Quasi-Configurations Derived by Special Arrangements of Lines
Abstract
:1. Introduction
2. Materials and Methods
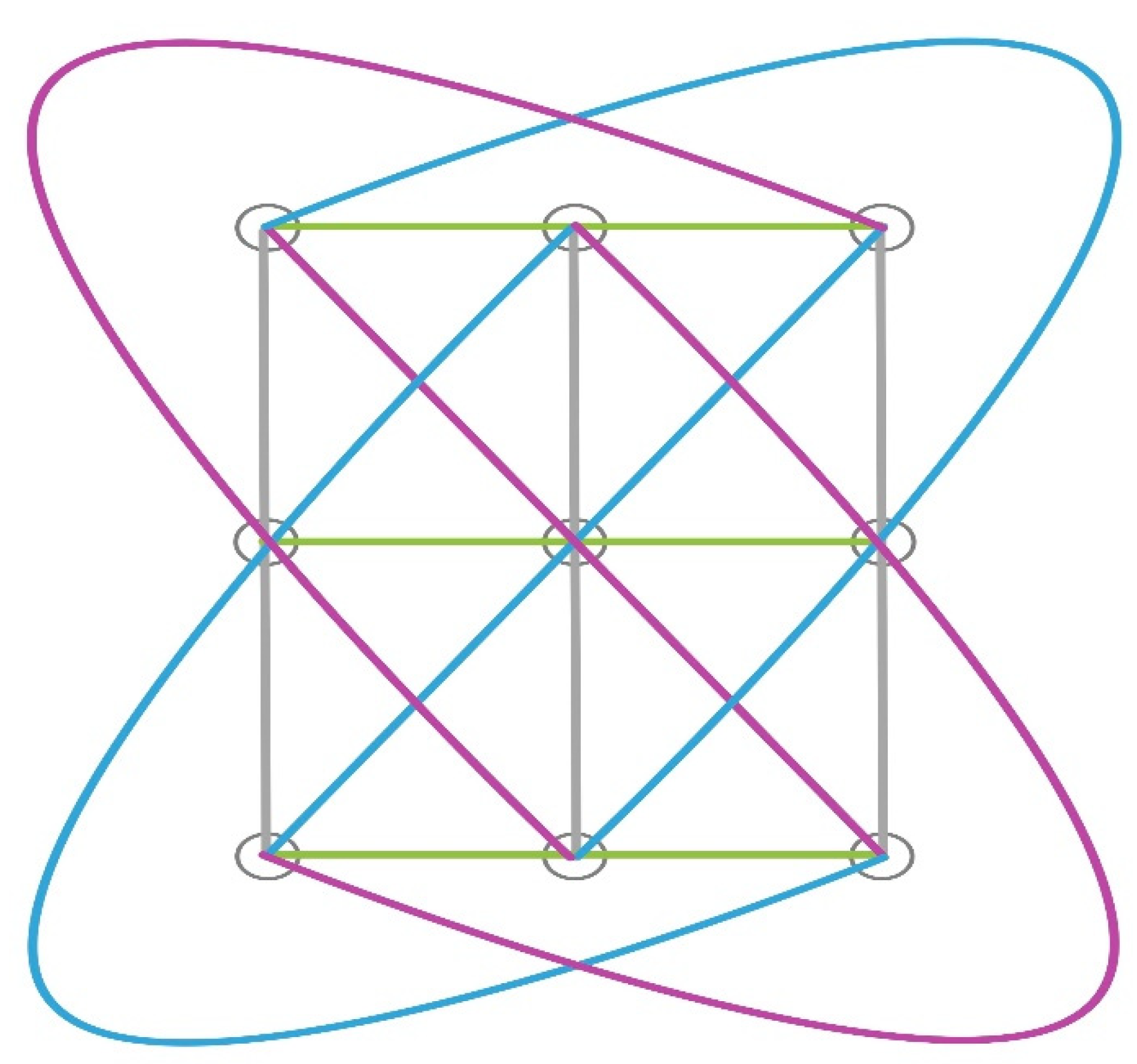
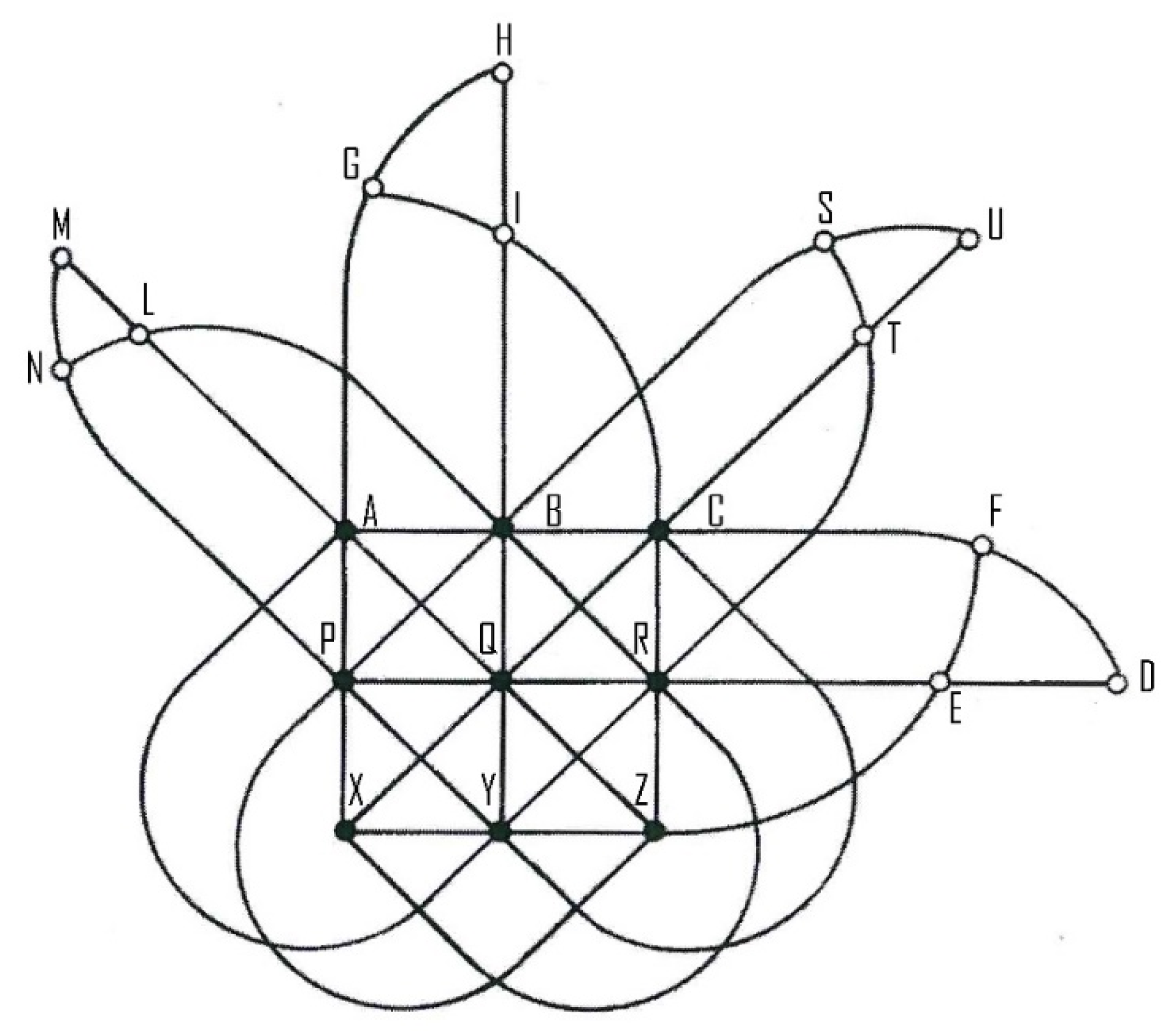
3. Ceva Arrangements of Lines
P: = (1,0,−1), Q: = (1,0,−ω2), R: = (1,0,−ω);
X: = (1,−1,0), Y: = (1,−ω,0), Z: = (1,−ω2,0).
APX: x + y + z = 0, BQY: x + ω2y + ωz = 0, CRZ: x + ωy + ω2z = 0;
AQZ: x + ωy + ωz = 0, BRX: x + y + ω2z = 0, CPY: x + ω2y + z = 0;
CQX: x + y + ωz = 0, ARY: x + ω2y + ω2z = 0, BPZ: x + ωy + z = 0;
G: = (1,ω,ω2), H: = (1,ω2,ω), I: = (1,1,1);
L: = (1,ω,1), M: = (1,1,ω), N: = (1,ω2,ω2);
U: = (1,ω,ω), T: = (1,ω2,1), S: = (1,1,ω2).
EGMT: y − ω2z = 0, EHSL: y − ωz = 0, EINU: y − z = 0;
FGNS: x − z = 0, FHMU: x − ω2z = 0, FILT: x − ωz = 0.
A∈EINU, B∈EGMT, C∈EHSL,
P∈FILT, Q∈FGNS, R∈FHMU,
4. Klein Arrangement of Lines
−x + y + ωz = 0, ωx + y + z = 0, −x + ωy + z = 0, −x − y + ωz = 0, x + z = 0, −x + ωy − z = 0, x + ωy + z = 0,
−x + z = 0, x − y + ωz = 0, x + y + ωz = 0, z = 0, −x + y = 0, x + y = 0, y = 0.
P8: = (1,−1,−ω), P9: = (1,ω−1,ω−1), P10: = (1,−ω,−1), P11: = (1,1,−ω), P12: = (1,0,1), P13: = (1,−ω,1), P14: = (1,ω,1),
P15: = (1,0,−1), P16: = (1,−1,ω), P17: = (1,1,ω), P18: = (0,0,1), P19: = (1,−1,0), P20: = (1,1,0), P21: = (0,1,0).
P11P17P18P20 x − y = 0, P6P10P15P21 x + z = 0, P1P2P7P9 y − z = 0, P8P16P18P19 x + y = 0,
P3P10P11P12 x − (ω + 1)y − z = 0, P4P14P15P16 x + (ω + 1)y + z = 0, P9P13P15P17 x − (ω + 1)y + z = 0,
P7P10P16P20 x − y + (ω + 1)z = 0, P2P4P6P11 (ω + 1)x − y + z = 0, P5P7P11P14 (ω + 1)x − y − z = 0,
P5P8 P9P10 (ω + 1)x + y + z = 0, P3P6P17P19 x + y + (ω + 1)z = 0, P2P3P13P16 (ω + 1)x + y − z = 0,
P6P7P8P12 x + (ω + 1)y − z = 0, P4P8P13P20 x − y − (ω + 1)z = 0, P9P11P14P19 x + y − (ω + 1)z = 0,
P3P14P20 x − y + (ω − 1)z = 0, P3P8P15 x + (−ω + 1)y + z = 0, P10P13P18 ωx + y = 0, P3P9P18 x − ωy = 0,
P6P9P20 x − y + (−ω + 1)z = 0, P7P13P19 x + y + (ω − 1)z = 0, P3P7P21 x + ωz = 0, P4P7P18 x + ωy = 0, P2P15P20 x − y + z = 0,
P7P11P15 x + (ω − 1)y + z = 0, P1P11P16 ωy + z = 0, P5P11P13 (ω − 1)x + y + z = 0, P8P11P21 ωx + z = 0, P2P12P19 x + y − z = 0,
P5P15P19 x + y + z = 0, P4P9P21 x − ωz = 0, P4P10P19 x + y + (−ω + 1)z = 0, P2P10P17 (ω − 1)x + y − z = 0,
P9P12P16 x + (−ω + 1)y − z = 0, P4P12P17 x + (ω − 1)y − z = 0, P5P6P16 (ω − 1)x − y − z = 0, P5P12P20 x − y − z = 0,
P16P17P21 ωx − z = 0.
P7 = (1,2,2) = 24, P8 = (1,6,4) = 38, P9 = (1,5,5) = 16, P10 = (1,4,6) = 50, P11 = (1,1,4) = 54, P12 = (1,0,1) = 13,
P13 = (1,4,1) = 30, P14 = (1,3,1) = 27, P15 = (1,0,6) = 43, P16 = (1,6,3) = 49, P17 = (1,1,3) = 25, P18 = (0,0,1) = 1,
P19 = (1,6,0) = 42, P20 = (1,1,0) = 35, P21 = (0,1,0) = 2,
5. Wiman Arrangement of Lines
P9: = (1,3,4), P10: = (1,14,13), P11: = (1,5,4), P12: = (1,12,18), P13: = (1,9,14), P14: = (1,15,2), P15: = (1,15,17),
P16: = (1,16,4), P17: = (1,5,13), P18: = (1,14,4), P19: = (1,7,18), P20: = (1,10,14), P21: = (1,4,2), P22: = (1,4,17),
P23: = (1,1,12), P24: = (1,14,15), P25: = (1,5,15), P26: = (1,12,1), P27: = (1,11,0), P28: = (1,8,8), P29: = (1,7,1),
P30: = (1,10,17), P31: = (1,18,12), P32: = (1,8,0), P33: = (1,11,8), P34: = (1,9,17), P35: = (1,0,7), P36: = (1,0,0),
P37: = (1,10,2), P38: = (0,1,11), P39: = (1,8,11), P40: = (1,11,11), P41: = (1,9,2), P42: = (0,1,8), P43: = (1,18,7),
P44: = (1,0,12), P45: = (1,1,7).
P7: = (1,9,5) = 311, P8: = (1,10,5) = 33, P9: = (1,3,4) = 232, P10: = (1,14,13) = 207, P11: = (1,5,4) = 237,
P12: = (1,12,18) = 199, P13: = (1,9,14) = 287, P14: = (1,15,2) = 254, P15: = (1,15,17) = 325, P16: = (1,16,4) = 346,
P17: = (1,5,13) = 271, P18: = (1,14,4) = 198, P19: = (1,7,18) = 41, P20: = (1,10,14) = 256, P21: = (1,4,2) = 159,
P22: = (1,4,17) = 279, P23: = (1,1,12) = 145, P24: = (1,14,15) = 28, P25: = (1,5,15) = 40, P26: = (1,12,1) = 209,
P27: = (1,11,0) = 272, P28: = (1,8,8) = 354, P29: = (1,7,1) = 148, P30: = (1,10,17) = 350,
P31: = (1,18,12) = 278, P32: = (1,8,0) = 267, P33: = (1,11,8) = 367, P34: = (1,9,17) = 363,
P35: = (1,0,7) = 253, P36: = (1,0,0) = 0, P37: = (1,10,2) = 128, P38: = (0,1,11) = 273,
P39: = (1,8,11) = 49, P40: = (1,11,11) = 7, P41: = (1,9,2) = 95, P42: = (0,1,8) = 268,
P43: = (1,18,7) = 352, P44: = (1,0,12) = 158, P45: = (1,1,7) = 324.
6. Conclusions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bokowski, J.; Pilaud, V. Quasi-configurations: Building blocks for point-line configurations. Ars Math. Contemp. 2016, 10, 99–112. [Google Scholar] [CrossRef]
- Berman, L.W.; Gévay, G.; Pisanski, T. On a new (21_4) polycyclic configuration. arXiv 2023, arXiv:2309.12992v2. [Google Scholar]
- Bauer, T.; Di Rocco, S.; Harbourne, B.; Huizenga, J.; Seceleanu, A.; Szemberg, T. Negative Curves on Symmetric Blowups of the Projective Plane, Resurgences, and Waldschmidt Constants. Int. Math. Res. Not. 2019, 2019, 7459–7514. [Google Scholar] [CrossRef]
- Grünbaum, B.; Rigby, J.F. The Real Configuration (214). J. Lond. Math. Soc. 1990, 2, 336–346. [Google Scholar] [CrossRef]
- Coxeter, H.S.M. My graph. Proc. London Math. Soc. 1983, 46, 117–136. [Google Scholar] [CrossRef]
- Ceva, G. De Lineis Rectis se Invicem Secantibus Statica Constructio, Mediolani, ex Typographia Ludouici Montiae, 1678. Available online: https://archive.org/details/ita-bnc-mag-00001346-001 (accessed on 1 April 2024).
- Klein, F. Uber die Transformation siebenter Ordnung der elliptischen Functionen. Math. Ann. 1879, 14, 428–471. [Google Scholar] [CrossRef]
- Gévay, G.; Pokora, P. Klein’s arrangements of lines and conics. Beitr Algebra Geom 2023, 65, 393–414. [Google Scholar] [CrossRef]
- Singer, J. A theorem in finite projective geometry and some applications to number theory. Trans. Amer. Math. Soc. 1938, 43, 377–385. Available online: https://www.ams.org/journals/tran/1938-043-03/S0002-9947-1938-1501951-4/S0002-9947-1938-1501951-4.pdf (accessed on 1 April 2024). [CrossRef]
- Hansen, T.; Mullen, G.L. Primitive Polynomials Over Finite Fields. Math. Comput. 1992, 59, 639–643. [Google Scholar] [CrossRef]
- De Clerck, F.; De Feyter, N. A characterization of the sets of internal and external points of a conic. Eur. J. Comb. 2007, 28, 1910–1921. [Google Scholar] [CrossRef]
- Hill, R.; Love, C.P. On the (22, 4)-arcs in PG(2, 7) and related codes. Discrete Math. 2003, 266, 253–261. [Google Scholar] [CrossRef]
- Van de Voorde, G. On sets without tangents and exterior sets of a conic. Discrete Math. 2011, 311, 2253–2258. [Google Scholar] [CrossRef]
- Bouyukliev, I.; Cheon, E.J.; Maruta, T.; Okazaki, T. On the (29, 5)-Arcs in PG(2,7) and Some Generalized Arcs in PG(2,q). Mathematics 2020, 8, 320. [Google Scholar] [CrossRef]
- Wiman, A. Zur Theorie der endlichen Gruppen von birationalen Transformationen in der Ebene. Math. Annalen 1896, 48, 195–240. [Google Scholar] [CrossRef]
- Bauer, T.; Di Rocco, S.; Harbourne, B.; Huizenga, J.; Lundman, A.; Pokora, P.; Szemberg, T. Bounded Negativity and Arrangements of Lines. Int. Math. Res. Not. 2015, 2015, 9456–9471. [Google Scholar] [CrossRef]
- Durante, N. On sets with few intersection numbers in finite projective and affine spaces. Electron. J. Combin. 2014, 21, P4–13. [Google Scholar] [CrossRef]


| ω0 = (1,0,0) | |||||||
|---|---|---|---|---|---|---|---|
| ω1 = (0,0,1) | ω2 = (0,1,0) | ω3 = (1,0,4) | ω4 = (0,1,3) | ω5 = (1,3,4) | ω6 = (1,6,1) | ω7 = (1,6,6) | ω8 = (1,1,6) |
| ω9 = (1,6,2) | ω10 = (1,5,6) | ω11 = (1,4,5) | ω12 = (1,3,0) | ω13 = (1,0,1) | ω14 = (0,1,5) | ω15 = (1,5,4) | ω16 = (1,5,5) |
| ω17 = (1,1,5) | ω18 = (1,5,2) | ω19 = (1,6,5) | ω20 = (1,2,6) | ω21 = (1,3,3) | ω22 = (1,1,1) | ω23 = (1,1,2) | ω24 = (1,2,2) |
| ω25 = (1,1,3) | ω26 = (1,3,2) | ω27 = (1,3,1) | ω28 = (1,5,1) | ω29 = (1,3,5) | ω30 = (1,4,1) | ω31 = (1,2,0) | ω32 = (1,0,3) |
| ω33 = (0,1,4) | ω34 = (1,4,4) | ω35 = (1,1,0) | ω36 = (1,0,2) | ω37 = (0,1,6) | ω38 = (1,6,4) | ω39 = (1,3,6) | ω40 = (1,2,1) |
| ω41 = (1,4,3) | ω42 = (1,6,0) | ω43 = (1,0,6) | ω44 = (0,1,2) | ω45 = (1,2,4) | ω46 = (1,2,3) | ω47 = (1,5,3) | ω48 = (1,2,5) |
| ω49 = (1,6,3) | ω50 = (1,4,6) | ω51 = (1,5,0) | ω52 = (1,0,5) | ω53 = (0,1,1) | ω54 = (1,1,4) | ω55 = (1,4,2) | ω56 = (1,4,0) |
| ℓ0 | 0 | 1 | 3 | 13 | 32 | 36 | 43 | 52 |
| 5 | 12 | 21 | 26 | 27 | 29 | 39 | 1 | 2 | 5 | 15 | 34 | 38 | 45 | 54 | 3 |
| 6 | 42 | 7 | 38 | 9 | 49 | 19 | 6 | 46 | 55 | 35 | 39 | 16 | 4 | ||
| 8 | 35 | 22 | 25 | 17 | 54 | 23 | 22 | 42 | 48 | 47 | 33 | 26 | 50 | ||
| 18 | 51 | 16 | 28 | 15 | 10 | 47 | 28 | 23 | 7 | 29 | 41 | 14 | 31 | ||
| 37 | 2 | 53 | 4 | 14 | 33 | 44 | 27 | 10 | 25 | 37 | 24 | 56 | 19 | ||
| 41 | 56 | 34 | 11 | 50 | 55 | 30 | 30 | 53 | 12 | 20 | 17 | 49 | 18 | ||
| 48 | 31 | 24 | 20 | 46 | 40 | 45 | 40 | 11 | 44 | 9 | 51 | 8 | 21 | ||
| 0 | 13 | ||||||||||||||
| 33 | 35 | 45 | 11 | 7 | 18 | 27 | 32 | 48 | 49 | 51 | 4 | 23 | 27 | 34 | 43 |
| 34 | 10 | 6 | 31 | 17 | 26 | 44 | 2 | 50 | 7 | 10 | 20 | 8 | 39 | ||
| 46 | 21 | 25 | 49 | 47 | 2 | 41 | 11 | 24 | 54 | 12 | 6 | 47 | 14 | ||
| 8 | 30 | 29 | 15 | 4 | 24 | 42 | 16 | 5 | 30 | 9 | 33 | 31 | 25 | ||
| 12 | 38 | 50 | 37 | 40 | 23 | 16 | 19 | 35 | 26 | 41 | 15 | 53 | 40 | ||
| 19 | 14 | 51 | 22 | 5 | 55 | 20 | 17 | 28 | 37 | 45 | 21 | 55 | 42 | ||
| 28 | 48 | 53 | 39 | 56 | 9 | 54 | 29 | 44 | 46 | 22 | 56 | 38 | 18 | ||
| 36 | 52 | ||||||||||||||
| ω0 = (1,0,0) | ω1 = (0,1,0) | ω2 = (0,0,1) | ||||||
|---|---|---|---|---|---|---|---|---|
| ω3 = (1,0,2) | ω4 = (1,5,2) | ω5 = (1,5,8) | ω6 = (1,6,13) | ω7 = (1,11,11) | ω8 = (1,13,12) | ω9 = (1,4,16) | ω10 = (1,3,14) | ω11 = (1,17,15) |
| ω12 = (1,7,7) | ω13 = (1,15,12) | ω14 = (1,4,5) | ω15 = (1,2,10) | ω16 = (1,1,4) | ω17 = (1,12,14) | ω18 = (1,17,16) | ω19 = (1,3,15) | ω20 = (1,7,4) |
| ω21 = (1,12,10) | ω22 = (1,1,14) | ω23 = (1,17,0) | ω24 = (0,1,17) | ω25 = (1,0,16) | ω26 = (1,3,2) | ω27 = (1,5,18) | ω28 = (1,14,15) | ω29 = (1,7,5) |
| ω30 = (1,2,16) | ω31 = (1,3,8) | ω32 = (1,6,1) | ω33 = (1,10,5) | ω34 = (1,2,3) | ω35 = (1,16,15) | ω36 = (1,7,0) | ω37 = (0,1,7) | ω38 = (1,0,17) |
| ω39 = (1,14,2) | ω40 = (1,5,15) | ω41 = (1,7,18) | ω42 = (1,9,8) | ω43 = (1,6,18) | ω44 = (1,9,18) | ω45 = (1,9,7) | ω46 = (1,15,4) | ω47 = (1,12,11) |
| ω48 = (1,13,6) | ω49 = (1,8,11) | ω50 = (1,13,11) | ω51 = (1,13,0) | ω52 = (0,1,13) | ω53 = (1,0,13) | ω54 = (1,11,2) | ω55 = (1,5,0) | ω56 = (0,1,5) |
| ω57 = (1,0,4) | ω58 = (1,12,2) | ω59 = (1,5,5) | ω60 = (1,2,12) | ω61 = (1,4,10) | ω62 = (1,1,6) | ω63 = (1,8,10) | ω64 = (1,1,10) | ω65 = (1,1,3) |
| ω66 = (1,16,18) | ω67 = (1,9,13) | ω68 = (1,11,6) | ω69 = (1,8,14) | ω70 = (1,17,5) | ω71 = (1,2,17) | ω72 = (1,14,11) | ω73 = (1,13,13) | ω74 = (1,11,12) |
| ω75 = (1,4,8) | ω76 = (1,6,7) | ω77 = (1,15,16) | ω78 = (1,3,9) | ω79 = (1,18,18) | ω80 = (1,9,12) | ω81 = (1,4,0) | ω82 = (0,1,4) | ω83 = (1,0,14) |
| ω84 = (1,17,2) | ω85 = (1,15,11) | ω86 = (1,13,10) | ω87 = (1,1,15) | ω88 = (1,7,9) | ω89 = (1,18,14) | ω90 = (1,17,4) | ω91 = (1,12,16) | ω92 = (1,3,0) |
| ω93 = (0,1,3) | ω94 = (1,0,18) | ω95 = (1,9,2) | ω96 = (1,5,9) | ω97 = (1,18,16) | ω98 = (1,3,18) | ω99 = (1,9,10) | ω100 = (1,1,11) | ω101 = (1,13,15) |
| ω102 = (1,7,17) | ω103 = (1,14,5) | ω104 = (1,2,11) | ω105 = (1,13,11) | ω106 = (1,18,8) | ω107 = (1,6,15) | ω108 = (1,7,6) | ω109 = (1,8,1) | ω110 = (1,10,6) |
| ω111 = (1,8,6) | ω112 = (1,8,9) | ω113 = (1,18,14) | ω114 = (1,11,10) | ω115 = (1,1,13) | ω116 = (1,11,13) | ω117 = (1,11,9) | ω118 = (1,18,10) | ω119 = (1,1,1) |
| ω120 = (1,10,12) | ω121 = (1,4,4) | ω122 = (1,12,12) | ω123 = (1,4,12) | ω124 = (1,4,18) | ω125 = (1,9,0) | ω126 = (0,1,9) | ω127 = (1,0,1) | ω128 = (1,10,2) |
| ω129 = (1,5,14) | ω130 = (1,17,11) | ω131 = (1,13,14) | ω132 = (1,17,14) | ω133 = (1,17,6) | ω134 = (1,8,5) | ω135 = (1,2,18) | ω136 = (1,9,1) | ω137 = (1,10,16) |
| ω138 = (1,3,13) | ω139 = (1,11,16) | ω140 = (1,3,16) | ω141 = (1,3,11) | ω142 = (1,13,3) | ω143 = (1,16,1) | ω144 = (1,10,10) | ω145 = (1,1,12) | ω146 = (1,4,6) |
| ω147 = (1,8,15) | ω148 = (1,7,1) | ω149 = (1,10,15) | ω150 = (1,7,15) | ω151 = (1,7,13) | ω152 = (1,11,3) | ω153 = (1,16,7) | ω154 = (1,15,14) | ω155 = (1,17,10) |
| ω156 = (1,1,0) | ω157 = (0,1,1) | ω158 = (1,0,12) | ω159 = (1,4,2) | ω160 = (1,5,3) | ω161 = (1,16,6) | ω162 = (1,8,16) | ω163 = (1,3,7) | ω164 = (1,15,9) |
| ω165 = (1,18,6) | ω166 = (1,8,13) | ω167 = (1,11,14) | ω168 = (1,17,18) | ω169 = (1,9,3) | ω170 = (1,16,13) | ω171 = (1,11,7) | ω172 = (1,15,15) | ω173 = (1,7,12) |
| ω174 = (1,4,11) | ω175 = (1,13,16) | ω176 = (1,3,3) | ω177 = (1,16,12) | ω178 = (1,4,9) | ω179 = (1,18,17) | ω180 = (1,14,17) | ω181 = (1,15,3) | ω182 = (1,16,14) |
| ω183 = (1,17,8) | ω184 = (1,6,9) | ω185 = (1,18,15) | ω186 = (1,7,14) | ω187 = (1,17,7) | ω188 = (1,15,10) | ω189 = (1,1,17) | ω190 = (1,14,16) | ω191 = (1,3,6) |
| ω192 = (1,8,7) | ω193 = (1,15,8) | ω194 = (1,6,16) | ω195 = (1,3,1) | ω196 = (1,10,13) | ω197 = (1,11,17) | ω198 = (1,14,4) | ω199 = (1,12,18) | ω200 = (1,9,15) |
| ω201 = (1,7,8) | ω202 = (1,6,6) | ω203 = (1,8,12) | ω204 = (1,4,15) | ω205 = (1,7,11) | ω206 = (1,13,17) | ω207 = (1,14,13) | ω208 = (1,11,4) | ω209 = (1,12,1) |
| ω210 = (1,10,8) | ω211 = (1,6,5) | ω212 = (1,2,14) | ω213 = (1,17,17) | ω214 = (1,14,12) | ω215 = (1,4,1) | ω216 = (1,10,4) | ω217 = (1,12,8) | ω218 = (1,6,17) |
| ω219 = (1,14,10) | ω220 = (1,1,16) | ω221 = (1,3,5) | ω222 = (1,2,8) | ω223 = (1,6,14) | ω224 = (1,17,9) | ω225 = (1,18,4) | ω226 = (1,12,9) | ω227 = (1,18,9) |
| ω228 = (1,18,3) | ω229 = (1,16,5) | ω230 = (1,2,15) | ω231 = (1,7,16) | ω232 = (1,3,4) | ω233 = (1,12,0) | ω234 = (0,1,12) | ω235 = (1,0,6) | ω236 = (1,8,2) |
| ω237 = (1,5,4) | ω238 = (1,12,5) | ω239 = (1,2,7) | ω240 = (1,15,13) | ω241 = (1,11,15) | ω242 = (1,7,3) | ω243 = (1,16,0) | ω244 = (0,1,16) | ω245 = (1,0,5) |
| ω246 = (1,2,2) | ω247 = (1,5,12) | ω248 = (1,4,3) | ω249 = (1,16,9) | ω250 = (1,18,5) | ω251 = (1,2,0) | ω252 = (0,1,2) | ω253 = (1,0,7) | ω254 = (1,15,2) |
| ω255 = (1,5,1) | ω256 = (1,10,14) | ω257 = (1,17,1) | ω258 = (1,10,1) | ω259 = (1,10,7) | ω260 = (1,15,0) | ω261 = (0,1,15) | ω262 = (1,0,9) | ω263 = (1,18,2) |
| ω264 = (1,5,16) | ω265 = (1,3,17) | ω266 = (1,14,6) | ω267 = (1,8,0) | ω268 = (0,1,8) | ω269 = (1,0,8) | ω270 = (1,6,2) | ω271 = (1,5,13) | ω272 = (1,11,0) |
| ω273 = (0,1,11) | ω274 = (1,0,15) | ω275 = (1,7,2) | ω276 = (1,5,18) | ω277 = (1,9,9) | ω278 = (1,18,12) | ω279 = (1,4,17) | ω280 = (1,14,1) | ω281 = (1,10,9) |
| ω282 = (1,18,11) | ω283 = (1,13,8) | ω284 = (1,6,4) | ω285 = (1,12,17) | ω286 = (1,14,18) | ω287 = (1,9,14) | ω288 = (1,17,3) | ω289 = (1,16,8) | ω290 = (1,6,3) |
| ω291 = (1,16,3) | ω292 = (1,16,11) | ω293 = (1,13,1) | ω294 = (1,10,18) | ω295 = (1,9,16) | ω296 = (1,3,10) | ω297 = (1,1,5) | ω298 = (1,2,4) | ω299 = (1,12,7) |
| ω300 = (1,15,11) | ω301 = (1,13,7) | ω302 = (1,15,7) | ω303 = (1,15,18) | ω304 = (1,9,4) | ω305 = (1,12,15) | ω306 = (1,7,10) | ω307 = (1,1,9) | ω308 = (1,18,1) |
| ω309 = (1,10,11) | ω310 = (1,13,18) | ω311 = (1,9,5) | ω312 = (1,2,1) | ω313 = (1,10,3) | ω314 = (1,16,10) | ω315 = (1,1,18) | ω316 = (1,9,11) | ω317 = (1,13,5) |
| ω318 = (1,2,9) | ω319 = (1,18,0) | ω320 = (0,1,18) | ω321 = (1,0,11) | ω322 = (1,13,2) | ω323 = (1,5,10) | ω324 = (1,1,7) | ω325 = (1,15,17) | ω326 = (1,14,3) |
| ω327 = (1,16,17) | ω328 = (1,14,17) | ω329 = (1,14,8) | ω330 = (1,6,10) | ω331 = (1,1,8) | ω332 = (1,6,8) | ω333 = (1,6,0) | ω334 = (0,1,6) | ω335 = (1,0,10) |
| ω336 = (1,1,2) | ω337 = (1,5,7) | ω338 = (1,15,1) | ω339 = (1,10,0) | ω340 = (0,1,10) | ω341 = (1,0,3) | ω342 = (1,16,2) | ω343 = (1,5,6) | ω344 = (1,8,4) |
| ω345 = (1,12,3) | ω346 = (1,16,4) | ω347 = (1,12,4) | ω348 = (1,12,13) | ω349 = (1,11,1) | ω350 = (1,10,17) | ω351 = (1,14,9) | ω352 = (1,18,7) | ω353 = (1,15,6) |
| ω354 = (1,8,8) | ω355 = (1,6,12) | ω356 = (1,4,7) | ω357 = (1,15,5) | ω358 = (1,2,13) | ω359 = (1,11,5) | ω360 = (1,2,5) | ω361 = (1,2,6) | ω362 = (1,8,18) |
| ω363 = (1,9,17) | ω364 = (1,14,14) | ω365 = (1,17,12) | ω366 = (1,4,13) | ω367 = (1,11,8) | ω368 = (1,6,11) | ω369 = (1,13,4) | ω370 = (1,12,6) | ω371 = (1,8,3) |
| ω372 = (1,16,16) | ω373 = (1,3,12) | ω374 = (1,4,14) | ω375 = (1,17,3) | ω376 = (1,11,18) | ω377 = (1,9,6) | ω378 = (1,8,17) | ω379 = (1,14,0) | ω380 = (0,1,14) |
| ℓ0 | 1 | 2 | 24 | 37 | 52 | 56 | 82 | 93 | 126 | 157 | 234 | 244 | 252 | 261 | 268 | 273 | 320 | 334 | 340 | 380 |
| ℓ1 | 0 | 2 | 3 | 25 | 38 | 53 | 57 | 83 | 94 | 127 | 158 | 235 | 245 | 253 | 262 | 269 | 274 | 321 | 335 | 341 |
| ℓ2 | 1 | 3 | 4 | 26 | 39 | 54 | 58 | 84 | 95 | 128 | 159 | 236 | 246 | 254 | 263 | 270 | 275 | 322 | 336 | 342 |
| ℓ3 | 2 | 4 | 5 | 27 | 40 | 55 | 59 | 85 | 96 | 129 | 160 | 237 | 247 | 255 | 264 | 271 | 276 | 323 | 337 | 343 |
| ℓ4 | 3 | 5 | 6 | 28 | 41 | 56 | 60 | 86 | 97 | 130 | 161 | 238 | 248 | 256 | 265 | 272 | 277 | 324 | 338 | 344 |
| ℓ5 | 4 | 6 | 7 | 29 | 42 | 57 | 61 | 87 | 98 | 131 | 162 | 239 | 249 | 257 | 266 | 273 | 278 | 325 | 339 | 345 |
| ℓ6 | 5 | 7 | 8 | 30 | 43 | 58 | 62 | 88 | 99 | 132 | 163 | 240 | 250 | 258 | 267 | 274 | 279 | 326 | 340 | 346 |
| ℓ7 | 6 | 8 | 9 | 31 | 44 | 59 | 63 | 89 | 100 | 133 | 164 | 241 | 251 | 259 | 268 | 275 | 280 | 327 | 341 | 347 |
| ℓ8 | 7 | 9 | 10 | 32 | 45 | 60 | 64 | 90 | 101 | 134 | 165 | 242 | 252 | 260 | 269 | 276 | 281 | 328 | 342 | 348 |
| ℓ9 | 8 | 10 | 11 | 33 | 46 | 61 | 65 | 91 | 102 | 135 | 166 | 243 | 253 | 261 | 270 | 277 | 282 | 329 | 343 | 349 |
| ℓ10 | 9 | 11 | 12 | 34 | 47 | 62 | 66 | 92 | 103 | 136 | 167 | 244 | 254 | 262 | 271 | 278 | 283 | 330 | 344 | 350 |
| ℓ11 | 10 | 12 | 13 | 35 | 48 | 63 | 67 | 93 | 104 | 137 | 168 | 245 | 255 | 263 | 272 | 279 | 284 | 331 | 345 | 351 |
| ℓ12 | 11 | 13 | 14 | 36 | 49 | 64 | 68 | 94 | 105 | 138 | 169 | 246 | 256 | 264 | 273 | 280 | 285 | 332 | 346 | 352 |
| ℓ13 | 12 | 14 | 15 | 37 | 50 | 65 | 69 | 95 | 106 | 139 | 170 | 247 | 257 | 265 | 274 | 281 | 286 | 333 | 347 | 353 |
| ℓ14 | 13 | 15 | 16 | 38 | 51 | 66 | 70 | 96 | 107 | 140 | 171 | 248 | 258 | 266 | 275 | 282 | 287 | 334 | 348 | 354 |
| ℓ15 | 14 | 16 | 17 | 39 | 52 | 67 | 71 | 97 | 108 | 141 | 172 | 249 | 259 | 267 | 276 | 283 | 288 | 335 | 349 | 355 |
| ℓ16 | 15 | 17 | 18 | 40 | 53 | 68 | 72 | 98 | 109 | 142 | 173 | 250 | 260 | 268 | 277 | 284 | 289 | 336 | 350 | 356 |
| ℓ17 | 16 | 18 | 19 | 41 | 54 | 69 | 73 | 99 | 110 | 143 | 174 | 251 | 261 | 269 | 278 | 285 | 290 | 337 | 351 | 357 |
| ℓ18 | 17 | 19 | 20 | 42 | 55 | 70 | 74 | 100 | 111 | 144 | 175 | 252 | 262 | 270 | 279 | 286 | 291 | 338 | 352 | 358 |
| ℓ19 | 18 | 20 | 21 | 43 | 56 | 71 | 75 | 101 | 112 | 145 | 176 | 253 | 263 | 271 | 280 | 287 | 292 | 339 | 353 | 359 |
| ℓ20 | 19 | 21 | 22 | 44 | 57 | 72 | 76 | 102 | 113 | 146 | 177 | 254 | 264 | 272 | 281 | 288 | 293 | 340 | 354 | 360 |
| ℓ21 | 20 | 22 | 23 | 45 | 58 | 73 | 77 | 103 | 114 | 147 | 178 | 255 | 265 | 273 | 282 | 289 | 294 | 341 | 355 | 361 |
| ℓ22 | 21 | 23 | 24 | 46 | 59 | 74 | 78 | 104 | 115 | 148 | 179 | 256 | 266 | 274 | 283 | 290 | 295 | 342 | 356 | 362 |
| ℓ23 | 22 | 24 | 25 | 47 | 60 | 75 | 79 | 105 | 116 | 149 | 180 | 257 | 267 | 275 | 284 | 291 | 296 | 343 | 357 | 363 |
| ℓ24 | 23 | 25 | 26 | 48 | 61 | 76 | 80 | 106 | 117 | 150 | 181 | 258 | 268 | 276 | 285 | 292 | 297 | 344 | 358 | 364 |
| ℓ25 | 24 | 26 | 27 | 49 | 62 | 77 | 81 | 107 | 118 | 151 | 182 | 259 | 269 | 277 | 286 | 293 | 298 | 345 | 359 | 365 |
| ℓ26 | 25 | 27 | 28 | 50 | 63 | 78 | 82 | 108 | 119 | 152 | 183 | 260 | 270 | 278 | 287 | 294 | 299 | 346 | 360 | 366 |
| ℓ27 | 26 | 28 | 29 | 51 | 64 | 79 | 83 | 109 | 120 | 153 | 184 | 261 | 271 | 279 | 288 | 295 | 300 | 347 | 361 | 367 |
| ℓ28 | 27 | 29 | 30 | 52 | 65 | 80 | 84 | 110 | 121 | 154 | 185 | 262 | 272 | 280 | 289 | 296 | 301 | 348 | 362 | 368 |
| ℓ29 | 28 | 30 | 31 | 53 | 66 | 81 | 85 | 111 | 122 | 155 | 186 | 263 | 273 | 281 | 290 | 297 | 302 | 349 | 363 | 369 |
| ℓ30 | 29 | 31 | 32 | 54 | 67 | 82 | 86 | 112 | 123 | 156 | 187 | 264 | 274 | 282 | 291 | 298 | 303 | 350 | 364 | 370 |
| ℓ31 | 30 | 32 | 33 | 55 | 68 | 83 | 87 | 113 | 124 | 157 | 188 | 265 | 275 | 283 | 292 | 299 | 304 | 351 | 365 | 371 |
| ℓ32 | 31 | 33 | 34 | 56 | 69 | 84 | 88 | 114 | 125 | 158 | 189 | 266 | 276 | 284 | 293 | 300 | 305 | 352 | 366 | 372 |
| ℓ33 | 32 | 34 | 35 | 57 | 70 | 85 | 89 | 115 | 126 | 159 | 190 | 267 | 277 | 285 | 294 | 301 | 306 | 353 | 367 | 373 |
| ℓ34 | 33 | 35 | 36 | 58 | 71 | 86 | 90 | 116 | 127 | 160 | 191 | 268 | 278 | 286 | 295 | 302 | 307 | 354 | 368 | 374 |
| ℓ35 | 34 | 36 | 37 | 59 | 72 | 87 | 91 | 117 | 128 | 161 | 192 | 269 | 279 | 287 | 296 | 303 | 308 | 355 | 369 | 375 |
| ℓ36 | 35 | 37 | 38 | 60 | 73 | 88 | 92 | 118 | 129 | 162 | 193 | 270 | 280 | 288 | 297 | 304 | 309 | 356 | 370 | 376 |
| ℓ37 | 36 | 38 | 39 | 61 | 74 | 89 | 93 | 119 | 130 | 163 | 194 | 271 | 281 | 289 | 298 | 305 | 310 | 357 | 371 | 377 |
| ℓ38 | 37 | 39 | 40 | 62 | 75 | 90 | 94 | 120 | 131 | 164 | 195 | 272 | 282 | 290 | 299 | 306 | 311 | 358 | 372 | 378 |
| ℓ39 | 38 | 40 | 41 | 63 | 76 | 91 | 95 | 121 | 132 | 165 | 196 | 273 | 283 | 291 | 300 | 307 | 312 | 359 | 373 | 379 |
| ℓ40 | 39 | 41 | 42 | 64 | 77 | 92 | 96 | 122 | 133 | 166 | 197 | 274 | 284 | 292 | 301 | 308 | 313 | 360 | 374 | 380 |
| ℓ41 | 0 | 40 | 42 | 43 | 65 | 78 | 93 | 97 | 123 | 134 | 167 | 198 | 275 | 285 | 293 | 302 | 309 | 314 | 361 | 375 |
| ℓ42 | 1 | 41 | 43 | 44 | 66 | 79 | 94 | 98 | 124 | 135 | 168 | 199 | 276 | 286 | 294 | 303 | 310 | 315 | 362 | 376 |
| ℓ43 | 2 | 42 | 44 | 45 | 67 | 80 | 95 | 99 | 125 | 136 | 169 | 200 | 277 | 287 | 295 | 304 | 311 | 316 | 363 | 377 |
| ℓ44 | 3 | 43 | 45 | 46 | 68 | 81 | 96 | 100 | 126 | 137 | 170 | 201 | 278 | 288 | 296 | 305 | 312 | 317 | 364 | 378 |
| ℓ45 | 4 | 44 | 46 | 47 | 69 | 82 | 97 | 101 | 127 | 138 | 171 | 202 | 279 | 289 | 297 | 306 | 313 | 318 | 365 | 379 |
| ℓ46 | 5 | 45 | 47 | 48 | 70 | 83 | 98 | 102 | 128 | 139 | 172 | 203 | 280 | 290 | 298 | 307 | 314 | 319 | 366 | 380 |
| ℓ47 | 0 | 6 | 46 | 48 | 49 | 71 | 84 | 99 | 103 | 129 | 140 | 173 | 204 | 281 | 291 | 299 | 308 | 315 | 320 | 367 |
| ℓ48 | 1 | 7 | 47 | 49 | 50 | 72 | 85 | 100 | 104 | 130 | 141 | 174 | 205 | 282 | 292 | 300 | 309 | 316 | 321 | 368 |
| ℓ49 | 2 | 8 | 48 | 50 | 51 | 73 | 86 | 101 | 105 | 131 | 142 | 175 | 206 | 283 | 293 | 301 | 310 | 317 | 322 | 369 |
| ℓ50 | 3 | 9 | 49 | 51 | 52 | 74 | 87 | 102 | 106 | 132 | 143 | 176 | 207 | 284 | 294 | 302 | 311 | 318 | 323 | 370 |
| ℓ51 | 4 | 10 | 50 | 52 | 53 | 75 | 88 | 103 | 107 | 133 | 144 | 177 | 208 | 285 | 295 | 303 | 312 | 319 | 324 | 371 |
| ℓ52 | 5 | 11 | 51 | 53 | 54 | 76 | 89 | 104 | 108 | 134 | 145 | 178 | 209 | 286 | 296 | 304 | 313 | 320 | 325 | 372 |
| ℓ53 | 6 | 12 | 52 | 54 | 55 | 77 | 90 | 105 | 109 | 135 | 146 | 179 | 210 | 287 | 297 | 305 | 314 | 321 | 326 | 373 |
| ℓ54 | 7 | 13 | 53 | 55 | 56 | 78 | 91 | 106 | 110 | 136 | 147 | 180 | 211 | 288 | 298 | 306 | 315 | 322 | 327 | 374 |
| ℓ55 | 8 | 14 | 54 | 56 | 57 | 79 | 92 | 107 | 111 | 137 | 148 | 181 | 212 | 289 | 299 | 307 | 316 | 323 | 328 | 375 |
| ℓ56 | 9 | 15 | 55 | 57 | 58 | 80 | 93 | 108 | 112 | 138 | 149 | 182 | 213 | 290 | 300 | 308 | 317 | 324 | 329 | 376 |
| ℓ57 | 10 | 16 | 56 | 58 | 59 | 81 | 94 | 109 | 113 | 139 | 150 | 183 | 214 | 291 | 301 | 309 | 318 | 325 | 330 | 377 |
| ℓ58 | 11 | 17 | 57 | 59 | 60 | 82 | 95 | 110 | 114 | 140 | 151 | 184 | 215 | 292 | 302 | 310 | 319 | 326 | 331 | 378 |
| ℓ59 | 12 | 18 | 58 | 60 | 61 | 83 | 96 | 111 | 115 | 141 | 152 | 185 | 216 | 293 | 303 | 311 | 320 | 327 | 332 | 379 |
| ℓ60 | 13 | 19 | 59 | 61 | 62 | 84 | 97 | 112 | 116 | 142 | 153 | 186 | 217 | 294 | 304 | 312 | 321 | 328 | 333 | 380 |
| ℓ61 | 0 | 14 | 20 | 60 | 62 | 63 | 85 | 98 | 113 | 117 | 143 | 154 | 187 | 218 | 295 | 305 | 313 | 322 | 329 | 334 |
| ℓ62 | 1 | 15 | 21 | 61 | 63 | 64 | 86 | 99 | 114 | 118 | 144 | 155 | 188 | 219 | 296 | 306 | 314 | 323 | 330 | 335 |
| ℓ63 | 2 | 16 | 22 | 62 | 64 | 65 | 87 | 100 | 115 | 119 | 145 | 156 | 189 | 220 | 297 | 307 | 315 | 324 | 331 | 336 |
| ℓ64 | 3 | 17 | 23 | 63 | 65 | 66 | 88 | 101 | 116 | 120 | 146 | 157 | 190 | 221 | 298 | 308 | 316 | 325 | 332 | 337 |
| ℓ65 | 4 | 18 | 24 | 64 | 66 | 67 | 89 | 102 | 117 | 121 | 147 | 158 | 191 | 222 | 299 | 309 | 317 | 326 | 333 | 338 |
| ℓ66 | 5 | 19 | 25 | 65 | 67 | 68 | 90 | 103 | 118 | 122 | 148 | 159 | 192 | 223 | 300 | 310 | 318 | 327 | 334 | 339 |
| ℓ67 | 6 | 20 | 26 | 66 | 68 | 69 | 91 | 104 | 119 | 123 | 149 | 160 | 193 | 224 | 301 | 311 | 319 | 328 | 335 | 340 |
| ℓ68 | 7 | 21 | 27 | 67 | 69 | 70 | 92 | 105 | 120 | 124 | 150 | 161 | 194 | 225 | 302 | 312 | 320 | 329 | 336 | 341 |
| ℓ69 | 8 | 22 | 28 | 68 | 70 | 71 | 93 | 106 | 121 | 125 | 151 | 162 | 195 | 226 | 303 | 313 | 321 | 330 | 337 | 342 |
| ℓ70 | 9 | 23 | 29 | 69 | 71 | 72 | 94 | 107 | 122 | 126 | 152 | 163 | 196 | 227 | 304 | 314 | 322 | 331 | 338 | 343 |
| ℓ71 | 10 | 24 | 30 | 70 | 72 | 73 | 95 | 108 | 123 | 127 | 153 | 164 | 197 | 228 | 305 | 315 | 323 | 332 | 339 | 344 |
| ℓ72 | 11 | 25 | 31 | 71 | 73 | 74 | 96 | 109 | 124 | 128 | 154 | 165 | 198 | 229 | 306 | 316 | 324 | 333 | 340 | 345 |
| ℓ73 | 12 | 26 | 32 | 72 | 74 | 75 | 97 | 110 | 125 | 129 | 155 | 166 | 199 | 230 | 307 | 317 | 325 | 334 | 341 | 346 |
| ℓ74 | 13 | 27 | 33 | 73 | 75 | 76 | 98 | 111 | 126 | 130 | 156 | 167 | 200 | 231 | 308 | 318 | 326 | 335 | 342 | 347 |
| ℓ75 | 14 | 28 | 34 | 74 | 76 | 77 | 99 | 112 | 127 | 131 | 157 | 168 | 201 | 232 | 309 | 319 | 327 | 336 | 343 | 348 |
| ℓ76 | 15 | 29 | 35 | 75 | 77 | 78 | 100 | 113 | 128 | 132 | 158 | 169 | 202 | 233 | 310 | 320 | 328 | 337 | 344 | 349 |
| ℓ77 | 16 | 30 | 36 | 76 | 78 | 79 | 101 | 114 | 129 | 133 | 159 | 170 | 203 | 234 | 311 | 321 | 329 | 338 | 345 | 350 |
| ℓ78 | 17 | 31 | 37 | 77 | 79 | 80 | 102 | 115 | 130 | 134 | 160 | 171 | 204 | 235 | 312 | 322 | 330 | 339 | 346 | 351 |
| ℓ79 | 18 | 32 | 38 | 78 | 80 | 81 | 103 | 116 | 131 | 135 | 161 | 172 | 205 | 236 | 313 | 323 | 331 | 340 | 347 | 352 |
| ℓ80 | 19 | 33 | 39 | 79 | 81 | 82 | 104 | 117 | 132 | 136 | 162 | 173 | 206 | 237 | 314 | 324 | 332 | 341 | 348 | 353 |
| ℓ81 | 20 | 34 | 40 | 80 | 82 | 83 | 105 | 118 | 133 | 137 | 163 | 174 | 207 | 238 | 315 | 325 | 333 | 342 | 349 | 354 |
| ℓ82 | 21 | 35 | 41 | 81 | 83 | 84 | 106 | 119 | 134 | 138 | 164 | 175 | 208 | 239 | 316 | 326 | 334 | 343 | 350 | 355 |
| ℓ83 | 22 | 36 | 42 | 82 | 84 | 85 | 107 | 120 | 135 | 139 | 165 | 176 | 209 | 240 | 317 | 327 | 335 | 344 | 351 | 356 |
| ℓ84 | 23 | 37 | 43 | 83 | 85 | 86 | 108 | 121 | 136 | 140 | 166 | 177 | 210 | 241 | 318 | 328 | 336 | 345 | 352 | 357 |
| ℓ85 | 24 | 38 | 44 | 84 | 86 | 87 | 109 | 122 | 137 | 141 | 167 | 178 | 211 | 242 | 319 | 329 | 337 | 346 | 353 | 358 |
| ℓ86 | 25 | 39 | 45 | 85 | 87 | 88 | 110 | 123 | 138 | 142 | 168 | 179 | 212 | 243 | 320 | 330 | 338 | 347 | 354 | 359 |
| ℓ87 | 26 | 40 | 46 | 86 | 88 | 89 | 111 | 124 | 139 | 143 | 169 | 180 | 213 | 244 | 321 | 331 | 339 | 348 | 355 | 360 |
| ℓ88 | 27 | 41 | 47 | 87 | 89 | 90 | 112 | 125 | 140 | 144 | 170 | 181 | 214 | 245 | 322 | 332 | 340 | 349 | 356 | 361 |
| ℓ89 | 28 | 42 | 48 | 88 | 90 | 91 | 113 | 126 | 141 | 145 | 171 | 182 | 215 | 246 | 323 | 333 | 341 | 350 | 357 | 362 |
| ℓ90 | 29 | 43 | 49 | 89 | 91 | 92 | 114 | 127 | 142 | 146 | 172 | 183 | 216 | 247 | 324 | 334 | 342 | 351 | 358 | 363 |
| ℓ91 | 30 | 44 | 50 | 90 | 92 | 93 | 115 | 128 | 143 | 147 | 173 | 184 | 217 | 248 | 325 | 335 | 343 | 352 | 359 | 364 |
| ℓ92 | 31 | 45 | 51 | 91 | 93 | 94 | 116 | 129 | 144 | 148 | 174 | 185 | 218 | 249 | 326 | 336 | 344 | 353 | 360 | 365 |
| ℓ93 | 32 | 46 | 52 | 92 | 94 | 95 | 117 | 130 | 145 | 149 | 175 | 186 | 219 | 250 | 327 | 337 | 345 | 354 | 361 | 366 |
| ℓ94 | 33 | 47 | 53 | 93 | 95 | 96 | 118 | 131 | 146 | 150 | 176 | 187 | 220 | 251 | 328 | 338 | 346 | 355 | 362 | 367 |
| ℓ95 | 34 | 48 | 54 | 94 | 96 | 97 | 119 | 132 | 147 | 151 | 177 | 188 | 221 | 252 | 329 | 339 | 347 | 356 | 363 | 368 |
| ℓ96 | 35 | 49 | 55 | 95 | 97 | 98 | 120 | 133 | 148 | 152 | 178 | 189 | 222 | 253 | 330 | 340 | 348 | 357 | 364 | 369 |
| ℓ97 | 36 | 50 | 56 | 96 | 98 | 99 | 121 | 134 | 149 | 153 | 179 | 190 | 223 | 254 | 331 | 341 | 349 | 358 | 365 | 370 |
| ℓ98 | 37 | 51 | 57 | 97 | 99 | 100 | 122 | 135 | 150 | 154 | 180 | 191 | 224 | 255 | 332 | 342 | 350 | 359 | 366 | 371 |
| ℓ99 | 38 | 52 | 58 | 98 | 100 | 101 | 123 | 136 | 151 | 155 | 181 | 192 | 225 | 256 | 333 | 343 | 351 | 360 | 367 | 372 |
| ℓ100 | 39 | 53 | 59 | 99 | 101 | 102 | 124 | 137 | 152 | 156 | 182 | 193 | 226 | 257 | 334 | 344 | 352 | 361 | 368 | 373 |
| ℓ101 | 40 | 54 | 60 | 100 | 102 | 103 | 125 | 138 | 153 | 157 | 183 | 194 | 227 | 258 | 335 | 345 | 353 | 362 | 369 | 374 |
| ℓ102 | 41 | 55 | 61 | 101 | 103 | 104 | 126 | 139 | 154 | 158 | 184 | 195 | 228 | 259 | 336 | 346 | 354 | 363 | 370 | 375 |
| ℓ103 | 42 | 56 | 62 | 102 | 104 | 105 | 127 | 140 | 155 | 159 | 185 | 196 | 229 | 260 | 337 | 347 | 355 | 364 | 371 | 376 |
| ℓ104 | 43 | 57 | 63 | 103 | 105 | 106 | 128 | 141 | 156 | 160 | 186 | 197 | 230 | 261 | 338 | 348 | 356 | 365 | 372 | 377 |
| ℓ105 | 44 | 58 | 64 | 104 | 106 | 107 | 129 | 142 | 157 | 161 | 187 | 198 | 231 | 262 | 339 | 349 | 357 | 366 | 373 | 378 |
| ℓ106 | 45 | 59 | 65 | 105 | 107 | 108 | 130 | 143 | 158 | 162 | 188 | 199 | 232 | 263 | 340 | 350 | 358 | 367 | 374 | 379 |
| ℓ107 | 46 | 60 | 66 | 106 | 108 | 109 | 131 | 144 | 159 | 163 | 189 | 200 | 233 | 264 | 341 | 351 | 359 | 368 | 375 | 380 |
| ℓ108 | 0 | 47 | 61 | 67 | 107 | 109 | 110 | 132 | 145 | 160 | 164 | 190 | 201 | 234 | 265 | 342 | 352 | 360 | 369 | 376 |
| ℓ109 | 1 | 48 | 62 | 68 | 108 | 110 | 111 | 133 | 146 | 161 | 165 | 191 | 202 | 235 | 266 | 343 | 353 | 361 | 370 | 377 |
| ℓ110 | 2 | 49 | 63 | 69 | 109 | 111 | 112 | 134 | 147 | 162 | 166 | 192 | 203 | 236 | 267 | 344 | 354 | 362 | 371 | 378 |
| ℓ111 | 3 | 50 | 64 | 70 | 110 | 112 | 113 | 135 | 148 | 163 | 167 | 193 | 204 | 237 | 268 | 345 | 355 | 363 | 372 | 379 |
| ℓ112 | 4 | 51 | 65 | 71 | 111 | 113 | 114 | 136 | 149 | 164 | 168 | 194 | 205 | 238 | 269 | 346 | 356 | 364 | 373 | 380 |
| ℓ113 | 0 | 5 | 52 | 66 | 72 | 112 | 114 | 115 | 137 | 150 | 165 | 169 | 195 | 206 | 239 | 270 | 347 | 357 | 365 | 374 |
| ℓ114 | 1 | 6 | 53 | 67 | 73 | 113 | 115 | 116 | 138 | 151 | 166 | 170 | 196 | 207 | 240 | 271 | 348 | 358 | 366 | 375 |
| ℓ115 | 2 | 7 | 54 | 68 | 74 | 114 | 116 | 117 | 139 | 152 | 167 | 171 | 197 | 208 | 241 | 272 | 349 | 359 | 367 | 376 |
| ℓ116 | 3 | 8 | 55 | 69 | 75 | 115 | 117 | 118 | 140 | 153 | 168 | 172 | 198 | 209 | 242 | 273 | 350 | 360 | 368 | 377 |
| ℓ117 | 4 | 9 | 56 | 70 | 76 | 116 | 118 | 119 | 141 | 154 | 169 | 173 | 199 | 210 | 243 | 274 | 351 | 361 | 369 | 378 |
| ℓ118 | 5 | 10 | 57 | 71 | 77 | 117 | 119 | 120 | 142 | 155 | 170 | 174 | 200 | 211 | 244 | 275 | 352 | 362 | 370 | 379 |
| ℓ119 | 6 | 11 | 58 | 72 | 78 | 118 | 120 | 121 | 143 | 156 | 171 | 175 | 201 | 212 | 245 | 276 | 353 | 363 | 371 | 380 |
| ℓ120 | 0 | 7 | 12 | 59 | 73 | 79 | 119 | 121 | 122 | 144 | 157 | 172 | 176 | 202 | 213 | 246 | 277 | 354 | 364 | 372 |
| ℓ121 | 1 | 8 | 13 | 60 | 74 | 80 | 120 | 122 | 123 | 145 | 158 | 173 | 177 | 203 | 214 | 247 | 278 | 355 | 365 | 373 |
| ℓ122 | 2 | 9 | 14 | 61 | 75 | 81 | 121 | 123 | 124 | 146 | 159 | 174 | 178 | 204 | 215 | 248 | 279 | 356 | 366 | 374 |
| ℓ123 | 3 | 10 | 15 | 62 | 76 | 82 | 122 | 124 | 125 | 147 | 160 | 175 | 179 | 205 | 216 | 249 | 280 | 357 | 367 | 375 |
| ℓ124 | 4 | 11 | 16 | 63 | 77 | 83 | 123 | 125 | 126 | 148 | 161 | 176 | 180 | 206 | 217 | 250 | 281 | 358 | 368 | 376 |
| ℓ125 | 5 | 12 | 17 | 64 | 78 | 84 | 124 | 126 | 127 | 149 | 162 | 177 | 181 | 207 | 218 | 251 | 282 | 359 | 369 | 377 |
| ℓ126 | 6 | 13 | 18 | 65 | 79 | 85 | 125 | 127 | 128 | 150 | 163 | 178 | 182 | 208 | 219 | 252 | 283 | 360 | 370 | 378 |
| ℓ127 | 7 | 14 | 19 | 66 | 80 | 86 | 126 | 128 | 129 | 151 | 164 | 179 | 183 | 209 | 220 | 253 | 284 | 361 | 371 | 379 |
| ℓ128 | 8 | 15 | 20 | 67 | 81 | 87 | 127 | 129 | 130 | 152 | 165 | 180 | 184 | 210 | 221 | 254 | 285 | 362 | 372 | 380 |
| ℓ129 | 0 | 9 | 16 | 21 | 68 | 82 | 88 | 128 | 130 | 131 | 153 | 166 | 181 | 185 | 211 | 222 | 255 | 286 | 363 | 373 |
| ℓ130 | 1 | 10 | 17 | 22 | 69 | 83 | 89 | 129 | 131 | 132 | 154 | 167 | 182 | 186 | 212 | 223 | 256 | 287 | 364 | 374 |
| ℓ131 | 2 | 11 | 18 | 23 | 70 | 84 | 90 | 130 | 132 | 133 | 155 | 168 | 183 | 187 | 213 | 224 | 257 | 288 | 365 | 375 |
| ℓ132 | 3 | 12 | 19 | 24 | 71 | 85 | 91 | 131 | 133 | 134 | 156 | 169 | 184 | 188 | 214 | 225 | 258 | 289 | 366 | 376 |
| ℓ133 | 4 | 13 | 20 | 25 | 72 | 86 | 92 | 132 | 134 | 135 | 157 | 170 | 185 | 189 | 215 | 226 | 259 | 290 | 367 | 377 |
| ℓ134 | 5 | 14 | 21 | 26 | 73 | 87 | 93 | 133 | 135 | 136 | 158 | 171 | 186 | 190 | 216 | 227 | 260 | 291 | 368 | 378 |
| ℓ135 | 6 | 15 | 22 | 27 | 74 | 88 | 94 | 134 | 136 | 137 | 159 | 172 | 187 | 191 | 217 | 228 | 261 | 292 | 369 | 379 |
| ℓ136 | 7 | 16 | 23 | 28 | 75 | 89 | 95 | 135 | 137 | 138 | 160 | 173 | 188 | 192 | 218 | 229 | 262 | 293 | 370 | 380 |
| ℓ137 | 0 | 8 | 17 | 24 | 29 | 76 | 90 | 96 | 136 | 138 | 139 | 161 | 174 | 189 | 193 | 219 | 230 | 263 | 294 | 371 |
| ℓ138 | 1 | 9 | 18 | 25 | 30 | 77 | 91 | 97 | 137 | 139 | 140 | 162 | 175 | 190 | 194 | 220 | 231 | 264 | 295 | 372 |
| ℓ139 | 2 | 10 | 19 | 26 | 31 | 78 | 92 | 98 | 138 | 140 | 141 | 163 | 176 | 191 | 195 | 221 | 232 | 265 | 296 | 373 |
| ℓ140 | 3 | 11 | 20 | 27 | 32 | 79 | 93 | 99 | 139 | 141 | 142 | 164 | 177 | 192 | 196 | 222 | 233 | 266 | 297 | 374 |
| ℓ141 | 4 | 12 | 21 | 28 | 33 | 80 | 94 | 100 | 140 | 142 | 143 | 165 | 178 | 193 | 197 | 223 | 234 | 267 | 298 | 375 |
| ℓ142 | 5 | 13 | 22 | 29 | 34 | 81 | 95 | 101 | 141 | 143 | 144 | 166 | 179 | 194 | 198 | 224 | 235 | 268 | 299 | 376 |
| ℓ143 | 6 | 14 | 23 | 30 | 35 | 82 | 96 | 102 | 142 | 144 | 145 | 167 | 180 | 195 | 199 | 225 | 236 | 269 | 300 | 377 |
| ℓ144 | 7 | 15 | 24 | 31 | 36 | 83 | 97 | 103 | 143 | 145 | 146 | 168 | 181 | 196 | 200 | 226 | 237 | 270 | 301 | 378 |
| ℓ145 | 8 | 16 | 25 | 32 | 37 | 84 | 98 | 104 | 144 | 146 | 147 | 169 | 182 | 197 | 201 | 227 | 238 | 271 | 302 | 379 |
| ℓ146 | 9 | 17 | 26 | 33 | 38 | 85 | 99 | 105 | 145 | 147 | 148 | 170 | 183 | 198 | 202 | 228 | 239 | 272 | 303 | 380 |
| ℓ147 | 0 | 10 | 18 | 27 | 34 | 39 | 86 | 100 | 106 | 146 | 148 | 149 | 171 | 184 | 199 | 203 | 229 | 240 | 273 | 304 |
| ℓ148 | 1 | 11 | 19 | 28 | 35 | 40 | 87 | 101 | 107 | 147 | 149 | 150 | 172 | 185 | 200 | 204 | 230 | 241 | 274 | 305 |
| ℓ149 | 2 | 12 | 20 | 29 | 36 | 41 | 88 | 102 | 108 | 148 | 150 | 151 | 173 | 186 | 201 | 205 | 231 | 242 | 275 | 306 |
| ℓ150 | 3 | 13 | 21 | 30 | 37 | 42 | 89 | 103 | 109 | 149 | 151 | 152 | 174 | 187 | 202 | 206 | 232 | 243 | 276 | 307 |
| ℓ151 | 4 | 14 | 22 | 31 | 38 | 43 | 90 | 104 | 110 | 150 | 152 | 153 | 175 | 188 | 203 | 207 | 233 | 244 | 277 | 308 |
| ℓ152 | 5 | 15 | 23 | 32 | 39 | 44 | 91 | 105 | 111 | 151 | 153 | 154 | 176 | 189 | 204 | 208 | 234 | 245 | 278 | 309 |
| ℓ153 | 6 | 16 | 24 | 33 | 40 | 45 | 92 | 106 | 112 | 152 | 154 | 155 | 177 | 190 | 205 | 209 | 235 | 246 | 279 | 310 |
| ℓ154 | 7 | 17 | 25 | 34 | 41 | 46 | 93 | 107 | 113 | 153 | 155 | 156 | 178 | 191 | 206 | 210 | 236 | 247 | 280 | 311 |
| ℓ155 | 8 | 18 | 26 | 35 | 42 | 47 | 94 | 108 | 114 | 154 | 156 | 157 | 179 | 192 | 207 | 211 | 237 | 248 | 281 | 312 |
| ℓ156 | 9 | 19 | 27 | 36 | 43 | 48 | 95 | 109 | 115 | 155 | 157 | 158 | 180 | 193 | 208 | 212 | 238 | 249 | 282 | 313 |
| ℓ157 | 10 | 20 | 28 | 37 | 44 | 49 | 96 | 110 | 116 | 156 | 158 | 159 | 181 | 194 | 209 | 213 | 239 | 250 | 283 | 314 |
| ℓ158 | 11 | 21 | 29 | 38 | 45 | 50 | 97 | 111 | 117 | 157 | 159 | 160 | 182 | 195 | 210 | 214 | 240 | 251 | 284 | 315 |
| ℓ159 | 12 | 22 | 30 | 39 | 46 | 51 | 98 | 112 | 118 | 158 | 160 | 161 | 183 | 196 | 211 | 215 | 241 | 252 | 285 | 316 |
| ℓ160 | 13 | 23 | 31 | 40 | 47 | 52 | 99 | 113 | 119 | 159 | 161 | 162 | 184 | 197 | 212 | 216 | 242 | 253 | 286 | 317 |
| ℓ161 | 14 | 24 | 32 | 41 | 48 | 53 | 100 | 114 | 120 | 160 | 162 | 163 | 185 | 198 | 213 | 217 | 243 | 254 | 287 | 318 |
| ℓ162 | 15 | 25 | 33 | 42 | 49 | 54 | 101 | 115 | 121 | 161 | 163 | 164 | 186 | 199 | 214 | 218 | 244 | 255 | 288 | 319 |
| ℓ163 | 16 | 26 | 34 | 43 | 50 | 55 | 102 | 116 | 122 | 162 | 164 | 165 | 187 | 200 | 215 | 219 | 245 | 256 | 289 | 320 |
| ℓ164 | 17 | 27 | 35 | 44 | 51 | 56 | 103 | 117 | 123 | 163 | 165 | 166 | 188 | 201 | 216 | 220 | 246 | 257 | 290 | 321 |
| ℓ165 | 18 | 28 | 36 | 45 | 52 | 57 | 104 | 118 | 124 | 164 | 166 | 167 | 189 | 202 | 217 | 221 | 247 | 258 | 291 | 322 |
| ℓ166 | 19 | 29 | 37 | 46 | 53 | 58 | 105 | 119 | 125 | 165 | 167 | 168 | 190 | 203 | 218 | 222 | 248 | 259 | 292 | 323 |
| ℓ167 | 20 | 30 | 38 | 47 | 54 | 59 | 106 | 120 | 126 | 166 | 168 | 169 | 191 | 204 | 219 | 223 | 249 | 260 | 293 | 324 |
| ℓ168 | 21 | 31 | 39 | 48 | 55 | 60 | 107 | 121 | 127 | 167 | 169 | 170 | 192 | 205 | 220 | 224 | 250 | 261 | 294 | 325 |
| ℓ169 | 22 | 32 | 40 | 49 | 56 | 61 | 108 | 122 | 128 | 168 | 170 | 171 | 193 | 206 | 221 | 225 | 251 | 262 | 295 | 326 |
| ℓ170 | 23 | 33 | 41 | 50 | 57 | 62 | 109 | 123 | 129 | 169 | 171 | 172 | 194 | 207 | 222 | 226 | 252 | 263 | 296 | 327 |
| ℓ171 | 24 | 34 | 42 | 51 | 58 | 63 | 110 | 124 | 130 | 170 | 172 | 173 | 195 | 208 | 223 | 227 | 253 | 264 | 297 | 328 |
| ℓ172 | 25 | 35 | 43 | 52 | 59 | 64 | 111 | 125 | 131 | 171 | 173 | 174 | 196 | 209 | 224 | 228 | 254 | 265 | 298 | 329 |
| ℓ173 | 26 | 36 | 44 | 53 | 60 | 65 | 112 | 126 | 132 | 172 | 174 | 175 | 197 | 210 | 225 | 229 | 255 | 266 | 299 | 330 |
| ℓ174 | 27 | 37 | 45 | 54 | 61 | 66 | 113 | 127 | 133 | 173 | 175 | 176 | 198 | 211 | 226 | 230 | 256 | 267 | 300 | 331 |
| ℓ175 | 28 | 38 | 46 | 55 | 62 | 67 | 114 | 128 | 134 | 174 | 176 | 177 | 199 | 212 | 227 | 231 | 257 | 268 | 301 | 332 |
| ℓ176 | 29 | 39 | 47 | 56 | 63 | 68 | 115 | 129 | 135 | 175 | 177 | 178 | 200 | 213 | 228 | 232 | 258 | 269 | 302 | 333 |
| ℓ177 | 30 | 40 | 48 | 57 | 64 | 69 | 116 | 130 | 136 | 176 | 178 | 179 | 201 | 214 | 229 | 233 | 259 | 270 | 303 | 334 |
| ℓ178 | 31 | 41 | 49 | 58 | 65 | 70 | 117 | 131 | 137 | 177 | 179 | 180 | 202 | 215 | 230 | 234 | 260 | 271 | 304 | 335 |
| ℓ179 | 32 | 42 | 50 | 59 | 66 | 71 | 118 | 132 | 138 | 178 | 180 | 181 | 203 | 216 | 231 | 235 | 261 | 272 | 305 | 336 |
| ℓ180 | 33 | 43 | 51 | 60 | 67 | 72 | 119 | 133 | 139 | 179 | 181 | 182 | 204 | 217 | 232 | 236 | 262 | 273 | 306 | 337 |
| ℓ181 | 34 | 44 | 52 | 61 | 68 | 73 | 120 | 134 | 140 | 180 | 182 | 183 | 205 | 218 | 233 | 237 | 263 | 274 | 307 | 338 |
| ℓ182 | 35 | 45 | 53 | 62 | 69 | 74 | 121 | 135 | 141 | 181 | 183 | 184 | 206 | 219 | 234 | 238 | 264 | 275 | 308 | 339 |
| ℓ183 | 36 | 46 | 54 | 63 | 70 | 75 | 122 | 136 | 142 | 182 | 184 | 185 | 207 | 220 | 235 | 239 | 265 | 276 | 309 | 340 |
| ℓ184 | 37 | 47 | 55 | 64 | 71 | 76 | 123 | 137 | 143 | 183 | 185 | 186 | 208 | 221 | 236 | 240 | 266 | 277 | 310 | 341 |
| ℓ185 | 38 | 48 | 56 | 65 | 72 | 77 | 124 | 138 | 144 | 184 | 186 | 187 | 209 | 222 | 237 | 241 | 267 | 278 | 311 | 342 |
| ℓ186 | 39 | 49 | 57 | 66 | 73 | 78 | 125 | 139 | 145 | 185 | 187 | 188 | 210 | 223 | 238 | 242 | 268 | 279 | 312 | 343 |
| ℓ187 | 40 | 50 | 58 | 67 | 74 | 79 | 126 | 140 | 146 | 186 | 188 | 189 | 211 | 224 | 239 | 243 | 269 | 280 | 313 | 344 |
| ℓ188 | 41 | 51 | 59 | 68 | 75 | 80 | 127 | 141 | 147 | 187 | 189 | 190 | 212 | 225 | 240 | 244 | 270 | 281 | 314 | 345 |
| ℓ189 | 42 | 52 | 60 | 69 | 76 | 81 | 128 | 142 | 148 | 188 | 190 | 191 | 213 | 226 | 241 | 245 | 271 | 282 | 315 | 346 |
| ℓ190 | 43 | 53 | 61 | 70 | 77 | 82 | 129 | 143 | 149 | 189 | 191 | 192 | 214 | 227 | 242 | 246 | 272 | 283 | 316 | 347 |
| ℓ191 | 44 | 54 | 62 | 71 | 78 | 83 | 130 | 144 | 150 | 190 | 192 | 193 | 215 | 228 | 243 | 247 | 273 | 284 | 317 | 348 |
| ℓ192 | 45 | 55 | 63 | 72 | 79 | 84 | 131 | 145 | 151 | 191 | 193 | 194 | 216 | 229 | 244 | 248 | 274 | 285 | 318 | 349 |
| ℓ193 | 46 | 56 | 64 | 73 | 80 | 85 | 132 | 146 | 152 | 192 | 194 | 195 | 217 | 230 | 245 | 249 | 275 | 286 | 319 | 350 |
| ℓ194 | 47 | 57 | 65 | 74 | 81 | 86 | 133 | 147 | 153 | 193 | 195 | 196 | 218 | 231 | 246 | 250 | 276 | 287 | 320 | 351 |
| ℓ195 | 48 | 58 | 66 | 75 | 82 | 87 | 134 | 148 | 154 | 194 | 196 | 197 | 219 | 232 | 247 | 251 | 277 | 288 | 321 | 352 |
| ℓ196 | 49 | 59 | 67 | 76 | 83 | 88 | 135 | 149 | 155 | 195 | 197 | 198 | 220 | 233 | 248 | 252 | 278 | 289 | 322 | 353 |
| ℓ197 | 50 | 60 | 68 | 77 | 84 | 89 | 136 | 150 | 156 | 196 | 198 | 199 | 221 | 234 | 249 | 253 | 279 | 290 | 323 | 354 |
| ℓ198 | 51 | 61 | 69 | 78 | 85 | 90 | 137 | 151 | 157 | 197 | 199 | 200 | 222 | 235 | 250 | 254 | 280 | 291 | 324 | 355 |
| ℓ199 | 52 | 62 | 70 | 79 | 86 | 91 | 138 | 152 | 158 | 198 | 200 | 201 | 223 | 236 | 251 | 255 | 281 | 292 | 325 | 356 |
| ℓ200 | 53 | 63 | 71 | 80 | 87 | 92 | 139 | 153 | 159 | 199 | 201 | 202 | 224 | 237 | 252 | 256 | 282 | 293 | 326 | 357 |
| ℓ201 | 54 | 64 | 72 | 81 | 88 | 93 | 140 | 154 | 160 | 200 | 202 | 203 | 225 | 238 | 253 | 257 | 283 | 294 | 327 | 358 |
| ℓ202 | 55 | 65 | 73 | 82 | 89 | 94 | 141 | 155 | 161 | 201 | 203 | 204 | 226 | 239 | 254 | 258 | 284 | 295 | 328 | 359 |
| ℓ203 | 56 | 66 | 74 | 83 | 90 | 95 | 142 | 156 | 162 | 202 | 204 | 205 | 227 | 240 | 255 | 259 | 285 | 296 | 329 | 360 |
| ℓ204 | 57 | 67 | 75 | 84 | 91 | 96 | 143 | 157 | 163 | 203 | 205 | 206 | 228 | 241 | 256 | 260 | 286 | 297 | 330 | 361 |
| ℓ205 | 58 | 68 | 76 | 85 | 92 | 97 | 144 | 158 | 164 | 204 | 206 | 207 | 229 | 242 | 257 | 261 | 287 | 298 | 331 | 362 |
| ℓ206 | 59 | 69 | 77 | 86 | 93 | 98 | 145 | 159 | 165 | 205 | 207 | 208 | 230 | 243 | 258 | 262 | 288 | 299 | 332 | 363 |
| ℓ207 | 60 | 70 | 78 | 87 | 94 | 99 | 146 | 160 | 166 | 206 | 208 | 209 | 231 | 244 | 259 | 263 | 289 | 300 | 333 | 364 |
| ℓ208 | 61 | 71 | 79 | 88 | 95 | 100 | 147 | 161 | 167 | 207 | 209 | 210 | 232 | 245 | 260 | 264 | 290 | 301 | 334 | 365 |
| ℓ209 | 62 | 72 | 80 | 89 | 96 | 101 | 148 | 162 | 168 | 208 | 210 | 211 | 233 | 246 | 261 | 265 | 291 | 302 | 335 | 366 |
| ℓ210 | 63 | 73 | 81 | 90 | 97 | 102 | 149 | 163 | 169 | 209 | 211 | 212 | 234 | 247 | 262 | 266 | 292 | 303 | 336 | 367 |
| ℓ211 | 64 | 74 | 82 | 91 | 98 | 103 | 150 | 164 | 170 | 210 | 212 | 213 | 235 | 248 | 263 | 267 | 293 | 304 | 337 | 368 |
| ℓ212 | 65 | 75 | 83 | 92 | 99 | 104 | 151 | 165 | 171 | 211 | 213 | 214 | 236 | 249 | 264 | 268 | 294 | 305 | 338 | 369 |
| ℓ213 | 66 | 76 | 84 | 93 | 100 | 105 | 152 | 166 | 172 | 212 | 214 | 215 | 237 | 250 | 265 | 269 | 295 | 306 | 339 | 370 |
| ℓ214 | 67 | 77 | 85 | 94 | 101 | 106 | 153 | 167 | 173 | 213 | 215 | 216 | 238 | 251 | 266 | 270 | 296 | 307 | 340 | 371 |
| ℓ215 | 68 | 78 | 86 | 95 | 102 | 107 | 154 | 168 | 174 | 214 | 216 | 217 | 239 | 252 | 267 | 271 | 297 | 308 | 341 | 372 |
| ℓ216 | 69 | 79 | 87 | 96 | 103 | 108 | 155 | 169 | 175 | 215 | 217 | 218 | 240 | 253 | 268 | 272 | 298 | 309 | 342 | 373 |
| ℓ217 | 70 | 80 | 88 | 97 | 104 | 109 | 156 | 170 | 176 | 216 | 218 | 219 | 241 | 254 | 269 | 273 | 299 | 310 | 343 | 374 |
| ℓ218 | 71 | 81 | 89 | 98 | 105 | 110 | 157 | 171 | 177 | 217 | 219 | 220 | 242 | 255 | 270 | 274 | 300 | 311 | 344 | 375 |
| ℓ219 | 72 | 82 | 90 | 99 | 106 | 111 | 158 | 172 | 178 | 218 | 220 | 221 | 243 | 256 | 271 | 275 | 301 | 312 | 345 | 376 |
| ℓ220 | 73 | 83 | 91 | 100 | 107 | 112 | 159 | 173 | 179 | 219 | 221 | 222 | 244 | 257 | 272 | 276 | 302 | 313 | 346 | 377 |
| ℓ221 | 74 | 84 | 92 | 101 | 108 | 113 | 160 | 174 | 180 | 220 | 222 | 223 | 245 | 258 | 273 | 277 | 303 | 314 | 347 | 378 |
| ℓ222 | 75 | 85 | 93 | 102 | 109 | 114 | 161 | 175 | 181 | 221 | 223 | 224 | 246 | 259 | 274 | 278 | 304 | 315 | 348 | 379 |
| ℓ223 | 76 | 86 | 94 | 103 | 110 | 115 | 162 | 176 | 182 | 222 | 224 | 225 | 247 | 260 | 275 | 279 | 305 | 316 | 349 | 380 |
| ℓ224 | 0 | 77 | 87 | 95 | 104 | 111 | 116 | 163 | 177 | 183 | 223 | 225 | 226 | 248 | 261 | 276 | 280 | 306 | 317 | 350 |
| ℓ225 | 1 | 78 | 88 | 96 | 105 | 112 | 117 | 164 | 178 | 184 | 224 | 226 | 227 | 249 | 262 | 277 | 281 | 307 | 318 | 351 |
| ℓ226 | 2 | 79 | 89 | 97 | 106 | 113 | 118 | 165 | 179 | 185 | 225 | 227 | 228 | 250 | 263 | 278 | 282 | 308 | 319 | 352 |
| ℓ227 | 3 | 80 | 90 | 98 | 107 | 114 | 119 | 166 | 180 | 186 | 226 | 228 | 229 | 251 | 264 | 279 | 283 | 309 | 320 | 353 |
| ℓ228 | 4 | 81 | 91 | 99 | 108 | 115 | 120 | 167 | 181 | 187 | 227 | 229 | 230 | 252 | 265 | 280 | 284 | 310 | 321 | 354 |
| ℓ229 | 5 | 82 | 92 | 100 | 109 | 116 | 121 | 168 | 182 | 188 | 228 | 230 | 231 | 253 | 266 | 281 | 285 | 311 | 322 | 355 |
| ℓ230 | 6 | 83 | 93 | 101 | 110 | 117 | 122 | 169 | 183 | 189 | 229 | 231 | 232 | 254 | 267 | 282 | 286 | 312 | 323 | 356 |
| ℓ231 | 7 | 84 | 94 | 102 | 111 | 118 | 123 | 170 | 184 | 190 | 230 | 232 | 233 | 255 | 268 | 283 | 287 | 313 | 324 | 357 |
| ℓ232 | 8 | 85 | 95 | 103 | 112 | 119 | 124 | 171 | 185 | 191 | 231 | 233 | 234 | 256 | 269 | 284 | 288 | 314 | 325 | 358 |
| ℓ233 | 9 | 86 | 96 | 104 | 113 | 120 | 125 | 172 | 186 | 192 | 232 | 234 | 235 | 257 | 270 | 285 | 289 | 315 | 326 | 359 |
| ℓ234 | 10 | 87 | 97 | 105 | 114 | 121 | 126 | 173 | 187 | 193 | 233 | 235 | 236 | 258 | 271 | 286 | 290 | 316 | 327 | 360 |
| ℓ235 | 11 | 88 | 98 | 106 | 115 | 122 | 127 | 174 | 188 | 194 | 234 | 236 | 237 | 259 | 272 | 287 | 291 | 317 | 328 | 361 |
| ℓ236 | 12 | 89 | 99 | 107 | 116 | 123 | 128 | 175 | 189 | 195 | 235 | 237 | 238 | 260 | 273 | 288 | 292 | 318 | 329 | 362 |
| ℓ237 | 13 | 90 | 100 | 108 | 117 | 124 | 129 | 176 | 190 | 196 | 236 | 238 | 239 | 261 | 274 | 289 | 293 | 319 | 330 | 363 |
| ℓ238 | 14 | 91 | 101 | 109 | 118 | 125 | 130 | 177 | 191 | 197 | 237 | 239 | 240 | 262 | 275 | 290 | 294 | 320 | 331 | 364 |
| ℓ239 | 15 | 92 | 102 | 110 | 119 | 126 | 131 | 178 | 192 | 198 | 238 | 240 | 241 | 263 | 276 | 291 | 295 | 321 | 332 | 365 |
| ℓ240 | 16 | 93 | 103 | 111 | 120 | 127 | 132 | 179 | 193 | 199 | 239 | 241 | 242 | 264 | 277 | 292 | 296 | 322 | 333 | 366 |
| ℓ241 | 17 | 94 | 104 | 112 | 121 | 128 | 133 | 180 | 194 | 200 | 240 | 242 | 243 | 265 | 278 | 293 | 297 | 323 | 334 | 367 |
| ℓ242 | 18 | 95 | 105 | 113 | 122 | 129 | 134 | 181 | 195 | 201 | 241 | 243 | 244 | 266 | 279 | 294 | 298 | 324 | 335 | 368 |
| ℓ243 | 19 | 96 | 106 | 114 | 123 | 130 | 135 | 182 | 196 | 202 | 242 | 244 | 245 | 267 | 280 | 295 | 299 | 325 | 336 | 369 |
| ℓ244 | 20 | 97 | 107 | 115 | 124 | 131 | 136 | 183 | 197 | 203 | 243 | 245 | 246 | 268 | 281 | 296 | 300 | 326 | 337 | 370 |
| ℓ245 | 21 | 98 | 108 | 116 | 125 | 132 | 137 | 184 | 198 | 204 | 244 | 246 | 247 | 269 | 282 | 297 | 301 | 327 | 338 | 371 |
| ℓ246 | 22 | 99 | 109 | 117 | 126 | 133 | 138 | 185 | 199 | 205 | 245 | 247 | 248 | 270 | 283 | 298 | 302 | 328 | 339 | 372 |
| ℓ247 | 23 | 100 | 110 | 118 | 127 | 134 | 139 | 186 | 200 | 206 | 246 | 248 | 249 | 271 | 284 | 299 | 303 | 329 | 340 | 373 |
| ℓ248 | 24 | 101 | 111 | 119 | 128 | 135 | 140 | 187 | 201 | 207 | 247 | 249 | 250 | 272 | 285 | 300 | 304 | 330 | 341 | 374 |
| ℓ249 | 25 | 102 | 112 | 120 | 129 | 136 | 141 | 188 | 202 | 208 | 248 | 250 | 251 | 273 | 286 | 301 | 305 | 331 | 342 | 375 |
| ℓ250 | 26 | 103 | 113 | 121 | 130 | 137 | 142 | 189 | 203 | 209 | 249 | 251 | 252 | 274 | 287 | 302 | 306 | 332 | 343 | 376 |
| ℓ251 | 27 | 104 | 114 | 122 | 131 | 138 | 143 | 190 | 204 | 210 | 250 | 252 | 253 | 275 | 288 | 303 | 307 | 333 | 344 | 377 |
| ℓ252 | 28 | 105 | 115 | 123 | 132 | 139 | 144 | 191 | 205 | 211 | 251 | 253 | 254 | 276 | 289 | 304 | 308 | 334 | 345 | 378 |
| ℓ253 | 29 | 106 | 116 | 124 | 133 | 140 | 145 | 192 | 206 | 212 | 252 | 254 | 255 | 277 | 290 | 305 | 309 | 335 | 346 | 379 |
| ℓ254 | 30 | 107 | 117 | 125 | 134 | 141 | 146 | 193 | 207 | 213 | 253 | 255 | 256 | 278 | 291 | 306 | 310 | 336 | 347 | 380 |
| ℓ255 | 0 | 31 | 108 | 118 | 126 | 135 | 142 | 147 | 194 | 208 | 214 | 254 | 256 | 257 | 279 | 292 | 307 | 311 | 337 | 348 |
| ℓ256 | 1 | 32 | 109 | 119 | 127 | 136 | 143 | 148 | 195 | 209 | 215 | 255 | 257 | 258 | 280 | 293 | 308 | 312 | 338 | 349 |
| ℓ257 | 2 | 33 | 110 | 120 | 128 | 137 | 144 | 149 | 196 | 210 | 216 | 256 | 258 | 259 | 281 | 294 | 309 | 313 | 339 | 350 |
| ℓ258 | 3 | 34 | 111 | 121 | 129 | 138 | 145 | 150 | 197 | 211 | 217 | 257 | 259 | 260 | 282 | 295 | 310 | 314 | 340 | 351 |
| ℓ259 | 4 | 35 | 112 | 122 | 130 | 139 | 146 | 151 | 198 | 212 | 218 | 258 | 260 | 261 | 283 | 296 | 311 | 315 | 341 | 352 |
| ℓ260 | 5 | 36 | 113 | 123 | 131 | 140 | 147 | 152 | 199 | 213 | 219 | 259 | 261 | 262 | 284 | 297 | 312 | 316 | 342 | 353 |
| ℓ261 | 6 | 37 | 114 | 124 | 132 | 141 | 148 | 153 | 200 | 214 | 220 | 260 | 262 | 263 | 285 | 298 | 313 | 317 | 343 | 354 |
| ℓ262 | 7 | 38 | 115 | 125 | 133 | 142 | 149 | 154 | 201 | 215 | 221 | 261 | 263 | 264 | 286 | 299 | 314 | 318 | 344 | 355 |
| ℓ263 | 8 | 39 | 116 | 126 | 134 | 143 | 150 | 155 | 202 | 216 | 222 | 262 | 264 | 265 | 287 | 300 | 315 | 319 | 345 | 356 |
| ℓ264 | 9 | 40 | 117 | 127 | 135 | 144 | 151 | 156 | 203 | 217 | 223 | 263 | 265 | 266 | 288 | 301 | 316 | 320 | 346 | 357 |
| ℓ265 | 10 | 41 | 118 | 128 | 136 | 145 | 152 | 157 | 204 | 218 | 224 | 264 | 266 | 267 | 289 | 302 | 317 | 321 | 347 | 358 |
| ℓ266 | 11 | 42 | 119 | 129 | 137 | 146 | 153 | 158 | 205 | 219 | 225 | 265 | 267 | 268 | 290 | 303 | 318 | 322 | 348 | 359 |
| ℓ267 | 12 | 43 | 120 | 130 | 138 | 147 | 154 | 159 | 206 | 220 | 226 | 266 | 268 | 269 | 291 | 304 | 319 | 323 | 349 | 360 |
| ℓ268 | 13 | 44 | 121 | 131 | 139 | 148 | 155 | 160 | 207 | 221 | 227 | 267 | 269 | 270 | 292 | 305 | 320 | 324 | 350 | 361 |
| ℓ269 | 14 | 45 | 122 | 132 | 140 | 149 | 156 | 161 | 208 | 222 | 228 | 268 | 270 | 271 | 293 | 306 | 321 | 325 | 351 | 362 |
| ℓ270 | 15 | 46 | 123 | 133 | 141 | 150 | 157 | 162 | 209 | 223 | 229 | 269 | 271 | 272 | 294 | 307 | 322 | 326 | 352 | 363 |
| ℓ271 | 16 | 47 | 124 | 134 | 142 | 151 | 158 | 163 | 210 | 224 | 230 | 270 | 272 | 273 | 295 | 308 | 323 | 327 | 353 | 364 |
| ℓ272 | 17 | 48 | 125 | 135 | 143 | 152 | 159 | 164 | 211 | 225 | 231 | 271 | 273 | 274 | 296 | 309 | 324 | 328 | 354 | 365 |
| ℓ273 | 18 | 49 | 126 | 136 | 144 | 153 | 160 | 165 | 212 | 226 | 232 | 272 | 274 | 275 | 297 | 310 | 325 | 329 | 355 | 366 |
| ℓ274 | 19 | 50 | 127 | 137 | 145 | 154 | 161 | 166 | 213 | 227 | 233 | 273 | 275 | 276 | 298 | 311 | 326 | 330 | 356 | 367 |
| ℓ275 | 20 | 51 | 128 | 138 | 146 | 155 | 162 | 167 | 214 | 228 | 234 | 274 | 276 | 277 | 299 | 312 | 327 | 331 | 357 | 368 |
| ℓ276 | 21 | 52 | 129 | 139 | 147 | 156 | 163 | 168 | 215 | 229 | 235 | 275 | 277 | 278 | 300 | 313 | 328 | 332 | 358 | 369 |
| ℓ277 | 22 | 53 | 130 | 140 | 148 | 157 | 164 | 169 | 216 | 230 | 236 | 276 | 278 | 279 | 301 | 314 | 329 | 333 | 359 | 370 |
| ℓ278 | 23 | 54 | 131 | 141 | 149 | 158 | 165 | 170 | 217 | 231 | 237 | 277 | 279 | 280 | 302 | 315 | 330 | 334 | 360 | 371 |
| ℓ279 | 24 | 55 | 132 | 142 | 150 | 159 | 166 | 171 | 218 | 232 | 238 | 278 | 280 | 281 | 303 | 316 | 331 | 335 | 361 | 372 |
| ℓ280 | 25 | 56 | 133 | 143 | 151 | 160 | 167 | 172 | 219 | 233 | 239 | 279 | 281 | 282 | 304 | 317 | 332 | 336 | 362 | 373 |
| ℓ281 | 26 | 57 | 134 | 144 | 152 | 161 | 168 | 173 | 220 | 234 | 240 | 280 | 282 | 283 | 305 | 318 | 333 | 337 | 363 | 374 |
| ℓ282 | 27 | 58 | 135 | 145 | 153 | 162 | 169 | 174 | 221 | 235 | 241 | 281 | 283 | 284 | 306 | 319 | 334 | 338 | 364 | 375 |
| ℓ283 | 28 | 59 | 136 | 146 | 154 | 163 | 170 | 175 | 222 | 236 | 242 | 282 | 284 | 285 | 307 | 320 | 335 | 339 | 365 | 376 |
| ℓ284 | 29 | 60 | 137 | 147 | 155 | 164 | 171 | 176 | 223 | 237 | 243 | 283 | 285 | 286 | 308 | 321 | 336 | 340 | 366 | 377 |
| ℓ285 | 30 | 61 | 138 | 148 | 156 | 165 | 172 | 177 | 224 | 238 | 244 | 284 | 286 | 287 | 309 | 322 | 337 | 341 | 367 | 378 |
| ℓ286 | 31 | 62 | 139 | 149 | 157 | 166 | 173 | 178 | 225 | 239 | 245 | 285 | 287 | 288 | 310 | 323 | 338 | 342 | 368 | 379 |
| ℓ287 | 32 | 63 | 140 | 150 | 158 | 167 | 174 | 179 | 226 | 240 | 246 | 286 | 288 | 289 | 311 | 324 | 339 | 343 | 369 | 380 |
| ℓ288 | 0 | 33 | 64 | 141 | 151 | 159 | 168 | 175 | 180 | 227 | 241 | 247 | 287 | 289 | 290 | 312 | 325 | 340 | 344 | 370 |
| ℓ289 | 1 | 34 | 65 | 142 | 152 | 160 | 169 | 176 | 181 | 228 | 242 | 248 | 288 | 290 | 291 | 313 | 326 | 341 | 345 | 371 |
| ℓ290 | 2 | 35 | 66 | 143 | 153 | 161 | 170 | 177 | 182 | 229 | 243 | 249 | 289 | 291 | 292 | 314 | 327 | 342 | 346 | 372 |
| ℓ291 | 3 | 36 | 67 | 144 | 154 | 162 | 171 | 178 | 183 | 230 | 244 | 250 | 290 | 292 | 293 | 315 | 328 | 343 | 347 | 373 |
| ℓ292 | 4 | 37 | 68 | 145 | 155 | 163 | 172 | 179 | 184 | 231 | 245 | 251 | 291 | 293 | 294 | 316 | 329 | 344 | 348 | 374 |
| ℓ293 | 5 | 38 | 69 | 146 | 156 | 164 | 173 | 180 | 185 | 232 | 246 | 252 | 292 | 294 | 295 | 317 | 330 | 345 | 349 | 375 |
| ℓ294 | 6 | 39 | 70 | 147 | 157 | 165 | 174 | 181 | 186 | 233 | 247 | 253 | 293 | 295 | 296 | 318 | 331 | 346 | 350 | 376 |
| ℓ295 | 7 | 40 | 71 | 148 | 158 | 166 | 175 | 182 | 187 | 234 | 248 | 254 | 294 | 296 | 297 | 319 | 332 | 347 | 351 | 377 |
| ℓ296 | 8 | 41 | 72 | 149 | 159 | 167 | 176 | 183 | 188 | 235 | 249 | 255 | 295 | 297 | 298 | 320 | 333 | 348 | 352 | 378 |
| ℓ297 | 9 | 42 | 73 | 150 | 160 | 168 | 177 | 184 | 189 | 236 | 250 | 256 | 296 | 298 | 299 | 321 | 334 | 349 | 353 | 379 |
| ℓ298 | 10 | 43 | 74 | 151 | 161 | 169 | 178 | 185 | 190 | 237 | 251 | 257 | 297 | 299 | 300 | 322 | 335 | 350 | 354 | 380 |
| ℓ299 | 0 | 11 | 44 | 75 | 152 | 162 | 170 | 179 | 186 | 191 | 238 | 252 | 258 | 298 | 300 | 301 | 323 | 336 | 351 | 355 |
| ℓ300 | 1 | 12 | 45 | 76 | 153 | 163 | 171 | 180 | 187 | 192 | 239 | 253 | 259 | 299 | 301 | 302 | 324 | 337 | 352 | 356 |
| ℓ301 | 2 | 13 | 46 | 77 | 154 | 164 | 172 | 181 | 188 | 193 | 240 | 254 | 260 | 300 | 302 | 303 | 325 | 338 | 353 | 357 |
| ℓ302 | 3 | 14 | 47 | 78 | 155 | 165 | 173 | 182 | 189 | 194 | 241 | 255 | 261 | 301 | 303 | 304 | 326 | 339 | 354 | 358 |
| ℓ303 | 4 | 15 | 48 | 79 | 156 | 166 | 174 | 183 | 190 | 195 | 242 | 256 | 262 | 302 | 304 | 305 | 327 | 340 | 355 | 359 |
| ℓ304 | 5 | 16 | 49 | 80 | 157 | 167 | 175 | 184 | 191 | 196 | 243 | 257 | 263 | 303 | 305 | 306 | 328 | 341 | 356 | 360 |
| ℓ305 | 6 | 17 | 50 | 81 | 158 | 168 | 176 | 185 | 192 | 197 | 244 | 258 | 264 | 304 | 306 | 307 | 329 | 342 | 357 | 361 |
| ℓ306 | 7 | 18 | 51 | 82 | 159 | 169 | 177 | 186 | 193 | 198 | 245 | 259 | 265 | 305 | 307 | 308 | 330 | 343 | 358 | 362 |
| ℓ307 | 8 | 19 | 52 | 83 | 160 | 170 | 178 | 187 | 194 | 199 | 246 | 260 | 266 | 306 | 308 | 309 | 331 | 344 | 359 | 363 |
| ℓ308 | 9 | 20 | 53 | 84 | 161 | 171 | 179 | 188 | 195 | 200 | 247 | 261 | 267 | 307 | 309 | 310 | 332 | 345 | 360 | 364 |
| ℓ309 | 10 | 21 | 54 | 85 | 162 | 172 | 180 | 189 | 196 | 201 | 248 | 262 | 268 | 308 | 310 | 311 | 333 | 346 | 361 | 365 |
| ℓ310 | 11 | 22 | 55 | 86 | 163 | 173 | 181 | 190 | 197 | 202 | 249 | 263 | 269 | 309 | 311 | 312 | 334 | 347 | 362 | 366 |
| ℓ311 | 12 | 23 | 56 | 87 | 164 | 174 | 182 | 191 | 198 | 203 | 250 | 264 | 270 | 310 | 312 | 313 | 335 | 348 | 363 | 367 |
| ℓ312 | 13 | 24 | 57 | 88 | 165 | 175 | 183 | 192 | 199 | 204 | 251 | 265 | 271 | 311 | 313 | 314 | 336 | 349 | 364 | 368 |
| ℓ313 | 14 | 25 | 58 | 89 | 166 | 176 | 184 | 193 | 200 | 205 | 252 | 266 | 272 | 312 | 314 | 315 | 337 | 350 | 365 | 369 |
| ℓ314 | 15 | 26 | 59 | 90 | 167 | 177 | 185 | 194 | 201 | 206 | 253 | 267 | 273 | 313 | 315 | 316 | 338 | 351 | 366 | 370 |
| ℓ315 | 16 | 27 | 60 | 91 | 168 | 178 | 186 | 195 | 202 | 207 | 254 | 268 | 274 | 314 | 316 | 317 | 339 | 352 | 367 | 371 |
| ℓ316 | 17 | 28 | 61 | 92 | 169 | 179 | 187 | 196 | 203 | 208 | 255 | 269 | 275 | 315 | 317 | 318 | 340 | 353 | 368 | 372 |
| ℓ317 | 18 | 29 | 62 | 93 | 170 | 180 | 188 | 197 | 204 | 209 | 256 | 270 | 276 | 316 | 318 | 319 | 341 | 354 | 369 | 373 |
| ℓ318 | 19 | 30 | 63 | 94 | 171 | 181 | 189 | 198 | 205 | 210 | 257 | 271 | 277 | 317 | 319 | 320 | 342 | 355 | 370 | 374 |
| ℓ319 | 20 | 31 | 64 | 95 | 172 | 182 | 190 | 199 | 206 | 211 | 258 | 272 | 278 | 318 | 320 | 321 | 343 | 356 | 371 | 375 |
| ℓ320 | 21 | 32 | 65 | 96 | 173 | 183 | 191 | 200 | 207 | 212 | 259 | 273 | 279 | 319 | 321 | 322 | 344 | 357 | 372 | 376 |
| ℓ321 | 22 | 33 | 66 | 97 | 174 | 184 | 192 | 201 | 208 | 213 | 260 | 274 | 280 | 320 | 322 | 323 | 345 | 358 | 373 | 377 |
| ℓ322 | 23 | 34 | 67 | 98 | 175 | 185 | 193 | 202 | 209 | 214 | 261 | 275 | 281 | 321 | 323 | 324 | 346 | 359 | 374 | 378 |
| ℓ323 | 24 | 35 | 68 | 99 | 176 | 186 | 194 | 203 | 210 | 215 | 262 | 276 | 282 | 322 | 324 | 325 | 347 | 360 | 375 | 379 |
| ℓ324 | 25 | 36 | 69 | 100 | 177 | 187 | 195 | 204 | 211 | 216 | 263 | 277 | 283 | 323 | 325 | 326 | 348 | 361 | 376 | 380 |
| ℓ325 | 0 | 26 | 37 | 70 | 101 | 178 | 188 | 196 | 205 | 212 | 217 | 264 | 278 | 284 | 324 | 326 | 327 | 349 | 362 | 377 |
| ℓ326 | 1 | 27 | 38 | 71 | 102 | 179 | 189 | 197 | 206 | 213 | 218 | 265 | 279 | 285 | 325 | 327 | 328 | 350 | 363 | 378 |
| ℓ327 | 2 | 28 | 39 | 72 | 103 | 180 | 190 | 198 | 207 | 214 | 219 | 266 | 280 | 286 | 326 | 328 | 329 | 351 | 364 | 379 |
| ℓ328 | 3 | 29 | 40 | 73 | 104 | 181 | 191 | 199 | 208 | 215 | 220 | 267 | 281 | 287 | 327 | 329 | 330 | 352 | 365 | 380 |
| ℓ329 | 0 | 4 | 30 | 41 | 74 | 105 | 182 | 192 | 200 | 209 | 216 | 221 | 268 | 282 | 288 | 328 | 330 | 331 | 353 | 366 |
| ℓ330 | 1 | 5 | 31 | 42 | 75 | 106 | 183 | 193 | 201 | 210 | 217 | 222 | 269 | 283 | 289 | 329 | 331 | 332 | 354 | 367 |
| ℓ331 | 2 | 6 | 32 | 43 | 76 | 107 | 184 | 194 | 202 | 211 | 218 | 223 | 270 | 284 | 290 | 330 | 332 | 333 | 355 | 368 |
| ℓ332 | 3 | 7 | 33 | 44 | 77 | 108 | 185 | 195 | 203 | 212 | 219 | 224 | 271 | 285 | 291 | 331 | 333 | 334 | 356 | 369 |
| ℓ333 | 4 | 8 | 34 | 45 | 78 | 109 | 186 | 196 | 204 | 213 | 220 | 225 | 272 | 286 | 292 | 332 | 334 | 335 | 357 | 370 |
| ℓ334 | 5 | 9 | 35 | 46 | 79 | 110 | 187 | 197 | 205 | 214 | 221 | 226 | 273 | 287 | 293 | 333 | 335 | 336 | 358 | 371 |
| ℓ335 | 6 | 10 | 36 | 47 | 80 | 111 | 188 | 198 | 206 | 215 | 222 | 227 | 274 | 288 | 294 | 334 | 336 | 337 | 359 | 372 |
| ℓ336 | 7 | 11 | 37 | 48 | 81 | 112 | 189 | 199 | 207 | 216 | 223 | 228 | 275 | 289 | 295 | 335 | 337 | 338 | 360 | 373 |
| ℓ337 | 8 | 12 | 38 | 49 | 82 | 113 | 190 | 200 | 208 | 217 | 224 | 229 | 276 | 290 | 296 | 336 | 338 | 339 | 361 | 374 |
| ℓ338 | 9 | 13 | 39 | 50 | 83 | 114 | 191 | 201 | 209 | 218 | 225 | 230 | 277 | 291 | 297 | 337 | 339 | 340 | 362 | 375 |
| ℓ339 | 10 | 14 | 40 | 51 | 84 | 115 | 192 | 202 | 210 | 219 | 226 | 231 | 278 | 292 | 298 | 338 | 340 | 341 | 363 | 376 |
| ℓ340 | 11 | 15 | 41 | 52 | 85 | 116 | 193 | 203 | 211 | 220 | 227 | 232 | 279 | 293 | 299 | 339 | 341 | 342 | 364 | 377 |
| ℓ341 | 12 | 16 | 42 | 53 | 86 | 117 | 194 | 204 | 212 | 221 | 228 | 233 | 280 | 294 | 300 | 340 | 342 | 343 | 365 | 378 |
| ℓ342 | 13 | 17 | 43 | 54 | 87 | 118 | 195 | 205 | 213 | 222 | 229 | 234 | 281 | 295 | 301 | 341 | 343 | 344 | 366 | 379 |
| ℓ343 | 14 | 18 | 44 | 55 | 88 | 119 | 196 | 206 | 214 | 223 | 230 | 235 | 282 | 296 | 302 | 342 | 344 | 345 | 367 | 380 |
| ℓ344 | 0 | 15 | 19 | 45 | 56 | 89 | 120 | 197 | 207 | 215 | 224 | 231 | 236 | 283 | 297 | 303 | 343 | 345 | 346 | 368 |
| ℓ345 | 1 | 16 | 20 | 46 | 57 | 90 | 121 | 198 | 208 | 216 | 225 | 232 | 237 | 284 | 298 | 304 | 344 | 346 | 347 | 369 |
| ℓ346 | 2 | 17 | 21 | 47 | 58 | 91 | 122 | 199 | 209 | 217 | 226 | 233 | 238 | 285 | 299 | 305 | 345 | 347 | 348 | 370 |
| ℓ347 | 3 | 18 | 22 | 48 | 59 | 92 | 123 | 200 | 210 | 218 | 227 | 234 | 239 | 286 | 300 | 306 | 346 | 348 | 349 | 371 |
| ℓ348 | 4 | 19 | 23 | 49 | 60 | 93 | 124 | 201 | 211 | 219 | 228 | 235 | 240 | 287 | 301 | 307 | 347 | 349 | 350 | 372 |
| ℓ349 | 5 | 20 | 24 | 50 | 61 | 94 | 125 | 202 | 212 | 220 | 229 | 236 | 241 | 288 | 302 | 308 | 348 | 350 | 351 | 373 |
| ℓ350 | 6 | 21 | 25 | 51 | 62 | 95 | 126 | 203 | 213 | 221 | 230 | 237 | 242 | 289 | 303 | 309 | 349 | 351 | 352 | 374 |
| ℓ351 | 7 | 22 | 26 | 52 | 63 | 96 | 127 | 204 | 214 | 222 | 231 | 238 | 243 | 290 | 304 | 310 | 350 | 352 | 353 | 375 |
| ℓ352 | 8 | 23 | 27 | 53 | 64 | 97 | 128 | 205 | 215 | 223 | 232 | 239 | 244 | 291 | 305 | 311 | 351 | 353 | 354 | 376 |
| ℓ353 | 9 | 24 | 28 | 54 | 65 | 98 | 129 | 206 | 216 | 224 | 233 | 240 | 245 | 292 | 306 | 312 | 352 | 354 | 355 | 377 |
| ℓ354 | 10 | 25 | 29 | 55 | 66 | 99 | 130 | 207 | 217 | 225 | 234 | 241 | 246 | 293 | 307 | 313 | 353 | 355 | 356 | 378 |
| ℓ355 | 11 | 26 | 30 | 56 | 67 | 100 | 131 | 208 | 218 | 226 | 235 | 242 | 247 | 294 | 308 | 314 | 354 | 356 | 357 | 379 |
| ℓ356 | 12 | 27 | 31 | 57 | 68 | 101 | 132 | 209 | 219 | 227 | 236 | 243 | 248 | 295 | 309 | 315 | 355 | 357 | 358 | 380 |
| ℓ357 | 0 | 13 | 28 | 32 | 58 | 69 | 102 | 133 | 210 | 220 | 228 | 237 | 244 | 249 | 296 | 310 | 316 | 356 | 358 | 359 |
| ℓ358 | 1 | 14 | 29 | 33 | 59 | 70 | 103 | 134 | 211 | 221 | 229 | 238 | 245 | 250 | 297 | 311 | 317 | 357 | 359 | 360 |
| ℓ359 | 2 | 15 | 30 | 34 | 60 | 71 | 104 | 135 | 212 | 222 | 230 | 239 | 246 | 251 | 298 | 312 | 318 | 358 | 360 | 361 |
| ℓ360 | 3 | 16 | 31 | 35 | 61 | 72 | 105 | 136 | 213 | 223 | 231 | 240 | 247 | 252 | 299 | 313 | 319 | 359 | 361 | 362 |
| ℓ361 | 4 | 17 | 32 | 36 | 62 | 73 | 106 | 137 | 214 | 224 | 232 | 241 | 248 | 253 | 300 | 314 | 320 | 360 | 362 | 363 |
| ℓ362 | 5 | 18 | 33 | 37 | 63 | 74 | 107 | 138 | 215 | 225 | 233 | 242 | 249 | 254 | 301 | 315 | 321 | 361 | 363 | 364 |
| ℓ363 | 6 | 19 | 34 | 38 | 64 | 75 | 108 | 139 | 216 | 226 | 234 | 243 | 250 | 255 | 302 | 316 | 322 | 362 | 364 | 365 |
| ℓ364 | 7 | 20 | 35 | 39 | 65 | 76 | 109 | 140 | 217 | 227 | 235 | 244 | 251 | 256 | 303 | 317 | 323 | 363 | 365 | 366 |
| ℓ365 | 8 | 21 | 36 | 40 | 66 | 77 | 110 | 141 | 218 | 228 | 236 | 245 | 252 | 257 | 304 | 318 | 324 | 364 | 366 | 367 |
| ℓ366 | 9 | 22 | 37 | 41 | 67 | 78 | 111 | 142 | 219 | 229 | 237 | 246 | 253 | 258 | 305 | 319 | 325 | 365 | 367 | 368 |
| ℓ367 | 10 | 23 | 38 | 42 | 68 | 79 | 112 | 143 | 220 | 230 | 238 | 247 | 254 | 259 | 306 | 320 | 326 | 366 | 368 | 369 |
| ℓ368 | 11 | 24 | 39 | 43 | 69 | 80 | 113 | 144 | 221 | 231 | 239 | 248 | 255 | 260 | 307 | 321 | 327 | 367 | 369 | 370 |
| ℓ369 | 12 | 25 | 40 | 44 | 70 | 81 | 114 | 145 | 222 | 232 | 240 | 249 | 256 | 261 | 308 | 322 | 328 | 368 | 370 | 371 |
| ℓ370 | 13 | 26 | 41 | 45 | 71 | 82 | 115 | 146 | 223 | 233 | 241 | 250 | 257 | 262 | 309 | 323 | 329 | 369 | 371 | 372 |
| ℓ371 | 14 | 27 | 42 | 46 | 72 | 83 | 116 | 147 | 224 | 234 | 242 | 251 | 258 | 263 | 310 | 324 | 330 | 370 | 372 | 373 |
| ℓ372 | 15 | 28 | 43 | 47 | 73 | 84 | 117 | 148 | 225 | 235 | 243 | 252 | 259 | 264 | 311 | 325 | 331 | 371 | 373 | 374 |
| ℓ373 | 16 | 29 | 44 | 48 | 74 | 85 | 118 | 149 | 226 | 236 | 244 | 253 | 260 | 265 | 312 | 326 | 332 | 372 | 374 | 375 |
| ℓ374 | 17 | 30 | 45 | 49 | 75 | 86 | 119 | 150 | 227 | 237 | 245 | 254 | 261 | 266 | 313 | 327 | 333 | 373 | 375 | 376 |
| ℓ375 | 18 | 31 | 46 | 50 | 76 | 87 | 120 | 151 | 228 | 238 | 246 | 255 | 262 | 267 | 314 | 328 | 334 | 374 | 376 | 377 |
| ℓ376 | 19 | 32 | 47 | 51 | 77 | 88 | 121 | 152 | 229 | 239 | 247 | 256 | 263 | 268 | 315 | 329 | 335 | 375 | 377 | 378 |
| ℓ377 | 20 | 33 | 48 | 52 | 78 | 89 | 122 | 153 | 230 | 240 | 248 | 257 | 264 | 269 | 316 | 330 | 336 | 376 | 378 | 379 |
| ℓ378 | 21 | 34 | 49 | 53 | 79 | 90 | 123 | 154 | 231 | 241 | 249 | 258 | 265 | 270 | 317 | 331 | 337 | 377 | 379 | 380 |
| ℓ379 | 0 | 22 | 35 | 50 | 54 | 80 | 91 | 124 | 155 | 232 | 242 | 250 | 259 | 266 | 271 | 318 | 332 | 338 | 378 | 380 |
| ℓ380 | 0 | 1 | 23 | 36 | 51 | 55 | 81 | 92 | 125 | 156 | 233 | 243 | 251 | 260 | 267 | 272 | 319 | 333 | 339 | 379 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Innamorati, S. Quasi-Configurations Derived by Special Arrangements of Lines. Axioms 2024, 13, 321. https://doi.org/10.3390/axioms13050321
Innamorati S. Quasi-Configurations Derived by Special Arrangements of Lines. Axioms. 2024; 13(5):321. https://doi.org/10.3390/axioms13050321
Chicago/Turabian StyleInnamorati, Stefano. 2024. "Quasi-Configurations Derived by Special Arrangements of Lines" Axioms 13, no. 5: 321. https://doi.org/10.3390/axioms13050321
APA StyleInnamorati, S. (2024). Quasi-Configurations Derived by Special Arrangements of Lines. Axioms, 13(5), 321. https://doi.org/10.3390/axioms13050321







