Weighted Convolution for Quaternion Linear Canonical Cosine Transform and Its Application
Abstract
:1. Introduction
2. Preliminaries
2.1. Quaternion Algebra
2.2. Linear Canonical Integral Transform
3. Quaternion Linear Canonical Cosine and Sine Transform
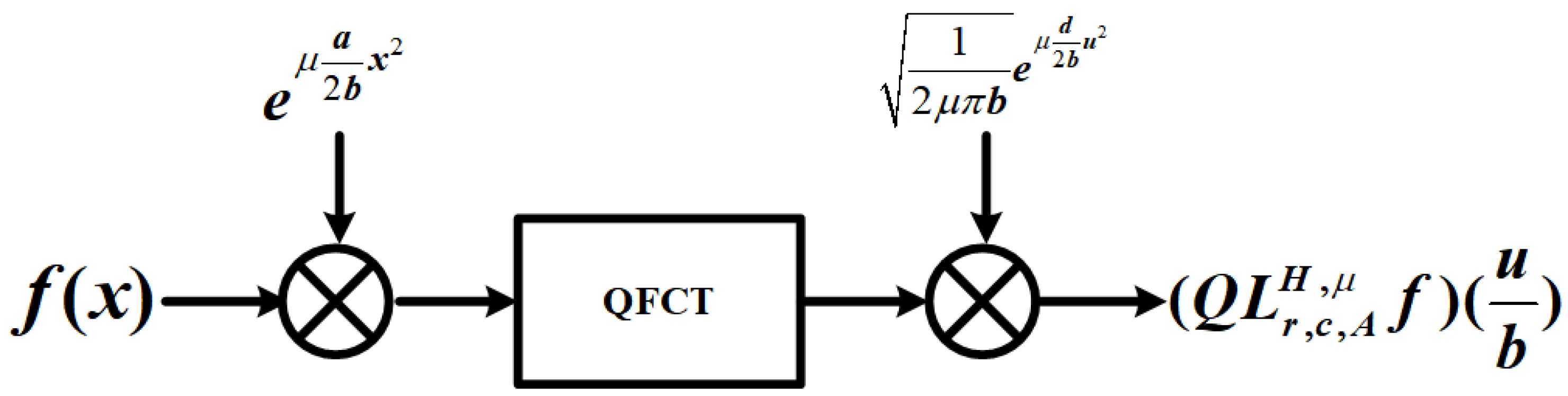
3.1. Definition of QLCcT and QLCsT
3.2. Properties for QLCcT
4. Convolution and Correlation for QLCcT
4.1. Convolution for QLCcT
4.2. Correlation for QLCcT
5. Example and Application for QLCcT
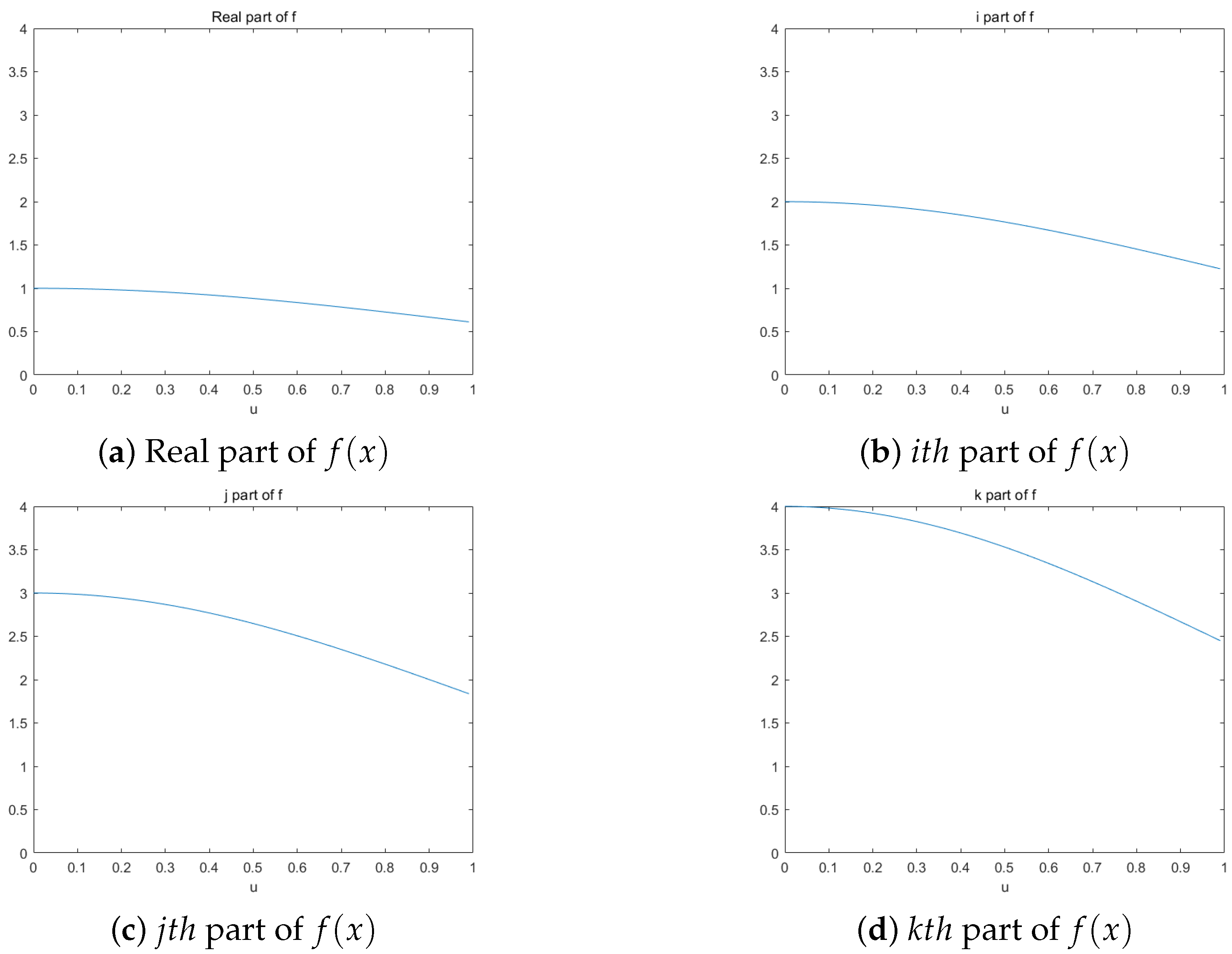
5.1. Example and Simulations for QLCcT
5.2. Application of QLCcT
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Moshinsky, M.; Quesne, C. Linear canonical transformations and their unitary representations. J. Math. Phys. 1971, 12, 1772–1780. [Google Scholar] [CrossRef]
- Feng, Q.; Li, B.Z. Convolution and correlation theorems for the two-dimensional linear canonical transform and its applications. IET Signal Process. 2016, 10, 125–132. [Google Scholar] [CrossRef]
- Xin, H.C.; Li, B.Z.; Bai, X. A Novel Sub-Nyquist FRI Sampling and Reconstruction Method in Linear Canonical Transform Domain. Circuits Syst. Signal Process. 2021, 40, 6173–6192. [Google Scholar] [CrossRef]
- Zhang, Z.C. Jittered Sampling in Linear Canonical Domain. IEEE Commun. Lett. 2020, 24, 1529–1533. [Google Scholar] [CrossRef]
- Chen, J.Y.; Li, B.Z. Fast numerical calculation of the offset linear canonical transform. J. Opt. Soc. Am. A—Opt. Image Sci. Vis. 2023, 40, 427–442. [Google Scholar] [CrossRef]
- Koç, A.; Bartan, B.; Ozaktas, H.M. Discrete Linear Canonical Transform Based on Hyperdifferential Operators. IEEE Trans. Signal Process. 2019, 67, 2237–2248. [Google Scholar] [CrossRef]
- Urynbassarova, D.; Teali, A.A. Convolution, Correlation, and uncertainty principles for the quaternion offset linear canonical transform. Mathematics 2023, 11, 2201. [Google Scholar] [CrossRef]
- Kundu, M.; Prasad, A.; Verma, R.K. Multidimensional linear canonical transform and convolution. J. Ramanujan Math. Soc. 2022, 37, 159–171. [Google Scholar]
- Wei, D.Y.; Li, Y.M. Convolution and Multichannel Sampling for the Offset Linear Canonical Transform and Their Applications. IEEE Trans. Signal Process. 2019, 67, 6009–6024. [Google Scholar] [CrossRef]
- Bhat, M.Y.; Dar, A.H. Multiresolution analysis for linear canonical S transform. Adv. Oper. Theory 2021, 6, 68. [Google Scholar] [CrossRef]
- Feng, Q.; Li, B.Z.; Rassias, J.M. Weighted Heisenberg-Pauli-Weyl uncertainty principles for the linear canonical transform. Signal Process. 2019, 165, 209–221. [Google Scholar] [CrossRef]
- Bruni, V.; Cardinali, M.L.; Vitulano, D. An MDL-Based Wavelet Scattering Features Selection for Signal Classification. Axioms 2022, 11, 376. [Google Scholar] [CrossRef]
- Miao, H.X. Generalized spectrum analysis of Chirp Cyclostationary signals associate with linear canonical transform. Signal Process. 2024, 218, 109385. [Google Scholar] [CrossRef]
- Xin, H.C.; Li, B.Z. On a new Wigner-Ville distribution associated with linear canonical transform. EURASIP J. Adv. Signal Process. 2021, 2021, 56. [Google Scholar] [CrossRef]
- Li, Z.W.; Gao, W.B. Inequalities for the Windowed Linear Canonical Transform of Complex Functions. Axioms 2023, 12, 554. [Google Scholar] [CrossRef]
- Bahri, M.; Karim, S.A.A. Novel Uncertainty Principles Concerning Linear Canonical Wavelet Transform. Mathematics 2022, 10, 3502. [Google Scholar] [CrossRef]
- Li, Y.M.; Jiang, M.J.; Wei, D.; Deng, Y. Novel Image Encryption Algorithm Based on Compressive Sensing and a Two-Dimensional Linear Canonical Transform. Fractal Fract. 2024, 8, 92. [Google Scholar] [CrossRef]
- Qasim, I.M.; Mohammed, E.A. Optical image encryption based on linear canonical transform with sparse representation. Opt. Commun. 2023, 533, 129262. [Google Scholar] [CrossRef]
- Pei, S.C.; Ding, J.J. Fractional cosine, sine, and Hartley transforms. IEEE Trans. Signal Process. 2002, 50, 1661–1680. [Google Scholar]
- Wang, R.B.; Feng, Q.; Ji, J.Y. The discrete convolution for fractional cosine-sine series and its application in convolution equations. AIMS Math. 2024, 9, 2641–2656. [Google Scholar] [CrossRef]
- Xiang, Y.; Yuan, S.; Feng, Q. Fractional Fourier cosine and sine Laplace weighted convolution and its application. IET Signal Process. 2023, 17, e12170. [Google Scholar] [CrossRef]
- Chen, B.J.; Yu, M.; Su, Q.T. Fractional quaternion cosine transform and its application in color image copy-move forgery detection. Multimed. Tools Appl. 2019, 78, 8057–8073. [Google Scholar] [CrossRef]
- Feng, Q.; Li, B.Z. Convolution theorem for fractional cosine-sine transform and its application. Math. Meth. Appl. Sci. 2017, 40, 3651–3665. [Google Scholar] [CrossRef]
- Pei, S.C.; Yeh, M.H. The discrete fractional cosine and sine transforms. IEEE Trans. Signal Process. 2001, 49, 1198–1207. [Google Scholar]
- Hamilton, W.R. Elements of Quaternions; Longmans Green: London, UK, 1866. [Google Scholar]
- Kantor, I.L.; Solodovnikov, A.S. Hypercomplex Number: An Elementary Introduction to Algebras; Springer: New York, NY, USA, 1989. [Google Scholar]
- Bujack, R.N.; Bie, H.D. Convolution products for hypercomplex Fourier transforms. J. Math. Imaging Vis. 2014, 48, 606–624. [Google Scholar] [CrossRef]
- Brahim, K.; Tefjeni, E. Uncertainty principle for the two sided quaternion windowed Fourier transform. J. Pseudo-Differ. Oper. 2020, 11, 159–185. [Google Scholar] [CrossRef]
- Xu, G.L.; Wang, X.T.; Xu, X.G. Fractional quaternion Fourier transform, convolution and correlation. Signal Process. 2008, 88, 2511–2517. [Google Scholar]
- Mei, Y.Y.; Feng, Q.; Gao, X.X.; Zhao, Y.B. Convolution theorem associated with the QWFRFT. Chin. J. Electron. 2023, 32, 485–492. [Google Scholar] [CrossRef]
- Bahri, M.; Ashino, R.; Makis, V. Two-dimensional quaternion linear canonical transform: Properties, convolution, correlation and uncertainty principle. J. Math. 2019, 2019, 1062979. [Google Scholar] [CrossRef]
- Hu, X.X.; Cheng, D.; Kou, K.I. Convolution theorem associated with quaternion linear canonical transforms and applications. Signal Process. 2023, 201, 108743. [Google Scholar] [CrossRef]
- Gao, W.B.; Li, B.Z. Quaternion windowed linear canonical transform of two-dimensional signals. Adv. Appl. Clifford Algebr. 2020, 30, 16. [Google Scholar] [CrossRef]
- Yang, H.H.; Feng, Q.; Wang, X.X.; Urynbassarova, D.; Teali, A.A. Reduced Biquaternion Windowed Linear Canonical Transform: Properties and Applications. Mathematics 2024, 12, 743. [Google Scholar] [CrossRef]
- Urynbassarova, D.; El Haoui, Y.; Zhang, F. Uncertainty Principles for Wigner-Ville Distribution Associated with the Quaternion Offset Linear Canonical Transform. Circuits Syst. Signal Process. 2023, 42, 385–404. [Google Scholar] [CrossRef]
- Bhat, M.Y.; Almanjahie, I.M.; Dar, A.H. Wigner-Ville distribution and ambiguity function associated with the quaternion offset linear canonical transform. Demonstr. Math. 2022, 55, 786–797. [Google Scholar] [CrossRef]
- Zayed, A. A convolution and product theorem for the fractional Fourier transform. IEEE Signal Process. Lett. 1998, 5, 101–103. [Google Scholar] [CrossRef]
- Bhandari, A.; Zayed, A. Convolution and product theorems for the special affine Fourier transform. In Frontiers in Orthogonal Polynomials and q-Series; World Scientific Publishers: New York, NY, USA, 2018; pp. 119–137. [Google Scholar]
- Nussbaumer, H.J. Fast Fourier Transform and Convolution Algorithms; Springer: New York, NY, USA, 1981. [Google Scholar]



| Order | Function | The Right-Side QLCcT |
|---|---|---|
| 1 | are constants. | |
| 2 | ||
| + | ||
| 3 | , where . | |
| 4 | ||
| + | ||
| 5 | ||
| 6 | ||
| 7 | ||
| 8 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, R.; Feng, Q. Weighted Convolution for Quaternion Linear Canonical Cosine Transform and Its Application. Axioms 2024, 13, 402. https://doi.org/10.3390/axioms13060402
Wang R, Feng Q. Weighted Convolution for Quaternion Linear Canonical Cosine Transform and Its Application. Axioms. 2024; 13(6):402. https://doi.org/10.3390/axioms13060402
Chicago/Turabian StyleWang, Rongbo, and Qiang Feng. 2024. "Weighted Convolution for Quaternion Linear Canonical Cosine Transform and Its Application" Axioms 13, no. 6: 402. https://doi.org/10.3390/axioms13060402
APA StyleWang, R., & Feng, Q. (2024). Weighted Convolution for Quaternion Linear Canonical Cosine Transform and Its Application. Axioms, 13(6), 402. https://doi.org/10.3390/axioms13060402






