The Unified Description of Abstract Convexity Structures
Abstract
:1. Introduction
2. Preliminaries
2.1. Abstract Convexity Space
- (1)
- ;
- (2)
- Ξ is closed under the intersection operation of its subsets, that is, if nonempty subset , then is in Ξ.
2.2. –Convex Space
- (1)
- for any , implies ;
- (2)
- for any , , we can discover a continuous mapping such that indicates . Where and represents the face of the n-dimensional standard simplex corresponding to . That is, if and , then .
- (1)
- For any , when , we have , i.e., ;
- (2)
- Define as , where and . For any , , where represents the face of the n-dimensional standard simplex corresponding to . For any , we can obtain , i.e., . Hence, we can discover a continuous mapping such that indicates .
2.3. –Convex Space
2.4. Order Convexity
- (1)
- ;
- (2)
- if , .
2.5. –Convex Space
2.6. –Convex
- (1)
- if , then ;
- (2)
- if and , then and for any , ;
- (3)
- if , and for some , then , where ;
- (4)
- if , the mapping from Δ to V is continuous, and
- (5)
- for all , there is a neighbourhood on the diagonal of V, such that for all n and , , , and hold for all .
2.7. Pasicki’s –Contractible Space
2.8. Komiya’s Convex Space
- (1)
- ;
- (2)
- , ;
- (3)
- , ;
- (4)
- .
2.9. Bielawski’s Simplicial Convexity
- (1)
- ;
- (2)
- , then .
- (1)
- for any , ;
- (2)
- for any , when , .
- (1)
- for all , ;
- (2)
- for all , , , , then .
2.10. Joó’s Pseudoconvexity
2.11. Horvath’s Pseudoconvex Space
- (1)
- for any , , .
- (2)
- for any , is continuous,
2.12. –Simplicial Convexity
2.13. –Space
2.14. –Convex Structure
2.15. –Space
- (1)
- for any , , , ;
- (2)
- a mapping , defined as , is continuous.
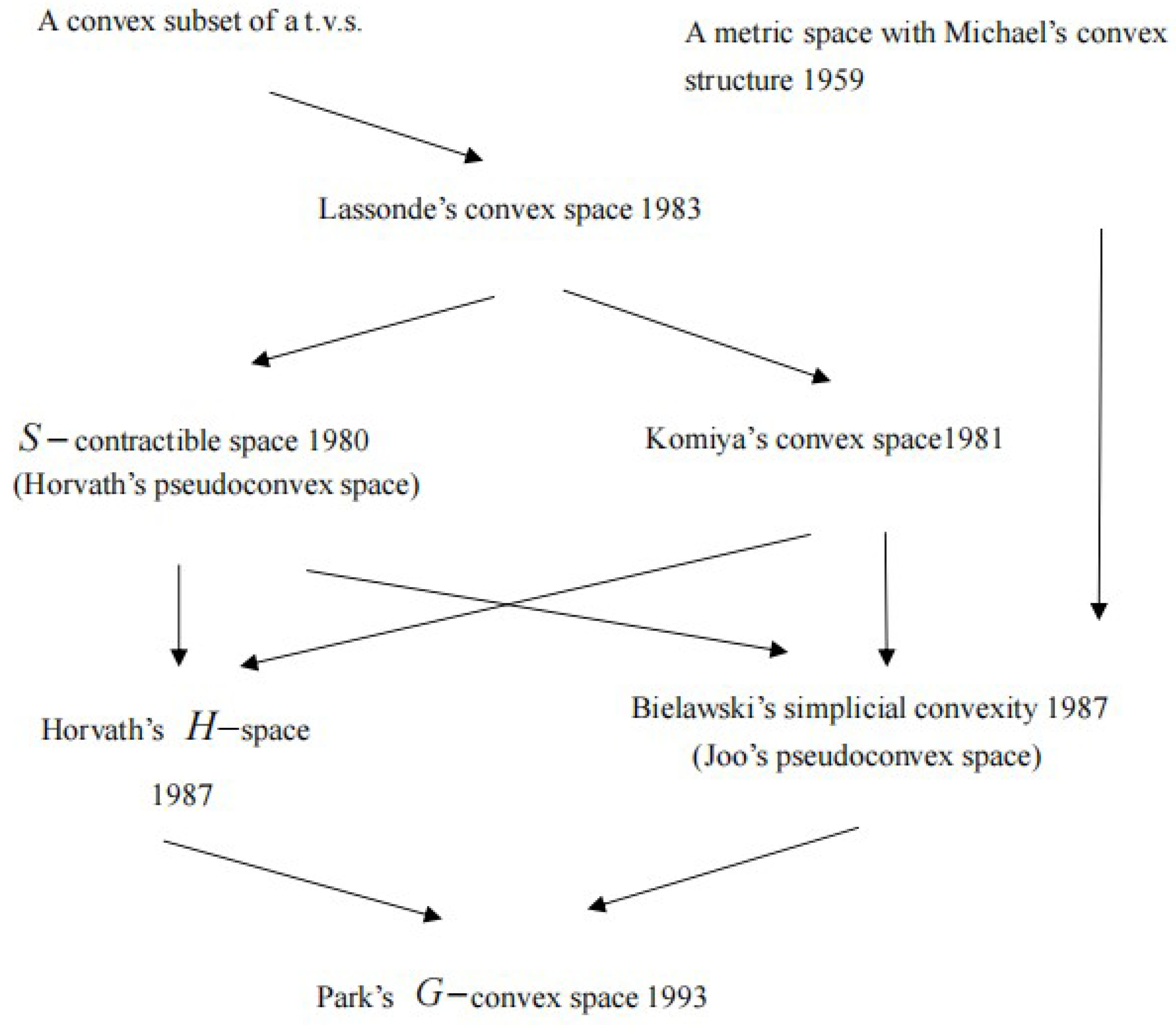
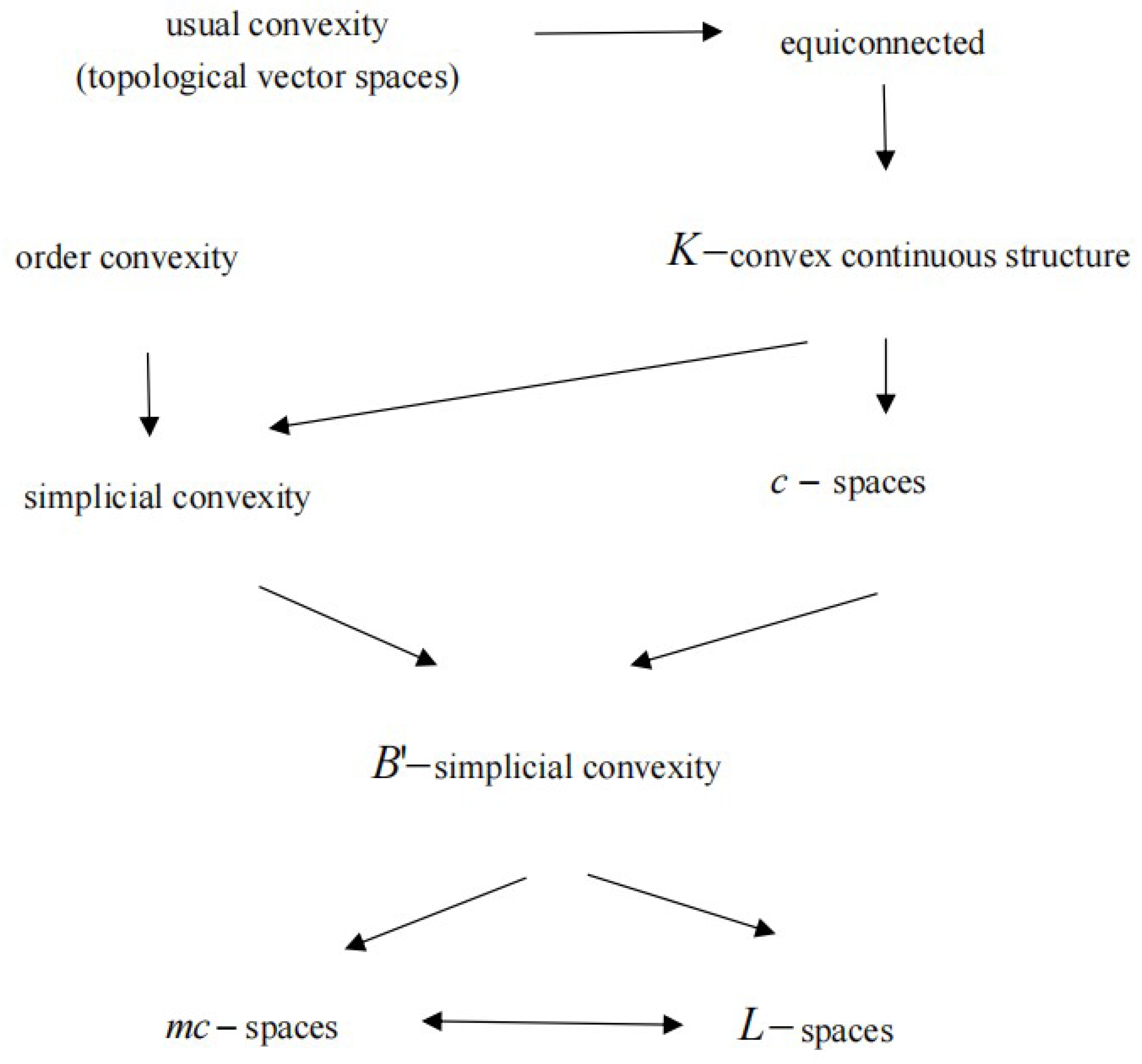
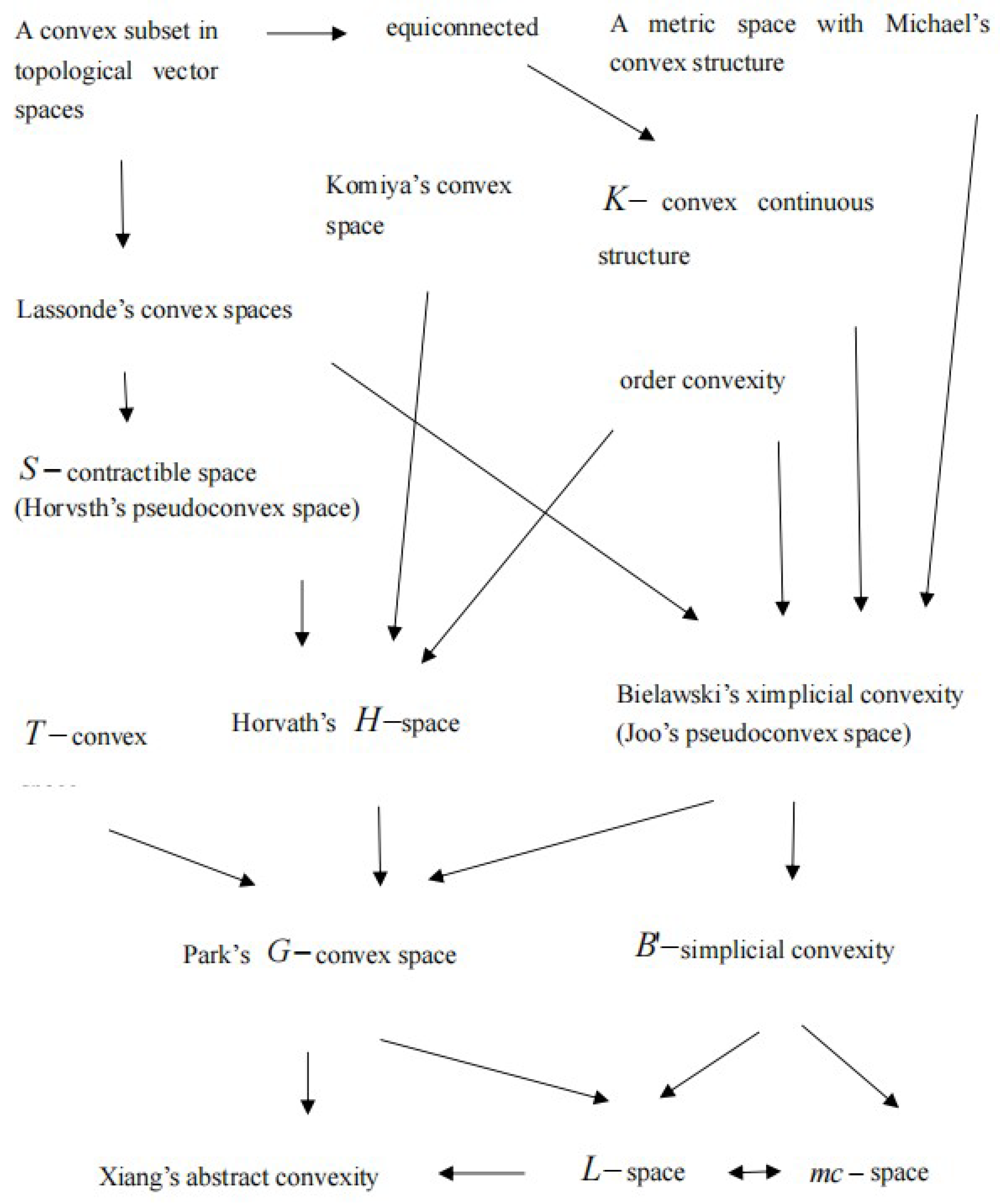
3. The Relation between the Various Convexities
- (1)
- for any , when , ;
- (2)
- for any , , we are capable of discovering a continuous mapping such that indicates .
- (1)
- ;
- (2)
- for a nonempty subset , it needs to be verified that . For any finite subset , we have for any . Because , i.e., is a –convex set, we can obtain . Thus, , and furthermore, .
- (1)
- –convex structure;
- (2)
- a convex structure on a topological semi-lattice space;
- (3)
- Michael’s convex strcture;
- (4)
- –convex structure.
- (1)
- –convex structure;
- (2)
- a convex structure on a topological semi-lattice space;
- (3)
- Michael’s convex strcture;
- (4)
- –convex structure;
- (5)
- –convex structure.
4. KKM Theory and Fixed Point Theory in Partially Convex Spaces
4.1. KKM Theory in Partially Convex Spaces
4.1.1. KKM Theory in –Convex Space
4.1.2. KKM Theory in –Space
- (1)
- for any , is compactly closed, i.e., for each compact subset , is closed in B;
- (2)
- there exists a compact set and an –compact set such that the weak –convex set Φ fulfills , .
- (1)
- for any , is compactly closed in V;
- (2)
- for any , is compactly open in V.
4.1.3. KKM Theory in Topological Ordered Space
- (1)
- is transferable closed values, for each ;
- (2)
- there exists such that cl is compact;
- (3)
- for any nonempty finite subset , .
4.2. Fixed Point Theory in Partially Convex Space
4.2.1. Fixed Point Theory in –Convex Space
4.2.2. Fixed Point Theory in –Space
- (1)
- for any , is an open set, and ;
- (2)
- for any , is –convex;
- (3)
- there exists such that is compact.
4.2.3. Fixed Point Theory in Topological Ordered Space
- (1)
- ;
- (2)
- for any , is nomempty. If , then .
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Michael, E. Convex structures and continuous selections. Can. J. Math. 1959, 11, 556–575. [Google Scholar] [CrossRef]
- Horvath, C.D. Some results on multivalued mappings and inequalities without convexity. In Nonlinear and Convex Analysis; CRC Press: Boca Raton, FL, USA, 1987; pp. 99–106. [Google Scholar]
- Tarafdar, E. A fixed point theorem in H-space and related results. Bull. Aust. Math. Soc. 1990, 42, 133–140. [Google Scholar] [CrossRef]
- Van de Vel, M. A selection theorem for topological convex structures. Trans. Am. Math. Soc. 1993, 336, 463–496. [Google Scholar] [CrossRef]
- Pasicki, L. Three fixed point theorems. Bull. Pol. Acad. Sci. 1980, 28, 173–175. [Google Scholar]
- Pasicki, L. Retracts in metric spaces. Proc. Am. Math. Soc. 1980, 78, 595–600. [Google Scholar] [CrossRef]
- Pasicki, L. A fixed point theory for multivalued mappings. Proc. Am. Math. Soc. 1981, 83, 781–789. [Google Scholar] [CrossRef]
- Komiya, H. Convexity on a topological space. Fundam. Math. 1981, 111, 107–113. [Google Scholar] [CrossRef]
- Lassonde, M. On the use of KKM multifunctions in fixed point theory and related topics. J. Math. Anal. Appl. 1983, 97, 151–201. [Google Scholar] [CrossRef]
- Horvath, C. Points fixes et coincidences pour les applications multivoques sans convexité. CR Acad. Sci. 1983, 296, 403–406. [Google Scholar]
- Joó, I. On some convexities. Acta Math. Hung. 1989, 54, 163–172. [Google Scholar] [CrossRef]
- Bielawski, R. Simplicial convexity and its applications. J. Math. Anal. Appl. 1987, 127, 155–171. [Google Scholar] [CrossRef]
- Park, S.; Kim, H. Admissible classes of multifunctions on generalized convex spaces. Proc. Collect. Nat. Sci. Seoul Natl. Univ. 1993, 18, 1–21. [Google Scholar]
- Park, S.; Kim, H. Coincidence theorems for admissible multifunctions on generalized convex spaces. J. Math. Anal. Appl. 1996, 197, 173–187. [Google Scholar] [CrossRef]
- Park, S. New topological versions of the Fan–Browder fixed point theorem. Nonlinear Anal. Theory Methods Appl. 2001, 47, 595–606. [Google Scholar] [CrossRef]
- Park, S. Fixed point theorems in locally G-convex spaces. Nonlinear Anal. Theory Methods Appl. 2002, 48, 869–879. [Google Scholar] [CrossRef]
- Park, S. Coincidence, almost fixed point, and minimax theorems on generalized convex spaces. J. Nonlinear Convex Anal. 2003, 4, 151–164. [Google Scholar]
- Park, S. The KKM, matching, and fixed point theorems in generalized convex spaces. Nonlinear Funct. Anal. Appl. 2006, 11, 139–154. [Google Scholar]
- Park, S. Five episodes related to generalized convex spaces. Nonlinear Funct. Anal. Appl. 1997, 2, 49–61. [Google Scholar]
- Park, S. A unified fixed point theory in generalized convex spaces. Acta Math. Sin. Engl. Ser. 2007, 23, 1509–1526. [Google Scholar] [CrossRef]
- Park, S. Continuous selection theorems in generalized convex spaces: Revisited. Nonlinear Anal. Forum 2011, 16, 21–33. [Google Scholar] [CrossRef]
- Briec, W.; Horvath, C.; De’partement, A. B-convexity. Optimization 2004, 53, 103–127. [Google Scholar] [CrossRef]
- Hong, M.S. Some properties of B-convexity. J. Nonlinear Sci. Appl. 2009, 2, 71–77. [Google Scholar]
- Xiang, S.W.; Yang, H. Some properties of abstract convexity structures on topological spaces. Nonlinear Anal. Theory Methods Appl. 2007, 67, 803–808. [Google Scholar] [CrossRef]
- Xiang, S.W.; Xia, S.Y. A further characteristic of abstract convexity structures on topological spaces. J. Math. Anal. Appl. 2007, 335, 716–723. [Google Scholar] [CrossRef]
- Xiang, S.W.; Xia, S.Y.; Chen, J. KKM lemmas and minimax inequality theorems in abstract convexity spaces. Fixed Point Theory Appl. 2013, 2013, 209. [Google Scholar] [CrossRef]
- Chen, Z.Y. Structure and Property of T-Convexity Spaces. J. Southwest Univ. Nat. Sci. 2012, 34, 106–108. [Google Scholar]
- Chen, Z.Y. KKM Lemma and Its Applications on T-Convexity Spaces. J. Nat. Sci. Hunan Norm. Univ. 2017, 40, 84–87. [Google Scholar]
- Chen, Z.Y. The existence theorems of Fan Ky’s points and Nash equilibrium points on T-Convex Spaces. Appl. Math. J. Chin. Univ. 2020, 35, 108–114. [Google Scholar]
- Chen, Z.Y. Fan Ky inequality theorem and its applications on T-convex spaces. Math. Pract. Theory 2021, 51, 211–218. [Google Scholar]
- Wang, C.C.; Chen, Z.Y.; Xia, S.Y.; Zhang, Z.Z.; Xu, G.J. KKM theorems under generalized H0-condition. J. Chongqing Norm. Univ. (Nat. Sci.) 2021, 38, 55–59. [Google Scholar]
- Chen, Z.Y. Fixed point theorems on locally T-convex spaces. J. Fuzhou Univ. (Nat. Sci. Ed.) 2019, 47, 157–160. [Google Scholar]
- Chen, Z.Y. Continuity selection and fixed point theorems on T-convex spaces. J. Northwest Norm. Univ. (Nat. Sci.) 2017, 53, 25–27. [Google Scholar]
- Xia, S.Y.; Wang, C.C.; Yang, Y.L.; Chen, Z.Y. R-KKM Lemma with an application in abstract convexity metric spaces. J. Southwest Norm. Univ. (Nat. Sci.) 2021, 46, 96–100. [Google Scholar]
- Llinares, J.V. Abstract convexity, some relations and applications. Optimization 2002, 51, 797–818. [Google Scholar] [CrossRef]
- Horvath, C.D. Contractibility and generalized convexity. J. Math. Anal. Appl. 1991, 156, 341–357. [Google Scholar] [CrossRef]
- Bardaro, C.; Ceppitelli, R. Some further generalizations of Knaster-Kuratowski-Mazurkiewicz theorem and minimax inequalities. J. Math. Anal. Appl. 1988, 132, 484–490. [Google Scholar] [CrossRef]
- Horvath, C.D.; Ciscar, J.V.L. Maximal elements and fixed points for binary relations on topological ordered spaces. J. Math. Econ. 1996, 25, 291–306. [Google Scholar] [CrossRef]
- Ben-El-Mechaiekh, H.; Chebbi, S.; Florenzano, M.; Llinares, J.V. Abstract convexity and fixed points. J. Math. Anal. Appl. 1998, 222, 138–150. [Google Scholar] [CrossRef]
- Horvath, C.D. Convexité généralisée et applications. Sém. Math. Supér 1990, 110, 81–99. [Google Scholar]
- Llinares, J.V. Unified treatment of the problem of existence of maximal elements in binary relations: A characterization. J. Math. Econ. 1998, 29, 285–302. [Google Scholar] [CrossRef]
- Park, S. The rise and fall of L-spaces. Adv. Theory Nonlinear Anal. Appl. 2020, 4, 152–166. [Google Scholar] [CrossRef]
- Park, S. The rise and fall of L-spaces, II. Adv. Theory Nonlinear Anal. Appl. 2021, 5, 7–24. [Google Scholar] [CrossRef]
- Park, S. The rise and fall of mc-spaces. Results Nonlinear Anal. 2021, 4, 21–32. [Google Scholar] [CrossRef]
- Knaster, B.; Kuratowski, K.; Mazurkiewicz, S. Ein Beweis des Fixpunktsatzes für n-dimensionale Simplexe. Fundam. Math. 1929, 14, 132–137. [Google Scholar] [CrossRef]
- Fan, K. A generalization of Tychonoff’s fixed point theorem. Math. Ann. 1961, 142, 305–310. [Google Scholar] [CrossRef]
- Park, S. Some coincidence theorems on acyclic multifunctions and applications to KKM theory. In Fixed Point Theory and Applications; World Scientific: Singapore, 1992; pp. 248–277. [Google Scholar]
- Park, S. Elements of the KKM theory for generalized convex spaces. J. Comput. Appl. Math. 2000, 7, 1–28. [Google Scholar] [CrossRef]
- Park, S.; Kim, H. Foundations of the KKM theory on generalized convex spaces. J. Math. Anal. Appl. 1997, 209, 551–571. [Google Scholar] [CrossRef]
- Park, S. New foundations of the KKM theory. J. Nonlinear Convex Anal. 2008, 9, 331–350. [Google Scholar]
- Ding, X.P. Generalized G-KKM theorems in generalized convex spaces and their applications. J. Math. Anal. Appl. 2002, 266, 21–37. [Google Scholar] [CrossRef]
- Tan, K.K. G-KKM theorem, minimax inequalities and saddle points. Nonlinear Anal. Theory Methods Appl. 1997, 30, 4151–4160. [Google Scholar]
- Ding, X.P.; Park, J.Y. Generalized vector equilibrium problems in generalized convex spaces. J. Optim. Theory Appl. 2004, 120, 327–353. [Google Scholar] [CrossRef]
- Park, S. Ninety years of the Brouwer fixed point theorem. Vietnam. J. Math 1999, 27, 187–222. [Google Scholar]
- Park, S. Review of recent studies on the KKM theory. Nonlinear Funct. Anal. Appl. 2013, 17, 459–470. [Google Scholar]
- Park, S. Review of recent studies on the KKM theory, II. Nonlinear Funct. Anal. Appl. 2014, 19, 145–157. [Google Scholar]
- Chang, S.; Ma, Y. Generalized KKM theorem on H-space with applications. J. Math. Anal. Appl. 1992, 163, 406–421. [Google Scholar] [CrossRef]
- Ding, X.P. New generalisations of an H-KKM type theorem and their applications. Bull. Aust. Math. Soc. 1993, 48, 451–464. [Google Scholar] [CrossRef]
- Ding, X.P.; Xia, F.Q. Generalized H-KKM type theorems in H-metric spaces with applications. Appl. Math. Mech. 2001, 22, 1140–1148. [Google Scholar] [CrossRef]
- Luo, Q. KKM and Nash equilibria type theorems in topological ordered spaces. J. Math. Anal. Appl. 2001, 264, 262–269. [Google Scholar] [CrossRef]
- Luo, Q. Ky Fan’s section theorem and its applications in topological ordered spaces. Appl. Math. Lett. 2004, 17, 1113–1119. [Google Scholar] [CrossRef]
- Kim, H. Applications of results on abstract convex spaces to topological ordered spaces. Bull. Korean Math. Soc. 2013, 50, 305–320. [Google Scholar] [CrossRef]
- Luo, Q. The applications of the Fan–Browder fixed point theorem in topological ordered spaces. Appl. Math. Lett. 2006, 19, 1265–1271. [Google Scholar] [CrossRef]
- Al-Homidan, S.; Ansari, Q.H.; Yao, J.C. Collectively fixed point and maximal element theorems in topological semilattice spaces. Appl. Anal. 2011, 90, 865–888. [Google Scholar] [CrossRef]
- Al-Homidan, S.; Ansari, Q.H. Fixed point theorems on product topological semilattice spaces, generalized abstract economies and systems of generalized vector quasi-equilibrium problems. Taiwan. J. Math. 2011, 15, 307–330. [Google Scholar] [CrossRef]
- Park, S. On the KKM theory of ordered spaces. Linear Nonlinear Anal. 2021, 7, 73–87. [Google Scholar]
- Brouwer, L.E.J. Über abbildung von mannigfaltigkeiten. Math. Ann. 1911, 71, 97–115. [Google Scholar] [CrossRef]
- Kakutani, S. A generalization of Brouwer’s fixed point theorem. Duke Math. J. 1941, 8, 457–459. [Google Scholar] [CrossRef]
- Browder, F.E. The fixed point theory of multi-valued mappings in topological vector spaces. Math. Ann. 1968, 177, 283–301. [Google Scholar] [CrossRef]
- Fan, K. Fixed-point and minimax theorems in locally convex topological linear spaces. Proc. Natl. Acad. Sci. USA 1952, 38, 121–126. [Google Scholar] [CrossRef] [PubMed]
- Glicksberg, I.L. A further generalization of the Kakutani fixed theorem, with application to Nash equilibrium points. Proc. Am. Math. Soc. 1952, 3, 170–174. [Google Scholar] [CrossRef]
- Altwaijry, N.; Ounaies, S.; Chebbi, S. Generalized convexity and applications to fixed points and equilibria. J. Fixed Point Theory Appl. 2018, 20, 1–13. [Google Scholar] [CrossRef]
- Fakhar, M.; Zafarani, J. Matching and fixed point theorems in L-convex spaces. Research Gate 2014, 1–7. [Google Scholar]
- Ding, X.P.; Park, J.Y. New collectively fixed point theorems and applications in G-convex spaces. Appl. Math. Mech. 2002, 23, 1237–1249. [Google Scholar]
- Balaj, M.; Lin, L.J. Fixed points, coincidence points and maximal elements with applications to generalized equilibrium problems and minimax theory. Nonlinear Anal. Theory Methods Appl. 2009, 70, 393–403. [Google Scholar] [CrossRef]
- Tarafdar, E. Fixed point theorems in H-spaces and equilibrium points of abstract economies. J. Aust. Math. Soc. 1993, 53, 252–260. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mo, C.; Yang, Y. The Unified Description of Abstract Convexity Structures. Axioms 2024, 13, 506. https://doi.org/10.3390/axioms13080506
Mo C, Yang Y. The Unified Description of Abstract Convexity Structures. Axioms. 2024; 13(8):506. https://doi.org/10.3390/axioms13080506
Chicago/Turabian StyleMo, Chunrong, and Yanlong Yang. 2024. "The Unified Description of Abstract Convexity Structures" Axioms 13, no. 8: 506. https://doi.org/10.3390/axioms13080506
APA StyleMo, C., & Yang, Y. (2024). The Unified Description of Abstract Convexity Structures. Axioms, 13(8), 506. https://doi.org/10.3390/axioms13080506




