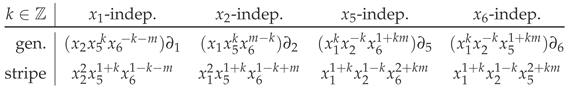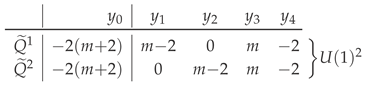Beyond Algebraic Superstring Compactification
Abstract
:1. Introduction, Rationale, and Summary
2. A Showcasing Deformation Family
2.1. From GLSM to Toric
2.1.1. The Superpotential
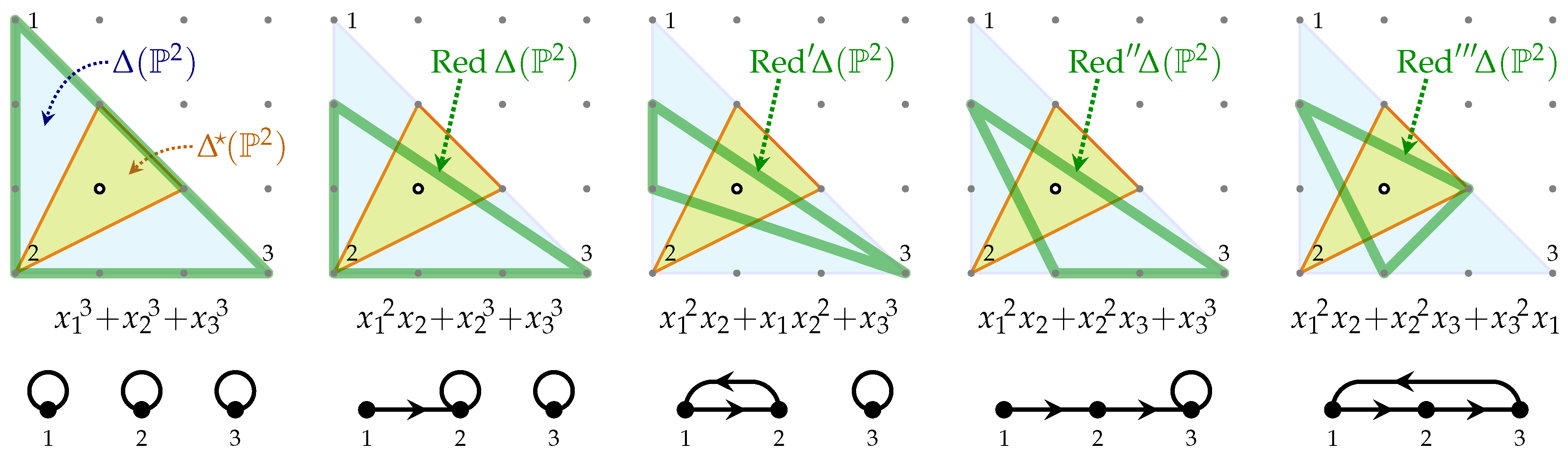
- Monomials independent of a particular variable occur along a straight-line “stripe” (hyperplane in higher dimensions). Therefore, each “stripe” is a suitable multiple of a single -derivative, :Since , each “stripe” acts as a boundary for that -deformation.
- “Cornerstone” monomials at the intersection of two “stripes” are independent of two variables; this hierarchy extends straightforwardly in higher dimensions. The tabulation (8) makes it clear that: (1) There is no - and -independent monomial. (2) There is an - and -independent monomial only for , and , respectively. (3) The “cornerstone” monomials are , , , and , and are circled in the plots in Figure 1.
- The above shows that deforming the fundamental monomial equips the system of anticanonical monomials with the hierarchical structure of a poset:
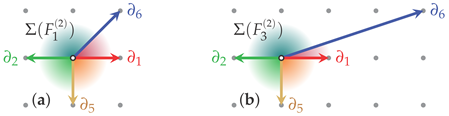
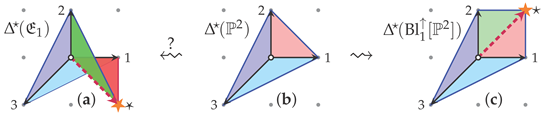
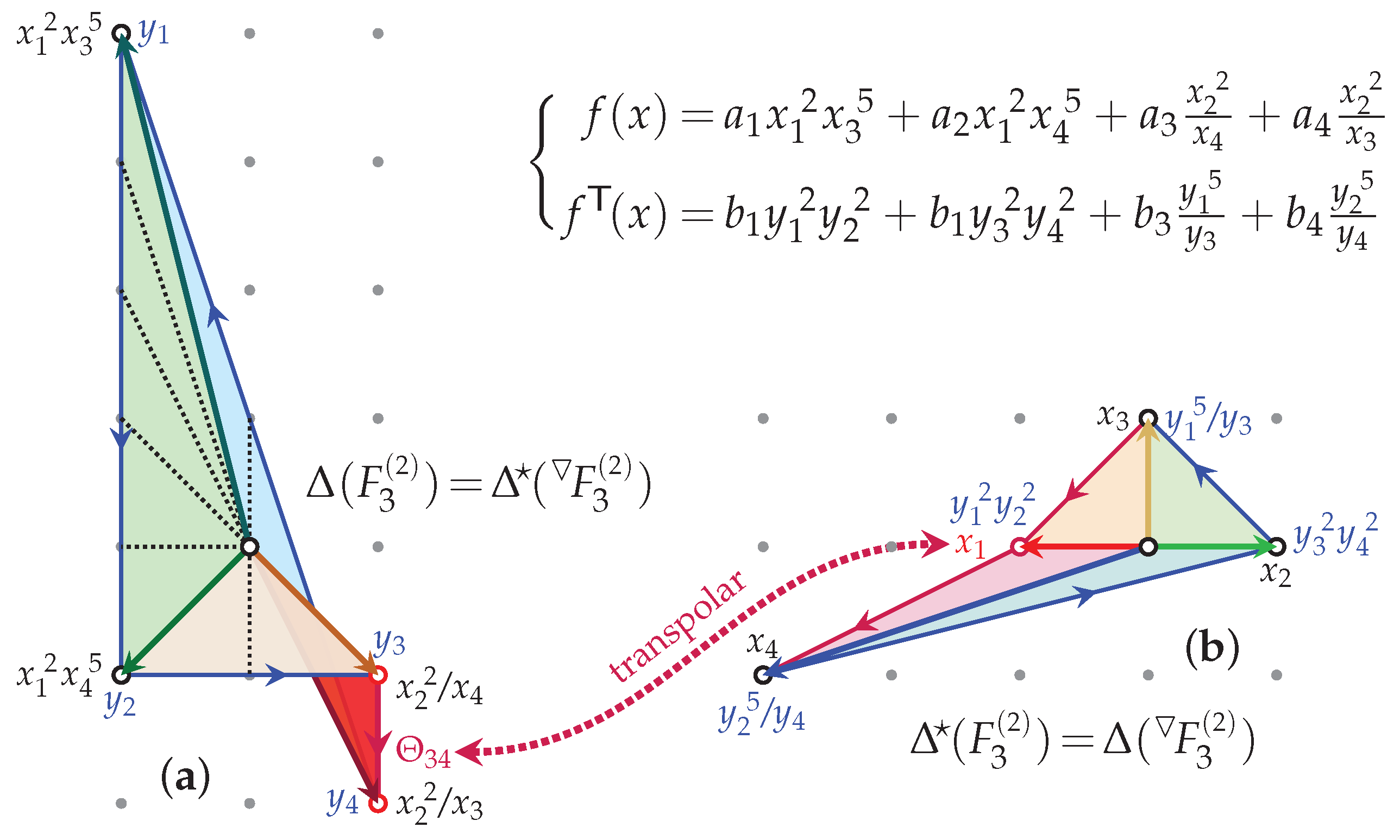
2.1.2. The Transpolar Operation
2.2. From Toric to Generalized Intersections

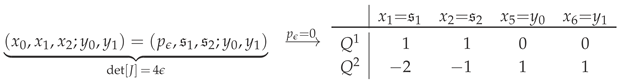
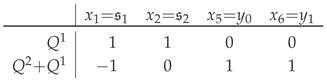
2.3. The Deformation Family Picture
- :
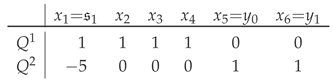
- :

- :
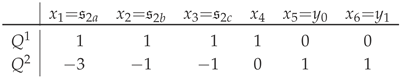
- :

2.4. The Deformation Family Picture, in Depth
2.5. The Deformation Family Picture, Layered
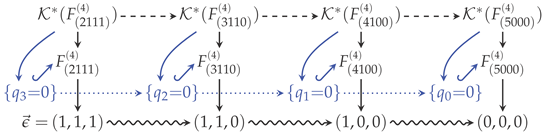
- The sequence of (discrete) deformations among the Hirzebruch scrolls, (middle row in (51), no arrows drawn to avoid clutter), as discussed above.
- The sequence of (likewise discrete) deformations (top row in (51), dashed arrows) among the indicated anticanonical bundles, . This induces corresponding deformations on the anticanonical sections, which provide candidates for the sequence described next.
- The sequence of discrete “leafs” in the double deformations of the defining Equations (lower middle row in (51), dotted arrows) among the indicated Calabi–Yau hypersurfaces: the -dependent anticanonical bundles provide sections for the “second” deformation of anticanonical hypersurfaces, .
- A well-understood embedding (“ambient”) space, X, chosen in (51).
- The anticanonical bundle, , and a collection of its sections, .
- The Calabi–Yau hypersurface—the zero locus, , of a selected anticanonical section.
2.6. Black Sheep in the Deformation Family
3. Transposition Mirrors
3.1. Transposition as Multitope Swapping
- Cox coordinates:
- Chart:
- A toric variety, X, is covered by an atlas of chars, each corresponding to a top-dimensional cone in the fan, . The lattice degree of a cone, , is the dimension-rescaled volume of the 0-apex pyramid generated by the cone’s lattice-primitive generators: .
- MPCP-desingularization:
- Degree-d cones encode -like affine charts (), a maximal projective crepant partial (MPCP)-desingularization (blowup) [92] of which is encoded by a subdivision into degree-1 subcones; each generator cone of the subdivision encodes (part of) an exceptional locus of the MPCP-desingularization.
- Charts and gluing:
- A toric variety, X is covered by an atlas of chars, each corresponding to a top-dimensional cone in the fan, . Charts overlap where their (top-dimensional) cones have a common facet (codimension-1 cone in the boundary), which then specifies how the charts are glued together. The so-defined poset structure in the atlas of charts covering X is in direct 1–1 correspondence to the poset of cones in the fan . A complete fan (where each codimension-1 facet adjoins two top-dimensional cones) corresponds to a compact toric space.
- Multifan layers:
- In multifans (as used in Refs. [60,61,62,63]), a common facet -cone, , adjoins two k-cones, that lie in distinct layers of a multifan that “flip-folds” at , so that contrary to appearances of a larger overlap. The hyperplane region spanned by the lattice-primitive generators of a cone is its (base) face in the spanning multitope of the multifan, which is assembled from the faces of all the cones and with the same poset structure; a precise correspondence with the rich (and varied) practices in the mathematical literature [70,71,72,73,74,75,76,77,78,79,80,81,94] remains to be determined.
3.2. Simplicial Reductions
3.3. Flip-Folded Layers
- The dotted lines in the diagram indicate the MPCP-desingularizations, so is a smooth manifold, but it does not stem from a global finite quotient. Corresponding to the four big (vertex-generated) cones, the four distinct charts (starting from the top-left vertex) correspond to the distinct MPCP desingularizations:
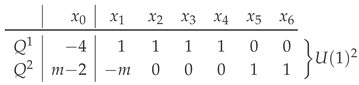
- has a maximal toric -action and a (1)-like GLSM specification:All other linear combinations with integral charges are, for , non-negative linear combinations of and , which are, thereby, the Mori vectors.
- The gluing and are, however, non-standard. In Figure 4a, the corresponding cones and (as well as and ) seem to partially overlap. This is a hallmark of multifans [70,71,72], which in general correspond to torus manifolds, and which are not algebraic varieties unless the multifan is in fact a (flat) fan.
- The multifan defined by the collection of central cones over the facets (“stripes” of anticanonical monomials that are independent of one of the Cox coordinates) is well defined as a poset if the cone is understood to flip-fold, into a (Riemann-sheet like) layer under and over the layer of . This way, and are 1-cones, consistently extending the “Separation Lemma” [48,49].
- This flip-folded character of the multifan centrally spanned by the multilayered multigonal object , which it star-subdivides, is well encoded by the continuous orientation of the closed cycle of cones,This is a key characteristic of the “generalized legal loops” [99], which in fact are the two-dimensional so-called VEX multitopes [60,61,62], the latter defined so that the transpolar operation acts within their class and always as an involution. Thereby, VEX multitopes and their (local) transpolar involution generalize (in a GLSM-motivated fashion) both (1) the (convex) reflexive polytopes [92] and their (standard, global) polar operation to non-convex, flip-folded, and otherwise multilayered VEX multitopes, as well as (2) the “generalized legal loops” and their (local) dual operation of Ref. [99] to all higher dimensions.
- Multifans do not uniquely encode torus manifolds, but it is not known how the continuous orientation of multifans and multitopes (item 5, above), such as depicted in Figure 4, correlates with various combinatorial data considered in the literature to more precisely specify the available choices among (unitary) torus manifolds.
 ” at —which is outside the cone that was being subdivided, and so, might be called a blowout. The 1-point blowup of may also be described as a connected sum , where the orientation of the second copy of has been reversed [100]. The toric diagram in (62, a) may then well correspond to , without which this reversal of orientation on the second copy does not admit a global complex structure (I am indebted to Prof. M. Masuda for suggesting this possibility). This is supported by the computation of the self-intersection of the divisors corresponding to the star-labeled vertices [62]: (1) , as standard for the exceptional divisor in a standard blow-up; (2) [D
” at —which is outside the cone that was being subdivided, and so, might be called a blowout. The 1-point blowup of may also be described as a connected sum , where the orientation of the second copy of has been reversed [100]. The toric diagram in (62, a) may then well correspond to , without which this reversal of orientation on the second copy does not admit a global complex structure (I am indebted to Prof. M. Masuda for suggesting this possibility). This is supported by the computation of the self-intersection of the divisors corresponding to the star-labeled vertices [62]: (1) , as standard for the exceptional divisor in a standard blow-up; (2) [D ]2 = +1, which is opposite from the exceptional divisor in a standard blow-up.
]2 = +1, which is opposite from the exceptional divisor in a standard blow-up.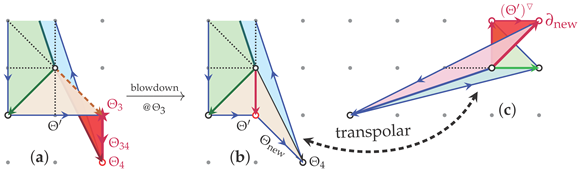
4. Concerns and Conclusions
One More Thing: An Algebraic Alternative
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
References
- Friedan, D.H. Nonlinear Models in 2+ϵ Dimensions. Phys. Rev. Lett. 1980, 45, 1057. [Google Scholar] [CrossRef]
- Friedan, D.H. Nonlinear Models in 2+ϵ Dimensions. Ann. Phys. 1985, 163, 318–419. [Google Scholar] [CrossRef]
- Polchinski, J. String Theory. Vol. 1: An Introduction to the Bosonic String; Cambridge Monographs on Mathematical Physics; Cambridge University Press: Cambridge, UK, 2007. [Google Scholar] [CrossRef]
- Polchinski, J. String Theory. Vol. 2: Superstring Theory and Beyond; Cambridge Monographs on Mathematical Physics; Cambridge University Press: Cambridge, UK, 2007. [Google Scholar] [CrossRef]
- Frenkel, I.B.; Garland, H.; Zuckerman, G.J. Semiinfinite Cohomology and String Theory. Proc. Nat. Acad. Sci. USA 1986, 83, 8442. [Google Scholar] [CrossRef]
- Bowick, M.J.; Rajeev, S.G. String Theory as the Kahler Geometry of Loop Space. Phys. Rev. Lett. 1987, 58, 535. [Google Scholar] [CrossRef]
- Bowick, M.J.; Rajeev, S.G. The Holomorphic Geometry of Closed Bosonic String Theory and Diff S1/S1. Nucl. Phys. 1987, B293, 348. [Google Scholar] [CrossRef]
- Bowick, M.J.; Rajeev, S. The Complex Geometry of String Theory and Loop Space. In Proceedings of the Johns Hopkins Workshop on Current Problems in Particle Theory, Lanzhou, China, 17–19 June 1987; Duan, Y.S., Domókos, G., Kövesi-Domókos, S., Eds.; World Scientific Publishing: Singapore, 1987. [Google Scholar]
- Oh, P.; Ramond, P. Curvature of Superdiff S1/S1. Phys. Lett. 1987, B195, 130–134. [Google Scholar] [CrossRef]
- Harari, D.; Hong, D.K.; Ramond, P.; Rodgers, V.G.J. The Superstring Diff S1/S1 and Holomorphic Geometry. Nucl. Phys. 1987, B294, 556–572. [Google Scholar] [CrossRef]
- Pilch, K.; Warner, N.P. Holomorphic Structure of Superstring Vacua. Class. Quant. Grav. 1987, 4, 1183. [Google Scholar] [CrossRef]
- Bowick, M.J.; Rajeev, S.G. Anomalies and Curvature in Complex Geometry. Nucl. Phys. 1988, B296, 1007–1033. [Google Scholar] [CrossRef]
- Bowick, M.J.; Lahiri, A. The Ricci Curvature of Diff S1/SL(2, R). J. Math. Phys. 1988, 29, 1979–1981. [Google Scholar] [CrossRef]
- Bowick, M.J.; Yang, K.Q. String Equations of Motion from Vanishing Curvature. Int. J. Mod. Phys. 1991, A6, 1319–1334. [Google Scholar] [CrossRef]
- Hübsch, T. Calabi–Yau Manifolds: A Bestiary for Physicists, 2nd ed.; World Scientific Publishing Europe Ltd.: London, UK, 2024. [Google Scholar]
- Candelas, P.; Horowitz, G.T.; Strominger, A.; Witten, E. Vacuum configurations for superstrings. Nucl. Phys. 1985, B258, 46–74. [Google Scholar] [CrossRef]
- Hull, C.M. Sigma Model Beta Functions and String Compactifications. Nucl. Phys. B 1986, 267, 266–276. [Google Scholar] [CrossRef]
- Strominger, A. Superstrings with Torsion. Nucl. Phys. B 1986, 274, 253. [Google Scholar] [CrossRef]
- Hull, C.M. Compactifications of the Heterotic Superstring. Phys. Lett. B 1986, 178, 357–364. [Google Scholar] [CrossRef]
- Becker, K.; Sethi, S. Torsional Heterotic Geometries. Nucl. Phys. B 2009, 820, 1–31. [Google Scholar] [CrossRef]
- Dasgupta, K.; Rajesh, G.; Sethi, S. M theory, orientifolds and G-flux. J. High Energy Phys. 1999, 8, 023. [Google Scholar] [CrossRef]
- Becker, K.; Becker, M.; Green, P.S.; Dasgupta, K.; Sharpe, E. Compactifications of heterotic strings on non-Kähler complex manifolds II. Nucl. Phys. 2004, B678, 19–100. [Google Scholar] [CrossRef]
- Becker, K.; Becker, M.; Dasgupta, K.; Green, P.S. Compactifications of heterotic theory on non-Kahler complex manifolds, I. J. High Energy Phys. 2003, 4, 007. [Google Scholar] [CrossRef]
- Witten, E. Phases of N = 2 theories in two-dimensions. Nucl. Phys. 1993, B403, 159–222. [Google Scholar] [CrossRef]
- Morrison, D.R.; Plesser, M.R. Summing the instantons: Quantum cohomology and mirror symmetry in toric varieties. Nucl. Phys. 1995, B440, 279–354. [Google Scholar] [CrossRef]
- Aspinwall, P.S.; Greene, B.R.; Morrison, D.R. Calabi–Yau Moduli Space, Mirror Manifolds and Space-Time Topology Change in String Theory. Nucl. Phys. 1994, B416, 414–480. [Google Scholar] [CrossRef]
- Hübsch, T.; Rahman, A. On the geometry and homology of certain simple stratified varieties. J. Geom. Phys. 2005, 53, 31–48. [Google Scholar] [CrossRef]
- Banagl, M. Intersection Spaces, Spatial Homology Truncation, and String Theory; Number 1997 in Lecture Notes in Mathematics; Springer: Berlin/Heidelberg, Germany, 2010. [Google Scholar]
- McNamara, J.; Vafa, C. Cobordism Classes and the Swampland. arXiv 2019, arXiv:1909.10355. [Google Scholar] [CrossRef]
- Berglund, P.; Hübsch, T.; Minic, D. On de Sitter Spacetime and String Theory. Int. J. Mod. Phys. D 2023, 32, 2330002. [Google Scholar] [CrossRef]
- Kasner, E. Finite Representation of the Solar Gravitational Field in Flat Space of Six Dimensions. Am. J. Math. 1921, 43, 130–133. [Google Scholar] [CrossRef]
- Fronsdal, C. Completion and Embedding of the Schwarzschild Solution. Phys. Rev. 1959, 116, 778–781. [Google Scholar] [CrossRef]
- Distler, J.; Kachru, S. (0, 2) Landau–Ginzburg theory. Nucl. Phys. B 1994, 413, 213–243. [Google Scholar] [CrossRef]
- Sharpe, E. A survey of recent developments in GLSMs. Int. J. Mod. Phys. A 2024, 39, 2446001. [Google Scholar] [CrossRef]
- Hübsch, T. Calabi–Yau Manifolds—Motivations and Constructions. Commun. Math. Phys. 1987, 108, 291–318. [Google Scholar] [CrossRef]
- Green, P.S.; Hübsch, T. Calabi–Yau Manifolds as Complete Intersections in Products of Projective Spaces. Comm. Math. Phys. 1987, 109, 99–108. [Google Scholar] [CrossRef]
- Candelas, P.; Dale, A.M.; Lütken, C.A.; Schimmrigk, R. Complete intersection Calabi–Yau manifolds. Nucl. Phys. 1988, B298, 493. [Google Scholar] [CrossRef]
- Candelas, P.; Lynker, M.; Schimmrigk, R. Calabi–Yau Manifolds in Weighted P(4). Nucl. Phys. 1990, B341, 383–402. [Google Scholar] [CrossRef]
- Kreuzer, M.; Skarke, H. On the classification of reflexive polyhedra. Commun. Math. Phys. 1997, 185, 495–508. [Google Scholar] [CrossRef]
- Skarke, H. Weight Systems for Toric Calabi–Yau Varieties and Reflexivity of Newton Polyhedra. Mod. Phys. Lett. A 1996, 11, 1637–1652. [Google Scholar] [CrossRef]
- Kreuzer, M.; Skarke, H. Classification of Reflexive Polyhedra in Three Dimensions. Adv. Theor. Math. Phys. 1998, 2, 853–871. [Google Scholar] [CrossRef]
- Kreuzer, M.; Skarke, H. Complete classification of reflexive polyhedra in four-dimensions. Adv. Theor. Math. Phys. 2000, 4, 1209–1230. [Google Scholar] [CrossRef]
- Kreuzer, M.; Skarke, H. Calabi–Yau Data. Available online: http://hep.itp.tuwien.ac.at/~kreuzer/CY/ (accessed on 25 January 2025).
- Constantin, A.; He, Y.H.; Lukas, A. Counting String Theory Standard Models. Phys. Lett. B 2019, 792, 258–262. [Google Scholar] [CrossRef]
- Griffiths, P.; Harris, J. Principles of Algebraic Geometry; Wiley Classics Library; John Wiley & Sons Inc.: New York, NY, USA, 1978. [Google Scholar]
- Danilov, V.I. The Geometry of Toric Varieties. Russ. Math. Surv. 1978, 33, 97–154. [Google Scholar] [CrossRef]
- Fulton, W. Introduction to Toric Varieties; Annals of Mathematics Studies; Princeton University Press: Princeton, NJ, USA, 1993. [Google Scholar]
- Ewald, G. Combinatorial Convexity and Algebraic Geometry; Springer: Berlin/Heidelberg, Germany, 1996. [Google Scholar]
- Cox, D.A.; Little, J.B.; Schenck, H.K. Toric Varieties; Graduate Studies in Mathematics; American Mathematical Society: Providence, RI, USA, 2011; Volume 124, Available online: https://www.mimuw.edu.pl/~jarekw/pragmatic2010/CoxLittleSchenckJan2010.pdf (accessed on 18 March 2025).
- Brooks, R.; Muhammad, F.; Gates, S.J., Jr. Unidexterous D = 2 Supersymmetry in Superspace. Nucl. Phys. 1986, B268, 599–620. [Google Scholar]
- Brooks, R.; Gates, S.J., Jr. Unidexterous D = 2 Supersymmetry in Superspace. 2. Quantization. Phys. Lett. 1987, B184, 217. [Google Scholar]
- Gates, S.J., Jr.; Brooks, R.; Muhammad, F. Unidexterous Superspace: The Flax of (Super)Strings. Phys. Lett. 1987, B194, 35. [Google Scholar]
- Gates, S.J., Jr.; Hübsch, T. Calabi–Yau Heterotic Strings and Unidexterous Sigma Models. Nucl. Phys. 1990, B343, 741–774. [Google Scholar] [CrossRef]
- Kusuki, Y. Modern Approach to 2D Conformal Field Theory. arXiv 2024, arXiv:2412.18307. [Google Scholar] [CrossRef]
- Berglund, P.; Hübsch, T. A generalized construction of mirror manifolds. Nucl. Phys. 1993, B393, 377–391. [Google Scholar] [CrossRef]
- Berglund, P.; Henningson, M. Landau–Ginzburg orbifolds, mirror symmetry and the elliptic genus. Nucl. Phys. 1995, B433, 311–332. [Google Scholar] [CrossRef]
- Krawitz, M.; Priddis, N.; Acosta, P.; Bergin, N.; Rathnakumara, H. FJRW-Rings and Mirror Symmetry. Comm. Math. Phys. 2009, 296, 145–174. [Google Scholar] [CrossRef]
- Borisov, L.A. Berglund-Hübsch mirror symmetry via vertex algebras. Comm. Math. Phys. 2013, 320, 73–99. [Google Scholar] [CrossRef]
- Berglund, P.; Hübsch, T. On Calabi–Yau generalized complete intersections from Hirzebruch varieties and novel K3-fibrations. Adv. Theor. Math. Phys. 2018, 22, 261–303. [Google Scholar] [CrossRef]
- Berglund, P.; Hübsch, T. A Generalized Construction of Calabi–Yau Models and Mirror Symmetry. SciPost 2018, 4, 9. [Google Scholar] [CrossRef]
- Berglund, P.; Hübsch, T. Hirzebruch Surfaces, Tyurin Degenerations and Toric Mirrors: Bridging Generalized Calabi–Yau Constructions. Adv. Theor. Math. Phys. 2022, 26, 2541–2598. [Google Scholar] [CrossRef]
- Berglund, P.; Hübsch, T. Chern Characteristics and Todd–Hirzebruch Identities for Transpolar Pairs of Toric Spaces. arXiv 2024, arXiv:2403.07139. [Google Scholar] [CrossRef]
- Hübsch, T. Ricci–Flat Mirror Hypersurfaces in Spaces of General Type. In Proceedings of the 11th Mathematical Physics Meeting, Belgrade, Serbia, 2–6 September 2024. [Google Scholar]
- Hirzebruch, F. Über eine Klasse von einfach-zusammenhängenden komplexen Mannigfaltigkeiten. Math. Ann. 1951, 124, 77–86. [Google Scholar]
- Gates, S.J., Jr.; Hübsch, T. Unidexterous Locally Supersymmetric Actions For Calabi–Yau Compactifications. Phys. Lett. 1989, B226, 100. [Google Scholar] [CrossRef]
- Hübsch, T. Chameleonic Sigma-Models. Phys. Lett. 1990, B247, 317–322. [Google Scholar] [CrossRef]
- Hübsch, T. How Singular a Space can Superstrings Thread? Mod. Phys. Lett. 1991, A6, 207–216. [Google Scholar] [CrossRef]
- Hübsch, T.; Yau, S.T. An SL(2,C) action on chiral rings and the mirror map. Mod. Phys. Lett. 1992, A7, 3277–3289. [Google Scholar] [CrossRef]
- Berglund, P.; Candelas, P.; de la Ossa, X.; Font, A.; Hübsch, T.; Jancic, D.; Quevedo, F. Periods for Calabi–Yau and Landau–Ginzburg Vacua. Nucl. Phys. 1994, B419, 352–403. [Google Scholar] [CrossRef]
- Masuda, M. Unitary toric manifolds, multi-fans and equivariant index. Tohoku Math. J. (2) 1999, 51, 237–265. [Google Scholar] [CrossRef]
- Masuda, M. From Convex Polytopes to Multi-Polytopes. Notes Res. Inst. Math. Anal. 2000, 1175, 1–15. [Google Scholar]
- Hattori, A.; Masuda, M. Theory of Multi-Fans. Osaka J. Math. 2003, 40, 1–68. [Google Scholar]
- Masuda, M.; Panov, T. On the cohomology of torus manifolds. Osaka J. Math. 2006, 43, 711–746. [Google Scholar]
- Hattori, A.; Masuda, M. Elliptic genera, torus manifolds and multi-fans. Int. J. Math. 2006, 16, 957–998. [Google Scholar] [CrossRef]
- Hattori, A. Elliptic genera, torus orbifolds and multi-fans; II. Int. J. Math. 2006, 17, 707–735. [Google Scholar] [CrossRef]
- Nishimura, Y. Multipolytopes and Convex Chains. Proc. Steklov Inst. Math. 2006, 252, 212–224. [Google Scholar] [CrossRef]
- Ishida, H. Invariant Stably Complex Structures on Topological Toric Manifolds. Osaka J. Math. 2013, 50, 795–806. [Google Scholar]
- Davis, M.W.; Januszkiewicz, T. Convex Polytopes, Coxeter Orbifolds and Torus Actions. Duke Math. J. 1991, 62, 417–451. [Google Scholar] [CrossRef]
- Ishida, H.; Fukukawa, Y.; Masuda, M. Topological toric manifolds. Mosc. Math. J. 2013, 13, 57–98. [Google Scholar] [CrossRef]
- Buchstaber, V.; Panov, T. Toric Topology; Number 204 in Mathematical Surveys and Monographs; American Mathematical Society: Providence, RI, USA, 2015. [Google Scholar]
- Jang, D. Four dimensional almost complex torus manifolds. arXiv 2023, arXiv:2310.11024. [Google Scholar] [CrossRef]
- Berglund, P.; Hübsch, T. On a Residue Representation of Deformation, Koszul and Chiral Rings. Int. J. Mod. Phys. A 1995, 10, 3381–3430. [Google Scholar] [CrossRef]
- Hori, K.; Knapp, J. A pair of Calabi–Yau manifolds from a two parameter non-Abelian gauged linear sigma model. arXiv 2016, arXiv:1612.06214. [Google Scholar] [CrossRef]
- Ruan, Y. Nonabelian gauged linear sigma model. Chin. Ann. Math. Ser. B 2017, 38, 963–984. [Google Scholar] [CrossRef]
- Anderson, L.B.; Apruzzi, F.; Gao, X.; Gray, J.; Lee, S.J. A New Construction of Calabi–Yau Manifolds: Generalized CICYs. Nucl. Phys. 2016, B906, 441–496. [Google Scholar] [CrossRef]
- Garbagnati, A.; van Geemen, B. A remark on generalized complete intersections. Nucl. Phys. 2017, B925, 135–143. [Google Scholar] [CrossRef]
- Kodaira, K.; Spencer, D.C. On Deformations of Complex Analytic Structures; Princeton University: Princeton, NJ, USA, 1957; pp. 328–401. [Google Scholar]
- Wall, C.T.C. Classifications Problems in Differential Topology V: On Certain 6-Manifolds. Invent. Math. 1966, 1, 355–374. [Google Scholar] [CrossRef]
- Tian, G.; Yau, S.T. Complete Kähler manifolds with zero Ricci curvature. I. J. Am. Math. Soc. 1990, 3, 579–609. [Google Scholar] [CrossRef]
- Tian, G.; Yau, S.T. Complete Kähler manifolds with zero Ricci curvature. II. Invent. Math. 1991, 106, 27–60. [Google Scholar] [CrossRef]
- Greene, B.R.; Plesser, M.R. Duality in Calabi–Yau Moduli Space. Nucl. Phys. 1990, B338, 15–37. [Google Scholar] [CrossRef]
- Batyrev, V.V. Dual polyhedra and mirror symmetry for Calabi–Yau hypersurfaces in toric varieties. J. Algebr. Geom. 1994, 3, 493–535. [Google Scholar]
- Cox, D.A. The Homogeneous Coordinate Ring of a Toric Variety. J. Algebr. Geom. 1995, 4, 17–50, Erratum in J. Algebr. Geom. 2014, 23, 393–398. [Google Scholar]
- Karshon, Y.; Tolman, S. The moment map and line bundles over presymplectic toric manifolds. J. Diff. Geom. 1993, 38, 465–484. [Google Scholar] [CrossRef]
- Arnold, V.; Gusein-Zade, S.; Varchenko, A. Singularities of Differentiable Maps; Birkhäuser: Boston, MA, USA, 1985; Volume 1. [Google Scholar]
- Kontsevich, M. Homological Algebra of Mirror Symmetry. In Proceedings of the International Congress of Mathematicians; Chatterji, S.D., Ed.; Birkhäuser Basel: Basel, Switzerland, 1995; pp. 120–139. [Google Scholar]
- Kontsevich, M. Mirror symmetry in dimension 3. Astérisque 1996, 237, 275–293. [Google Scholar]
- Aspinwall, P.S.; Bridgeland, T.; Craw, A.; Douglas, M.R.; Kapustin, A.; Moore, G.W.; Gross, M.; Segal, G.; Szendroi, B.; Wilson, P.M.H. Dirichlet Branes and Mirror Symmetry; Clay Mathematics Monographs; AMS: Providence, RI, USA, 2009; Volume 4. [Google Scholar]
- Poonen, B.; Rodriguez-Villegas, F. Lattice Polygons and the Number 12. Am. Math. Mon. 2000, 107, 238–250. [Google Scholar] [CrossRef]
- Huybrechts, D. Complex Geometry; Springer: Berlin/Heidelberg, Germany, 2005. [Google Scholar] [CrossRef]
- Rahman, A. A Perverse Sheaf Approach Toward a Cohomology Theory for String Theory. Adv. Theor. Math. Phys. 2009, 13, 667–693. [Google Scholar] [CrossRef]
- Collins, T.C.; Gukov, S.; Picard, S.; Yau, S.T. Special Lagrangian cycles and Calabi–Yau transitions. Comm. Math. Phys. 2023, 401, 769–802. [Google Scholar] [CrossRef]
- Anderson, L.B.; Brodie, C.R.; Gray, J. Branes and Bundles through Conifold Transitions and Dualities in Heterotic String Theory. Phys. Rev. D 2023, 108, 106019. [Google Scholar]
- Friedman, B.; Picard, S.; Suan, C. Gromov–Hausdorff continuity of non-Kähler Calabi–Yau conifold transitions. arXiv 2024, arXiv:2404.11840. [Google Scholar] [CrossRef]
- Picard, S. Calabi–Yau threefolds across quadratic singularities. arXiv 2025, arXiv:2501.19313. [Google Scholar] [CrossRef]
- Hibi, T. Star-Shaped Complexes and Ehrhart Polynomials. Proc. Am. Math. Soc. 1995, 123, 723–726. [Google Scholar] [CrossRef]
- Kodaira, K. Complex Manifolds and Deformations of Complex Structures; Springer: New York, NY, USA, 1986; pp. 43–76. [Google Scholar] [CrossRef]
- Reid, M.A. The moduli space of 3-folds with K = 0 may nevertheless be irreducible. Math. Ann. 1987, 278, 329–334. [Google Scholar] [CrossRef]
- Friedman, R. On threefolds with trivial canonical bundle. In Complex Geometry and Lie Theory; Carlson, J.A., Clemens, C.H., Morrison, D.R., Eds.; American Mathematical Society: Providence, RI, USA, 1991; Volume 53, pp. 103–134. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hübsch, T. Beyond Algebraic Superstring Compactification. Axioms 2025, 14, 236. https://doi.org/10.3390/axioms14040236
Hübsch T. Beyond Algebraic Superstring Compactification. Axioms. 2025; 14(4):236. https://doi.org/10.3390/axioms14040236
Chicago/Turabian StyleHübsch, Tristan. 2025. "Beyond Algebraic Superstring Compactification" Axioms 14, no. 4: 236. https://doi.org/10.3390/axioms14040236
APA StyleHübsch, T. (2025). Beyond Algebraic Superstring Compactification. Axioms, 14(4), 236. https://doi.org/10.3390/axioms14040236