Theoretical and Numerical Investigations on Static Characteristics of Aerostatic Porous Journal Bearings
Abstract
:1. Introduction
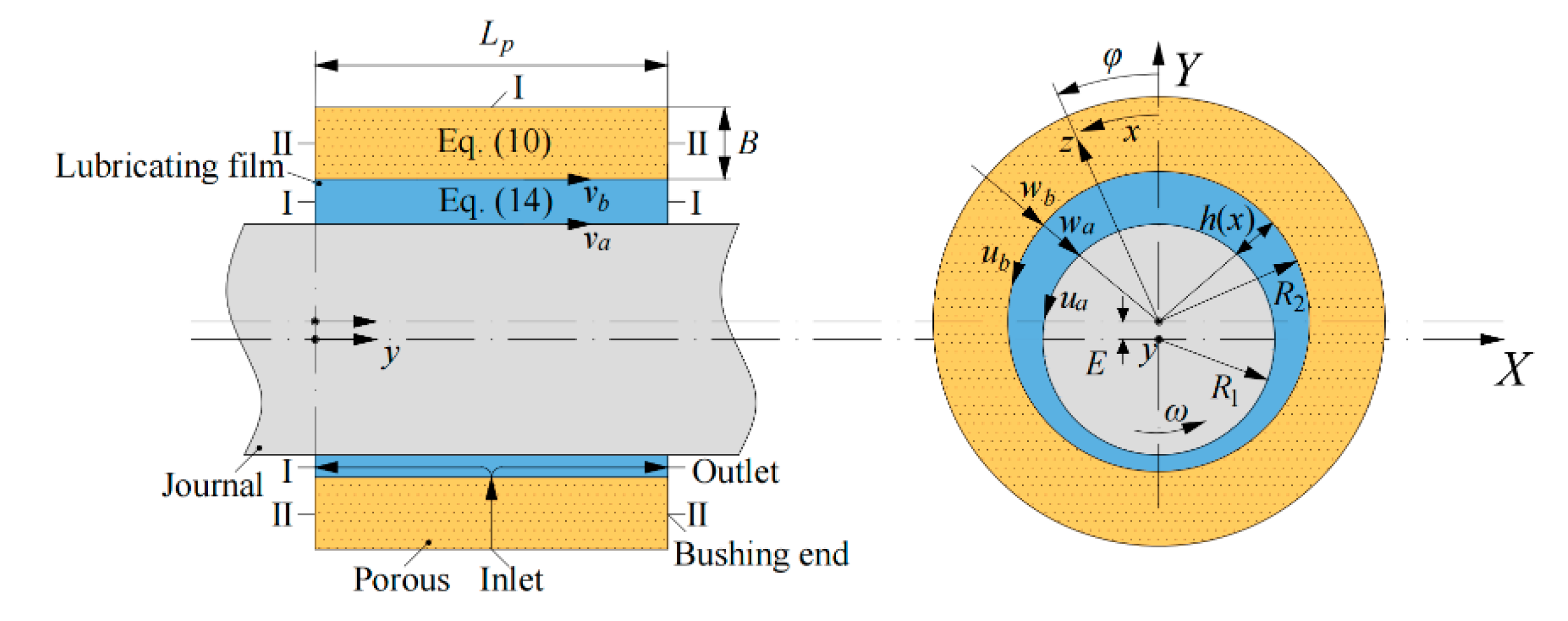
2. Porous Journal Bearings
3. Theoretical Modeling
3.1. Reynolds Lubrication Equation
3.2. Flow Model of Porous Bushing
3.3. Flow Model of Lubricating film
3.4. Boundary Condition
3.5. Ideal Gas State Equation
4. Numerical Solution
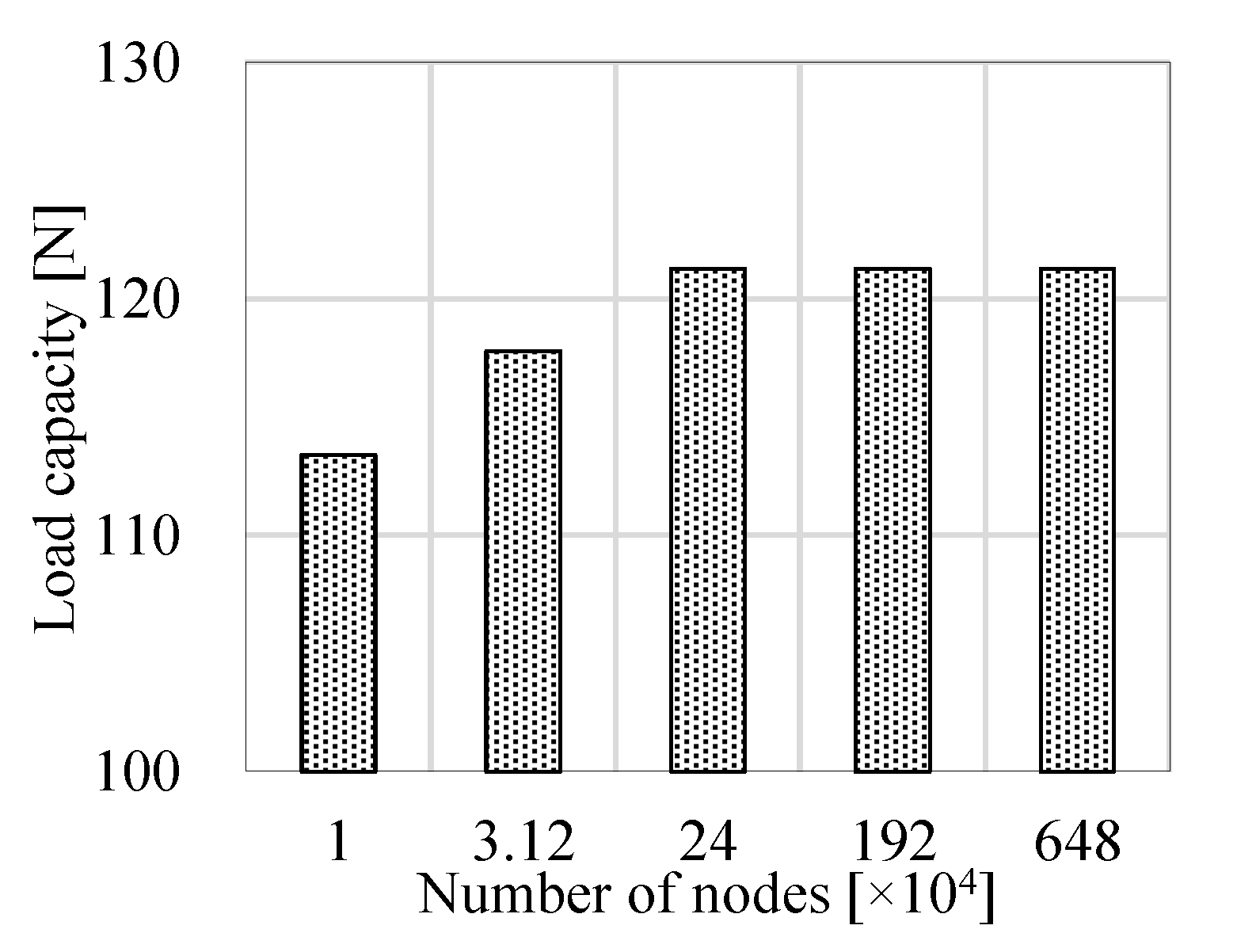
4.1. Grid Generation
4.2. Difference Scheme
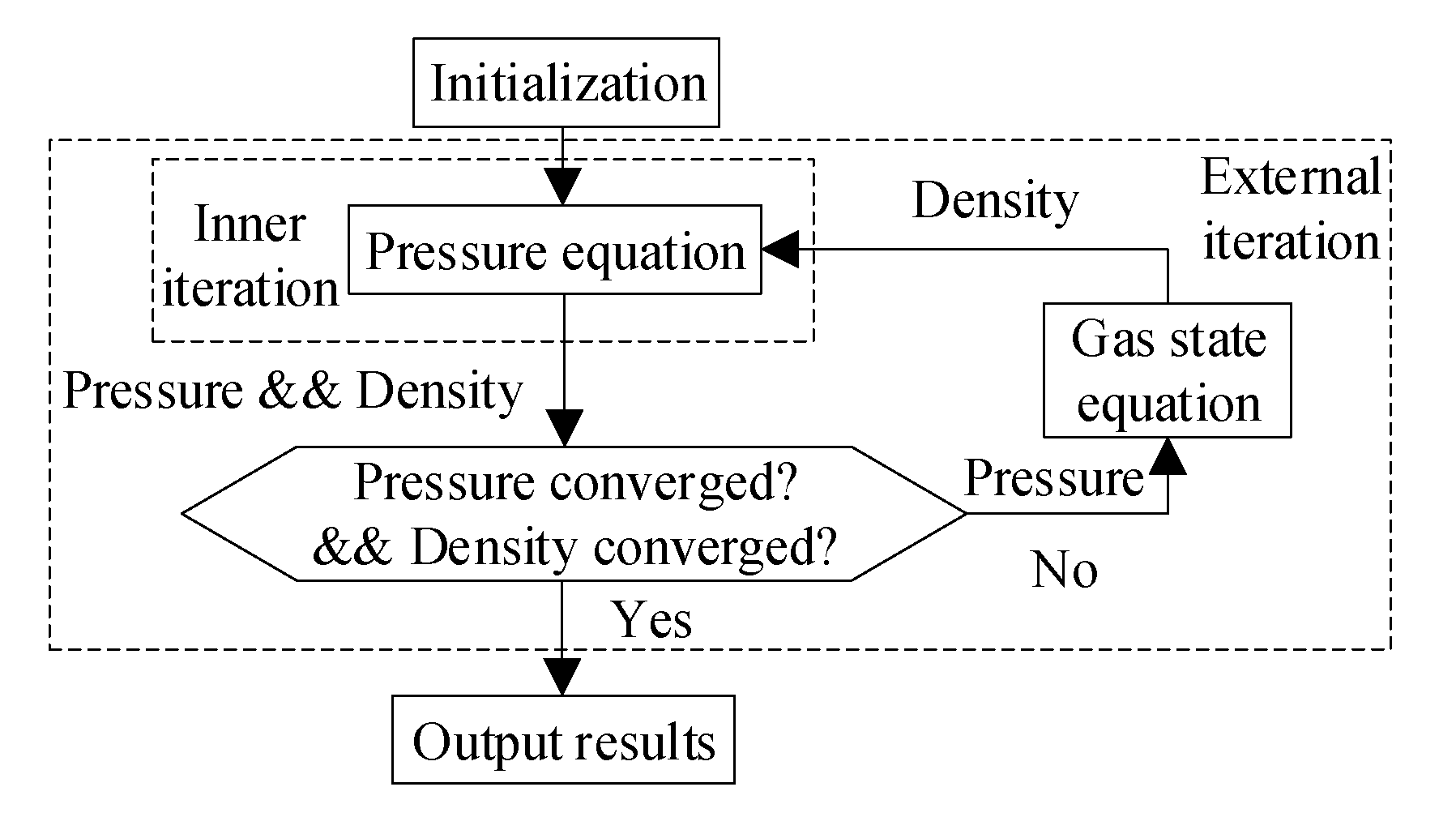
4.3. Pressure–Density Coupling Calculation Method
4.4. Static Characteristics
5. Results and Discussion
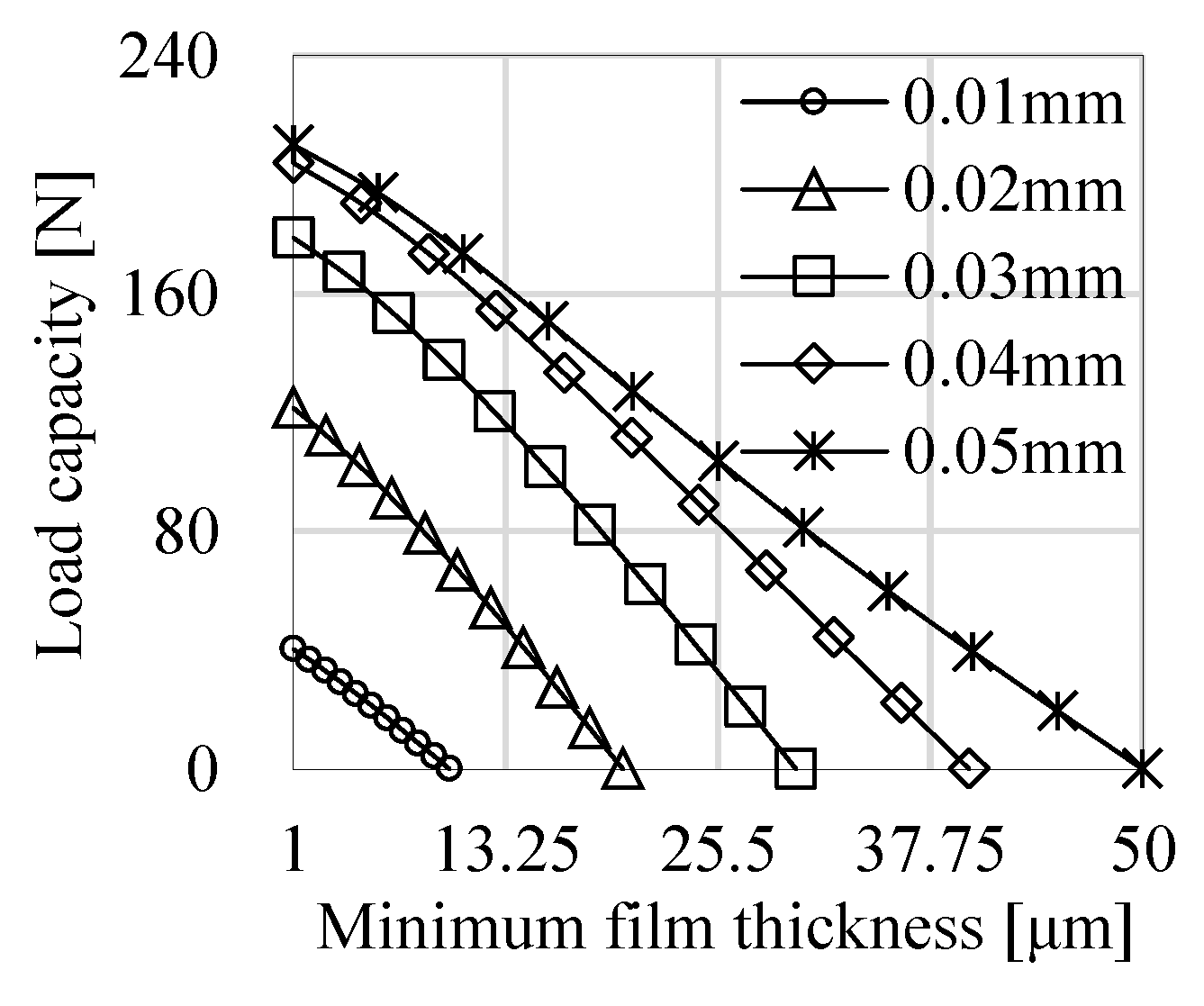
5.1. Effects of Nominal Clearance on Static Characteristics
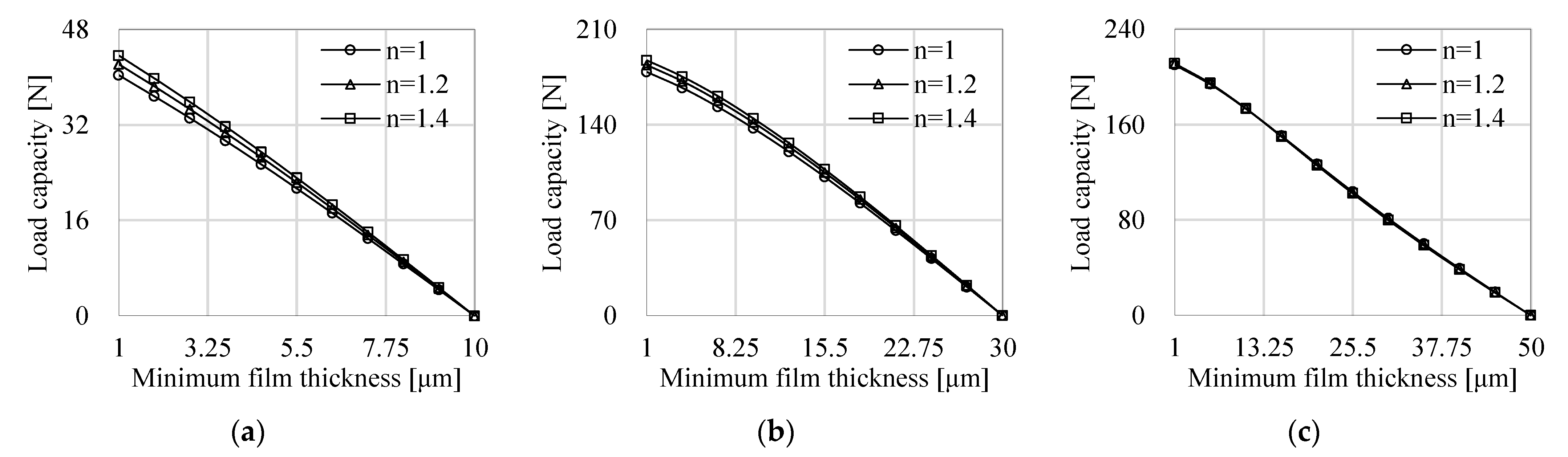
5.2. Effects of Gas Polytropic Index on Static Characteristics
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| B | porous bushing thickness |
| E | eccentricity |
| FX | X-direction load capacity |
| FY | Y-direction load capacity |
| F | load capacity |
| h | radial gap function |
| h0 | nominal clearance |
| i | index for circumferential difference scheme |
| j | index for axial difference scheme |
| k | index for radial difference scheme |
| Lp | porous bushing length |
| ṁ | mass flow rate |
| n | rotating speed |
| Nx | circumferential grid node number |
| Ny | axial grid node number |
| Nz | radial grid node number |
| P | power consumption |
| p | pressure |
| ps | feeding pressure |
| pb | backing pressure |
| pv | virtual node |
| pi,j,k | internal node |
| ∆p | feeding pressure difference (∆p = ps − pb) |
| q | grid growth ratio |
| R1 | journal radius |
| R2 | bearing inside radius |
| Rg | gas constant |
| T | temperature |
| u | circumferential velocity |
| ua | journal circumferential velocity component |
| ub | bushing circumferential velocity component |
| ub,s | bushing circumferential slip velocity component |
| v | axial velocity |
| va | journal axial velocity component |
| vb | bushing axial velocity component |
| vb,s | bushing axial slip velocity component |
| w | radial velocity |
| wa | journal radial velocity component |
| wb | bushing radial velocity component |
| wb,s | bushing radial slip velocity component |
| x | circumferential coordinate |
| y | axial coordinate |
| z | radial coordinate |
| Δzk | grid thickness of layer k |
| Greek symbols | |
| ρ | density |
| μ | lubricant dynamic viscosity |
| υ | lubricant kinematic viscosity |
| α | permeability |
| φ | circumferential angle |
| ω | journal angular velocity |
| Superscripts | |
| n | gas polytropic index |
| Subscripts | |
| a | journal component |
| b | bushing component |
| i | free index |
| j | dummy index |
| s | slip velocity |
| x | circumferential |
| y | axial |
| z | radial |
References
- Gu, Y.; Böhle, M.; Schimpf, A.; Yuan, S. Aerostatic bearing with porous restrictor: Research status and future perspectives. J. Drain. Irrig. Mach. Eng. 2021, 39, 818–825. [Google Scholar] [CrossRef]
- Gu, Y.; Böhle, M.; Schimpf, A.; Yuan, S. Theoretical modeling and numerical solution of hydrostatic radial bearing with porous restrictor. J. Vib. Shock. 2021, 40, 16–24. [Google Scholar] [CrossRef]
- Lee, C.C.; You, H.I. Characteristics of externally pressurized porous gas bearings considering structure permeability. Tribol. Trans. 2009, 52, 768–776. [Google Scholar] [CrossRef]
- San Andres, L.; Cable, T.A.; Zheng, Y.; De Santiago, O.; Devitt, D. Assessment of porous type gas bearings: Measurements of bearing performance and rotor vibrations. In Proceedings of the ASME Turbo Expo 2016: Turbomachinery Technical Conference and Exposition, Seoul, Korea, 13–17 June 2016. [Google Scholar] [CrossRef]
- Liu, W.; Feng, K.; Huo, Y.; Guo, Z. Measurements of the rotordynamic response of a rotor supported on porous type gas bearing. J. Eng. Gas Turbines Power 2018, 140, 102501. [Google Scholar] [CrossRef]
- Wang, B.; Wang, Y.; Liu, Y.; Zhu, C.; Yuan, X. Static and dynamic characteristics of tilting-pad thrust bearing on inclined shaft. J. Xi’an Jiaotong Univ. 2020, 54, 129–138. [Google Scholar] [CrossRef]
- Hu, X.; Lü, P.; Feng, K.; Zhao, X. Static and dynamic performance of laminated gas foil thrust bearing. J. Aerosp. Power 2018, 37, 236–241. [Google Scholar] [CrossRef]
- Sneck, H.J.; Yen, K.T. The externally pressurized, porous wall, gas-lubricated journal bearing. ASLE Trans. 1964, 7, 288–298. [Google Scholar] [CrossRef]
- Majumdar, B.C. Analysis of externally pressurized porous gas journal bearings—I. Wear 1975, 33, 25–35. [Google Scholar] [CrossRef]
- Singh, K.C.; Rao, N.S.; Majumdar, B.C. Effect of slip flow on the steady-state performance of aerostatic porous journal bearings. J. Tribol. 1984, 106, 156–162. [Google Scholar] [CrossRef]
- Prakash, J.; Gururajan, K. Effect of velocity slip in an infinitely long rough porous journal bearing. Tribol. Trans. 1999, 42, 661–667. [Google Scholar] [CrossRef]
- Naduvinamani, N.B.; Hiremath, P.S.; Gurubasavaraj, G. Squeeze film lubrication of a short porous journal bearing with couple stress fluids. Tribol. Int. 2001, 34, 739–747. [Google Scholar] [CrossRef]
- Saha, N.; Majumdar, B.C. Study of externally-pressurized gas-lubricated two-layered porous journal bearings: A steady state analysis. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2002, 216, 151–158. [Google Scholar] [CrossRef]
- Elsharkawy, A.A. Effects of misalignment on the performance of flexible porous journal bearings. Tribol. Trans. 2003, 46, 119–127. [Google Scholar] [CrossRef]
- Lu, Z.; Du, J.; Sun, Y. Analysis on aerostatic porous spherical bearings static performance. Chin. J. Mech. Eng. 2004, 2004, 115–119. [Google Scholar] [CrossRef]
- Ruan, H.; Zhang, D.; Jing, X. Analysis on operation stability of porous aerostatic journal bearing. Bearing 2006, 2006, 1–3. [Google Scholar] [CrossRef]
- Miyatake, M.; Yoshimoto, S.; Sato, J. Whirling instability of a rotor supported by aerostatic porous journal bearings with a surface-restricted layer. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2006, 220, 95–103. [Google Scholar] [CrossRef]
- Nicoletti, R.; Silveira, Z.C.; Purquerio, B.M. Modified Reynolds equation for aerostatic porous radial bearings with quadratic Forchheimer pressure-flow assumption. J. Tribol. 2008, 130, 031701. [Google Scholar] [CrossRef]
- Nicoletti, R.; Purquerio, B.D.M.; Silveira, Z.D.C. The effect of permeability distribution on the numerical analysis of aerostatic ceramic porous bearings. Lubr. Sci. 2013, 25, 185–194. [Google Scholar] [CrossRef]
- Lee, C.C.; You, H.I. Geometrical design considerations on externally pressurized porous gas bearings. Tribol. Trans. 2010, 53, 386–391. [Google Scholar] [CrossRef]
- Nishitani, Y.; Yoshimoto, S.; Somaya, K. Numerical investigation of static and dynamic characteristics of water hydrostatic porous thrust bearings. Int. J. Autom. Technol. 2011, 5, 773–779. [Google Scholar] [CrossRef]
- Chien, S.Y.; Cramer, M.; Untaroiu, A. A compressible thermohydrodynamic analysis of journal bearings lubricated with supercritical CO2. In Proceedings of the ASME 2017 Fluids Engineering Division Summer Meeting, Waikoloa, HI, USA, 30 July–3 August 2017. [Google Scholar] [CrossRef]
- Feng, K.; Wu, Y.; Liu, W.; Zhao, X.; Li, W. Theoretical investigation on porous tilting pad bearings considering tilting pad motion and porous material restriction. Precis. Eng. 2018, 53, 26–37. [Google Scholar] [CrossRef]
- Feng, K.; Li, W.; Huo, Y.; Huo, C. Thermal characteristic analysis of aerostatic porous journal bearings with surface-restricted layer. J. Mech. Eng. 2018, 54, 216–224. [Google Scholar] [CrossRef]
- Böhle, M.; Gu, Y.; Schimpf, A. Two flow models for designing hydrostatic bearings with porous material. In Proceedings of the ASME-JSME-KSME 2019 8th Joint Fluids Engineering Conference, San Francisco, CA, USA, 28 July–1 August 2019. [Google Scholar] [CrossRef]
- Bhattacharjee, B.; Chakraborti, P.; Choudhuri, K. Evaluation of the performance characteristics of double-layered porous micropolar fluid lubricated journal bearing. Tribol. Int. 2019, 138, 415–423. [Google Scholar] [CrossRef]
- Wu, D.; Tao, J. Analysis of static performance of porous graphite aerostatic thrust bearing. China Mech. Eng. 2010, 21, 2296–2301. [Google Scholar]
- Cui, H.; Wang, Y.; Yue, X.; Huang, M.; Wang, W. Effects of manufacturing errors on the static characteristics of aerostatic journal bearings with porous restrictor. Tribol. Int. 2017, 115, 246–260. [Google Scholar] [CrossRef]
- Pei, H.; Long, W.; Yang, S.; Gong, L. Formation mechanism of micro-vibration in aerostatic bearings. J. Vib. Shock. 2018, 37, 71–78. [Google Scholar] [CrossRef]
- Böhle, M. Numerical investigation of the flow in hydrostatic journal bearings with porous material. In Proceedings of the ASME 2018 5th Joint US-European Fluids Engineering Division Summer Meeting, Montreal, Qc, Canada, 15–20 July 2018. [Google Scholar] [CrossRef]
- Hamrock, B.J.; Schmid, S.R.; Jacobson, B.O. Fundamentals of Fluid Film Lubrication, 2nd ed.; Marcel Dekker, Inc.: New York, NY, USA, 2004. [Google Scholar] [CrossRef]
- Sparrow, E.M.; Beavers, G.S.; Hwang, I.T. Effect of velocity slip on porous-walled squeeze films. J. Lubr. Technol. 1972, 94, 260–264. [Google Scholar] [CrossRef]
- Ferziger, J.H.; Perić, M.; Street, R.L. Computational Methods for Fluid Dynamics, 3rd ed.; Springer: Berlin/Heidelberg, Germany, 2002. [Google Scholar] [CrossRef]
- Tao, W. Numerical Heat Transfer, 2nd ed.; Xi’an Jiaotong University Press: Xi’an, China, 2001. (In Chinese) [Google Scholar]





| Parameter | Case A | Case B | Case C | Case D | Case E |
|---|---|---|---|---|---|
| Journal radius R1 (mm) | 15 | 15 | 15 | 15 | 15 |
| Nominal clearance h0 (mm) | 0.01 | 0.02 | 0.03 | 0.04 | 0.05 |
| Bearing inside radius R2 (mm) | 15.01 | 15.02 | 15.03 | 15.04 | 15.05 |
| Porous bushing thickness B (mm) | 5 | ||||
| Porous bushing length Lp (mm) | 38 | ||||
| Dynamic viscosity of lubricant μ (Pa·s) | 1.7894 × 10−5 | ||||
| Permeability α (m2) | 1 × 10−13 | ||||
| Rotating speed n (r·min−1) | 5000 | ||||
| Feeding pressure ps (bar) | 4 | ||||
| Background pressure pb (bar) | 1 | ||||
| Feeding pressure difference ∆p = ps − pb (bar) | 3 | ||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gu, Y.; Cheng, J.; Xie, C.; Li, L.; Zheng, C. Theoretical and Numerical Investigations on Static Characteristics of Aerostatic Porous Journal Bearings. Machines 2022, 10, 171. https://doi.org/10.3390/machines10030171
Gu Y, Cheng J, Xie C, Li L, Zheng C. Theoretical and Numerical Investigations on Static Characteristics of Aerostatic Porous Journal Bearings. Machines. 2022; 10(3):171. https://doi.org/10.3390/machines10030171
Chicago/Turabian StyleGu, Yandong, Jinwu Cheng, Chaojie Xie, Longyu Li, and Changgeng Zheng. 2022. "Theoretical and Numerical Investigations on Static Characteristics of Aerostatic Porous Journal Bearings" Machines 10, no. 3: 171. https://doi.org/10.3390/machines10030171
APA StyleGu, Y., Cheng, J., Xie, C., Li, L., & Zheng, C. (2022). Theoretical and Numerical Investigations on Static Characteristics of Aerostatic Porous Journal Bearings. Machines, 10(3), 171. https://doi.org/10.3390/machines10030171






