Effect of Strain Hardening and Ellipticity on Elastic–Plastic Contact Behaviour between Ellipsoids and Rigid Planes
Abstract
:1. Introduction
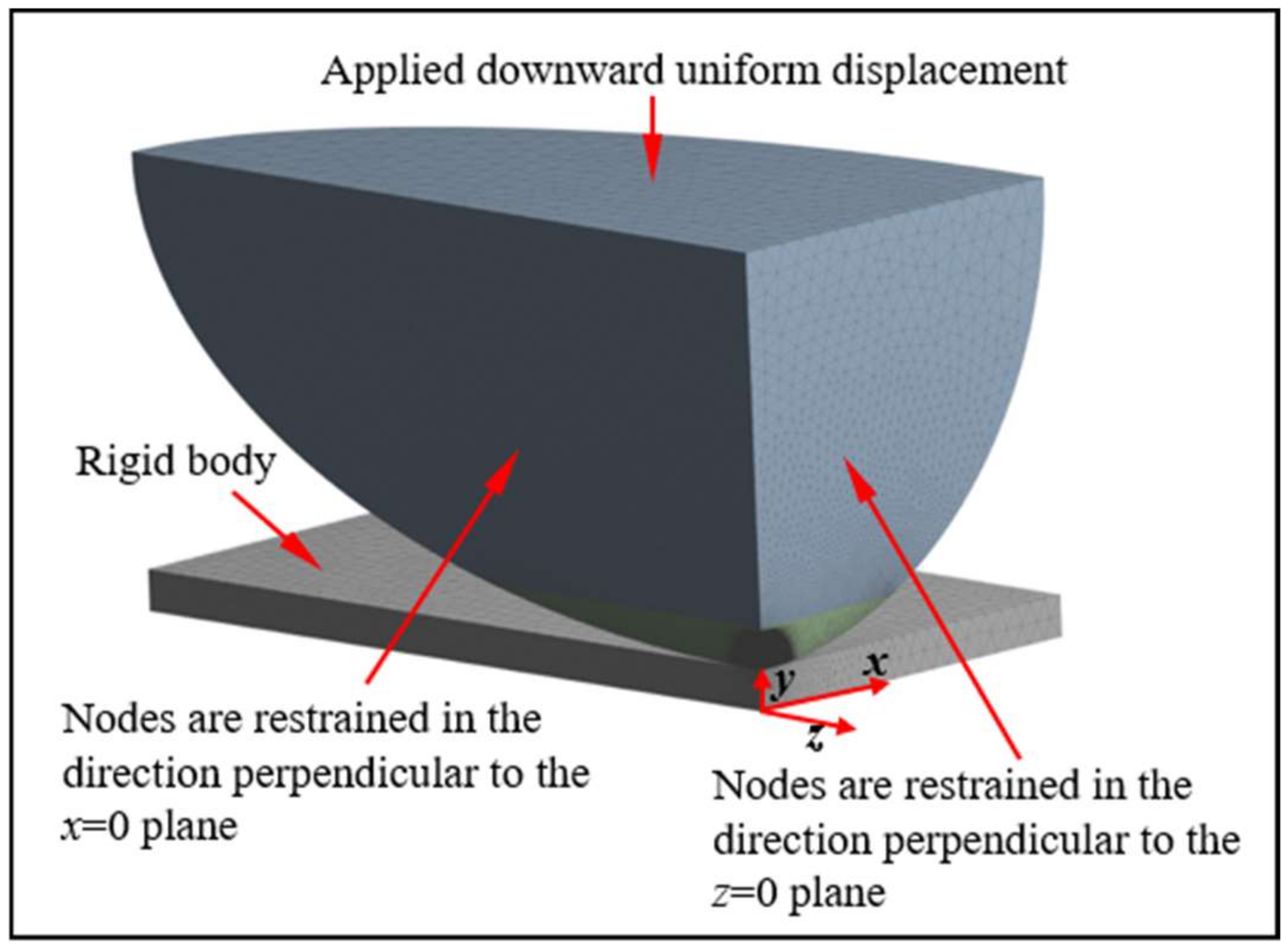
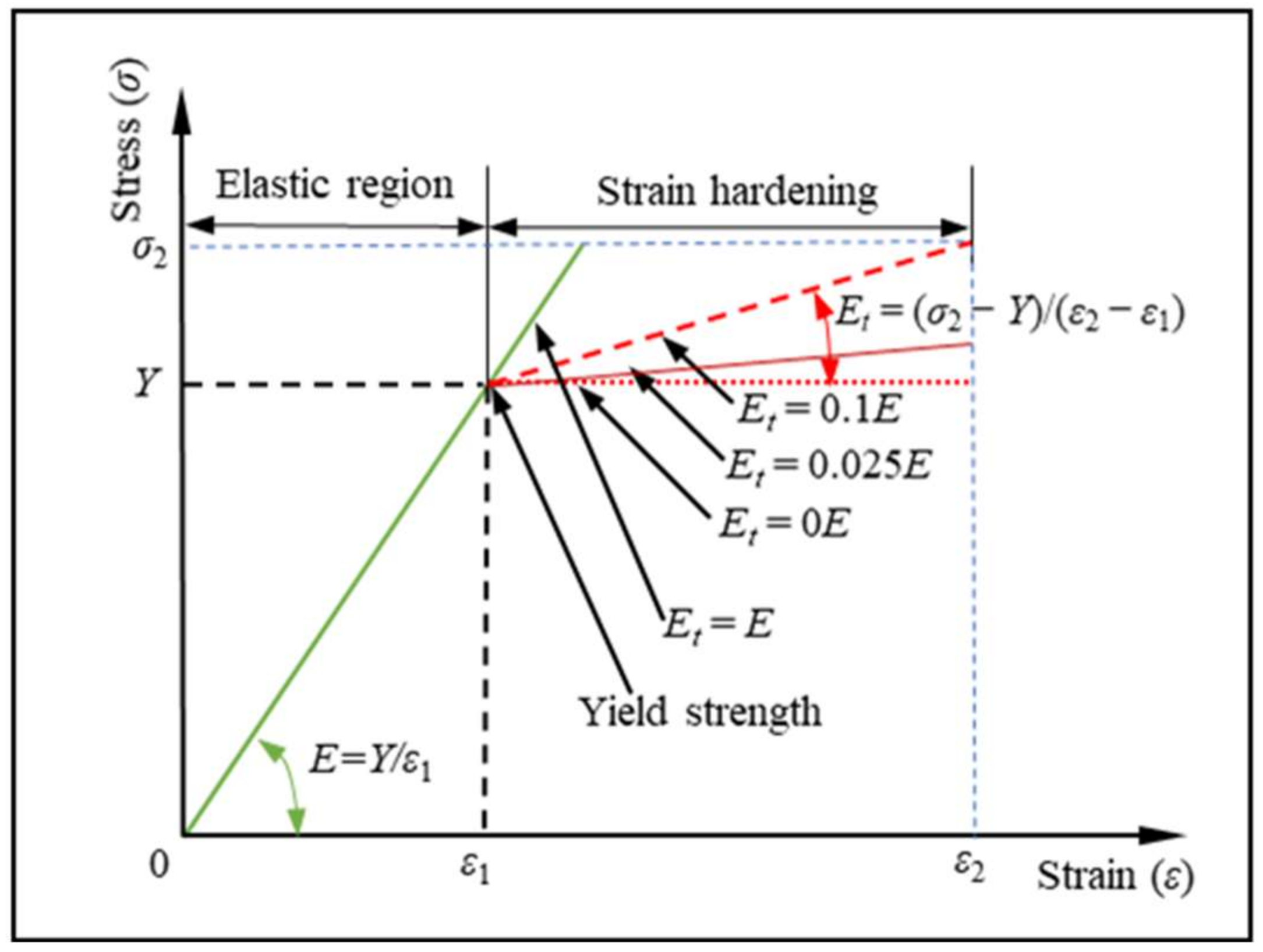
2. Numerical Model
3. Numerical Results and Discussion
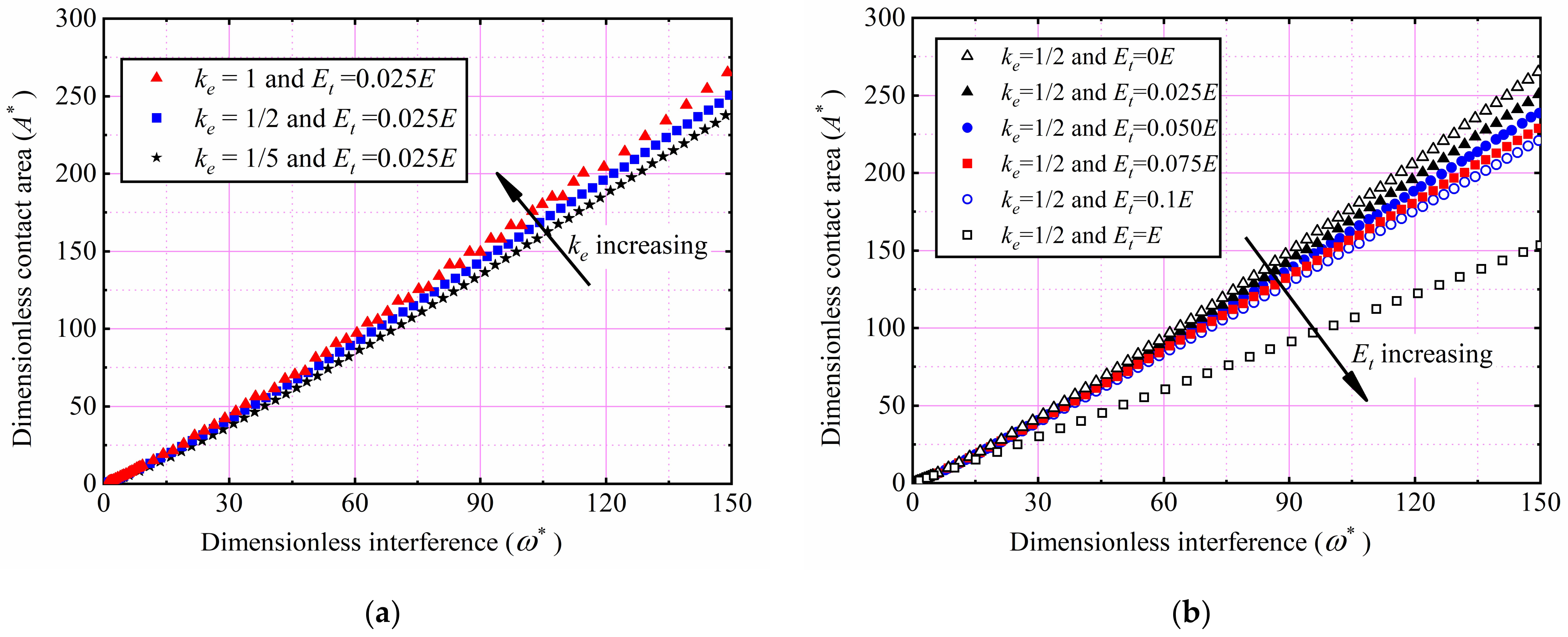
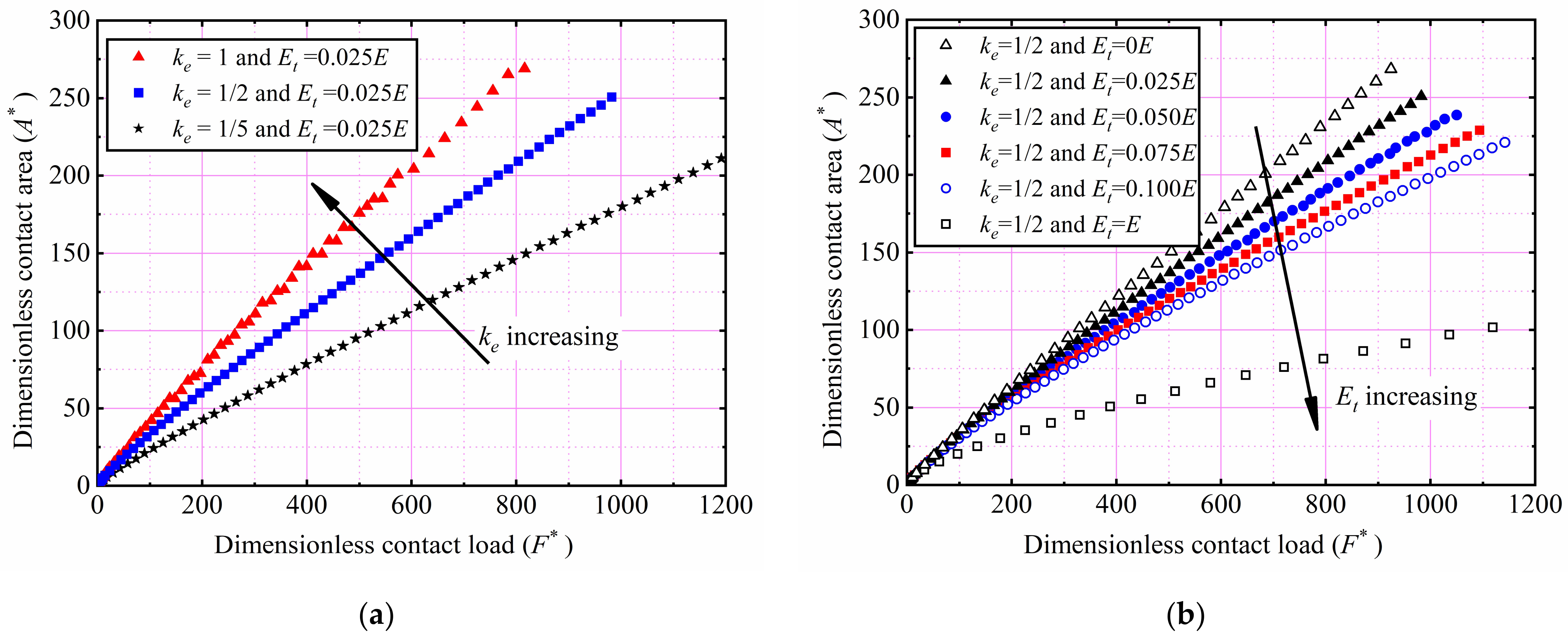
3.1. Contact Parameters
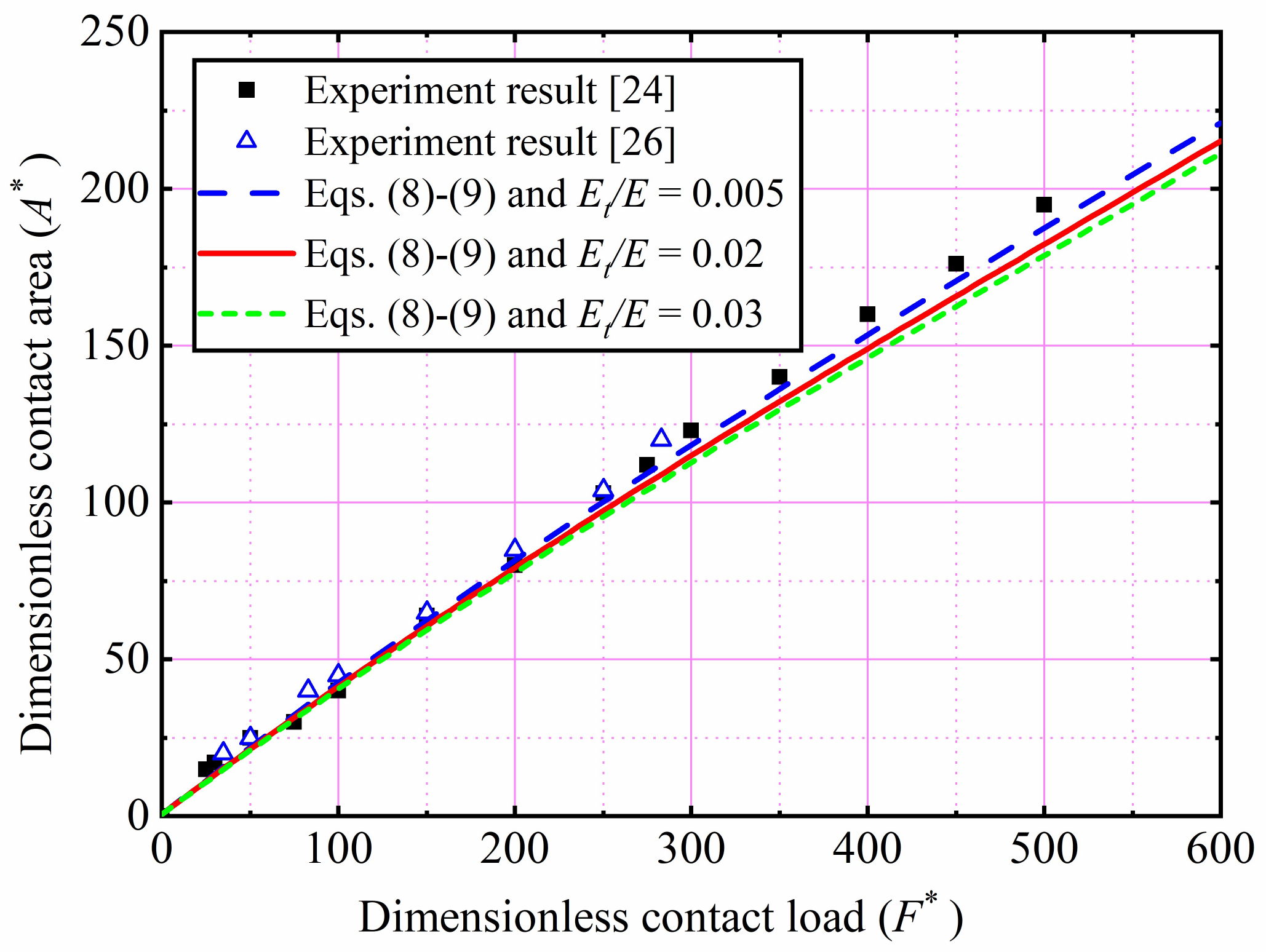
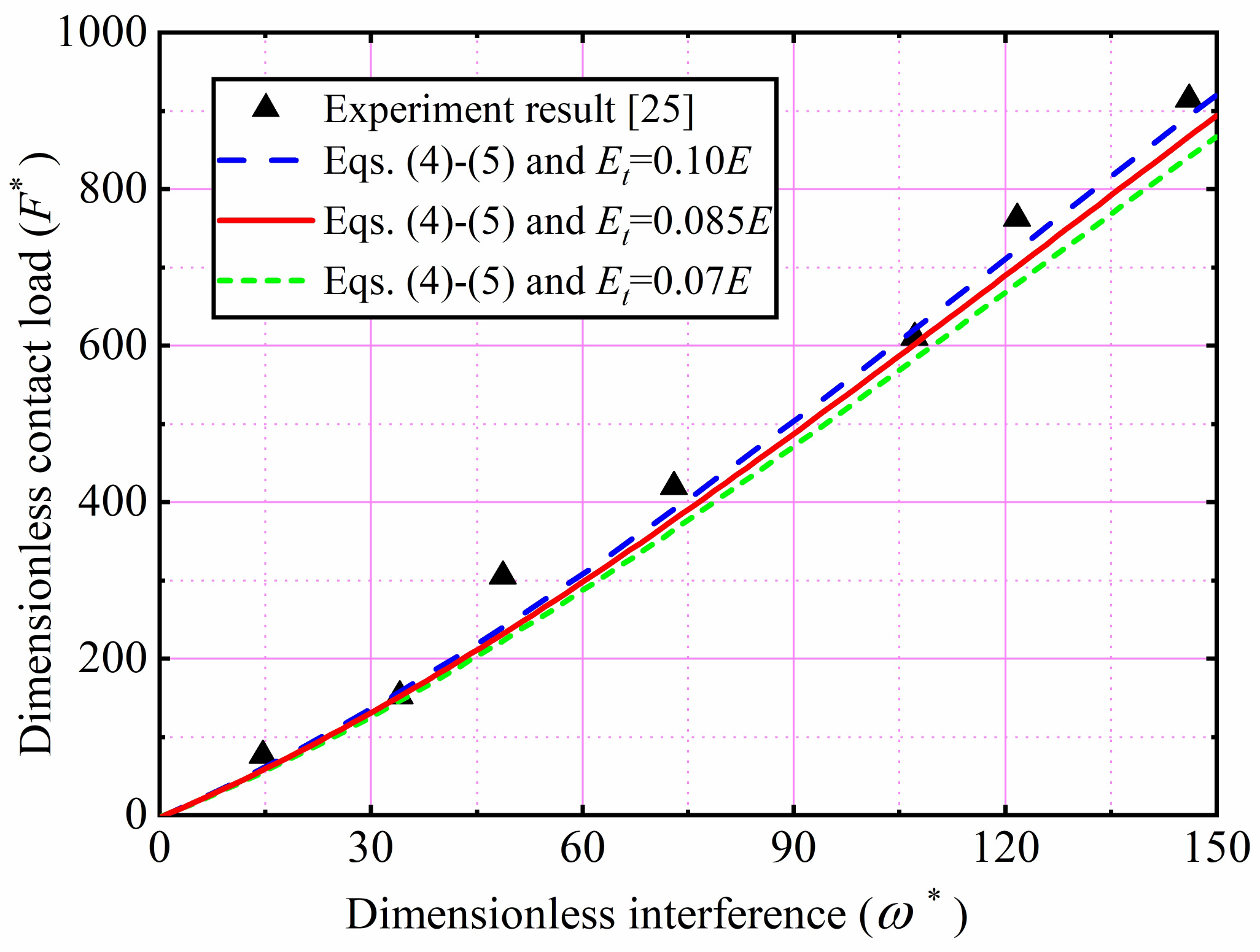
3.2. Comparison with Experimental Results
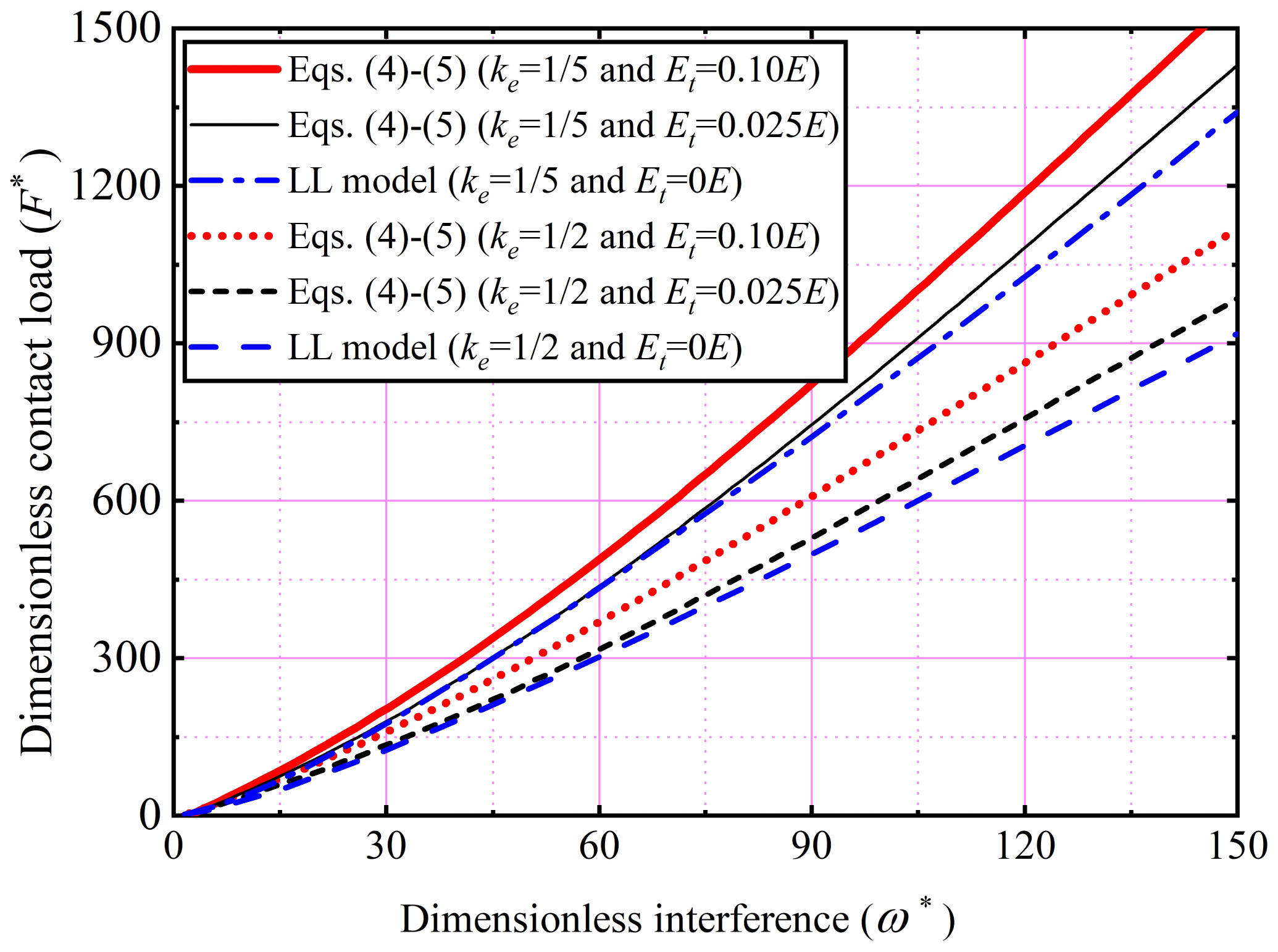
3.3. Comparison with Previous Numerical Models
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Salari, S.; Beheshti, A. Asperity-based contact and static friction with provision for creep: A review. Surf. Interfaces 2021, 24, 101144. [Google Scholar] [CrossRef]
- Flores, P. Contact mechanics for dynamical systems: A comprehensive review. Multibody Syst. Dyn. 2022, 54, 127–177. [Google Scholar] [CrossRef]
- Zhao, G.; Li, S.; Xiong, Z.; Gao, W.; Han, Q. A statistical model of elastic-plastic contact between rough surfaces. Trans. Can. Soc. Mech. Eng. 2019, 43, 38–46. [Google Scholar] [CrossRef]
- Zhang, Y.F.; Fang, B.; Kong, L.F.; Li, Y. Effect of the ring misalignment on the service characteristics of ball bearing and rotor system. Mech. Mach. Theory 2020, 151, 103889. [Google Scholar] [CrossRef]
- Li, G.; Wang, Z.H.; Zhu, W.D. Prediction of surface wear of involute gears based on a modified fractal method. J. Tribol. 2019, 141, 031603. [Google Scholar] [CrossRef]
- Yu, G.; Mao, H.; Jiang, L.; Liu, W.; Valerii, T. Fractal contact mechanics model for the rough surface of a Beveloid gear with elliptical asperities. Appl. Sci. 2022, 12, 4071. [Google Scholar] [CrossRef]
- Chung, J.C.; Lin, J.F. Fractal model developed for elliptic elastic-plastic asperity microcontacts of rough surfaces. J. Tribol. 2004, 126, 646–654. [Google Scholar] [CrossRef]
- Liu, Y.; An, Q.; Shang, D.; Bai, L.; Huang, M.; Huang, S. Research on normal contact stiffness of rough joint surfaces machined by turning and grinding. Metals 2022, 12, 669. [Google Scholar] [CrossRef]
- Zhang, Y.; Lu, H.; Zhang, X.; Ling, H.; Fan, W.; Bao, L.; Guo, Z. A normal contact stiffness model of machined joint surfaces considering elastic, elasto-plastic and plastic factors. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2020, 234, 1007–1016. [Google Scholar] [CrossRef]
- Ghaednia, H.; Jackson, R.L. The effect of nanoparticles on the real area of contact, friction, and wear. J. Tribol. 2013, 135, 041603. [Google Scholar] [CrossRef]
- Zhang, H.; Etsion, I. Evolution of adhesive wear and friction in elastic-plastic spherical contact. Wear 2021, 478–479, 203915. [Google Scholar] [CrossRef]
- Yuan, Y.; Cheng, Y.; Liu, K.; Gan, L. A revised Majumdar and Bushan model of elastoplastic contact between rough surfaces. Appl. Surf. Sci. 2017, 425, 1138–1157. [Google Scholar] [CrossRef]
- Hertz, H. Ueber die berührung fester elastischer körper. J. Reine Angew. Math. 1881, 92, 156–171. [Google Scholar]
- Zhao, J.; Fu, P.; Zhang, X.; Zhou, L.; Wang, P.; Kan, Q. An effective method for calculating elasto-plastic contact pressure and contact patch size under elliptical, circular and line contact conditions. Appl. Math. Model. 2021, 95, 541–574. [Google Scholar] [CrossRef]
- Ghaednia, H.; Wang, X.; Saha, S.; Xu, Y.; Sharma, A.; Jackson, R.L. A review of elastic-plastic contact mechanics. Appl. Mech. Rev. 2017, 69, 2017. [Google Scholar] [CrossRef] [Green Version]
- Li, W.; Zhang, Z.; Zhang, C.; Meng, D.; He, P. The influence of surface topography on friction squeal—A review. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2022, 1175653436. [Google Scholar] [CrossRef]
- Greenwood, J.A.; Williamson, J.B.P. Contact of nominally flat surfaces. Proc. R. Soc. A Math. Phys. Eng. Sci. 1966, 295, 300–319. [Google Scholar]
- Abbott, E.J.; Firestone, F.A. Specifying surface quality-a method based on accurate measurement and comparison. J. Appl. Mech. 1933, 55, 569–572. [Google Scholar]
- Chang, W.R.; Etsion, I.; Bogy, D.B. An elastic-plastic model for the contact of rough surfaces. J. Tribol. 1987, 109, 257–263. [Google Scholar] [CrossRef]
- Zhao, Y.; Maietta, D.M.; Chang, L. An asperity microcontact model incorporating the transition from elastic deformation to fully plastic flow. J. Tribol. 2000, 122, 86–93. [Google Scholar] [CrossRef]
- Wang, H.; Yin, X.; Hao, H.; Chen, W.; Yu, B. The correlation of theoretical contact models for normal elastic-plastic impacts. Int. J. Solids Struct. 2020, 182, 15–33. [Google Scholar] [CrossRef]
- Li, L.; Wang, J.; Shi, X.; Tong, M.; Li, L. A modified elastoplastic contact stiffness model considering continuous smooth contact characteristics and substrate deformation. Acta Mech. Solida Sin. 2021, 34, 754–765. [Google Scholar] [CrossRef]
- Weng, P.; Yin, X.; Hu, W.; Yuan, H.; Chen, C.; Ding, H.; Yu, B.; Xie, W.; Jiang, L.; Wang, H. Piecewise linear deformation characteristics and a contact model for elastic-plastic indentation considering indenter elasticity. Tribol. Int. 2021, 162, 107–114. [Google Scholar] [CrossRef]
- Ovcharenko, A.; Halperin, G.; Verberne, G.; Etsion, I. In situ investigation of the contact area in elastic–plastic spherical contact during loading-unloading. Tribol. Lett. 2007, 25, 153–160. [Google Scholar] [CrossRef]
- Bartier, O.; Hernot, X.; Mauvoisin, G. Theoretical and experimental analysis of contact radius for spherical indentation. Mech. Mater. 2010, 42, 640–656. [Google Scholar] [CrossRef] [Green Version]
- Chaudhri, M.M.; Hutchings, I.M.; Makin, P.L. Plastic compression of spheres. Philos. Mag. A 1984, 49, 493–503. [Google Scholar] [CrossRef]
- Popov, V.L. Analysis of impact on composite structures with the method of dimensionality reduction. Facta Univ. Ser. Mech. Eng. 2015, 13, 39–46. [Google Scholar]
- Lyashenko, I.A. Dynamic model of elastoplastic normal collision of a spherical particle with a half-space with allowance for adhesion interaction in a contact zone. Phys. Solid State 2019, 61, 163–173. [Google Scholar] [CrossRef]
- Kogut, L.; Etsion, I. Elastic-plastic contact analysis of a sphere and a rigid flat. J. Appl. Mech. 2002, 69, 657–662. [Google Scholar] [CrossRef] [Green Version]
- Jackson, R.L.; Green, I. A finite element study of elasto-plastic hemispherical contact against a rigid flat. J. Tribol. 2005, 127, 343–354. [Google Scholar] [CrossRef] [Green Version]
- Quicksall, J.J.; Jackson, R.L.; Green, I. Elasto-plastic hemispherical contact models for various mechanical properties. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2004, 218, 313–322. [Google Scholar] [CrossRef]
- Olemskoi, A.I.; Khomenko, A.V. Phenomenological equations of the glass transition in liquids. Tech. Phys. 2000, 45, 672–676. [Google Scholar] [CrossRef]
- Shankar, S.; Mayuram, M.M. Effect of strain hardening in elastic–plastic transition behavior in a hemisphere in contact with a rigid flat. Int. J. Solids Struct. 2008, 45, 3009–3020. [Google Scholar] [CrossRef] [Green Version]
- Shankar, S.; Mayuram, M.M. A finite element based study on the elastic-plastic transition behavior in a hemisphere in contact with a rigid flat. J. Tribol. 2008, 130, 044502. [Google Scholar] [CrossRef]
- Li, X.Y.; Liang, L.; Wu, S.J. A numerical and effective method for the contact stress calculation of elliptical partial slip. J. Mech. Sci. Technol. 2015, 29, 517–525. [Google Scholar] [CrossRef]
- Bush, A.W.; Gibson, R.D.; Keogh, G.P. Strongly anisotropic rough surfaces. J. Lubr. Technol. 1979, 101, 15–20. [Google Scholar] [CrossRef]
- Horng, J.H. An elliptic elastic-plastic asperity microcontact model for rough surfaces. J. Tribol. 1998, 120, 82–88. [Google Scholar] [CrossRef]
- Jamari, J.; Schipper, D.J. An elastic-plastic contact model of ellipsoid bodies. Tribol. Lett. 2006, 21, 262–271. [Google Scholar] [CrossRef]
- Wen, Y.; Tang, J.; Zhou, W.; Zhu, C. A new elliptical microcontact model considering elastoplastic deformation. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2018, 232, 1352–1364. [Google Scholar] [CrossRef]
- Wen, Y.; Tang, J.; Zhou, W.; Li, L. A reconstruction and contact analysis method of three-dimensional rough surface based on ellipsoidal asperity. J. Tribol. 2020, 142, 041502. [Google Scholar] [CrossRef]
- Jeng, Y.; Wang, P. An elliptical microcontact model considering elastic, elastoplastic, and plastic deformation. J. Tribol. 2003, 125, 232–240. [Google Scholar] [CrossRef]
- Chung, J.C. Elastic-plastic contact analysis of an ellipsoid and a rigid flat. Tribol. Int. 2010, 43, 491–502. [Google Scholar] [CrossRef]
- Li, P.; Zhou, L.; Cui, F.; Wang, Q.; Guo, M.; Zhai, Y.; Li, Y.; Hua, D. Elasto-plastic analysis of the contact region between a rigid ellipsoid and a semi-flat surface. Adv. Mech. Eng. 2018, 10, 168781401879739. [Google Scholar] [CrossRef] [Green Version]
- Jackson, R.L.; Kogut, L. A comparison of flattening and indentation approaches for contact mechanics modeling of single asperity contacts. J. Tribol. 2006, 128, 209–212. [Google Scholar] [CrossRef]
- Becker, V.; Kamlah, M. A theoretical model for the normal contact force of two elastoplastic ellipsoidal bodies. J. Appl. Mech. 2021, 88, 3. [Google Scholar] [CrossRef]
- Lin, L.P.; Lin, J.F. An elliptical elastic-plastic microcontact model developed for an ellipsoid in contact with a smooth rigid flat. J. Tribol. 2007, 129, 772–782. [Google Scholar] [CrossRef]
- Song, Z.; Komvopoulos, K. An elastic-plastic analysis of spherical indentation: Constitutive equations for single-indentation unloading and development of plasticity due to repeated indentation. Mech. Mater. 2014, 76, 93–101. [Google Scholar] [CrossRef]
- Etsion, I.; Kligerman, Y.; Kadin, Y. Unloading of an elastic-plastic loaded spherical contact. Int. J. Solids Struct. 2005, 42, 716–3729. [Google Scholar] [CrossRef]
- Sahoo, P.; Chatterjee, B. A finite element study of elastic-plastic hemispherical contact behavior against a rigid flat under varying modulus of elasticity and sphere radius. Engineering 2010, 2, 205–211. [Google Scholar] [CrossRef] [Green Version]





| ke | Et | E (GPa) | Y (GPa) | R (mm) | ν | |
|---|---|---|---|---|---|---|
| 1 | 1/5 | 0; 0.025E; 0.05E; 0.075E; 0.1E | 207 | 0.7 | 1 | 0.3 |
| 2 | 1/2 | |||||
| 3 | 1 |
| Hertz | Simulation | Hertz | Simulation | Hertz | Simulation | |
|---|---|---|---|---|---|---|
| ke | 1/5 | 1/5 | 1/2 | 1/2 | 1 | 1 |
| F* | 6.98 | 6.95 | 2.61 | 2.63 | 1 | 0.986 |
| ω* | 3.21 | 3.19 | 1.85 | 1.83 | 1 | 0.99 |
| A* | 1.82 | 1.83 | 1.44 | 1.47 | 1 | 0.988 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, J.; Zhang, W.; Wang, C.; Liu, D.; Zhu, L. Effect of Strain Hardening and Ellipticity on Elastic–Plastic Contact Behaviour between Ellipsoids and Rigid Planes. Machines 2022, 10, 488. https://doi.org/10.3390/machines10060488
Chen J, Zhang W, Wang C, Liu D, Zhu L. Effect of Strain Hardening and Ellipticity on Elastic–Plastic Contact Behaviour between Ellipsoids and Rigid Planes. Machines. 2022; 10(6):488. https://doi.org/10.3390/machines10060488
Chicago/Turabian StyleChen, Jian, Wangyang Zhang, Chenglong Wang, Di Liu, and Linbo Zhu. 2022. "Effect of Strain Hardening and Ellipticity on Elastic–Plastic Contact Behaviour between Ellipsoids and Rigid Planes" Machines 10, no. 6: 488. https://doi.org/10.3390/machines10060488






