A Decoupling Algorithm-Based Technology for Predicting and Regulating the Unbalance of Aircraft Rotor Assembly Considering Manufacturing Errors
Abstract
:1. Introduction
2. Rotor Unbalance Prediction Model
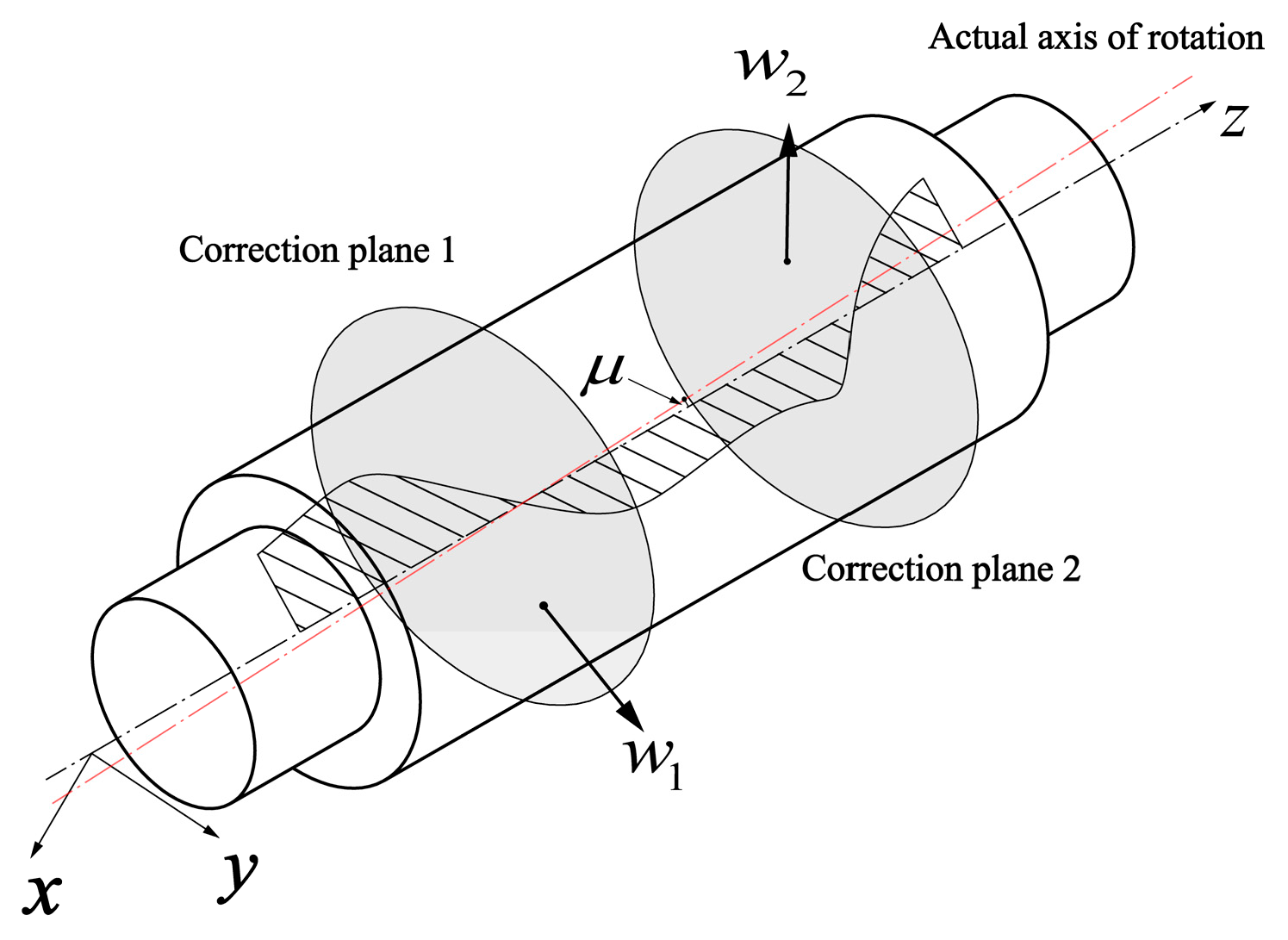
2.1. Decoupling Algorithm of Rotor Unbalance
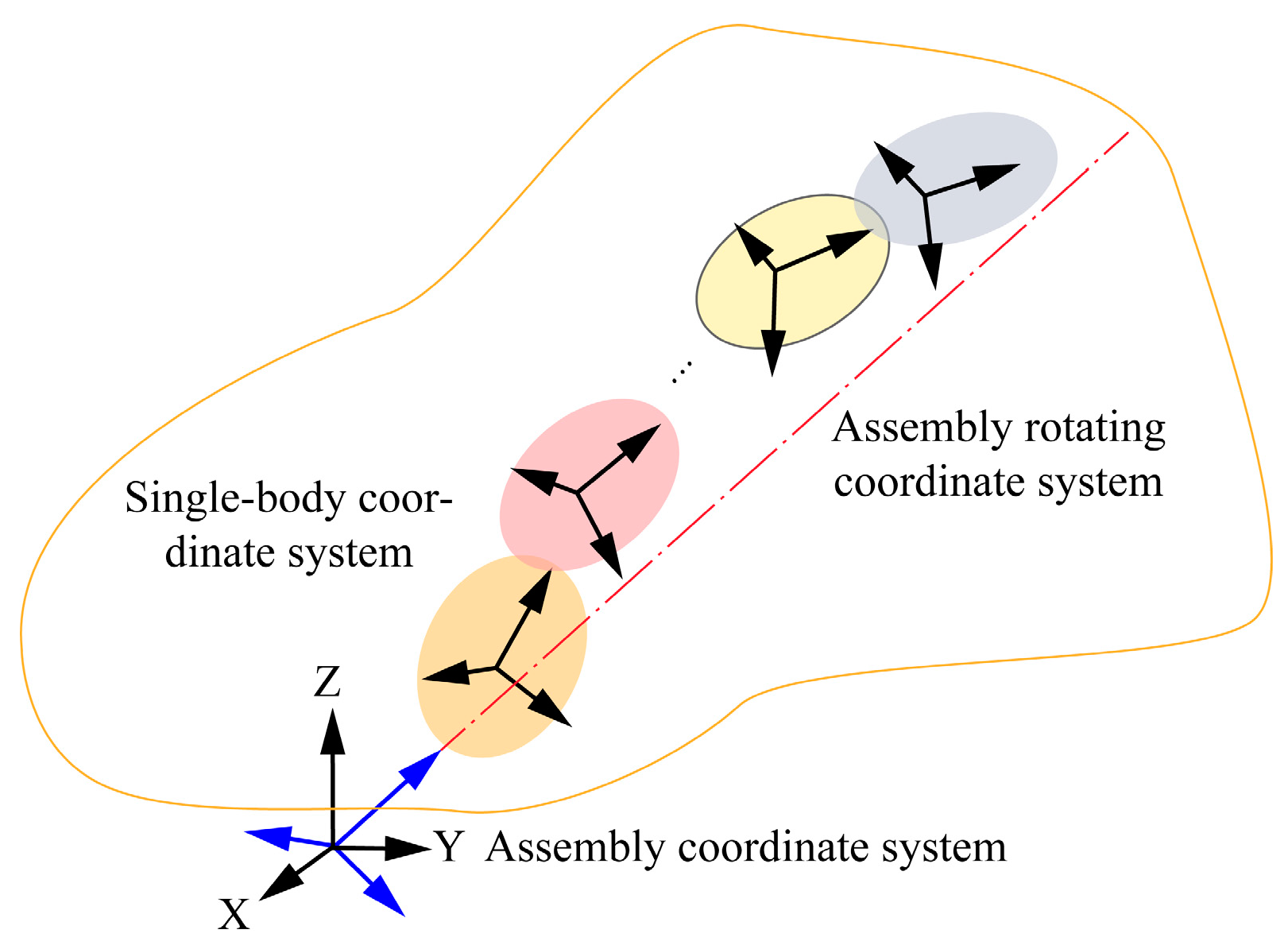
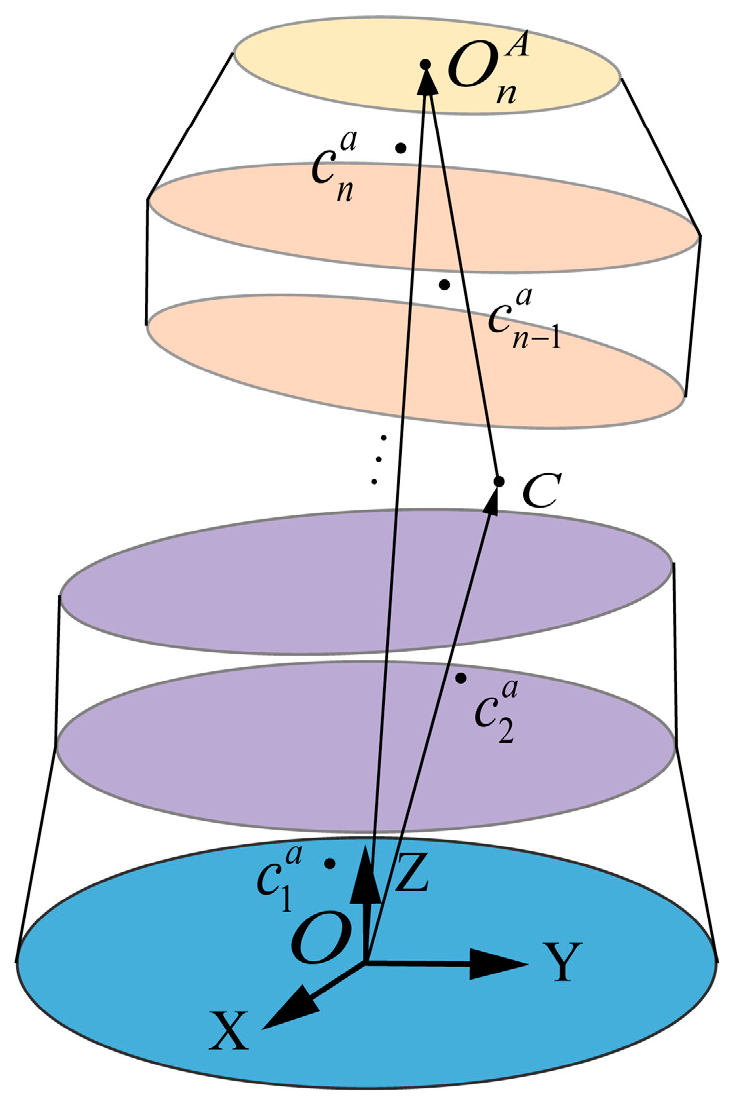
2.2. Prediction Model for Multi-Stage Rotor Unbalance
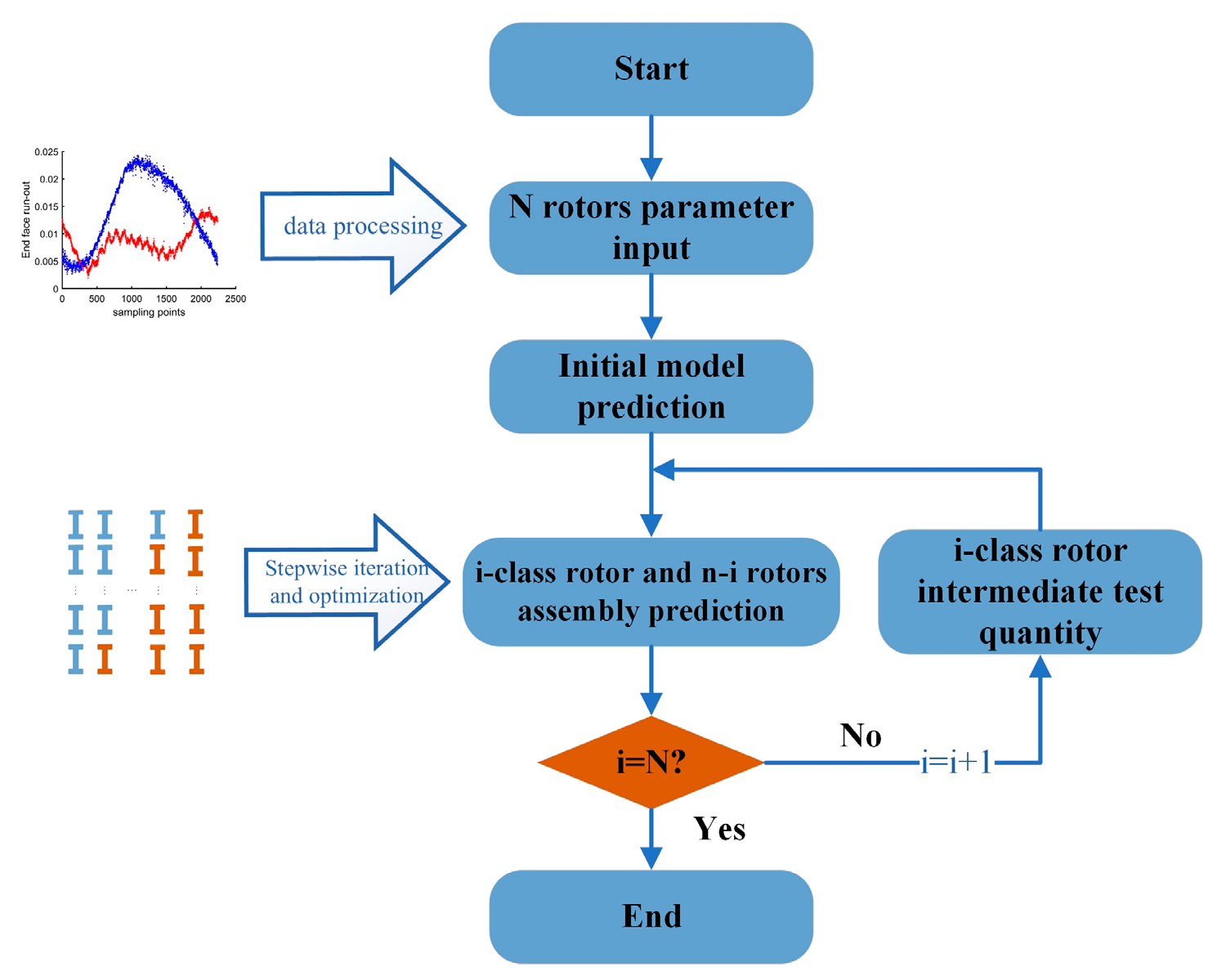
3. Model Correction Based on Experimental Values as Inputs
3.1. Unbalance Measurement Method during the Assembly Synchronization Process
3.2. Method of Model Correction with Intermediate Test Measurements as Input
4. Experimental Design
4.1. Measurement of Rotor Unbalance
4.2. Measurement of Rotor Manufacturing Errors
5. Discussion
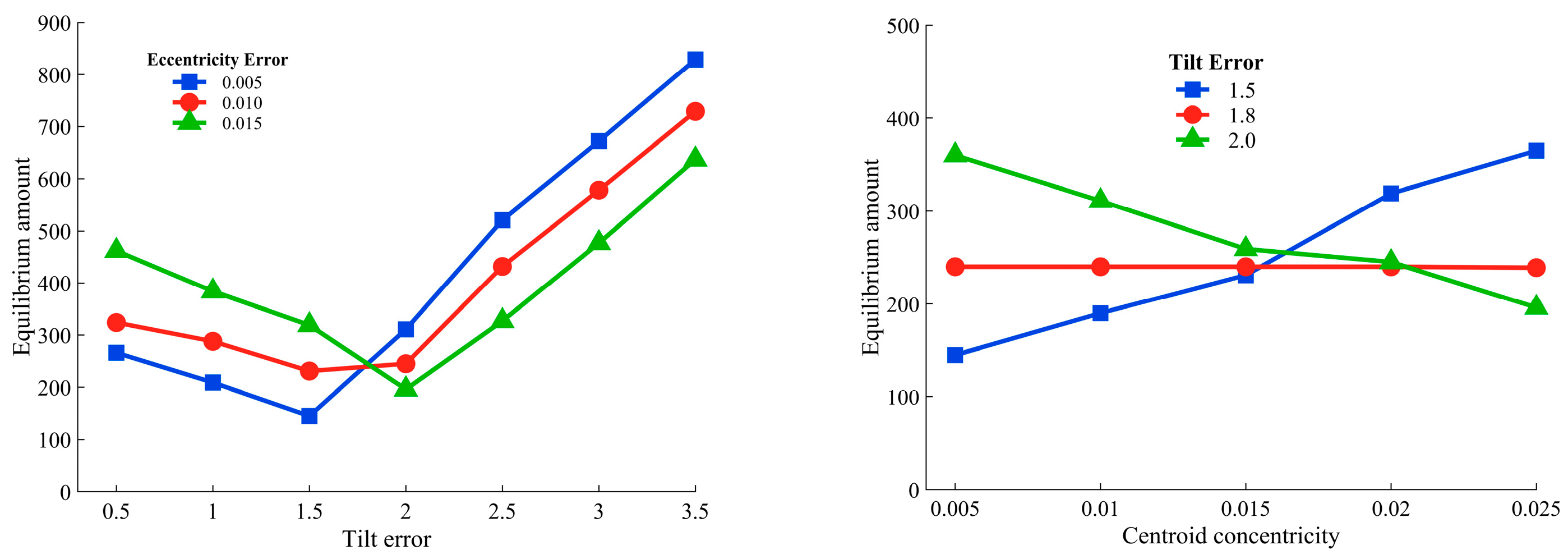
5.1. The Relationship between Unbalance and Centroid Concentricity
5.2. The Impact of Manufacturing Errors on Unbalance
6. Conclusions
- (1)
- After model correction, the assembly unbalance decreased by 42.2% compared to the control group. The unbalance levels after model correction reduced by 15.8% compared to before correction, confirming the effectiveness of the model adjustment. The theoretical values after model correction matched experimental values by 91.3%, and the average error is reduced by 15.3% compared to before correction.
- (2)
- The relationship between the concentricity of the center of mass and unbalance levels was explored. As concentricity decreased, assembly unbalance initially decreased and then increased. During rotor assembly, both factors should be reasonably considered.
- (3)
- The impact of manufacturing errors on unbalance levels and concentricity was studied. With increasing tilt error, unbalance initially decreased and then increased. When tilt error falls within a reasonable range, eccentricity error has minimal impact on unbalance. As tilt error increases, the concentricity at the point of optimal unbalance initially decreases and then increases. As eccentricity error increases from 0.005 to 0.015, the concentricity at the point of optimal unbalance remains around 0.06. To simultaneously control rotor concentricity while achieving optimal unbalance, particular attention should be paid to tilt errors during rotor machining and subsequent assembly. These research findings can effectively support the quality adjustment of multi-stage rotor assemblies.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Sun, W.; Li, T.; Yang, D.; Sun, Q.; Huo, J. Dynamic investigation of aeroengine high pressure rotor system considering assembly characteristics of bolted joints. Eng. Fail. Anal. 2020, 112, 104510. [Google Scholar] [CrossRef]
- Guptak, K.; Guptak, D. Unbalance response of a dual rotor system: Theory and experiment. J. Vib. Acoust. 1993, 115, 427–435. [Google Scholar] [CrossRef]
- Chen, X.; Zhai, J.; Zhang, H.; Han, Q. Simulation study on unbalance vibration characteristics of dual-rotor system. SN Appl. Sci. 2020, 2, 1423. [Google Scholar] [CrossRef]
- Ma, P.; Zhai, J.; Wang, Z.; Zhang, H.; Han, Q. Unbalance vibration characteristics and sensitivity analysis of the dual-rotor system in aeroengines. J. Aerosp. Eng. 2021, 34, 04020094. [Google Scholar] [CrossRef]
- Wang, A.; Bi, Y.; Cheng, X.; Yang, J.; Meng, G.; Xia, Y.; Feng, Y. Continuous rotor dynamics of multi-disc and multi-span rotors: A theoretical and numerical In-vestigation of the Identification of rotor unbalance from unbalance responses. Appl. Sci. 2022, 12, 4251. [Google Scholar] [CrossRef]
- Zhang, H.; Feng, G.; Wang, H.; Gu, F.; Sinha, J. Proceedings of the IncoME-VI and TEPEN 2021: Performance Engineering and Maintenance Engineering; Springer Nature: Berlin/Heidelberg, Germany, 2022. [Google Scholar]
- He, Q.; Peng, H.; Zhai, P.; Zhen, Y. The effects of unbalance orientation angle on the stability of the lateral torsion coupling vibration of an accelerated rotor with a transverse breathing crack. Mech. Syst. Signal Process. 2016, 75, 330–344. [Google Scholar] [CrossRef]
- Liu, Y.; Zhang, M.; Sun, C.; Hu, M.; Chen, D.; Liu, Z.; Tan, J. A method to minimize stage-by-stage initial unbalance in the aero engine assembly of multistage rotors. Aerosp. Sci. Technol. 2019, 85, 270–276. [Google Scholar] [CrossRef]
- Wang, D.; Wang, N.; Chen, K. Unbalance response of a magnetic suspended dual-rotor system. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2019, 233, 5758–5772. [Google Scholar] [CrossRef]
- Deepthikumar, M.B.; Sekhar, A.S.; Srikanthan, M.R. Modal balancing of flexible rotors with bow and distributed unbalance. J. Sound Vib. 2013, 332, 6216–6233. [Google Scholar] [CrossRef]
- Deepthikumar, M.B.; Sekhar, A.S.; Srikanthan, M.R. Balancing of flexible rotor with bow using transfer matrix method. J. Vib. Control 2014, 20, 225–240. [Google Scholar] [CrossRef]
- Wang, L.K.; Bin, G.F.; Han, Q.K.; Li, X.J. Experimental Investigation of Unbalance Location on Vibration Characteristics of Double Overhung Rotor with Oil Film Bearings. In Energy and Mechanical Engineering: Proceedings of the 2015 International Conference on Energy and Mechanical Engineering; World Scientific: Singapore, 2016; pp. 1022–1030. [Google Scholar]
- Mu, X.; Wang, Y.; Yuan, B.; Sun, W.; Liu, C.; Sun, Q. A New assembly precision prediction method of aeroengine high-pressure rotor system considering manufacturing error and deformation of parts. J. Manuf. Syst. 2021, 61, 112–124. [Google Scholar] [CrossRef]
- Sun, C.; Chen, D.; Li, C.; Liu, Y.; Liu, Z.; Hu, M.; Tan, J. A novel constrained optimization-build method for precision assembly of aircraft engine. Assem. Autom. 2019, 40, 869–879. [Google Scholar] [CrossRef]
- Zhang, Y.; Li, M.; Hu, Y. Model-based balancing method of rotors using differential evolution algorithm. In IOP Conference Series: Materials Science and Engineering; IOP Publishing: Bristol, UK, 2020; Volume 751, p. 012046. [Google Scholar]
- Zhang, Z.; Zhang, Z.; Jin, X.; Zhang, Q. A novel modelling method of geometric errors for precision assembly. Int. J. Adv. Manuf. Technol. 2018, 94, 1139–1160. [Google Scholar] [CrossRef]
- Li, M.; Wang, Y.; Sun, Q.; Mu, X. Assembly accuracy prediction and optimization of aero-engine rotor under the separation condition of assembly and measurement. Int. J. Adv. Manuf. Technol. 2022, 120, 3103–3112. [Google Scholar] [CrossRef]
- Zhu, Z.; Qiao, L. Analysis and control of assembly precision in different assembly sequences. Procedia CIRP 2015, 27, 117–123. [Google Scholar] [CrossRef]
- Sun, C.; Hu, M.; Liu, Y.; Zhang, M.; Liu, Z.; Chen, D.; Tan, J. A method to control the amount of unbalance propagation in precise cylindrical components assembly. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 2019, 233, 2458–2468. [Google Scholar] [CrossRef]
- Sun, C.; Liu, Z.; Liu, Y.; Wang, X.; Tan, J. An adjustment method of geometry and mass centers for precision rotors assembly. IEEE Access 2019, 7, 169992–170002. [Google Scholar] [CrossRef]
- Li, R.; Liu, Y.; Sun, C.; Wang, D.; Tan, J. Measurement and adjustment of the centroid deviation of aero-engine rotors based on unbalance decoupling. Measurement 2023, 212, 112660. [Google Scholar] [CrossRef]
- Chen, Y.; Cui, J.; Sun, X. An unbalance optimization method for a multi-stage rotor based on an assembly error propagation model. Appl. Sci. 2021, 11, 887. [Google Scholar] [CrossRef]
- Lan, L.; Liu, X.; Wang, Q. Fault detection and classification of the rotor unbalance based on dynamics features and support vector machine. Meas. Control 2023, 56, 1075–1086. [Google Scholar] [CrossRef]
- Sudhakar, G.; Sekhar, A.S. Identification of unbalance in a rotor bearing system. J. Sound Vib. 2011, 330, 2299–2313. [Google Scholar] [CrossRef]
- Wang, M.; Wang, J.; Tang, W.; Zhang, X.; Peng, Y.; Li, Z. Mass and center of gravity measurement of large size revolving bodies and error separation method. In Proceedings of the 2019 IEEE 3rd Information Technology, Networking, Electronic and Automation Control Conference (ITNEC), Chengdu, China, 15–17 March 2019; IEEE: Piscataway, NJ, USA, 2019; pp. 1638–1641. [Google Scholar]
- Zhang, X.; Wang, M.; Tang, W.; Wang, J. A flexible measurement technique for testing the mass and center of gravity of large-sized objects. Meas. Sci. Technol. 2019, 31, 015006. [Google Scholar] [CrossRef]
- Li, S.; Chu, W.; Huang, X. Measurement method of aircraft barycenter based on multi posture. Sens. Rev. 2020, 40, 217–226. [Google Scholar] [CrossRef]
- Na, Q.; Hu, S.; Tao, J.; Luo, Y. Adaptive quasi-Monte Carlo method for uncertainty evaluation in centroid measurement of planetary rovers. Trans. Inst. Meas. Control 2021, 43, 623–634. [Google Scholar] [CrossRef]
- Tang, L.; Shangguan, W.B. An improved pendulum method for the determination of the center of gravity and inertia tensor for irregular-shaped bodies. Measurement 2011, 44, 1849–1859. [Google Scholar] [CrossRef]
- Previati, G.; Gobbi, M.; Mastinu, G. Measurement of the mass properties of rigid bodies by means of multi-filar pendulums–influence of test rig flexibility. Mech. Syst. Signal Process. 2019, 121, 31–43. [Google Scholar] [CrossRef]
- Gobbi, M.; Mastinu, G.; Previati, G. A method for measuring the inertia properties of rigid bodies. Mech. Syst. Signal Process. 2011, 25, 305–318. [Google Scholar] [CrossRef]
- Sun, Q.; Zhao, B.; Liu, X.; Mu, X.; Zhang, Y. Assembling deviation estimation based on the real mating status of assembly. Comput.-Aided Des. 2019, 115, 244–255. [Google Scholar] [CrossRef]


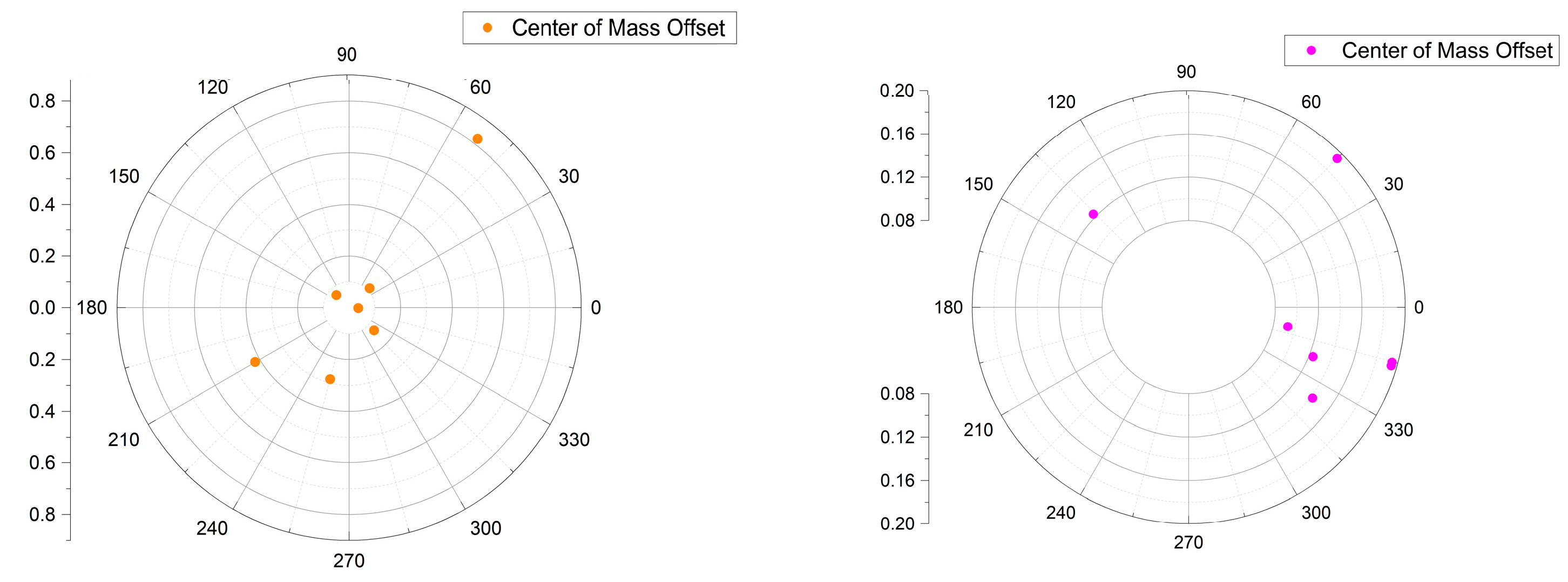

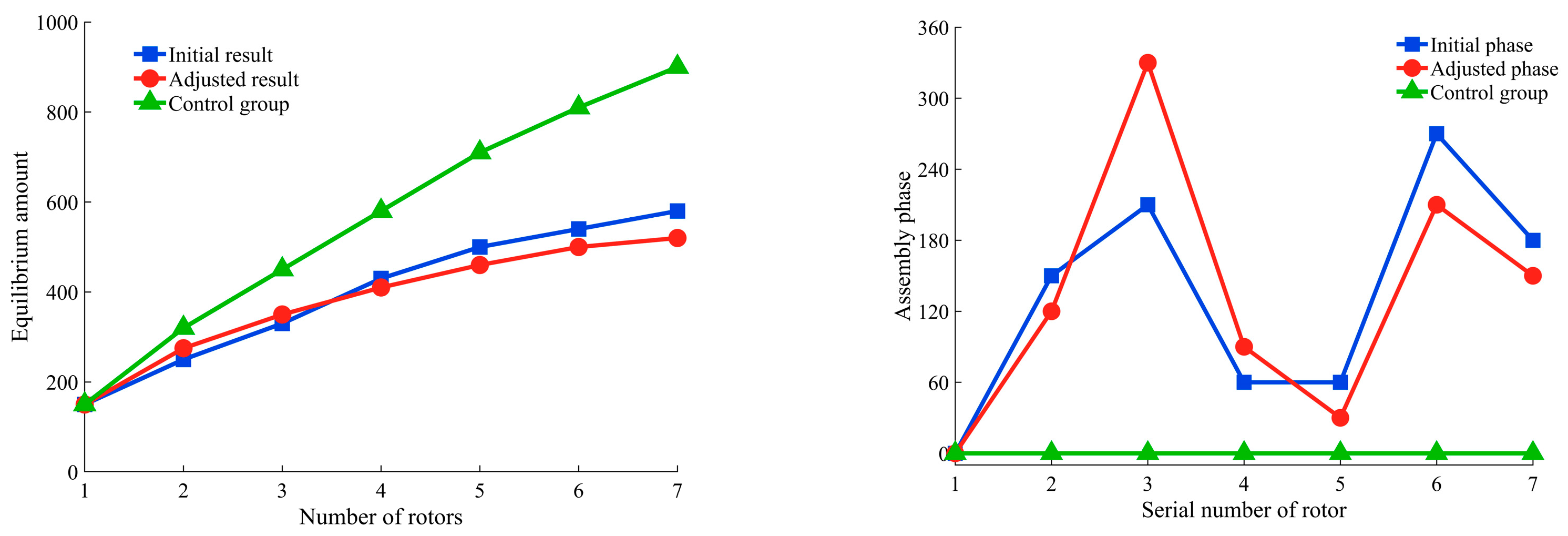


| Rotors | (°) | (°) | ||
|---|---|---|---|---|
| 1 | 169 | 84 | 147 | 256 |
| 2 | 273 | 225 | 98 | 72 |
| 3 | 229 | 200 | 77 | 230 |
| 4 | 78 | 215 | 165 | 188 |
| 5 | 164 | 305 | 118 | 322 |
| 6 | 53 | 77 | 284 | 174 |
| 7 | 120 | 95 | 254 | 33 |
| Rotors | Eccentricity Error | Tilt Error | ||
|---|---|---|---|---|
| (mm) | (mm) | (e − 5) | (e − 5) | |
| 1 | 0.0071 | −0.0080 | 0.9258 | 3.0230 |
| 2 | −0.0163 | 0.0012 | 1.8970 | 3.6620 |
| 3 | 0.0020 | 0.0113 | 0.6221 | 2.2835 |
| 4 | 0.0125 | −0.0015 | 1.4430 | 0.0398 |
| 5 | −0.0065 | −0.0027 | 1.3600 | 0.3945 |
| 6 | −0.0031 | 0.0037 | 0.3258 | 1.5660 |
| 7 | 0.0094 | −0.0045 | 0.7441 | 1.8358 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, Y.; Mu, X.; Liu, J.; Sun, Q.; Zhou, P.; Fang, G. A Decoupling Algorithm-Based Technology for Predicting and Regulating the Unbalance of Aircraft Rotor Assembly Considering Manufacturing Errors. Machines 2023, 11, 970. https://doi.org/10.3390/machines11100970
Zhao Y, Mu X, Liu J, Sun Q, Zhou P, Fang G. A Decoupling Algorithm-Based Technology for Predicting and Regulating the Unbalance of Aircraft Rotor Assembly Considering Manufacturing Errors. Machines. 2023; 11(10):970. https://doi.org/10.3390/machines11100970
Chicago/Turabian StyleZhao, Yingjie, Xiaokai Mu, Jian Liu, Qingchao Sun, Ping Zhou, and Guozhen Fang. 2023. "A Decoupling Algorithm-Based Technology for Predicting and Regulating the Unbalance of Aircraft Rotor Assembly Considering Manufacturing Errors" Machines 11, no. 10: 970. https://doi.org/10.3390/machines11100970
APA StyleZhao, Y., Mu, X., Liu, J., Sun, Q., Zhou, P., & Fang, G. (2023). A Decoupling Algorithm-Based Technology for Predicting and Regulating the Unbalance of Aircraft Rotor Assembly Considering Manufacturing Errors. Machines, 11(10), 970. https://doi.org/10.3390/machines11100970









