Dynamic Characteristics of a Rotating Blade with a Dovetail Fixture
Abstract
1. Introduction
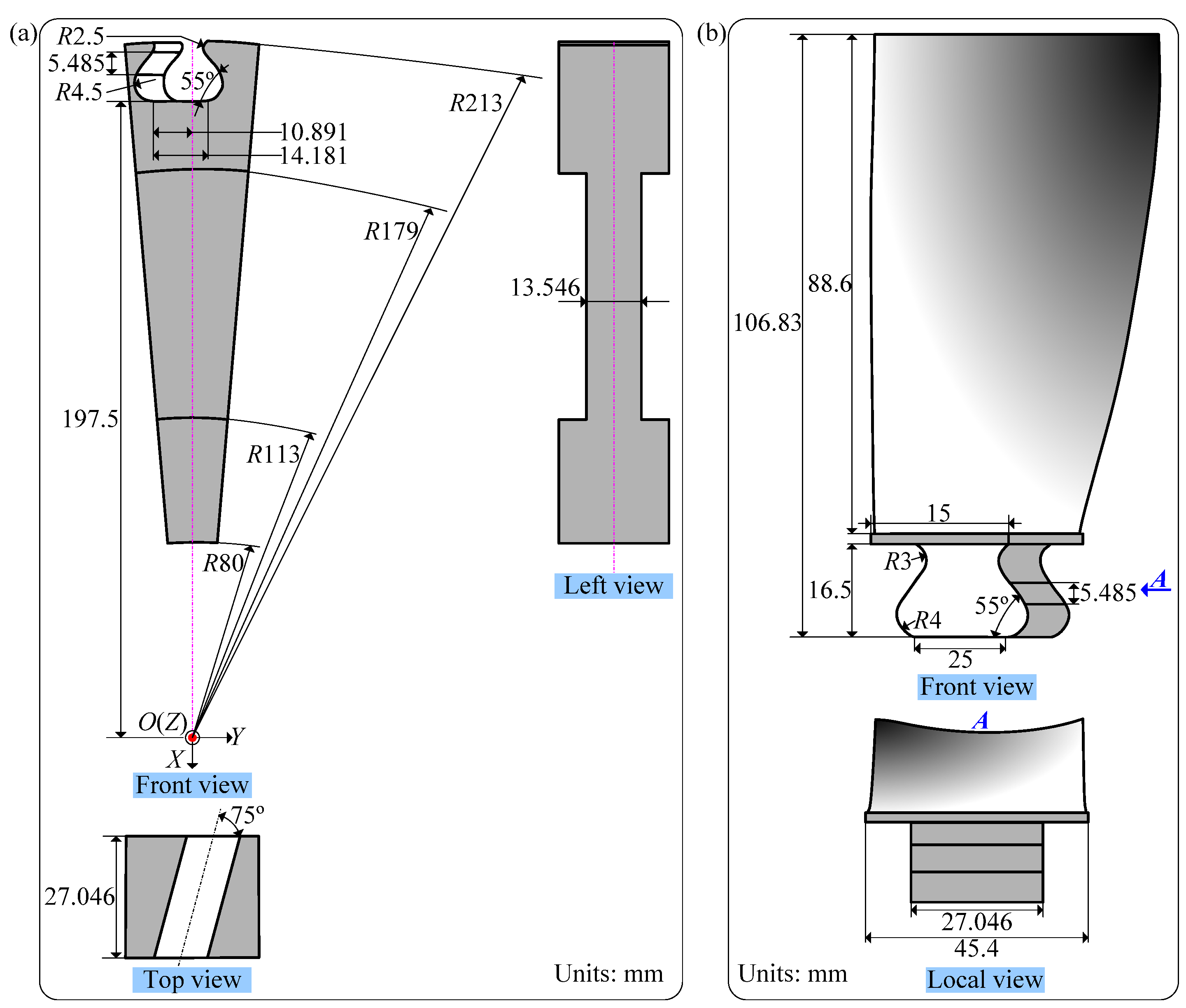
2. Finite Element Model
3. Dynamic Characteristic Analysis
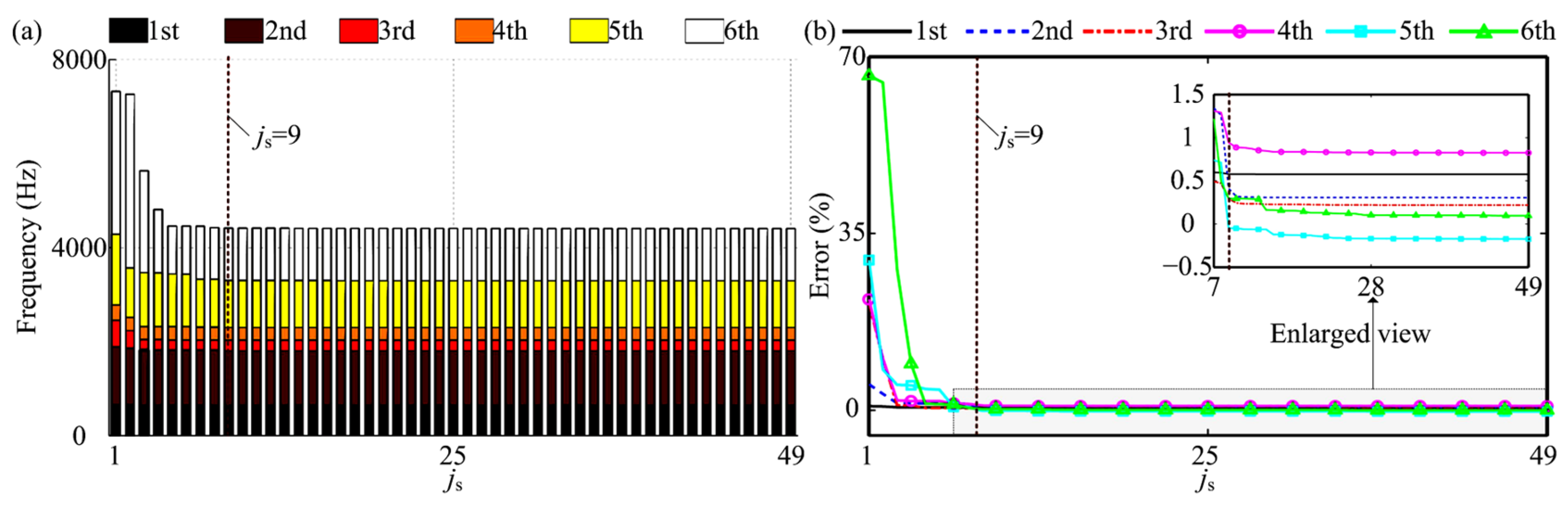
3.1. Modal Characteristics
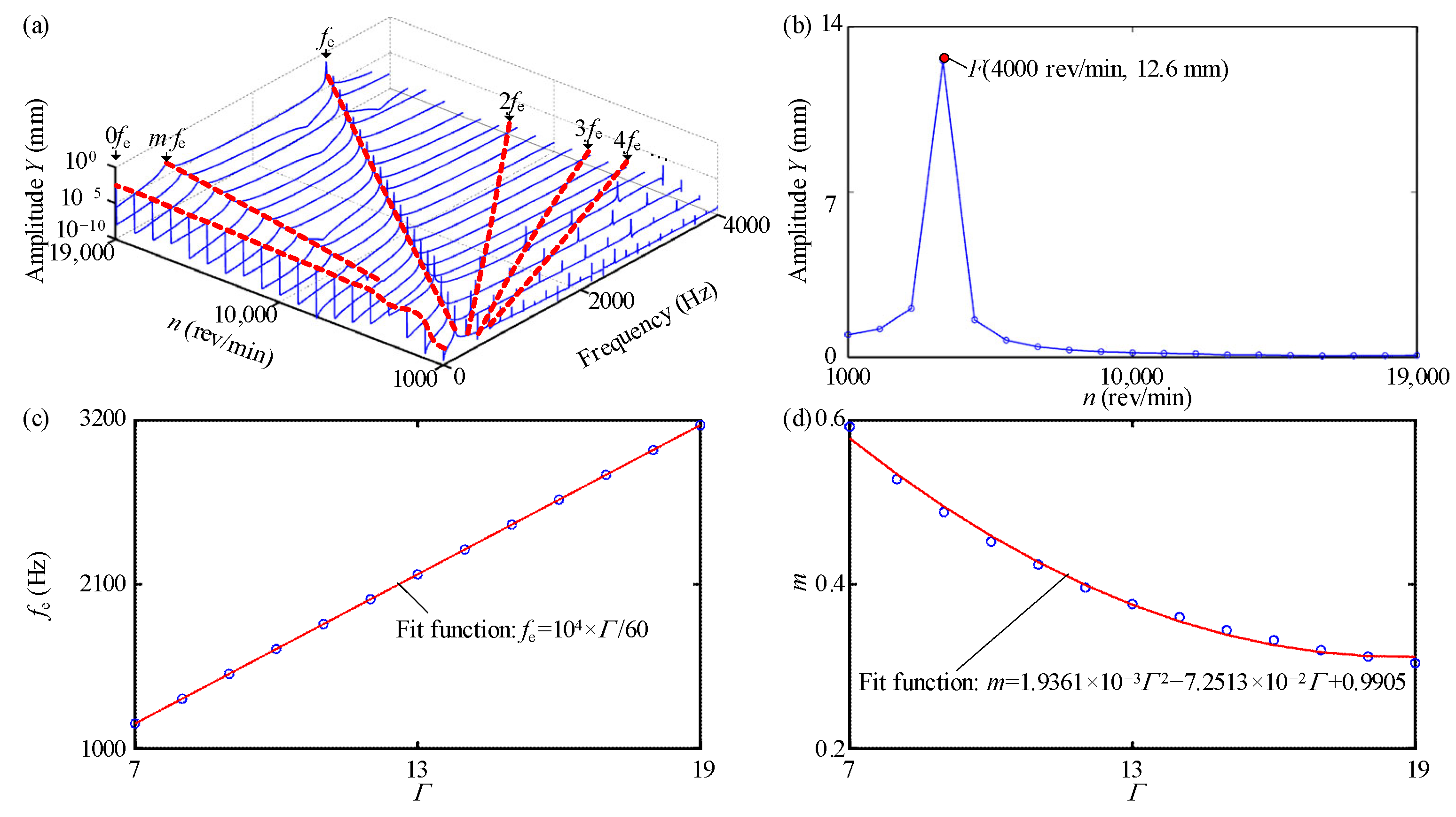
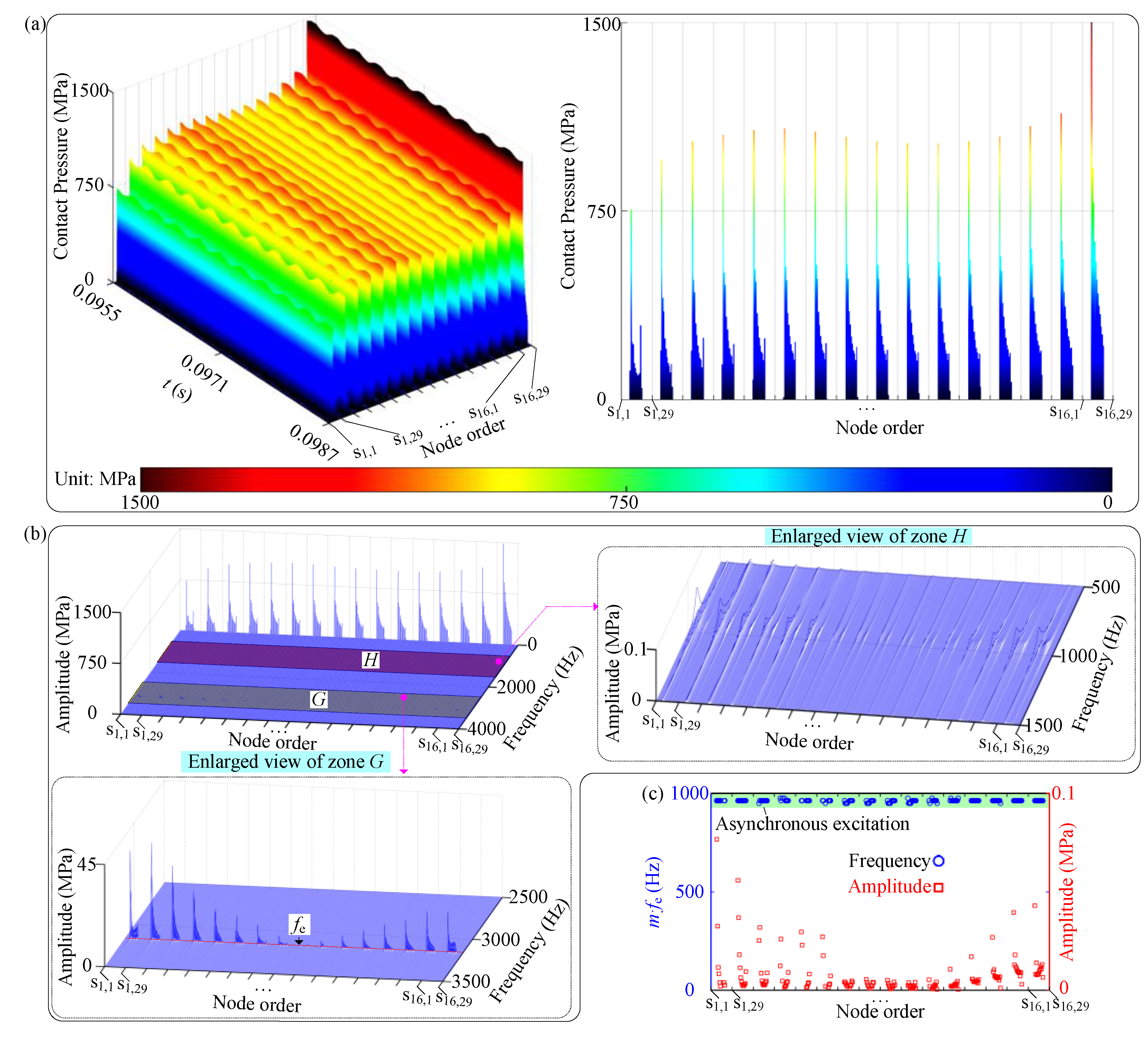
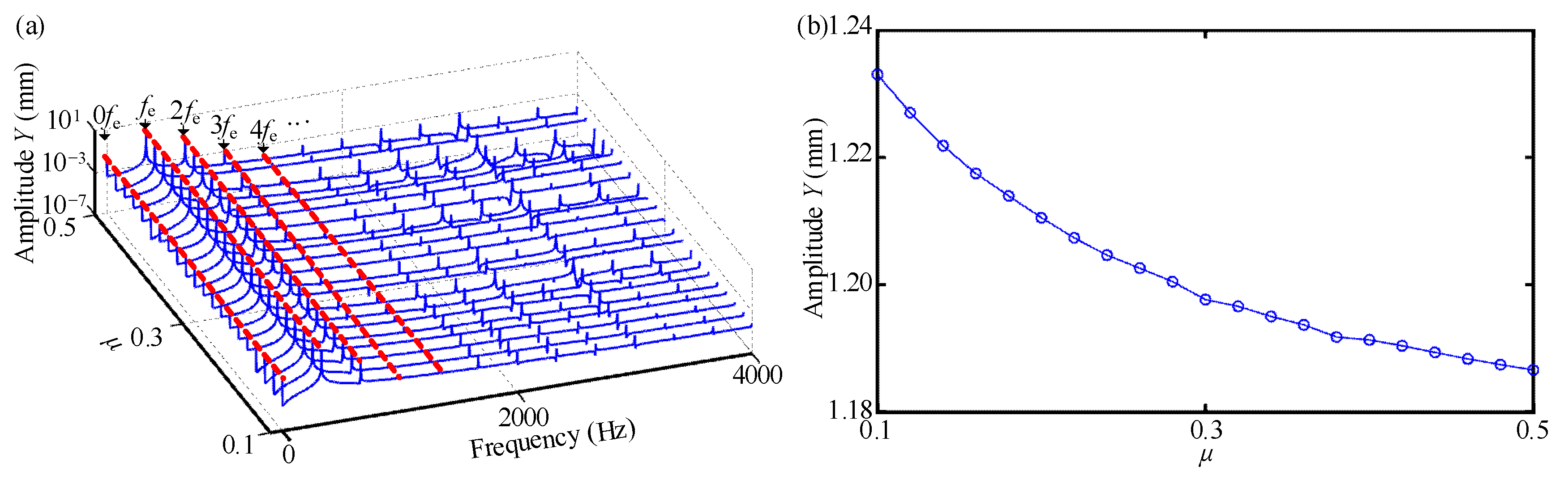
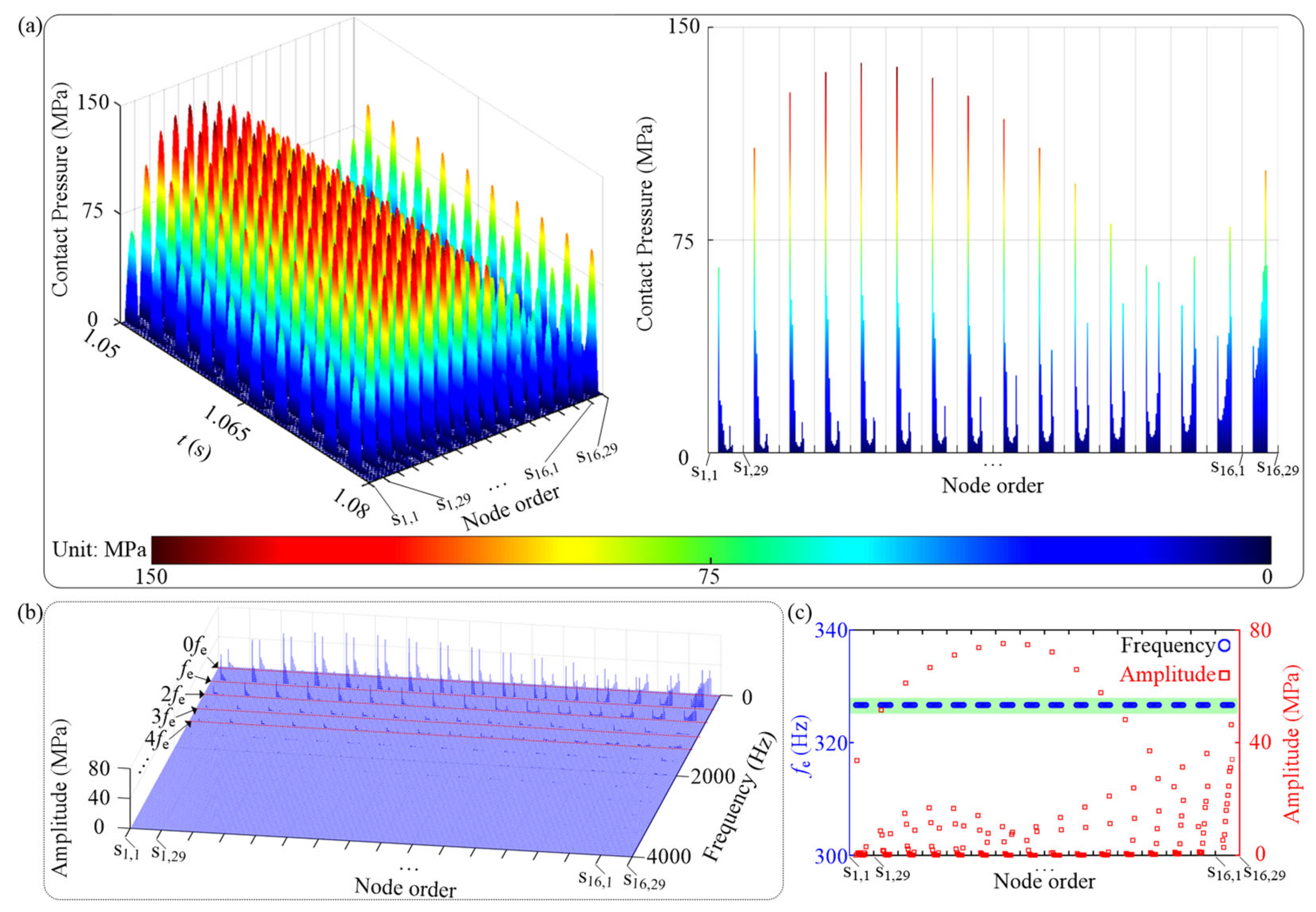
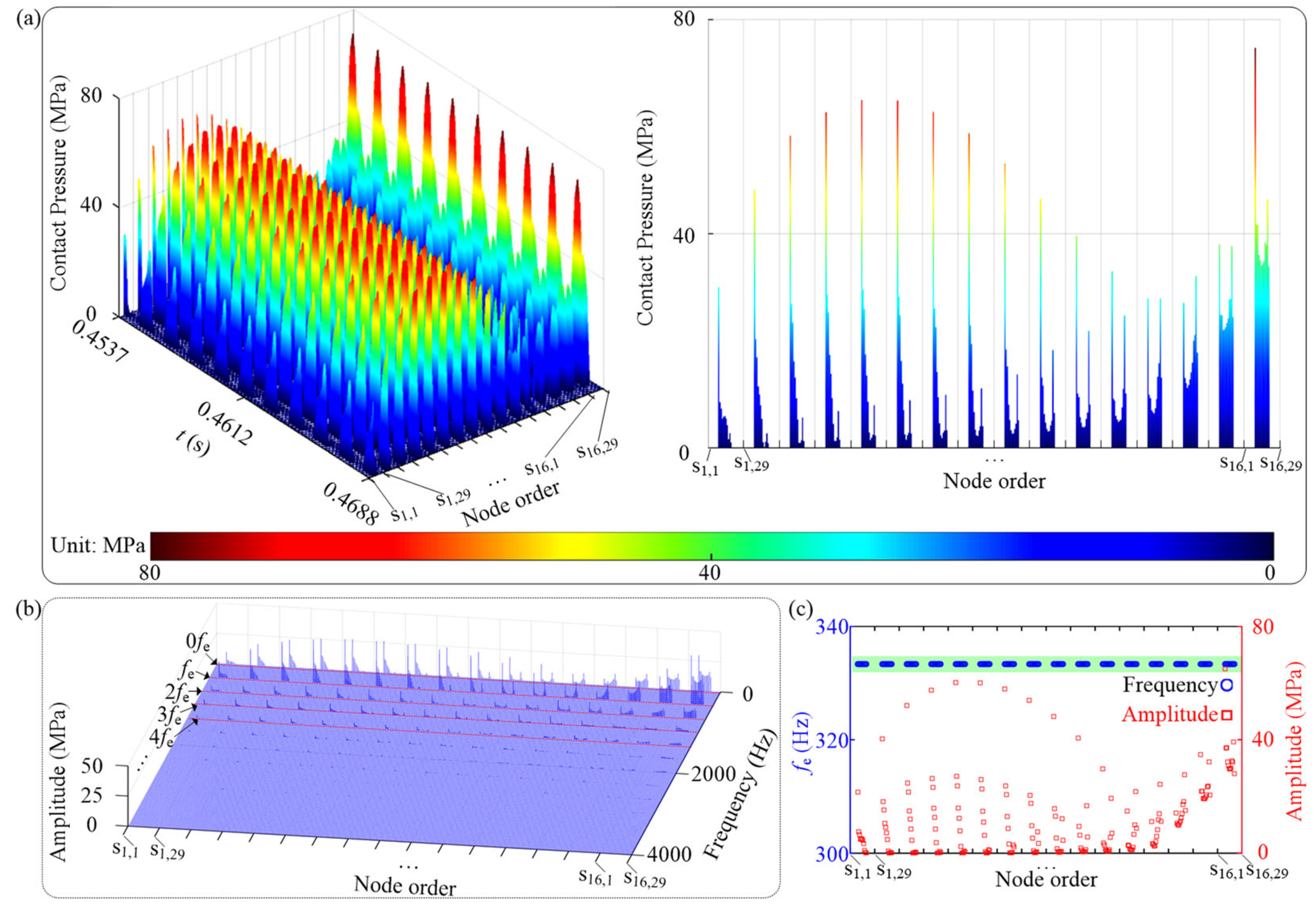
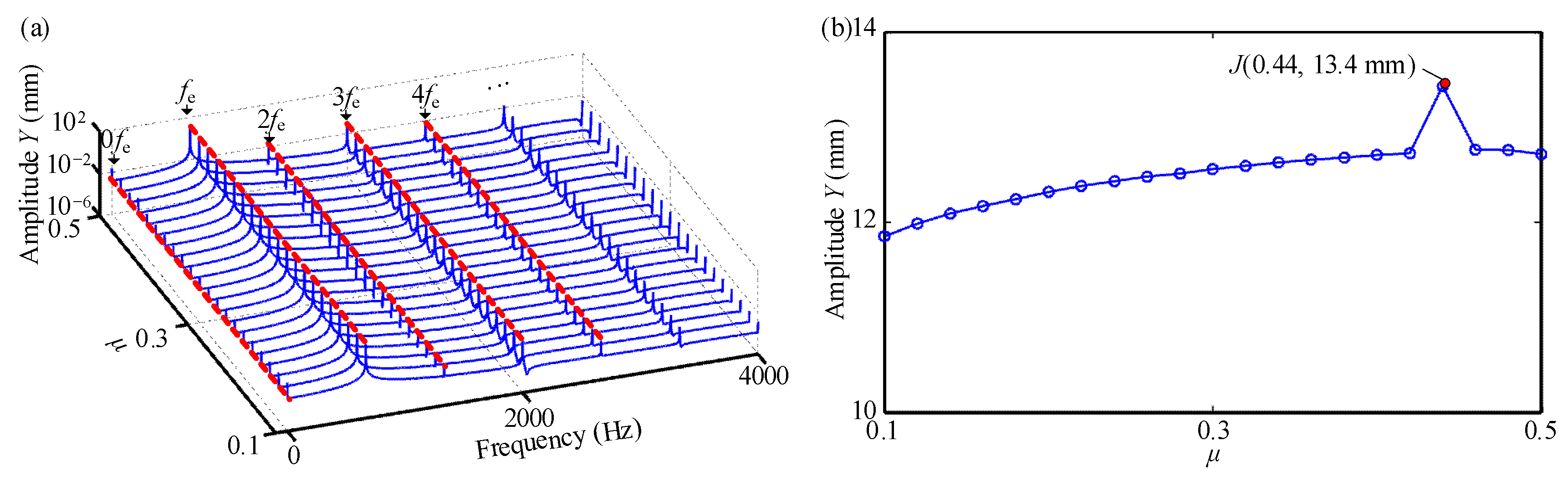
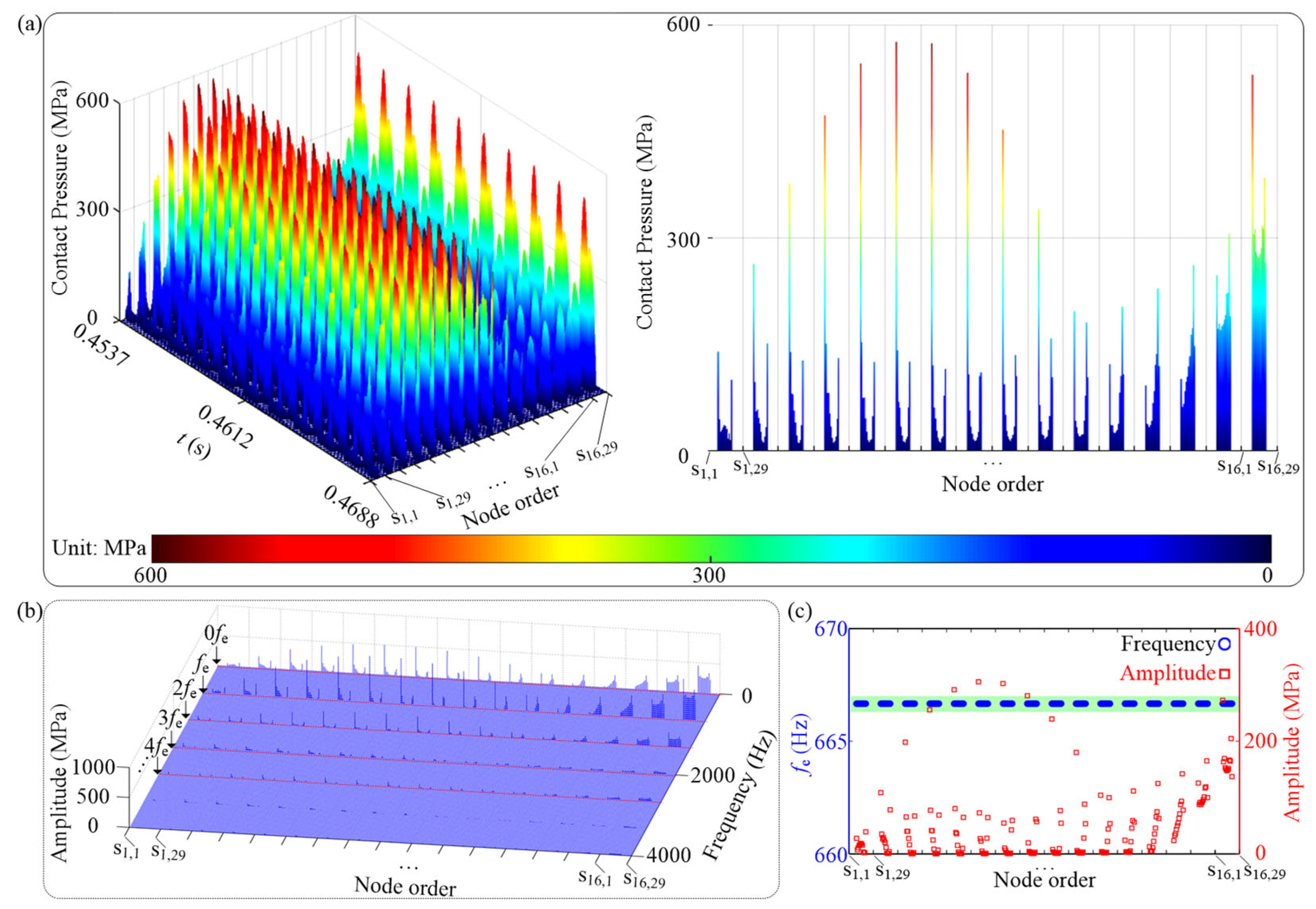
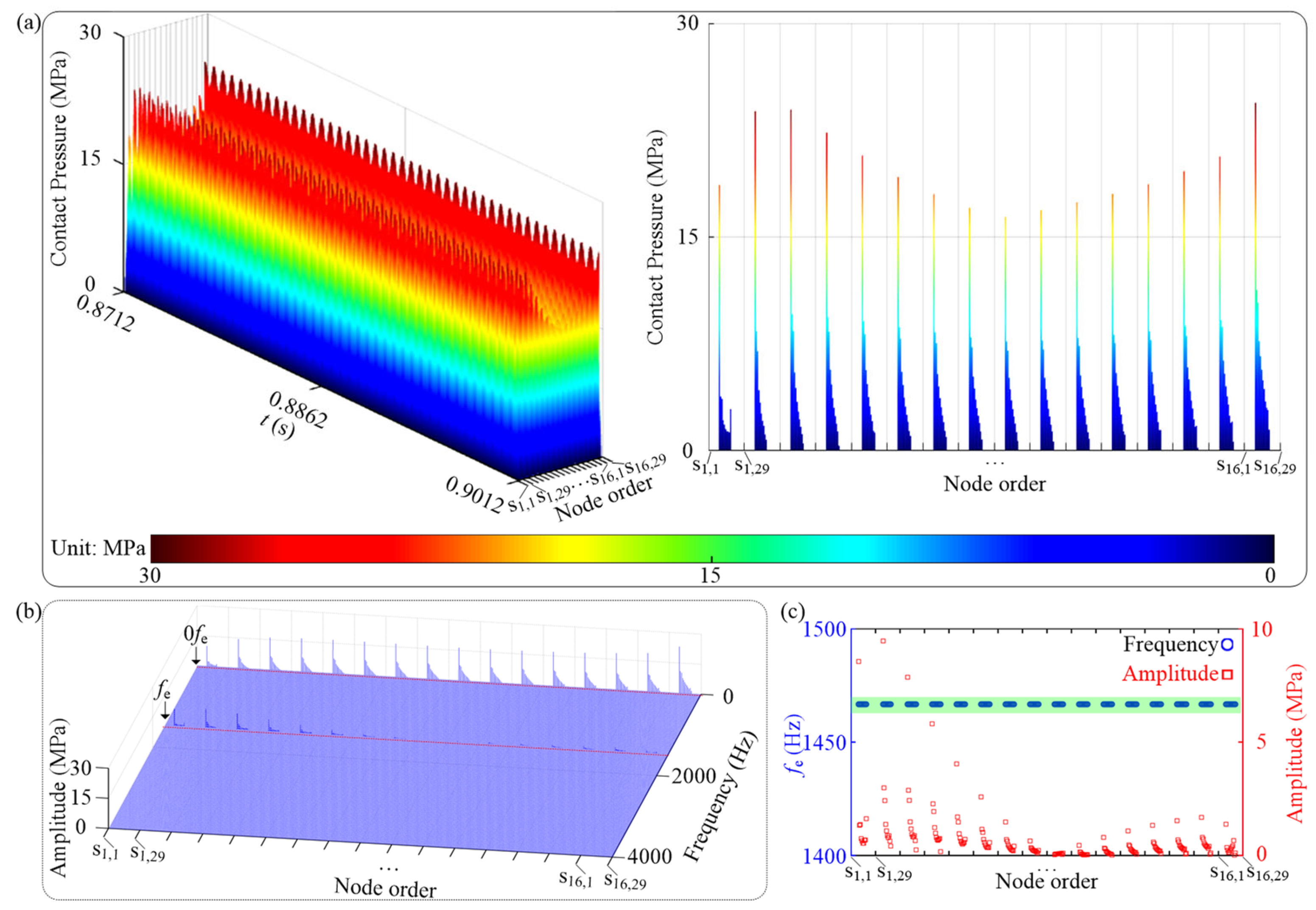
3.2. Nonlinear Vibration Characteristics
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Ma, H.; Wang, D.; Tai, X.Y.; Wen, B.C. Vibration response analysis of blade-disk dovetail structure under blade tip rubbing condition. J. Vib. Control 2017, 23, 252–271. [Google Scholar] [CrossRef]
- Quaegebeur, S.; Chouvion, B.; Thouverez, F. Nonlinear dynamic analysis of three-dimensional bladed-disks with frictional contact interfaces based on cyclic reduction strategies. Int. J. Solids Struct. 2022, 236–237, 111277. [Google Scholar] [CrossRef]
- Li, C.F.; Shen, Z.C.; Zhong, B.F.; Wen, B.C. Study on the nonlinear characteristics of a rotating flexible blade with dovetail interface feature. Shock. Vib. 2018, 2018, 4923898. [Google Scholar] [CrossRef]
- Li, C.F.; Liu, X.W.; Tang, Q.S.; Chen, Z.L. Modeling and nonlinear dynamics analysis of a rotating beam with dry friction support boundary conditions. J. Sound Vib. 2021, 498, 115978. [Google Scholar] [CrossRef]
- She, H.X.; Li, C.F.; Tang, Q.S.; Wen, B.C. Veering and merging analysis of nonlinear resonance frequencies of an assembly bladed disk system. J. Sound Vib. 2021, 493, 115818. [Google Scholar] [CrossRef]
- Shangguan, B.; Xu, Z. Experimental study of friction damping of blade with loosely assembled dovetail attachment. Proc. Inst. Mech. Eng. Part A J. Power Energy 2012, 226, 738–750. [Google Scholar] [CrossRef]
- Shangguan, B.; Yu, F.L.; Duan, J.Y.; Gao, S.; Xiao, J.F. A fractal contact friction model and nonlinear vibration response studies of loosely assembled blade with dovetail root. In Proceedings of the ASME Turbo Expo 2016: Turbomachinery Technical Conference and Exposition, Seoul, Republic of Korea, 13–17 June 2016. [Google Scholar]
- Lassalle, M.; Firrone, C.M. A parametric study of limit cycle oscillation of a bladed disk caused by flutter and friction at the blade root joints. J. Fluids Struct. 2018, 76, 349–366. [Google Scholar] [CrossRef]
- Zucca, S.; Firrone, C.M.; Gola, M.M. Numerical assessment of friction damping at turbine blade root joints by simultaneous calculation of the static and dynamic contact loads. Nonlinear Dyn. 2012, 67, 1934–1955. [Google Scholar] [CrossRef]
- Appaji Gowda, B.M.; Yeshovanth, H.R.; Siddaraju, C. Investigation and efficient modeling of an dovetail attachment in aero-engine. Procedia Mater. Sci. 2014, 5, 1873–1879. [Google Scholar] [CrossRef]
- Chen, J.J.; Zang, C.P.; Zhou, B.; Petrov, E.P. A study of friction microslip modeling for dynamic analysis of bladed discs with root joints. J. Mech. Eng. Sci. 2018, 233, 2599–2641. [Google Scholar] [CrossRef]
- Chen, J.J.; Zang, C.P.; Zhou, B.; Petrov, E.P. High-fidelity calculation of modal damping caused by friction at blade roots for single blades and tuned bladed disc assemblies. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2021, 235, 2810–2831. [Google Scholar] [CrossRef]
- Joannin, C.; Chouvion, B.; Thouverze, F.; Mbaye, M.; Ousty, J.P. Nonlinear modal analysis of mistuned periodic structures subjected to dry friction. J. Eng. Gas Turbines Power 2016, 138, 072504. [Google Scholar] [CrossRef]
- Schwarz, S.; Kohlmann, L.; Hartung, A.; Gross, J.; Scheel, M.; Krack, M. Validation of a turbine blade component test with frictional contacts by phase-locked-loop and force-controlled measurements. J. Eng. Gas Turbines Power 2020, 142, 051006. [Google Scholar] [CrossRef]
- Liu, T.Y.; Zhang, D.; Xie, Y.H. A nonlinear vibration analysis of forced response for a bladed-disk with dry friction dampers. J. Low Freq. Noise Vib. Act. Control 2019, 38, 1522–1539. [Google Scholar] [CrossRef]
- Canale, G.; Kinawy, M.; Maligno, A.; Sathujoda, P.; Citarella, R. Study of mixed-mode cracking of dovetail root of an aero-engine blade like structure. Appl. Sci. 2019, 9, 3825. [Google Scholar] [CrossRef]
- Yuan, J.; Schwingshackl, C.; Salles, L.; Wong, C.; Patsias, S. Reduced order method based on an adaptive formulation and its application to fan blade system with dovetail joints. In Proceedings of the ASME Turbo Expo 2020: Turbomachinery Technical Conference and Exposition, London, UK, 22–26 June 2020. [Google Scholar]
- Anandavel, K.; Prakash, R.V.; Davis, A. Effect of preloading on the contact stress distribution of a dovetail interface. World Acad. Sci. Eng. Technol. 2010, 4, 1107–1112. [Google Scholar]
- Fernandes, R.; El-Borgi, S.; Ahmed, K.; Friswell, M.I.; Jamia, N. Static fracture and modal analysis simulation of a gas turbine compressor blade and bladed disk system. Adv. Model. Simul. Eng. Sci. 2016, 3, 30. [Google Scholar] [CrossRef]
- Wei, D.S.; Ma, M.D.; Zhang, H.; Hu, C.; Wang, Y.R. Study of the variation of contact state near the contact boundary in a dovetail attachment under different loads. Eng. Fail. Anal. 2019, 105, 518–526. [Google Scholar] [CrossRef]






| Cases | Analysis Type | Gravity | Constant Parameters | Varying Parameters |
|---|---|---|---|---|
| 1 | Prestressed modal | No | n = 4000 rev/min, μ = 0.3, P0 = 0 MPa | js∈[1, 49], Δjs = 1; |
| 2 | js = 10, μ = 0.3, P0 = 0 MPa | n∈[1000, 20,000] rev/min, Δn = 1000 rev/min; | ||
| 3 | Transient | Yes | js = 10, P0 = 0.1 MPa, μ = 0.3, k = 10 | fe = k·fr, n∈[1000, 20,000] rev/min, Δn = 1000 rev/min; |
| 4 | js = 10, P0 = 0.1 MPa, k = 10 | fe = k·fr, n = 2000 rev/min, 4000 rev/min; μ∈[0.1, 0.5], Δμ = 0.02 | ||
| 5 | js = 10, P0 = 0.1 MPa, n = 2000 rev/min, μ = 0.3 | fe = k·fr, k∈[4, 44] rev/min, Δk = 2 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fan, X.; Liang, W.; Zeng, J.; Yang, Y.; Ma, H.; Fan, C.; Fu, S. Dynamic Characteristics of a Rotating Blade with a Dovetail Fixture. Machines 2023, 11, 643. https://doi.org/10.3390/machines11060643
Fan X, Liang W, Zeng J, Yang Y, Ma H, Fan C, Fu S. Dynamic Characteristics of a Rotating Blade with a Dovetail Fixture. Machines. 2023; 11(6):643. https://doi.org/10.3390/machines11060643
Chicago/Turabian StyleFan, Xiaoyu, Wenchao Liang, Jin Zeng, Yang Yang, Hui Ma, Chenguang Fan, and Shunguo Fu. 2023. "Dynamic Characteristics of a Rotating Blade with a Dovetail Fixture" Machines 11, no. 6: 643. https://doi.org/10.3390/machines11060643
APA StyleFan, X., Liang, W., Zeng, J., Yang, Y., Ma, H., Fan, C., & Fu, S. (2023). Dynamic Characteristics of a Rotating Blade with a Dovetail Fixture. Machines, 11(6), 643. https://doi.org/10.3390/machines11060643








