Mass Flow Function Correlation for Solid and Honeycomb Land Labyrinth Seals including Fin Front Angle, Clearance, Fin Number and Honeycomb Geometry
Abstract
:1. Introduction
2. Experimental Methods
3. Results and Discussions
3.1. Solid Land Labyrinth Seal
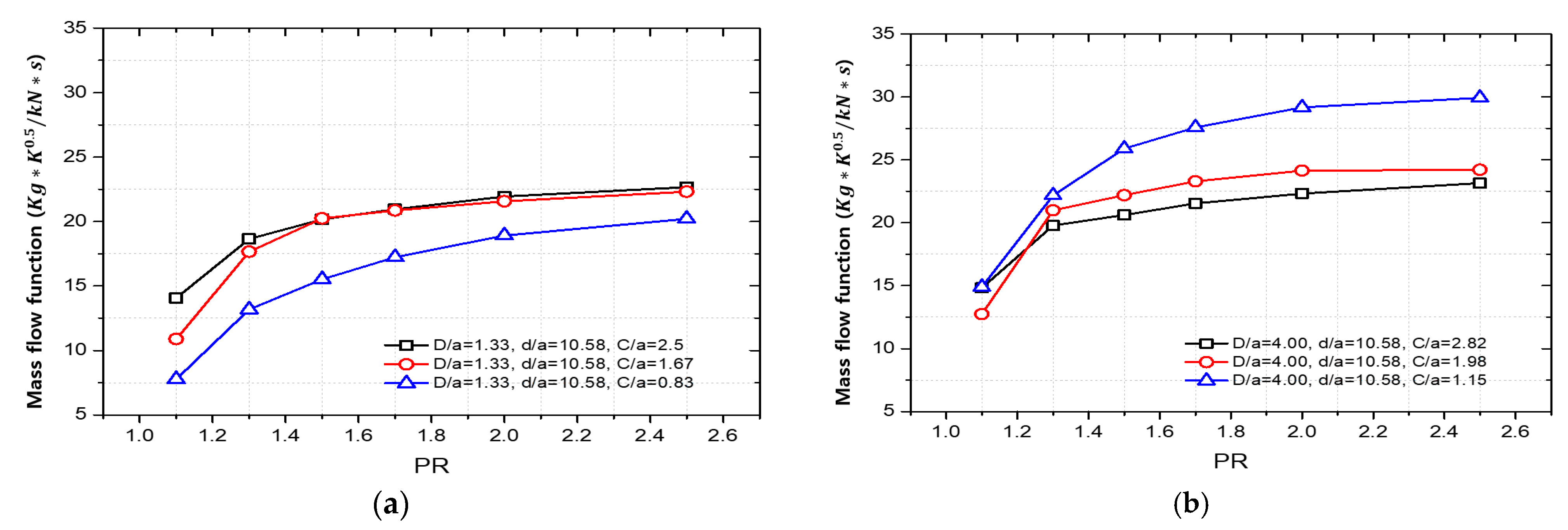
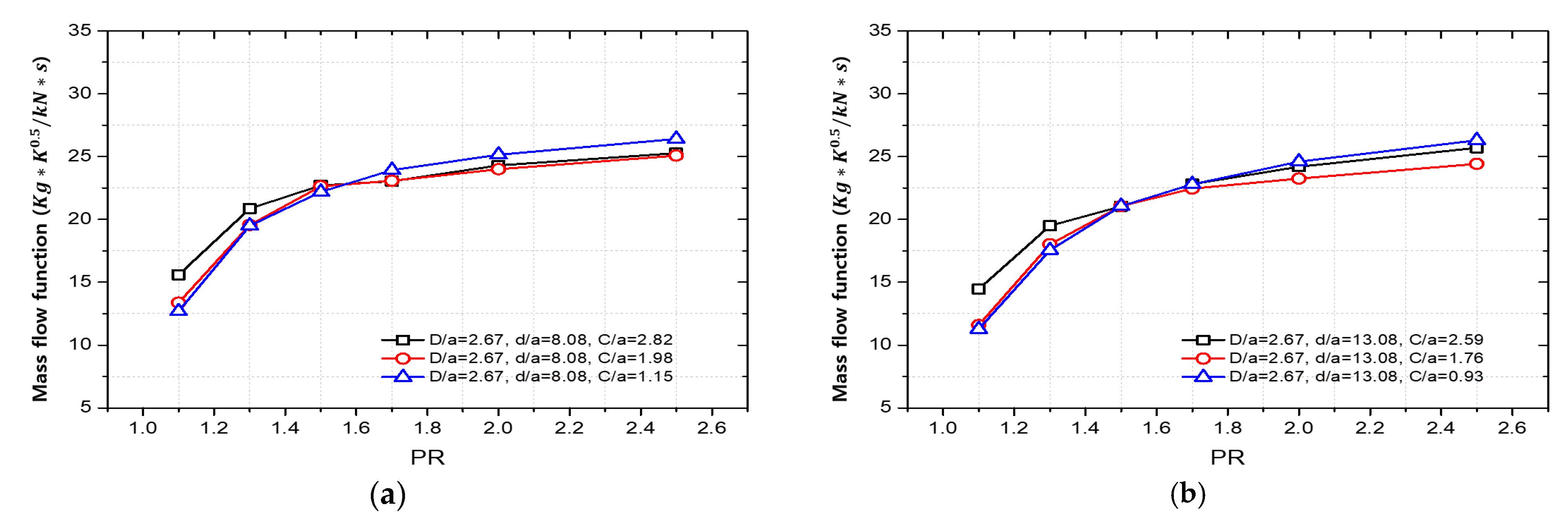
3.2. Honeycomb Land Labyrinth Seal
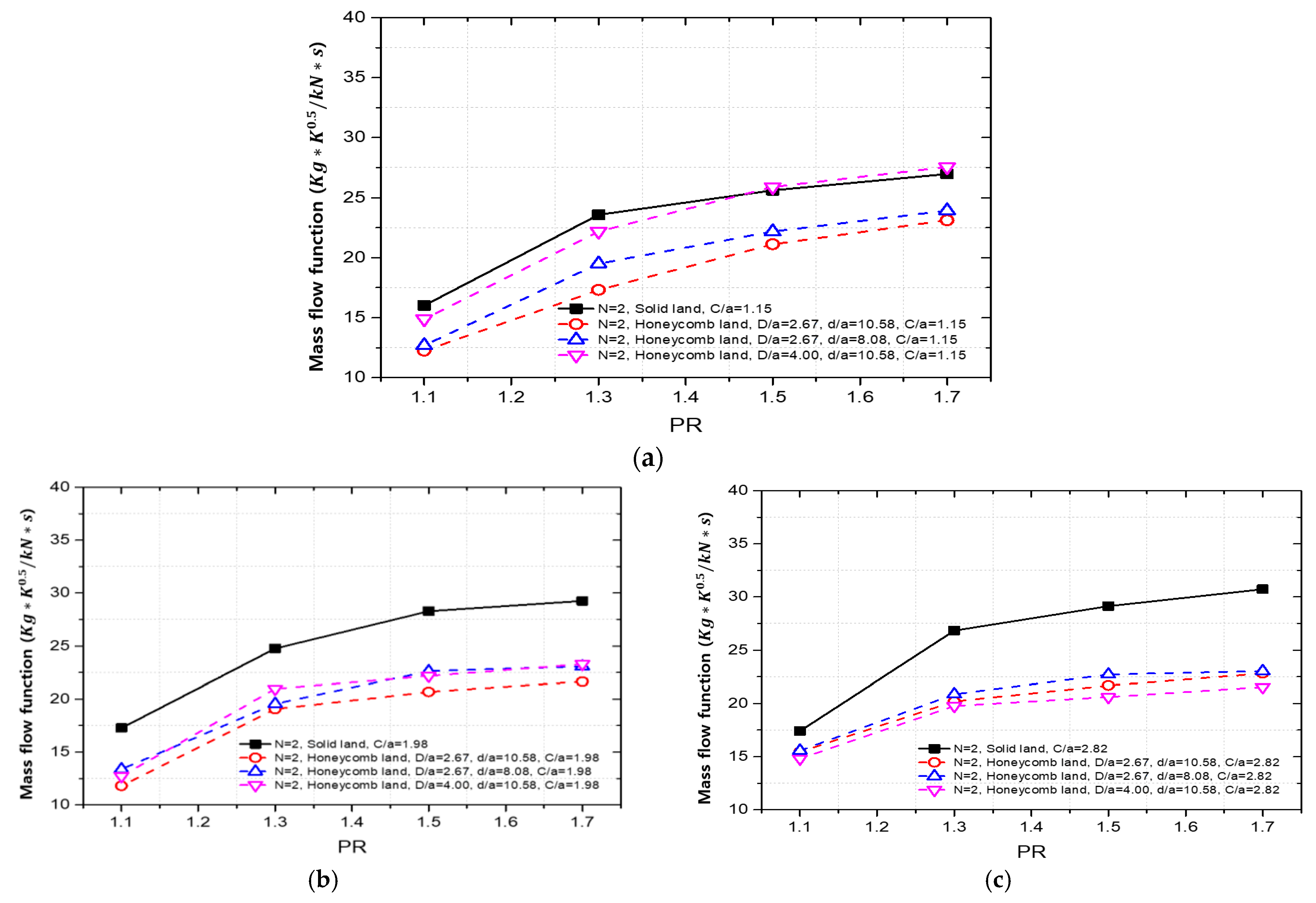
3.3. Comparison of Solid Land and Honeycomb Land Labyrinth Seal
4. Conclusions
- (1)
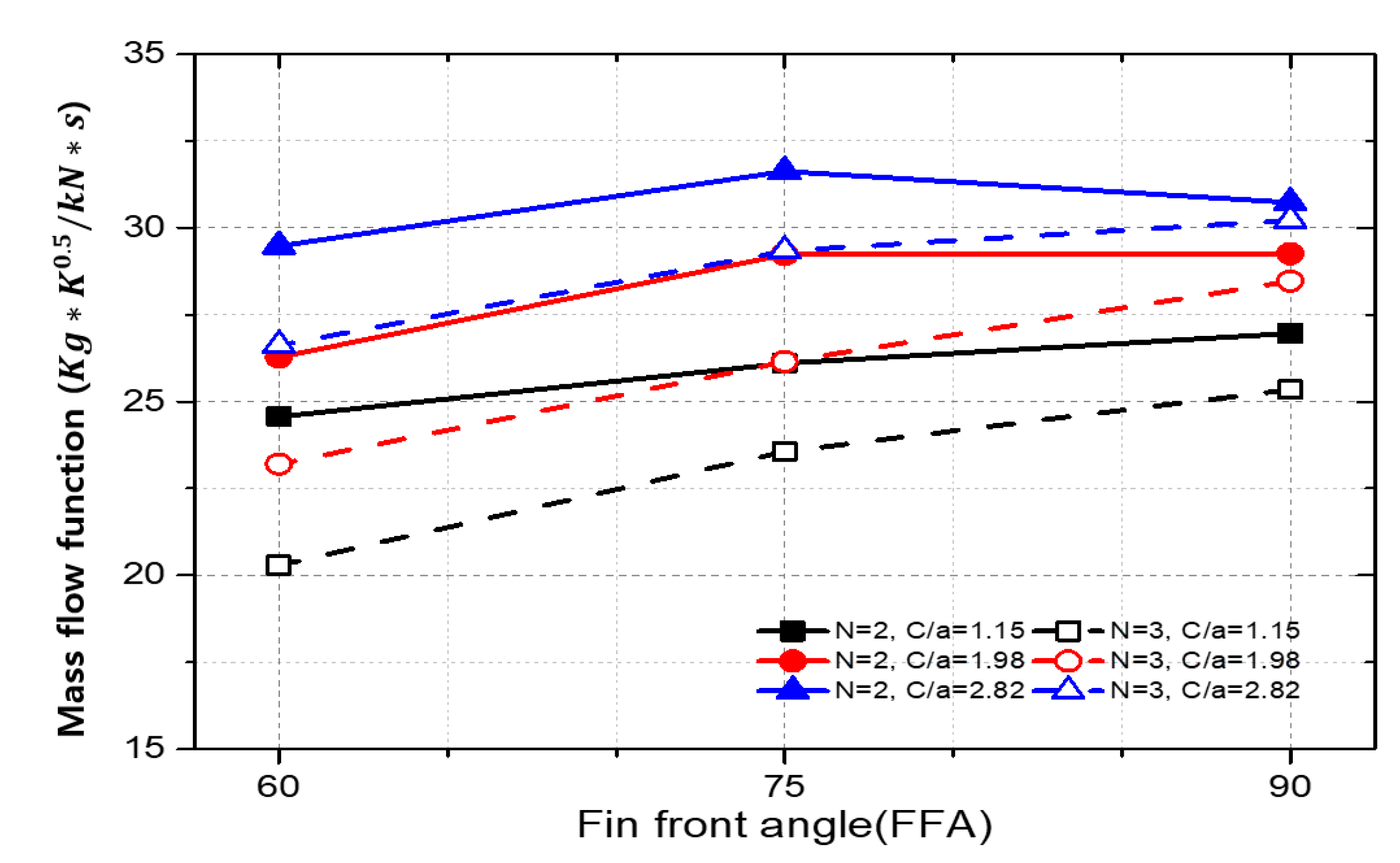
- Regarding the solid land labyrinth seal, the mass flow function exhibited its lowest value at the FFA (forward facing angle) of 60°. Additionally, the mass flow function demonstrated a decreasing trend as the clearance decreased.
- (2)
- In both solid and honeycomb lands, employing three fins resulted in a higher sealing performance compared to using two fins.
- (3)
- The effect of the honeycomb cell diameter on the flow rate function varied depending on the clearance, while the effect of cell depth on the flow rate function was relatively minor compared to the influence of cell diameter.
- (4)
- In general, the honeycomb land labyrinth seal exhibited lower mass flow function values compared to the solid land seal with the same number of fins, FFA, and clearance.
- (5)
- Based on the experimental results, correlation equations were derived for both solid and honeycomb lands. It is expected that these equations can be applied to various seal designs.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Clearance area | |
| Fin tip thickness | |
| Clearance | |
| Honeycomb cell diameter | |
| Honeycomb cell depth | |
| FFA | Fin front angle |
| Fin height | |
| Mass flow rate | |
| Number of fins | |
| Total pressure | |
| Pitch of fin | |
| PR | Pressure ratio |
| Total temperature | |
| Honeycomb cell thickness | |
| Fin front angle (degree) | |
| Mass flow function ( |
References
- Denton, J.D. The 1993 IGTI Scholar Lecture: Loss Mechanisms in Turbomachines. ASME J. Turbomach. 1993, 115, 621–656. [Google Scholar] [CrossRef]
- Chupp, R.E.; Hendricks, R.C.; Lattime, S.B.; Steinetz, B.M. Sealing in Turbomachinery, NASA Technical Report, NASA/TM-2006-214341; NASA: Cleveland, OH, USA, 2006. [Google Scholar] [CrossRef]
- Wadia, A.R.; Booth, T.C. Rotor-Tip Leakage: Part II—Design Optimization Through Viscous Analysis and Experiment. J. Eng. Power 1982, 104, 162–169. [Google Scholar] [CrossRef]
- Steinetz, B.M.; Hendricks, R.C.; Braun, M.J. Turbomachine Sealing and Secondary Flows, Part 1—Review of Sealing Performance, Customer, Engine Designer, and Research Issues, NASA Technical Report, NASA/TM-2004-211991/Part1; NASA: Cleveland, OH, USA, 2004. [Google Scholar]
- Stocker, H.L. Determining and improving labyrinth seal performance in current and advanced high performance gas turbines. AGARD Seal Technol. Gas Turbine Eng. 1978, 237, 13.1–13.22. [Google Scholar]
- Zimmermann, H.; Wolff, K.H. Air System Correlations: Part 1—Labyrinth seals. In ASME International Gas Turbine and Aeroengine Congress and Exhibition, stockholm, sweden, Volume 4: Heat Transfer; Electric Power; American Society of Mechanical Engineers: Atlanta, GA, USA, 1998; V004T09A048. [Google Scholar]
- Vermes, G. A fluid mechanics approach to the labyrinth seal leakage problem. ASME J. Eng. Power 1960, 83, 161–169. [Google Scholar] [CrossRef]
- Kim, T.S.; Kang, Y.; Moon, H.K. Aerodynamic Performance of Double-Sided Labyrinth Seal, Fluid Machinery and Fluid Me-Chanics: 4th International Symposium; Springer: Berlin/Heidelberg, Germany, 2009; pp. 377–382. [Google Scholar]
- Micio, M.; Facchini, B.; Innocenti, L.; Simonetti, F. Experimental Investigation on Leakage Loss and Heat Transfer in a Straight through labyrinth seal. In Proceedings of the ASME Turbo Expo: Turbine Technical Conference and Exposition, Vancouver, LM, Canada, 6–10 June 2011; Volume 54655, pp. 967–979. [Google Scholar] [CrossRef]
- Michaud, M.; Vakili, A.; Meganathan, A.; Zielke, R.; Shuster, L.; Terrell, J. An experimental study of labyrinth seal flow. In Proceedings of the International Joint Power Generation Conference collocated with Turbo Expo 2003, Atlanta, GA, USA, 16–19 June 2003; Volume 36924, pp. 497–504. [Google Scholar]
- Vakili, A.D.; Meganathan, A.J.; Michaud, M.; Radhakrishnan, S. An experimental and numerical study of labyrinth seal flow. In ASME Turbo Expo 2005: Power for Land, Sea, and Air Volume 3: Turbo Expo 2005, Parts A and B; ASME: New York, NY, USA, 2005; pp. 1121–1128. [Google Scholar]
- Kang, Y.; Kim, T.S.; Kang, S.Y.; Moon, H.K. Aerodynamic performance of stepped labyrinth seals for gas turbine applications. In ASME Turbo Expo 2005: Power for Land, Sea, and Air Volume 4: Heat Transfer, Parts A and B; ASME: New York, NY, USA, 2010; pp. 1191–1199. [Google Scholar]
- Li, J.; Yan, X.; Li, G.; Feng, Z. Effects of pressure ratio and sealing clearance on leakage flow characteristics in the rotating honeycomb labyrinth seal. In ASME Turbo Expo: Power for Land, Sea, and Air, Montreal, Canada Volume 4: Turbo Expo 2007, Parts A and B; ASME: New York, NY, USA, 2007; pp. 1199–1206. [Google Scholar] [CrossRef]
- Bunker, R.S. Axial Turbine Blade Tips: Function, Design, and Durability. J. Propuls. Power 2006, 22, 271–285. [Google Scholar] [CrossRef]
- Kaczyński, P.; Szwaba, R.; Wasilczuk, F.; Flaszyński, P.; Doerffer, P. Leakage flow reduction in different configuration of labyrinth seal on a turbine blade tip. J. Phys. Conf. Ser. 2018, 1101, 012012. [Google Scholar] [CrossRef]
- Frączek, D.; Bochon, K.; Wróblewski, W. Influence of Honeycomb Land Geometry on Seal Performance. In Proceedings of the ASME Turbo Expo 2016: Turbomachinery Technical Conference and Exposition, Seoul, South Korea, 13–17 June 2016; Volume 5A: Heat Transfer. [Google Scholar]
- Yan, X.; Chen, X.; He, K. Influence of Shroud Seal Dimensions on Aerodynamic Performance of Steam Turbine Stages: Part I—Honeycomb Seal. In Proceedings of the ASME Turbo Expo 2016: Turbomachinery Technical Conference and Exposition, Seoul, South Korea, 13–17 June 2016; Volume 8: Microturbines, Turbochargers and Small Turbomachines, Steam Turbines. [Google Scholar]
- Desando, A.; Rapisarda, A.; Campagnoli, E.; Taurino, R. Numerical Analysis of Honeycomb Labyrinth Seals: Cell Geometry and Fin Tip Thickness Impact on the Discharge Coefficient. In Proceedings of the ASME Turbo Expo 2015: Turbine Technical Conference and Exposition, Montreal, QC, Canada, 15–19 June 2015; Volume 5C: Heat Transfer. [Google Scholar]
- Kuwamura, Y.; Matsumoto, K.; Uehara, H.; Ooyama, H.; Tanaka, Y.; Nishimoto, S. Development of New High-Performance Labyrinth seal Using Aerodynamic Approach. In Proceedings of the ASME Turbo Expo: Turbine Technical Conference and Exposition, San Antonio, TX, USA, 3–7 June 2013; Volume 5B: Oil and Gas Applications, Stream Turbines. [Google Scholar]
- Lee, S.I.; Kang, Y.J.; Kim, W.J.; Kwak, J.S. Effects of tip clearance, number of teeth, and tooth front angle on the sealing performance of straight and stepped labyrinth seals. J. Mech. Sci. Technol. 2021, 35, 1539–1547. [Google Scholar] [CrossRef]
- Li, J.; Kong, S.; Yan, X.; Obi, S.; Feng, Z. Numerical Investigations on Leakage Performance of the Rotating Labyrinth Honeycomb Seal. ASME J. Eng. Gas Turbines Power 2010, 132, 062501. [Google Scholar] [CrossRef]
- Zhang, L.; Zhu, H.; Liu, C.; Tong, F. Experimental and Numerical Investigation on Leakage Characteristic of Stepped Labyrinth Seal. In Proceedings of the ASME Turbo Expo 2016: Turbomachinery Technical Conference and Exposition, Seoul, South Korea, 13–17 June 2016; Volume 5A: Heat Transfer. [Google Scholar]
- Chun, Y.H.; Ahn, J. Optimizing the Geometric Parameters of a Stepped Labyrinth Seal to Minimize the Discharge Coefficient. Processes 2022, 10, 2019. [Google Scholar] [CrossRef]
- Hu, B.; Yao, J.; An, N.; Mi, H.; Bai, X. Numerical investigation on the effects of HC geometry on the flow and heat transfer in the disk cavity system at the turbine vane root. Appl. Therm. Eng. 2023, 230, 120794. [Google Scholar] [CrossRef]
- Kim, T.-S.; Kang, S.-Y. Investigation of Leakage Characteristics of Straight and Stepped Labyrinth Seals. Int. J. Fluid Mach. Syst. 2010, 3, 253–259. [Google Scholar] [CrossRef]
- Kline, S.J. The Purposes of Uncertainty Analysis. ASME J. Fluids Eng. 1985, 107, 153–160. [Google Scholar] [CrossRef]
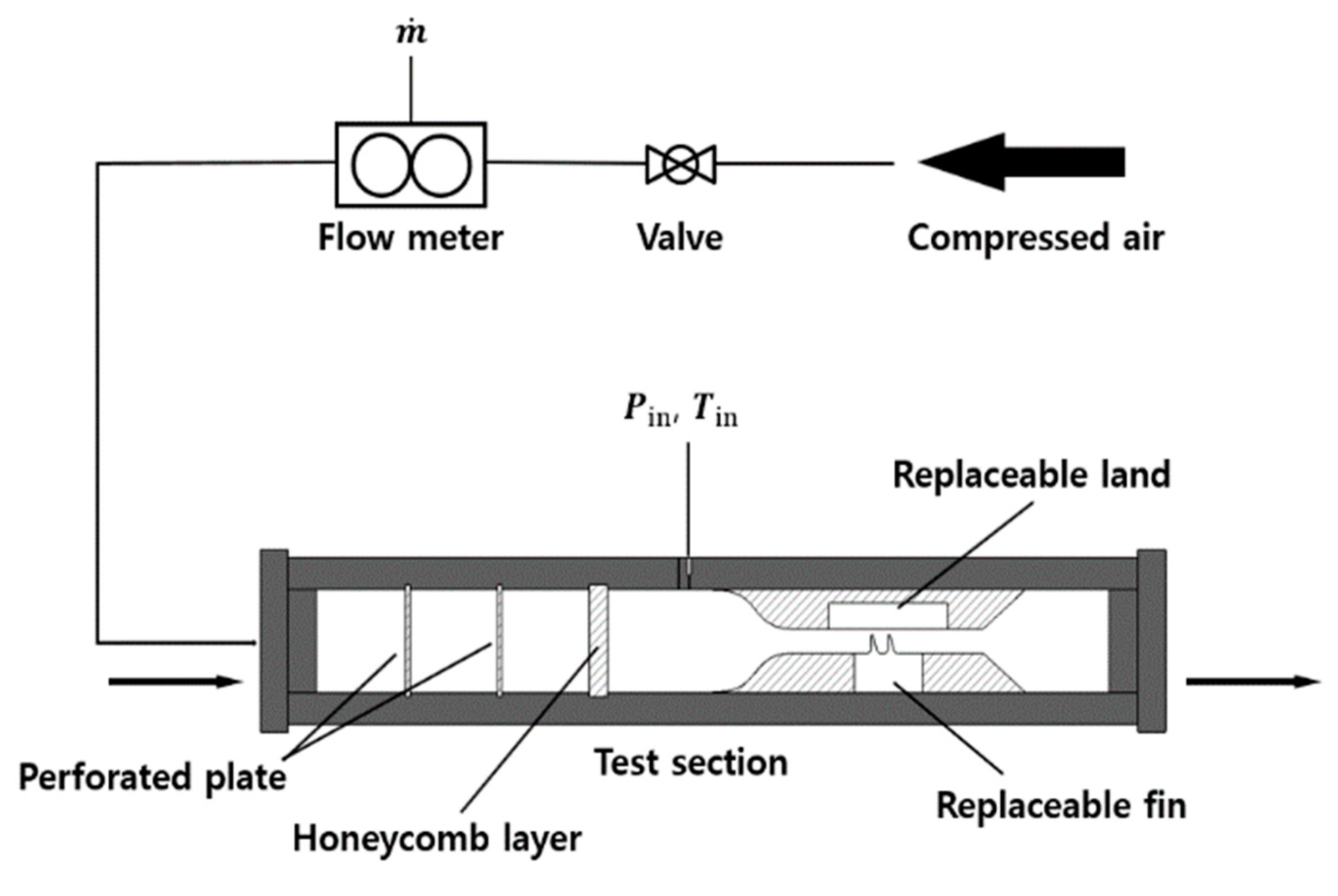
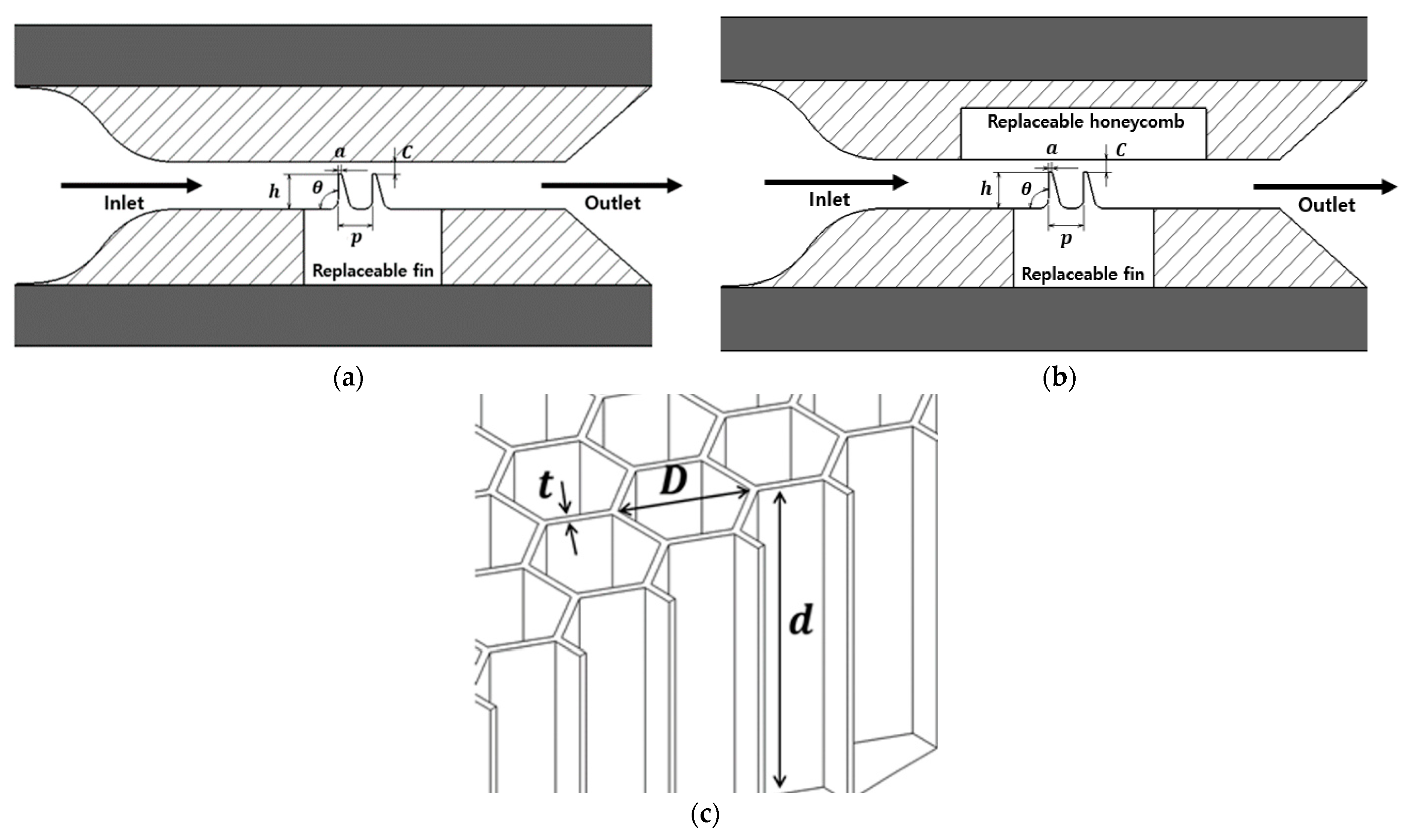
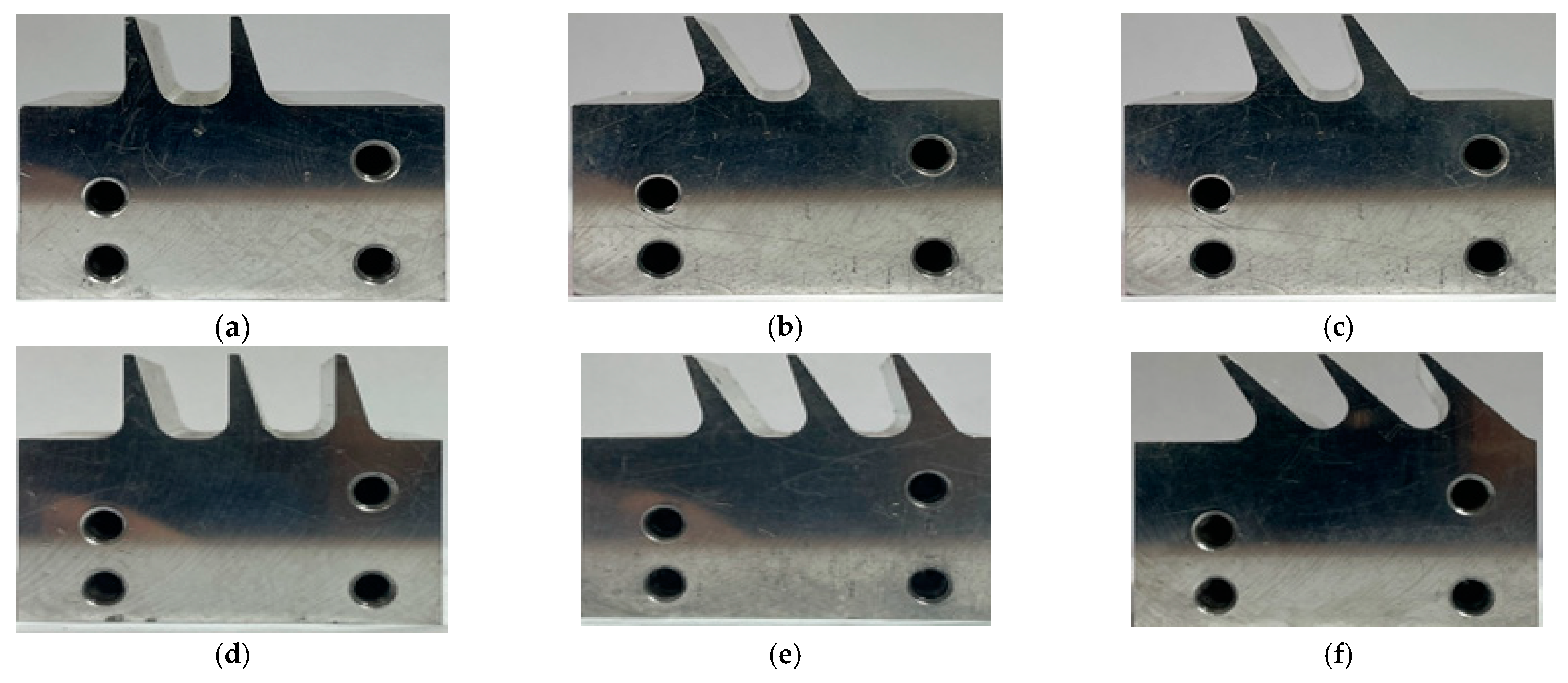



| Seal Type | Solid Land | Honeycomb Land |
|---|---|---|
| FFA (θ) | 60°, 75°, 90° | |
| Number of fin (N) | 2, 3 | |
| Fin tip thickness (a) | 1.2 mm | |
| Pitch (p/a) | 10 | |
| Clearance (C/a) | 1.16~2.82 | 1.15~3.65 |
| Honeycomb cell thickness (t/a) | - | 0.25 |
| Honeycomb cell diameter (D/a) | - | 1.33~4.00 |
| Honeycomb cell depth (d/a) | - | 8.08~13.08 |
| Parameters | Constraints |
|---|---|
| FFA (θ) | 90~60° |
| Clearance (C/a) | 1.1~2.82 |
| Number of fin (N) | 2, 3 |
| PR | 1.1~1.7 |
| 0.9822 | |
| i | 0.1915 |
| j | 22.5635 |
| k | 0.2250 |
| l | −0.2230 |
| m | 0.4594 |
| Parameters | Constraints |
|---|---|
| FFA (θ) | 90~60° |
| Clearance (C/a) | 0.83~1.98 |
| Number of fin (N) | 2, 3 |
| Cell diameter (D/a) | 1.33~2.67 |
| Cell depth (d/a) | 8.08~13.08 |
| PR | 1.1~1.7 |
| 0.9621 | |
| i | 0.1019 |
| j | 34.1676 |
| k | 0.0465 |
| l | 0.1790 |
| m | −0.2072 |
| n | 0.4824 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, H.J.; Kang, Y.J.; Kim, W.; Jo, Y.R.; Park, S.; Kwak, J.S. Mass Flow Function Correlation for Solid and Honeycomb Land Labyrinth Seals including Fin Front Angle, Clearance, Fin Number and Honeycomb Geometry. Machines 2023, 11, 880. https://doi.org/10.3390/machines11090880
Kim HJ, Kang YJ, Kim W, Jo YR, Park S, Kwak JS. Mass Flow Function Correlation for Solid and Honeycomb Land Labyrinth Seals including Fin Front Angle, Clearance, Fin Number and Honeycomb Geometry. Machines. 2023; 11(9):880. https://doi.org/10.3390/machines11090880
Chicago/Turabian StyleKim, Hyeok Je, Young Jun Kang, Woojun Kim, Ye Rim Jo, Suhyeon Park, and Jae Su Kwak. 2023. "Mass Flow Function Correlation for Solid and Honeycomb Land Labyrinth Seals including Fin Front Angle, Clearance, Fin Number and Honeycomb Geometry" Machines 11, no. 9: 880. https://doi.org/10.3390/machines11090880
APA StyleKim, H. J., Kang, Y. J., Kim, W., Jo, Y. R., Park, S., & Kwak, J. S. (2023). Mass Flow Function Correlation for Solid and Honeycomb Land Labyrinth Seals including Fin Front Angle, Clearance, Fin Number and Honeycomb Geometry. Machines, 11(9), 880. https://doi.org/10.3390/machines11090880







