Investigation of the Effect of Pumping Depth and Frequency of Flapping Hydrofoil on Suspended Matter Discharge Characteristics
Abstract
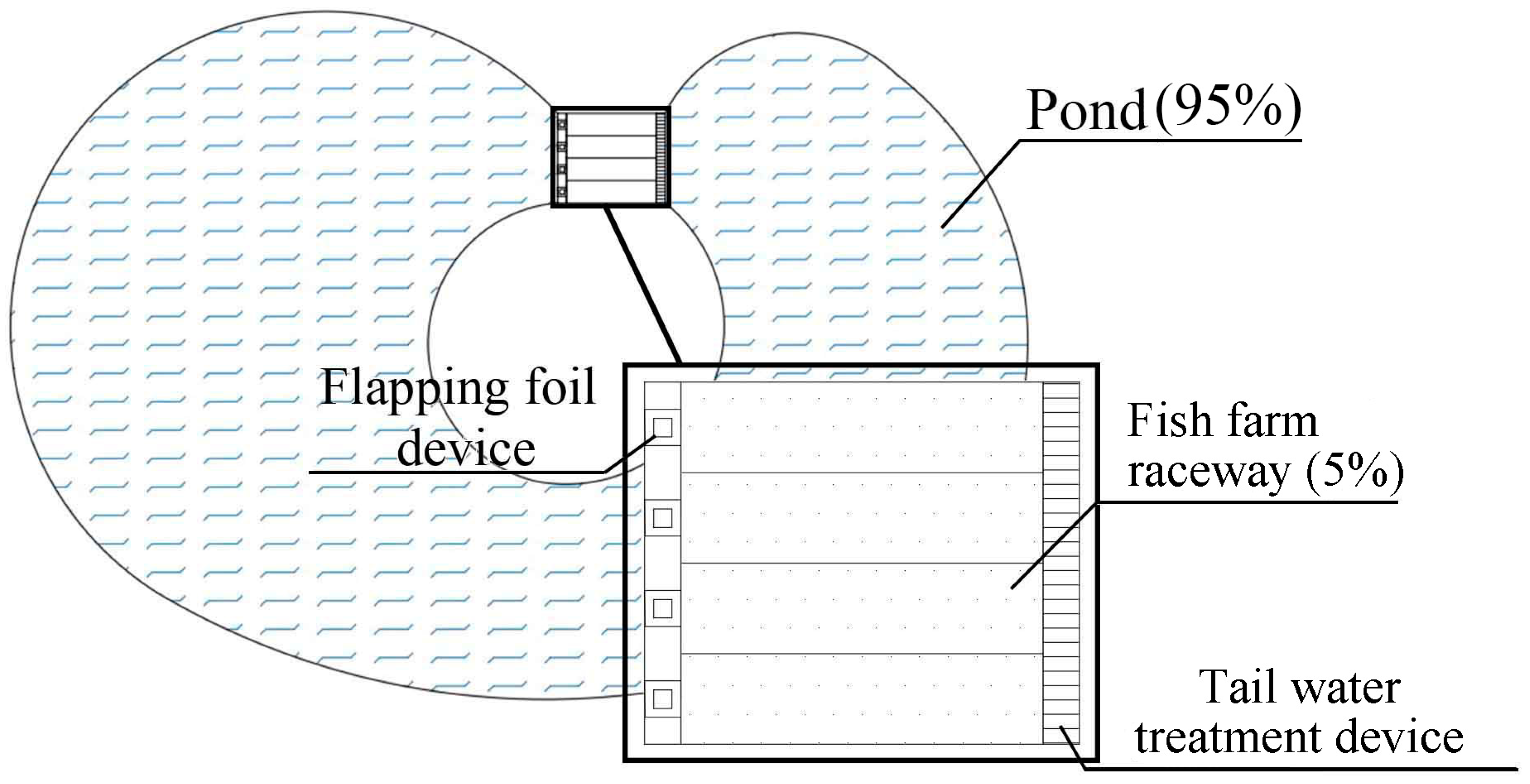
:1. Introduction
2. Modelling
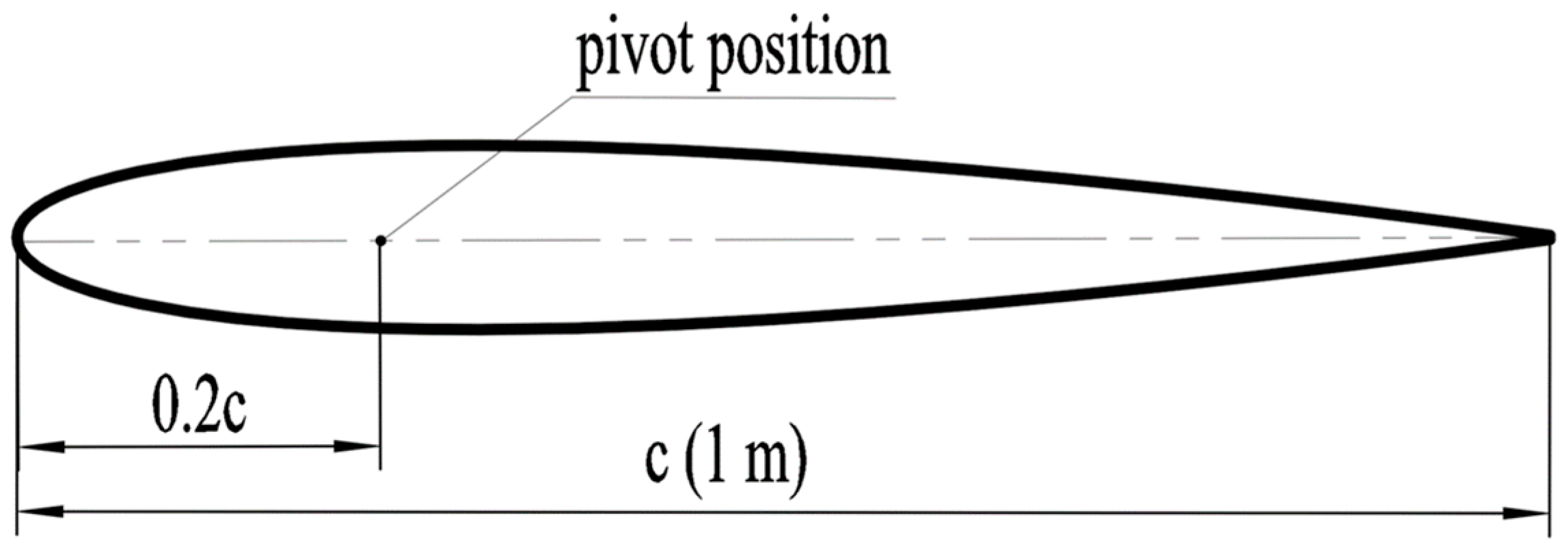
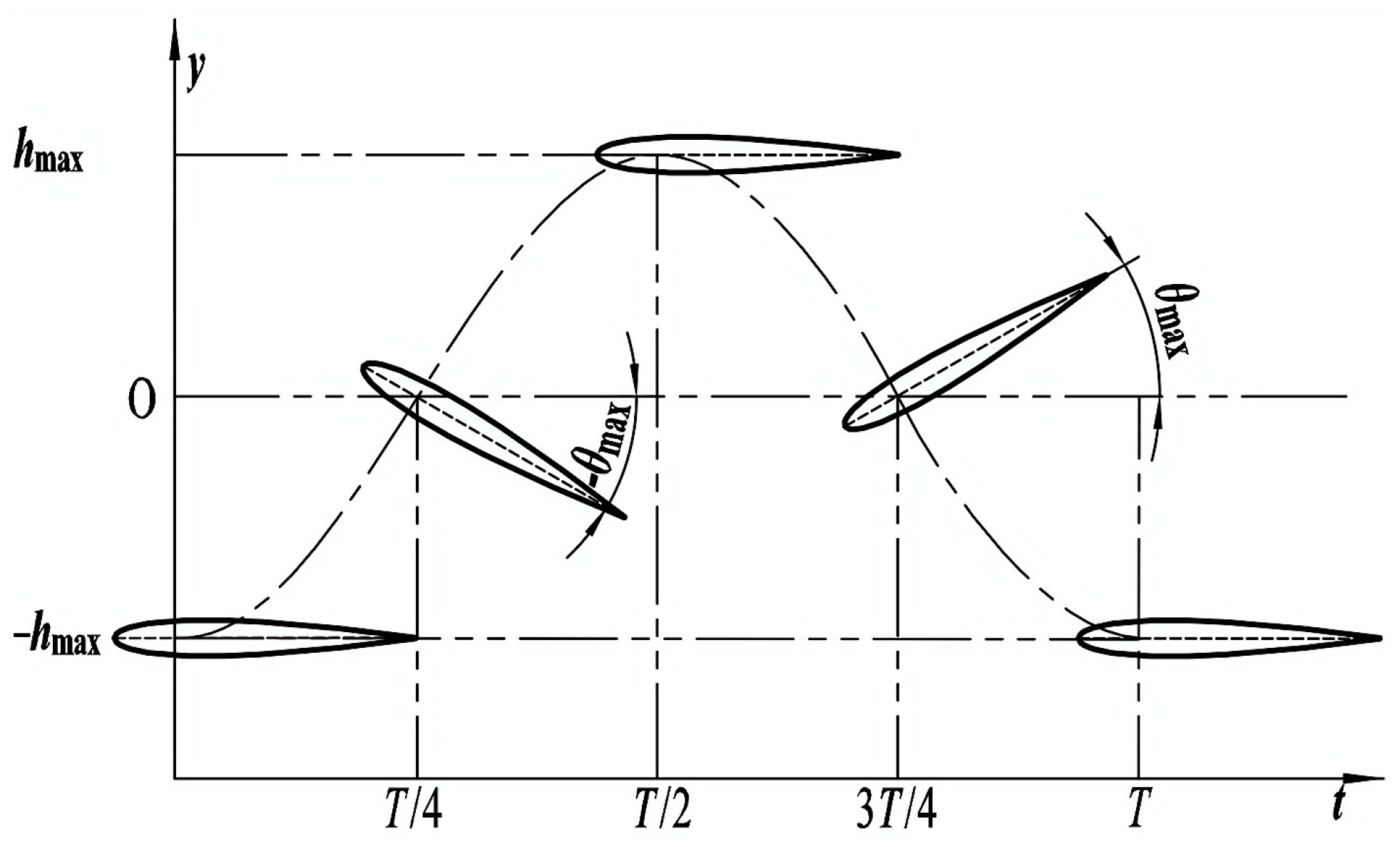
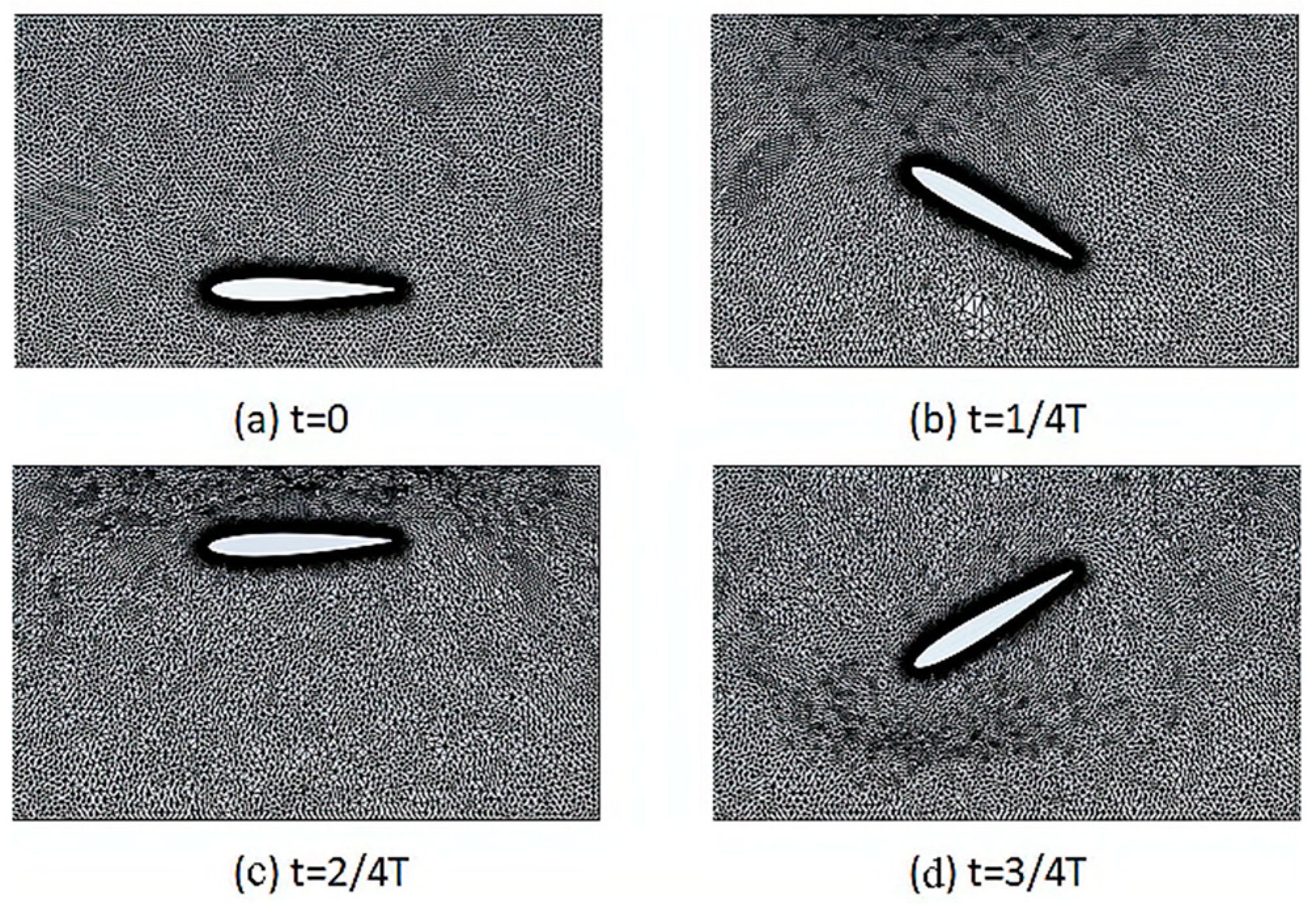
2.1. Description of Flapping Hydrofoil Motion
2.2. Particle Tracking Models and Discrete Phase Parameters
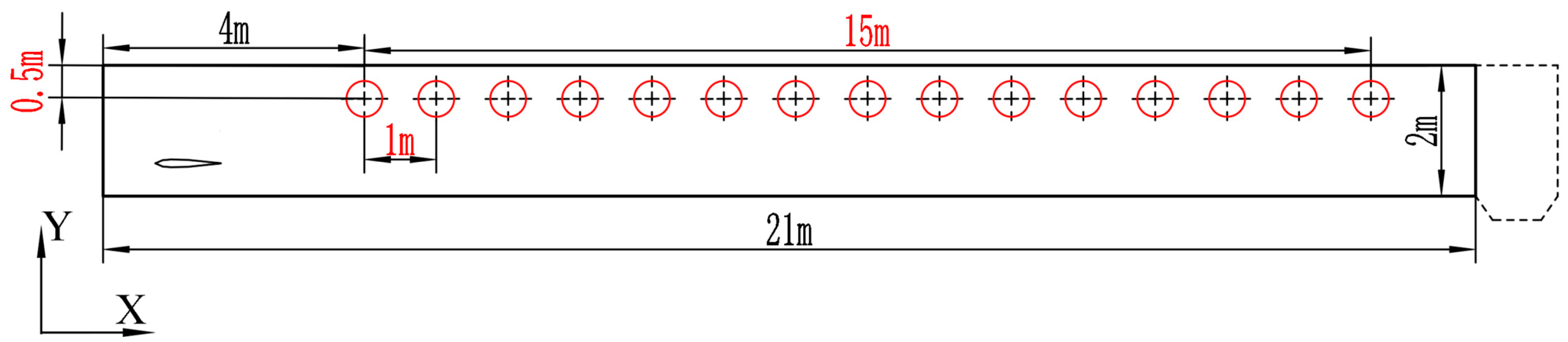
2.3. Evaluation of Indicators and Particle Placement Strategy
3. Numerical Method and Validation
3.1. Control Equations and Turbulence Modelling
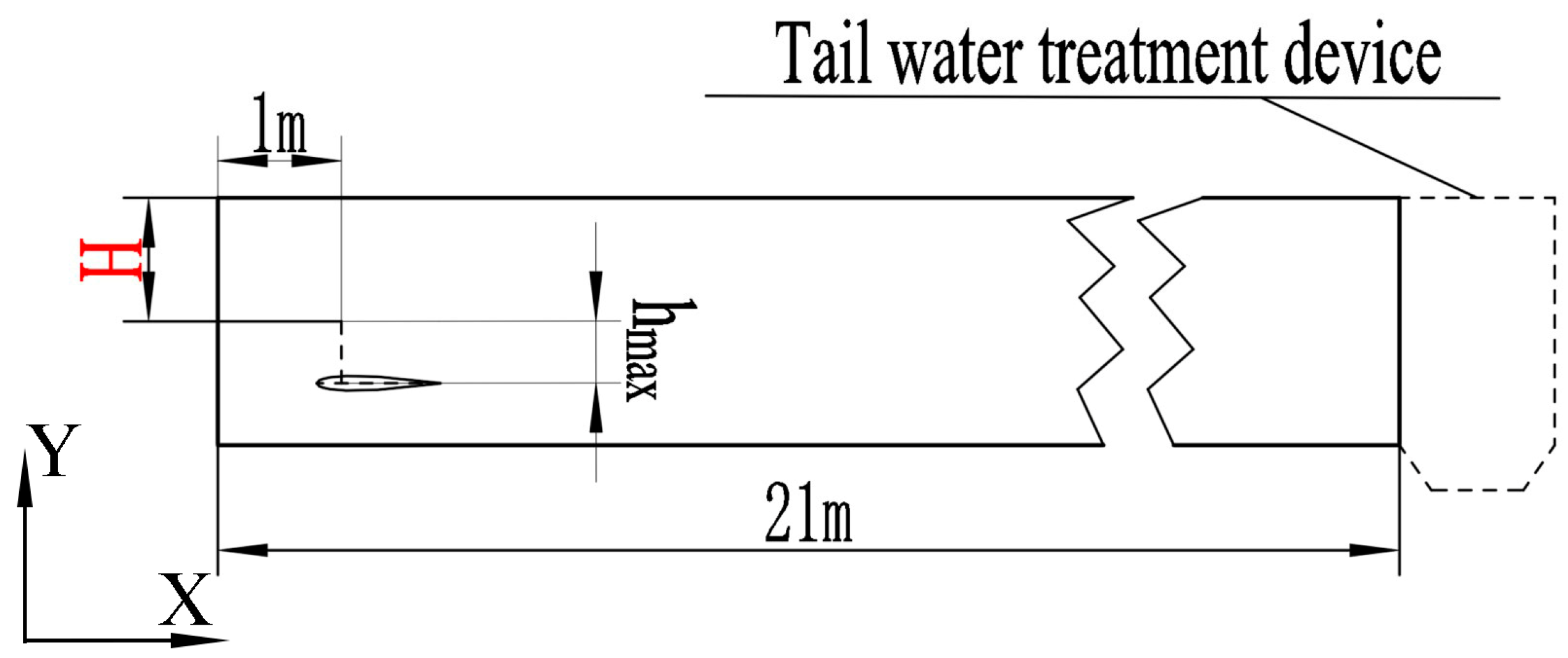
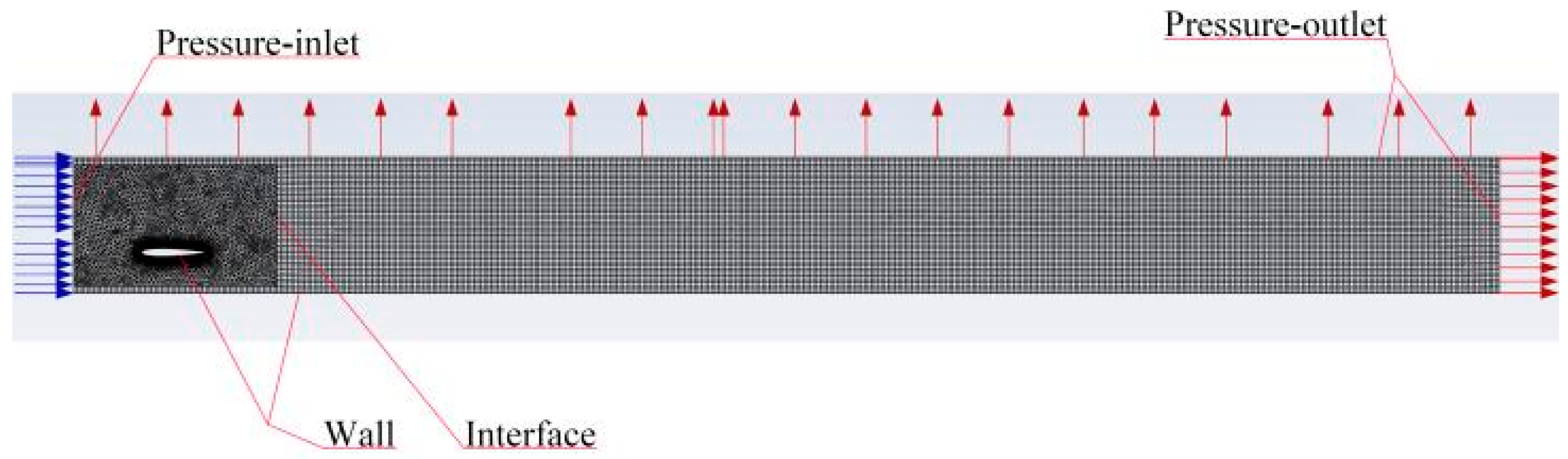
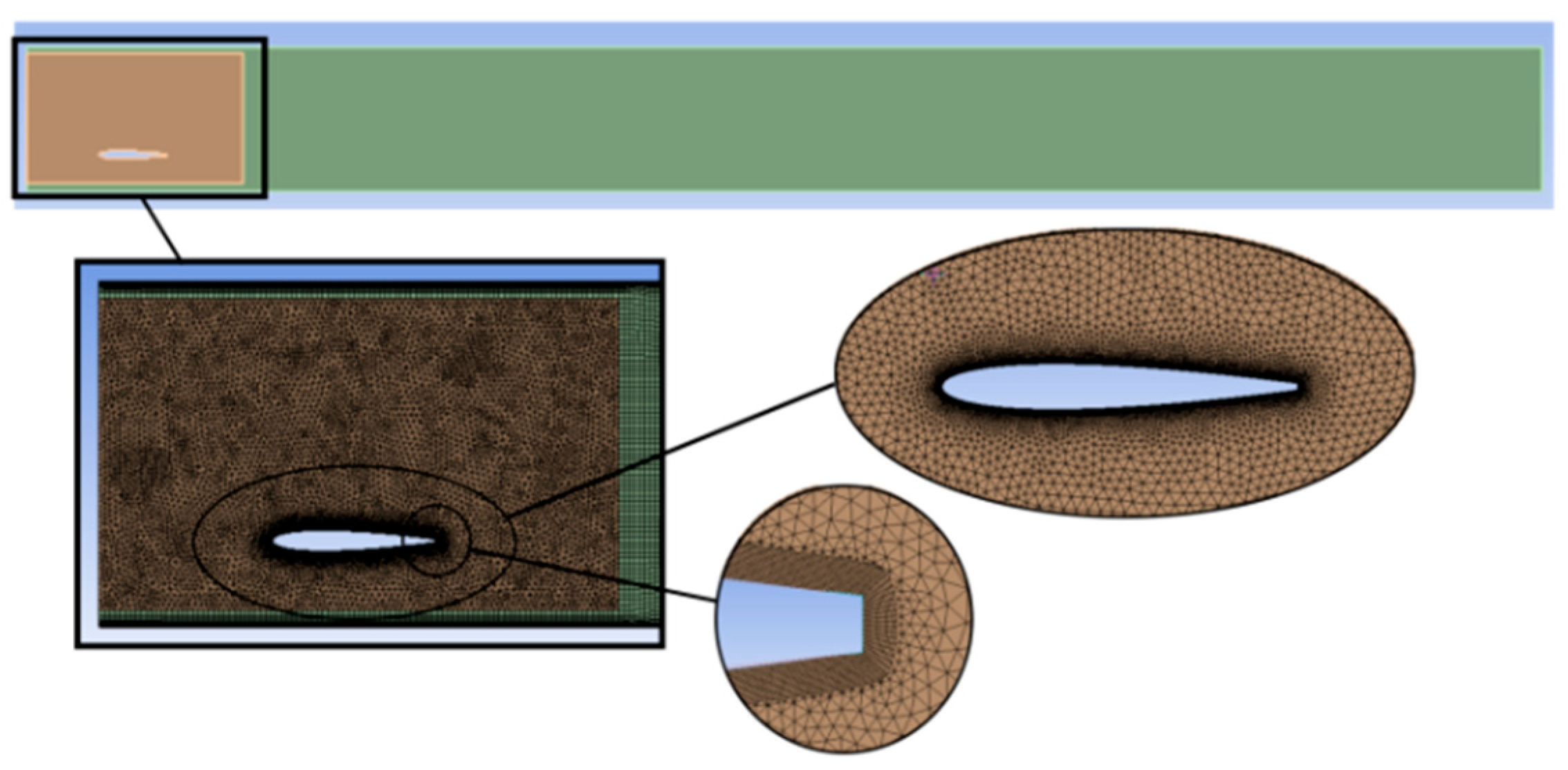
3.2. Computational Domain and Meshing
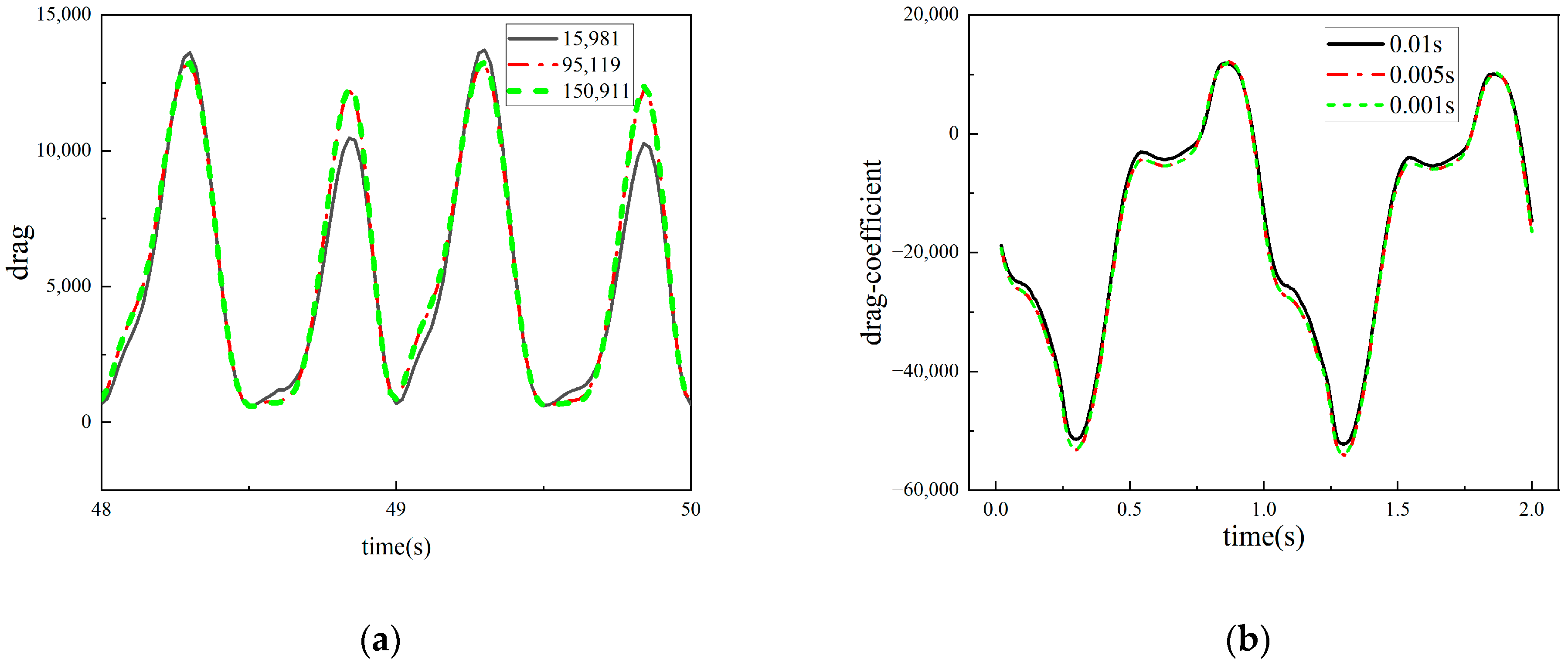
3.3. Grid-Independent Verification
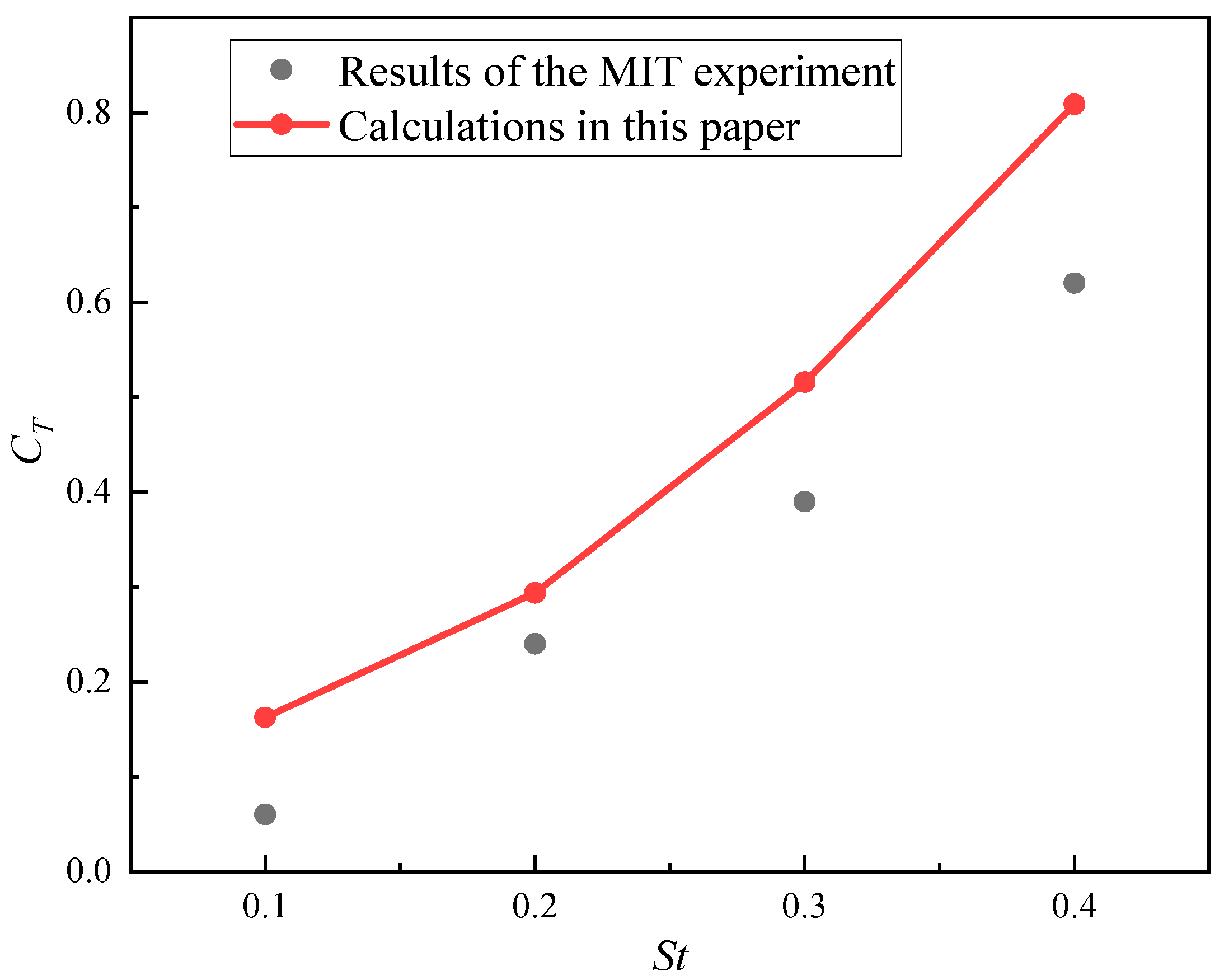
3.4. Validation
4. Results & Discussion
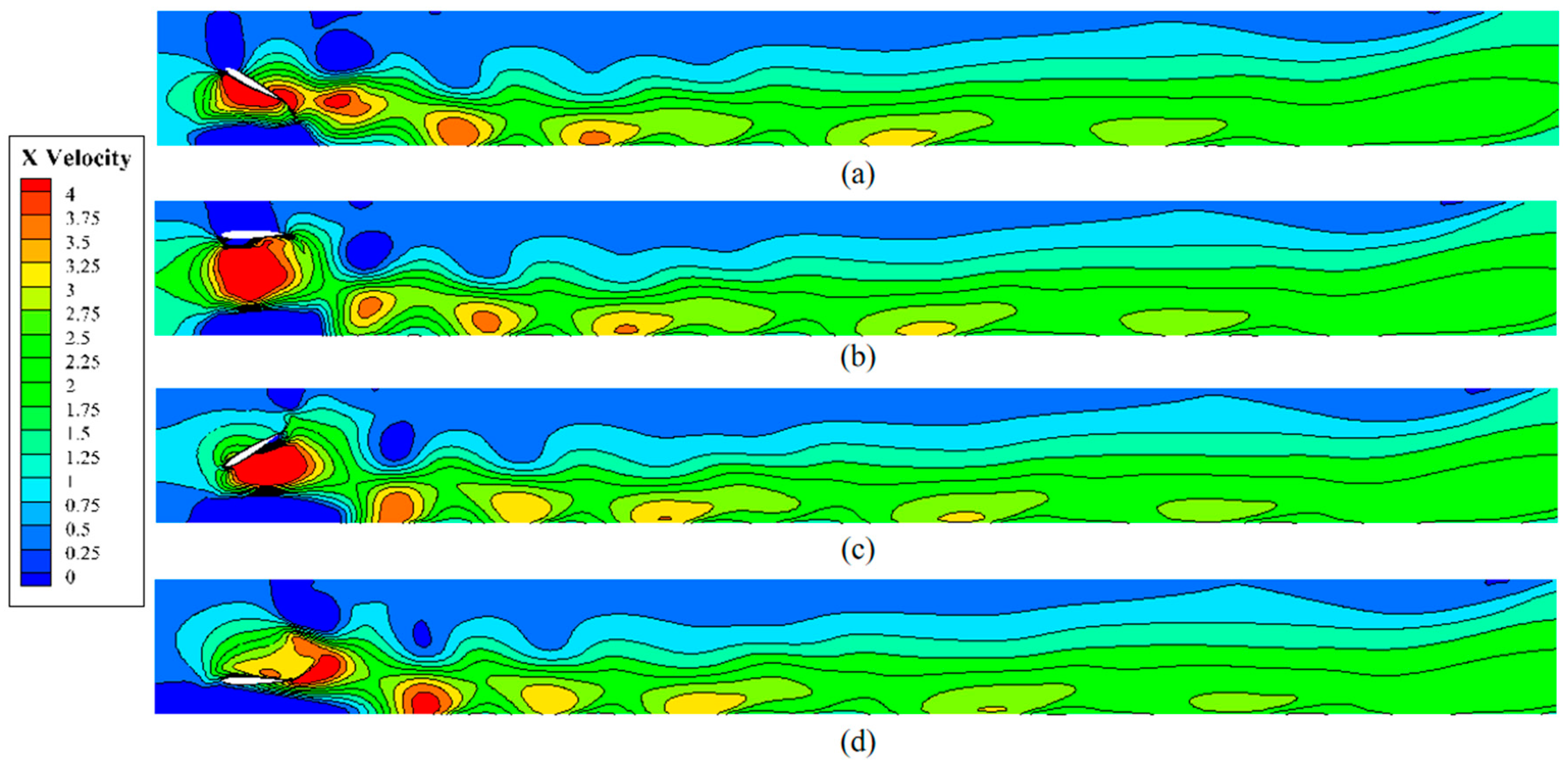
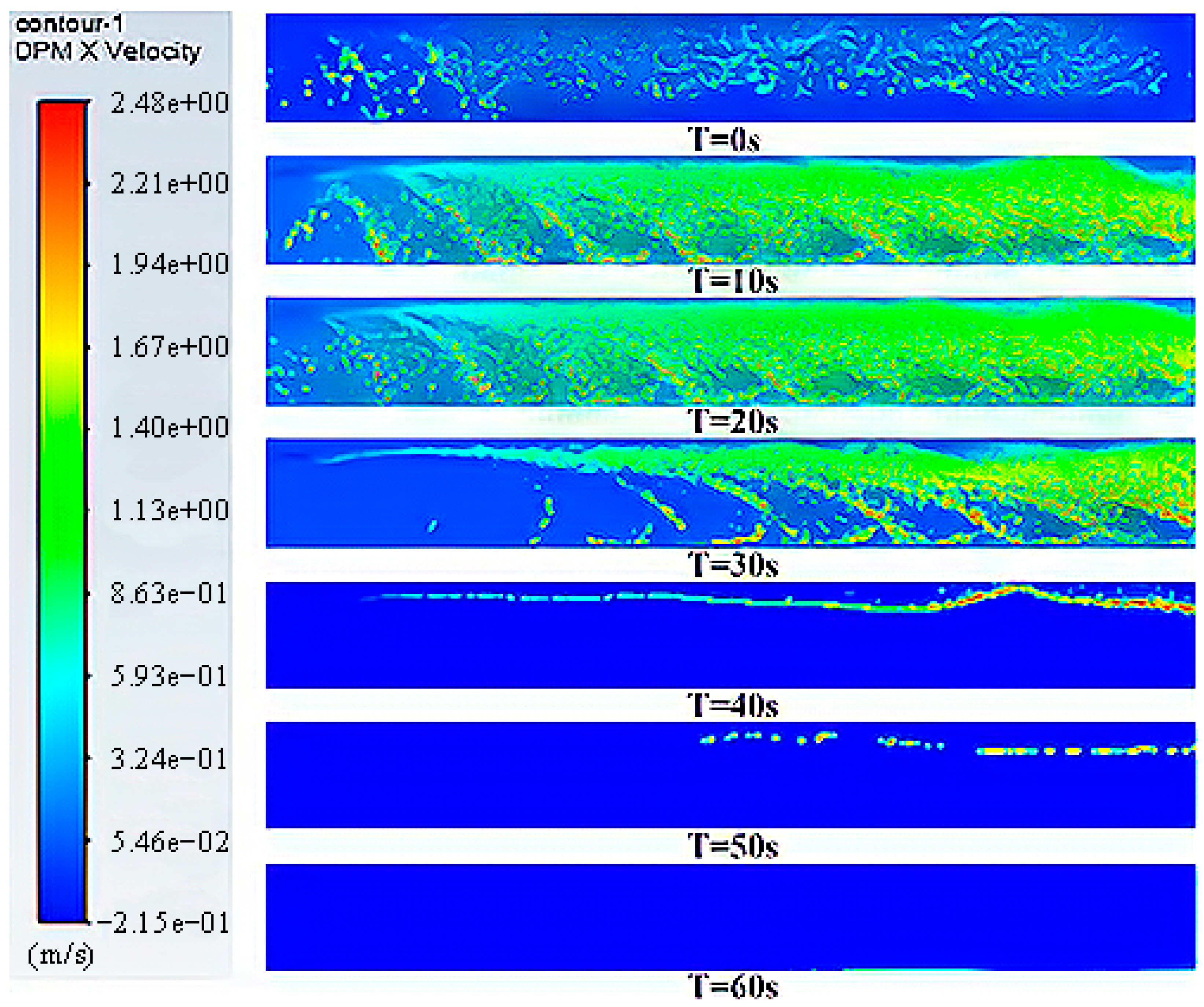
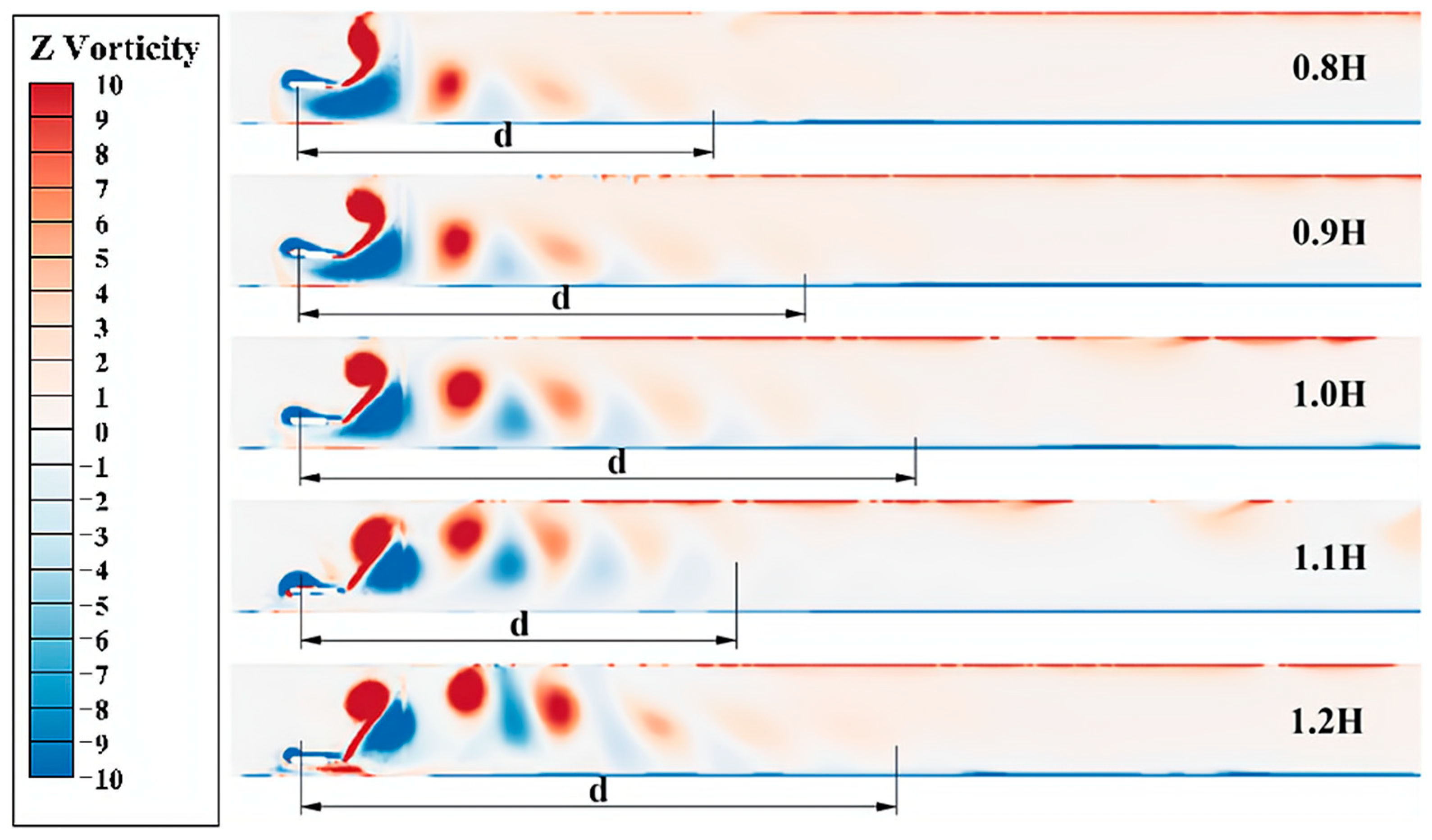
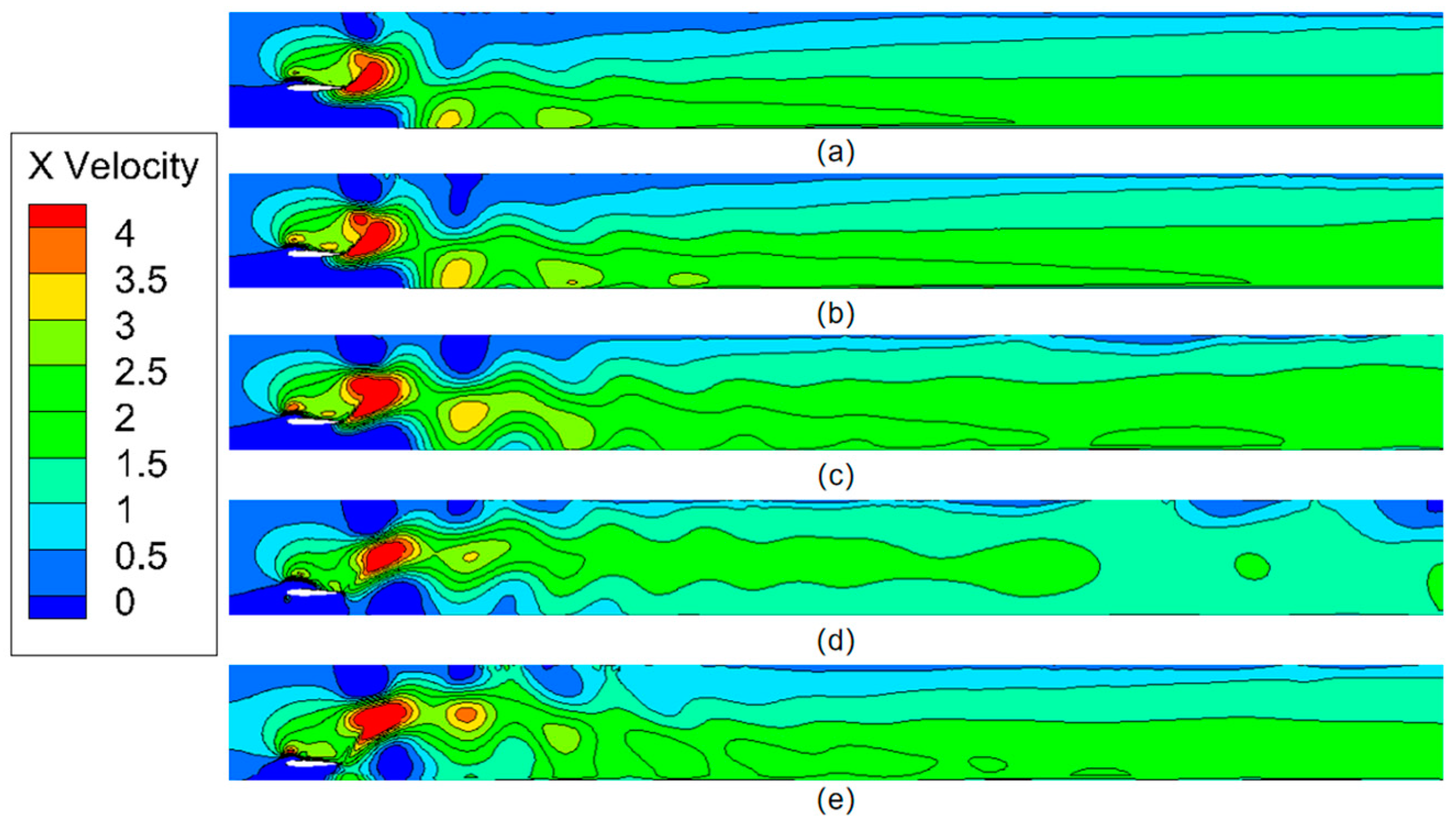
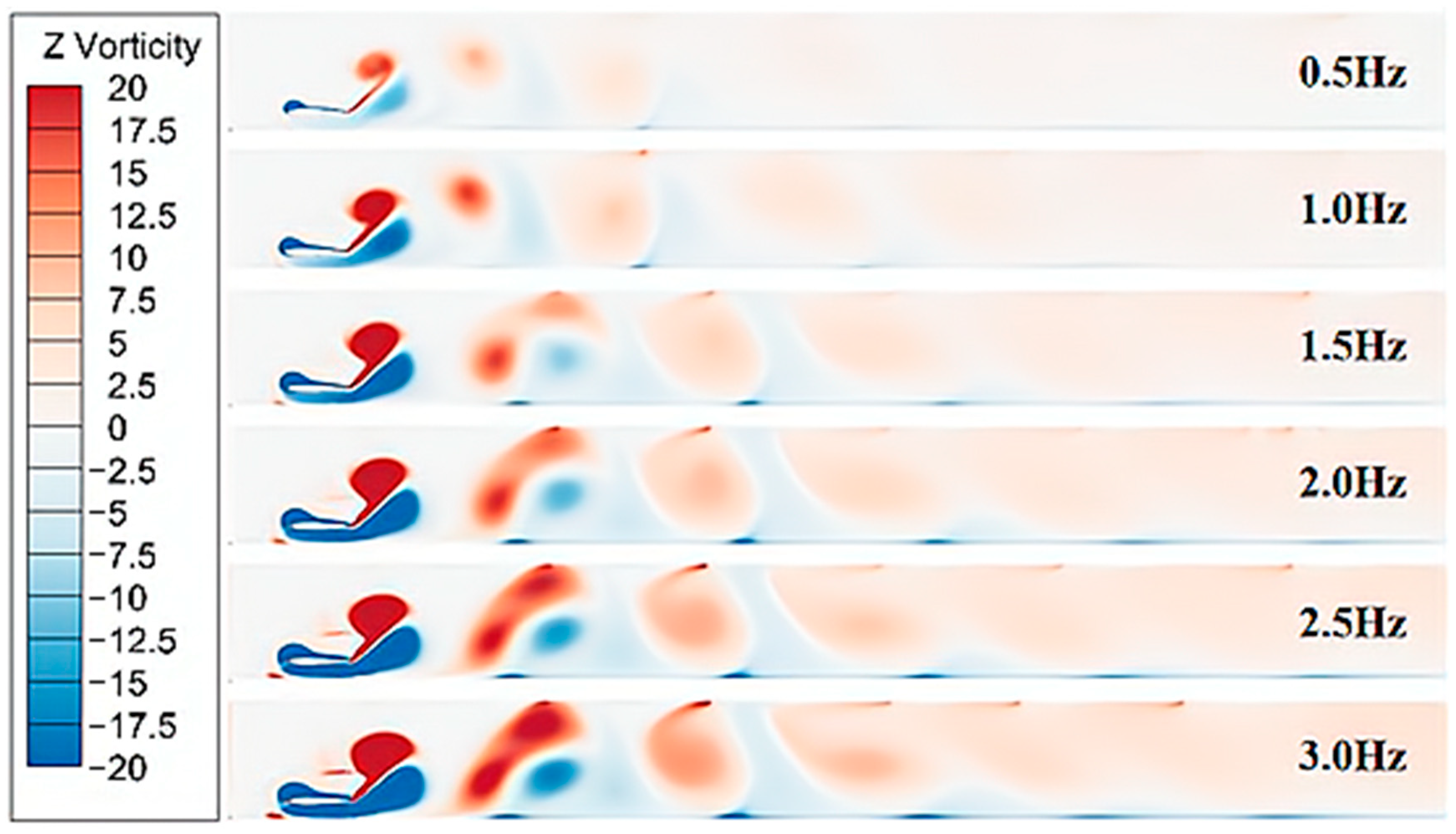
4.1. Impact of Flapping Hydrofoil Motion on the Distribution of Suspended Particles in Water
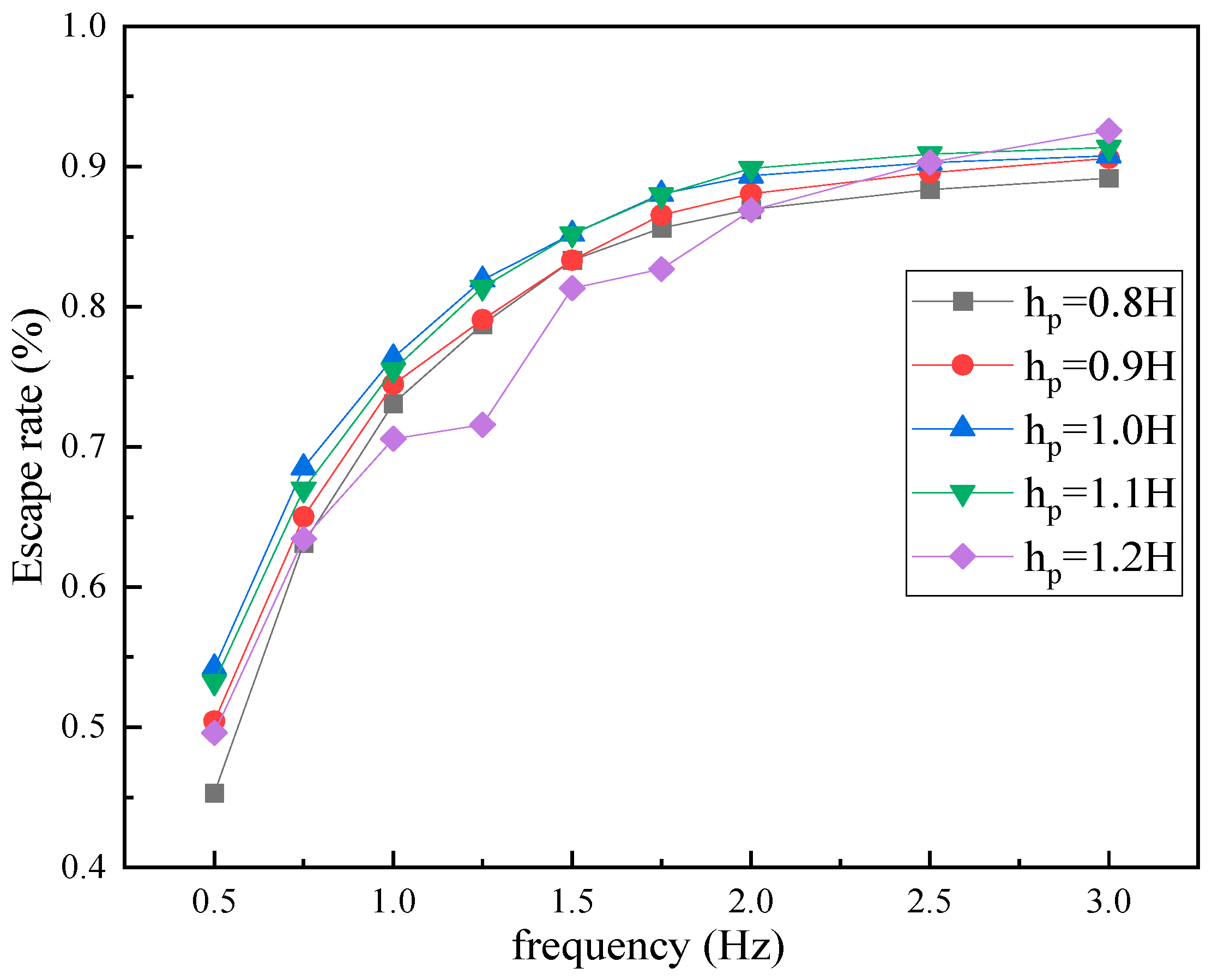
4.2. Impact of Pumping Depth on the Efficiency of Discharging Suspended Particles
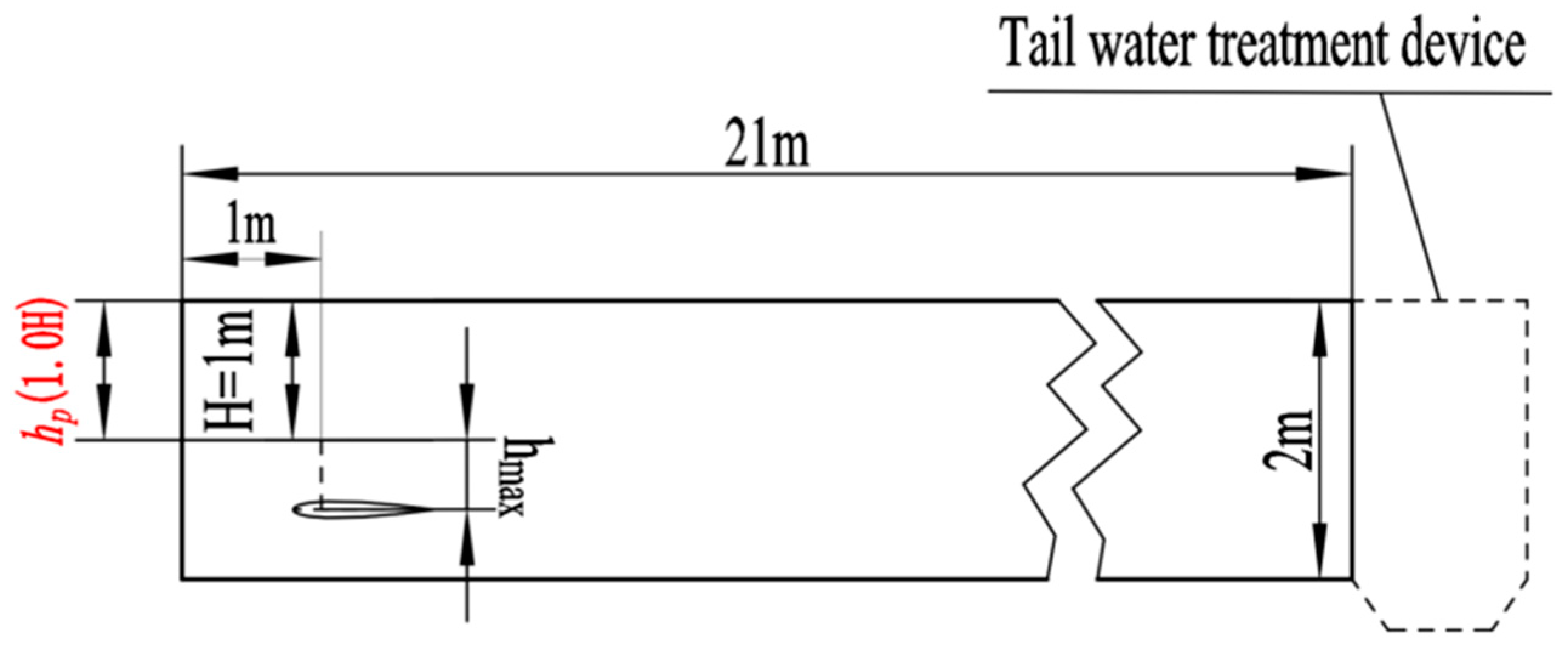
4.2.1. Variable Setting for Pumping Depth
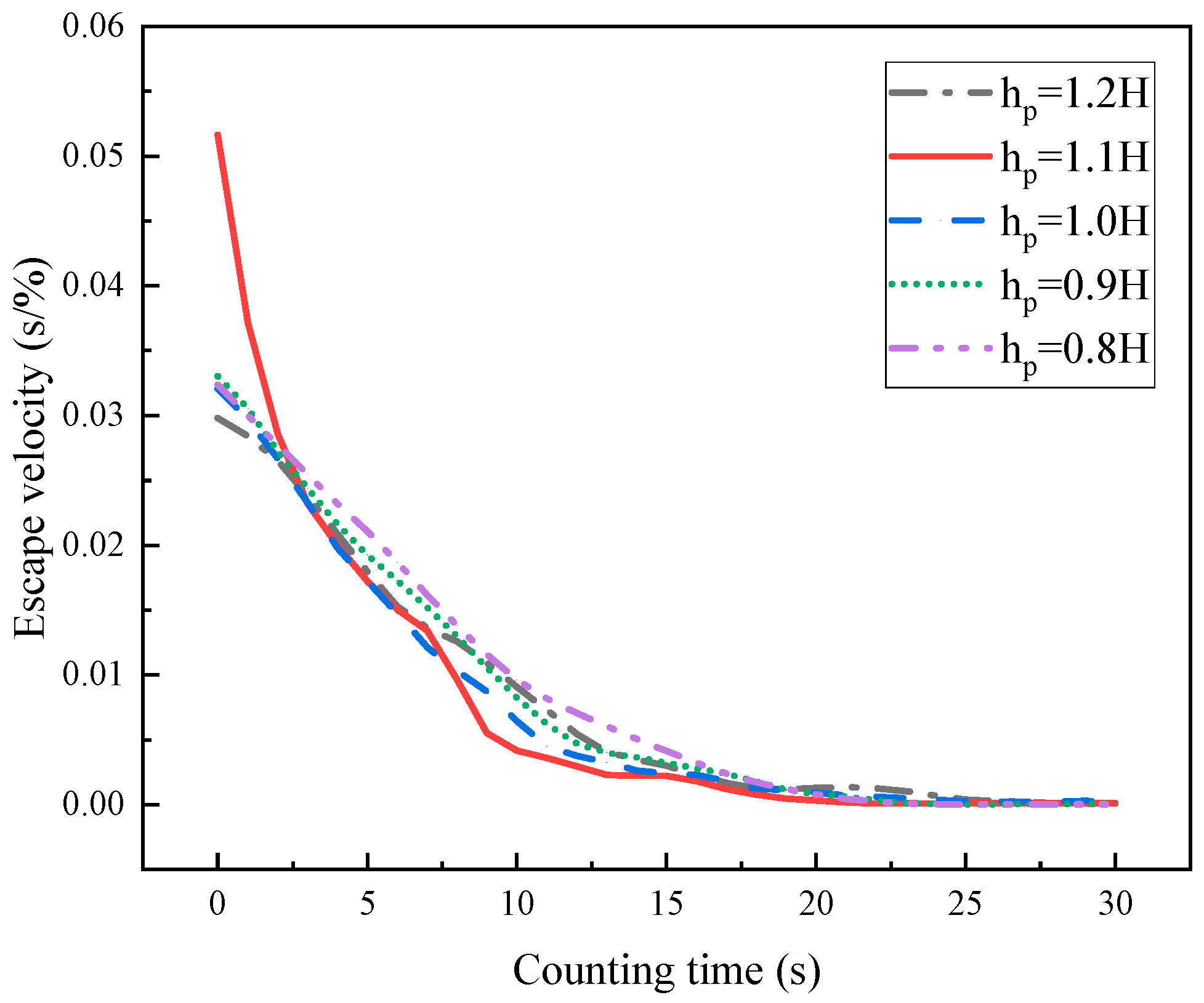
4.2.2. Effect of Pumping Depth on Particle Escape Rates and Escape Rates
4.3. Analysis of the Combined Effect of Pumping Depth and Pumping Frequency
5. Conclusions
- The flapping hydrofoil generates a gradually dissipating Kármán vortex street during the pumping process, effectively addressing the issue of insufficient flow velocity in ecological water bodies. Additionally, the thrust generated by vortex dissipation enhances the discharge efficiency of suspended particles;
- Excessive depth of the flapping hydrofoil pumping leads to significant interference from the bottom, while a shallow pumping depth results in the downward dissipation of the Kármán vortex street due to gravity, consuming more energy and resulting in inadequate thrust generated by the flapping hydrofoil;
- At a pumping depth of 1.1 H, the flapping hydrofoil motion produces a more complete and stable reversed Kármán vortex street, which effectively reduces the effect of runway end-wall shear on the near-bottom flow velocity and enhances the overall flow velocity of the raceway, which in turn leads to a higher rate of escape velocity of suspended particles at this point;
- The depth and frequency of the flapping hydrofoil pumping water have a combined effect on the particle discharge. At low frequency (0.5 Hz), the closer the pumping depth of the flapping hydrofoil is to 1.0 H, the better the effect of the flapping hydrofoil discharge; as the frequency increases, the deeper the pumping depth of the flapping hydrofoil is, the better the effect of the suspended particles’ discharge;
- In raceway aquaculture sewage discharge, this paper suggests the reference values of pumping depth and frequency of 1.1 H and 2.0 Hz. This combination of pumping parameters for flapping hydrofoils can produce stable reversed Kármán vortex street structures, and without energy wastage due to a high frequency without significant pumping efficiency improvement, the flapping hydrofoils can obtain good pumping efficiency and suspended pollutant discharge results.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wu, X.; Zhang, X.; Tian, X.; Li, X.; Lu, W. A review on fluid dynamics of flapping foils. Ocean Eng. 2020, 195, 106712. [Google Scholar] [CrossRef]
- Yang, G.; Ma, R.; Zhang, L.; Jiang, J.; Yao, S.; Zhang, M.; Zeng, H. Lake status, major problems and protection strategy in China. J. Lake Sci. 2010, 22, 799–810. [Google Scholar]
- Barsi, D.; Fink, R.; Odry, P.; Ubaldi, M.; Zunino, P. Flow regulation of low head hydraulic propeller turbines by means of variable rotational speed: Aerodynamic motivations. Machines 2023, 11, 202. [Google Scholar] [CrossRef]
- Liu, S.; Cao, H.; Chen, Y.; Ni, S.; Zhao, G.; Jiang, C. Numerical examination of the dynamic evolution of fluctuations in cavitation and pressure in a centrifugal pump during startup. Machines 2023, 11, 67. [Google Scholar] [CrossRef]
- Anderson, J.M.; Streitlien, K.; Barrett, D.S.; Triantafyllou, M.S. Oscillating foils of high propulsive efficiency. J. Fluid Mech. 1998, 360, 41–72. [Google Scholar] [CrossRef]
- Cheng, J.; Li, J.; Wen, H.; Wen, S.; Ni, M.; Ren, Y.; Ding, H.; Song, Z.; Liu, M.; Lai, C.; et al. Effects of culture density on growth, muscle fractions and blood physiological and biochemical indices of juvenile hybrid sturgeon. J. Fish. Sci. China 2015, 22, 433–441. [Google Scholar]
- Zhou, L.; Tang, R.; Liu, X.; Cheng, G.; Gu, Z.; Liu, S. Research progress of suspended particulate matter removal equipment in recirculating aquaculture system. J. Chin. Agric. Mech. 2023, 44, 78–86. [Google Scholar]
- Guo, K.; Zhao, W.; Dong, S.L.; Jiang, Z.Q. Structure of suspended particles and organic carbon storage in jellyfish-shellfish-fish-prawn polyculture ponds. Acta Ecol. Sin. 2016, 36, 1872–1880. [Google Scholar]
- Huggins, D.L.; Piedrahita, R.H.; Rumsey, T. Analysis of sediment transport modeling using computational fluid dynamics (CFD) for aquaculture raceways. Aquac. Eng. 2004, 31, 277–293. [Google Scholar] [CrossRef]
- Brinker, A.; Rösch, R. Factors determining the size of suspended solids in a flow-through fish farm. Aquac. Eng. 2005, 33, 1–19. [Google Scholar] [CrossRef]
- Li, W.; Cheng, X.; Xie, J.; Wang, Z.; Yu, D. Hydrodynamics of an in-pond raceway system with an aeration plug-flow device for application in aquaculture: An experimental study. R. Soc. Open Sci. 2019, 6, 182061. [Google Scholar] [CrossRef] [PubMed]
- Hua, E.; Chen, W.; Tang, S. Water Pushing Flow Characteristics of Flapping Hydrofoil Device in Small River. Trans. Chin. Soc. Agric. Mach. 2022, 53, 154–162. [Google Scholar]
- Zhang, K.; Xie, J.; Yu, D.; Wang, G.; Gong, W.; Li, Z.; Yu, E.; Tian, J.; Xia, Y. Study on food web of grass carp (Ctenopharyngodon idellus) push-water aquaculture ecosystem by stable isotope analysis. South China Fish. Sci. 2020, 16, 9. [Google Scholar]
- Knoller, R. Die gesetzedes luftwiderstandes. Flug-Und Mot. 1909, 3, 1–7. [Google Scholar]
- Ding, H.; Song, B.; Tian, W. Analysis of underwater bionic flapping-wing propulsion performance. J. Northwest. Polytech. Univ. 2013, 31, 150–156. [Google Scholar]
- Du, X.; Zhang, Z. Numerical analysis of influence of four flapping modes on propulsion performance of underwater flapping foils. Eng. Mech. 2018, 35, 8. [Google Scholar]
- Xiao, Q.; Wang, J.; Jiang, B.; Ding, Y.; Yang, X. Study on nonlinear correlation in modal coefficients of the bionic airfoil. Machines 2023, 11, 88. [Google Scholar] [CrossRef]
- Hua, E.; Su, Z.; Xie, R.; Chen, W.; Tang, S.; Luo, H. Optimization and experimental verification of pivot position of flapping hydrofoil. J. Hydroelectr. Eng. 2023, 42, 128–138. [Google Scholar]
- Hua, E.; Tang, S.; Chen, W.; Xie, R.; Guo, X. Analysis on influence of swinging modes of swing hydrofoil on water propulsion. J. Hydroelectr. Eng. 2022, 41, 93–103. [Google Scholar]
- Hu, J.; Zhu, F.; Yao, R.; Gui, F.; Liu, B.; Zhang, Z.; Feng, D. Optimization of the inlet pipe layout of circular recirculating water aquaculture tank based on STAR-CCM+. Trans. Chin. Soc. Agric. Eng. 2021, 37, 244–251. [Google Scholar]
- Song, X.; Wu, D. Methods of controlling the density of fish feed. Fish. Mod. 2005, 44–45. [Google Scholar]
- Zhang, J.; Wu, T.; Xu, D.; Gao, Y.; Tang, W. Feeding on feces, diets and cage farming sediments of Paralichthys olivaceus by Japanese scallop Patinopecten yessoensis. Prog. Fish. Sci. 2013, 34, 96–102. [Google Scholar]
- Li, A.; Wang, S.; Wang, W. Study on settling rule of formation sand in the liquid fluids. Pet. Geol. Recovery Effic. 2001, 1, 70–73. [Google Scholar]
- Shaheed, R.; Mohammadian, A.; Kheirkhah Gildeh, H. A comparison of standard k–ε and realizable k–ε turbulence models in curved and confluent channels. Environ. Fluid Mech. 2019, 19, 543–568. [Google Scholar] [CrossRef]
- Read, D.A.; Hover, F.S.; Triantafyllou, M.S. Forces on oscillating foils for propulsion and maneuvering. J. Fluids Struct. 2003, 17, 163–183. [Google Scholar] [CrossRef]
- Li, Y.; Wang, J.; Chen, J. Numerical Investigation of Pitching Foil’s Propulsive Performance with Wall Effect. Nav. Archit. Ocean Eng. 2020, 36, 26–31. [Google Scholar]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hua, E.; Xiang, M.; Wang, T.; Song, Y.; Lu, C.; Sun, Q. Investigation of the Effect of Pumping Depth and Frequency of Flapping Hydrofoil on Suspended Matter Discharge Characteristics. Machines 2024, 12, 300. https://doi.org/10.3390/machines12050300
Hua E, Xiang M, Wang T, Song Y, Lu C, Sun Q. Investigation of the Effect of Pumping Depth and Frequency of Flapping Hydrofoil on Suspended Matter Discharge Characteristics. Machines. 2024; 12(5):300. https://doi.org/10.3390/machines12050300
Chicago/Turabian StyleHua, Ertian, Mingwang Xiang, Tao Wang, Yabo Song, Caiju Lu, and Qizong Sun. 2024. "Investigation of the Effect of Pumping Depth and Frequency of Flapping Hydrofoil on Suspended Matter Discharge Characteristics" Machines 12, no. 5: 300. https://doi.org/10.3390/machines12050300
APA StyleHua, E., Xiang, M., Wang, T., Song, Y., Lu, C., & Sun, Q. (2024). Investigation of the Effect of Pumping Depth and Frequency of Flapping Hydrofoil on Suspended Matter Discharge Characteristics. Machines, 12(5), 300. https://doi.org/10.3390/machines12050300




