Mathematical Complexities in Modelling Damage in Spur Gears
Abstract
:1. Introduction
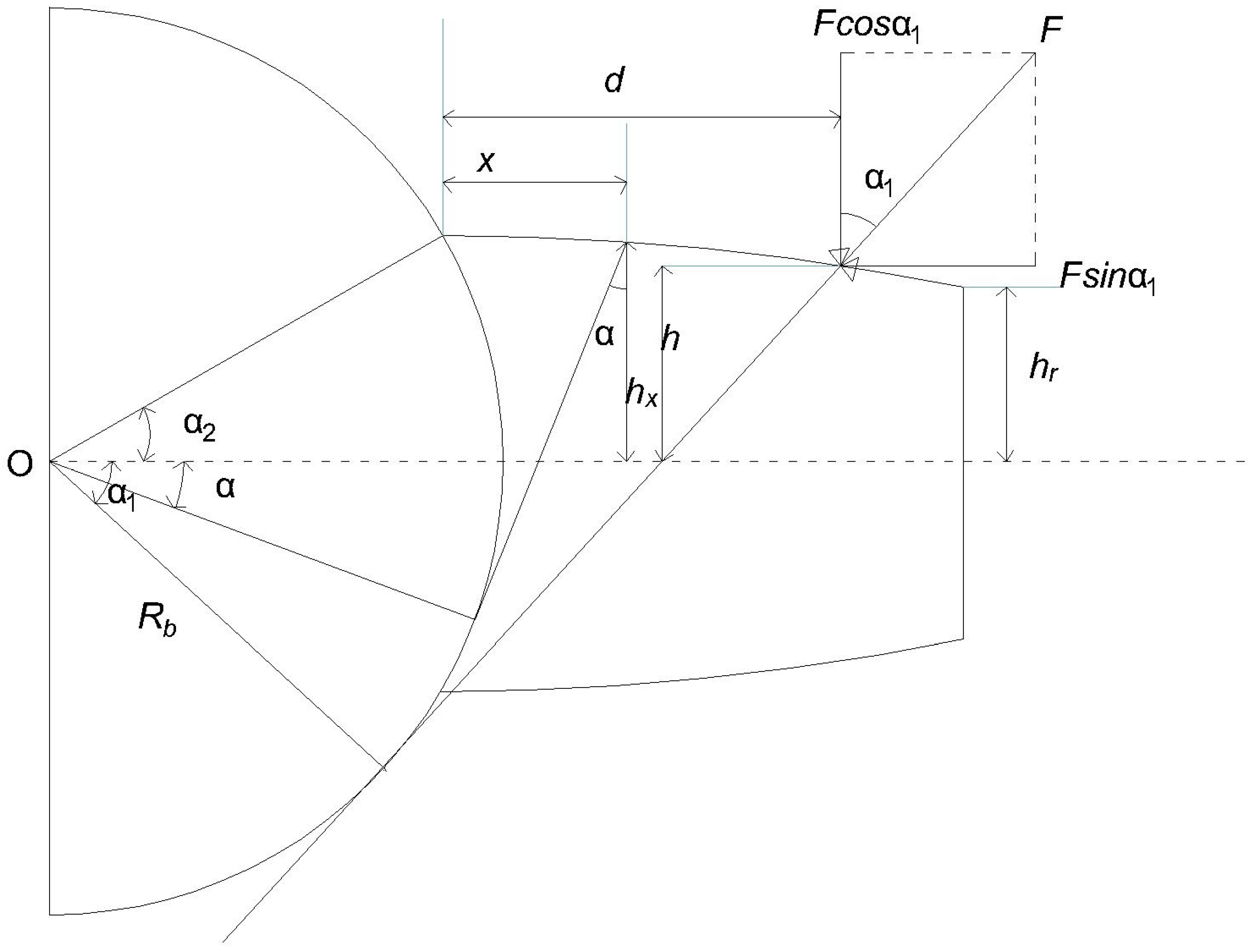
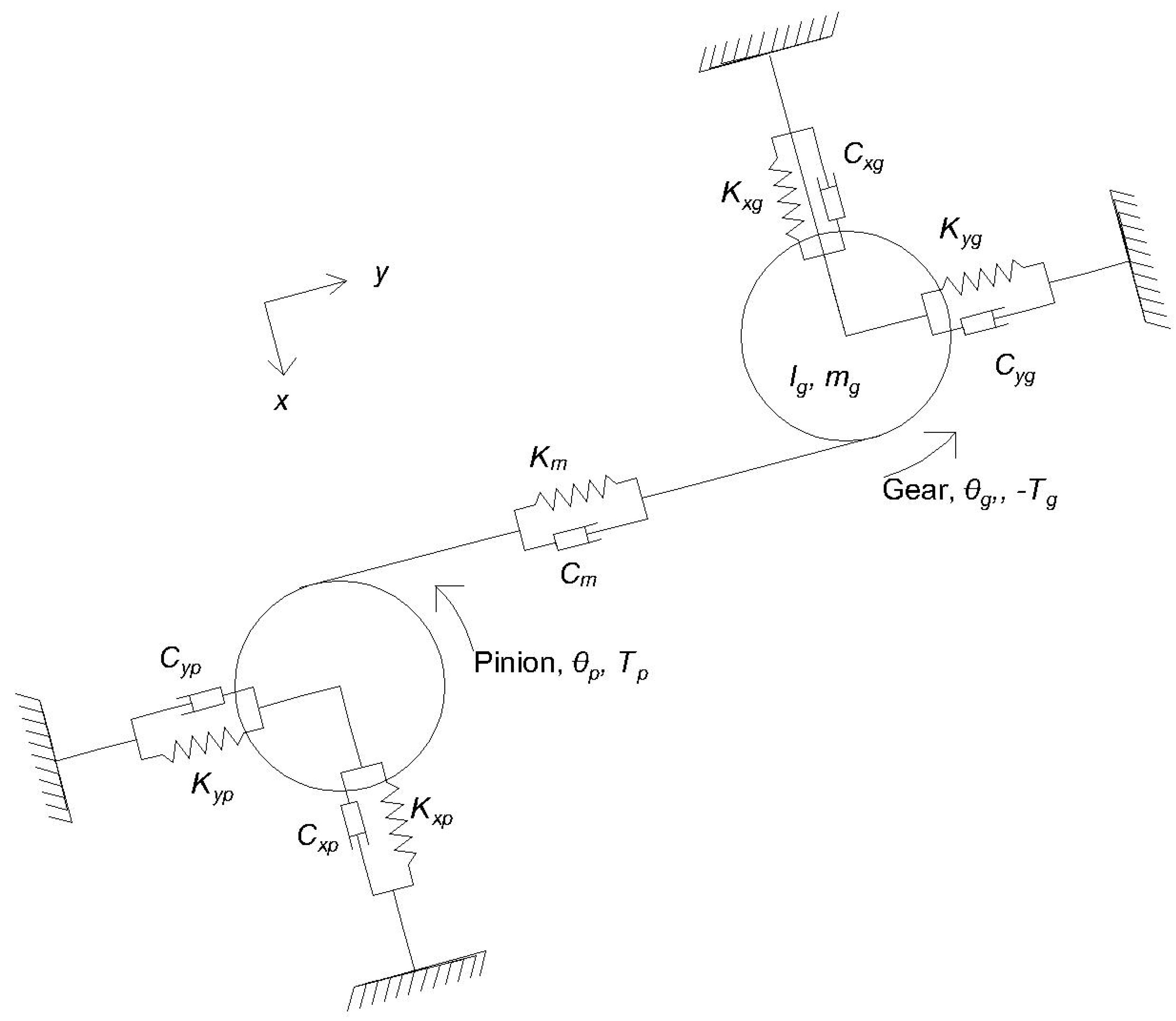
2. Analytical Models of Healthy Gears
- p = pinion, g = gear, x and y represent two translational directions, represent the rotational direction
- m = mass (kg)
- r = Base circle (mm)
- C = Radial damping (Ns/m)
- K = Radial stiffness (N/m)
- F = Frictional Force (N)
- T = Torque (Nm)
- M = Moments due to frictional forces (Nm)
- Km = Total mesh stiffness (N/m) and Cm = mesh damping (Ns/m)
- G = Shear modulus (N/mm2)
- Ax = Area moment of inertia =
- Ix = Moment of inertia =
- E = Elastic modulus (N/mm2)
- L = Tooth width (mm)
- = Deflection of the tooth fillet
- F = Force on tooth fillet (N)
- = the comprehensive mesh stiffness
- = the total mesh stiffness without lubrication
- = stiffness of the lubrication film
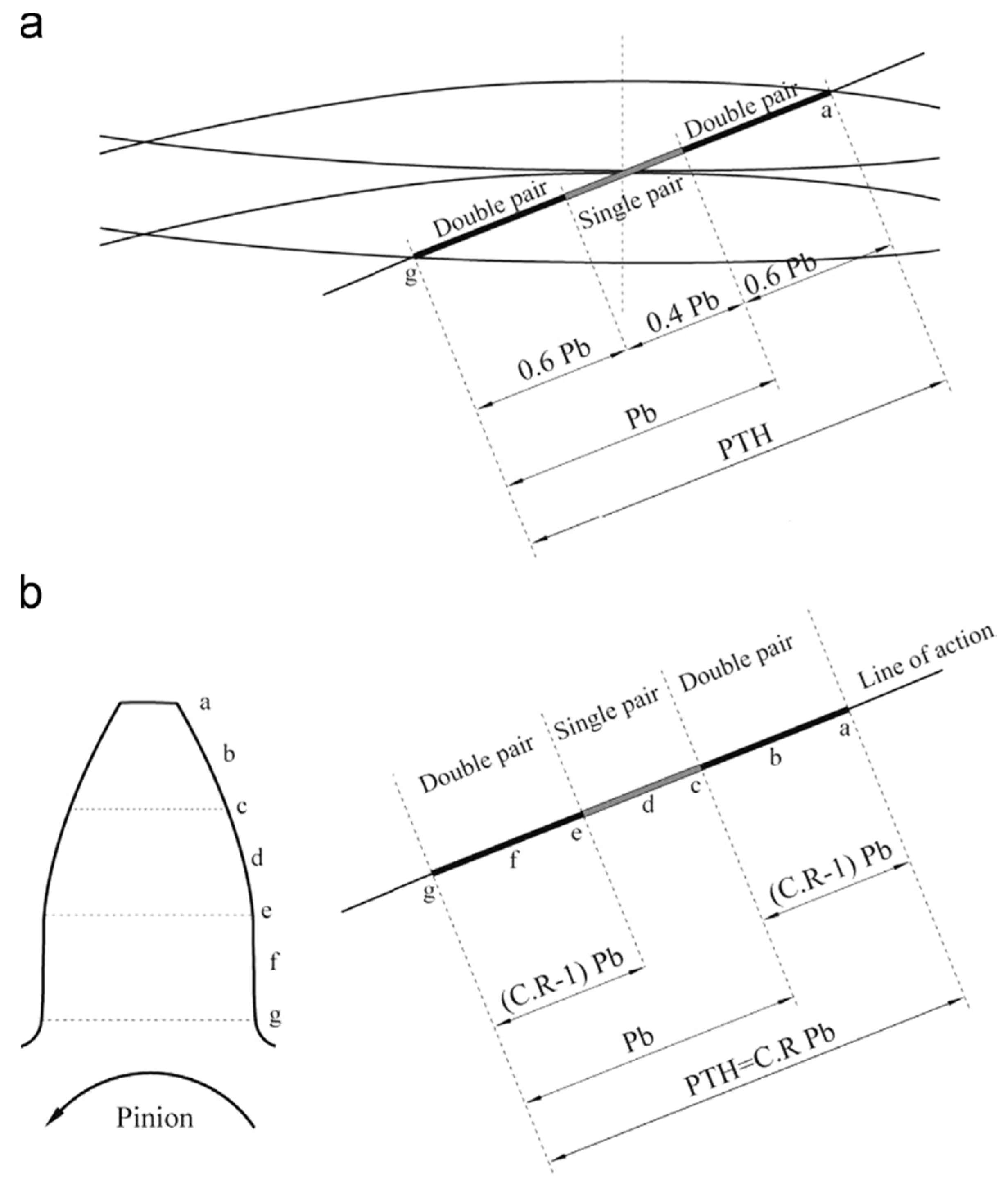
- C.R. = Contact ratio
- PTH = Path of contact
- Pb = Base circle circular pitch
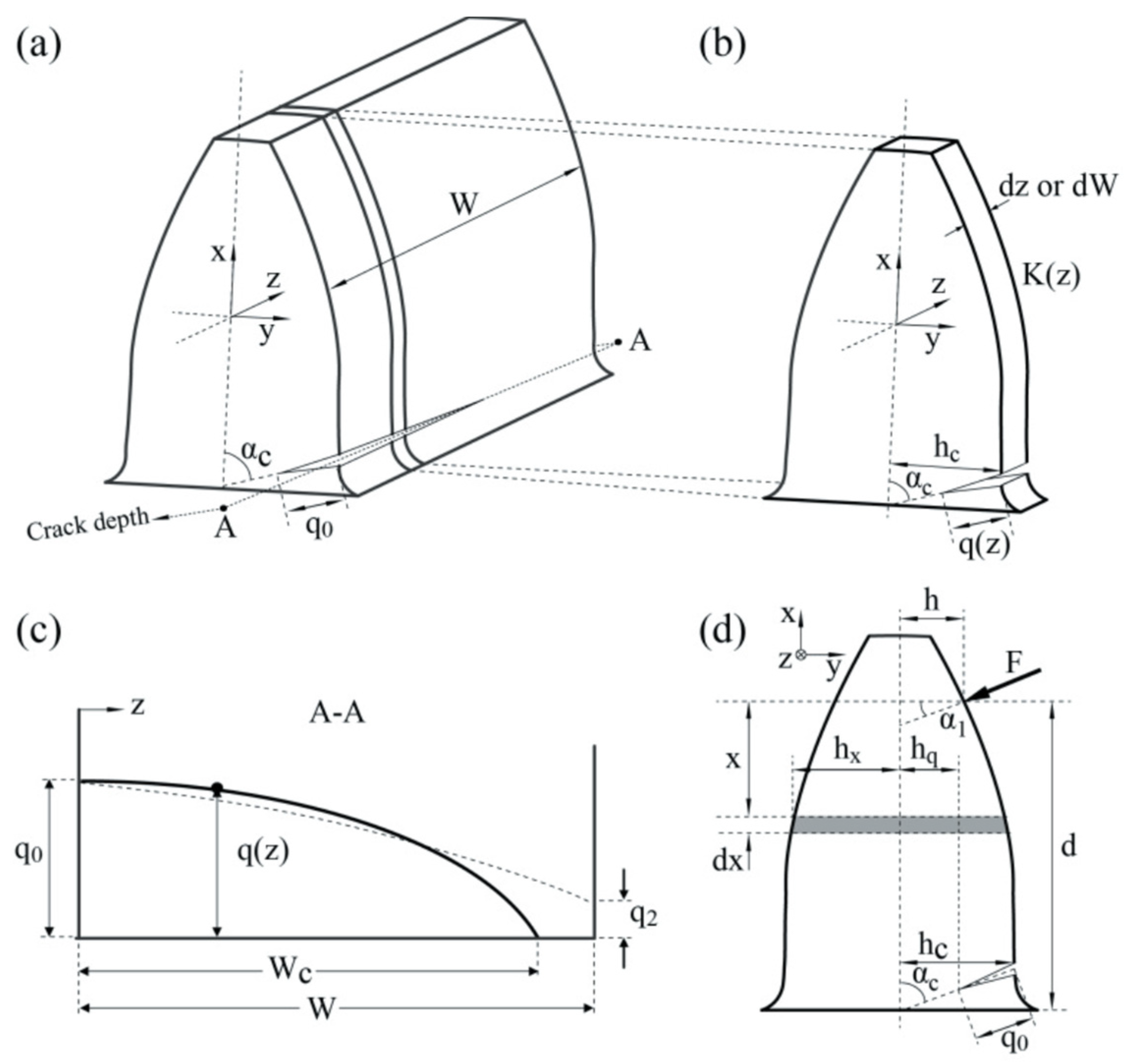
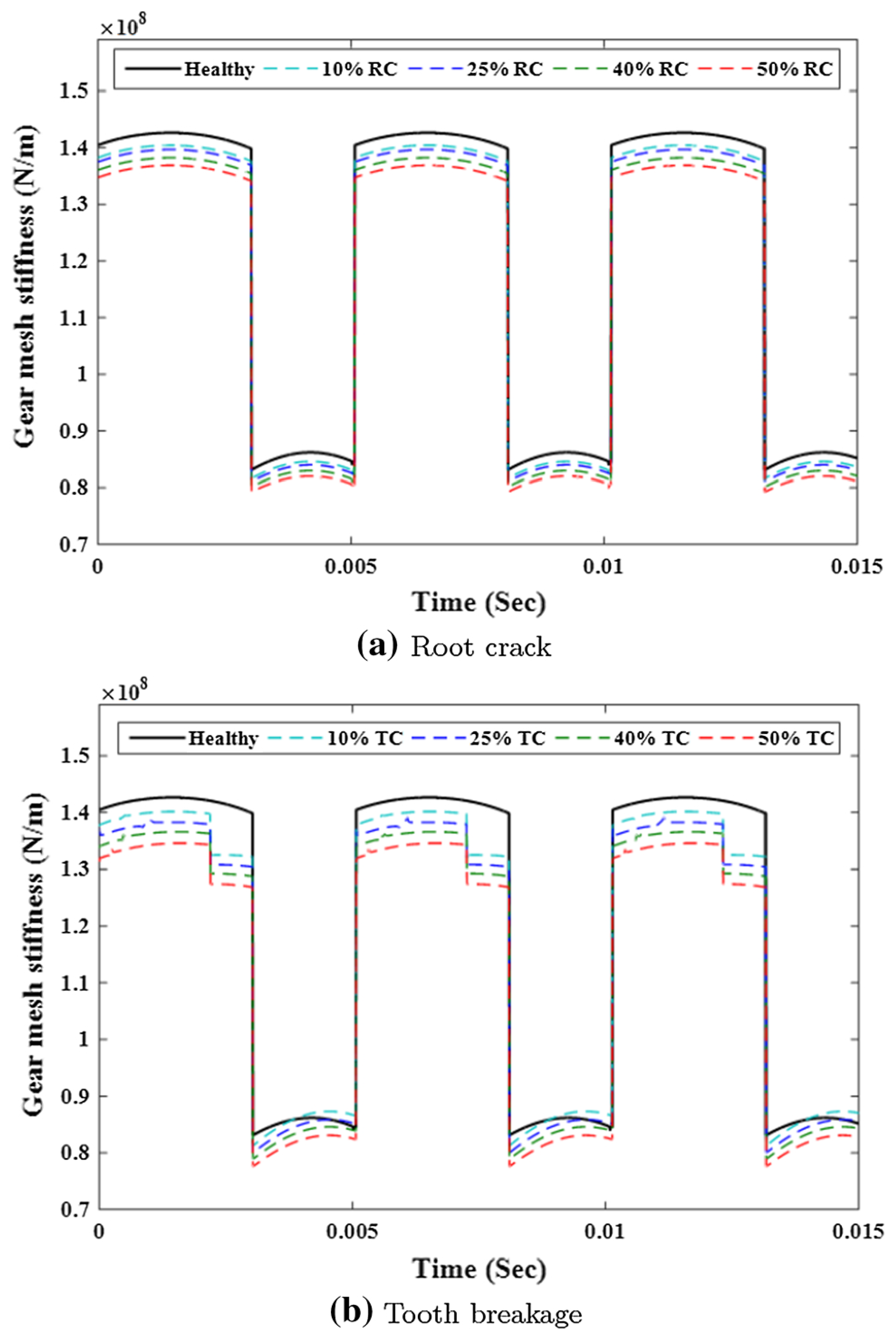
3. Analytical Models with Single Faults
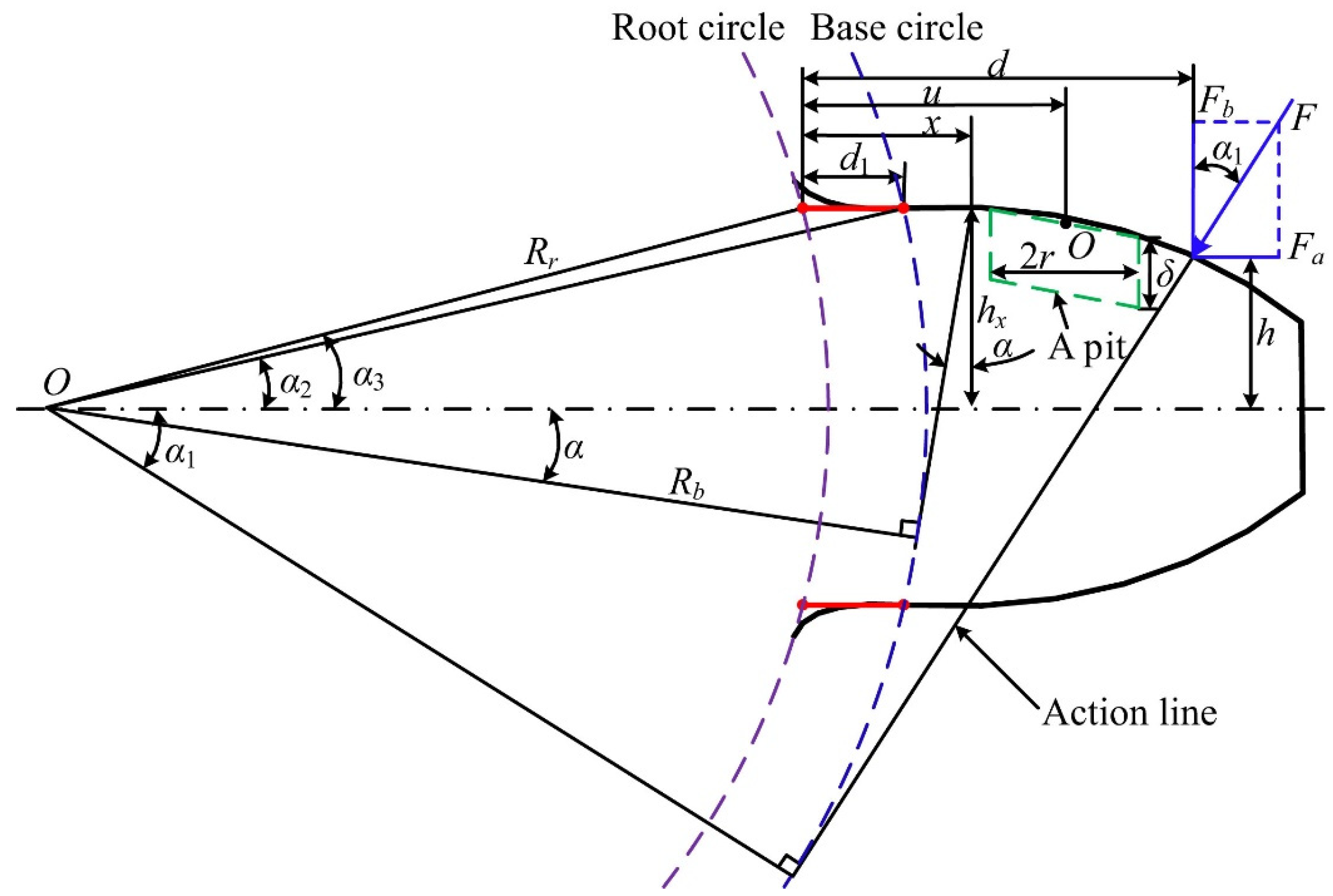
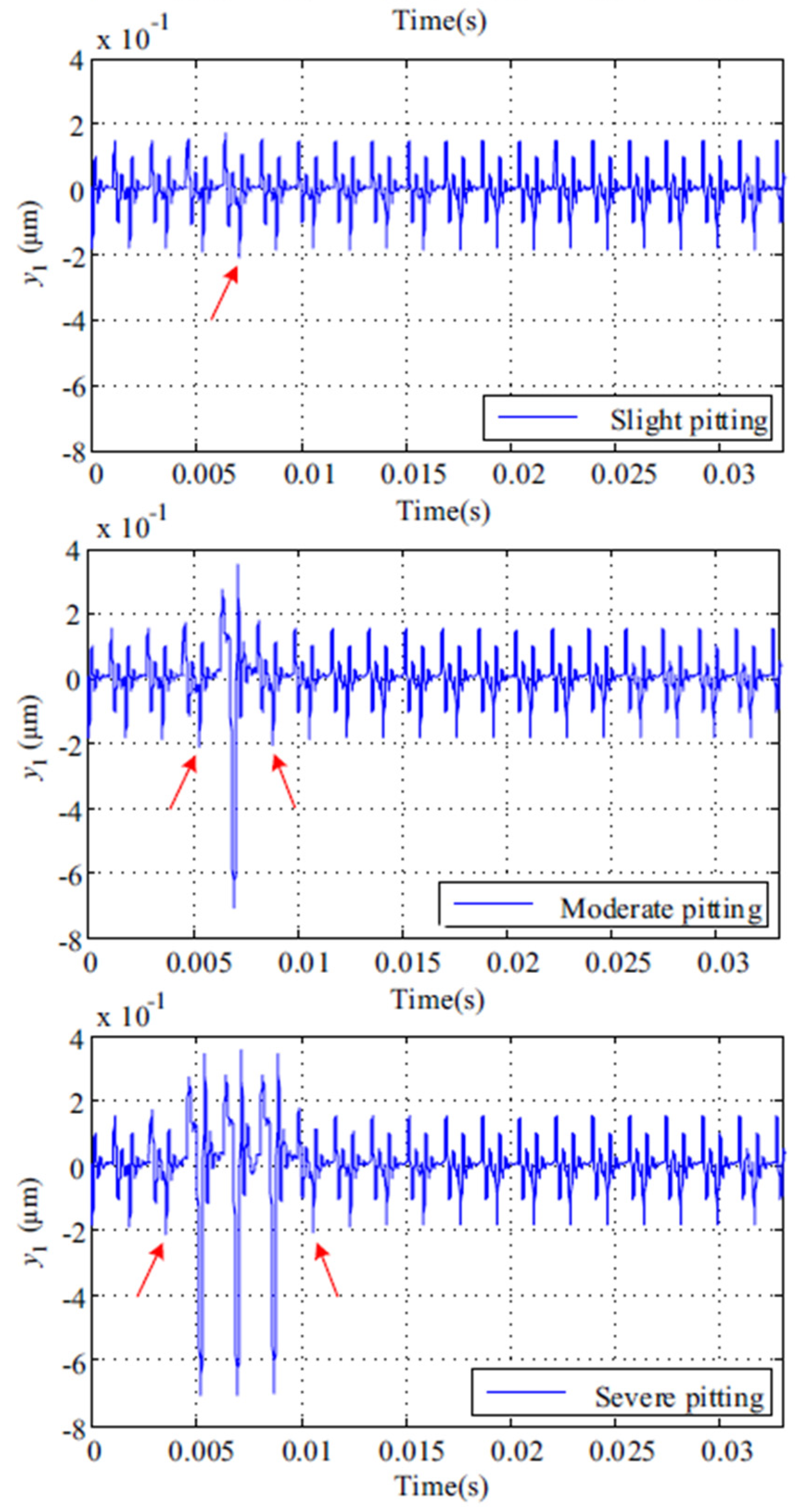
3.1. Gear Pitting Analytical Models
- = Change in length
- = Change in Area
- = Change in Area Moment of inertia
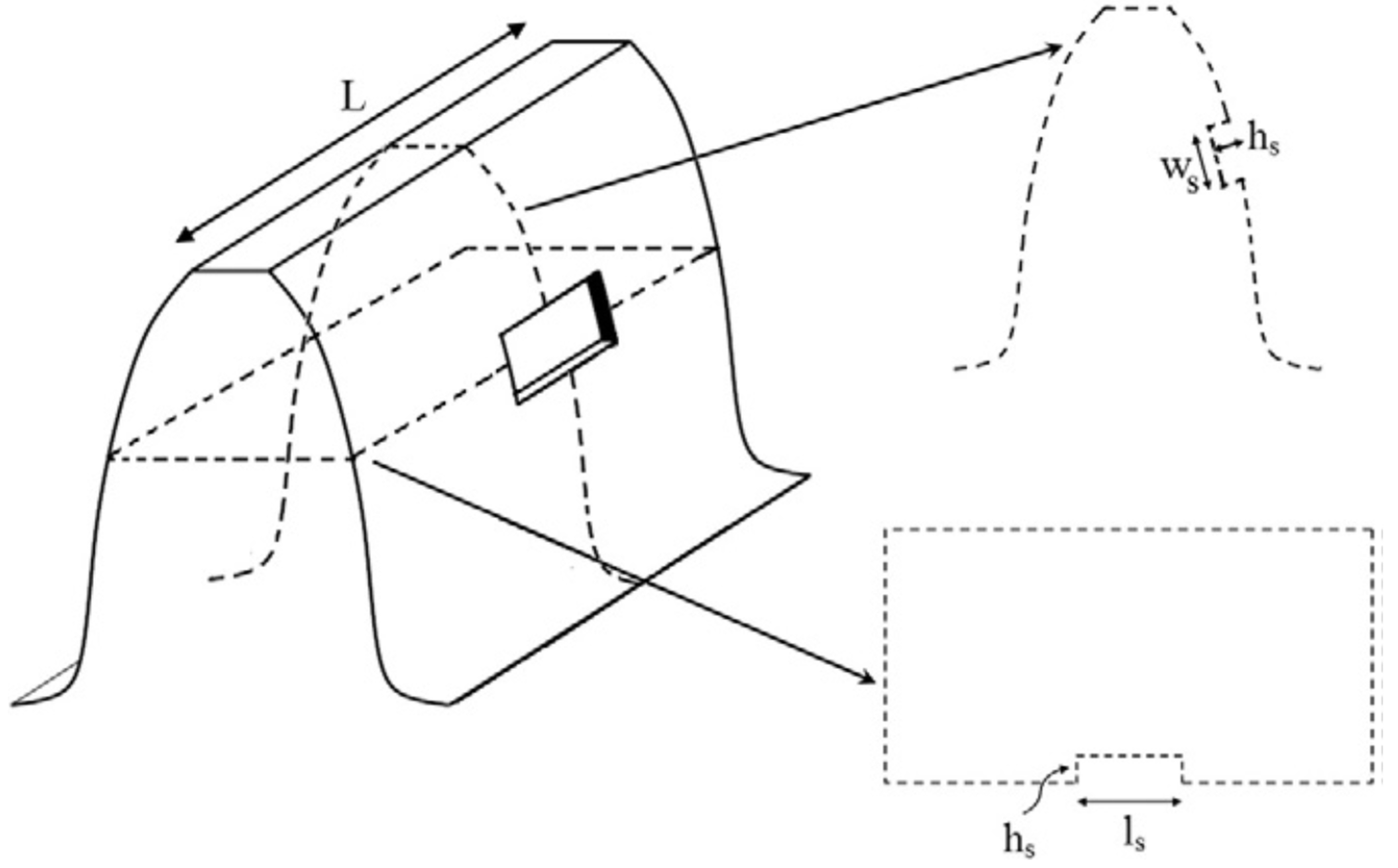
3.2. Gear Spalling Analytical Models
3.3. Gear Tooth Breakage Analytical Models
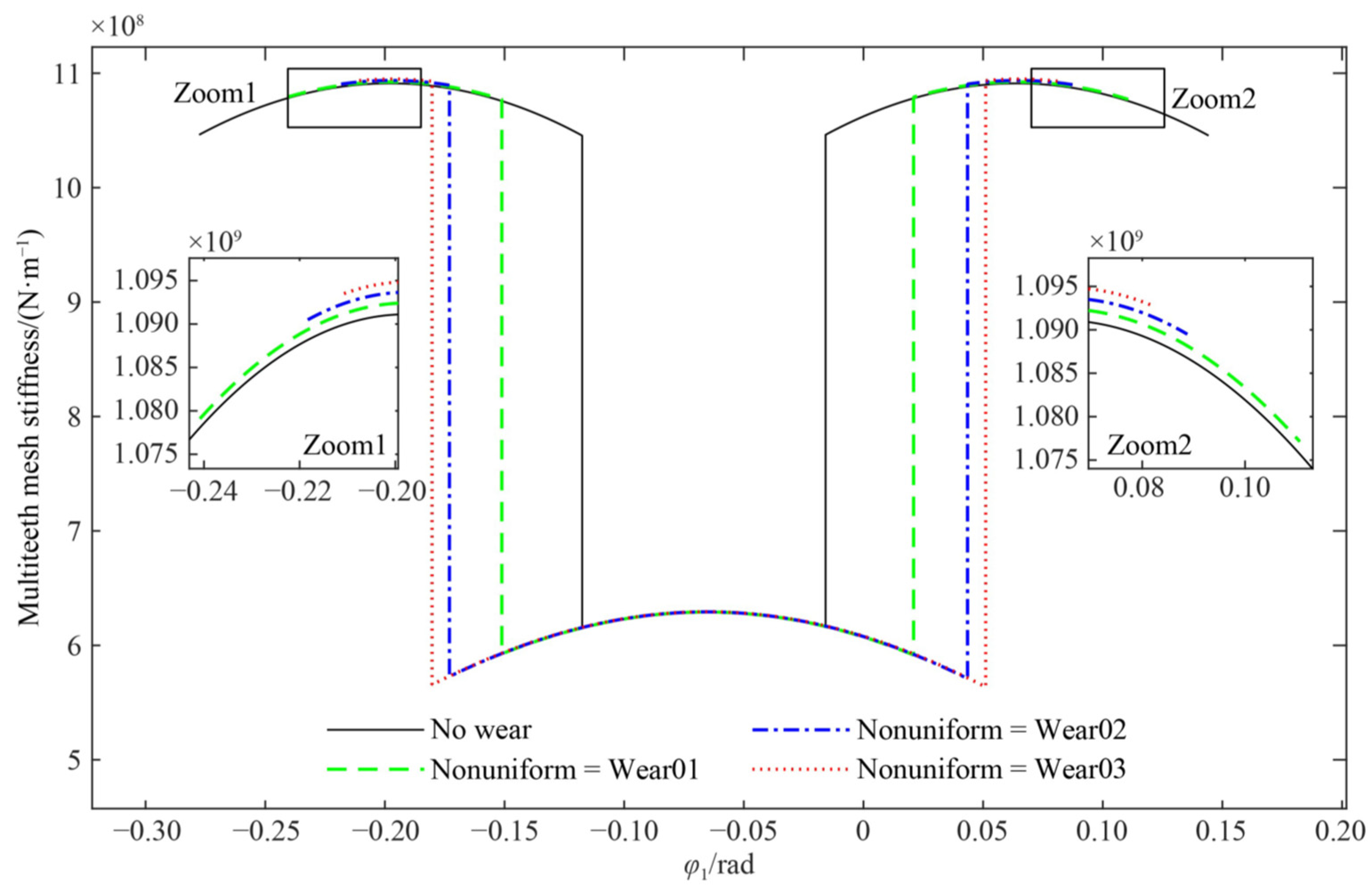
3.4. Gear Abrasive Wear Analytical Models
- = Pressure angle
- = Geometric transmission error
- = Dynamic transmission error (DTE)
- e = Static transmission error (STE)
- = Elastic deformation
- = Comprehensive error
- = Wear depth
3.5. Gear Adhesive Wear Analytical Models
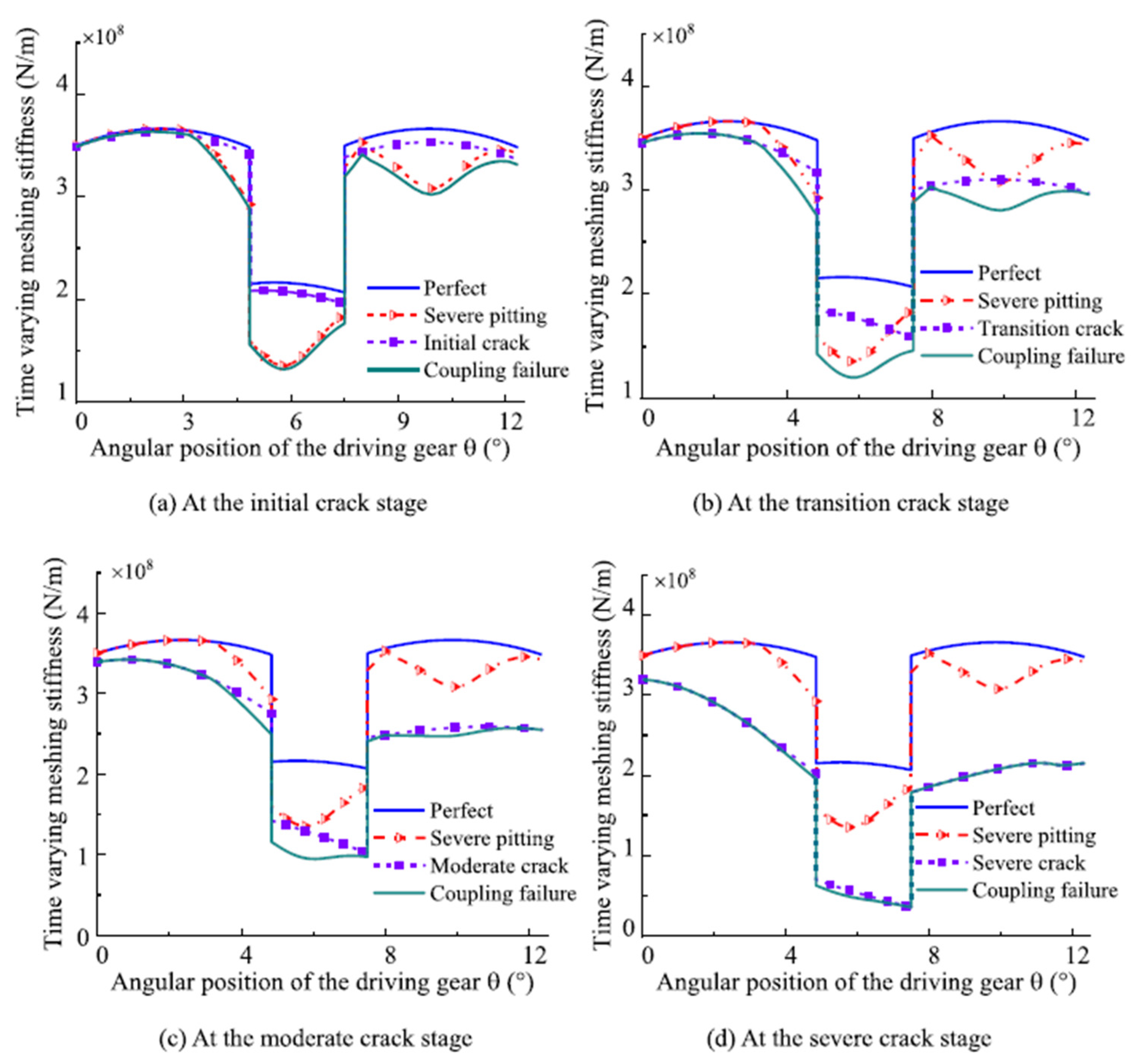
4. Analytical Models with Combined Faults
5. Failure Models Comparison
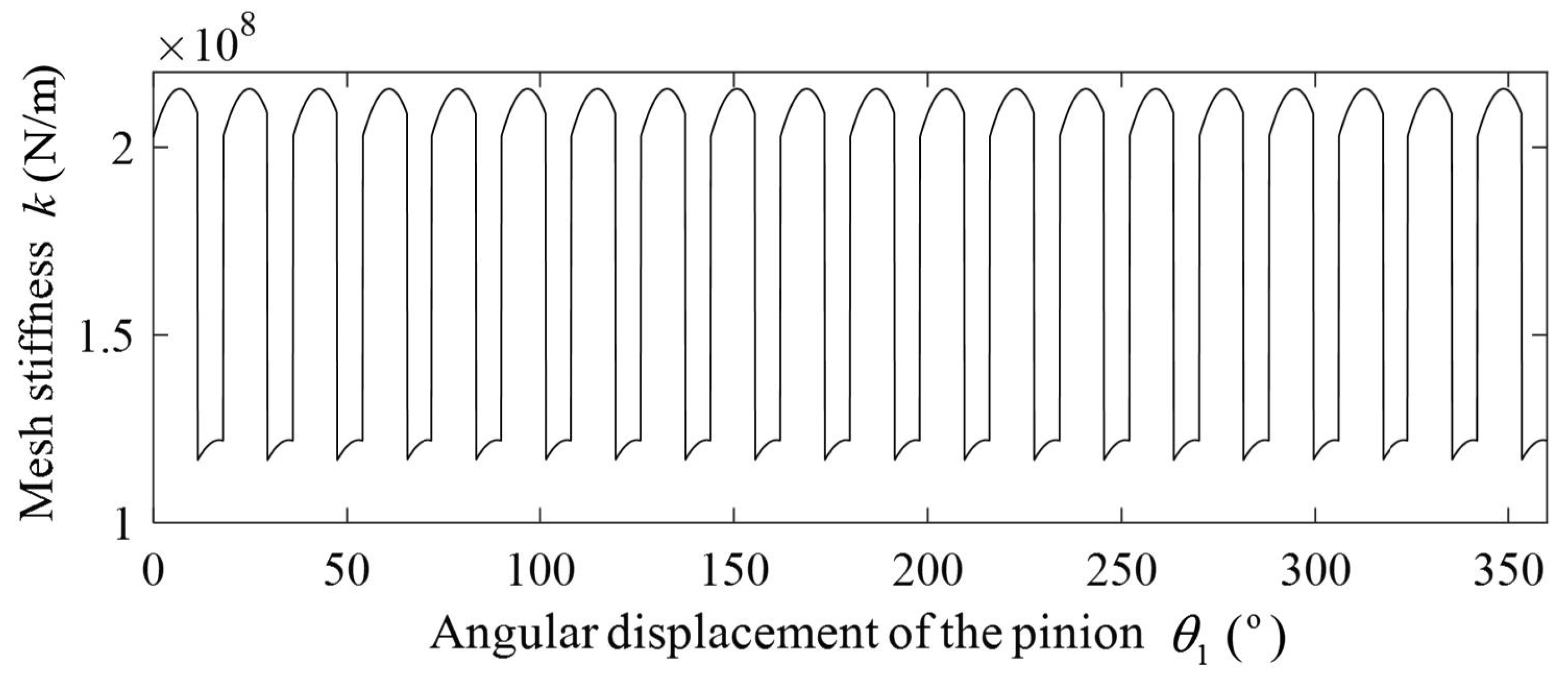
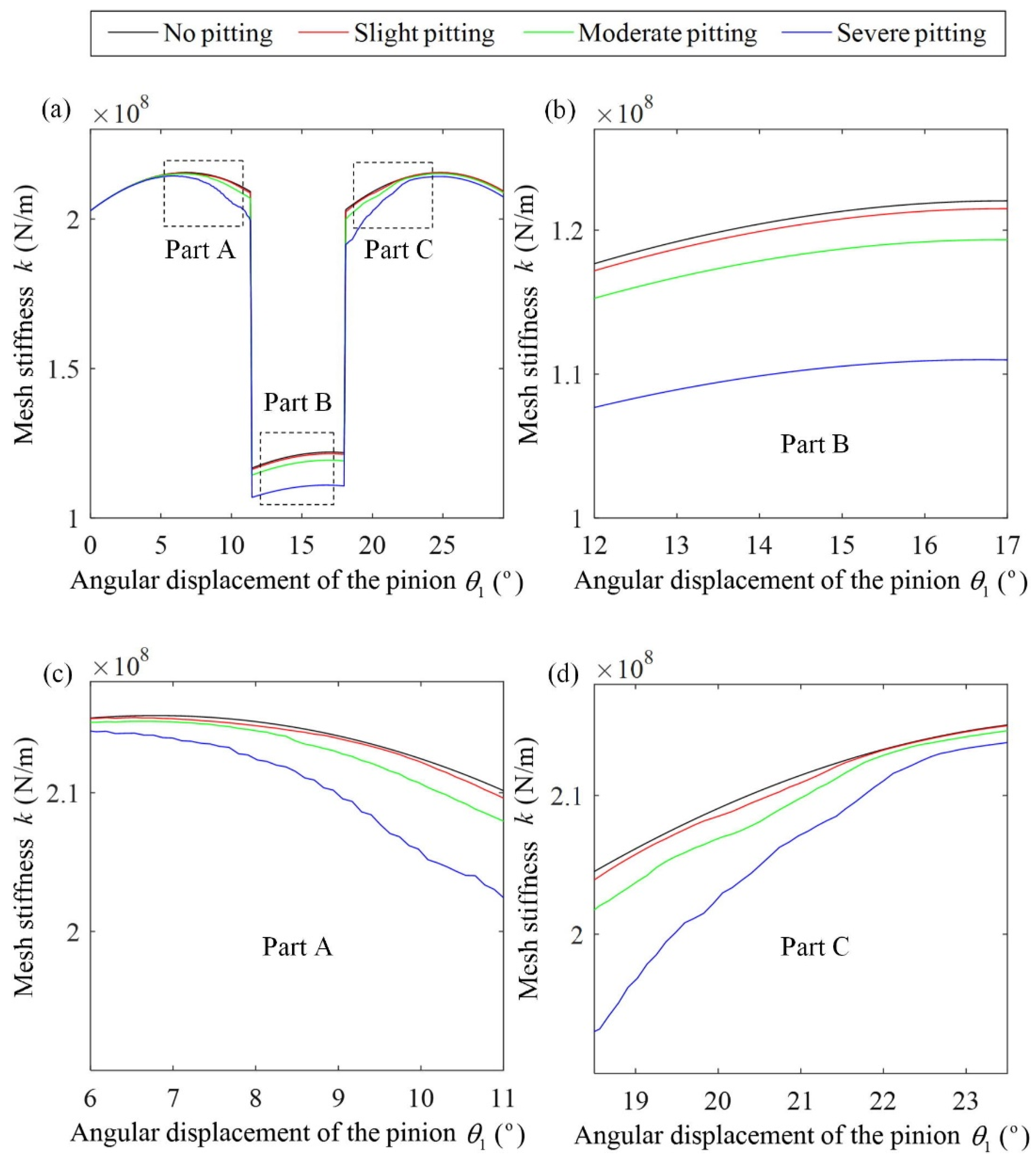
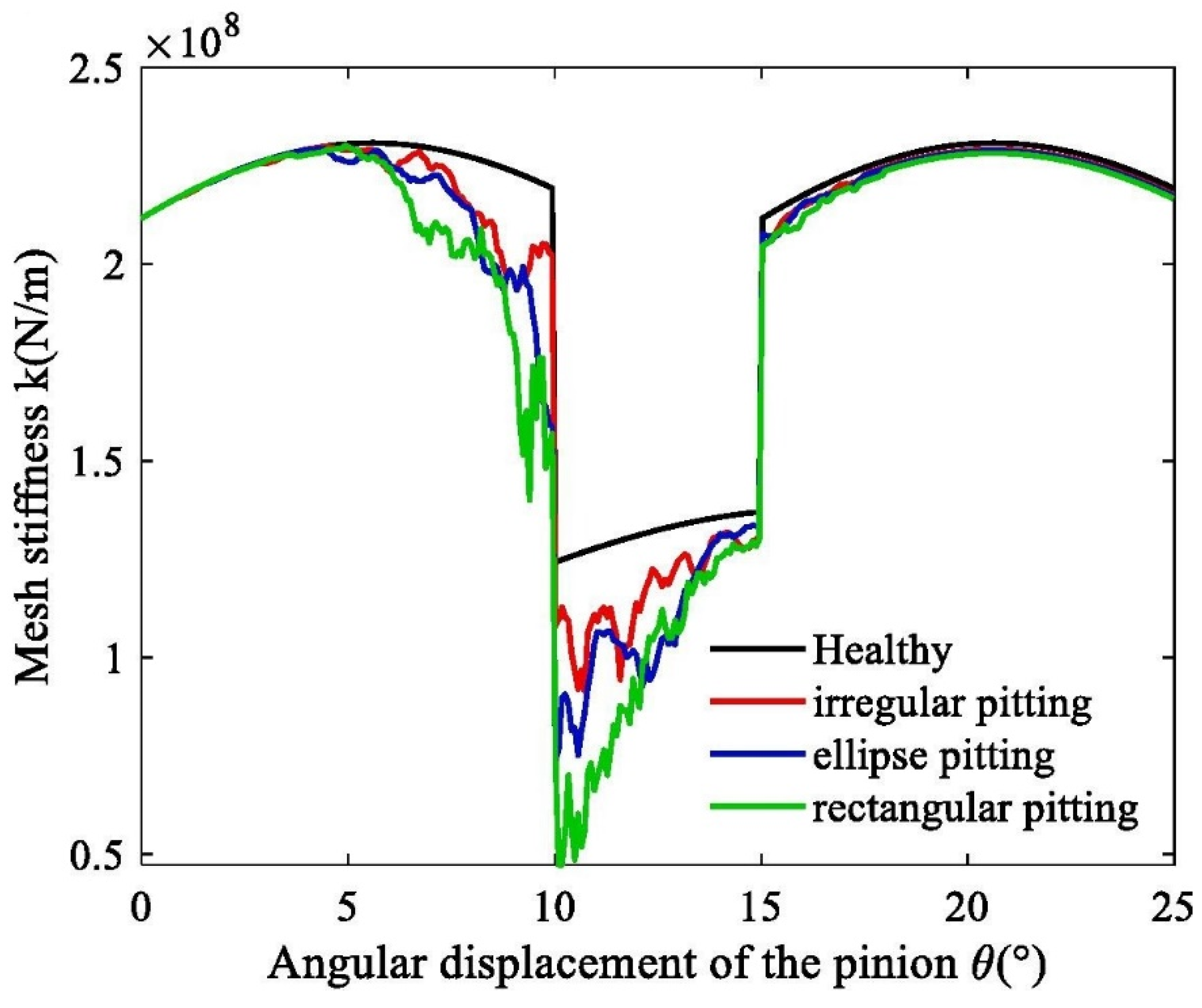
5.1. Comparison of TVMS
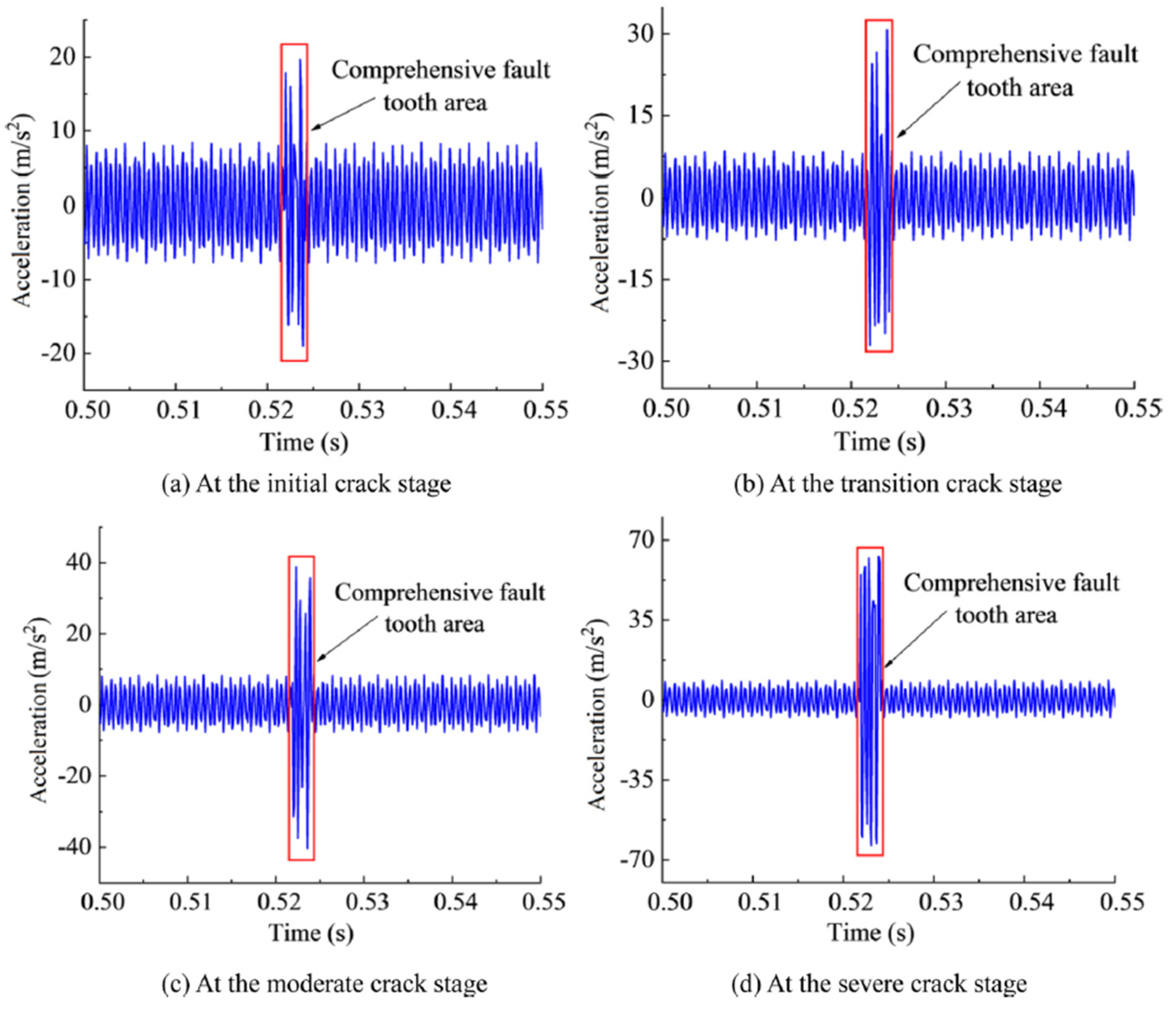
5.2. Comparison of Time Domain Responses
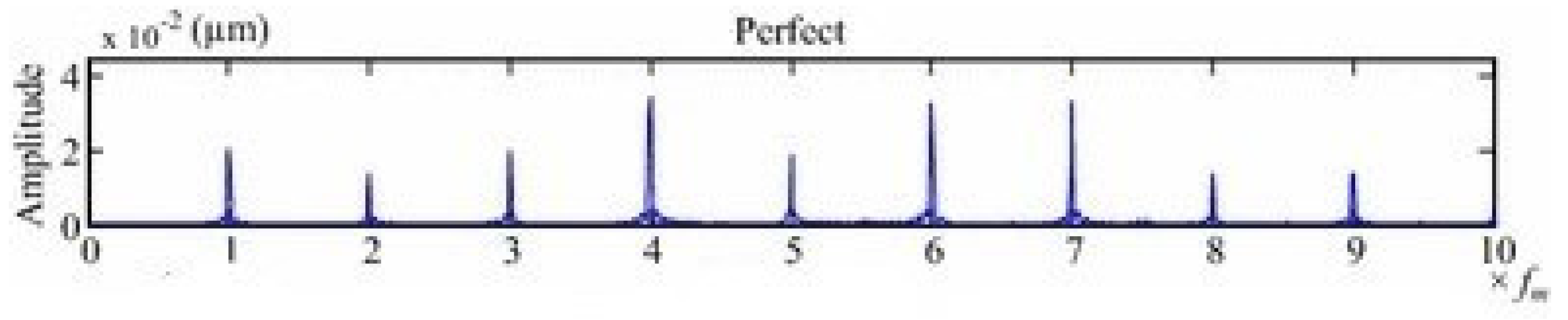
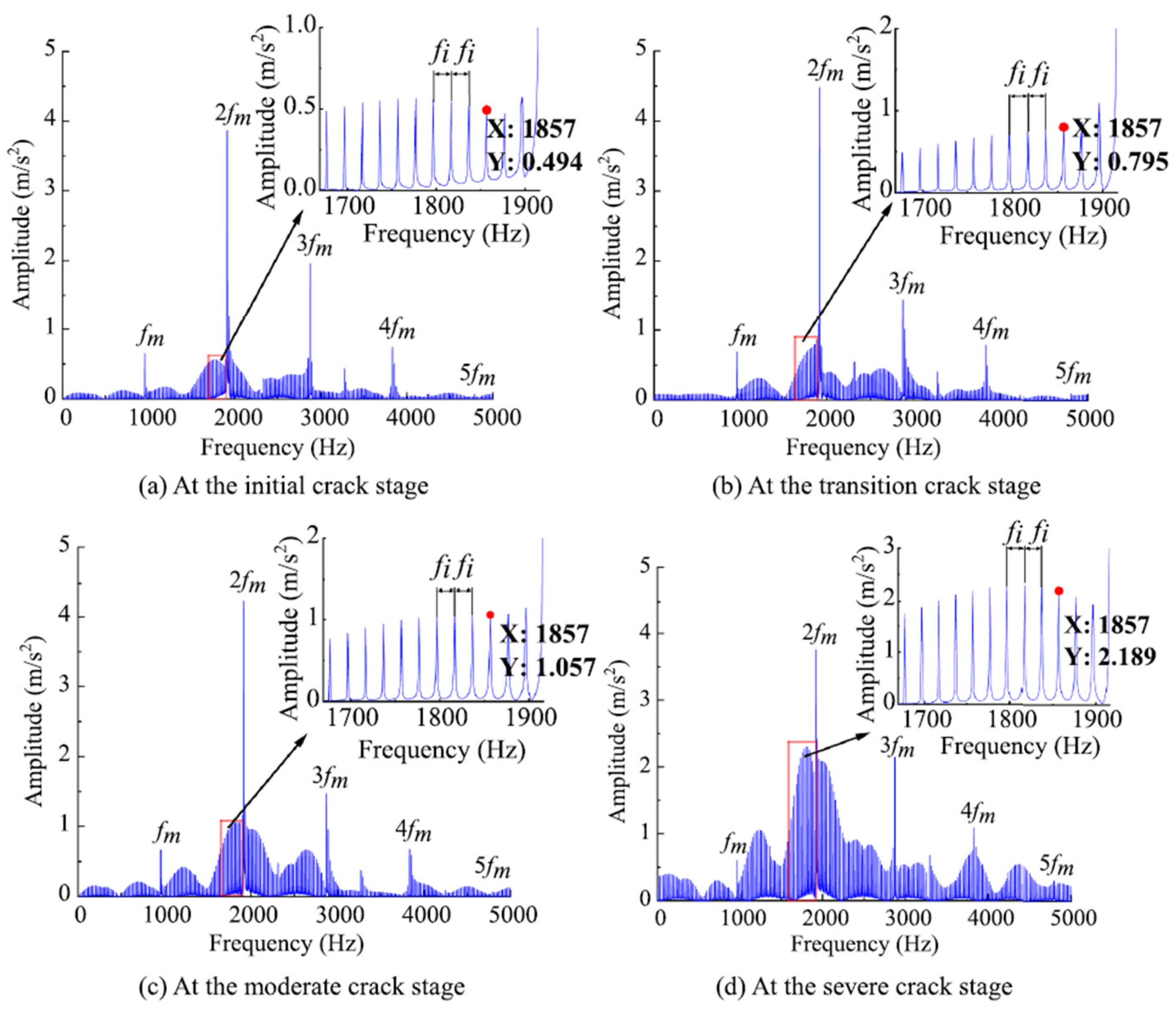
5.3. Comparison of Frequency Domain Responses
6. Key Findings and Challenges
6.1. Key Findings from Existing Literature
6.2. Current Challenges
6.2.1. The Dynamics of Fault Initiation and Progression
6.2.2. Assumptions in Fault Geometry
6.2.3. Fault Location
6.2.4. Reliability of Existing Models for Health Monitoring
6.3. Identified Research Needs
- Analytical modelling methods that can model the irregular shapes of gear faults are required to be able the provide realistic results. Currently, most models assume regular shapes for gear faults.
- Mathematical models to obtain responses for gears undergoing scuffing are not available. Research to develop these models by FEM or analytically will provide valuable information for gear health monitoring.
- Further investigations on the reliability of the TVMS for obtaining dynamic responses for abrasive wear need to be conducted. Current information has conflicting results on how wear affects the TVMS of a gear system.
- Gear fault models majorly provide responses where there is a fault on a single tooth on the pinion, a few works have been conducted on multiple teeth of the pinion. Responses for gears with faults on multiple teeth of the pinion and wheel will provide useful insight for gear health monitoring.
- Developing models that can cater to multiple fault conditions would help in estimating the remaining useful life of gears undergoing similar conditions in actual gear operations.
7. Conclusions
- The correct evaluation of the fault state time-varying mesh stiffness and in some cases, the transmission error is the key requirement to providing the dynamic response required.
- The study has also shown that the geometry and location of the fault are the main inputs to determining the correct time-varying mesh stiffness.
- The dynamic models for pitting, spalling and tooth root crack share very similar inputs and this indicates a high possibility for developing a combined model for these faults.
- The determination of TVMS or static transmission error for the case of scuffing needs to be developed as models providing a dynamic response for scuffing are not readily available.
- Developing multiple fault dynamic models to include abrasive wear and adhesive wear/scuffing may require additional evaluation like determining wear depth or a new meshing plane.
- Current works in gear analytical modelling are mainly single-fault models. This review recommends that efforts to combine multiple failure modes in gear analytical modelling be focused on in future studies. This would help provide accurate dynamic responses for a wide range of fault conditions that are obtainable for gears in operations. It would furthermore guarantee progress in gear health monitoring.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Khan, M.A.; Shahid, M.A.; Ahmed, S.A.; Khan, S.Z.; Khan, K.A.; Ali, S.A.; Tariq, M. Gear misalignment diagnosis using statistical features of vibration and airborne sound spectrums. Measurement 2019, 145, 419–435. [Google Scholar] [CrossRef]
- Kishore, K.; Sharma, A.; Mukhopadhyay, G. Failure Analysis of a Gearbox of a Conveyor Belt. J. Fail. Anal. Prev. 2020, 20, 1237–1243. [Google Scholar] [CrossRef]
- Feng, W.; Feng, Z.; Mao, L. Failure analysis of a secondary driving helical gear in transmission of electric vehicle. Eng. Fail. Anal. 2020, 117, 104934. [Google Scholar] [CrossRef]
- Parey, A.; Jain, N.K.; Koria, S.C. Failure analysis of air cooled condenser gearbox. Case Stud. Eng. Fail. Anal. 2014, 2, 150–156. [Google Scholar] [CrossRef]
- Manda, P.; Singh, S.; Singh, A.K. Failure Analysis of Cooler Fan Drive Gear System of Helicopter. Mater. Today Proc. 2018, 5, 5254–5261. [Google Scholar] [CrossRef]
- Ghosh, D.; Mondal, A.; Hussain, N.; De, S.K.; Ray, S.; Subramanian, C. Failure analysis of helical gear pinion. Mater. Today Proc. 2022, 66, 3714–3717. [Google Scholar] [CrossRef]
- Netpu, S.; Srichandr, P. Failure of a helical gear in a power plant. Eng. Fail. Anal. 2013, 32, 81–90. [Google Scholar] [CrossRef]
- Shantiswaroop, P.M.; Savanur, S.A.; Sahoo, B.; Beura, C. Fatigue Failure of a Spiral Bevel Gear in a Typical Low-Bypass Turbofan Engine. In Structural Integrity Assessment; Lecture Notes in Mechanical Engineering; Springer: Singapore, 2020; pp. 639–649. [Google Scholar] [CrossRef]
- Salawu, E.Y.; Ajayi, O.O.; Inegbenebor, A.O. Forensic Investigation of a Failed Intermediate Starwheel Spur Gear Tooth in a Filler Machine. Procedia Manuf. 2019, 35, 97–102. [Google Scholar] [CrossRef]
- Rajinikanth, V.; Soni, M.K.; Mahato, B.; Rao, M.A. Microstructural investigation of rolling contact fatigue (RCF) on a failed planetary gear of a windmill gearbox. Eng. Fail. Anal. 2021, 121, 105167. [Google Scholar] [CrossRef]
- Wang, Q.; Zhu, Y.; Zhang, Z.; Fu, C.; Dong, C.; Su, H. Partial Load: A Key Factor Resulting in the Failure of Gear in the Wind Turbine Gearbox. J. Fail. Anal. Prev. 2016, 16, 109–122. [Google Scholar] [CrossRef]
- Rajinikanth, V.; Soni, M.K.; Mahato, B.; Rao, M.A. Study of microstructural degradation of a failed pinion gear at a cement plant. Eng. Fail. Anal. 2019, 95, 117–126. [Google Scholar] [CrossRef]
- Jonck, J.; Slabbert, G.A. Analysis of a failed spur gear from a Vibro-Hammer. Eng. Fail. Anal. 2013, 34, 511–518. [Google Scholar] [CrossRef]
- Mohammed, O.D.; Rantatalo, M. Gear fault models and dynamics-based modelling for gear fault detection—A review. Eng. Fail. Anal. 2020, 117, 104798. [Google Scholar] [CrossRef]
- Khan, M.A.; Cooper, D.; Starr, A. BS-ISO helical gear fatigue life estimation and wear quantitative feature analysis. Strain 2009, 45, 358–363. [Google Scholar] [CrossRef]
- Lias, M.R.; Rao, T.V.V.L.N.; Awang, M.; Khan, M.A. The stress distribution of gear tooth due to axial misalignment condition. J. Appl. Sci. 2012, 12, 2404–2410. [Google Scholar] [CrossRef]
- Fernandes, P.J.L. Tooth bending fatigue failures in gears. Eng. Fail. Anal. 1996, 3, 219–225. [Google Scholar] [CrossRef]
- Bonaiti, L.; Bayoumi, A.B.M.; Concli, F.; Rosa, F.; Gorla, C. Gear root bending strength: A comparison between single tooth bending fatigue tests and meshing gears. J. Mech. Des. 2021, 143, 103402. [Google Scholar] [CrossRef]
- Onsy, A.; Bicker, R.; Shaw, B. Predictive Health Monitoring of Gear Surface Fatigue Failure Using Model-Based Parametric Method Algorithms; An Experimental Validation. SAE Int. J. Aerosp. 2013, 6, 1–7. [Google Scholar] [CrossRef]
- Loutas, T.H.; Roulias, D.; Pauly, E.; Kostopoulos, V. The combined use of vibration, acoustic emission and oil debris on-line monitoring towards a more effective condition monitoring of rotating machinery. Mech. Syst. Signal Process. 2011, 25, 1339–1352. [Google Scholar] [CrossRef]
- Jamadar, I.M.; Nithin, R.; Nagashree, S.; Prasad, V.P.; Preetham, M.; Samal, P.K.; Singh, S. Spur Gear Fault Detection Using Design of Experiments and Support Vector Machine (SVM) Algorithm. J. Fail. Anal. Prev. 2023, 23, 2014–2028. [Google Scholar] [CrossRef]
- Ozturk, H.; Yesilyurt, I.; Sabuncu, M. Investigation of effectiveness of some vibration-based techniques in early detection of real-time fatigue failure in gears. Shock Vib. 2010, 17, 741–757. [Google Scholar] [CrossRef]
- Khan, M.A.; Starr, A.G. Theoretical and Experimental Working Life Comparison for a Helical Gear under Linear Pitting Failure. Appl. Mech. Mater. 2007, 7–8, 95–100. [Google Scholar] [CrossRef]
- Fernandes, P.J.L.; McDuling, C. Surface contact fatigue failures in gears. Eng. Fail. Anal. 1997, 4, 99–107. [Google Scholar] [CrossRef]
- Weibring, M.; Gondecki, L.; Tenberge, P. Simulation of fatigue failure on tooth flanks in consideration of pitting initiation and growth. Tribol. Int. 2019, 131, 299–307. [Google Scholar] [CrossRef]
- Ding, Y.; Rieger, N.F. Spalling formation mechanism for gears. Wear 2003, 254, 1307–1317. [Google Scholar] [CrossRef]
- Wu, X.; Luo, Y.; Li, Q.; Shi, J. A new analytical model for evaluating the time-varying mesh stiffness of helical gears in healthy and spalling cases. Eng. Fail. Anal. 2022, 131, 105842. [Google Scholar] [CrossRef]
- Kumar, A.; Gandhi, C.P.; Zhou, Y.; Kumar, R.; Xiang, J. Latest developments in gear defect diagnosis and prognosis: A review. Meas. J. Int. Meas. Confed. 2020, 158, 107735. [Google Scholar] [CrossRef]
- Podgornik, B. Adhesive Wear Failures. In Failure Analysis and Prevention; ASM International: Almere, The Netherlands, 2020; pp. 1–20. [Google Scholar] [CrossRef]
- Ognjanovic, M. Progressive Gear Teeth Wear and Failure Probability Modelling. Tribol. Ind. 2004, 26, 44–49. [Google Scholar]
- Feng, K.; Ji, J.C.; Ni, Q.; Beer, M. A review of vibration-based gear wear monitoring and prediction techniques. Mech. Syst. Signal Process. 2023, 182, 109605. [Google Scholar] [CrossRef]
- Goswami, P.; Nandan Rai, R. A systematic review on failure modes and proposed methodology to artificially seed faults for promoting PHM studies in laboratory environment for an industrial gearbox. Eng. Fail. Anal. 2023, 146, 107076. [Google Scholar] [CrossRef]
- Cubillo, A.; Perinpanayagam, S.; Esperon-Miguez, M. A review of physics-based models in prognostics: Application to gears and bearings of rotating machinery. In Advances in Mechanical Engineering; SAGE Publications Inc.: Thousand Oaks, CA, USA, 2016; Volume 8. [Google Scholar] [CrossRef]
- Kundu, P.; Darpe, A.K.; Kulkarni, M.S. A review on diagnostic and prognostic approaches for gears. In Structural Health Monitoring; SAGE Publications Ltd.: Thousand Oaks, CA, USA, 2021; Volume 20, pp. 2853–2893. [Google Scholar] [CrossRef]
- Liang, X.; Zuo, M.J.; Feng, Z. Dynamic modeling of gearbox faults: A review. Mech. Syst. Signal Process. 2018, 98, 852–876. [Google Scholar] [CrossRef]
- Mohammed, O.D.; Rantatalo, M.; Aidanpää, J.O.; Kumar, U. Vibration signal analysis for gear fault diagnosis with various crack progression scenarios. Mech. Syst. Signal Process. 2013, 41, 176–195. [Google Scholar] [CrossRef]
- Marafona, J.D.M.; Marques, P.M.T.; Martins, R.C.; Seabra, J.H.O. Mesh stiffness models for cylindrical gears: A detailed review. Mech. Mach. Theory 2021, 166, 104472. [Google Scholar] [CrossRef]
- Chen, Z.; Shao, Y. Dynamic simulation of spur gear with tooth root crack propagating along tooth width and crack depth. Eng. Fail. Anal. 2011, 18, 2149–2164. [Google Scholar] [CrossRef]
- Mohammed, O.D.; Rantatalo, M.; Aidanpää, J.O. Dynamic modelling of a one-stage spur gear system and vibration-based tooth crack detection analysis. Mech. Syst. Signal Process. 2015, 54–55, 293–305. [Google Scholar] [CrossRef]
- Kumar, V.; Kumar, A.; Kumar, S.; Sarangi, S. TVMS calculation and dynamic analysis of carburized spur gear pair. Mech. Syst. Signal Process. 2022, 166, 108436. [Google Scholar] [CrossRef]
- Luo, Y.; Baddour, N.; Liang, M. Dynamical modeling and experimental validation for tooth pitting and spalling in spur gears. Mech. Syst. Signal Process. 2019, 119, 155–181. [Google Scholar] [CrossRef]
- Saxena, A.; Parey, A.; Chouksey, M. Time varying mesh stiffness calculation of spur gear pair considering sliding friction and spalling defects. Eng. Fail. Anal. 2016, 70, 200–211. [Google Scholar] [CrossRef]
- Dai, H.; Long, X.; Chen, F.; Xun, C. An improved analytical model for gear mesh stiffness calculation. Mech. Mach. Theory 2021, 159, 104262. [Google Scholar] [CrossRef]
- Lei, Y.; Liu, Z.; Wang, D.; Yang, X.; Liu, H.; Lin, J. A probability distribution model of tooth pits for evaluating time-varying mesh stiffness of pitting gears. Mech. Syst. Signal Process. 2018, 106, 355–366. [Google Scholar] [CrossRef]
- Liang, X.H.; Liu, Z.L.; Pan, J.; Zuo, M.J. Spur Gear Tooth Pitting Propagation Assessment Using Model-based Analysis. Chin. J. Mech. Eng. (Engl. Ed.) 2017, 30, 1369–1382. [Google Scholar] [CrossRef]
- Liang, X.; Zhang, H.; Liu, L.; Zuo, M.J. The influence of tooth pitting on the mesh stiffness of a pair of external spur gears. Mech. Mach. Theory 2016, 106, 1–15. [Google Scholar] [CrossRef]
- Yakeu Happi, K.H.; Tchomeni Kouejou, B.X.; Anyika Alugongo, A. Experimental study and comparative analysis of pitting fault in spur gear system. J. Vibroengi. 2023, 25, 1480–1501. [Google Scholar] [CrossRef]
- Liang, X.; Zuo, M.J.; Feng, Z.; Liu, L. A mesh stiffness evaluation model to reflect tooth pitting growth of a pair of external spur gears. In Proceedings of the 2016 Prognostics and System Health Management Conference, PHM-Chengdu 2016, Chengdu, China, 19–21 October 2016; Institute of Electrical and Electronics Engineers Inc.: Piscataway, NJ, USA, 2017. [Google Scholar] [CrossRef]
- Qu, Y.; Zhang, H.; Hong, L.; Tan, Y.; Zhou, Z. Dynamic Modeling and Fault Feature Analysis of Pitted Gear System. In Proceedings of the 2018 IEEE International Conference on Prognostics and Health Management, ICPHM 2018, Seattle, WA, USA, 11–13 June 2018; Institute of Electrical and Electronics Engineers Inc.: Piscataway, NJ, USA, 2018. [Google Scholar] [CrossRef]
- Liang, X.; Pan, J.; Liu, Z.; Zuo, M.J. Dynamic Modeling of Gear Tooth Pitting Propagation to Neighbouring and Mating Teeth; Dynamic Modeling of Gear Tooth Pitting Propagation to Neighbouring and Mating Teeth. In Proceedings of the 2018 IEEE International Conference on Sensing, Diagnostics, Prognostics, and Control, Xi’an, China, 15–17 August 2018. [Google Scholar] [CrossRef]
- Liu, J.; Wang, C.; Wu, W. Research on Meshing Stiffness and Vibration Response of Pitting Fault Gears with Different Degrees. Int. J. Rotating Mach. 2020, 2020, 4176430. [Google Scholar] [CrossRef]
- Meng, Z.; Wang, F.; Shi, G. A novel evolution model of pitting failure and effect on time -varying meshing stiffness of spur gears. Eng. Fail. Anal. 2021, 120, 105068. [Google Scholar] [CrossRef]
- Hou, J.; Yang, S.; Li, Q.; Liu, Y. Effect of a Novel Tooth Pitting Model on Mesh Stiffness and Vibration Response of Spur Gears. Mathematics 2022, 10, 471. [Google Scholar] [CrossRef]
- Liu, W.; Zhu, R.; Zhou, W.; Shang, Y. Probability distribution model of gear time-varying mesh stiffness with random pitting of tooth surface. Eng. Fail. Anal. 2021, 130, 105782. [Google Scholar] [CrossRef]
- Chen, T.; Wang, Y.; Chen, Z. A novel distribution model of multiple teeth pits for evaluating time-varying mesh stiffness of external spur gears. Mech. Syst. Signal Process. 2019, 129, 479–501. [Google Scholar] [CrossRef]
- Meng, F.; Xia, H.; Zhang, X.; Wang, J. A new tooth pitting modeling method based on matrix equation for evaluating time-varying mesh stiffness. Eng. Fail. Anal. 2022, 142, 106799. [Google Scholar] [CrossRef]
- El Yousfi, B.; Soualhi, A.; Medjaher, K.; Guillet, F. New approach for gear mesh stiffness evaluation of spur gears with surface defects. Eng. Fail. Anal. 2020, 116, 104740. [Google Scholar] [CrossRef]
- Ma, R.; Chen, Y.; Cao, Q. Research on dynamics and fault mechanism of spur gear pair with spalling defect. J. Sound Vib. 2012, 331, 2097–2109. [Google Scholar] [CrossRef]
- Wan, Z.; Zheng, J.; Li, J.; Man, Z. Fault feature analysis of gear tooth spalling based on dynamic simulation and experiments. Materials 2021, 14, 6053. [Google Scholar] [CrossRef] [PubMed]
- Luo, W.; Qiao, B.; Shen, Z.; Yang, Z.; Cao, H.; Chen, X. Investigation on the influence of spalling defects on the dynamic performance of planetary gear sets with sliding friction. Tribol. Int. 2021, 154, 106639. [Google Scholar] [CrossRef]
- Xiang, L.; An, C.; Zhang, Y.; Hu, A. Failure dynamic modelling and analysis of planetary gearbox considering gear tooth spalling. Eng. Fail. Anal. 2021, 125, 105444. [Google Scholar] [CrossRef]
- Luo, W.; Qiao, B.; Shen, Z.; Yang, Z.; Chen, X. Time-varying mesh stiffness calculation of a planetary gear set with the spalling defect under sliding friction. Meccanica 2020, 55, 245–260. [Google Scholar] [CrossRef]
- Cui, L.; Liu, T.; Huang, J.; Wang, H. Improvement on meshing stiffness algorithms of gear with peeling. Symmetry 2019, 11, 609. [Google Scholar] [CrossRef]
- Wang, F.; Dai, P.; Yan, S.; Wang, J.; Niu, L. Investigation on double shock in vibration response of the gear pair with spalling defects. Proc. Inst. Mech. Eng. C J. Mech. Eng. Sci. 2023, 237, 4882–4894. [Google Scholar] [CrossRef]
- Wang, S.; Zhu, R. An improved mesh stiffness calculation model of spur gear pair under mixed EHL friction with spalling effect. Vibroeng. Procedia 2020, 33, 176–181. [Google Scholar] [CrossRef]
- Chen, Y.; Jin, Y.; Kang, R.; Gong, W.; Yang, Y. The Time-varying Mesh Stiffness Modeling of Gear System with Spalling Defects in Different Positions. In Proceedings of the 2017 4th International Conference on Transportation Information and Safety (ICTIS): Conference Proceedings, Banff, AB, Canada, 8–10 August 2017; pp. 658–663. [Google Scholar]
- Shi, L.; Wen, J.; Pan, B.; Xiang, Y.; Zhang, Q.; Lin, C. Dynamic characteristics of a gear system with double-teeth spalling fault and its fault feature analysis. Appl. Sci. 2020, 10, 7058. [Google Scholar] [CrossRef]
- Yu, W.; Mechefske, C.K.; Timusk, M. A new dynamic model of a cylindrical gear pair with localized spalling defects. Nonlinear Dyn. 2018, 91, 2077–2095. [Google Scholar] [CrossRef]
- Wang, L.; Deng, C.; Xu, J.; Yin, L.; Yu, W.; Ding, X.; Shao, Y.; Huang, W.; Yang, X. Effects of spalling fault on dynamic responses of gear system considering three-dimensional line contact elasto-hydrodynamic lubrication. Eng. Fail. Anal. 2022, 132, 105930. [Google Scholar] [CrossRef]
- Yang, L.; Chen, Q.; Yin, L.; Wang, L.; Shao, Y. Dynamic characteristic of spur gear system with spalling fault considering tooth pitch error. Qual. Reliab. Eng. Int. 2022, 38, 2921–2938. [Google Scholar] [CrossRef]
- Luo, Y.; Baddour, N.; Liang, M. Evaluation of time-varying mesh stiffness of gears with tooth spalls modeled as spherical shapes. In Proceedings of the Proceedings-2018 Prognostics and System Health Management Conference, PHM-Chongqing 2018, Chongqing, China, 23–25 October 2018; Institute of Electrical and Electronics Engineers Inc.: Piscataway, NJ, USA, 2019; pp. 94–99. [Google Scholar] [CrossRef]
- Cheng, Z.; Huang, K.; Xiong, Y.; Sang, M. Dynamic Analysis of a High-Contact-Ratio Spur Gear System with Localized Spalling and Experimental Validation. Machines 2022, 10, 154. [Google Scholar] [CrossRef]
- Luo, Y.; Baddour, N.; Han, G.; Jiang, F.; Liang, M. Evaluation of the time-varying mesh stiffness for gears with tooth spalls with curved-bottom features. Eng. Fail. Anal. 2018, 92, 430–442. [Google Scholar] [CrossRef]
- Huangfu, Y.; Chen, K.; Ma, H.; Li, X.; Han, H.; Zhao, Z. Meshing and dynamic characteristics analysis of spalled gear systems: A theoretical and experimental study. Mech. Syst. Signal Process. 2020, 139, 106640. [Google Scholar] [CrossRef]
- Meng, Z.; Shi, G.; Wang, F. Vibration response and fault characteristics analysis of gear based on time-varying mesh stiffness. Mech. Mach. Theory 2020, 148, 103786. [Google Scholar] [CrossRef]
- Doğan, O.; Karpat, F. Crack detection for spur gears with asymmetric teeth based on the dynamic transmission error. Mech. Mach. Theory 2019, 133, 417–431. [Google Scholar] [CrossRef]
- Chaari, F.; Fakhfakh, T.; Haddar, M. Analytical modelling of spur gear tooth crack and influence on gearmesh stiffness. Eur. J. Mech.-A/Solids 2009, 28, 461–468. [Google Scholar] [CrossRef]
- Chen, Z.; Zhai, W.; Shao, Y.; Wang, K.; Sun, G. Analytical model for mesh stiffness calculation of spur gear pair with non-uniformly distributed tooth root crack. Eng. Fail. Anal. 2016, 66, 502–514. [Google Scholar] [CrossRef]
- Ning, J.; Chen, Z.; Wang, Y.; Li, Y.; Zhai, W. Vibration feature of spur gear transmission with non-uniform depth distribution of tooth root crack along tooth width. Eng. Fail. Anal. 2021, 129, 105713. [Google Scholar] [CrossRef]
- Cui, L.; Huang, J.; Zhai, H.; Zhang, F. Research on the meshing stiffness and vibration response of fault gears under an angle-changing crack based on the universal equation of gear profile. Mech. Mach. Theory 2016, 105, 554–567. [Google Scholar] [CrossRef]
- Onkareshwar, M.; Inturi, V.; Rajendra, S.P.; Penumakala, P.K.; Sabareesh, G.R. Effect of local gear tooth failures on gear mesh stiffness and vibration response of a single-stage spur gear pair. In Proceedings of the 14th International Conference on Vibration Problems, Crete, Greece, 1–4 September 2019; Lecture Notes in Mechanical Engineering. Springer Science and Business Media Deutschland GmbH: Berlin/Heidelberg, Germany, 2021; pp. 1095–1103. [Google Scholar] [CrossRef]
- Mohammed, O.D.; Rantatalo, M. Dynamic response and time-frequency analysis for gear tooth crack detection. Mech. Syst. Signal Process. 2016, 66–67, 612–624. [Google Scholar] [CrossRef]
- Inturi, V.; Penumakala, P.K.; Sabareesh, G.R. Effect of Multiple Defects and Multi-component Failure on the Dynamic Behaviour of a Wind Turbine Gearbox. Arab. J. Sci. Eng. 2022, 47, 8969–8983. [Google Scholar] [CrossRef]
- Yang, L.T.; Shao, Y.M.; Jiang, W.W.; Zhang, L.K.; Wang, L.M.; Xu, J. Effects of tooth surface crack propagation on meshing stiffness and vibration characteristic of spur gear system. Appl. Sci. 2021, 11, 1968. [Google Scholar] [CrossRef]
- Liu, Y.; Shi, Z.; Shen, G.; Zhen, D.; Wang, F.; Gu, F. Evaluation model of mesh stiffness for spur gear with tooth tip chipping fault. Mech. Mach. Theory 2021, 158, 104238. [Google Scholar] [CrossRef]
- Chaari, F.; Baccar, W.; Abbes, M.S.; Haddar, M. Effect of spalling or tooth breakage on gearmesh stiffness and dynamic response of a one-stage spur gear transmission. Eur. J. Mech. A/Solids 2008, 27, 691–705. [Google Scholar] [CrossRef]
- Zhang, L.; Shao, Y. Mesh Stiffness Calculation of Spur Gears with Tooth Surface Crack. In Proceedings of the ASME 2019 International Design Engineering Technical Conferences and Computers and Information in Engineering Conference IDETC/CIE2019, Anaheim, CA, USA, 18–21 August 2019; Available online: http://asmedigitalcollection.asme.org/IDETC-CIE/proceedings-pdf/IDETC-CIE2019/59308/V010T11A011/6454683/v010t11a011-detc2019-97857.pdf (accessed on 18 February 2024).
- Onishchenko, V. Tooth wear modeling and prognostication parameters of engagement of spur gear power transmissions. Mech. Mach. Theory 2008, 43, 1639–1664. [Google Scholar] [CrossRef]
- Osman, T.; Velex, P. Static and dynamic simulations of mild abrasive wear in wide-faced solid spur and helical gears. Mech. Mach. Theory 2010, 45, 911–924. [Google Scholar] [CrossRef]
- Feng, K.; Borghesani, P.; Smith, W.A.; Randall, R.B.; Chin, Z.Y.; Ren, J.; Peng, Z. Vibration-based updating of wear prediction for spur gears. Wear 2019, 426–427, 1410–1415. [Google Scholar] [CrossRef]
- Shen, Z.; Yang, L.; Qiao, B.; Luo, W.; Chen, X.; Yan, R. Mesh relationship modeling and dynamic characteristic analysis of external spur gears with gear wear. Front. Mech. Eng. 2022, 17, 9. [Google Scholar] [CrossRef]
- Shen, Z.; Qiao, B.; Yang, L.; Luo, W.; Yang, Z.; Chen, X. Fault mechanism and dynamic modeling of planetary gear with gear wear. Mech. Mach. Theory 2021, 155, 104098. [Google Scholar] [CrossRef]
- Wang, Y.; Li, K.; Qiao, B.; Shen, Z.; Chen, X. Theoretical Investigation of Mesh Relationship and Mesh Stiffness of Internal Spur Gears with Tooth Wear. Appl. Sci. 2023, 13, 2022. [Google Scholar] [CrossRef]
- Dong, N.; Cui, Q.; Zhou, J.; Tong, R.; Wang, H.; Lu, F. Study on wear evolution of spur gears considering dynamic meshing stiffness. J. Mech. Sci. Technol. 2023, 37, 3393–3408. [Google Scholar] [CrossRef]
- Ren, J.; Yuan, H. A Dynamic Wear Prediction Model for Studying the Interactions between Surface Wear and Dynamic Response of Spur Gears. Coatings 2022, 12, 1250. [Google Scholar] [CrossRef]
- Zhao, J.; Yu, X.; Sheng, W.; Li, Z.; Zhang, H.; Zhu, R. Effect of Machined Tooth Surface Mixed Lubrication Sliding Wear on Gear Dynamic Characteristics. Machines 2023, 11, 25. [Google Scholar] [CrossRef]
- Chen, W.; Lei, Y.; Fu, Y.; Hou, L. A study of effects of tooth surface wear on time-varying mesh stiffness of external spur gear considering wear evolution process. Mech. Mach. Theory 2021, 155, 104055. [Google Scholar] [CrossRef]
- Chang, L.; Yu, Q.; Jeng, Y.R. Modeling and Analysis of High-Load and High-Speed Involute Spur Gear Systems in Adverse Lubrication. Tribol. Trans. 2018, 61, 325–334. [Google Scholar] [CrossRef]
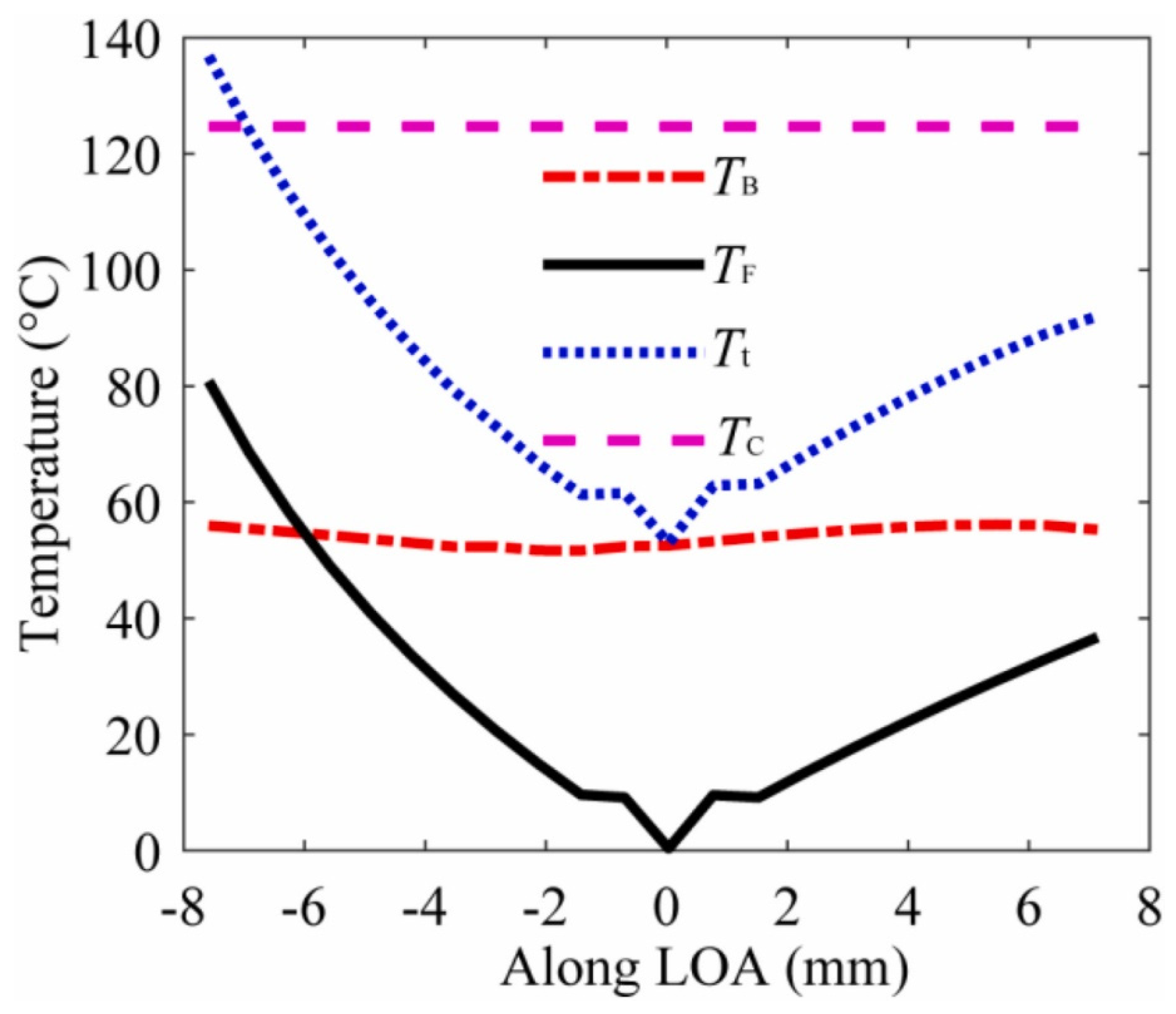
- Li, S.; Kahraman, A. A scuffing model for spur gear contacts. Mech. Mach. Theory 2021, 156, 104161. [Google Scholar] [CrossRef]
- Zhou, C.; Xing, M.; Wang, H.; Hu, B. A novel thermal network model for predicting the contact temperature of spur gears. Int. J. Therm. Sci. 2021, 161, 106703. [Google Scholar] [CrossRef]
- Chen, T.; Zhu, C.; Liu, H.; Wei, P.; Zhu, J.; Xu, Y. Simulation and experiment of carburized gear scuffing under oil jet lubrication. Eng. Fail. Anal. 2022, 139, 106406. [Google Scholar] [CrossRef]
- Li, W.; Tian, J. Unsteady-state temperature field and sensitivity analysis of gear transmission. Tribol. Int. 2017, 116, 229–243. [Google Scholar] [CrossRef]
- Xiao, Z.; Zhou, C.; Li, Z.; Zheng, M. Thermo-mechanical characteristics of high-speed and heavy-load modified gears with elasto-hydrodynamic contacts. Tribol. Int. 2019, 131, 406–414. [Google Scholar] [CrossRef]
- Li, S.; Kolivand, A.; Wei, J. Determination of Critical Temperature of Scuffing for AISI 8620 Steel Gear Contacts Lubricated by Dexron 6 through Computational Simulation of Experiment. J. Tribol. 2022, 144, 081201. [Google Scholar] [CrossRef]
- Ouyang, T.; Huang, H.; Zhang, N.; Mo, C.; Chen, N. A model to predict tribo-dynamic performance of a spur gear pair. Tribol. Int. 2017, 116, 449–459. [Google Scholar] [CrossRef]
- Chang, J.; Liu, S.; Hu, X.; Dai, Y. Evolution of surface spur gear tooth temperature based on three-dimensional finite element model. J. Braz. Soc. Mech. Sci. Eng. 2019, 41, 370. [Google Scholar] [CrossRef]
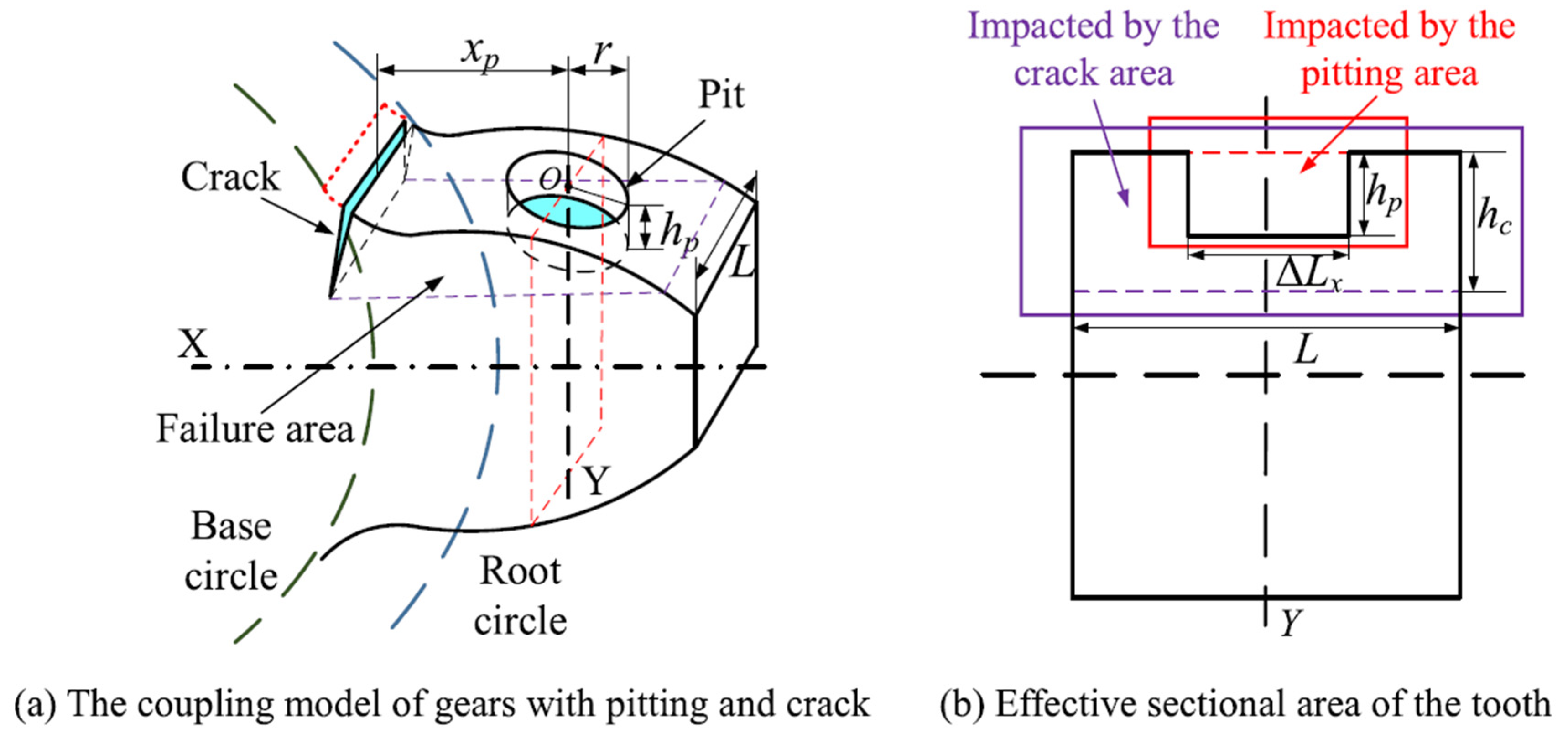
- Ouyang, T.; Wang, G.; Cheng, L.; Wang, J.; Yang, R. Comprehensive diagnosis and analysis of spur gears with pitting-crack coupling faults. Mech. Mach. Theory 2022, 176, 104968. [Google Scholar] [CrossRef]
- Zai, B.A.; Khan, M.A.; Khan, K.A.; Mansoor, A. A novel approach for damage quantification using the dynamic response of a metallic beam under thermo-mechanical loads. J. Sound Vib. 2020, 469, 115134. [Google Scholar] [CrossRef]
- Zai, B.A.; Khan, M.A.; Khan, S.Z.; Asif, M.; Khan, K.A.; Saquib, A.N.; Mansoor, A.; Shahzad, M.; Mujtaba, A. Prediction of Crack Depth and Fatigue Life of an Acrylonitrile Butadiene Styrene Cantilever Beam Using Dynamic Response. J. Test. Eval. 2020, 48, 1520–1536. [Google Scholar] [CrossRef]
- He, F.; Thakur, V.K.; Khan, M. Evolution and new horizons in modeling crack mechanics of 3D printing polymeric structures. Mater. Today Chem. 2021, 20, 100393. [Google Scholar] [CrossRef]
- Ma, H.; Zeng, J.; Feng, R.; Pang, X.; Wen, B. An improved analytical method for mesh stiffness calculation of spur gears with tip relief. Mech. Mach. Theory 2016, 98, 64–80. [Google Scholar] [CrossRef]















Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Oreavbiere, A.; Khan, M. Mathematical Complexities in Modelling Damage in Spur Gears. Machines 2024, 12, 346. https://doi.org/10.3390/machines12050346
Oreavbiere A, Khan M. Mathematical Complexities in Modelling Damage in Spur Gears. Machines. 2024; 12(5):346. https://doi.org/10.3390/machines12050346
Chicago/Turabian StyleOreavbiere, Aselimhe, and Muhammad Khan. 2024. "Mathematical Complexities in Modelling Damage in Spur Gears" Machines 12, no. 5: 346. https://doi.org/10.3390/machines12050346
APA StyleOreavbiere, A., & Khan, M. (2024). Mathematical Complexities in Modelling Damage in Spur Gears. Machines, 12(5), 346. https://doi.org/10.3390/machines12050346





