Influence of Machine Tool Operating Conditions on the Resulting Circularity and Positioning Accuracy
Abstract
:1. Introduction
2. Materials and Methods
2.1. State of the Art and Research Approach
- -
- Geometric accuracy
- -
- Positioning accuracy
- -
- Circular interpolation accuracy
- -
- Volumetric accuracy
- -
- Machining accuracy
- -
- Production accuracy
- Accuracy of circular interpolation
- Machining tools errors
- -
- Geometric and kinematic errors
- -
- Machine assembly-induced errors
- -
- Errors induced by thermal distortions
- -
- Dynamic errors
- -
- Cutting force-induced errors
- -
- Fixture dependent errors
2.2. Experimental Setup
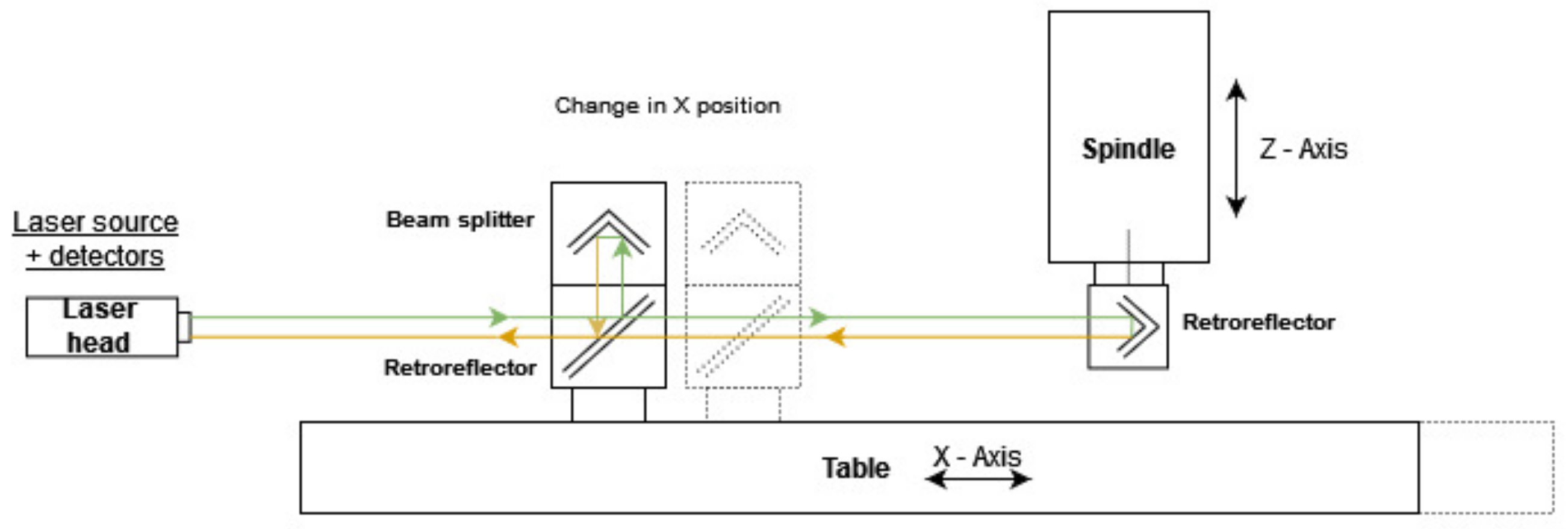
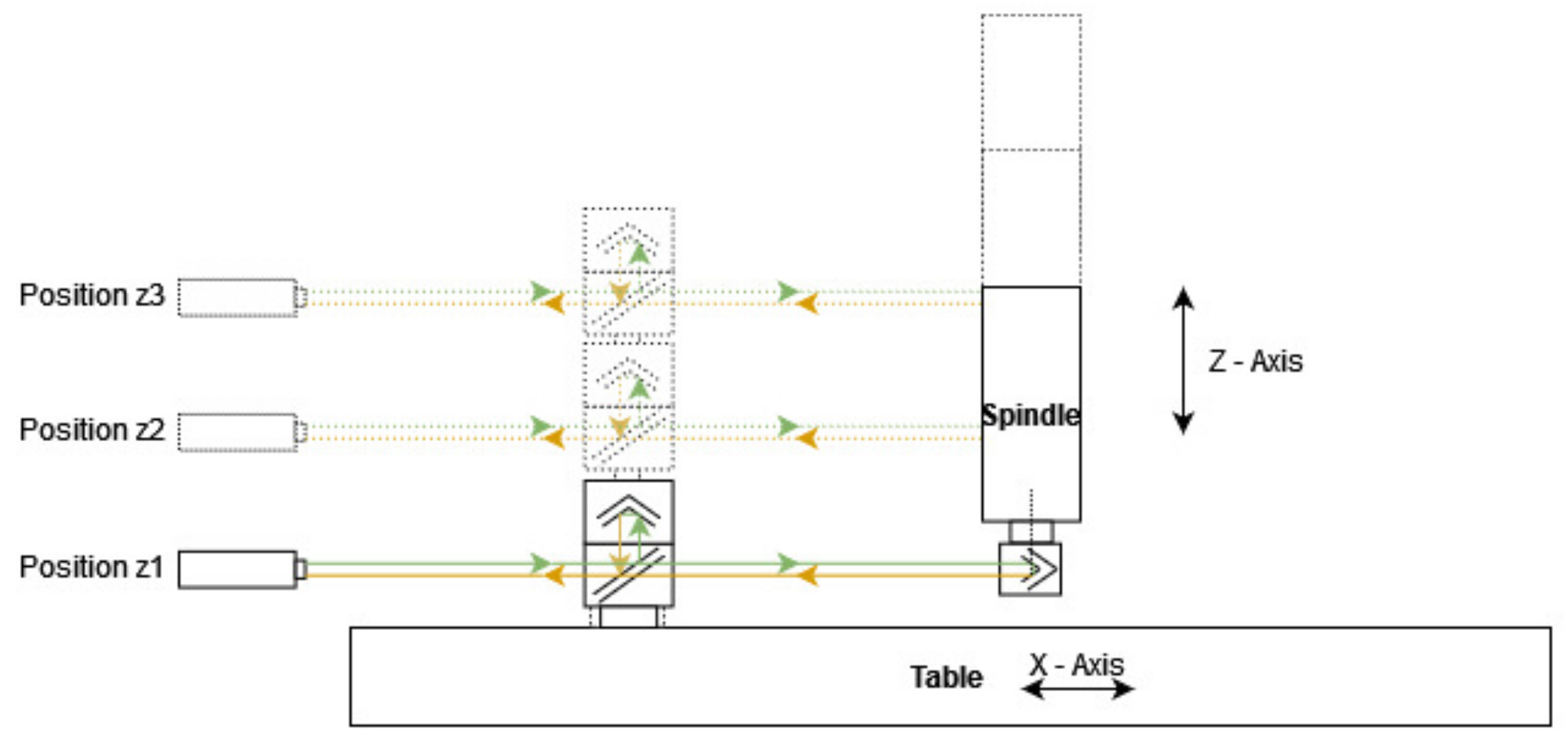
2.2.1. Measurement Setup 1
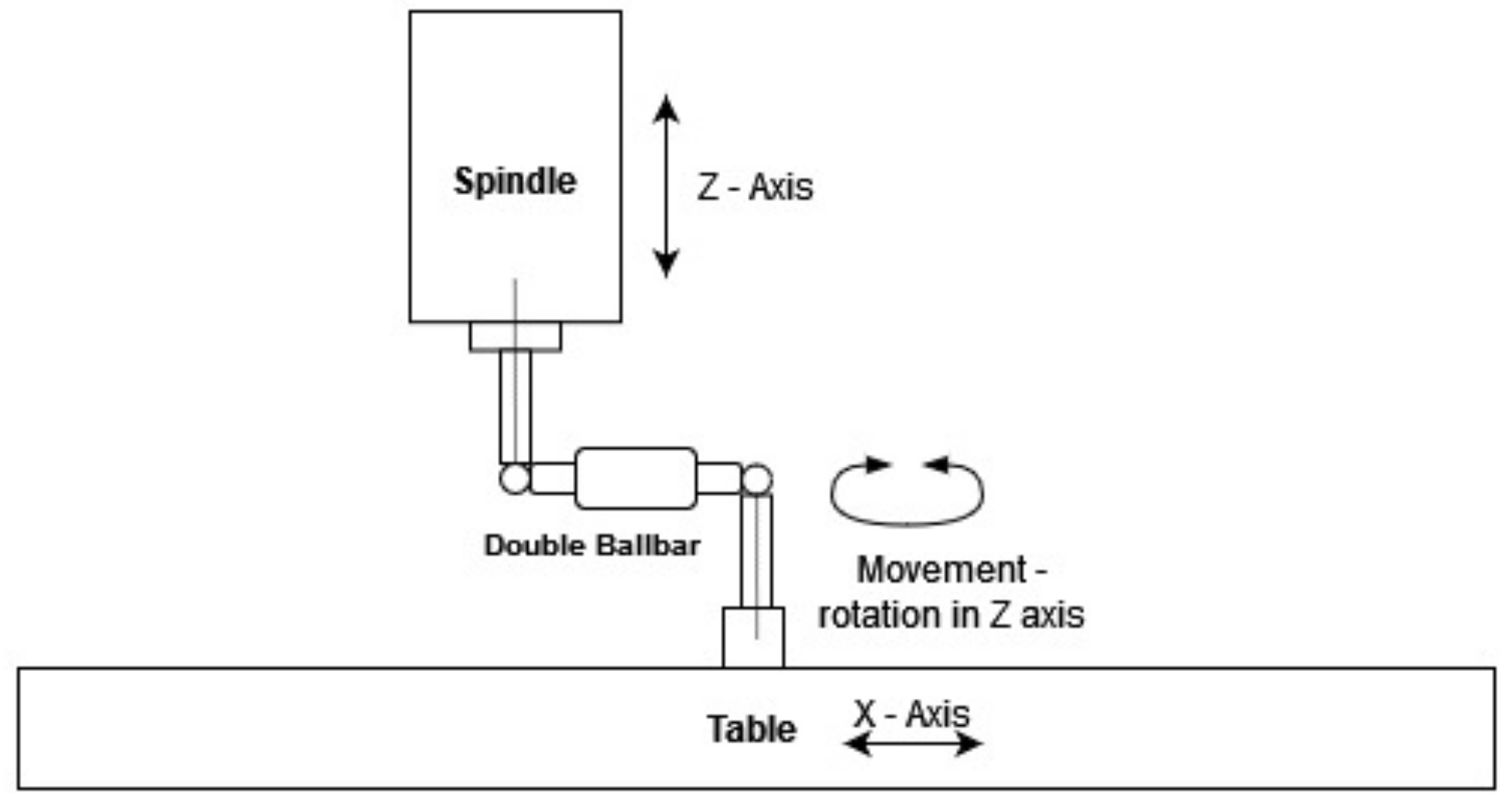
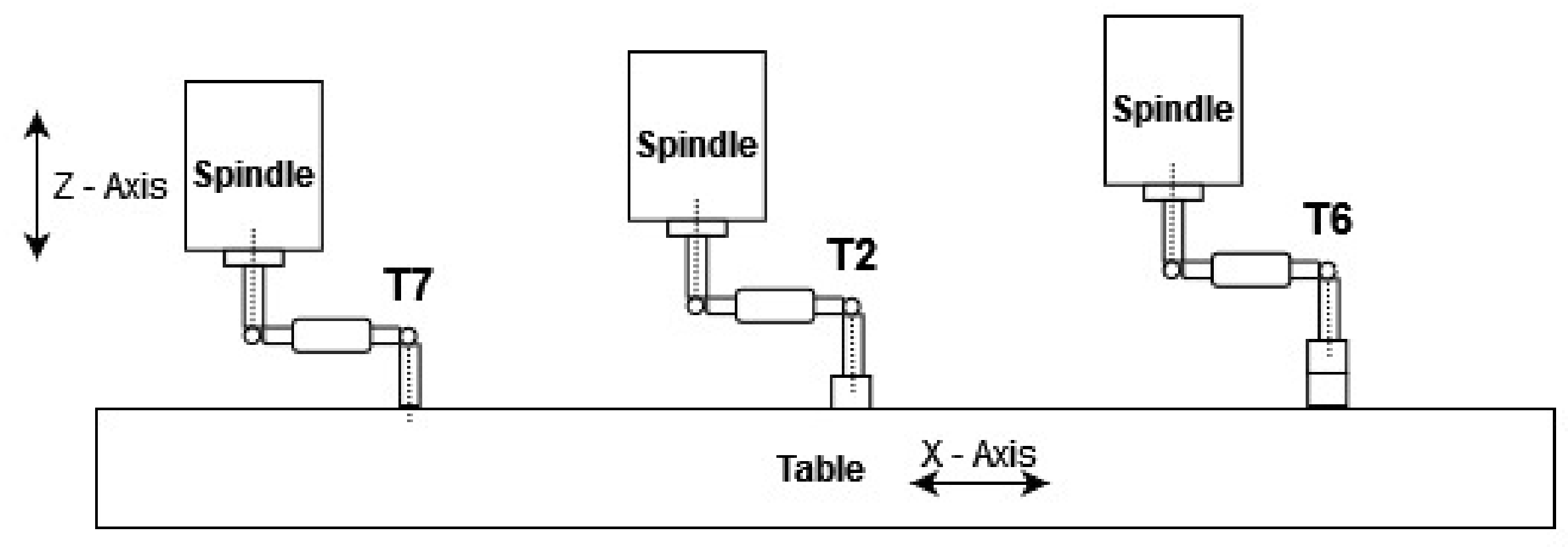
2.2.2. Measurement Setup 2
2.2.3. Measurement Setup 3
2.2.4. Measurement Setup 4
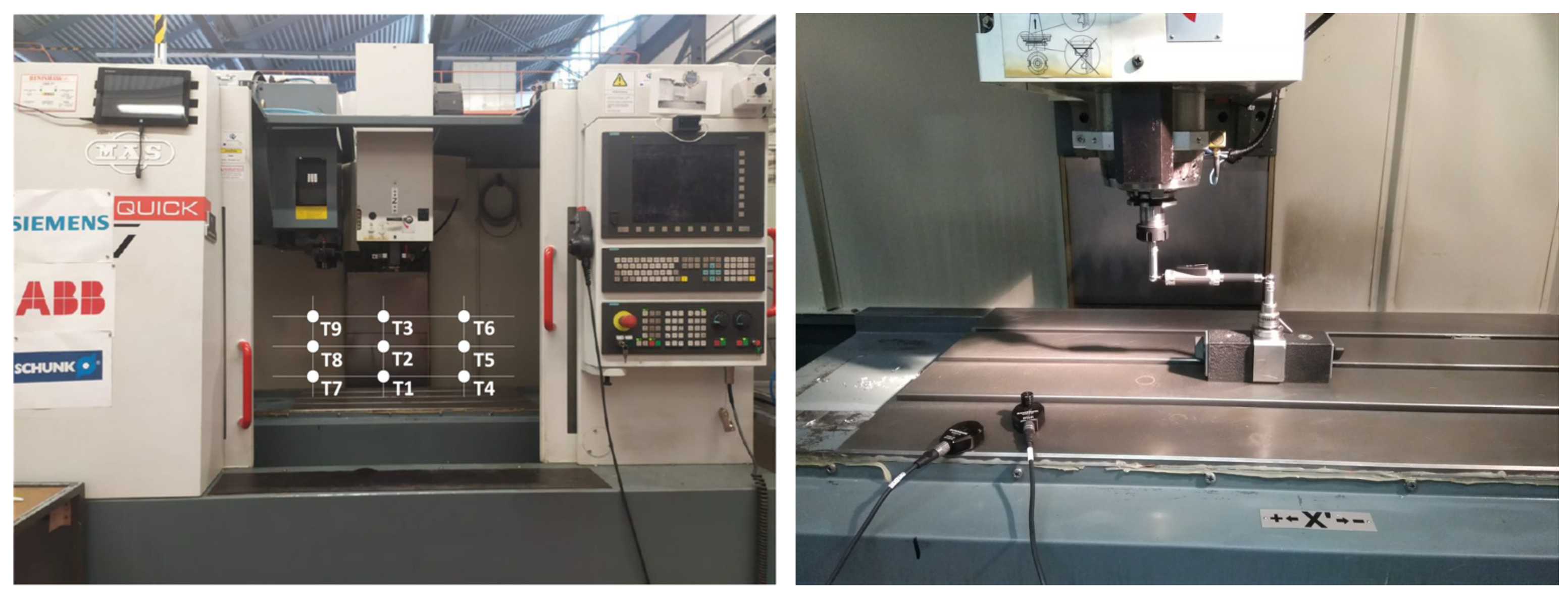
2.3. Demonstrator
2.4. Measurement Devices
3. Results
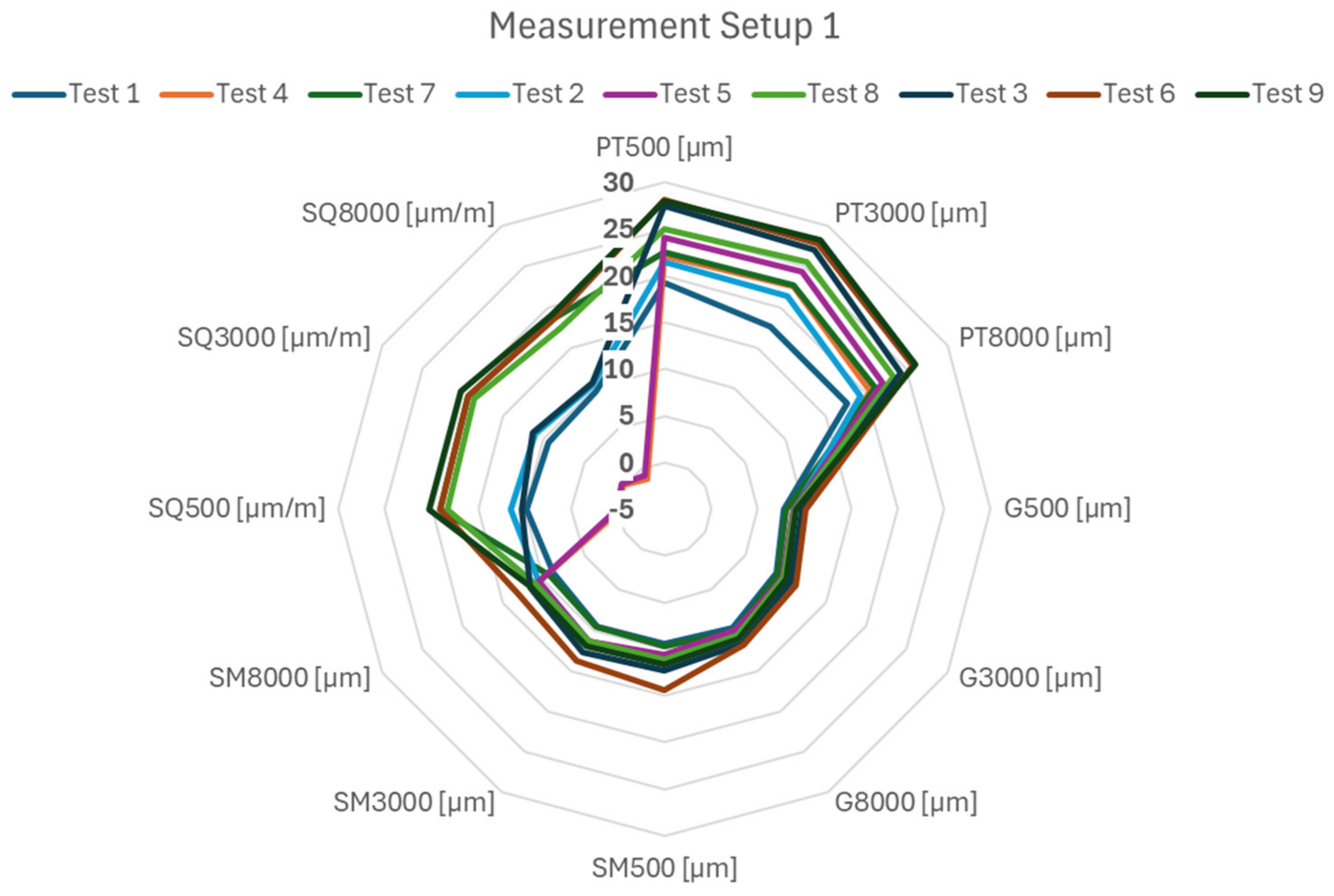
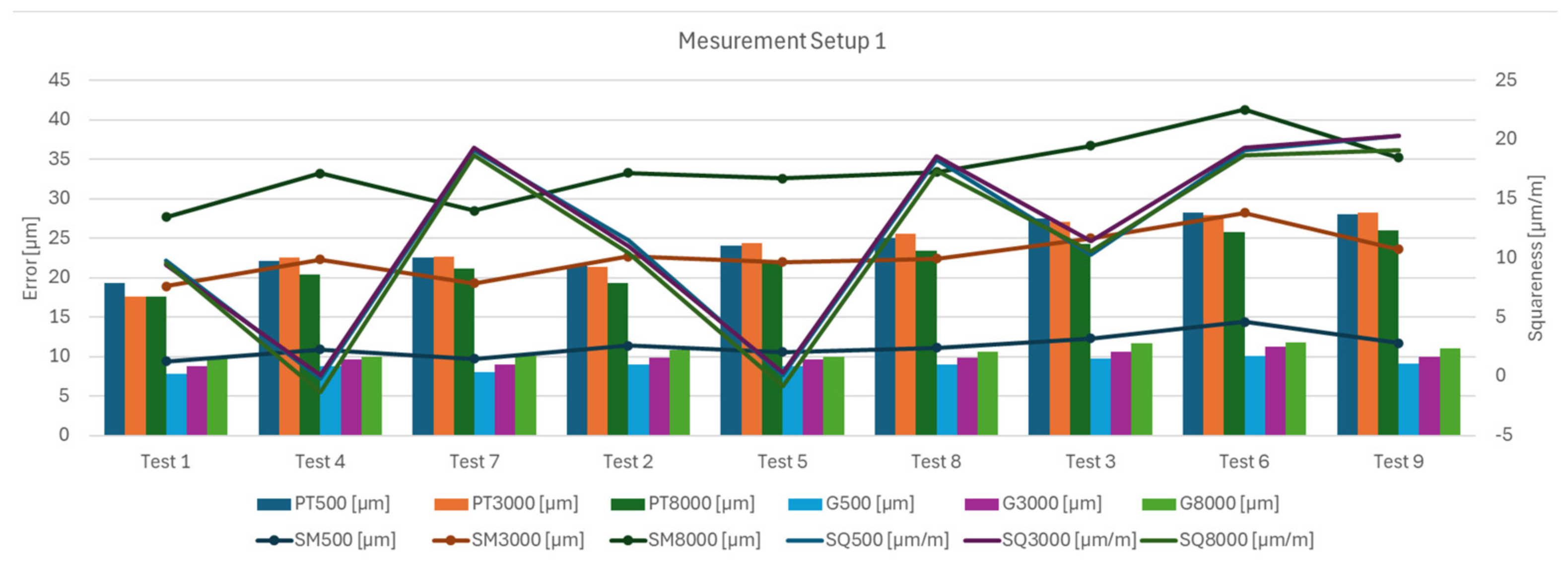
3.1. Measurement Setup 1
3.2. Measurement Setup 2
3.3. Measurement Setup 3
3.4. Measurement Setup 4
3.5. Prediction of Geometric Accuracy
4. Discussion
5. Conclusions
- -
- operating conditions such as feed rate, workpiece position, and machine setup influence the final behaviour of the CNC machine tool;
- -
- the modifications were monitored on the parameters set by the ISO 230-2 and ISO 230-4 procedures;
- -
- the influence of temperature (heat sources) was also observed in the results;
- -
- the results can be further used to predict the dimensional and form accuracy of workpieces under finishing machining conditions.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Dostal, P.; Sadilek, M.; Dubsky, J.; Szkandera, P. Accuracy of Machine Tools. MM Sci. J. 2020, 2020, 3832–3836. [Google Scholar] [CrossRef]
- Gao, W.; Ibaraki, S.; Donmez, M.A.; Kono, D.; Mayer, J.R.R.; Chen, Y.L.; Szipka, K.; Archenti, A.; Linares, J.M.; Suzuki, N. Machine Tool Calibration: Measurement, Modeling, and Compensation of Machine Tool Errors. Int. J. Mach. Tools Manuf. 2023, 187, 104017. [Google Scholar] [CrossRef]
- Schwenke, H.; Knapp, W.; Haitjema, H.; Weckenmann, A.; Schmitt, R.; Delbressine, F. Geometric Error Measurement and Compensation of Machines—An Update. CIRP Ann. 2008, 57, 660–675. [Google Scholar] [CrossRef]
- ISO 230-2; Test Code for Machine Tools—Part 2: Determination of Accuracy and Repeatability of Positioning of Numerically Controlled Axes. International Organization for Standardization: Geneva, Switzerland, 2014.
- Zhang, Z.; Jiang, F.; Luo, M.; Wu, B.; Zhang, D.; Tang, K. Geometric Error Measuring, Modeling, and Compensation for CNC Machine Tools: A Review. Chin. J. Aeronaut. 2024, 37, 163–198. [Google Scholar] [CrossRef]
- ISO 230-4; Test Code for Machine Tools—Part 4: Circular Tests for Numerically Controlled Machine Tools. International Organization for Standardization: Geneva, Switzerland, 2005.
- Bryan, J.B. A Simple Method for Testing Measuring Machines and Machine Tools Part 1: Principles and Applications. Precis. Eng. 1982, 4, 61–69. [Google Scholar] [CrossRef]
- Wang, Z.; Wang, D.; Yu, S.; Li, X.; Dong, H. A Reconfigurable Mechanism Model for Error Identification in the Double Ball Bar Tests of Machine Tools. Int. J. Mach. Tools Manuf. 2021, 165, 103737. [Google Scholar] [CrossRef]
- Holub, M.; Jankovych, R.; Vetiska, J.; Sramek, J.; Blecha, P.; Smolik, J.; Heinrich, P. Experimental Study of the Volumetric Error Effect on the Resulting Working Accuracy—Roundness. Appl. Sci. 2020, 10, 6233. [Google Scholar] [CrossRef]
- Zheng, F.; Zhang, B.; Zhao, Y.; Li, J.; Long, F.; Feng, Q. Efficient Method for Identifying Key Errors Based on 21-Geometric-Error Measurement of Three Linear Axes of Machine Tools. Appl. Sci. 2024, 14, 2982. [Google Scholar] [CrossRef]
- Wang, W.; Yue, S.; Yang, H.; Xu, K.; Sun, T.; Lu, K.; Chen, Z.; Wang, C.; Cui, X.; Ju, B. Fast Detection of Geometric Errors for Three-Axis Machine Tools with Combined Double-Ball Bars Based on Spatial Circle Detection. Meas. Sci. Technol. 2024, 35, 035003. [Google Scholar] [CrossRef]
- Archenti, A. Prediction of Machined Part Accuracy from Machining System Capability. CIRP Ann. 2014, 63, 505–508. [Google Scholar] [CrossRef]
- Ramesh, R.; Mannan, M.A.; Poo, A.N. Error Compensation in Machine Tools—A Review: Part I: Geometric, Cutting-Force Induced and Fixture-Dependent Errors. Int. J. Mach. Tools Manufact. 2000, 40, 1235–1256. [Google Scholar] [CrossRef]
- VDI/DGQ 3441; Statistical Testing of the Operational and Positional Accuracy of Machine Tools. Verein Deutscher Ingenieure (VDI): Dusseldorf, Germany, 1982.
- Okafor, A.C.; Ertekin, Y.M. Derivation of Machine Tool Error Models and Error Compensation Procedure for Three Axes Vertical Machining Center Using Rigid Body Kinematics. Int. J. Mach. Tools Manuf. 2000, 40, 1199–1213. [Google Scholar] [CrossRef]
- Peterka, J.; Kuruc, M.; Kolesnyk, V.; Dehtiarov, I.; Moravcikova, J.; Vopat, T.; Pokorny, P.; Jurina, F.; Simna, V. Selected Aspects of Precision Machining on CNC Machine Tools. Machines 2023, 11, 946. [Google Scholar] [CrossRef]
- Holub, M.; Blecha, P.; Bradac, F.; Kana, R. Volumetric Compensation of Three-Axis Vertical Machining Centre. MM Sci. J. 2015, 2015, 677–681. [Google Scholar] [CrossRef]
- Wiessner, M.; Blaser, P.; Böhl, S.; Mayr, J.; Knapp, W.; Wegener, K. Thermal Test Piece for 5-Axis Machine Tools. Precis. Eng. 2018, 52, 407–417. [Google Scholar] [CrossRef]
- Mareš, M.; Horejš, O.; Havlík, L. Thermal Error Compensation of a 5-Axis Machine Tool Using Indigenous Temperature Sensors and CNC Integrated Python Code Validated with a Machined Test Piece. Precis. Eng. 2020, 66, 21–30. [Google Scholar] [CrossRef]
- Usop, Z.; Sarhan, A.A.D.; Mardi, N.A.; Wahab, M.N.A. Measuring of Positioning, Circularity and Static Errors of a CNC Vertical Machining Centre for Validating the Machining Accuracy. Measurement 2015, 61, 39–50. [Google Scholar] [CrossRef]
- Quan, L.; Zhao, W. A Review on Positioning Uncertainty in Motion Control for Machine Tool Feed Drives. Precis. Eng. 2024, 88, 428–448. [Google Scholar] [CrossRef]
- Paweł, M.; Bartosz, P. Rapid Method to Determine Accuracy and Repeatability of Positioning of Numerically Controlled Axes. Int. J. Mach. Tools Manuf. 2019, 137, 1–12. [Google Scholar] [CrossRef]
- Renishaw. XL-80 and XC-80 Error Budget and Uncertainty Calculations; Renishaw: Wotton-under-Edge, UK, 2013. [Google Scholar]
- Renishaw. QC20-W Error Budget & Uncertainty Calculations; Renishaw: Wotton-under-Edge, UK, 2013. [Google Scholar]





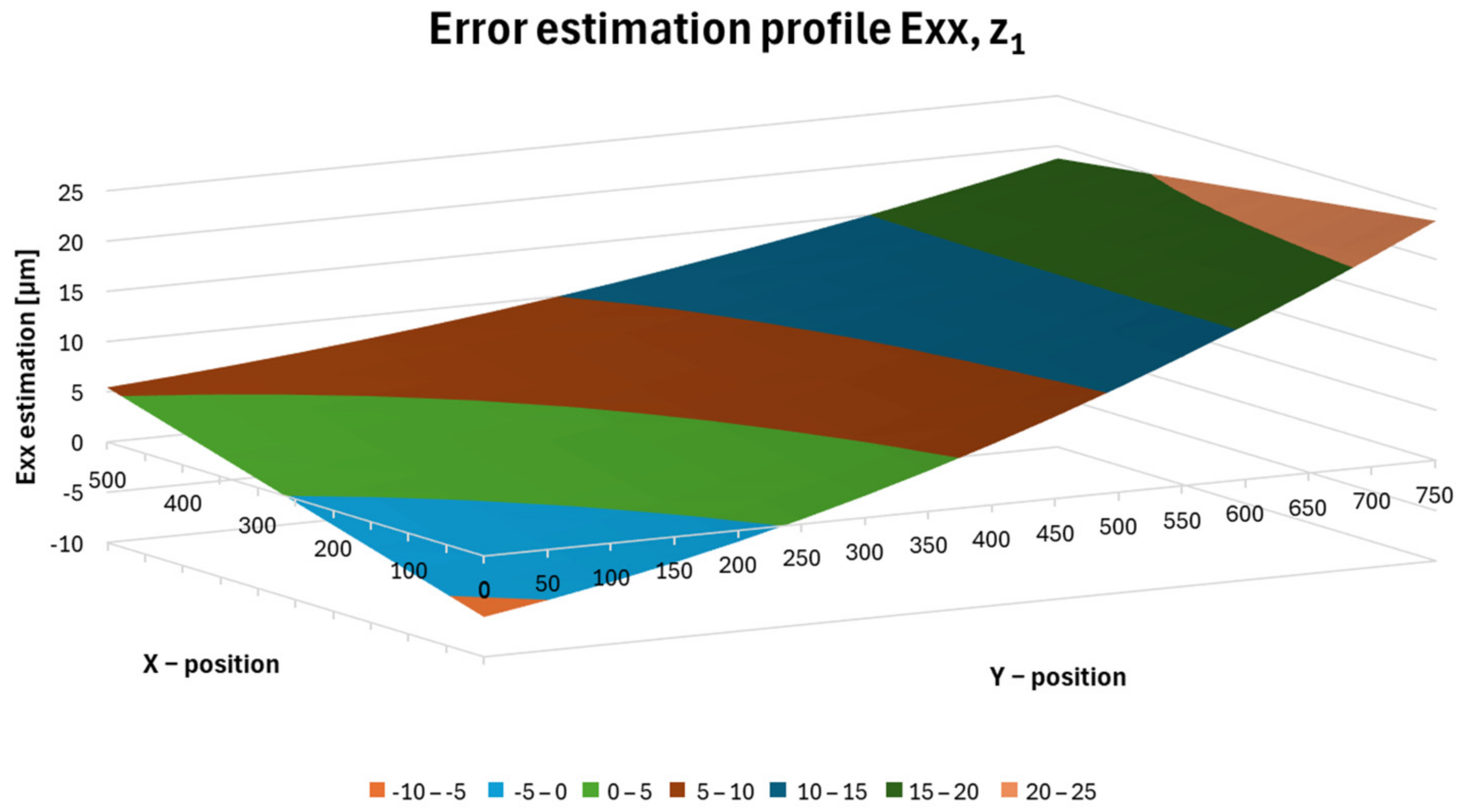




| Working range | X axis [mm] | 754 |
| Y axis [mm] | 500 | |
| Z axis [mm] | 550 | |
| Accuracy | Positioning accuracy [mm] | 0.012 |
| Repeatability [mm] | 0.005 | |
| Spindle | Maximal RPM [min−1] | 10,000 |
| Feed | Working feed [mm/min] | 1–30,000 |
| Rapid feed [mm/min] | 30,000 |
| Laser frequency accuracy | ±0.05 ppm |
| Linear measurement accuracy | ±0.5 ppm |
| Linear resolution | 0.1 nm |
| Expanded uncertainty (k = 2) | 0.486 ppm |
| Estimate of expanded uncertainty | 0.2 µm + 0.3 × L µm/m |
| Range of measurement | 80 m |
| Maximal velocity | 4 m/s |
| Sensor resolution | 0.1 µm |
| Estimate of expanded uncertainty | (0.70 μm + 0.30% L) µm |
| Range of measurement | ±1.0 mm |
| Maximal sample rate | 1000 Hz |
| Position [mm] | Nr. | Feed Rate f [mm/min] | Positioning Tolerance [µm] | Circularity [µm] | Scaling Mismatch [µm] | Squareness [µm] | |
|---|---|---|---|---|---|---|---|
| Test 1 | X = 397, Y = 254, Z = −460 | 1 | 500 | 19.3 | 7.8 | 9.4 | 9.8 |
| 2 | 3000 | 17.6 | 8.8 | 9.5 | 9.4 | ||
| 3 | 8000 | 17.6 | 9.7 | 8.8 | 9.6 | ||
| Test 2 | X = 397, Y = 254, Z = −405 | 4 | 500 | 21.5 | 9.0 | 11.4 | 11.5 |
| 5 | 3000 | 21.4 | 9.8 | 11.3 | 11.0 | ||
| 6 | 8000 | 19.3 | 10.8 | 10.6 | 10.4 | ||
| Test 3 | X = 397, Y = 254, Z = −350 | 7 | 500 | 27.5 | 9.7 | 12.3 | 10.3 |
| 8 | 3000 | 27.1 | 10.6 | 12.7 | 11.4 | ||
| 9 | 8000 | 24.3 | 11.7 | 11.7 | 10.6 | ||
| Test 4 | X = 600, Y = 254, Z = −460 | 10 | 500 | 22.1 | 8.8 | 10.9 | −0.2 |
| 11 | 3000 | 22.6 | 9.6 | 11.4 | 0.1 | ||
| 12 | 8000 | 20.4 | 10.0 | 10.9 | −1.3 | ||
| Test 5 | X = 600, Y = 254, Z = −405 | 13 | 500 | 24.1 | 8.8 | 10.6 | 0.1 |
| 14 | 3000 | 24.4 | 9.6 | 11.4 | 0.3 | ||
| 15 | 8000 | 22.0 | 10.0 | 10.6 | −0.8 | ||
| Test 6 | X = 600, Y = 254, Z = −350 | 16 | 500 | 28.2 | 10.1 | 14.4 | −1.3 |
| 17 | 3000 | 27.9 | 11.3 | 13.8 | −1.6 | ||
| 18 | 8000 | 25.8 | 11.8 | 13.1 | −2.4 | ||
| Test 7 | X = 152, Y = 254, Z = −460 | 19 | 500 | 22.5 | 8.0 | 9.7 | 19.1 |
| 20 | 3000 | 22.7 | 9.0 | 9.6 | 19.3 | ||
| 21 | 8000 | 21.1 | 10.1 | 9.2 | 18.7 | ||
| Test 8 | X = 152, Y = 254, Z = −405 | 22 | 500 | 25.0 | 9.0 | 11.1 | 18.3 |
| 23 | 3000 | 25.6 | 9.8 | 11.3 | 18.6 | ||
| 24 | 8000 | 23.4 | 10.6 | 11.0 | 17.4 | ||
| Test 9 | X = 152, Y = 254, Z = −350 | 25 | 500 | 28.0 | 9.1 | 11.7 | 20.3 |
| 26 | 3000 | 28.3 | 10.0 | 11.9 | 20.3 | ||
| 27 | 8000 | 26.0 | 11.0 | 11.6 | 19.1 |
| Position 1 | Position 2 | Position 3 | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Position Z [mm] | −460 | −405 | −350 | ||||||
| Feed rate f [mm/min] | 500 | 3000 | 8000 | 500 | 3000 | 8000 | 500 | 3000 | 8000 |
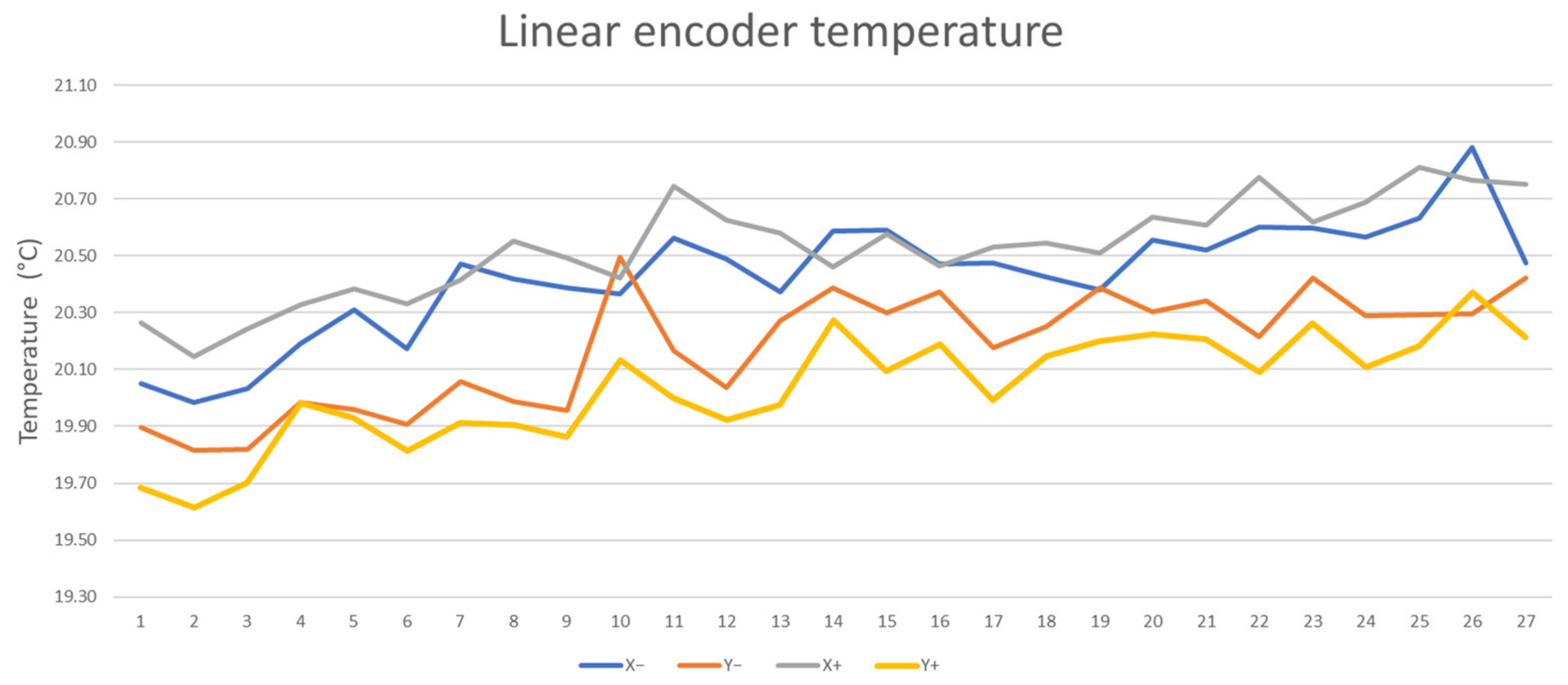
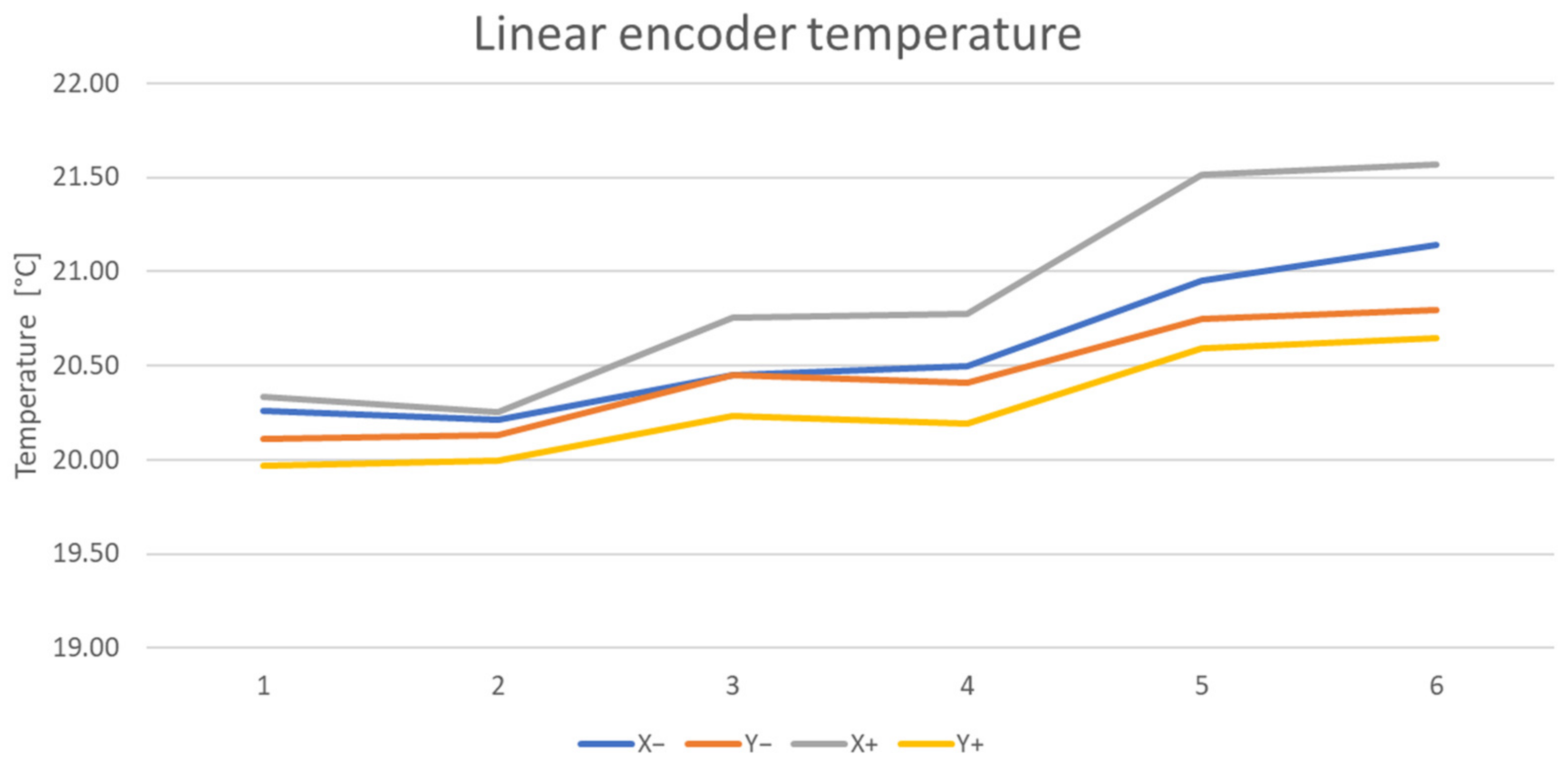
| Temperature X − [°C] | 19.7 | 19.6 | 19.7 | 19.7 | 19.6 | 19.6 | 19.8 | 19.9 | 19.9 |
| Temperature X + [°C] | 19.5 | 19.6 | 19.6 | 19.7 | 19.7 | 19.6 | 20.0 | 20.1 | 20.2 |
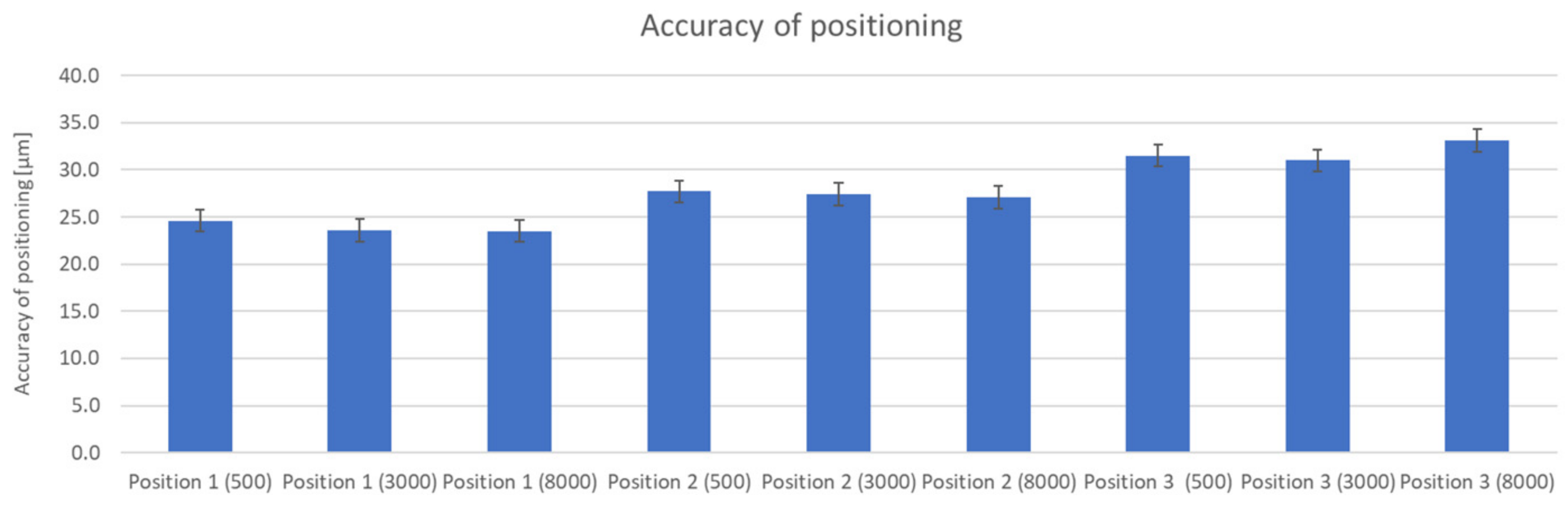
| Accuracy of positioning A [µm] | 24.6 | 23.6 | 23.5 | 27.7 | 27.4 | 27.1 | 31.5 | 31.0 | 33.1 |
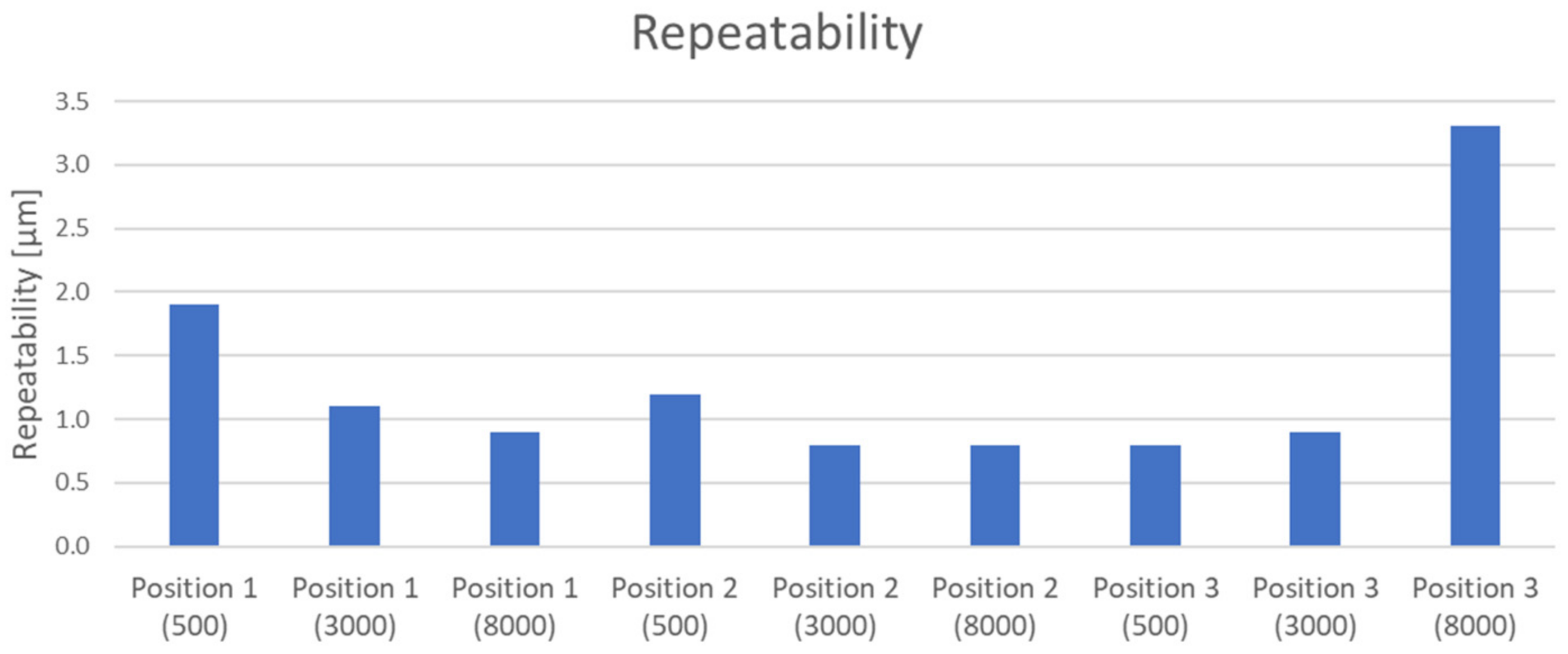
| Repeatability R [µm] | 1.9 | 1.1 | 0.9 | 1.2 | 0.8 | 0.8 | 0.8 | 0.9 | 3.3 |
| Systematic positioning deviation E [µm] | 23.6 | 23.3 | 23.1 | 27.4 | 27.0 | 26.9 | 31.1 | 30.8 | 32.5 |
| Reversal value B [µm] | 0.4 | 0.4 | 0.3 | 0.3 | 0.3 | 0.3 | 0.2 | 0.4 | 2.7 |
| Mean positioning deviation M [µm] | 0.3 | 0.2 | 0.2 | 0.2 | 0.2 | 0.2 | 0.1 | 0.2 | 2.0 |
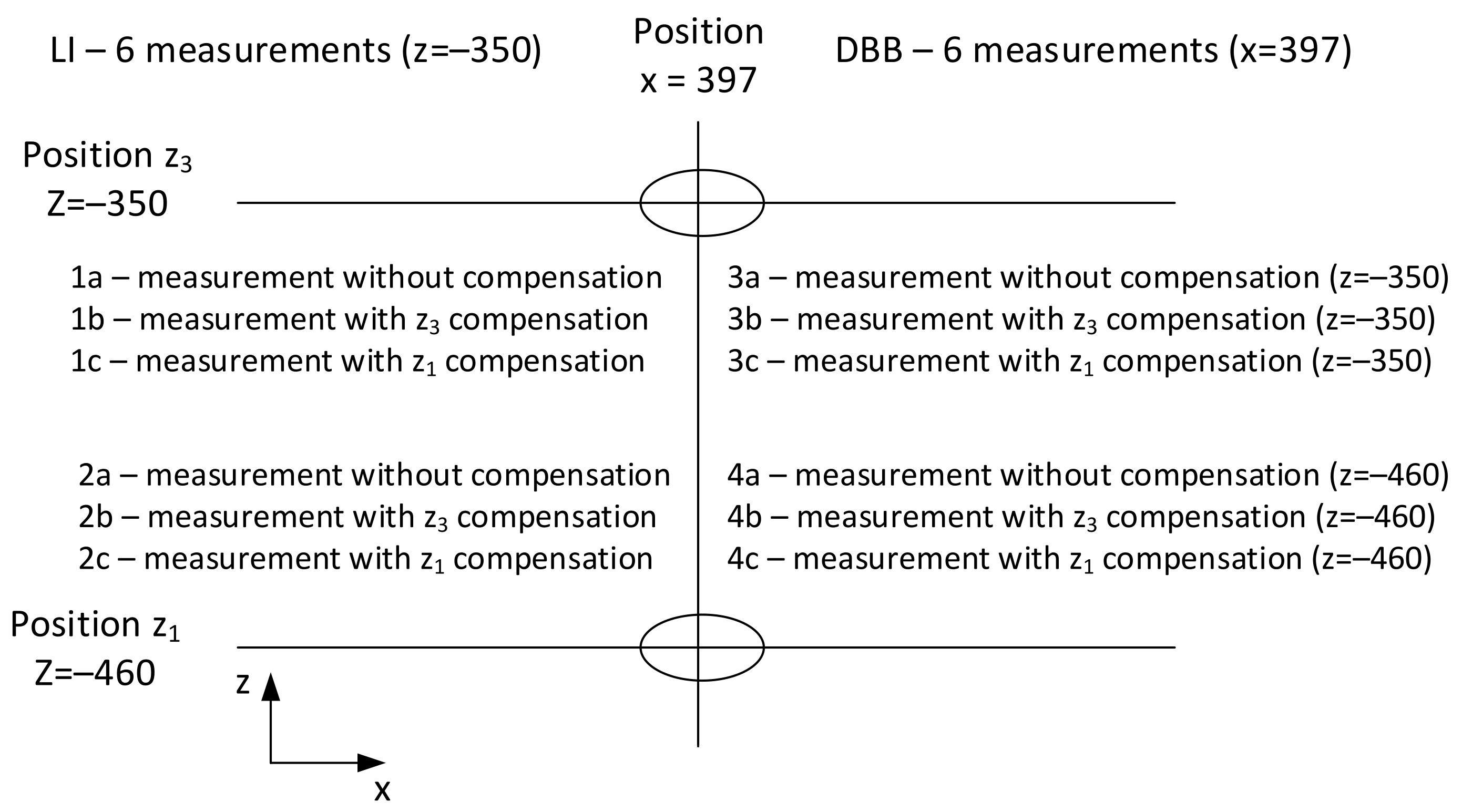
| Position z3 (1a, 1b, 1c) | Position z1 (2a, 2b, 2c) | |||||
|---|---|---|---|---|---|---|
| Position Z [mm] | −350 | −460 | ||||
| Feed rate f [mm/min] | 3000 | 3000 | ||||
| Compensation [-] | off | on by z3 | z1 | off | z3 | on by z1 |
| Temperature X − [°C] | 20.5 | 20.6 | 20.6 | 20.5 | 20.6 | 20.7 |
| Temperature X + [°C] | 20.4 | 20.6 | 20.7 | 20.6 | 20.6 | 20.8 |
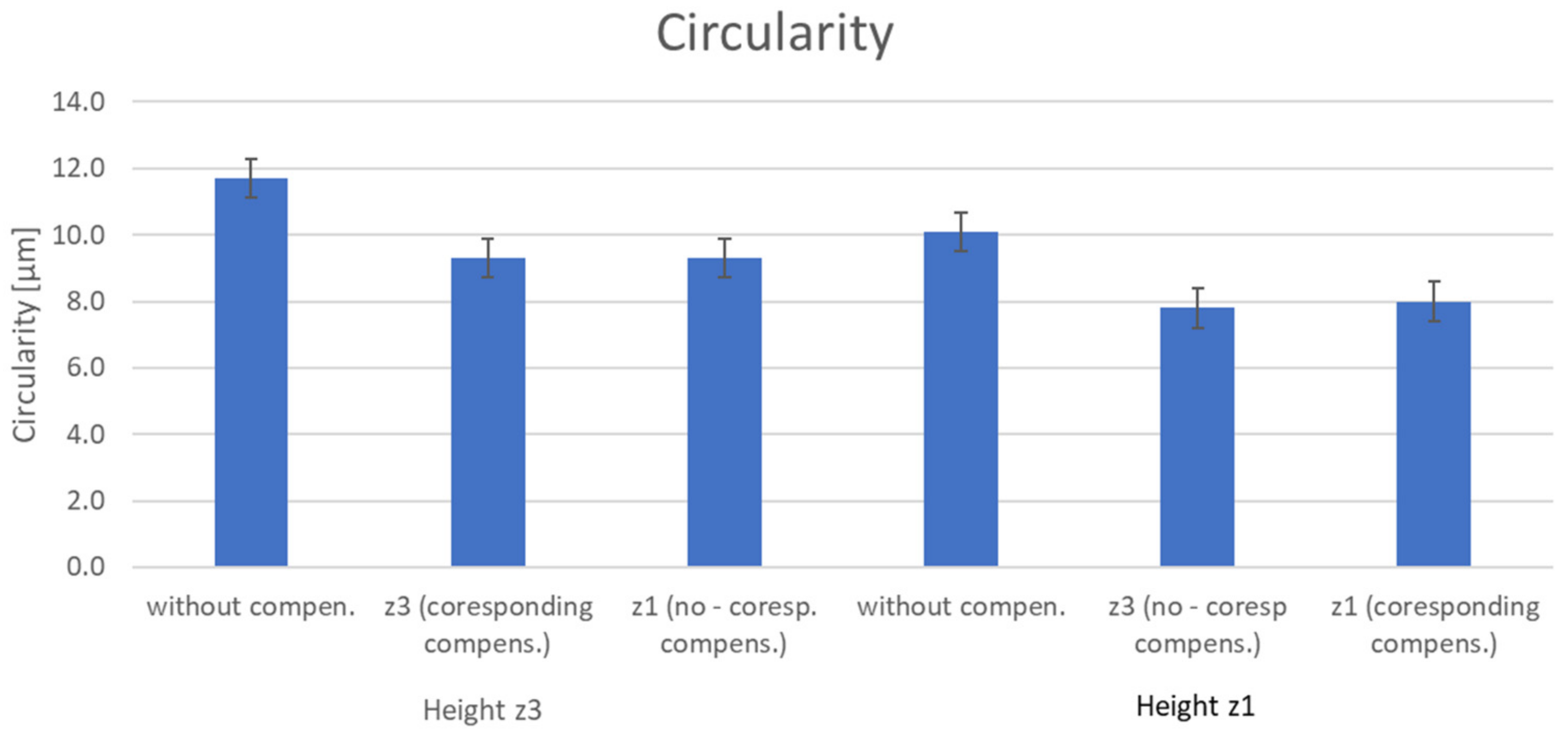
| Accuracy of positioning A [µm] | 29.5 | 1.4 | 7.7 | 21.4 | 7.6 | 1.4 |
| Repeatability R [µm] | 2.5 | 1.0 | 1.4 | 0.9 | 0.8 | 1.0 |
| Systematic positioning deviation E [µm] | 28.3 | 1.1 | 6.9 | 21.1 | 7.3 | 1.0 |
| Reversal value B [µm] | 0.5 | 0.3 | 0.4 | 0.3 | 0.3 | 0.3 |
| Mean positioning deviation M [µm] | 0.3 | 0.2 | 0.2 | 0.2 | 0.2 | 0.2 |
| Nr. of Measurement | Position z3 (3a, 3b, 3c) | Position z1 (4a, 4b, 4c) | ||||
|---|---|---|---|---|---|---|
| Position X, Y, Z [mm] | 397, 254, −350 | 397, 254, −460 | ||||
| Feed rate f [mm/min] | 3000 | 3000 | ||||
| Compensation [-] | off | on by z3 | z1 | off | z3 | on by z1 |
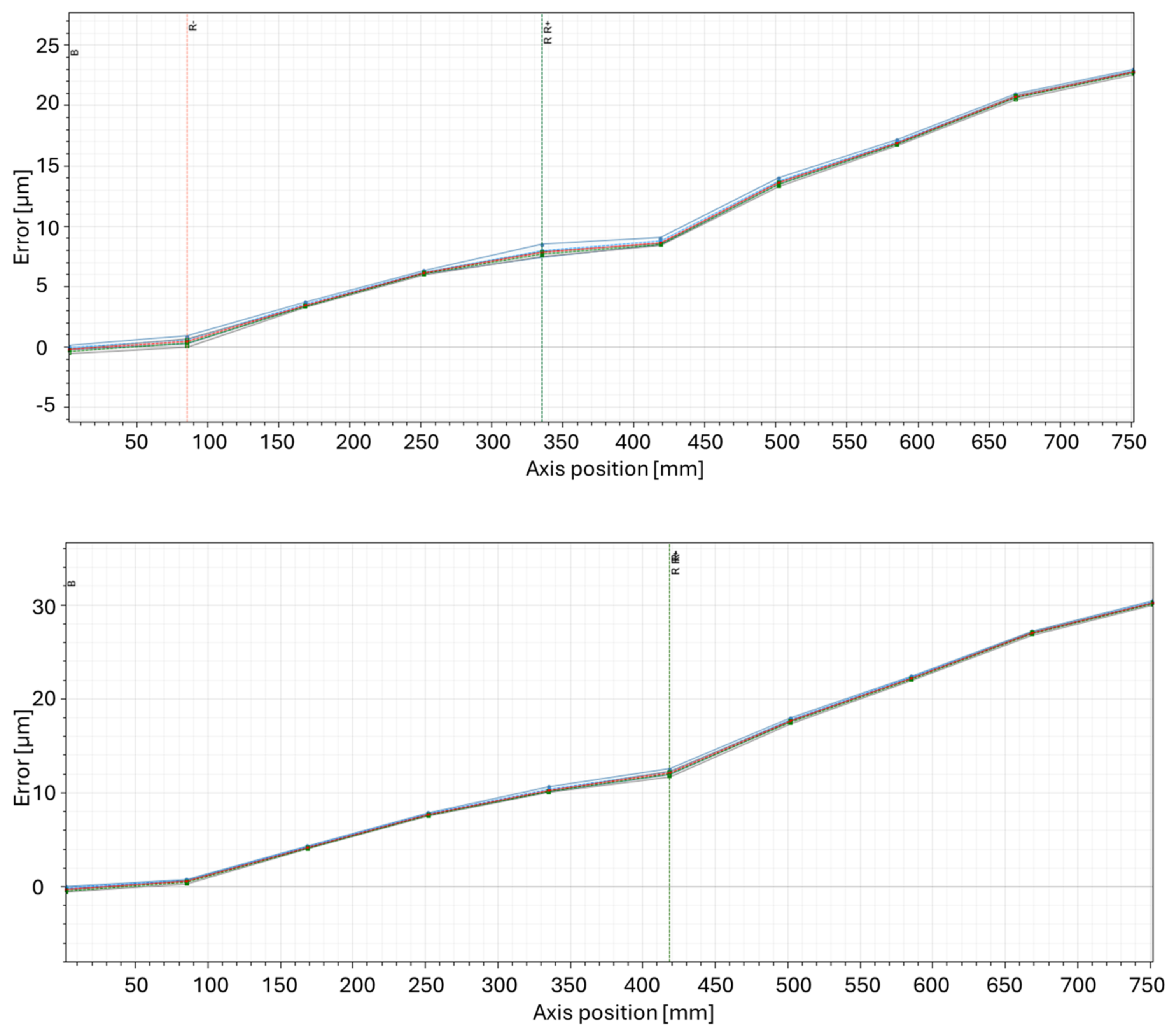
| Positioning tolerance [µm] | 22.4 | 12.9 | 14.1 | 19.4 | 17.3 | 14.7 |
| Circularity [µm] | 11.7 | 9.3 | 9.3 | 10.1 | 7.8 | 8.0 |
| Scaling mismatch [µm] | 13.3 | 2.8 | 4.6 | 12.0 | 1.6 | 2.8 |
| Squareness [µm/m] | 6.8 | 6.9 | 6.8 | 9.4 | 9.1 | 9.4 |
| Maes. t0 | Meas. t1 | Meas. T2 | ||||
|---|---|---|---|---|---|---|
| Position Z [mm] | −350 | −460 | −460 | |||
| Feed rate f [mm/min] | 3000 | 3000 | 3000 | |||
| Compensation [-] | off | on | off | on | off | on |
| Temperature X − [°C] | 20.1 | 20.2 | 20.6 | 20.6 | 20.9 | 20.9 |
| Temperature X + [°C] | 20.2 | 20.2 | 20.6 | 20.8 | 21.6 | 21.3 |
| Accuracy of positioning A [µm] | 22.7 | 1.7 | 21.3 | 1.4 | 20.5 | 1.3 |
| Repeatability R [µm] | 0.7 | 0.9 | 0.7 | 1.2 | 0.7 | 0.8 |
| Systematic positioning deviation E [µm] | 22.3 | 1.4 | 21.0 | 1.0 | 20.1 | 0.8 |
| Reversal value B [µm] | 0.3 | 0.0 | 0.2 | 0.3 | 0.2 | 0.2 |
| Mean positioning deviation M [µm] | 0.2 | 0.2 | 0.2 | 0.2 | 0.1 | 0.1 |
| Maes. t0 | Meas. t1 | Meas. t2 | ||||
|---|---|---|---|---|---|---|
| Position X, Z [mm] | 397, −460 | 397, −460 | 397, −460 | |||
| Feed rate f [mm/min] | 3000 | 3000 | 3000 | |||
| Compensation [-] | off | on | off | on | off | on |
| Positioning tolerance [µm] | 18.7 | 12.6 | 20.7 | 9.8 | 22.9 | 9.7 |
| Circularity [µm] | 11.3 | 8.5 | 10.7 | 7.3 | 10.6 | 6.6 |
| Scaling mismatch [µm] | 11.4 | 3.8 | 11.5 | 3.0 | 11.9 | 3.3 |
| Squareness [µm/m] | 7.7 | 9.1 | 8.8 | 9.1 | 9.1 | 9.3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sarvas, M.; Holub, M.; Marek, T.; Prochazka, J.; Bradac, F.; Blecha, P. Influence of Machine Tool Operating Conditions on the Resulting Circularity and Positioning Accuracy. Machines 2024, 12, 352. https://doi.org/10.3390/machines12050352
Sarvas M, Holub M, Marek T, Prochazka J, Bradac F, Blecha P. Influence of Machine Tool Operating Conditions on the Resulting Circularity and Positioning Accuracy. Machines. 2024; 12(5):352. https://doi.org/10.3390/machines12050352
Chicago/Turabian StyleSarvas, Matej, Michal Holub, Tomas Marek, Jan Prochazka, Frantisek Bradac, and Petr Blecha. 2024. "Influence of Machine Tool Operating Conditions on the Resulting Circularity and Positioning Accuracy" Machines 12, no. 5: 352. https://doi.org/10.3390/machines12050352
APA StyleSarvas, M., Holub, M., Marek, T., Prochazka, J., Bradac, F., & Blecha, P. (2024). Influence of Machine Tool Operating Conditions on the Resulting Circularity and Positioning Accuracy. Machines, 12(5), 352. https://doi.org/10.3390/machines12050352









