Experimental Vibration Analysis of a Small Scale Vertical Wind Energy System for Residential Use
Abstract
1. Introduction
- there is no need to constantly align the turbine with wind direction;
- better behaviour with turbulent or disturbed flow;
- less noise emissions [10].
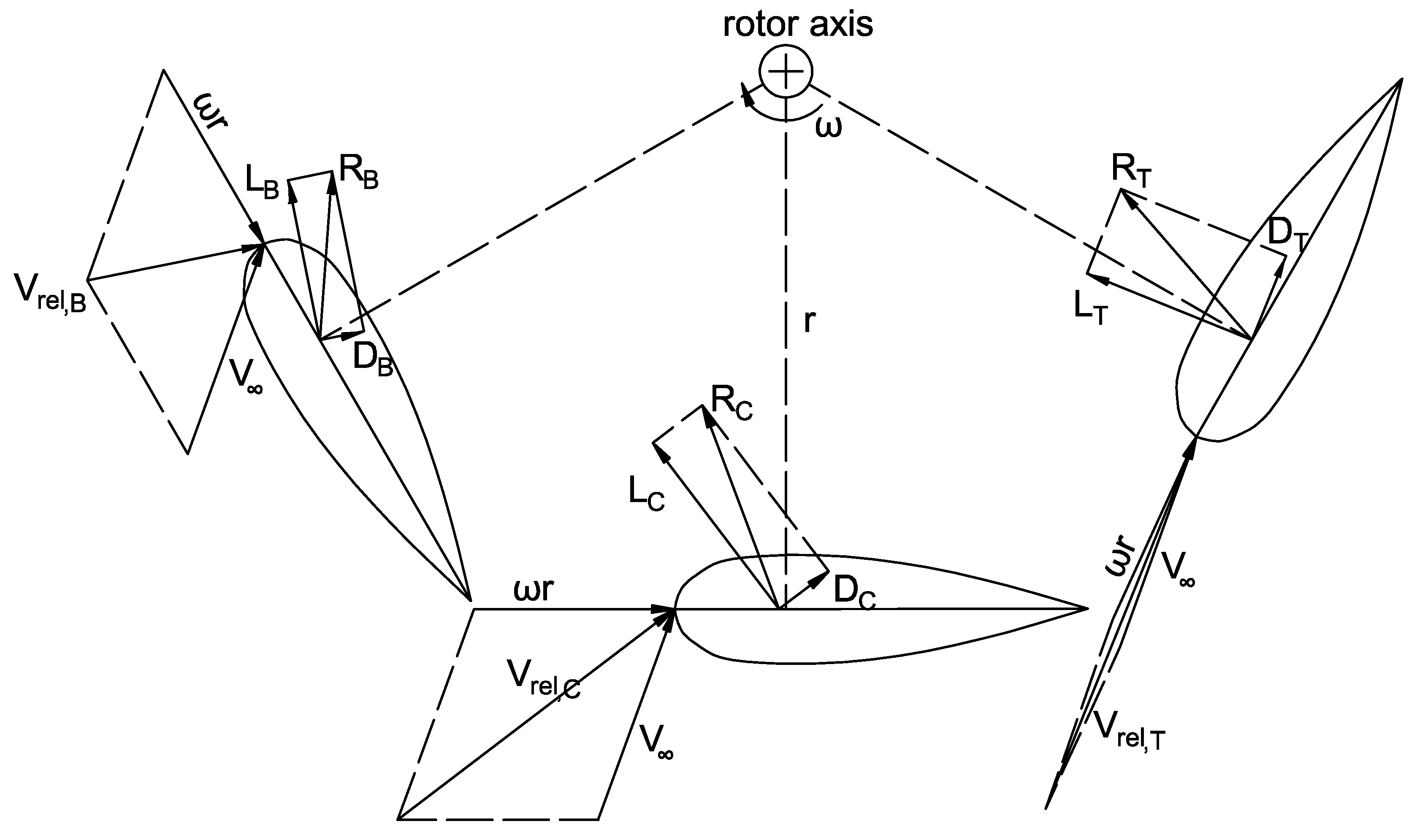
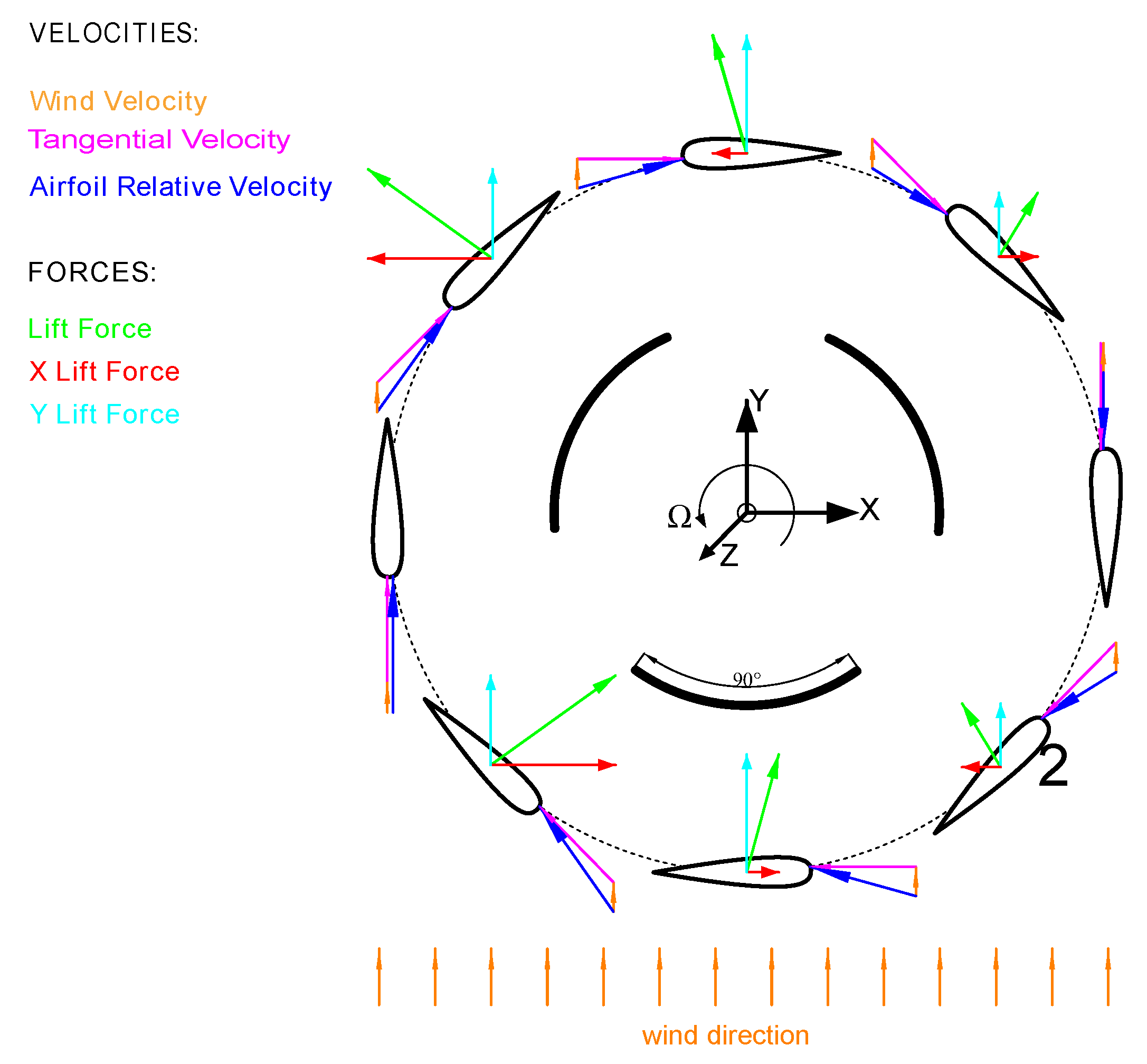
2. Aerodynamics of Vertical Axis Small Wind Turbines
- Savonius;
- Darrieus.
- constructive simplicity;
- cheap initial and maintenance costs;
- low vision impact for urban applications;
- startup with low wind speed independent of the wind direction;
- high torque.
- symmetric, non cambered airfoil;
- the airfoil mean line is perpendicular to the turbine radius;
- steady and uniform wind speed.
3. Methods and Facilities
- Vertical axis wind turbine installed on a small building;
- Decoupler for damping the tower vibrations;
- Accelerometers: three uniaxial and one triaxial;
- Microphone;
- Ultrasonic Doppler anemometer( UDA);
- Amperometer and voltmeter;
- Data acquisition (DAQ) system.
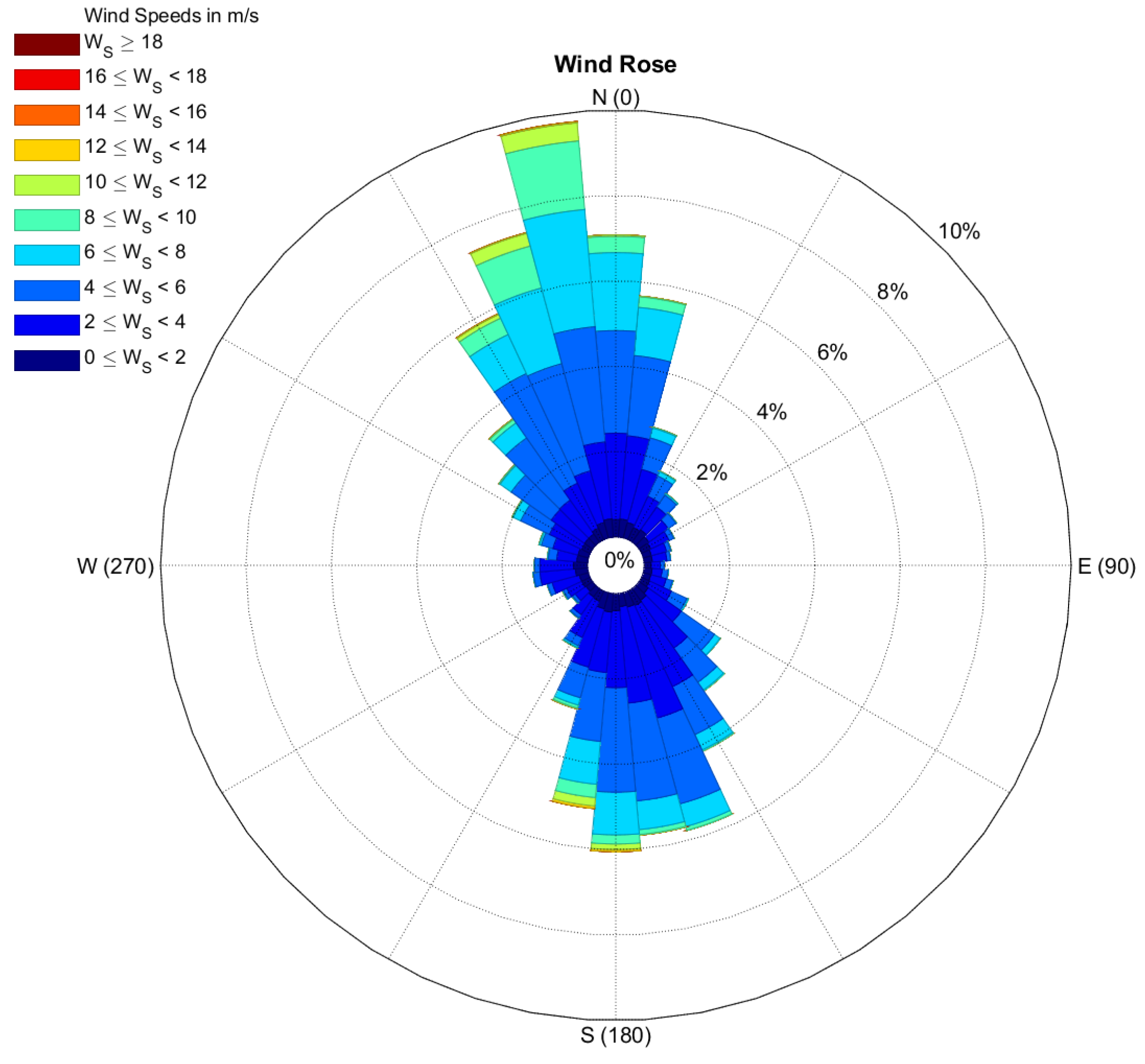
3.1. Wind Turbine, Building and Decoupler System
3.2. Vibration and Noise Measurement
3.3. UDA, Instruments for Electrical Measurements and DAQ
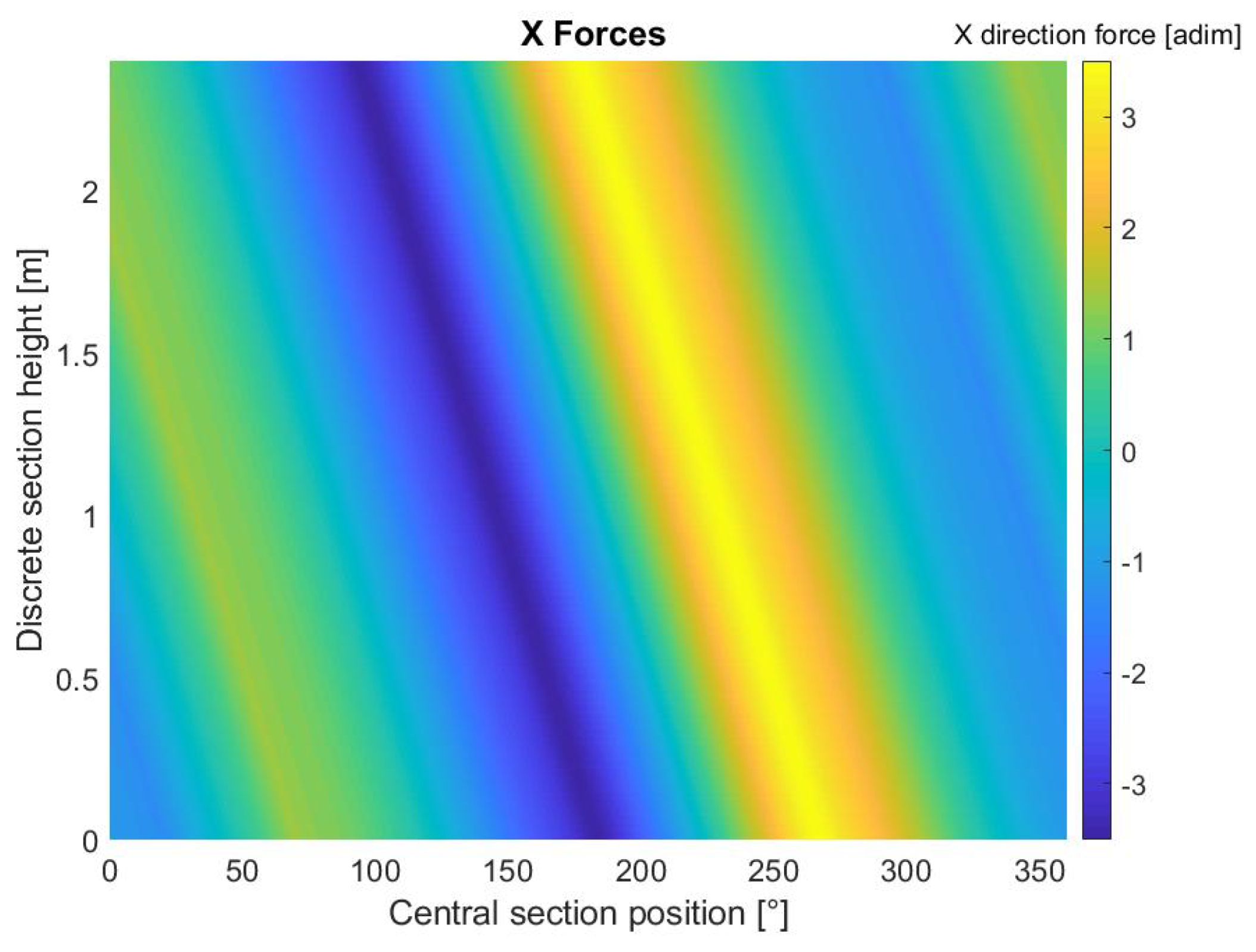
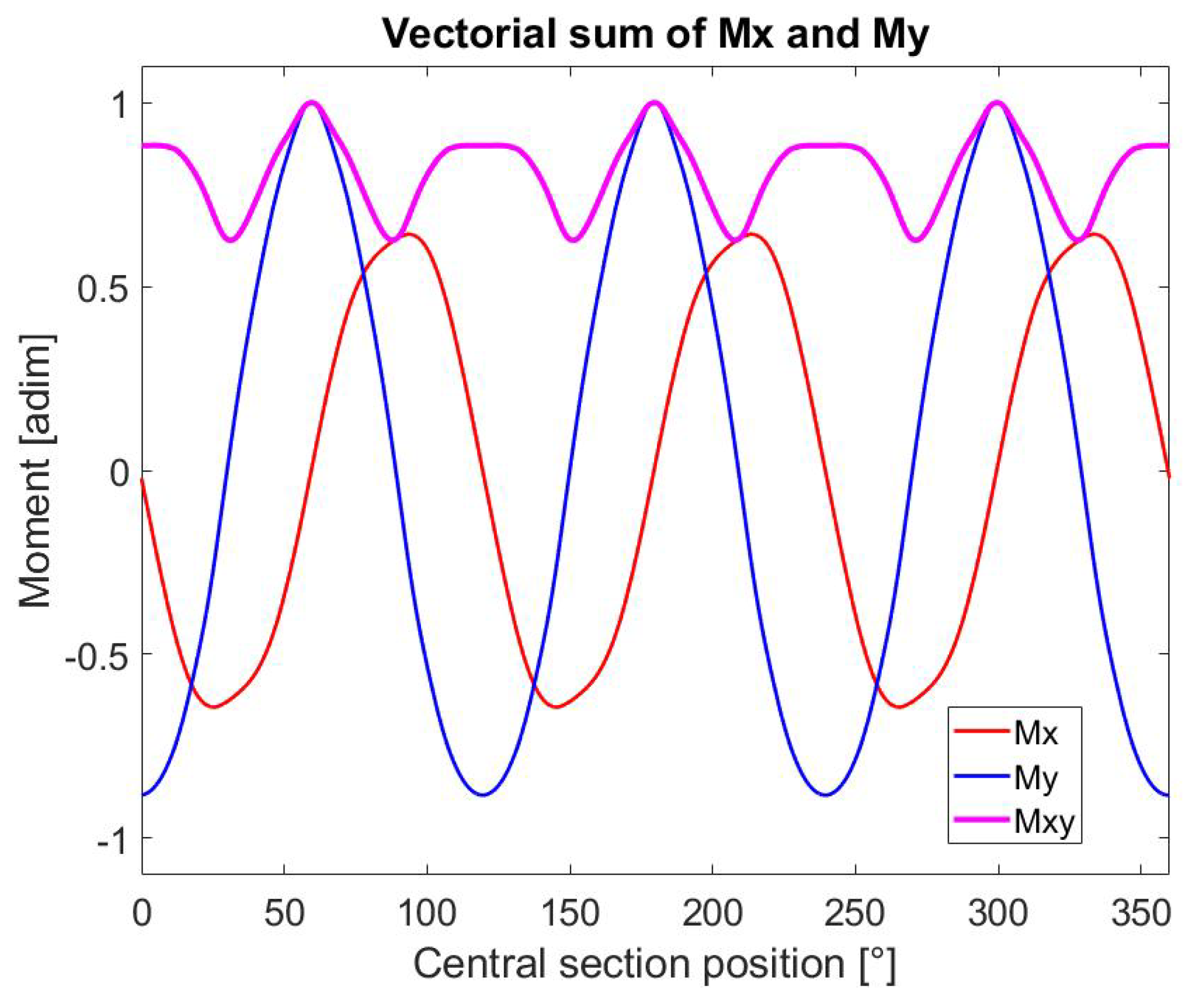
4. Results
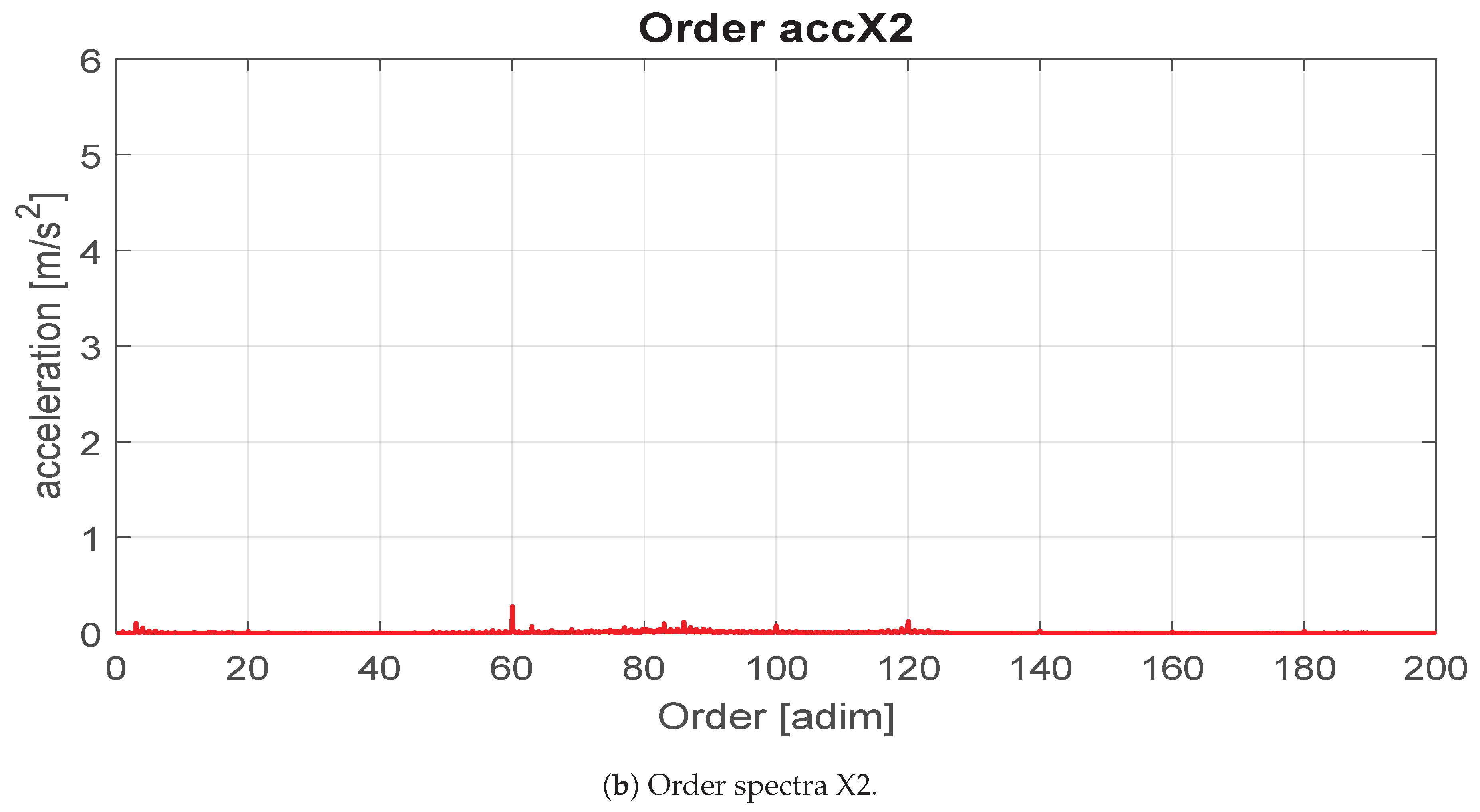
Accelerations of Layers 1 and 2
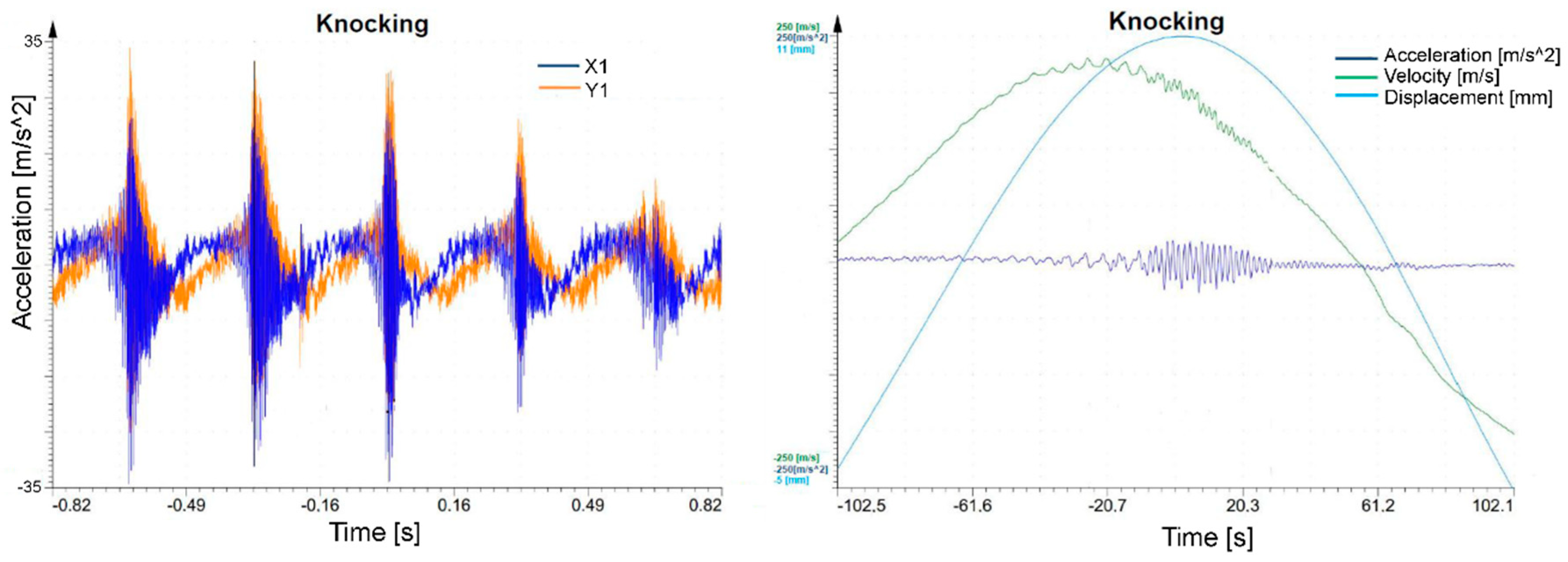
5. Discussion
5.1. Order 1P
5.2. Order 3P
5.3. Order 6P
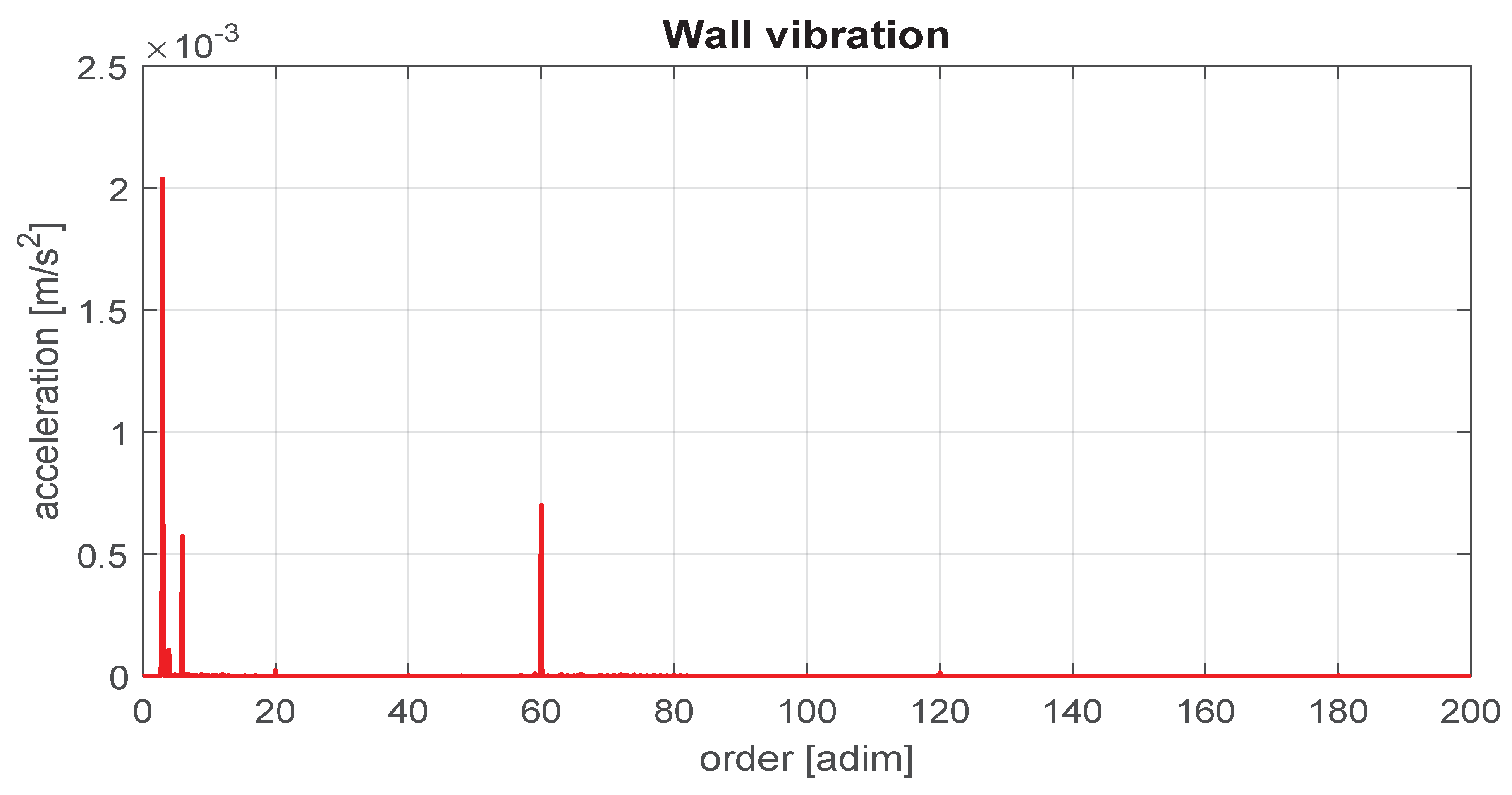
5.4. Orders 60th and 120th
5.5. Decoupler Overload
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| a.s.l. | above sea level |
| AoA | angle of attack |
| DAQ | data acquisition system |
| FFT | fast Fourier transform |
| HAWT | horizontal axis wind turbine |
| HAWST | horizontal axis small wind turbine |
| LES | large eddy simulation |
| OSG | on-site generation |
| OT | order tracking |
| PIV | particle image velocimetry |
| RANS | Reynold averaged Navier–Stokes |
| UDA | ultrasonic Doppler anemometry |
| VAWT | vertical axis wind turbine |
| VASWT | vertical axis small wind turbine |
References
- Tummala, A.; Velamati, R.K.; Sinha, D.K.; Indraja, V.; Krishna, V.H. A review on small scale wind turbines. Renew. Sustain. Energy Rev. 2016, 56, 1351–1371. [Google Scholar] [CrossRef]
- Li, Q.; Maeda, T.; Kamada, Y.; Ogasawara, T.; Nakai, A.; Kasuya, T. Investigation of power performance and wake on a straight-bladed vertical axis wind turbine with field experiments. Energy 2017, 141, 1113–1123. [Google Scholar] [CrossRef]
- Pagnini, L.; Piccardo, G.; Repetto, M.P. Full scale behavior of a small size vertical axis wind turbine. Renew. Energy 2018, 127, 41–55. [Google Scholar] [CrossRef]
- Pagnini, L.C.; Burlando, M.; Repetto, M.P. Experimental power curve of small-size wind turbines in turbulent urban environment. Appl. Energy 2015, 154, 112–121. [Google Scholar] [CrossRef]
- Ishugah, T.; Li, Y.; Wang, R.; Kiplagat, J. Advances in wind energy resource exploitation in urban environment: A review. Renew. Sustain. Energy Rev. 2014, 37, 613–626. [Google Scholar] [CrossRef]
- Sunderland, K.; Woolmington, T.; Blackledge, J.; Conlon, M. Small wind turbines in turbulent (urban) environments: A consideration of normal and Weibull distributions for power prediction. J. Wind Eng. Ind. Aerodyn. 2013, 121, 70–81. [Google Scholar] [CrossRef]
- Battisti, L.; Ricci, M. Wind Energy Exploitation in Urban Environment: TUrbWind 2017 Colloquium; Springer: Cham, Switzwerland, 2018. [Google Scholar]
- Zhang, L.; Zhu, K.; Zhong, J.; Zhang, L.; Jiang, T.; Li, S.; Zhang, Z. Numerical Investigations of the Effects of the Rotating Shaft and Optimization of Urban Vertical Axis Wind Turbines. Energies 2018, 11, 1870. [Google Scholar] [CrossRef]
- Pope, K.; Dincer, I.; Naterer, G. Energy and exergy efficiency comparison of horizontal and vertical axis wind turbines. Renew. Energy 2010, 35, 2102–2113. [Google Scholar] [CrossRef]
- Eriksson, S.; Bernhoff, H.; Leijon, M. Evaluation of different turbine concepts for wind power. Renew. Sustain. Energy Rev. 2008, 12, 1419–1434. [Google Scholar] [CrossRef]
- Castellani, F.; Astolfi, D.; Natili, F.; Mari, F. The Yawing Behavior of Horizontal-Axis Wind Turbines: A Numerical and Experimental Analysis. Machines 2019, 7, 15. [Google Scholar] [CrossRef]
- Mabrouk, I.B.; El Hami, A.; Walha, L.; Zghal, B.; Haddar, M. Dynamic vibrations in wind energy systems: Application to vertical axis wind turbine. Mech. Syst. Sig. Proc. 2017, 85, 396–414. [Google Scholar] [CrossRef]
- Mabrouk, I.B.; El Hami, A. Effect of number of blades on the dynamic behavior of a Darrieus turbine geared transmission system. Mech. Syst. Sig. Proc. 2019, 121, 562–578. [Google Scholar] [CrossRef]
- Mabrouk, I.B.; El Hami, A. Dynamic response analysis of Darrieus wind turbine geared transmission system with unsteady wind inflow. Renew. Energy 2019, 131, 482–493. [Google Scholar] [CrossRef]
- Wang, Y.; Lu, W.; Dai, K.; Yuan, M.; Chen, S.E. Dynamic Study of a Rooftop Vertical Axis Wind Turbine Tower Based on an Automated Vibration Data Processing Algorithm. Energies 2018, 11, 3135. [Google Scholar] [CrossRef]
- Kotulski, L.; Jablonski, A.; Staszewski, W.; Jabłoński, A.; Dziedziech, K.; Czop, P. Comparison of requirements for vibration-based condition monitoring of a vertical-axis vs. horizontal-axis wind turbine. Diagnostyka 2018, 19, 95–100. [Google Scholar] [CrossRef]
- Tian, W.; Song, B.; VanZwieten, J.H.; Pyakurel, P. Computational Fluid Dynamics Prediction of a Modified Savonius Wind Turbine with Novel Blade Shapes. Energies 2015, 8, 7915–7929. [Google Scholar] [CrossRef]
- Altan, B.D.; Atılgan, M. An experimental and numerical study on the improvement of the performance of Savonius wind rotor. Energy Convers. Manag. 2008, 49, 3425–3432. [Google Scholar] [CrossRef]
- Battisti, L.; Brighenti, A.; Benini, E.; Castelli, M.R. Analysis of different blade architectures on small VAWT performance. In Journal of Physics: Conference Series; IOP Publishing: Bristol, UK, 2016; Volume 753, p. 062009. [Google Scholar]
- Battisti, L.; Persico, G.; Dossena, V.; Paradiso, B.; Castelli, M.R.; Brighenti, A.; Benini, E. Experimental benchmark data for H-shaped and troposkien VAWT architectures. Renew. Energy 2018, 125, 425–444. [Google Scholar] [CrossRef]
- Gupta, R.; Biswas, A.; Sharma, K. Comparative study of a three-bucket Savonius rotor with a combined three-bucket Savonius–three-bladed Darrieus rotor. Renew. Energy 2008, 33, 1974–1981. [Google Scholar] [CrossRef]
- Dixon, S.L. Fluid Mechanics and Thermodynamics of Turbomachinery, 7th ed.; Butterworth-Heinemann: Oxford, UK, 2014; ISBN 0-7506-7059-2. [Google Scholar]
- Timmer, W.; Bak, C. 4-Aerodynamic characteristics of wind turbine blade airfoils. In Advances in Wind Turbine Blade Design and Materials; Brøndsted, P., Nijssen, R.P., Eds.; Woodhead Publishing Series in Energy; Woodhead Publishing: Sawston, UK, 2013; pp. 109–149. [Google Scholar]
- Imiela, M.; Faßmann, B.; Heilers, G.; Wilke, G. Numerical airfoil catalogue including 360° airfoil polars and aeroacoustic footprints. Wind Energy Sci. Discuss. 2017, 2017, 1–34. [Google Scholar] [CrossRef]
- KC, A.; Whale, J.; Urmee, T. Urban wind conditions and small wind turbines in the built environment: A review. Renew. Energy 2019, 131, 268–283. [Google Scholar] [CrossRef]
- Heiduschke, A.; Kubowitz, P.; Hamann, M.; Thompson, R.; Haller, P. Tubular timber poles for small wind turbines. In Proceedings of the 11th World Conference on Timber Engineering 2010 (WCTE 2010), Trentino, Italy, 20–24 June 2010; Volume 3, pp. 1821–1825. [Google Scholar]
- Wang, K.; Heyns, P. The combined use of order tracking techniques for enhanced Fourier analysis of order components. Mech. Syst. Sig. Proc. 2011, 25, 803–811. [Google Scholar] [CrossRef]
- Ramlau, R.; Niebsch, J. Imbalance Estimation Without Test Masses for Wind Turbines. J. Sol. Energy Eng. 2009, 131, 011010. [Google Scholar] [CrossRef]
- Wauters, J.; Degroote, J. On the study of transitional low-Reynolds number flows over airfoils operating at high angles of attack and their prediction using transitional turbulence models. Prog. Aerosp. Sci. 2018, 103, 52–68. [Google Scholar] [CrossRef]
- Yang, Y.; Guo, Z.; Zhang, Y.; Jinyama, H.; Li, Q. Numerical investigation of the tip vortex of a straight-bladed vertical axis wind turbine with double-blades. Energies 2017, 10, 1721. [Google Scholar] [CrossRef]
- Castellani, F.; Astolfi, D.; Becchetti, M.; Berno, F.; Cianetti, F.; Cetrini, A. Experimental and numerical vibrational analysis of a horizontal-axis micro-wind turbine. Energies 2018, 11, 456. [Google Scholar] [CrossRef]












© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Castellani, F.; Astolfi, D.; Peppoloni, M.; Natili, F.; Buttà, D.; Hirschl, A. Experimental Vibration Analysis of a Small Scale Vertical Wind Energy System for Residential Use. Machines 2019, 7, 35. https://doi.org/10.3390/machines7020035
Castellani F, Astolfi D, Peppoloni M, Natili F, Buttà D, Hirschl A. Experimental Vibration Analysis of a Small Scale Vertical Wind Energy System for Residential Use. Machines. 2019; 7(2):35. https://doi.org/10.3390/machines7020035
Chicago/Turabian StyleCastellani, Francesco, Davide Astolfi, Mauro Peppoloni, Francesco Natili, Daniele Buttà, and Alexander Hirschl. 2019. "Experimental Vibration Analysis of a Small Scale Vertical Wind Energy System for Residential Use" Machines 7, no. 2: 35. https://doi.org/10.3390/machines7020035
APA StyleCastellani, F., Astolfi, D., Peppoloni, M., Natili, F., Buttà, D., & Hirschl, A. (2019). Experimental Vibration Analysis of a Small Scale Vertical Wind Energy System for Residential Use. Machines, 7(2), 35. https://doi.org/10.3390/machines7020035






