An Empirical Study on the Friction of Reciprocating Rod Seals at Predefined Lubrication Conditions and Shear Rates
Abstract
:1. Introduction
2. Materials and Methods
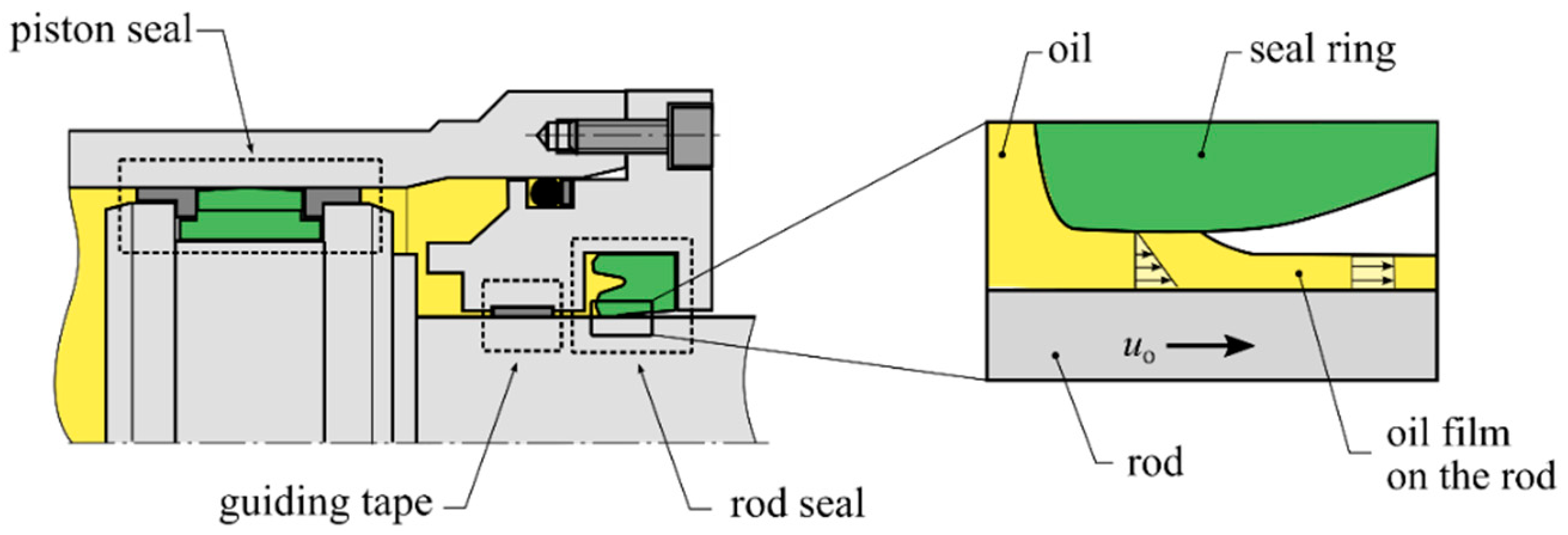
2.1. Components of the Analyzed Sealing Systems
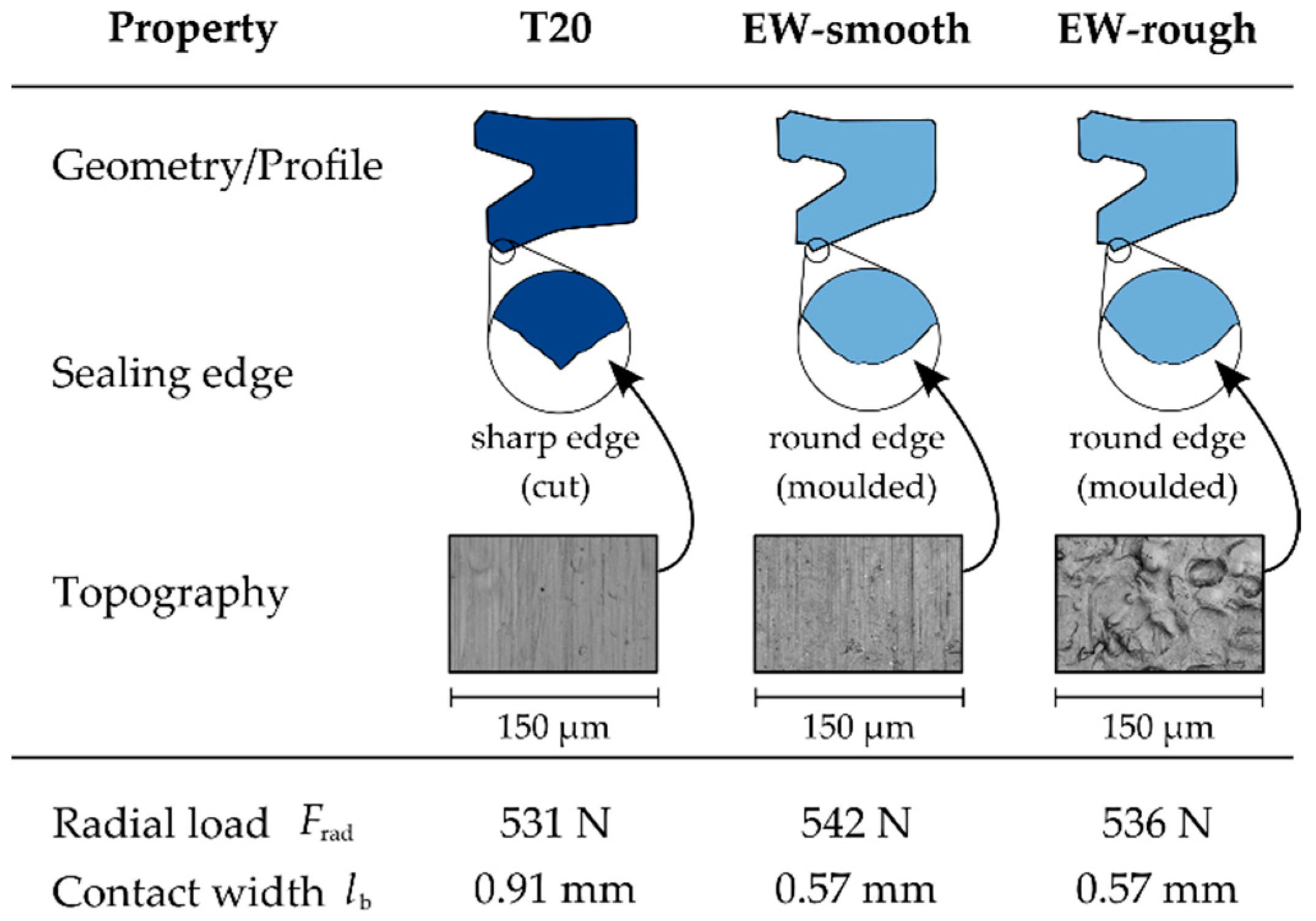
2.1.1. Seal Rings
2.1.2. Hydraulic Oil
2.1.3. Hydraulic Rod
2.2. Ellipsometer Used for Film Thickness Meausrements
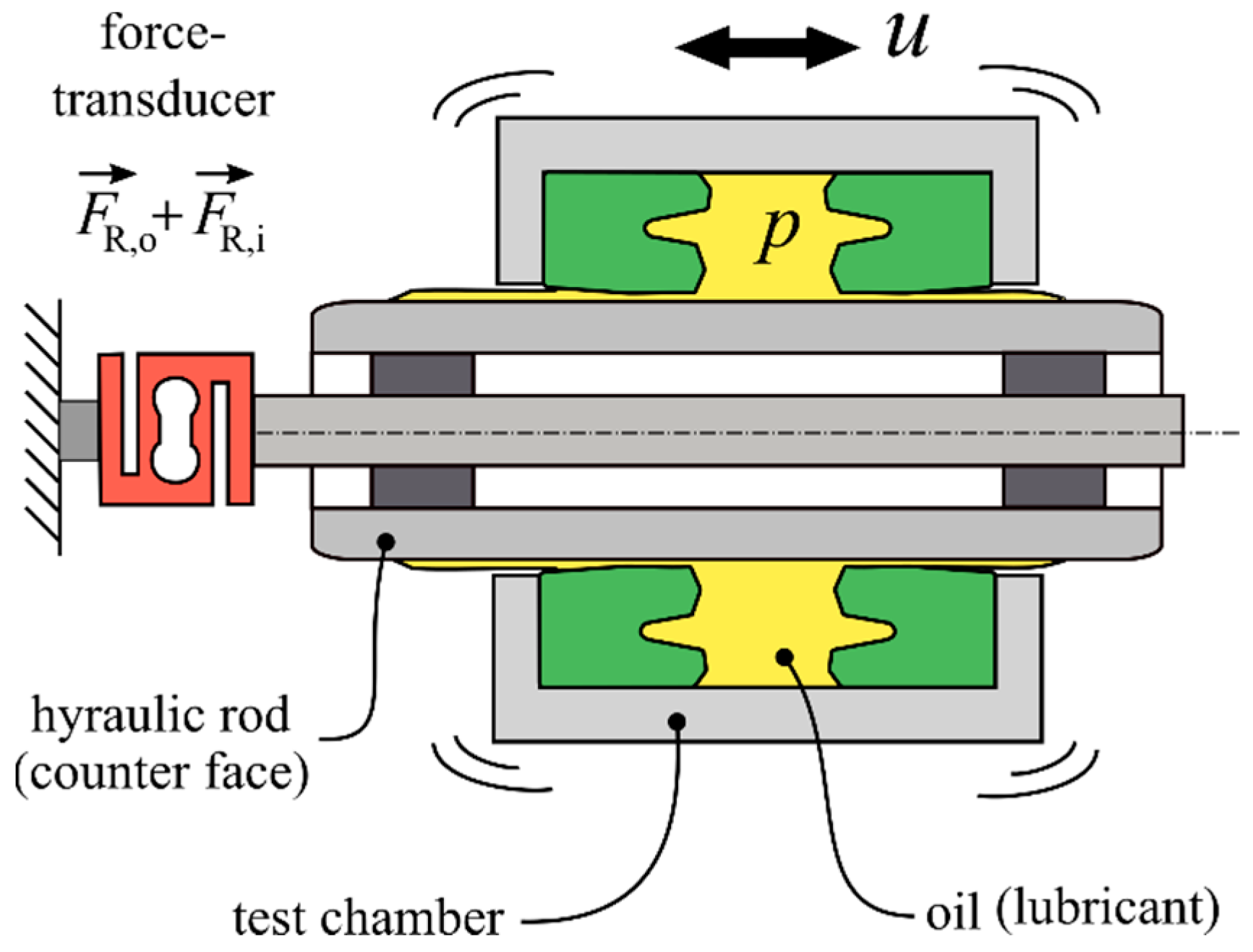
2.3. Test Rigs for Hydraulic Rod Seals
2.3.1. Common Test Rig for Friction Measurement on Two Seals
2.3.2. New Test Rig
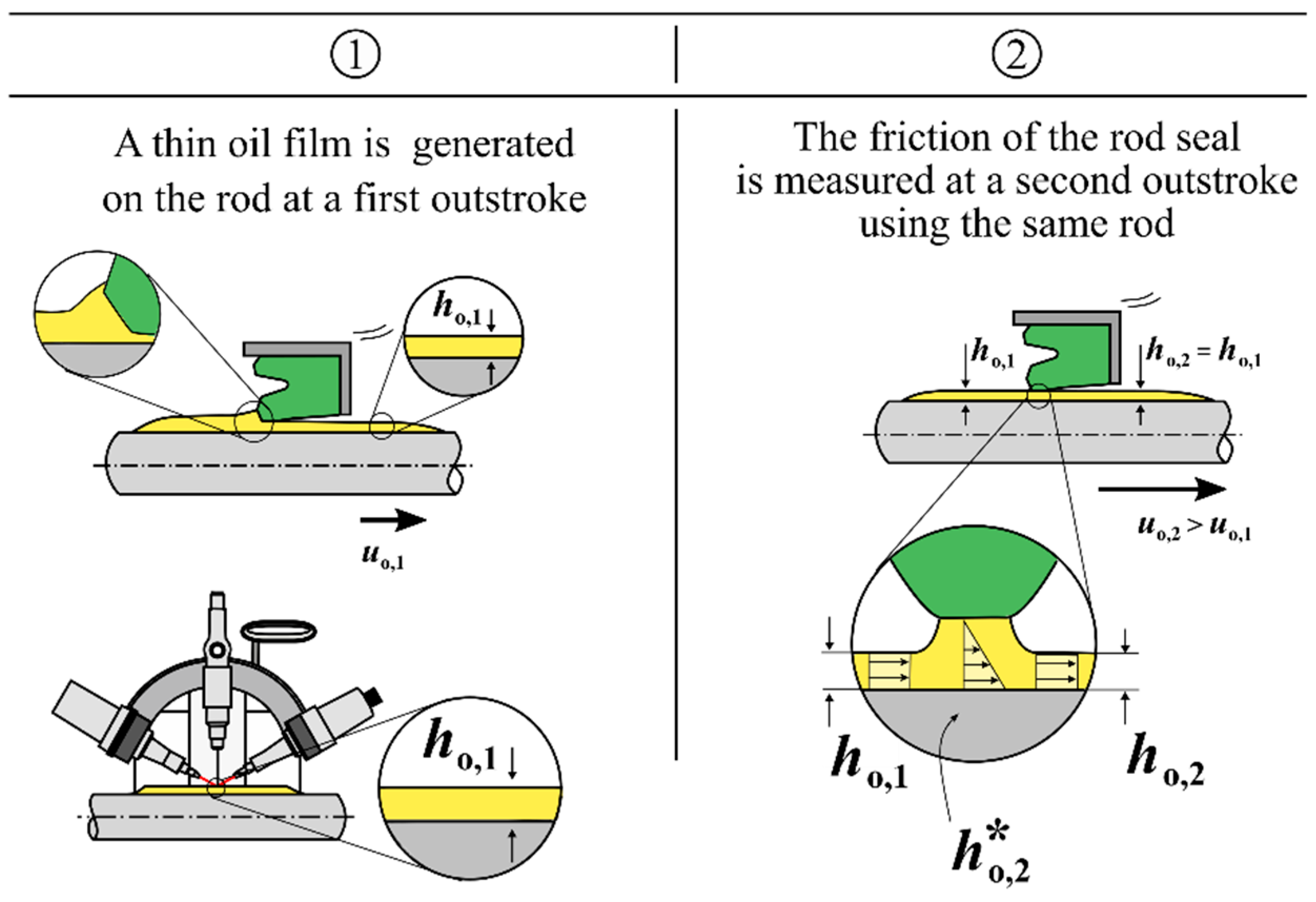
2.4. Novel Measurement Procedure
3. Results
3.1. Common Test Method
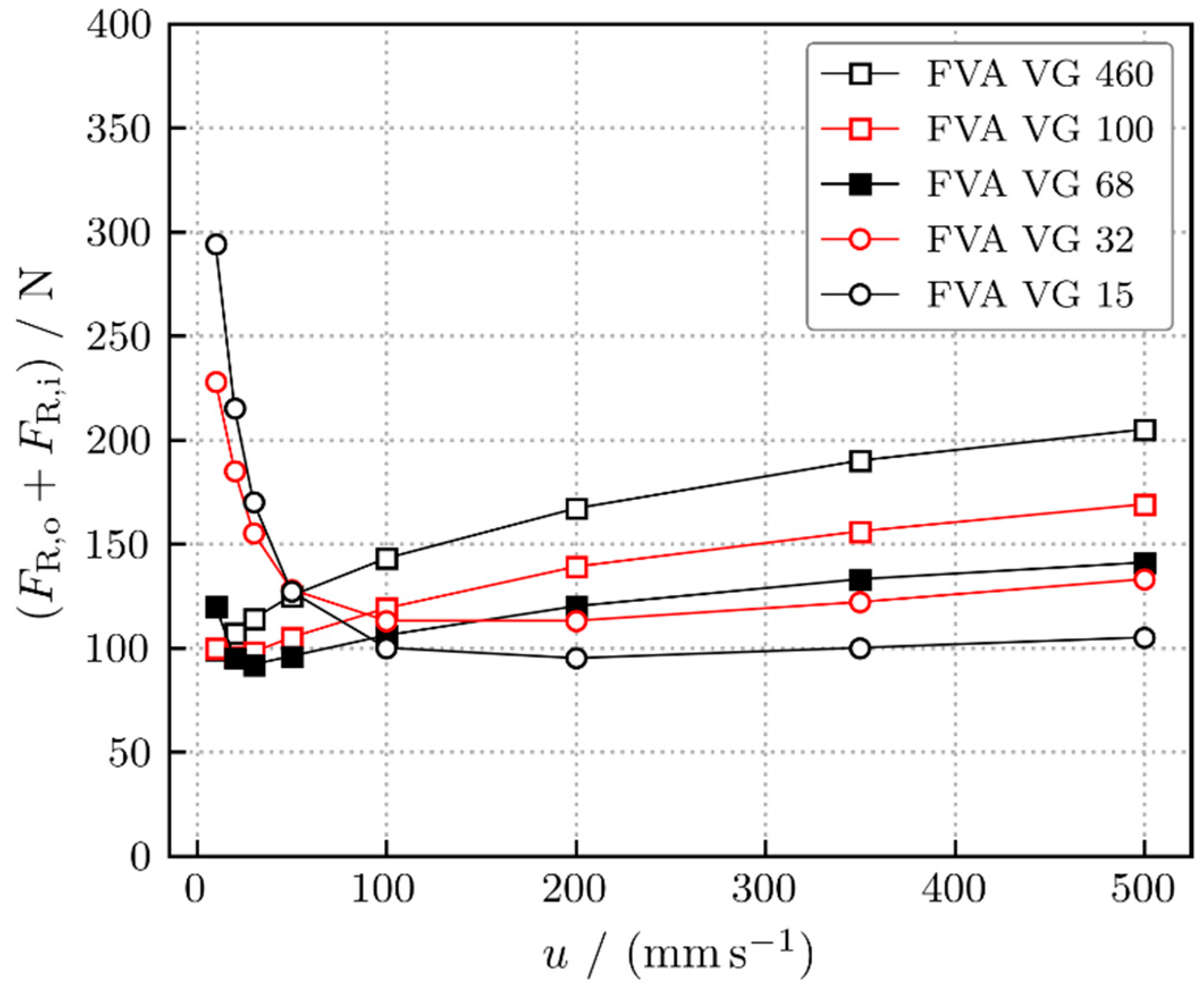
3.1.1. Measured Friction
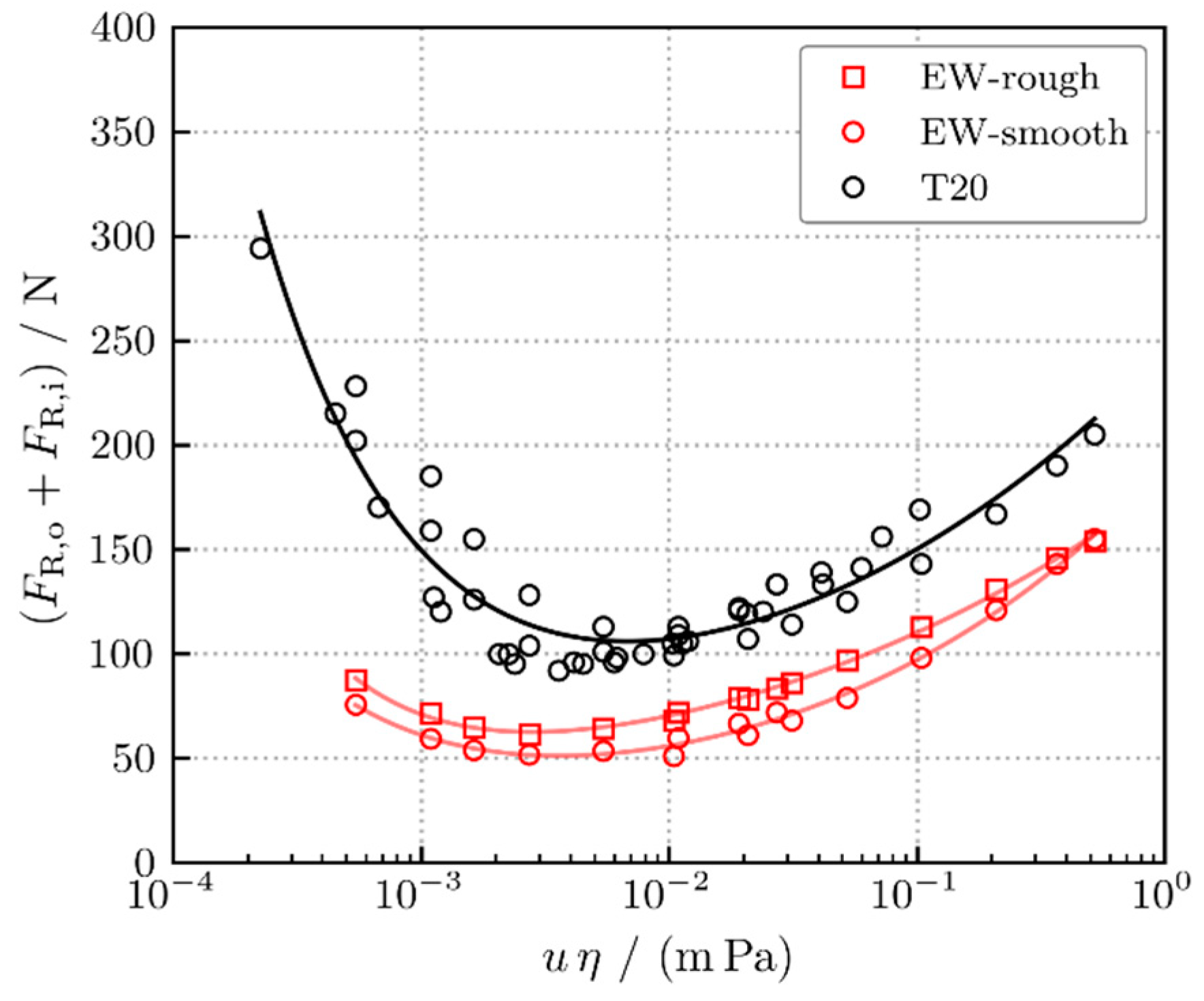
3.1.2. Measured Friction vs. Hydrodynamic Parameter
3.2. New Procedure
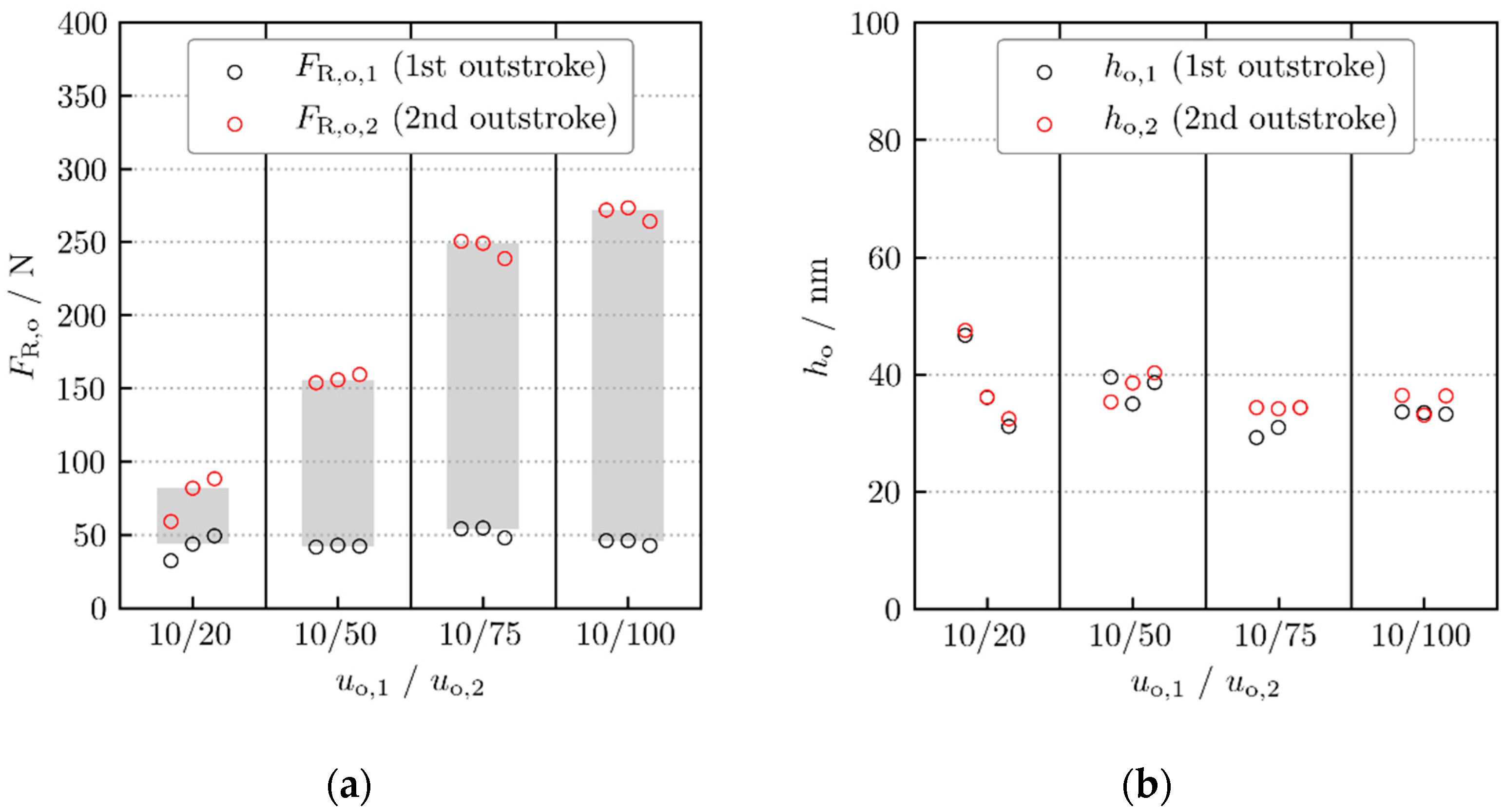
3.2.1. Measured Friction and Film Thickness
3.2.2. Measured Friction vs. Calculated Fluid Friction
4. Discussion
4.1. New Measurement Procedure
4.2. On the Analyis of Friction of Rod Seals
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Contact area between a seal ring and a rod | |
| Nominal diameter of a rod | |
| Newtonian fluid friction | |
| Measured friction at the test rig for two seals | |
| Friction of one rod seal at instroke | |
| Friction of one rod seal at outstroke | |
| Hydrodynamic parameter | |
| Film thickness on the rod after the 1st outstroke when using the new procedure | |
| Film thickness on the rod after the 2nd outstroke when using the new procedure | |
| Contact width between the seal ring and the rod, measured in axial direction | |
| Ambient pressure | |
| Operating pressure | |
| Rod speed at outstroke | |
| Rod speed at 1st outstroke when using the new procedure | |
| Rod speed at 2nd outstroke when using the new procedure | |
| Shear rate | |
| Dynamic viscosity | |
| Temperature |
Appendix A
| U-Cup | Oil | Rod Speed in mms−1 | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 10 | 20 | 30 | 50 | 100 | 200 | 350 | 500 | |||
| Friction Force in N | ||||||||||
| T20 | FVA1 | 22 | 294 | 215 | 170 | 127 | 100 | 95 | 100 | 105 |
| T20 | FVA2 | 54 | 228 | 185 | 155 | 128 | 113 | 113 | 122 | 133 |
| T20 | FVA2 | 54 | 202 | 159 | 126 | 104 | 101 | 109 | 121 | 134 |
| T20 | FVA3 | 205 | 100 | 96 | 98 | 105 | 119 | 139 | 157 | 169 |
| T20 | FVA4 | 1040 | 99 | 107 | 114 | 125 | 143 | 167 | 190 | 205 |
| T20 | ISO VG 68 | 119 | 120 | 95 | 92 | 96 | 107 | 121 | 133 | 141 |
| EW-rough | FVA2 | 54 | 87 | 71 | 65 | 61 | 64 | 72 | 79 | 84 |
| EW-rough | FVA4 | 1040 | 68 | 78 | 86 | 97 | 113 | 131 | 146 | 154 |
| EW-smooth | FVA2 | 54 | 75 | 59 | 54 | 52 | 54 | 60 | 67 | 72 |
| EW-smooth | FVA4 | 1040 | 51 | 61 | 68 | 79 | 98 | 121 | 143 | 156 |
| U-Cup | Oil | U-Cup | Oil | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| T20 | FVA1 | 24 | 10 | 0.4 | 70.7 | T20 | FVA3 | 234 | 40 | 14.8 | 71.1 |
| T20 | FVA1 | 24 | 10 | 0.8 | 61.8 | T20 | FVA3 | 234 | 100 | 7.8 | 222.5 |
| T20 | FVA1 | 24 | 10 | 0.9 | 57.3 | T20 | FVA3 | 234 | 100 | 8.0 | 210 |
| T20 | FVA1 | 24 | 20 | 1.1 | 49.4 | T20 | FVA3 | 234 | 100 | 9.2 | 189.4 |
| T20 | FVA1 | 24 | 20 | 2.5 | 41.5 | T20 | FVA3 | 234 | 100 | 29.3 | 74.8 |
| T20 | FVA1 | 24 | 20 | 3.6 | 41.4 | T20 | FVA3 | 234 | 100 | 33.8 | 61.6 |
| T20 | FVA1 | 24 | 40 | 4.3 | 53.5 | T20 | FVA3 | 234 | 100 | 35.2 | 63.4 |
| T20 | FVA1 | 24 | 40 | 4.6 | 51.1 | T20 | FVA3 | 234 | 200 | 28.2 | 122.3 |
| T20 | FVA1 | 24 | 40 | 5.1 | 48.8 | T20 | FVA3 | 234 | 200 | 30.1 | 123.6 |
| T20 | FVA2 | 60 | 20 | 4.1 | 41.2 | T20 | FVA3 | 234 | 200 | 34.7 | 100.3 |
| T20 | FVA2 | 60 | 20 | 5.1 | 37.3 | T20 | FVA3 | 234 | 200 | 34.7 | 100.0 |
| T20 | FVA2 | 60 | 20 | 5.3 | 38.8 | T20 | FVA3 | 234 | 200 | 35.7 | 112.7 |
| T20 | FVA2 | 60 | 40 | 7.0 | 45.4 | T20 | FVA3 | 234 | 200 | 36.0 | 104.3 |
| T20 | FVA2 | 60 | 40 | 7.1 | 48.8 | T20 | FVA3 | 234 | 200 | 55.0 | 65.0 |
| T20 | FVA2 | 60 | 40 | 7.5 | 48.7 | T20 | FVA3 | 234 | 200 | 60.3 | 66.6 |
| T20 | FVA2 | 60 | 100 | 11.7 | 50.0 | T20 | FVA3 | 234 | 200 | 70.9 | 63.0 |
| T20 | FVA2 | 60 | 100 | 11.7 | 54.6 | T20 | FVA4 | 1214 | 20 | 32.4 | 88.3 |
| T20 | FVA2 | 60 | 100 | 12.7 | 48.3 | T20 | FVA4 | 1214 | 20 | 36.1 | 81.2 |
| T20 | FVA2 | 60 | 200 | 10.2 | 100.8 | T20 | FVA4 | 1214 | 20 | 47.5 | 59.1 |
| T20 | FVA2 | 60 | 200 | 10.7 | 95.1 | T20 | FVA4 | 1214 | 50 | 35.3 | 153.8 |
| T20 | FVA2 | 60 | 200 | 11.5 | 90.4 | T20 | FVA4 | 1214 | 50 | 38.5 | 155.8 |
| T20 | FVA2 | 60 | 200 | 20.1 | 53.4 | T20 | FVA4 | 1214 | 50 | 40.3 | 159.5 |
| T20 | FVA2 | 60 | 200 | 21.0 | 56.7 | T20 | FVA4 | 1214 | 75 | 34.1 | 249.2 |
| T20 | FVA2 | 60 | 200 | 21.5 | 47.4 | T20 | FVA4 | 1214 | 75 | 34.3 | 238.7 |
| T20 | FVA3 | 234 | 10 | 2.7 | 63.5 | T20 | FVA4 | 1214 | 75 | 34.3 | 250.6 |
| T20 | FVA3 | 234 | 10 | 2.8 | 56.2 | T20 | FVA4 | 1214 | 100 | 33.1 | 273.4 |
| T20 | FVA3 | 234 | 10 | 3.5 | 74.6 | T20 | FVA4 | 1214 | 100 | 36.3 | 264.2 |
| T20 | FVA3 | 234 | 20 | 7.0 | 65.5 | T20 | FVA4 | 1214 | 100 | 36.4 | 271.9 |
| T20 | FVA3 | 234 | 20 | 8.0 | 65.2 | T20 | FVA4 | 1214 | 100 | 218.4 | 46.7 |
| T20 | FVA3 | 234 | 20 | 8.4 | 57.1 | T20 | FVA4 | 1214 | 100 | 232.6 | 47.7 |
| T20 | FVA3 | 234 | 40 | 10.5 | 76.6 | T20 | FVA4 | 1214 | 100 | 232.6 | 46.2 |
| T20 | FVA3 | 234 | 40 | 11.6 | 81.9 |
| U-Cup | Oil | U-Cup | Oil | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| EW-smooth | FVA2 | 60 | 80 | 12.0 | 46.7 | EW-rough | FVA2 | 60 | 80 | 13.3 | 38.4 |
| FVA2 | 60 | 80 | 13.0 | 44.0 | FVA2 | 60 | 80 | 15.6 | 36.6 | ||
| FVA3 | 234 | 75 | 9.7 | 102.8 | FVA3 | 234 | 75 | 7.8 | 118.4 | ||
| FVA3 | 234 | 75 | 13.4 | 84.0 | FVA3 | 234 | 75 | 11.2 | 84.8 |
References
- Müller, H.K.; Nau, B.S. Fluid Sealing Technology: Principles and Applications; Marcel Dekker: New York, NY, USA, 1998; ISBN 0-8247-9969-0. [Google Scholar]
- Bauer, F. Tribologie—Prägnant Und Praxisrelevant; Springer Vieweg: Wiesbaden, Germany, 2021; ISBN 978-3-658-32920-4. [Google Scholar]
- Flitney, R. Reciprocating Seals. In Seals and Sealing Handbook; Butterworth-Heinemann: Oxford, UK, 2014; pp. 289–367. ISBN 978-0-08-099416-1. [Google Scholar]
- Gee, M.L.; McGuiggan, P.M.; Israelachvili, J.N.; Homola, A.M. Liquid to Solidlike Transitions of Molecularly Thin Films under Shear. J. Chem. Phys. 1990, 93, 1895–1906. [Google Scholar] [CrossRef]
- Shen, M.; Luo, J.; Wen, S. Effects of Surface Physicochemical Properties on the Tribological Properties of Liquid Paraffin Film in the Nanoscale. Surf. Interface Anal. 2001, 32, 286–288. [Google Scholar] [CrossRef]
- Luo, J.; Wen, S.; Xuanyu, S. Substrate Surface Energy Effects on a Liquid Lubricant Film at Nanometre Scale. Lubr. Sci. 1998, 11, 936–1198. [Google Scholar]
- Neto, C.; Evans, D.R.; Bonaccurso, E.; Butt, H.-J.; Craig, V.S.J. Boundary Slip in Newtonian Liquids: A Review of Experimental Studies. Rep. Prog. Phys. 2005, 68, 2859–2897. [Google Scholar] [CrossRef]
- Lauga, E.; Brenner, M.; Stone, H. Microfluidics: The No-Slip Boundary Condition. In Springer Handbook of Experimental Fluid Mechanics; Springer: Berlin/Heidelberg, Germany, 2007; pp. 1219–1235. ISBN 978-3-540-30299-5. [Google Scholar]
- Lee, T.; Charrault, E.; Neto, C. Interfacial Slip on Rough, Patterned and Soft Surfaces: A Review of Experiments and Simulations. Adv. Colloid Interface Sci. 2014, 210, 21–38. [Google Scholar] [CrossRef]
- Spikes, H.A. Slip at the Wall—Evidence and Tribological Implications. Tribol. Ser. 2003, 41, 525–535. [Google Scholar] [CrossRef]
- Spikes, H. Friction Modifier Additives. Tribol. Lett. 2015, 60, 5. [Google Scholar] [CrossRef] [Green Version]
- Porter, R.S.; Johnson, J.F. Viscosity Performance of Lubricating Base Oils at Shears Developed in Machine Elements. Wear 1961, 4, 32–40. [Google Scholar] [CrossRef]
- Panwar, P.; Michael, P.; Devlin, M.; Martini, A. Critical Shear Rate of Polymer-Enhanced Hydraulic Fluids. Lubricants 2020, 8, 102. [Google Scholar] [CrossRef]
- Bair, S.; Qureshi, F. The Generalized Newtonian Fluid Model and Elastohydrodynamic Film Thickness. J. Tribol. 2003, 125, 70–75. [Google Scholar] [CrossRef]
- Wang, J.; Li, Y.; Lian, Z. Numerical Investigations on the Sealing Performance of a Reciprocating Seal Based on the Inverse Lubrication Method. J. Tribol. 2019, 141, 112201. [Google Scholar] [CrossRef]
- Kaiser, F. Ein Simulationsmodell zur Analyse des Schmierfilms von Stangendichtungen. Ph.D. Thesis, TU Kaiserslautern, Kaiserslautern, Germany, 2015. [Google Scholar]
- Crudu, M.; Fatu, A.; Cananau, S.; Hajjam, M.; Pascu, A.; Cristescu, C. A Numerical and Experimental Friction Analysis of Reciprocating Hydraulic “U” Rod Seals. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2012, 226, 785–794. [Google Scholar] [CrossRef]
- Fatu, A.; Hajjam, M. Numerical Modelling of Hydraulic Seals by Inverse Lubrication Theory. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2011, 225, 1159–1173. [Google Scholar] [CrossRef]
- El Gadari, M.; Hajjam, M. Effect of the Grooved Rod on the Friction Force of U-Cup Hydraulic Rod Seal with Rough Lip. Tribol. Trans. 2018, 61, 661–670. [Google Scholar] [CrossRef]
- Huang, P. Numerical Calculation of Elastohydrodynamic Lubrication; Wiley-VCH: Singapore, 2015; ISBN 978-1-118-92096-1. [Google Scholar]
- Stupkiewicz, S.; Marciniszyn, A. Elastohydrodynamic Lubrication and Finite Configuration Changes in Reciprocating Elastomeric Seals. Tribol. Int. 2009, 42, 615–627. [Google Scholar] [CrossRef]
- Spikes, H.; Jie, Z. History, Origins and Prediction of Elastohydrodynamic Friction. Tribol. Lett. 2014, 56, 1–25. [Google Scholar] [CrossRef] [Green Version]
- Huang, Y.; Salant, R.F. Simulation of a Hydraulic Rod Seal with a Textured Rod and Starvation. Tribol. Int. 2016, 95, 306–315. [Google Scholar] [CrossRef] [Green Version]
- Gropp, A. Simulation of Surface Topography in Sealing Systems for Reciprocating Rods. In Proceedings of the 19th International Sealing Conference, Stuttgart, Germany, 12–13 October 2016; VDMA Fluidtechnik: Stuttgart, Germany, 2016; pp. 193–203. [Google Scholar]
- ISO 7986:1997-07; Hydraulic Fluid Power—Sealing Devices—Standard Test Methods to Assess the Performance of Seals Used in Oil Hydraulic Reciprocating Applications. Beuth Verlag GmbH: Berlin, Germany, 1997.
- Feuchtmüller, O.; Dakov, N.; Hörl, L.; Bauer, F. Remarks on Modeling the Oil Film Generation of Rod Seals. Lubricants 2021, 9, 95. [Google Scholar] [CrossRef]
- Nikas, G.K. Eighty Years of Research on Hydraulic Reciprocating Seals: Review of Tribological Studies and Related Topics since the 1930s. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2010, 224, 1–23. [Google Scholar] [CrossRef]
- Visscher, M.; Kanters, A.F.C. Literature Review and Discussion on Measurements of Leakage, Lubricant Film Thickness and Friction of Reciprocating Elastomeric Seals. Lubr. Eng. 1990, 46, 785–791. [Google Scholar]
- Feuchtmüller, O.; Hörl, L.; Bauer, F. Oil Film Generation of a Hydraulic Rod Seal: An Experimental Study Using Ellipsometry. Tribol. Int. 2021, 162, 107102. [Google Scholar] [CrossRef]
- Hörl, L.; Haas, W.; Nißler, U. A Comparison of Test Methods for Hydraulic Rod Seals. Seal. Technol. 2009, 2009, 8–13. [Google Scholar] [CrossRef]
- Feldmeth, S.; Stoll, M.; Bauer, F. How to Measure the Radial Load of Radial Lip Seals. In Proceedings of the 61. Tribologie-Fachtagung, Göttingen, Germany, 28–30 September 2020; pp. 5–12. [Google Scholar]
- DIN 3761-9; Radial-Wellendichtringe für Kraftfahrzeuge, Prüfung Radialkraft-Meßgerät-Digital. Beuth Verlag GmbH: Berlin, Germany, 1984.
- SAE J1901:1988-06; Recommended Practice: Lip Force Measurement—Radial Lips Seals. Society of Automotive Engineers: Warrendale, PA, USA, 1988; (Cancelled in 2002-10).
- FVA Heft 660; Referenzölkatalog (Datensammlung). Forschungsvereiningung Antriebstechnik eV.: Frankfurt, Germany, 2007.
- Lang, C.M. Untersuchungen an Berührungsdichtungen für Hydraulische Arbeitszylinder. Ph.D. Thesis, University of Stuttgart, Stuttgart, Germany, 1960. [Google Scholar]
- Messner, N. Untersuchung von Hydraulik-Stangendichtungen aus Polytetrafluoräthylen. Ph.D. Thesis, Universität Stuttgart, Stuttgart, Germany, 1985. [Google Scholar]
- Prokop, H.-J. Zum Abdicht-und Reibungsverhalten von Hydraulik-Stangendichtungen aus Polytetrafluoräthylen. Ph.D. Thesis, Universität Stuttgart, Stuttgart, Germany, 1989. [Google Scholar]
- Salant, R.F.; Maser, N.; Yang, B. Numerical Model of a Reciprocating Hydraulic Rod Seal. J. Tribol. 2006, 129, 91–97. [Google Scholar] [CrossRef]
- Ottink, K.; Wennehorst, B.; Poll, G. Analysis of Rod Seals by Application of the Light Induced Fluorescence Method. In Proceedings of the 16th International Sealing Conference, Stuttgart, Germany, 12–13 October 2010; VDMA Fluidtechnik: Stuttgart, Germany, 2010; pp. 101–111. [Google Scholar]
- Goerres, M.; Murrenhoff, H. Schmierfilmdickenmessung Mit Hilfe Der Interferenz- Und Fluoreszenzmethode. In Proceedings of the 13th International Sealing Conference, Stuttgart, Germany, 5–6 October 2004; VDMA Fluidtechnik: Stuttgart, Germany, 2004; pp. 254–266. [Google Scholar]
- Fowell, M.T.; Myant, C.; Spikes, H.A.; Kadiric, A. A Study of Lubricant Film Thickness in Compliant Contacts of Elastomeric Seal Materials Using a Laser Induced Fluorescence Technique. Tribol. Int. 2014, 80, 76–89. [Google Scholar] [CrossRef]
- Yoshimura, K.; Suzuki, N.; Mizuta, H. Oil Film Formation of Reciprocating Seals Observed by Interferometry. Tribol. Online 2014, 9, 106–112. [Google Scholar] [CrossRef] [Green Version]
- Blok, H.; Koens, H.J. The Breathing Film between a Flexible Seal and a Reciprocating Rod. Proc. Inst. Mech. Eng. 1965, 180, 221–223. [Google Scholar] [CrossRef]
- Kaneta, M.; Todoroki, H.; Nishikawa, H.; Kanzaki, Y.; Kawahara, Y. Tribology of Flexible Seals for Reciprocating Motion. J. Tribol. 2000, 122, 787–795. [Google Scholar] [CrossRef]
- Suzuki, N.; Mizuta, H.; Sato, Y. An Experimental Approach to the Sealing Mechanism of the Reciprocating Seals. In Proceedings of the 17th International Sealing Conference, Stuttgart, Germany, 13–14 September 2012; VDMA Fluidtechnik: Stuttgart, Germany, 2012; pp. 483–490. [Google Scholar]
- Meyer, F.; Loyen, C.F. The Creep of Oil on Steel Followed by Ellipsometry. Wear 1975, 33, 317–323. [Google Scholar] [CrossRef]



| Oil | Viscosity Class | Dynamic Viscosity mPas | ||
|---|---|---|---|---|
| FVA1 | ISO VG 15 | 28 | 22 | 18 |
| FVA2 | ISO VG 32 | 72 | 54 | 42 |
| FVA2 + FVA3 | ISO VG 68 | 161 | 119 | 90 |
| FVA3 | ISO VG 100 | 288 | 205 | 150 |
| FVA4 | ISO VG 460 | 1543 | 1040 | 720 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Feuchtmüller, O.; Hörl, L.; Bauer, F. An Empirical Study on the Friction of Reciprocating Rod Seals at Predefined Lubrication Conditions and Shear Rates. Lubricants 2022, 10, 56. https://doi.org/10.3390/lubricants10040056
Feuchtmüller O, Hörl L, Bauer F. An Empirical Study on the Friction of Reciprocating Rod Seals at Predefined Lubrication Conditions and Shear Rates. Lubricants. 2022; 10(4):56. https://doi.org/10.3390/lubricants10040056
Chicago/Turabian StyleFeuchtmüller, Oliver, Lothar Hörl, and Frank Bauer. 2022. "An Empirical Study on the Friction of Reciprocating Rod Seals at Predefined Lubrication Conditions and Shear Rates" Lubricants 10, no. 4: 56. https://doi.org/10.3390/lubricants10040056
APA StyleFeuchtmüller, O., Hörl, L., & Bauer, F. (2022). An Empirical Study on the Friction of Reciprocating Rod Seals at Predefined Lubrication Conditions and Shear Rates. Lubricants, 10(4), 56. https://doi.org/10.3390/lubricants10040056






