Study on Factors Influencing Film Formation of Grease and Calculation Model for Grease Film Thickness
Abstract
:1. Introduction
2. Materials and Methods
2.1. Experimental Materials
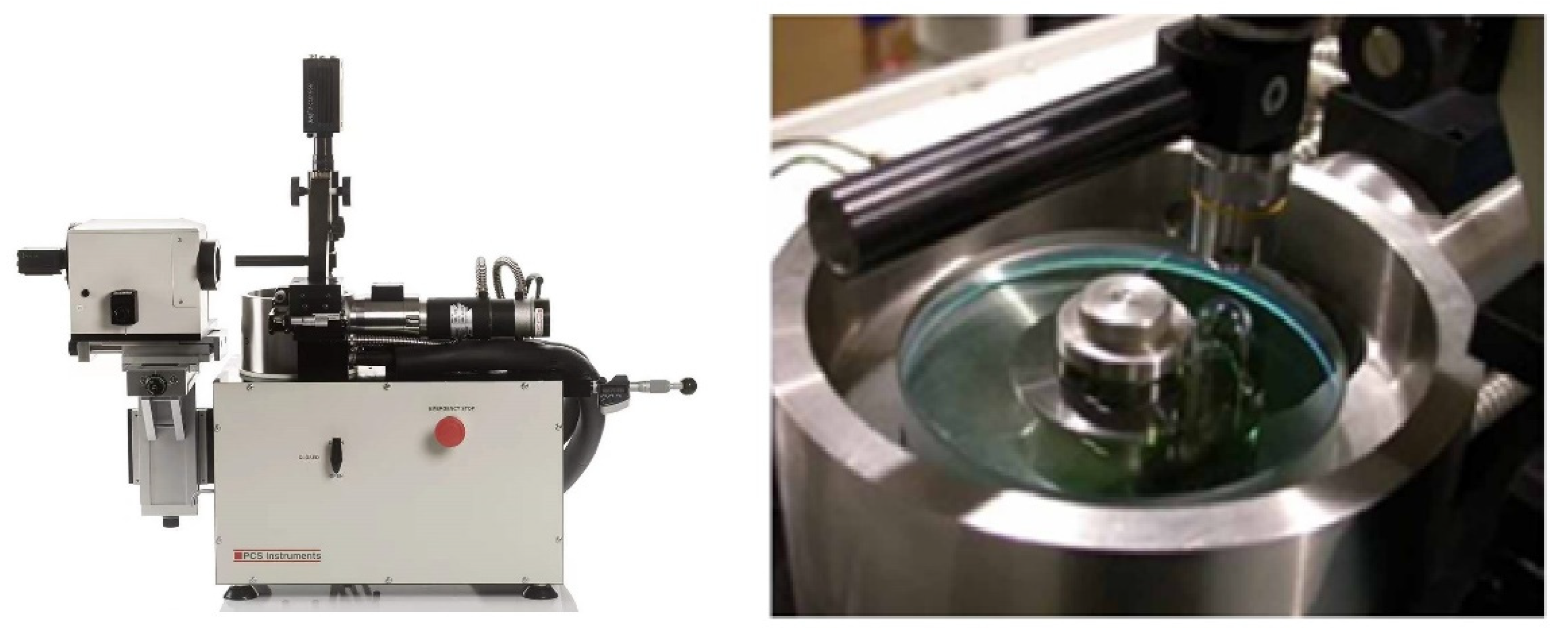
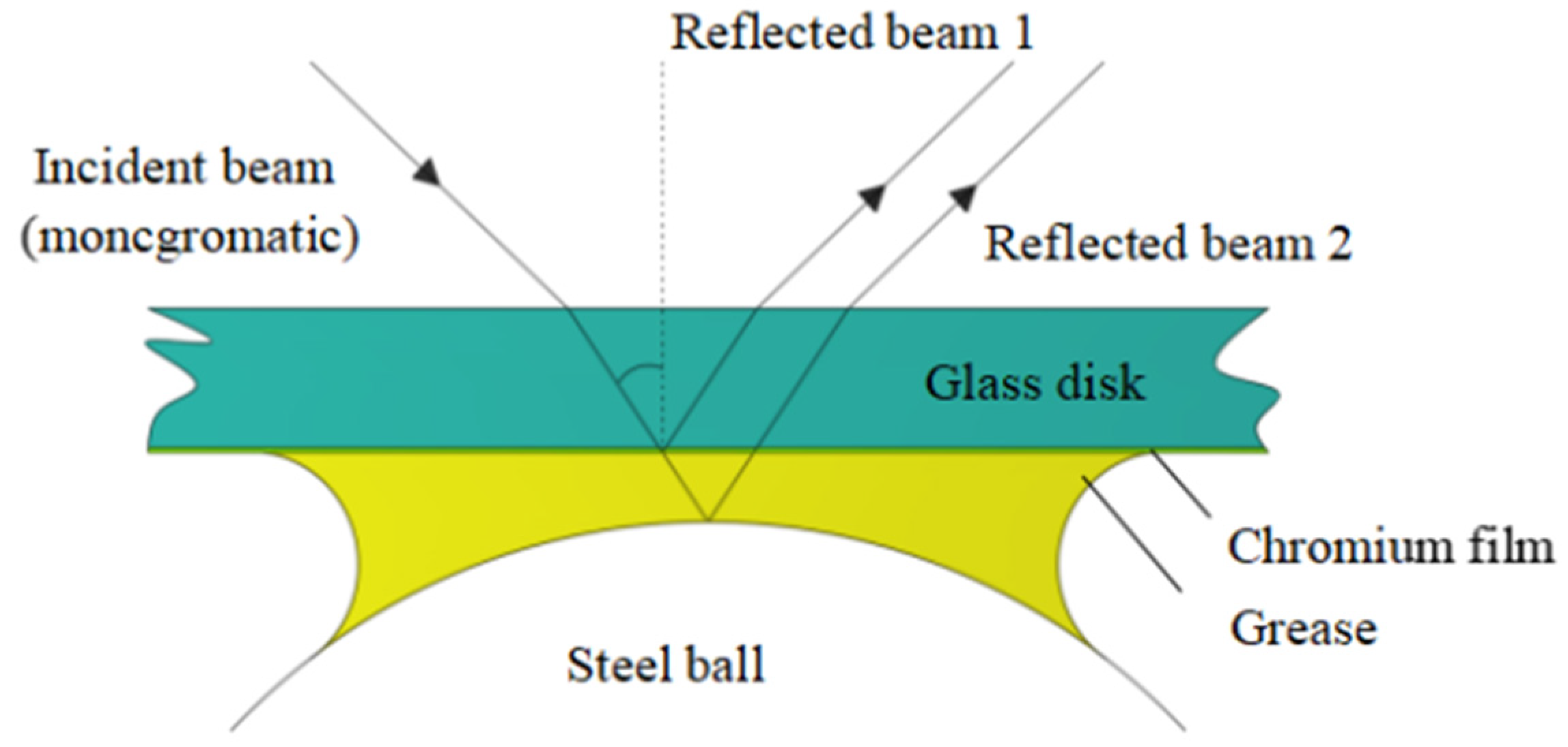
2.2. Experimental Method
- The measuring accuracy can reach ±1 nm;
- The spacer layer imaging method (SLIM) is used to observe the oil film-forming in the experiment process;
- The maximum load is 50 N and the maximum contact pressure is 0.7 GPa for glass disc;
- The maximum rolling speed is 4 m/s;
- The temperature range: Ambient~150 °C;
- The volume of the test sample was 120 mL.
3. Results and Discussion
3.1. Results
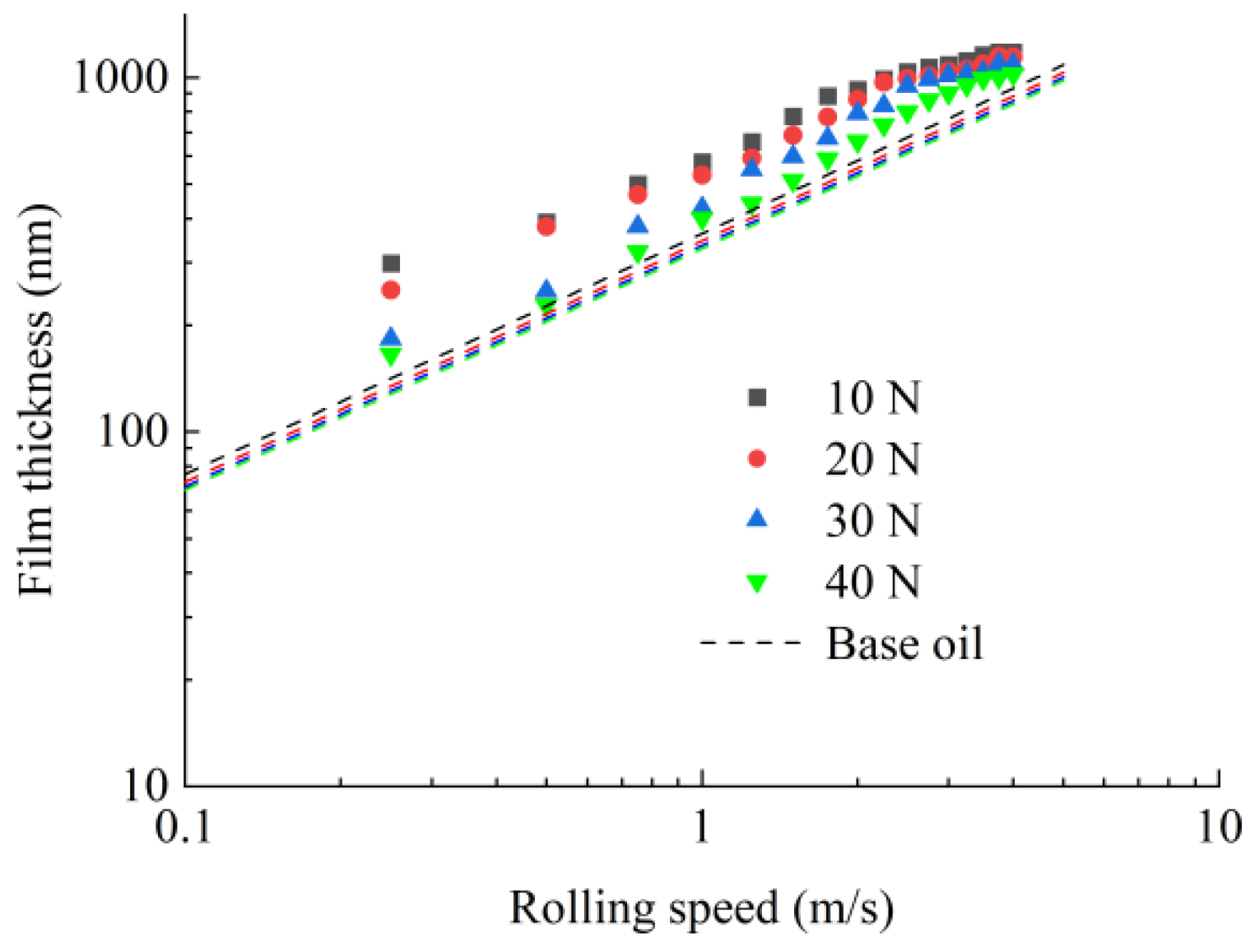
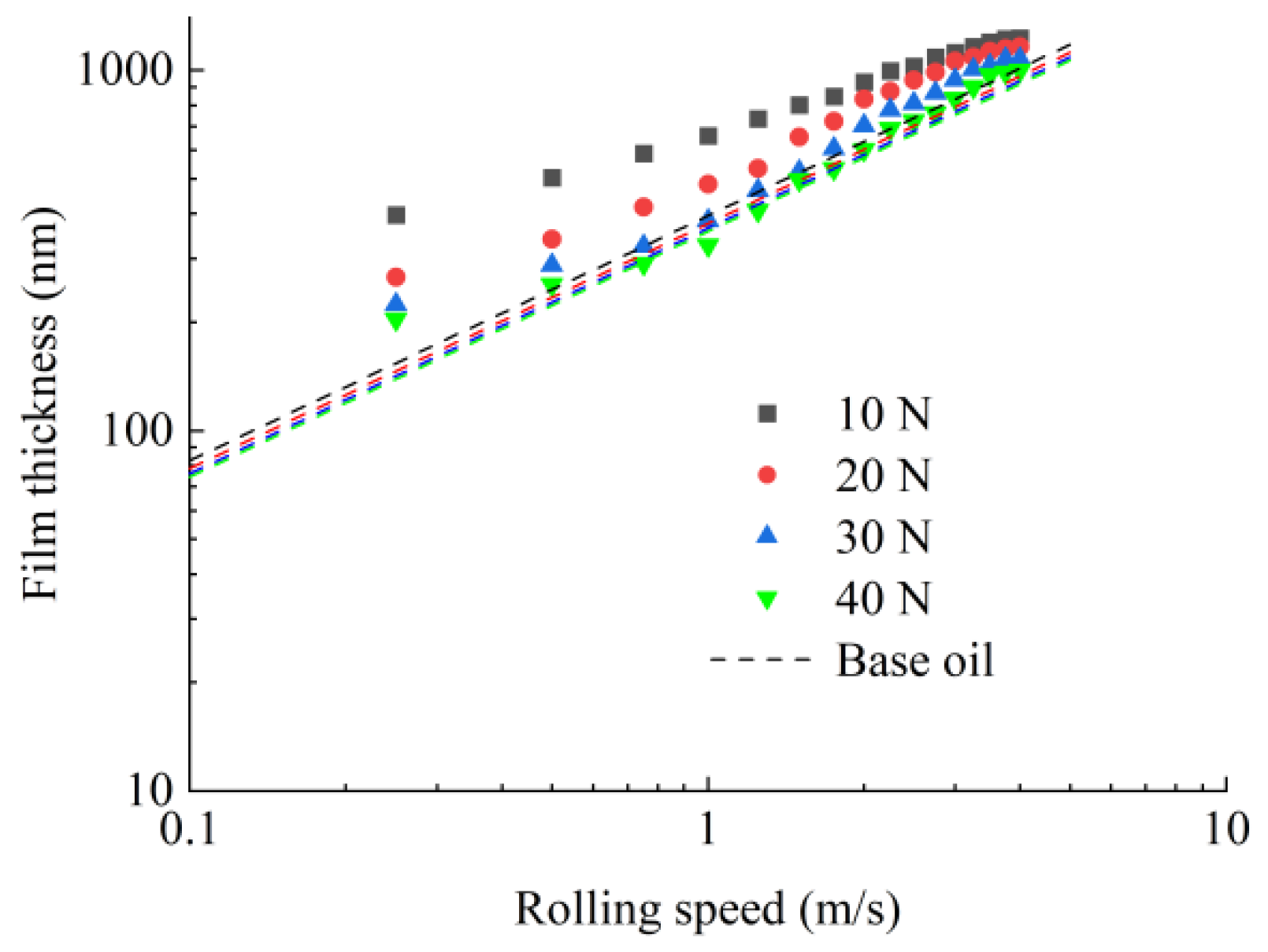
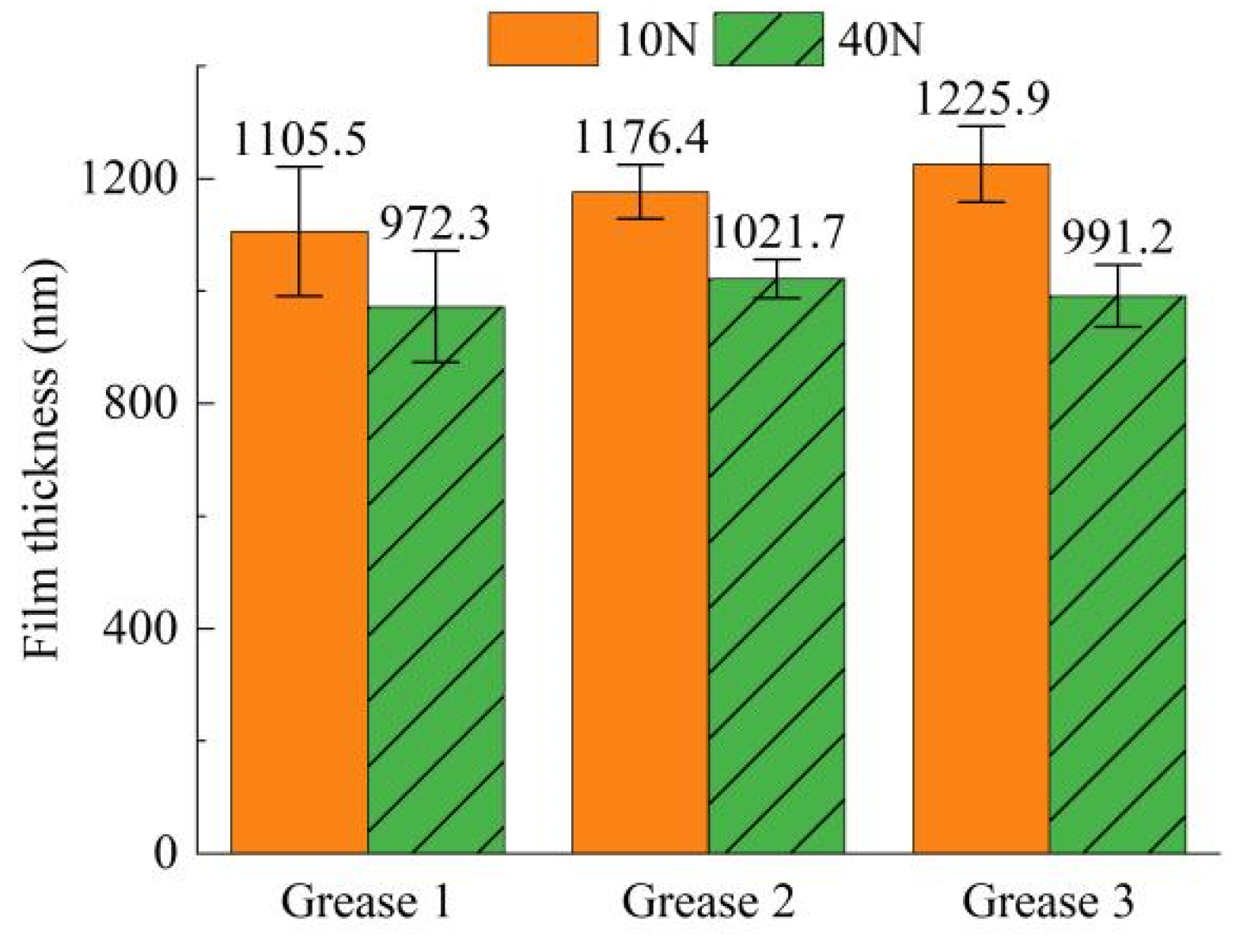
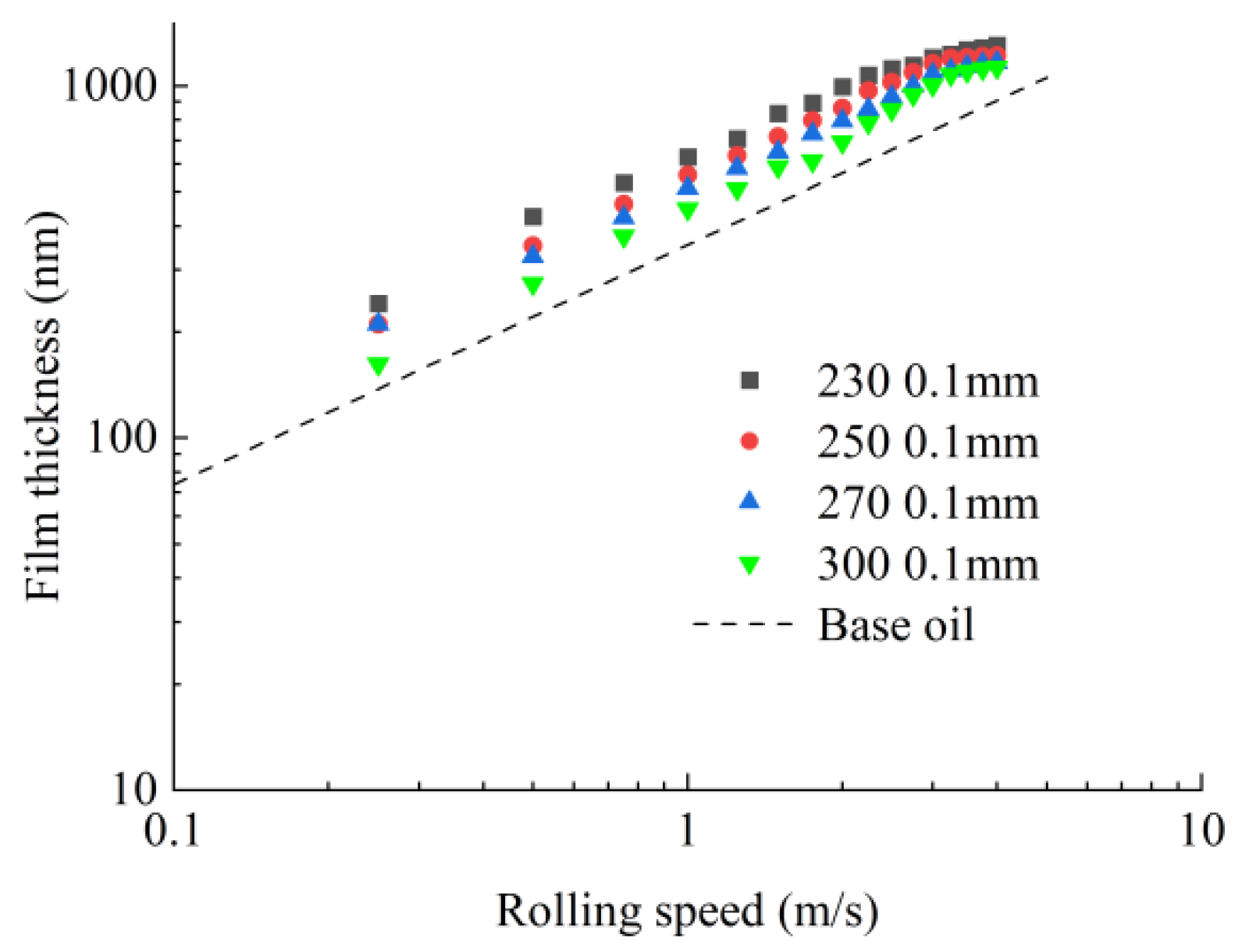
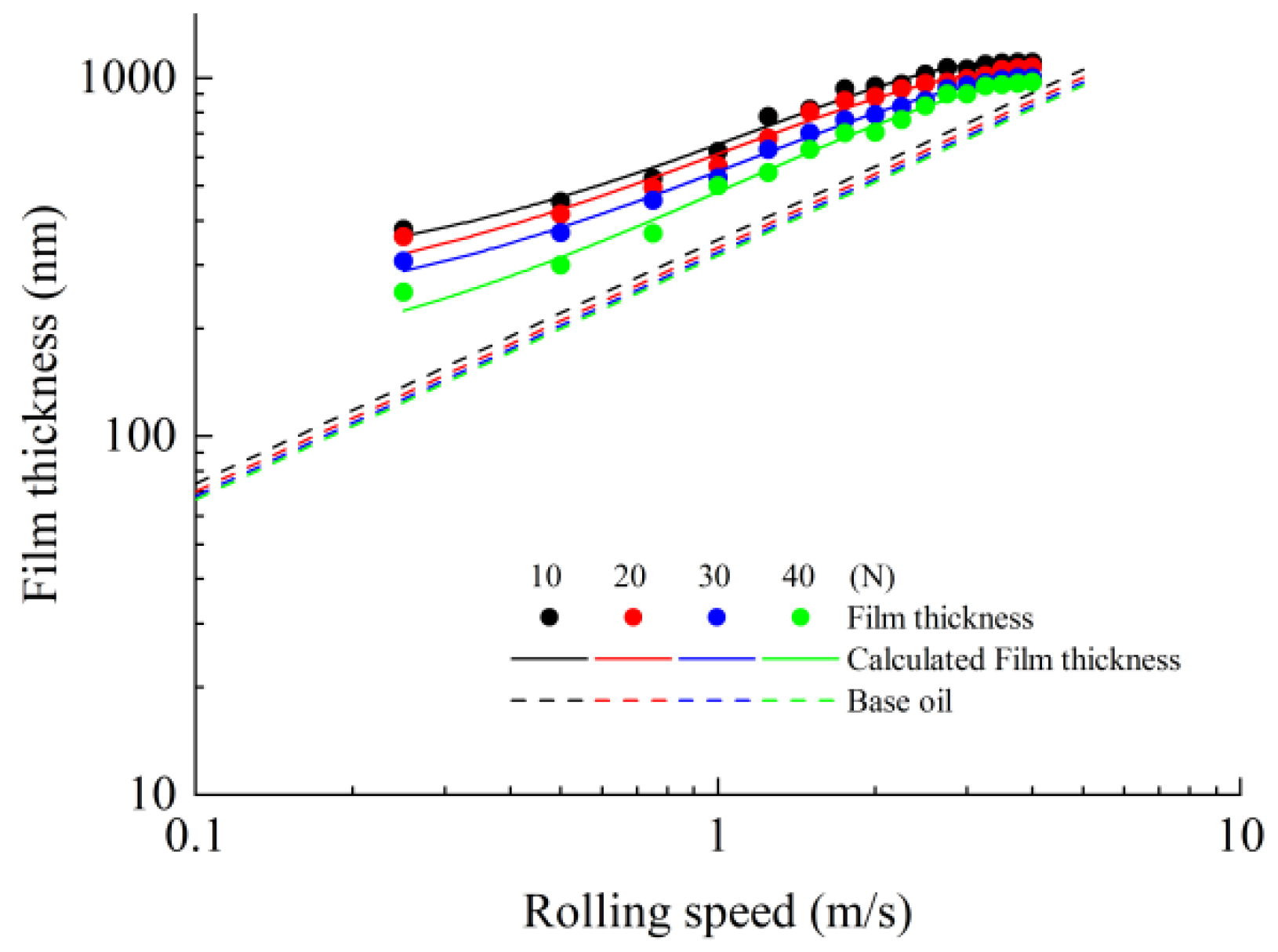
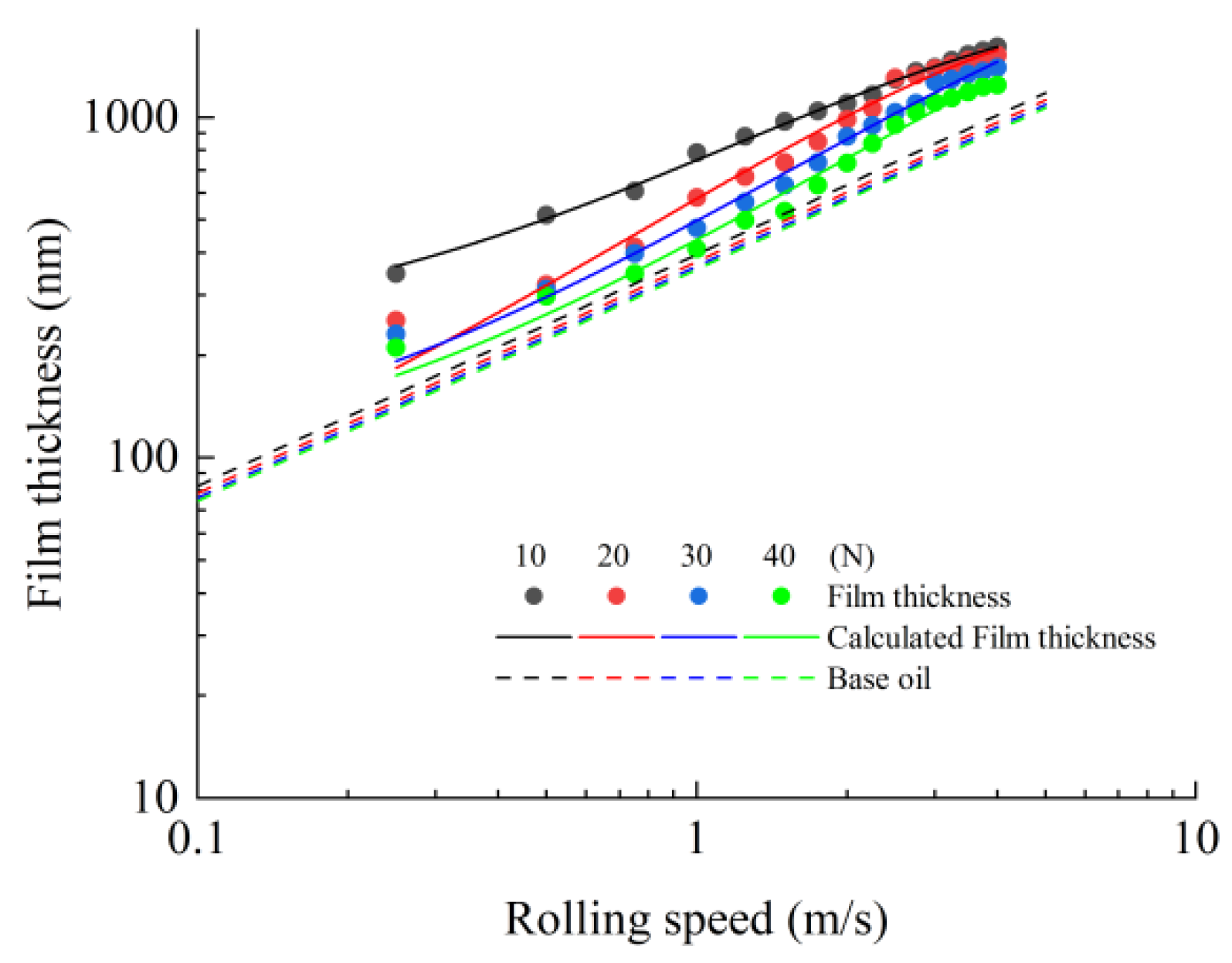
3.1.1. Effect of Rolling Speed and Normal Load on Grease Film Thickness
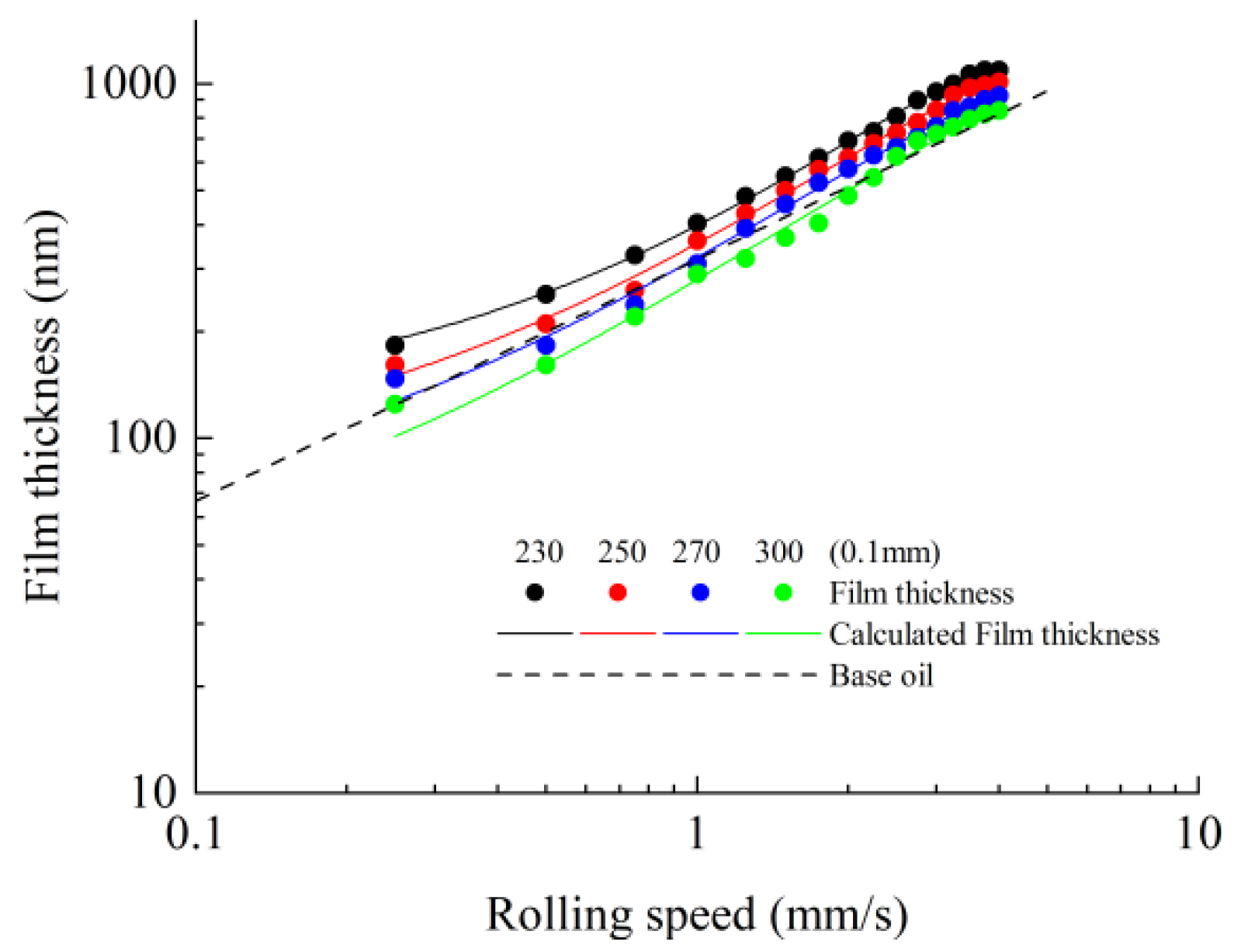
3.1.2. The Effect of Consistency on Grease Film Thickness
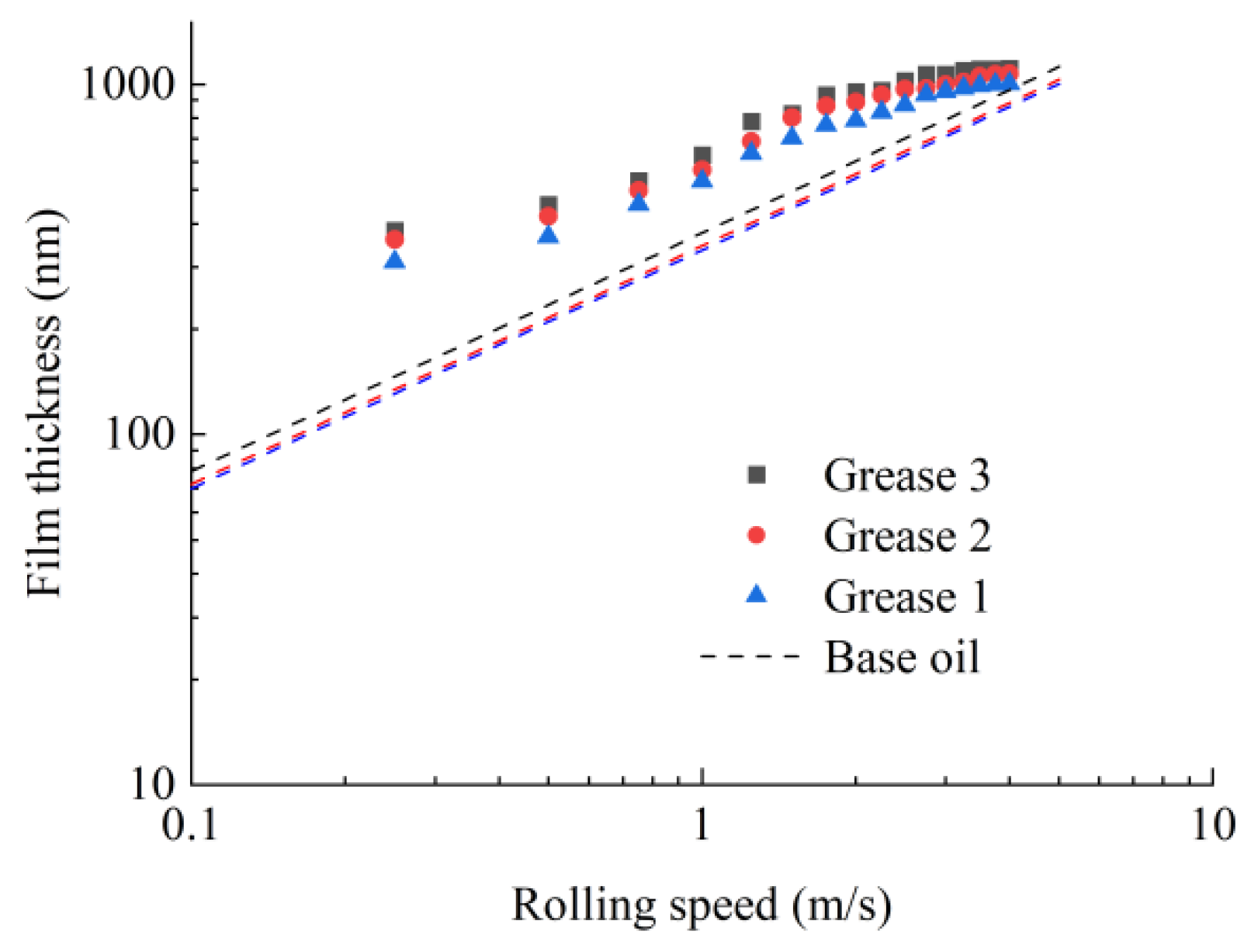
3.1.3. The Effect of Base Oil Type on Grease Film Thickness
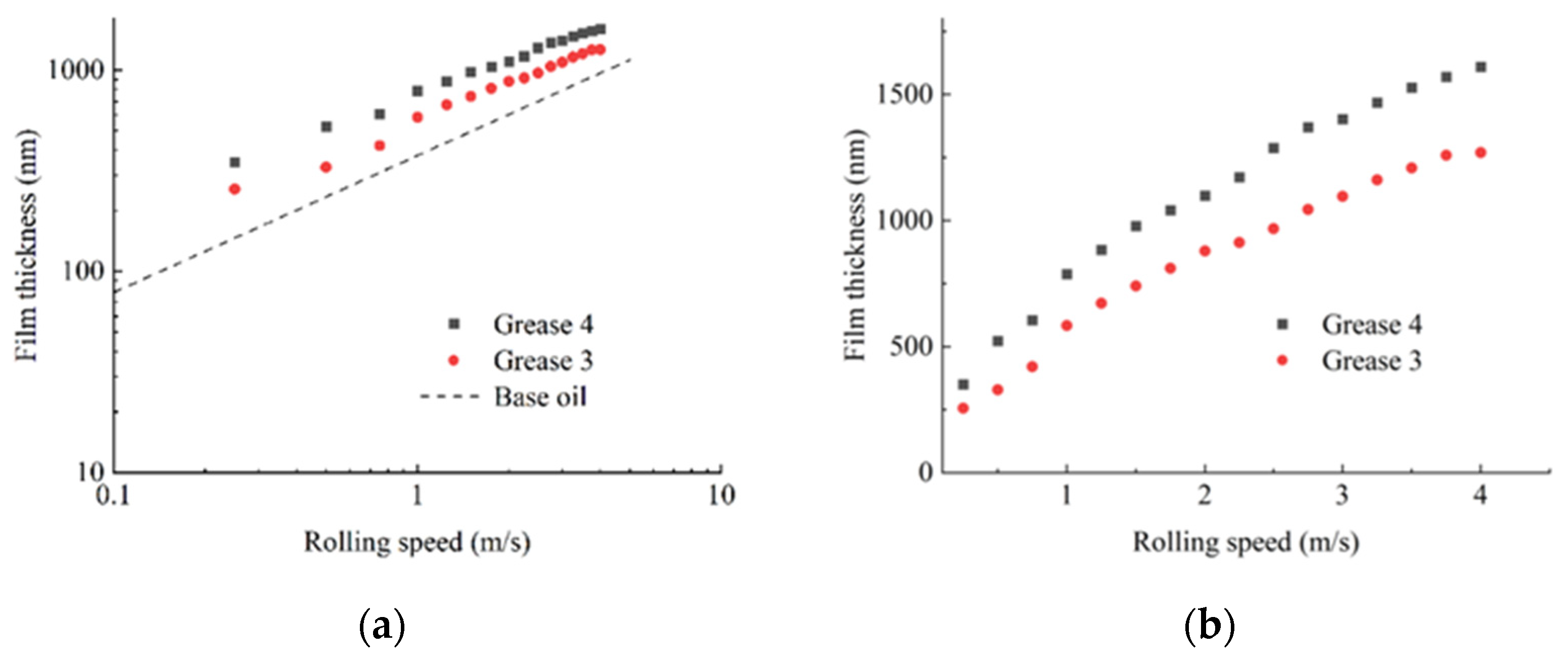
3.1.4. The Effect of Thickener Type on Grease Film Thickness
3.2. Discussion
4. Calculation Model for Grease Film Thickness
4.1. Mathematical Model
4.2. The Film Thickness Calculation Model for Two Greases
4.3. Verifying Calculation Model
5. Conclusions
- (1)
- The degree of film enhancement comparing to its base oil in fully flooded lubrication will depend on thickener type and consistency (or concentration);
- (2)
- In the high-speed region (above 0.25 m/s), when the rolling speed is less than 3 m/s, the grease film thickness increases with increasing rolling speed. The growth rate gradually decreases, then approaches to a fixed value when the rolling speed is beyond 3 m/s, which may be because at this point, the contact region is filled with enough thickener, and no more thickener can enter the region and the variation of grease film thickness remains almost unchanged with a further increase in speed;
- (3)
- The lithium-based grease with mineral oil forms the largest film thickness, followed by ester oil and the smallest by PAO oil. The larger the atmospheric viscosity and pressure-viscosity coefficient of the base oil, the higher the film thickness of the greases with the same thickener;
- (4)
- The grease film thicknesses with the same base oil and different thickeners are determined by the size of thickener particles at the same consistency or concentration. The fiber skeleton of mineral oil-polyurea grease is smaller than that of mineral oil-lithium grease, which results in a thicker film of mineral oil-polyurea grease than that of mineral oil-lithium grease;
- (5)
- The larger the consistency or concentration of thickeners (the smaller the cone penetration), the thicker the grease film thickness whose base oil has the same type and viscosity along with the same type of thickener. The reason is that the greater the consistency of the grease (the more the content of the thickener), the greater the effective viscosity of the grease at the contact and the thicker the grease film thickness;
- (6)
- A new calculation model for grease film thickness is proposed, which considers the influence factors such as working conditions, grease type and consistency. The calculation model has a high calculation accuracy and applicability to predict the grease film thickness compared to the base oil film thickness predicted by the Hamrock–Dowson formula.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Glovnea, R.P.; Olver, A.V.; Spikes, H.A. Experimental Investigation of the Effect of Speed and Load on Film Thickness in Elastohydrodynamic Contact. Tribol. Trans. 2005, 48, 328–335. [Google Scholar] [CrossRef]
- Křupka, I.; Hartl, M.; Liška, M. Influence of Contact Pressure on Central and Minimum Film Thickness Within Ultrathin Film Lubricated Contacts. J. Tribol.-Trans. Asme 2005, 127, 890–892. [Google Scholar] [CrossRef]
- Kochi, T.; Sakai, M.; Nogi, T.; Dong, D.; Kimura, Y. Experimental Study on the Physics of Thick EHL Film Formation with Grease at Low Speeds. Tribol. Lett. 2019, 67, 55. [Google Scholar] [CrossRef]
- Gerstenberger, J.; Poll, G. Rolling Bearing Lubrication with Grease at Low Temperatures. In Tribology Series, Proceedings of the 27th Leeds–Lyon Symposium on Tribology, Institut National des Sciences Appliquées de Lyon, Lyon, France, 5–8 September 2000; Dalmaz, G., Lubrecht, A.A., Dowson, D., Priest, M., Eds.; Elsevie: Amsterdam, The Netherlands, 2001; Volume 39, pp. 303–312. [Google Scholar] [CrossRef]
- Cen, H.; Lugt, P.M. Film Thickness in a Grease Lubricated Ball Bearing. Tribol. Int. 2019, 134, 26–35. [Google Scholar] [CrossRef]
- Eriksson, P.; Wikström, V.; Larsson, R. Grease Passing through an Elastohydrodynamic Contact under Pure Rolling Conditions. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2000, 214, 309–316. [Google Scholar] [CrossRef]
- Cann, P.M. Grease Lubrication of Rolling Element Bearings-Role of the Grease Thickener. Lubr. Sci. 2007, 19, 183–196. [Google Scholar] [CrossRef]
- Gonçalves, D.; Graça, B.; Campos, A.V.; Seabra, J.; Leckner, J.; Westbroek, R. On the Film Thickness Behaviour of Polymer Greases at Low and High Speeds. Tribol. Int. 2015, 90, 435–444. [Google Scholar] [CrossRef]
- Morales-Espejel, G.E.; Lugt, P.M.; Pasaribu, H.R.; Cen, H. Film Thickness in Grease Lubricated Slow Rotating Rolling Bearings. Tribol. Int. 2014, 74, 7–19. [Google Scholar] [CrossRef]
- Bhat, S.A.; Charoo, M.S. Effect of Additives on the Tribological Properties of Various Greases-A Review. Mater. Today Proc. 2019, 18, 4416–4420. [Google Scholar] [CrossRef]
- Vengudusamy, B.; Enekes, C.; Spallek, R. On the Film Forming and Friction Behaviour of Greases in Rolling/Sliding Contacts. Tribol. Int. 2019, 129, 323–337. [Google Scholar] [CrossRef]
- Fischer, D.; Jacobs, G.; Stratmann, A.; Burghardt, G. Effect of Base Oil Type in Grease Composition on the Lubricating Film Formation in EHD Contacts. Lubricants 2018, 6, 32. [Google Scholar] [CrossRef] [Green Version]
- Li, G. Study on Film-forming Mechanisms and Tribological Properties of Grease in the Harsh Conditions. Ph.D. Thesis, Tsinghua University, Beijing, China, 2010. [Google Scholar]
- Kimura, Y.; Endo, T.; Dong, D. EHL with Grease at Low Speeds. In Advanced Tribology: Proceedings of CIST2008 & ITS-IFToMM2008, Beijing, China, 24–27 September 2008; Luo, J., Meng, Y., Shao, T., Zhao, Q., Eds.; Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar] [CrossRef]
- Kanazawa, Y.; Sayles, R.S.; Kadiric, A. Film Formation and Friction in Grease Lubricated Rolling-Sliding Non-Conformal Contacts. Tribol. Int. 2017, 109, 505–518. [Google Scholar] [CrossRef]
- Saatchi, A.; Shiller, P.J.; Eghtesadi, S.A.; Liu, T.B.; Doll, G.L. A Fundamental Study of Oil Release Mechanism in Soap and Non-soap Thickened Greases. Tribol. Int. 2017, 110, 333–340. [Google Scholar] [CrossRef]
- Huang, L.; Guo, D.; Wen, S.; Wan, G.T.Y. Effects of Slide/Roll Ratio on the Behaviours of Grease Reservoir and Film Thickness of Point Contact. Tribol. Lett. 2014, 54, 263–271. [Google Scholar] [CrossRef]
- Yin, Y.; Duan, Q.; Ji, X.; Liu, Z. Grease Film Thickness Calculation and Analysis for Roller Bearing Clutch. Lubr. Eng. 2008, 33, 30–34+39. [Google Scholar]
- van Zoelen, M.T.; Venner, C.H.; Lugt, P.M. Prediction of Film Thickness Decay in Starved Elasto-hydrodynamically Lubricated Contacts Using a Thin Layer Flow Model. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2009, 223, 541–552. [Google Scholar] [CrossRef]
- Yang, Q.; Chen, Y.; Huang, P. A Novel Method to Determine EHL Film Thickness with Optical Interference. Appl. Mech. Mater. 2013, 456, 549–554. [Google Scholar] [CrossRef]
- Xue, H.; Wang, J.; Hong, Y. Elastohydrodynamic Lubrication Analysis on Line Contact Lubricated with Grease. Lubr. Eng. 2017, 42, 12–16+33. [Google Scholar]
- Wang, D.; Yang, J.; Wei, P.; Pu, W. A Mixed EHL Model of Grease Lubrication Considering Surface Roughness and the Study of Friction Behavior. Tribol. Int. 2021, 154, 106710. [Google Scholar] [CrossRef]
- Hamrock, B.J.; Dowson, D. Isothermal Elastohydrodynamic Lubrication of Point Contacts, Part III—Fully Flooded Results. J. Tribol.-Trans. Asme 1977, 99, 264–276. [Google Scholar] [CrossRef]
- Vengudusamy, B.; Kuhn, M.; Rankl, M.; Spallek, R. Film Forming Behaviour of Greases under Starved and Fully Flooded EHL Conditions. Tribol. Trans. 2016, 59, 62–71. [Google Scholar] [CrossRef]
- DeLaurentis, N.D.; Kadiric, A.; Lugt, P.; Cann, P. The Influence of Bearing Grease Composition on Friction in Rolling/Sliding Concentrated Contacts. Tribol. Int. 2016, 94, 624–632. [Google Scholar] [CrossRef] [Green Version]
- Cyriac, F.; Lugt, P.M.; Bosman, R.; Padberg, C.J.; Venner, C.H. Effect of Thickener Particle Geometry and Concentration on the Grease EHL Film Thickness at Medium Speeds. Tribol. Lett. 2016, 60, 18. [Google Scholar] [CrossRef] [Green Version]
- Gunsel, S.; Korcek, S.; Smeeth, M.; Spikes, H.A. The Elastohydrodynamic Friction and Film Forming Properties of Lubricant Base Oils. Tribol. Trans. 1999, 42, 559–569. [Google Scholar] [CrossRef]
- Cann, P.; Lubrecht, A.A. An analysis of the mechanisms of grease lubrication in rolling element bearings. Lubr. Sci. 1999, 11, 227–245. [Google Scholar] [CrossRef]




| Type of Grease | Thickener | Base Oil | Cone Penetration (0.1 mm) | Concentration (Mass %) |
|---|---|---|---|---|
| Grease 1 | Lithium 12-Hydroxystearate | PAO oil | 270 | 11% |
| Grease 2 | Lithium 12-Hydroxystearate | Ester oil | 270 | 12% |
| Grease 3 | Lithium 12-Hydroxystearate | Mineral oil | 270 | 8% |
| Grease 4 | Cyclohexylamine, octadecylamine and isocyanate | Mineral oil | 270 | 9% |
| Grease 5 | Cyclohexylamine, octadecylamine and isocyanate | PAO oil | 230 250 270 300 | 13% 12% 11% 9% |
| ɑ | β | μ | δ |
|---|---|---|---|
| A0: 2.2698 × 10−11 | B0: 1.2613 × 10−12 | C0: −2.0530 × 10−9 | D0: 3.5269 × 10−8 |
| A1: 9.1593 × 10−6 | B1: 1.0021 × 10−4 | C1: 8.2771 × 10−4 | D1: 1.7401 × 10−3 |
| A2: −2.63 × 10−2 | B2: −2.46 × 10−2 | C2: −2.72 × 10−2 | D2: −3.47 × 10−2 |
| ɑ | β | μ | δ |
|---|---|---|---|
| A0: −4.5269 × 10−11 | B0: 1.6603 × 10−12 | C0: 3.2045 × 10−8 | D0: 4.0610 × 10−8 |
| A1: 2.5547 × 10−4 | B2: 1.4218 × 10−4 | C1: 9.9042 × 10−5 | D1: 1.0156 × 10−4 |
| A2: −0.5028 | B2: −0.4626 | C2: −0.4548 | D2: −0.4791 |
| A3: −2.9881 × 10−2 | B3: −3.9442 × 10−2 | C3: −3.1540 × 10−2 | D3: −2.7927 × 10−2 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Z.; Wang, Y.; Lin, J.; Wang, D. Study on Factors Influencing Film Formation of Grease and Calculation Model for Grease Film Thickness. Lubricants 2022, 10, 123. https://doi.org/10.3390/lubricants10060123
Zhang Z, Wang Y, Lin J, Wang D. Study on Factors Influencing Film Formation of Grease and Calculation Model for Grease Film Thickness. Lubricants. 2022; 10(6):123. https://doi.org/10.3390/lubricants10060123
Chicago/Turabian StyleZhang, Zhe, Yanshuang Wang, Jianghai Lin, and Dongfeng Wang. 2022. "Study on Factors Influencing Film Formation of Grease and Calculation Model for Grease Film Thickness" Lubricants 10, no. 6: 123. https://doi.org/10.3390/lubricants10060123
APA StyleZhang, Z., Wang, Y., Lin, J., & Wang, D. (2022). Study on Factors Influencing Film Formation of Grease and Calculation Model for Grease Film Thickness. Lubricants, 10(6), 123. https://doi.org/10.3390/lubricants10060123






