Thermo-Hydrodynamic Lubrication Analysis of Slipper Pair Considering Wear Profile
Abstract
:1. Introduction
2. System theory
2.1. Definition of the Coordinate System
2.2. Kinematic Analysis
2.3. Reynolds Equation
2.4. Non-Uniform Clearance of Oil Film Thickness
2.5. Force Analysis
2.6. Energy and Heat Transfer Equation
2.7. Viscosity Temperature Equation
2.8. Flow Balance Equation
3. Numerical Solution
4. Results
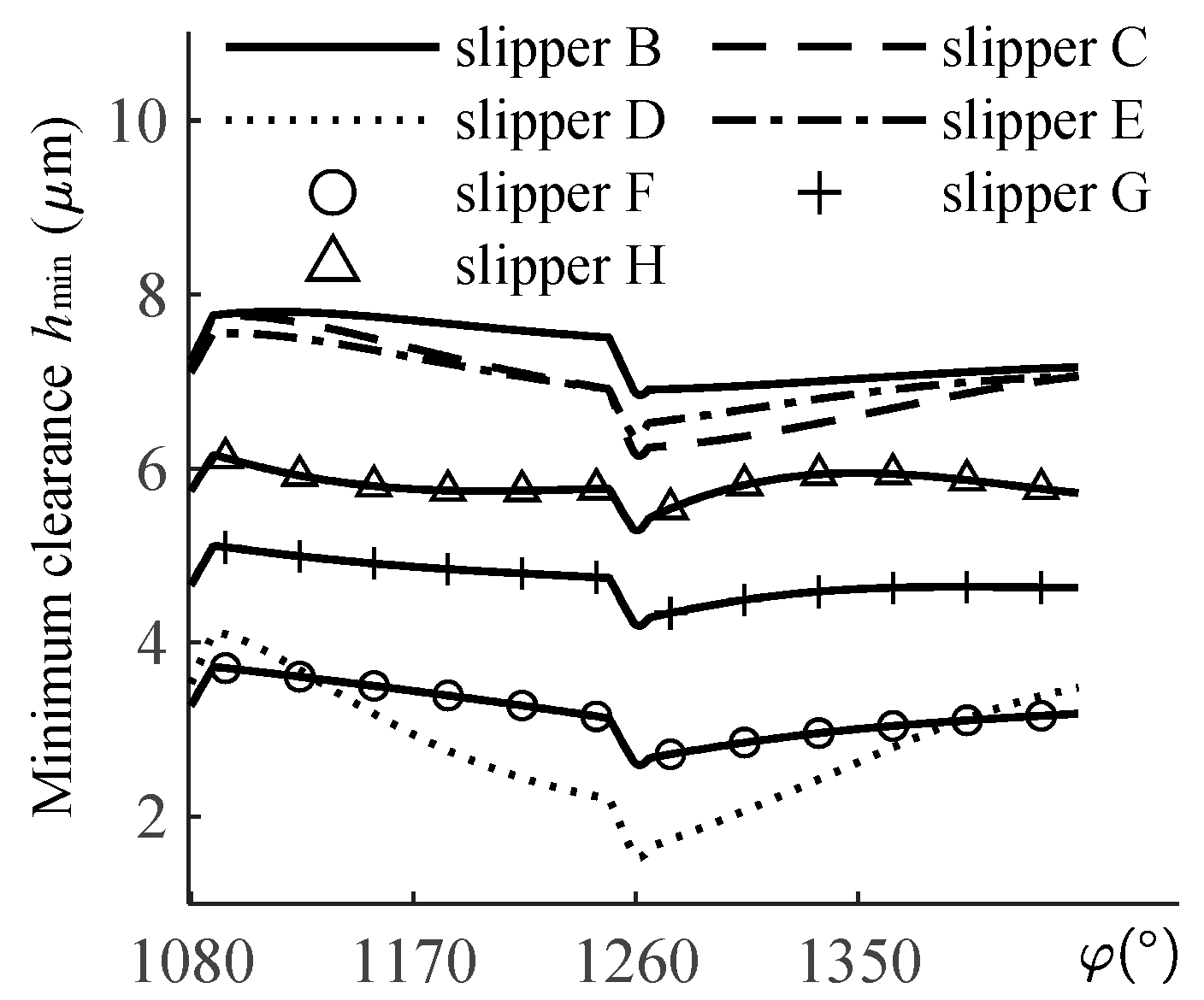
4.1. Effect of Wear Profile on the Thickness under Slipper Sealing Land
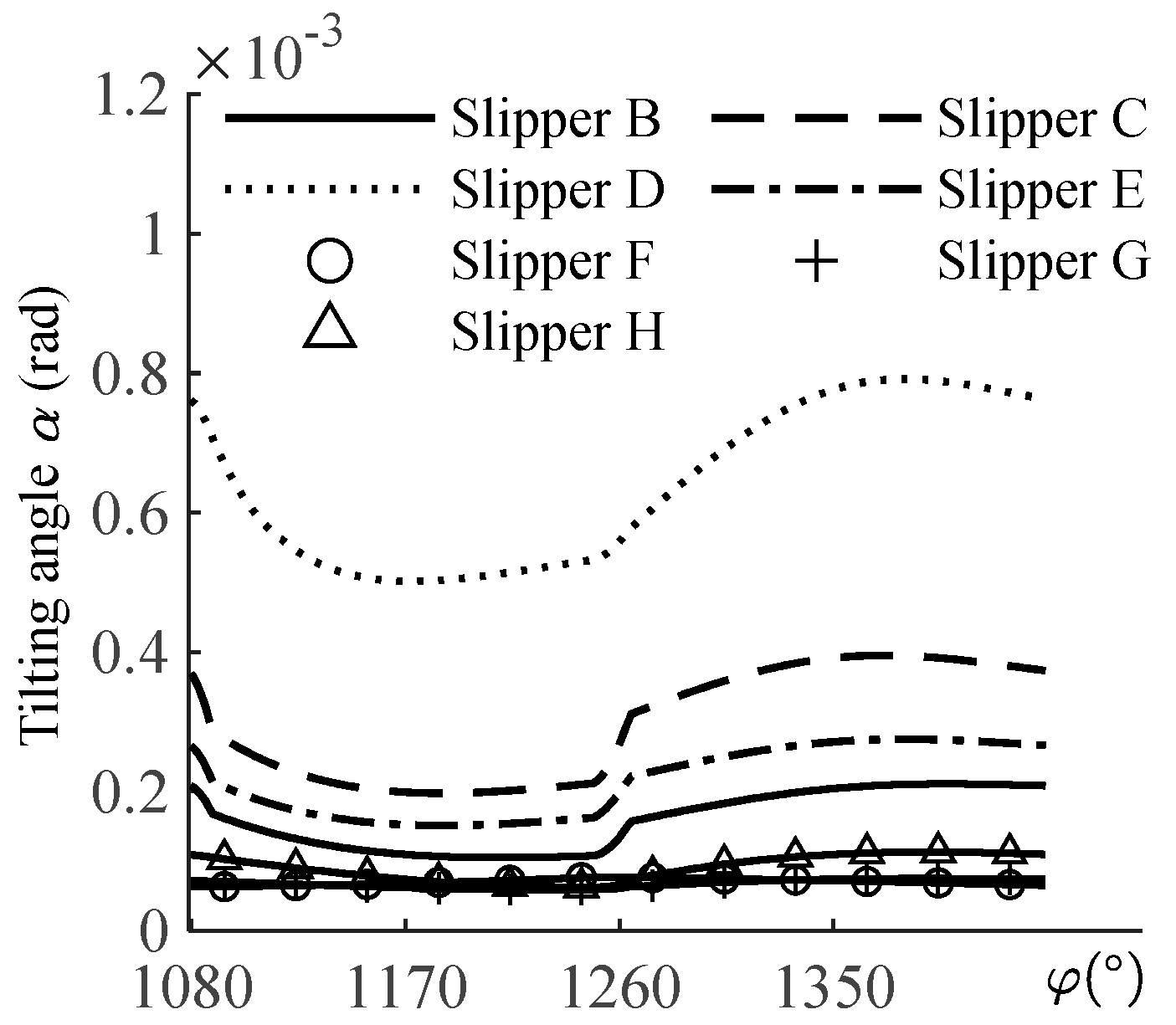
4.2. Effect of Wear Profile on the Sliding Attitude of the Slipper
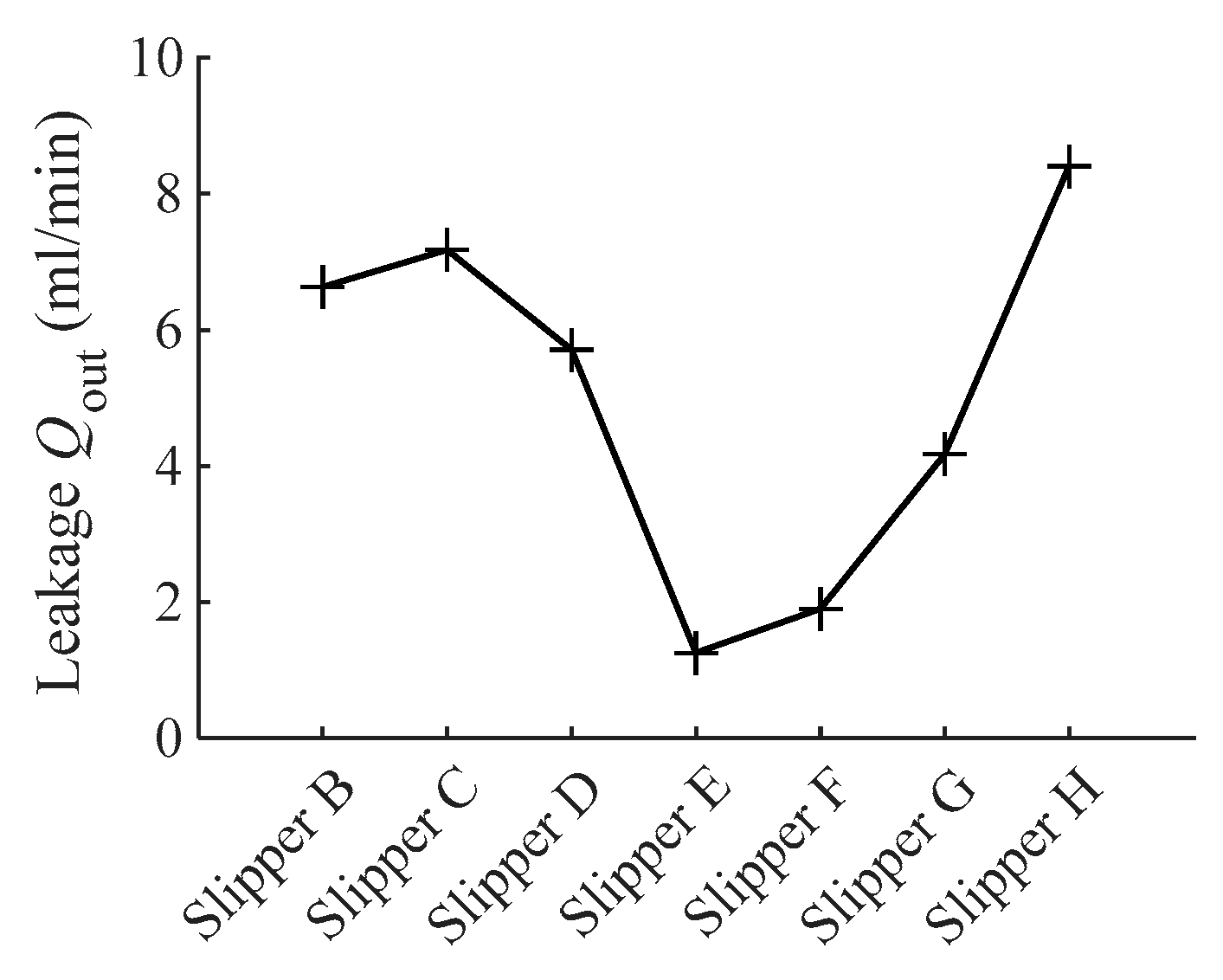
4.3. Effect of Wear Profile on Leakage of Slipper
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Ransegnola, T.; Shang, L.; Vacca, A. A study of piston and slipper spin in swashplate type axial piston machines. Tribol. Int. 2022, 167, 107420. [Google Scholar] [CrossRef]
- Xu, B.; Zhang, J.H. Effect of case drain pressure on slipper/swashplate pair within axial piston pump. J. Zhejiang Univ.—Sci. A Appl. Phys. Eng. 2015, 16, 1001–1014. [Google Scholar] [CrossRef]
- Wu, H.C.; He, Y.Y.; Jian, X.S. Numerical Analysis of Influence of Slipper Bottom Structure on Oil Film Performance of Slipper Pair of Axial Piston Pump. Lubr. Eng. 2014, 39, 1–5. [Google Scholar]
- Li, Z.; Xu, S.; Gong, G.; Bi, Y.; Xu, L.; Zhang, L.; Ren, Z. Study on Friction Characteristics of Slipper Pair of Large Displacement High-Pressure Piston Pump. Lubricants 2022, 10, 363. [Google Scholar] [CrossRef]
- Mo, H.; Guo, Y.; Quan, S. Effect of Texture on Total Energy Consumption of High, Frequency Hydraulic Impact Piston Pair. Coatings 2022, 12, 340. [Google Scholar] [CrossRef]
- Chao, Q.; Zhang, J.; Xu, B.; Wang, Q.; Huang, H. Test rigs and experimental studies of the slipper bearing in axial piston pumps: A review. Measurement 2018, 132, 135–149. [Google Scholar] [CrossRef]
- Harris, R.; Edge, K.A.; Tilley, D.G. Predicting the behavior of slipper pads in swashplate-type axial piston pumps. J. Dyn. Syst. Meas. Control. ASME 1996, 118, 41–47. [Google Scholar] [CrossRef]
- Borghi, M.; Specchia, E.; Zardin, B. Numerical analysis of the dynamic behavior of axial piston pumps and motors slipper bearings. Int. J. Passeng. Cars–Mech. Syst. SAE 2009, 2, 1285–1302. [Google Scholar] [CrossRef]
- Hooke, C.J.; Kakoullis, Y.P.S. The Effects of centrifugal load and ball friction on the lubrication of slippers in axial piston pumps. In Proceedings of the 6th International Fluid Power Symposium, Cambridge, UK, 8–10 April 1981. [Google Scholar]
- Hooke, C.J.; Kakoullis, Y.P. The Effects of non-flatness on the performance of slippers in Axial Piston Pumps. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 1983, 197, 239–247. [Google Scholar] [CrossRef]
- Manring, N.D.; Johnson, R.E.; Cherukuri, H.P. The impact of linear deformations on stationary hydrostatic thrust bearings. J. Tribol. ASME 2002, 124, 874–877. [Google Scholar] [CrossRef]
- Wieczorek, U.; Ivantysynova, M. CASPAR—A Computer Aided Design Tool for Axial Piston Machines. In Proceedings of the Bath Workshop on Power Transmission and Motion Control PTMC 2000, Bath, UK, 1 November 2000; pp. 113–126. [Google Scholar]
- Schenk, A. Predicting Lubrication Performance between the Slipper and Swashplate in Axial Piston Hydraulic Machines; Purdue University: West Lafayette, IN, USA, 2014. [Google Scholar]
- Schenk, A.; Ivantysynova, M. A Transient Thermoelastohydrodynamic Lubrication Model for the Slipper/Swashplate in Axial Piston Machines. J. Tribol. 2015, 137, 031701. [Google Scholar] [CrossRef]
- Liu, H.; Yuan, S.H.; Jing, C.B.; Zhao, Y. Effects of Wear Profile and Elastic Deformation on the Slipper’s Dynamic Characteristics. J. Mech. Eng. 2013, 49, 75–83. [Google Scholar] [CrossRef]
- Koc, E.; Hooke, C.J.; Li, K.Y. Slipper Balance in Axial Piston Pumps and Motors. J. Tribol. 1992, 114, 766–772. [Google Scholar] [CrossRef]
- Ivantysyn, R.; Shorbagy, A.; Weber, J. Investigation of the wear behavior of the slipper in an axial piston pump using simulation and measurement. In Proceedings of the 12th International Fluid Power Conference 2020, Dresden, Germany, 9–11 March 2020; pp. 315–326. [Google Scholar]
- Darbani, A.A.; Shang, L.; Beale, J.R.; Ivantysynova, M. Slipper Surface Geometry Optimization of the Slipper/Swashplate Interface of Swashplate-Type Axial Piston Machines. Int. J. Fluid Power 2019, 20, 245–270. [Google Scholar] [CrossRef]
- Chao, Q.; Zhang, J.; Xu, B.; Wang, Q.; Lyu, F.; Li, K. Integrated slipper retainer mechanism to eliminate slipper wear in high-speed axial piston pumps. Front. Mech. Eng. 2022, 17, 1. [Google Scholar] [CrossRef]
- Li, H.L.; Liu, F.G.; Zhang, B.M. Improvement of Slipper Wear Failure Mode. Hydraul. Pneum. Seals 2015, 35, 75–78. [Google Scholar]
- Zhang, J.; Chao, Q.; Wang, Q.; Xu, B.; Chen, Y.; Li, Y. Experimental investigations of the slipper spin in an axial piston pump. Measurement 2017, 102, 112–120. [Google Scholar] [CrossRef]
- Koc, E.; Hooke, C.J. Considerations in the design of partially hydrostatic slipper bearings. Tribol. Int. 1997, 30, 815–823. [Google Scholar] [CrossRef]
- Tang, H.S.; Yin, Y.B.; Liu, J. Thermal-structural coupling characteristics of axial piston pump slipper pair. J. Aerosp. Power 2016, 31, 1913–1920. [Google Scholar]
- Chao, Q. Derivation of the Reynolds equation in cylindrical coordinates applicable to the slipper/swash plate interface in axial piston pumps. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2020, 235, 798–807. [Google Scholar] [CrossRef]
- Christensen, H. Elastohydrodynamic Theory of Spherical Bodies in Normal Approach. Trans. Asme J. Lub. 1970, 92, 145–154. [Google Scholar] [CrossRef]
- Tang, H.S.; Ren, Y.; Xiang, J.W. A novel model for predicting thermoelastohydrodynamic lubrication characteristics of slipper pair in axial piston pump. Int. J. Mech. Sci. 2017, 124–125, 109–121. [Google Scholar] [CrossRef]
- Chao, Q.; Xu, Z.; Tao, J.; Liu, C. Capped piston: A promising design to reduce compressibility effects, pressure ripple and cavitation for high-speed and high-pressure axial piston pumps. Alex. Eng. J. 2023, 62, 509–521. [Google Scholar] [CrossRef]
- Tang, H.S.; Yin, Y.B.; Zhang, Y.; Li, J. Parametric analysis of thermal effect on hydrostatic slipper bearing capacity of axial piston pump. J. Cent. South Univ. 2016, 23, 333–343. [Google Scholar] [CrossRef]
- Roelands, C.J.A.; Vulger, J.C.; Waterman, H.I. The Viscosity-Temperature-Pressure Relationship of Lubricating Oils and Its Correlation with Chemical Constitution. Trans. ASME J. Trib. Tech. 1963, 85, 601–605. [Google Scholar] [CrossRef]
- Xu, Y.M. Oil Film Theory and the Design of Friction Pairs for Hydraulic Pumps and Motors; China Machine Press: Beijing, China, 1987; pp. 25–27. [Google Scholar]
- Liu, S.; Hu, J. Tribo-dynamic model of slipper bearing. Appl. Math. Model. 2015, 39, 548–558. [Google Scholar]
- Fu, J.F.; Li, H.C.; Zeng, X.Q.; Liu, X. Numerical simulation of hybrid lubrication characteristics of slipper pair of aviation fuel piston pump. J. Beijing Univ. Aeronaut. Astronaut. 2018, 44, 939–950. [Google Scholar]
- Hu, J.B.; Zhou, H.C.; Wei, C.; Liu, H. Theoretical and Experimental Research on the Formation of Lubrication Film for Slipper Bearings of Axial Piston Pumps. Trans. Beijing Inst. Technol. 2015, 35, 231–235. [Google Scholar]








| Name | Unit | Value |
|---|---|---|
| Inner radius of sealing land r1 | mm | 6.4 |
| Outer radius of sealing land r2 | mm | 12.8 |
| Radius of ball head R | mm | 44 |
| Outer radius of piston rp | mm | 9.5 |
| Pressure of piston pp | Mpa | 10 |
| Angular velocity of ball head ω | rpm | 1500 |
| Inclination of swash plate β | ° | 14 |
| Initial viscosity of hydraulic oil μ0 | mm2/s | 30.69 |
| Types | Profile Parameters | Types | Profile Parameters | ||||
|---|---|---|---|---|---|---|---|
| Δhi μm | Δho μm | λr | Δhi μm | Δho μm | λr | ||
| A | 0 | 0.01 | 0.1 | E | 0 | 2 | 0.5 |
| B | 0 | 1 | 0.1 | F | 1 | 1 | 0.5 |
| C | 0 | 2 | 0.1 | G | 2 | 2 | 0.5 |
| D | 0 | 8 | 0.1 | H | 5 | 5 | 0.5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mo, H.; Hu, Y.; Quan, S. Thermo-Hydrodynamic Lubrication Analysis of Slipper Pair Considering Wear Profile. Lubricants 2023, 11, 190. https://doi.org/10.3390/lubricants11050190
Mo H, Hu Y, Quan S. Thermo-Hydrodynamic Lubrication Analysis of Slipper Pair Considering Wear Profile. Lubricants. 2023; 11(5):190. https://doi.org/10.3390/lubricants11050190
Chicago/Turabian StyleMo, Hu, Yanping Hu, and Song Quan. 2023. "Thermo-Hydrodynamic Lubrication Analysis of Slipper Pair Considering Wear Profile" Lubricants 11, no. 5: 190. https://doi.org/10.3390/lubricants11050190
APA StyleMo, H., Hu, Y., & Quan, S. (2023). Thermo-Hydrodynamic Lubrication Analysis of Slipper Pair Considering Wear Profile. Lubricants, 11(5), 190. https://doi.org/10.3390/lubricants11050190




