1. Introduction
As stated in Amontons’ law and Reyes–Archard’s’ law, friction and wear in many mechanical contacts turn out to be approximately proportional to the load but are independent of the apparent contact area, unless normal stresses are very large [
1,
2]. These two observations can be easily rationalized for randomly rough surfaces [
3]: The distribution of contact stresses in macroscopic contacts—when normalized to the true contact area—barely changes with load, unless the relative contact area
clearly exceeds 10% [
4,
5,
6]. Thus, increasing the normal load does not mean (much) more extreme local contact stresses but a larger number of contact spots [
5,
7,
8]. Loosely speaking, increasing load leads to more of the same, i.e., doubling the load—at small loads—is expected to induce roughly twice the number of contact patches having a given real contact area
A. Amontons’ law and Reyes–Archard’s law follow unless friction and wear processes are non-local or sliding dynamics [
9] or wear [
10,
11] are strongly effected by the number-density of contact patches.
The analysis of the contact-patch-size distribution
supposedly started with an experimental work by Dieterich and Kilgore [
12], who found evidence for an algebraic decay with increasing patch area
A—in contacts between quartz, calcite, and glass with acrylic plastic—according to
The value of the exponent
was found to be between one and three. However, when the fitting range included large domains, a value of
turned out to be most likely. Simple models for contacts produced by cuts through randomly rough, self-affine surfaces [
13,
14] also found power law distributions, in which the exponent
was found to obey
, where
H is the Hurst roughness exponent, which is defined in the
Section 2.2. This result reproduced the experimental observation of an increasing exponent
with decreasing
H. First contact-mechanics simulations of randomly rough rough surfaces could not confirm this trend [
7]. However, in hindsight, these simulations may have suffered from insufficiently fine spatial discretization cells, and perhaps more importantly—given the results of this study—the power law may have been fitted to the branch of the
distribution on which
A is too large to obey the power-law scaling. Later, the same group considered larger-scale simulations as well as finer resolution and also included plasticity, which led them to revise their estimate to
[
5].
Ten years ago, Campaña [
8] ran even larger-scale simulations of system sizes going up to
discretization points of the elastic solid, allowing him to obtain good resolution of contact at small scales. This proved to be important to identify a new scaling regime at small
A, where
turned out approximately constant and where individual clusters on that branch obeyed the area-load relation for Hertzian contact, namely
. For larger-scale clusters, he identified the scaling relation of Equation (
1) to hold. These patches showed a linear
relation on an
individual basis. Campaña’s data were again consistent with a decrease of
with
H, however, no clear claim was made, or could be made, about whether the
relation also applied to elastic contacts. Similarly, a recent study by Molinari’s group [
15] found
to be close to but not necessarily identical with the exponent obtained from cuts through Gaussian surfaces. As Campaña superimposed different distributions function
for different loads, the impression could arise that the predominant effect of doubling the load is an approximate doubling the number of contact patches of a given size.
The increasing-load-only-leads-to-more-of-the-same dictum cannot be exact. For any given finite contact, there exists a largest contact patch, the size of which should generally increase with load. Two growth mechanisms exist: A continuous growth process due to contact lines being pushed out further when a patch carries more load—as it happens, for example, in an isolated Hertzian contact—and a discontinuous mechanism, which is due to the merging of two patches into a single one. The latter process requires contact formation at a saddle point, as described in Ref. [
16], and as modeled quantitatively in an advanced bearing model, which accounts for the merging of and the elastic interaction between contact patches [
17,
18].
The existence of a largest patch implies that the
cannot extend to arbitrarily large
A. In fact, numerical data produced for
with high local resolution reveals a strong reduction of
for large patch sizes compared to the self-affine scaling regime [
8,
15,
19]. This brings up the question of whether there is a characteristic patch size
above which the scaling relation no longer holds even in an infinitely large system, or, whether the existence of a largest cluster is merely a finite-size effect, as a prominent theorist suggested in a personal communication to the authors.
In this paper, we argue that there is a maximum characteristic patch size, above which the relation breaks down. The main reason, on which we also base our estimate for characteristic contact areas is related to the following argument: Contact seizes to be connected with a significant probability when the expected (square of the) stress at a distance r from a given contact point has fallen below the (square of the) pressure , at which contact percolates macroscopically. By definition, this distance is finite when the mean contact pressure is less than and infinite (in the thermodynamic limit) when .
The remainder of the article is organized as follows:
Section 2 introduces our contact model and the methods with which we solve the model; it defines the quantities of interest and also contains our theoretical estimates on the range of validity for the self-affine scaling regime. Results are presented in
Section 3, while conclusions are drawn in
Section 4.
3. Results
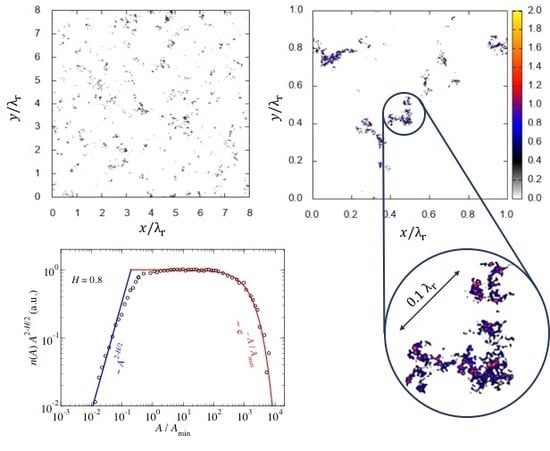
To set the stage for a later discussion, results of a medium-sized contact-mechanics simulation are shown in
Figure 3, in which the relative contact area has been obtained to
. The figure reveals that the real contact area becomes roughly isotropic for a
surface when averaged over apparent contacts clearly exceeding
. It also shows that there are many more small than large contact patches. However, most contact points belong to large patches. Moreover, the linear size of the largest cluster shown in the circular call-out box corresponds roughly to twice the value of
r, at which
has fallen to
, which defines a rough estimate of a characteristic patch radius.
We begin our quantitative analysis with a reproduction of the trends published by Campaña on the load-area relation of individual contact patches in
Figure 4. An added value with respect to the original data is the realization that the estimate for the contact area at which the Hertzian
scaling crosses over to the linear
relation for self-affine patches is quite reasonable. After coarse graining load and area over many patches in an interval
, we always found the
running averages to lie within 20% of the running averages, deviations being obviously largest near the transition from the Hertzian to the self-affine branch.
Figure 4 also gives a clue as to why the dimensionless proportionality coefficient
increases with decreasing
H. A smaller value for
H clearly leads to more small patches lying above the (extrapolated) line
, which was drawn in
Figure 4 both times assuming a value of
. At the same time, the relative weight of large clusters becomes smaller. It turns out that
is not sensitive to the dimensionless numbers
and
as long as the latter remains sufficiently large in order for the surface to qualify as self-affine.
We next analyze the contact-patch-size distribution
in
Figure 5. The product
states the probability that a randomly picked contact patch has a size between
A and
, where
is assumed to be infinitesimally small. When measuring
at sufficiently small loads, we find that all data, i.e., many more datasets than the ones shown in
Figure 5, are consistent with the Equation (
1) and
dependence identified for cuts through Gaussian surfaces
in a non-negligible range of values for
.
An exponent in the range
indicates that the mean contact area is determined by the small patches on the self-affine branch, while the characteristic contact area is determined by the large patches and strongly affected by the value
above which power law scaling no longer holds. We also note that the contact area at which the
scaling relation changes is within a few 10% of the contact area at which the
relation crosses from Hertzian to linear. In addition, the probability of very large clusters to occur is strongly suppressed compared to the scaling at intermediate
A. In fact, for
, the relation
where
is a fitting parameter turning out close to
, gives a satisfactory representation of the complete range of
-data for
and
, provided the reference area
is appropriately chosen.
The next question to be tackled is to what degree the characteristic patch size changes with the normal pressure. The increasing-load-only-leads-to-more-of-the-same dictum is certainly counterintuitive as each existing patch, including the largest one, is expected to grow with increasing load.
Figure 6 reveals that
in fact increases with pressure from small to large loads. The pressure dependence of
becomes particularly significant near the percolation transition, above which
is macroscopically large, whereas it is finite (even in an infinite system) for
.
It is interesting to note that, near the percolation threshold, the relation for both and appears to satisfy a power law with a value of . At very small pressures, however, the functional form of —and thus of —seems to differ between and . The is consistent with a rather weak exponential increase of with , while the low-pressure data are better described with a power law and .
Before discussing the results in detail, we note that acquiring statistics is a non-trivial undertaking, since a few large clusters dominate the statistics for the value of
. Moreover, one disorder realization gives exactly one data point. It is not obvious how to perform a disorder average without increasing
, which is highly demanding computationally. For these reasons, it is currently difficult to determine with certainty whether the low-pressure
dependence is indeed exponential for
and a power law for
. For similar reasons, it is much more difficult to determine critical exponents near the percolation threshold for elastic contacts than for simple percolation models, such as the random-on/off-bond model defined on lattices. Some aspects pertaining to this issue have been touched upon in the context of the Reynolds flow through the thin gap between a randomly rough substrate and an elastic manifold that is squeezed against it [
34,
38].
The growth of with load well below the percolation threshold, i.e., at loads where the stress distribution function is only weakly dependent on load, may affect the likelihood of plastic events. A larger contact patch effectively corresponds to a larger indenter exerting the same mean stress on the deformable body as a smaller indenter. Since plasticity, in contrast to elasticity, is not scale free, plastic events are very likely to be superlinear in load once the pressure is sufficiently large for to noticeably grow with p.
Another dimensionless variable, which affects the patch-size distribution and thus the characteristic patch size quite substantially, is the dimensionless ratio
(see
Figure 7). This time, a qualitative difference is clearly borne out for the two investigated Hurst exponents. For
, a systematic increase of
with
in form of a
power law is observed in the investigated range of
. In contrast, the
system shows a rather weak
dependence at fixed load, which is roughly logarithmic. The difference between the largest and smallest value of
for the
system is a factor of two, while it is more than a factor of 200 for the
system.
This difference in scaling of can be rationalized as follows: For , the dominant part of the elastic energy needed to conform two surfaces is dominated by the short-wavelength deformations, while the opposite holds for . Consequently, a substrate bears many patches of size on a scale , since it is not energetically expensive to form contact at distant asperities. In contrast, a substrate only has at best a few dominant mesoscale asperities in contact over an area of order . Due to this qualitative difference, we expect that not only the functional form of the laws differ between Hurst exponents above and below , but also the functional form of the low-pressure dependence, even if the currently available data on by itself is too meager to provide strong support for this latter claim.
It still needs to be shown that the radius of a characteristic cluster correlates with the distance at which the stress ACF drops to a value near
. Towards this end, the stress ACF is shown in
Figure 8 at different values of
. It can be seen that the
stress ACF has decayed to a rather small value at a relatively short distance, i.e., to a value well below
on a distance that is of
. Subsequently, there is little dependence of the stress ACF for
. This is different for the
surface, where stress correlations are much longer ranged than for
, and, moreover, distinctly more sensitive to the ratio
.
Figure 9 tests our hypothesis that contact patch radii defined as
should satisfy
. Towards this end, we show
at a fixed load for a variety of ratios of
. It turns out that the inequality is well obeyed and that the value of
only depends weakly, i.e., logarithmically, on
.
For completeness,
Figure 10 shows how the stress ACF changes with dimensionless pressure, when all other (dimensionless) parameters are kept constant. Similar curves (with a slightly different definition of the stress ACF) have been reported before, see, e.g., Figures 7 and 8 in Ref. [
25], where we also tested and thereby validated the suitability of Persson theory with respect to the prediction of stress-ACF’s.
Curves such as those shown in
Figure 10 are then used to deduce the value of
, which then enters the test of our hypothesis that the radius of characteristic patches satisfies
at
. Another such test is shown in
Figure 11, which includes percolated contacts.
As argued in
Section 2.3,
exceeds
for
. Because of the relatively small system system sizes investigated here (the relevant number is
, at least for
), the standard deviation with respect to the quite accurate literature estimate [
34] of
is non-negligible. For the two investigated samples (different values of
H but identical seeds used for the random number generator defining the surface realization), percolation occurred slightly below the value of
, which was obtained using much larger values of
. The transition between percolated and non-percolated contact occurs, as argued in the theory section, near
.
4. Conclusions
In this article, we have analyzed and rationalized the distribution of contact patch sizes in contacts between adhesionless, randomly rough, elastic solids. Our simulations revealed that the scaling relation known for the number density of large contact patches, i.e.,
, breaks down at a characteristic (maximum) patch size
, where the scaling crosses over to a superexponential decay. Our theoretical considerations allow the range of validity for the scaling relation to be crudely estimated. Likewise, it allows the characteristic contact-patch size to be estimated from any theory that allows a reliable calculation of the stress ACF. Thus far, Persson’s approach to contact mechanics has been successful in this regard [
25,
33,
39,
40], while bearing models that do not incorporate pairwise elastic interactions between asperities are doomed to fail by design [
40]. Additional theory is nonetheless required to explain the observed dependence of the exponent
on the Hurst exponent, which we identify as
. Since the total contact area is readily predicted, as are
and
, this theory would have to explain either the exponent
directly or indirectly through the prediction of the prefactor for the number density
.
The dependence of the exponent
on
H is consistent with results deduced from cuts through Gaussian surfaces [
13,
14]. This is quite surprising, because the exponents describing the spatial correlation of contact differ quite substantially between those valid for elastic contacts from those obtained from cuts through the surface [
19,
40]. However, it seems as if changes to the model can alter the precise value of exponents. For example, the problem assigned in the contact-mechanics challenge [
19] included moderate adhesion (large enough to increase
by 50%, but too small to induce substantial stickiness) reduced the value for a
surface from
to
. This result can be rationalized with the argument that adhesion favors the existence of large patches, because a small separation between two clusters become energetically unfavorable, whereby small contact patches are removed and added to large patches.
Our simulations demonstrated that the increasing-load-only-leads-to-more-of-the-same (ILOL2MOTS) dictum—which is approximately valid for the contact mechanics of self-affine, randomly surfaces at small stresses—starts to be a poor approximation well below the percolation pressure. As proposed in the Introduction, this observation provides
a possible explanation for why Amontons’ law or Ryes–Archard’s law break down at large loads, even if the pressure is sufficiently small for the stress distribution (within the contact) to remain essentially unchanged when
is multiplied or divided by a factor of two. We certainly do not mean to claim that it is the only possible explanation and perhaps not even a very common one. After all, in many practical applications, the generation of debris and the subsequent third-body distribution—as discussed, for example, by Blau in the context of running-in [
41]—may be more critical than surface topography and contact mechanics.
When considering debris particles, whose presence leads to more wear and thus to more debris particles and higher friction, it is clear that the ILOL2MOTS dictum may not even hold at low pressures. In addition, elastic coupling between asperities can affect the friction-load [
9] or the wear-load [
11] dependence under certain circumstances However, when these mechanisms are irrelevant, our findings suggest that the dictum may still break down at a lower pressure than would be expected based on the study of the stress distribution function alone. The latter can look essentially indistinguishable for a system with, say, 0.05% and 10% relative contact area. However, since the way in which stress is spatially distributed differs between the two characteristic patch sizes are distinctly smaller at 0.05% than at 10% contact area, it is clear that any size dependence of plasticity can be revealed before (predicted, elastic) contact stresses start to raise. This issue is discussed in more detail in the next paragraph with an emphasis on Amontons’ law.
In systems where plasticity (and thus wear) are negligibly small at small loads, dissipation must be expected to occur predominantly within the boundary lubricant. Friction then results as a surface integral over the shear stress, which is a function of normal stress—and potentially velocity. Since the normal, interfacial stress distribution in a randomly rough contact barely changes with load (when normalized to the real contact area), not only the contact area but also friction can turn out linear in load even when the local dependence of shear on normal stress is non-linear. This argument is no longer correct, when the characteristic patch size exceeds a critical value, above which plasticity is no longer negligible or independent of patch size. Additional dissipation mechanisms come into play so that friction increases superlinearly with load. Thoughts along these lines might also matter for adhesive surfaces, for which the existence of a critical contact-patch size has been proposed, below which plastic deformation within the bulk prevails and above which surfaces yield through adhesive wear [
10,
15].
Let us conclude this work with an answer to the question how much
grows—at small stresses for a
surface—when the roughness, i.e., the root-mean-square (rms) height
is doubled. The doubling can be achieved, for example, by leaving
,
as well as the load
L unchanged, while the height spectrum
is replaced with
. Dimensional analysis reveals that—within linear elasticity and the small-slope approximation—the effect on contact mechanics is the same as if the load were doubled or the apparent contact were halved and all geometric surface parameters kept unchanged [
26]. Under such a transformation,
would roughly increase by a factor
, which amounts to an increase of approximately 50% for
of a generic
surface.
The doubling of the rms-height can also be achieved by leaving the pressure and the prefactor of constant, while increasing the ratio . For sufficiently small , this means that also the reduced pressure remains unchanged. It is readily found that scales with , so that doubling the roughness necessitates an increase of for our surface. This in turn means an increase of by approximately .
An increase of by a factor of 3.7 (induced by the doubling of the rms-height through a pertinent change of ) might not sound much more dramatic than an increase by 1.5 (induced by scaling all heights at a given in-plane position with a factor of 2). However, if is changed by a factor of say 100 (the difference between two surfaces being polished to either 1 m or 0.1 mm), these numbers change to 12.5 and 5600, respectively. Thus, polishing not only reduces the local stress by a reduction of root-mean-square height gradients but also by a lowering of the roll-off wavevector leading to smaller, more resilient contact patches.