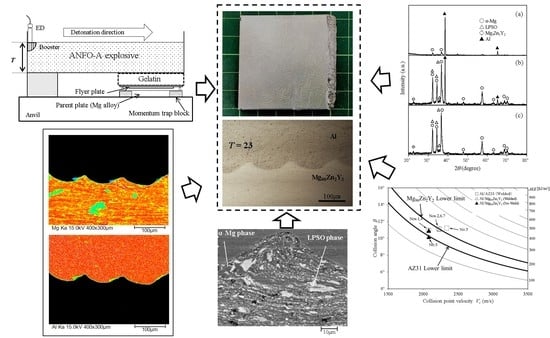
Explosive Welding of Thin Aluminum Plate onto Magnesium Alloy Plate Using a Gelatin Layer as a Pressure-Transmitting Medium
Abstract
1. Introduction
2. Materials and Methods
3. Results and Discussion
3.1. Microstructure of the Weld Interface
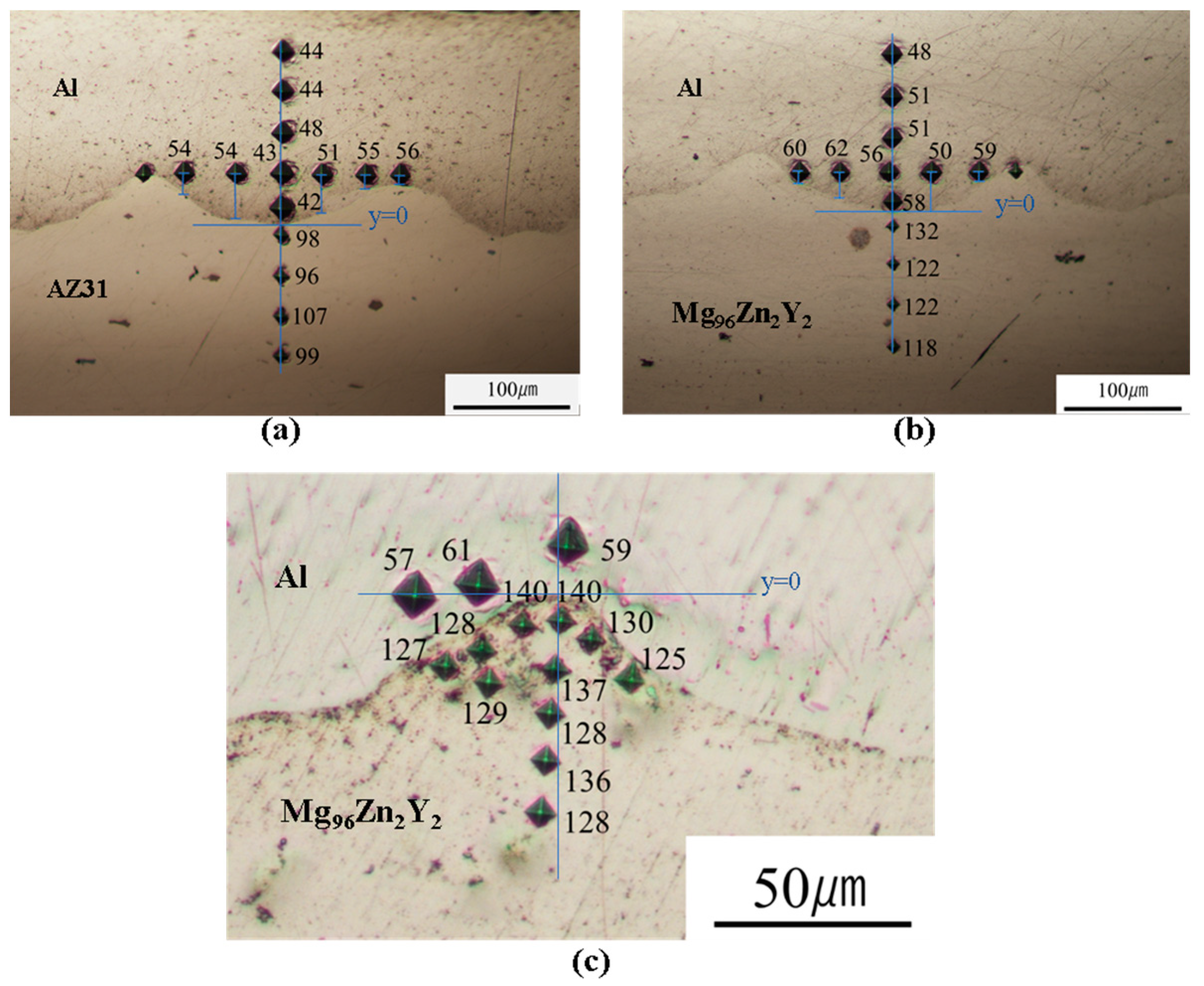
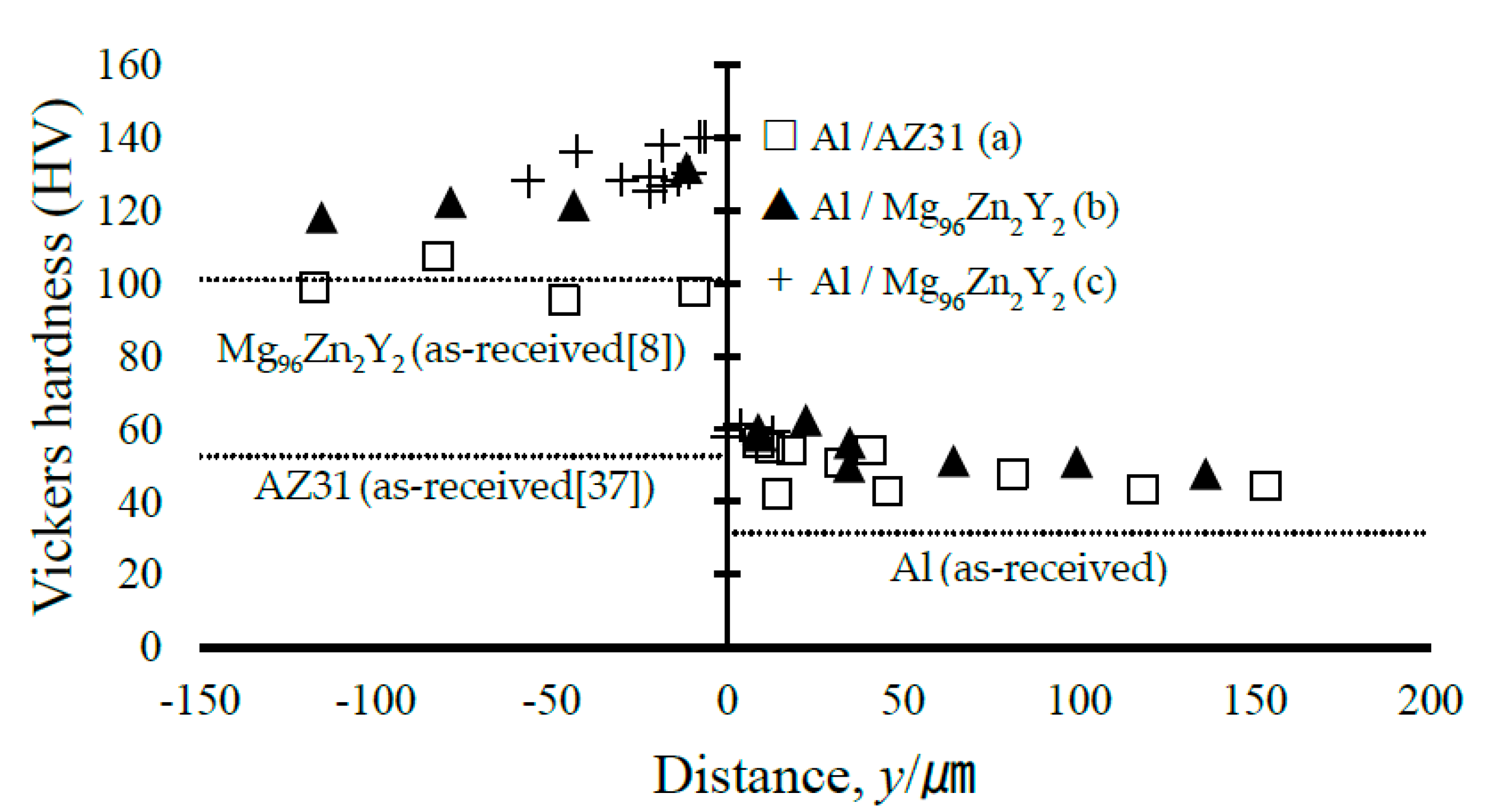
3.2. Quality of Bonding Across Interface
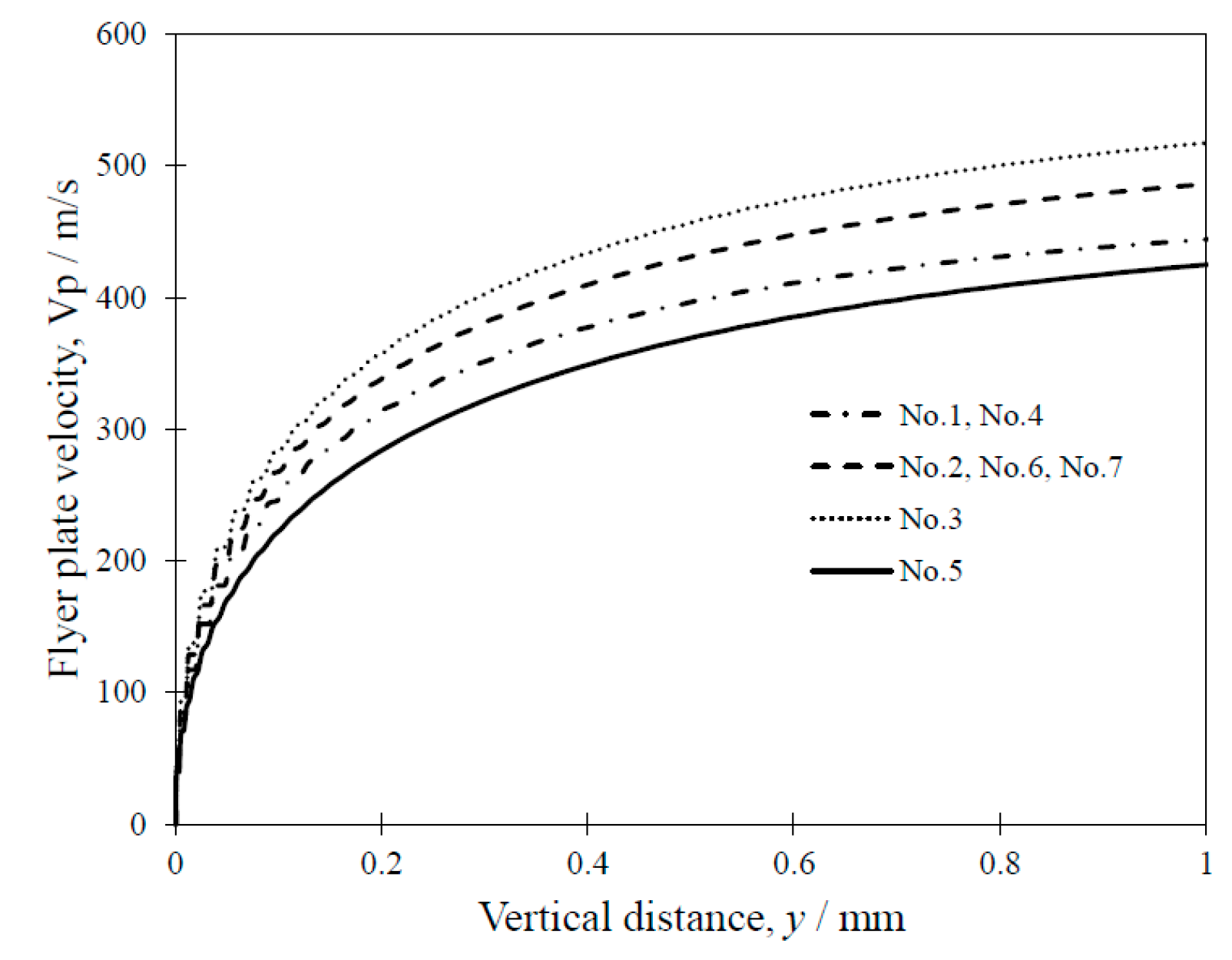
3.3. Welding Conditions
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Mordike, B.L.; Ebert, T. Magnesium: Properties—Applications—Potential. Mater. Sci. Eng. A 2001, 302, 37–45. [Google Scholar] [CrossRef]
- Yamasaki, M.; Hashimoto, K.; Hagihara, K.; Kawamura, Y. Effect of multimodal microstructure evolution on mechanical properties of Mg–Zn–Y extruded alloy. Acta Mater. 2011, 59, 3646–3658. [Google Scholar] [CrossRef]
- Noda, M.; Mayama, T.; Kawamura, Y. Evolution of mechanical properties and microstructure in extruded Mg 96Zn2Y2 alloys by annealing. Mater. Trans. 2009, 50, 2526–2531. [Google Scholar] [CrossRef]
- Hagihara, K.; Kinoshita, A.; Sugino, Y.; Yamasaki, M.; Kawamura, Y.; Yasuda, H.Y.; Umakoshi, Y. Effect of long-period stacking ordered phase on mechanical properties of Mg97Zn1Y2 extruded alloy. Acta Mater. 2010, 58, 6282–6293. [Google Scholar] [CrossRef]
- Abe, E.; Ono, A.; Itoi, T.; Yamasaki, M.; Kawamura, Y. Polytypes of long-period stacking structures synchronized with chemical order in a dilute Mg–Zn–Y alloy. Philos. Mag. Lett. 2011, 91, 690–696. [Google Scholar] [CrossRef]
- Hagihara, K.; Yokotani, N.; Umakoshi, Y. Plastic deformation behavior of Mg12YZn with 18R long-period stacking ordered structure. Intermetallics 2010, 18, 267–276. [Google Scholar] [CrossRef]
- Kawamura, Y.; Yamasaki, M. Formation and Mechanical Properties of Mg97Zn1RE2 Alloys with Long-Period Stacking Ordered Structure. Mater. Trans. 2007, 48, 2986–2992. [Google Scholar] [CrossRef]
- Noda, M.; Kawamura, Y.; Mayama, T.; Funami, K. Thermal Stability and Mechanical Properties of Extruded Mg-Zn-Y Alloys with a Long-Period Stacking Order Phase and Plastic Deformation. In New Features on Magnesium Alloys; InTechOpen: London, UK, 2012. [Google Scholar]
- Liu, F.; Liang, W.; Li, X.; Zhao, X.; Zhang, Y.; Wang, H. Improvement of corrosion resistance of pure magnesium via vacuum pack treatment. J. Alloys Compd. 2008, 461, 399–403. [Google Scholar] [CrossRef]
- Borrisutthekul, R.; Miyashita, Y.; Mutoh, Y. Dissimilar material laser welding between magnesium alloy AZ31B and aluminum alloy A5052-O. Sci. Technol. Adv. Mater. 2005, 6, 199–204. [Google Scholar] [CrossRef]
- Mahendran, G.; Balasubramanian, V.; Senthilvelan, T. Developing diffusion bonding windows for joining AZ31B magnesium–AA2024 aluminium alloys. Mater. Des. 2009, 30, 1240–1244. [Google Scholar] [CrossRef]
- Çam, G.; Mistikoglu, S. Recent Developments in Friction Stir Welding of Al-alloys. J. Mater. Eng. Perform. 2014, 23, 1936–1953. [Google Scholar] [CrossRef]
- Kong, C.Y.; Soar, R.C.; Dickens, P.M. Optimum process parameters for ultrasonic consolidation of 3003 aluminium. J. Mater. Process. Technol. 2004, 146, 181–187. [Google Scholar] [CrossRef]
- Mariani, E.; Ghassemieh, E. Microstructure evolution of 6061 O Al alloy during ultrasonic consolidation: An insight from electron backscatter diffraction. Acta Mater. 2010, 58, 2492–2503. [Google Scholar] [CrossRef]
- Higashi, Y.; Iwamoto, C.; Kawamura, Y. Microstructure evolution and mechanical properties of extruded Mg96Zn2Y2 alloy joints with ultrasonic spot welding. Mater. Sci. Eng. A 2016, 651, 925–934. [Google Scholar] [CrossRef]
- Luo, C.; Liang, W.; Chen, Z.; Zhang, J.; Chi, C.; Yang, F. Effect of high temperature annealing and subsequent hot rolling on microstructural evolution at the bond-interface of Al/Mg/Al alloy laminated composites. Mater. Charact. 2013, 84, 34–40. [Google Scholar] [CrossRef]
- Carpenter, S.H.; Wittman, R.H. Explosion Welding. Annu. Rev. Mater. Sci. 1975, 5, 177–199. [Google Scholar] [CrossRef]
- Blazynski, T.Z. Explosive Welding, Forming, and Compaction; Applied Science: London, UK, 1983; ISBN 9401197512. [Google Scholar]
- Habib, M.A.; Keno, H.; Uchida, R.; Mori, A.; Hokamoto, K. Cladding of titanium and magnesium alloy plates using energy-controlled underwater three layer explosive welding. J. Mater. Process. Tech. 2015, 217, 310–316. [Google Scholar] [CrossRef]
- Mori, A.; Tamaru, K.; Hokamoto, K.; Fujita, M. Underwater Explosive Welding, Discussion Based on Weldable Window. AIP Conf. Proc. 2006, 845, 1543–1546. [Google Scholar]
- Mori, A.; Hokamoto, K.; Fujita, M. Characteristics of the New Explosive Welding Technique Using Underwater Shock Wave-Based on Numerical Analysis. Mater. Sci. Forum 2004, 465, 307–312. [Google Scholar] [CrossRef]
- Hokamoto, K.; Fujita, M.; Shimokawa, H.; Okugawa, H. A new method for explosive welding of Al/ZrO2 joint using regulated underwater shock wave. J. Mater. Process. Technol. 1999, 85, 175–179. [Google Scholar] [CrossRef]
- Hokamoto, K.; Nakata, K.; Mori, A.; Tsuda, S.; Tsumura, T.; Inoue, A. Dissimilar material welding of rapidly solidified foil and stainless steel plate using underwater explosive welding technique. J. Alloys Compd. 2009, 472, 507–511. [Google Scholar] [CrossRef]
- Mori, A.; Nishi, M.; Hokamoto, K. Underwater shock wave weldability window for Sn-Cu plates. J. Mater. Process. Technol. 2019, 267, 152–158. [Google Scholar]
- Nagayama, K.; Mori, Y.; Motegi, Y.; Nakahara, M. Shock hugoniot for biological materials. Shock Waves 2006, 15, 267–275. [Google Scholar] [CrossRef]
- Shepherd, C.J.; Appleby-Thomas, G.J.; Hazell, P.J.; Allsop, D.F. The dynamic behaviour of ballistic gelatin. In Proceedings of the AIP Conference Proceedings, Nashville, TN, USA, 28 June–3 July 2009; Volume 1195, pp. 1399–1402. [Google Scholar]
- Inao, D.; Tanaka, S.; Yamashita, T.; Hokamoto, K. Visualization of shockwave behavior in water and gelatin. Measurement 2019, 148, 106929. [Google Scholar] [CrossRef]
- Parchuri, P.; Kotegawa, S.; Yamamoto, H.; Ito, K.; Mori, A.; Hokamoto, K. Benefits of intermediate-layer formation at the interface of Nb/Cu and Ta/Cu explosive clads. Mater. Des. 2019, 166, 107610. [Google Scholar] [CrossRef]
- Crossland, B. Explosive Welding of Metals and Its Application; Clarendon Press: Oxford, UK; New York, NY, USA, 1982; ISBN 9780198591191. [Google Scholar]
- Hokamoto, K.; Izuma, T.; Fujita, M. New explosive welding technique to weld aluminum alloy and stainless steel plates using a stainless steel intermediate plate. Metall. Mater. Trans. A 1993, 24, 2289–2297. [Google Scholar] [CrossRef]
- Akbari Mousavi, A.A.; Al-Hassani, S.T.S. Numerical and experimental studies of the mechanism of the wavy interface formations in explosive/impact welding. J. Mech. Phys. Solids 2005, 53, 2501–2528. [Google Scholar] [CrossRef]
- Deribas, A. Physics of explosive hardening and welding. Nauk. Novosib. 1980, 220. [Google Scholar]
- Yoshimoto, S.; Yamasaki, M.; Kawamura, Y. Microstructure and Mechanical Properties of Extruded Mg-Zn-Y Alloys with 14H Long Period Ordered Structure. Mater. Trans. 2006, 47, 959–965. [Google Scholar] [CrossRef]
- Ghaderi, S.H.; Mori, A.; Hokamoto, K. Analysis of Explosively Welded Aluminum-AZ31 Magnesium Alloy Joints. Mater. Trans. 2008, 49, 1142–1147. [Google Scholar] [CrossRef]
- Bataev, I.A.; Lazurenko, D.V.; Tanaka, S.; Hokamoto, K.; Bataev, A.A.; Guo, Y.; Jorge, A.M. High cooling rates and metastable phases at the interfaces of explosively welded materials. Acta Mater. 2017, 135, 277–289. [Google Scholar] [CrossRef]
- Hoseini Athar, M.M.; Tolaminejad, B. Weldability window and the effect of interface morphology on the properties of Al/Cu/Al laminated composites fabricated by explosive welding. Mater. Des. 2015, 86, 516–525. [Google Scholar] [CrossRef]
- Ghaderi, S.H.; Mori, A.; Hokamoto, K. Explosion Joining of Magnesium Alloy AZ31 and Aluminum. Mater. Sci. Forum 2008, 566, 291–296. [Google Scholar] [CrossRef]
- Kennedy, J. Explosive Output for Driving Metal. Behav. Util. Explos. Eng. Des. 1972, 109–124. [Google Scholar]
- Cowan, G.R.; Bergmann, O.R.; Holtzman, A.H. Mechanism of Bond Zone Wave Formation in Explosion-Clad Metals. Metall. Mater. Trans. B 1971, 2, 3145–3155. [Google Scholar] [CrossRef]
- Gurney, R.W. The Initial Velocities of Fragments from Bombs, Shell, and Grenades. Army Ballist. Res. Lab Aberd. Proving Ground Md. 1943, 450. [Google Scholar]
- Chadwick, M.D.; Howd, D.; Wildsmith, G.; Cairns, J.H. Explosive welding of tubes and tube plates. Br. Weld. J. 1968, 15, 480–492. [Google Scholar]
- Liu, R.Q.; Nie, J.X.; Jiao, Q.J. Study on Lee-Tarver Model Parameters of CL-20 Explosive Ink. In Proceedings of the Smart Innovation, Systems and Technologies, Jilin, China, 18–20 July 2019; Springer: Singapore, 2019; Volume 157, pp. 205–215. [Google Scholar]
- Hino, K. Fragmentation of rock through blasting. J. Ind. Explos. Soc. Jpn. 1956, 17, 2–11. [Google Scholar]
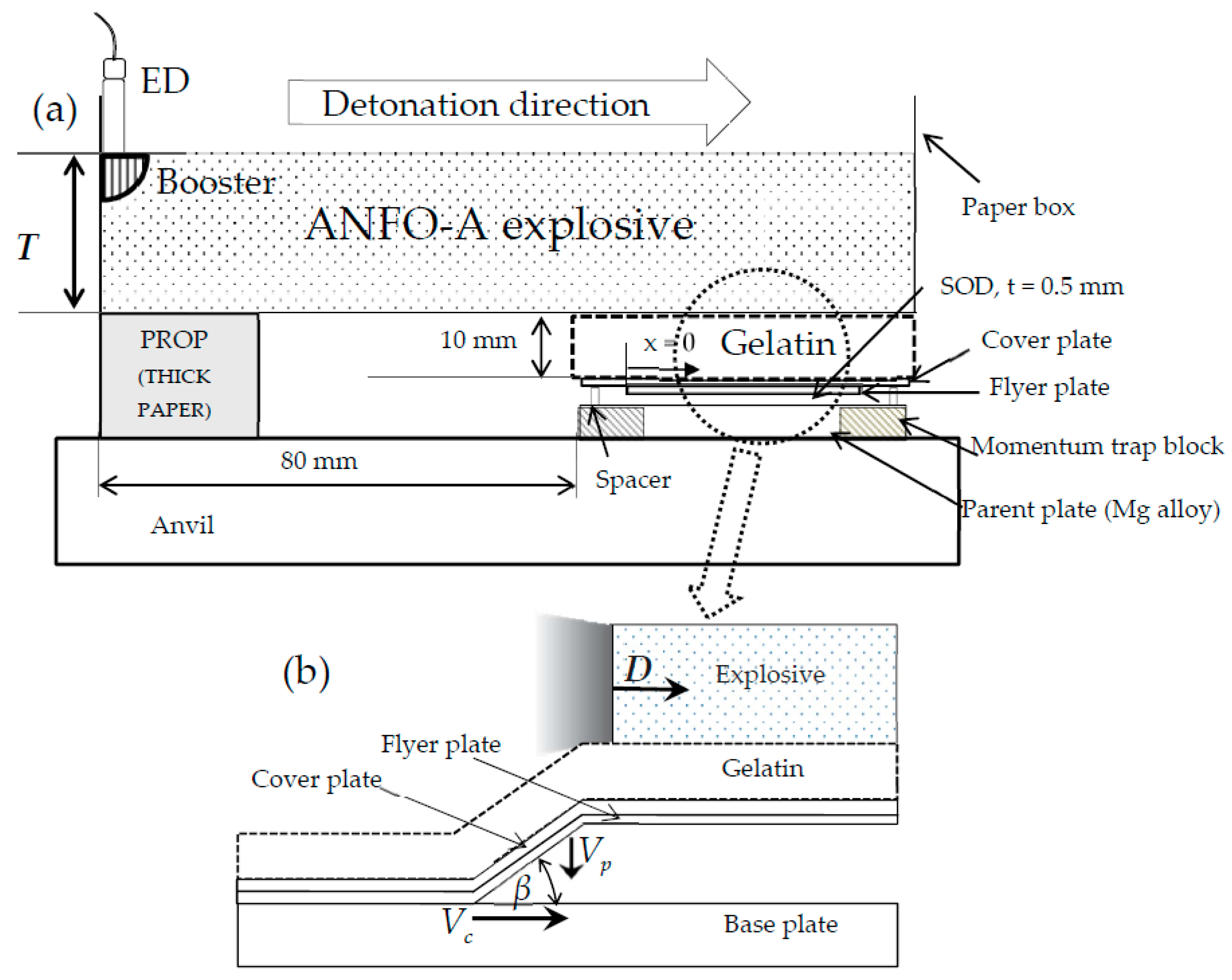









| No. | Parent Plate (l × w × t, mm3) | Flyer Plate (t, mm) | Cover Plate (t, mm) | Explosive Thickness (T, mm) | Weld | |
|---|---|---|---|---|---|---|
| 1 | AZ31 | 50 × 50 × 5 | Al (0.2) | JIS-SUS304 (0.2) | 18 | Yes |
| 2 | AZ31 | 50 × 50 × 5 | Al (0.2) | JIS-SUS304 (0.2) | 23 | Yes |
| 3 | AZ31 | 50 × 50 × 5 | Al (0.2) | JIS-SUS304 (0.2) | 29 | Yes |
| 4 | Mg96Zn2Y2 | 50 × 50 × 5 | Al (0.2) | JIS-SUS304 (0.2) | 18 | No |
| 5 | Mg96Zn2Y2 | 50 × 50 × 5 | Al (0.2) | JIS-SUS304 (0.3) | 18 | No |
| 6 | Mg96Zn2Y2 | 50 × 50 × 5 | Al (0.2) | JIS-SUS304 (0.2) | 23 | Yes |
| 7 | Mg96Zn2Y2 | 95 × 50 × 5 | Al (0.2) | JIS-SUS304 (0.2) | 23 | Yes |
| Material | Mg | Zn | Y | Al |
|---|---|---|---|---|
| AZ31 | bal. | 0.7–0.13 | - | 2.5–3.5 |
| Mg96Zn2Y2 | bal. | 4.9 | 6.36 | 0.25 |
| No. | Thickness of Explosive (T) | Combination | Wavelength (Average) | Amplitude (Average) |
|---|---|---|---|---|
| No. 1 | T = 18 mm | Al/AZ31 | 102 μm | 20 μm |
| No. 2 | T = 23 mm | Al/AZ31 | 167 μm | 27 μm |
| No. 3 | T = 29 mm | Al/AZ31 | 216 μm | 51 μm |
| No. 6 | T = 23 mm | Al/Mg96Zn2Y2 | 161 μm | 35 μm |
| Pressure Medium | Reference Density | Gruneisen Coefficient (Γ) | Sound Velocity | Material Constant (s) |
|---|---|---|---|---|
| 20 wt.% gelatin | 1060 | 0.00 | 1570 | 1.77 |
| Thickness of Explosive (T) | Kinetic Energy Lost (ΔKE) kJ/m2 |
|---|---|
| T = 18 mm (Cover plate thickness 0.2 mm) | 168 |
| T = 18 mm (Cover plate thickness 0.3 mm) | 200 |
| T = 23 mm | 199 |
| T = 29 mm | 224 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Inao, D.; Mori, A.; Tanaka, S.; Hokamoto, K. Explosive Welding of Thin Aluminum Plate onto Magnesium Alloy Plate Using a Gelatin Layer as a Pressure-Transmitting Medium. Metals 2020, 10, 106. https://doi.org/10.3390/met10010106
Inao D, Mori A, Tanaka S, Hokamoto K. Explosive Welding of Thin Aluminum Plate onto Magnesium Alloy Plate Using a Gelatin Layer as a Pressure-Transmitting Medium. Metals. 2020; 10(1):106. https://doi.org/10.3390/met10010106
Chicago/Turabian StyleInao, Daisuke, Akihisa Mori, Shigeru Tanaka, and Kazuyuki Hokamoto. 2020. "Explosive Welding of Thin Aluminum Plate onto Magnesium Alloy Plate Using a Gelatin Layer as a Pressure-Transmitting Medium" Metals 10, no. 1: 106. https://doi.org/10.3390/met10010106
APA StyleInao, D., Mori, A., Tanaka, S., & Hokamoto, K. (2020). Explosive Welding of Thin Aluminum Plate onto Magnesium Alloy Plate Using a Gelatin Layer as a Pressure-Transmitting Medium. Metals, 10(1), 106. https://doi.org/10.3390/met10010106