Determination of Wide Burnishing Energy-Force Parameters based on Constructing the Kinematically Admissible Velocity Field
Abstract
:1. Introduction
2. Theoretical Background
- (a)
- Among all kinematically admissible velocity fields in the deformation zone, there is only one which causes the total power calculated as a product of stress and strain reaches minimum. This total power is the sum of the power of plastic deformation, power at tool-workpiece interface, and the power dissipated at the velocity discontinuity surfaces.
- (b)
- Strain rate field calculated from kinematically admissible velocity field is also kinematically admissible.
- (c)
- Real power of deformation supplied to the system by the external forces is always lower than power calculated as sum of powers by first item. If the velocity field is determined with an error, the power calculated from this field will be larger than the real power of the process. The minimum of the power is obtained for the real (correct) velocity field.
- (a)
- Work piece of rigid-plastic material without strain hardening;
- (b)
- Task is solved in plain conditions (plain-deformed state);
- (c)
- Plastic deformation site is a second-order curve bounded by the indenter surface
- (d)
- Microroughness is neglected; the indenter penetrates flat surface at depth t(Figure 3).
- —plastic deformation, sliding along the flow rate discontinuity surfaces, friction forces, and external forces power respectively;
- = 2 —shear deformation rate intensity in the deformation site of volume W;
- —discontinuity surface of velocity peripheral component ;
- —friction surface (in the roller and medium contact area) with friction coefficient ;—surface on which external forces are active.
- —plastic deformation zone volume.
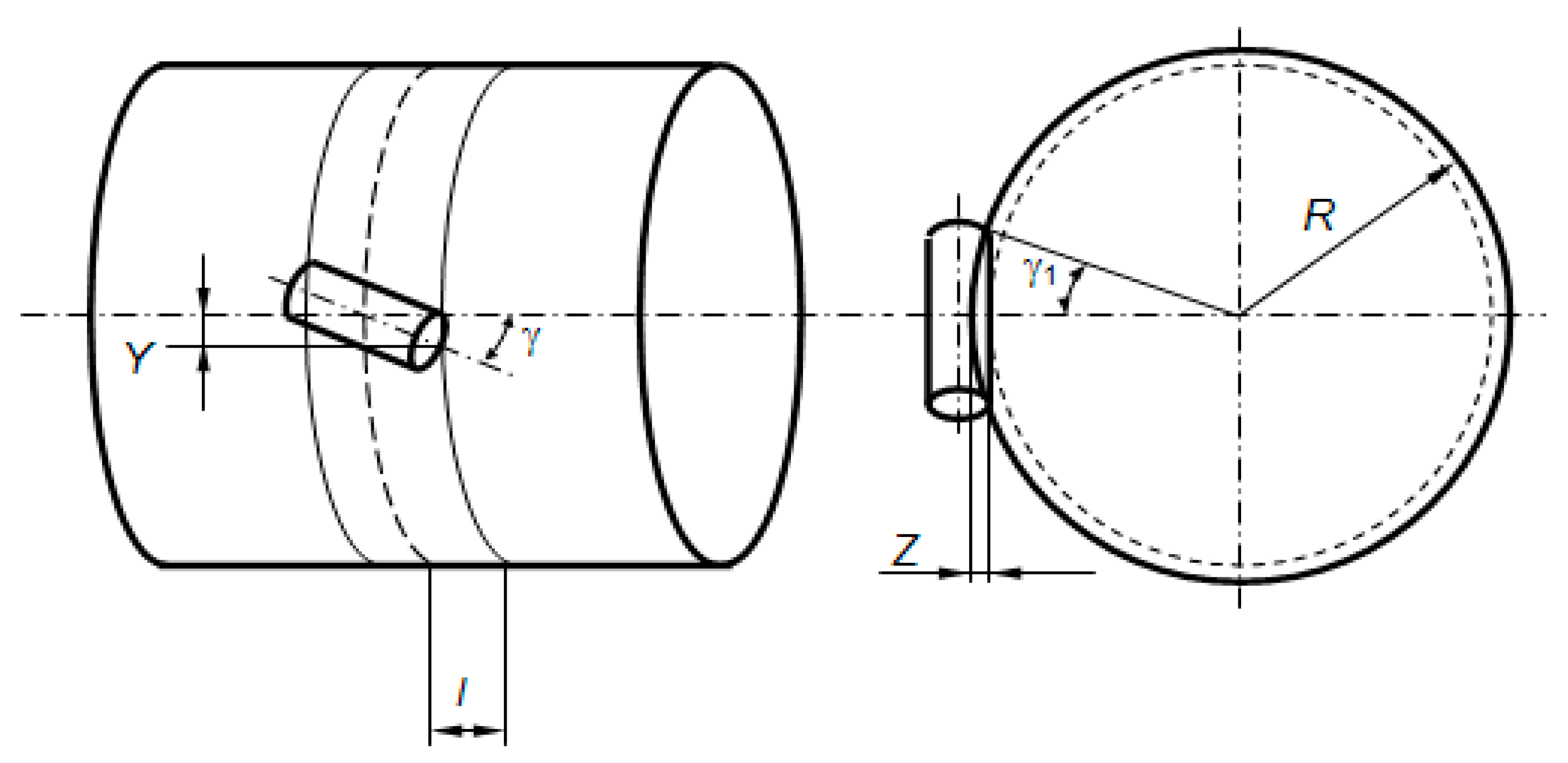
3. Experimental Procedure
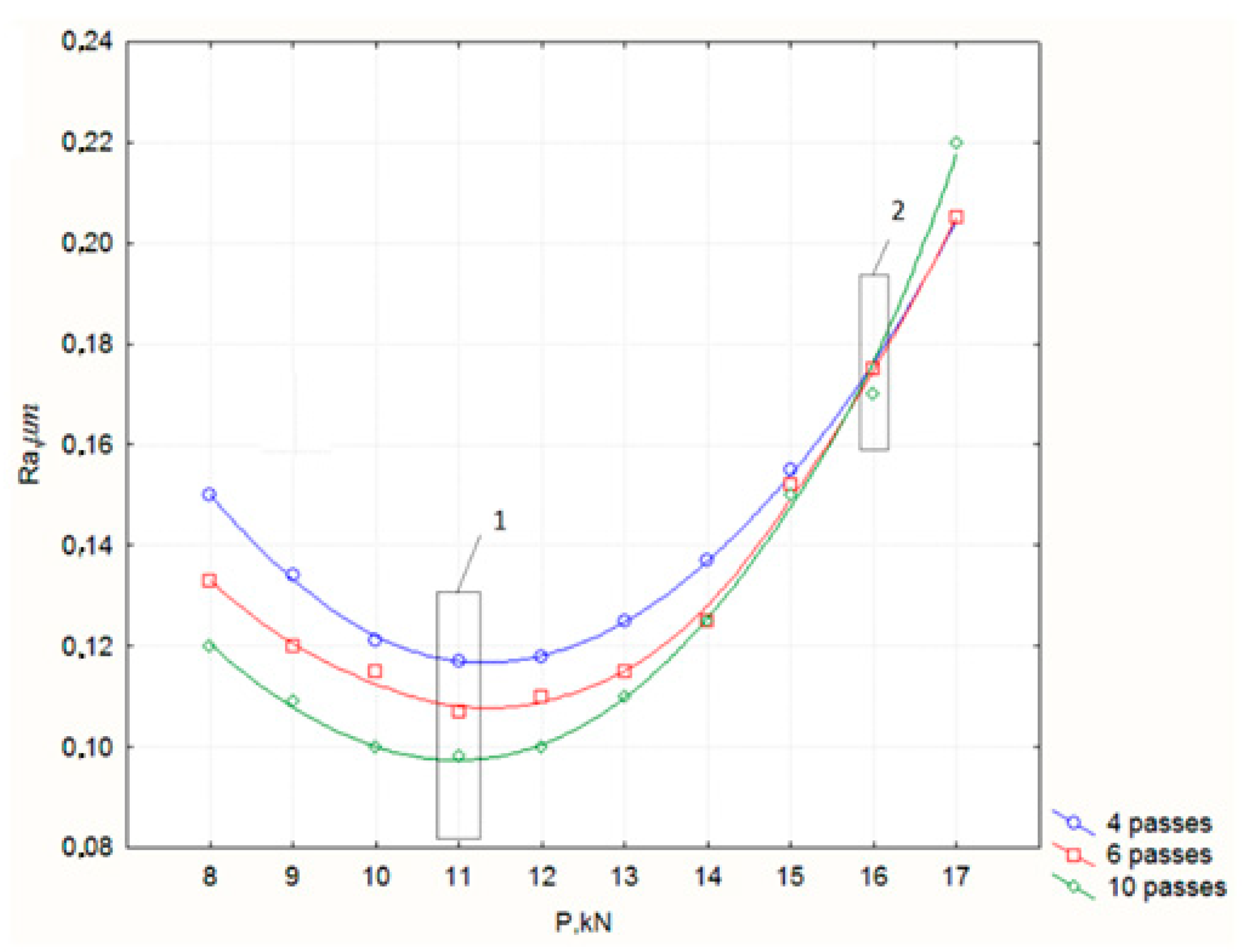
4. Discussion
- (1)
- The pressure on indenter, found by the analytical method, is up to 9% greater than the pressure determined by the finite elements method, which is explained by implementing the upper bound approach in the analytical method;
- (2)
- The pressure on indenter, found experimentally using Equation (37), is on average 20% less than the pressure calculated by the finite elements method.
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Yen, Y.C.; Sartkulvanich, P.; Altan, T. Finite Element Modeling of Roller Burnishing Process. CIRP Ann. 2005, 54, 237–240. [Google Scholar] [CrossRef]
- Prevey, P.S.; Ravindranath, R.A.; Shepard, M.; Gabb, T. Case Studies of Fatigue Life Improvement Using Low Plasticity Burnishing in Gas Turbine Engine Applications. Trans. ASME 2006, 128, 866–872. [Google Scholar] [CrossRef] [Green Version]
- Hassan, A.M.; Maqableh, A.M. The Effects of Initial Burnishing Parameters on Non Ferrous Components. J. Mater. Process. Technol. 2000, 102, 115–121. [Google Scholar] [CrossRef]
- El-Axir, M.H.; EI-CKhabeery, M.M. Influence of Orthogonal Burnishing Parameters on Surface Characteristics for Various 4. Materials. J. Mater. Process. Technol. 2003, 132, 82–89. [Google Scholar] [CrossRef]
- Nemat, M.; Lyons, A.C. An Investigation of the Surface Topography of Ball Burnished Mild Steel and Aluminum. Int. J. Adv. Manuf. Technol. 2000, 16, 469–473. [Google Scholar]
- Shiou, F.J.; Chen, C.H. Determination of Optimal Ball Burnishing Parameters for Plastic Injection Moulding Steel. Int. J. Adv. Manuf. Technol. 2003, 3, 177–185. [Google Scholar]
- Roettger, K. Walzen Hartgedrehter Oberflaechen. Ph.D. Thesis, RWTH Aachen, North Rhine-Westphalia, Gremany, 2002. [Google Scholar]
- Howell, M.; Hahn, G.T.; Rubin, C.A.; McDowell, D.L. Finite Element Analysis of Rolling Contact for Nonlinear Kinematic Hardening Bearing Steel. J. Tribol. 1995, 117, 729–736. [Google Scholar] [CrossRef]
- Shiratori, M.; Ito, M.; Hashimoto, M. Elastic-Plastic Analysis of Rolling Contact for Surface Hardened Steel. Trans. Jpn. Soc. Mech. Eng. A. 1995, 61, 1064–1069. [Google Scholar] [CrossRef] [Green Version]
- Marshall, E.A. Rolling Contact with Plastic Deformation. J. Mech. Phys. Solids 1968, 16, 243–254. [Google Scholar] [CrossRef]
- Collins, I.F. A Simplified Analysis of the Rolling of Cylinder on a Rigid/Perfectly Plastic Half-Space. Int. J. Mech. Sci. 1972, 14, 1–14. [Google Scholar] [CrossRef]
- Nepershin, R.I. The Rolling and Slipping of a Cylinder along the Boundary of an Ideally Plastic Half-Space. J. Appl. Math. Mech. 2003, 67, 293–301. [Google Scholar] [CrossRef]
- Nepershin, R.I. Plastic Deformation of Surface Layer during Rigid Cylinder Rolling and Sliding. J. Frict. Wear 2013, 34, 204–207. [Google Scholar] [CrossRef]
- Nepershin, R.I. Surface Plastic Deformation by Sliding Elliptical Cylinder. J. Mater. Sci. Chem. Eng. 2015, 3, 1–7. [Google Scholar]
- Pietrzyk, M.; Madej, L.; Rauch, L.; Szeliga, D. Computational Materials Engineering. In Achieving High Accuracy and Efficiency in Metals Processing Simulations; Elsevier: Amsterdam, The Netherlands, 2015; 381p. [Google Scholar]
- Prager, W. The Theory of Plasticity: A Survey of Recent Achievements. Proc. Inst. Mech. Eng. 1955, 169, 41–57. [Google Scholar] [CrossRef]
- Kudo, H. Upper-bound Approach to Metal Forming Processes-to Date and in the Future. In Metal Forming and Impact Mechanics; Reid, S.R., Ed.; Pergamon Press: Oxford, UK, 1985; pp. 19–45. [Google Scholar]
- Johnson, W.; Mellor, P.B. Engineering Plasticity; Ellis Horwood Ltd.: Chichester, UK, 1983; 646p. [Google Scholar]
- Avitzur, B. Metal Forming Processes and Analysis; McGraw-Hill Book Co.: New York, NY, USA, 1968; pp. 102–111. [Google Scholar]
- Grechnikov, F.; Khaimovich, A. The study of plastic deformation at high deformation rates in upset forging of cylinders. Key Eng. Mater. 2016, 684, 74–79. [Google Scholar] [CrossRef]
- Abulkhanov, S.R.; Skuratov, D.L.; Khaimovich, A.I.; Shvetcov, A.N. Analytical approach to determining of parameters which characterize surface layer quality of the parts hardened by a traveling diamond sphere. Int. J. Eng. Technol. 2014, 6, 2191–2200. [Google Scholar]
- Druker, D.C.; Providence, R.I. Coulomb friction. J. Appl. Mech. 1954, 21, 71–74. [Google Scholar]








| Depth t, mm | Indenter Radius R, mm | R/t | Average Pressure by Equation (33) Analytical Calculation (Yield Stress = 1 MPa) | Average Pressure by Equation (35) Finite Elements Calculation (Yield Stress = 1 MPa) | Relative Error |
|---|---|---|---|---|---|
| 0.004 | 2 | 500 | 1.981 | 1.85 | 7.1% |
| 0.008 | 2 | 500 | 2.002 | 1.99 | 0.6% |
| 0.004 | 4 | 1250 | 1.973 | 1.81 | 9.0% |
| 0.008 | 4 | 1250 | 1.981 | 1.89 | 4.8% |
| 0.02 | 4 | 200 | 2.016 | 2.1 | −4.0% |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bobrovskij, I.; Khaimovich, A.; Bobrovskij, N.; D’yakonov, A. Determination of Wide Burnishing Energy-Force Parameters based on Constructing the Kinematically Admissible Velocity Field. Metals 2020, 10, 46. https://doi.org/10.3390/met10010046
Bobrovskij I, Khaimovich A, Bobrovskij N, D’yakonov A. Determination of Wide Burnishing Energy-Force Parameters based on Constructing the Kinematically Admissible Velocity Field. Metals. 2020; 10(1):46. https://doi.org/10.3390/met10010046
Chicago/Turabian StyleBobrovskij, Igor, Alexander Khaimovich, Nikolaj Bobrovskij, and Aleksander D’yakonov. 2020. "Determination of Wide Burnishing Energy-Force Parameters based on Constructing the Kinematically Admissible Velocity Field" Metals 10, no. 1: 46. https://doi.org/10.3390/met10010046
APA StyleBobrovskij, I., Khaimovich, A., Bobrovskij, N., & D’yakonov, A. (2020). Determination of Wide Burnishing Energy-Force Parameters based on Constructing the Kinematically Admissible Velocity Field. Metals, 10(1), 46. https://doi.org/10.3390/met10010046





