Recent Development of Superplasticity in Aluminum Alloys: A Review
Abstract
1. Introduction
2. Influencing Factors on Al Alloys Superplasticity
2.1. Effect of Initial Grain Size on the Superplasticity of Al Alloys
2.2. Effect of Temperature on the Superplasticity of Al Alloys
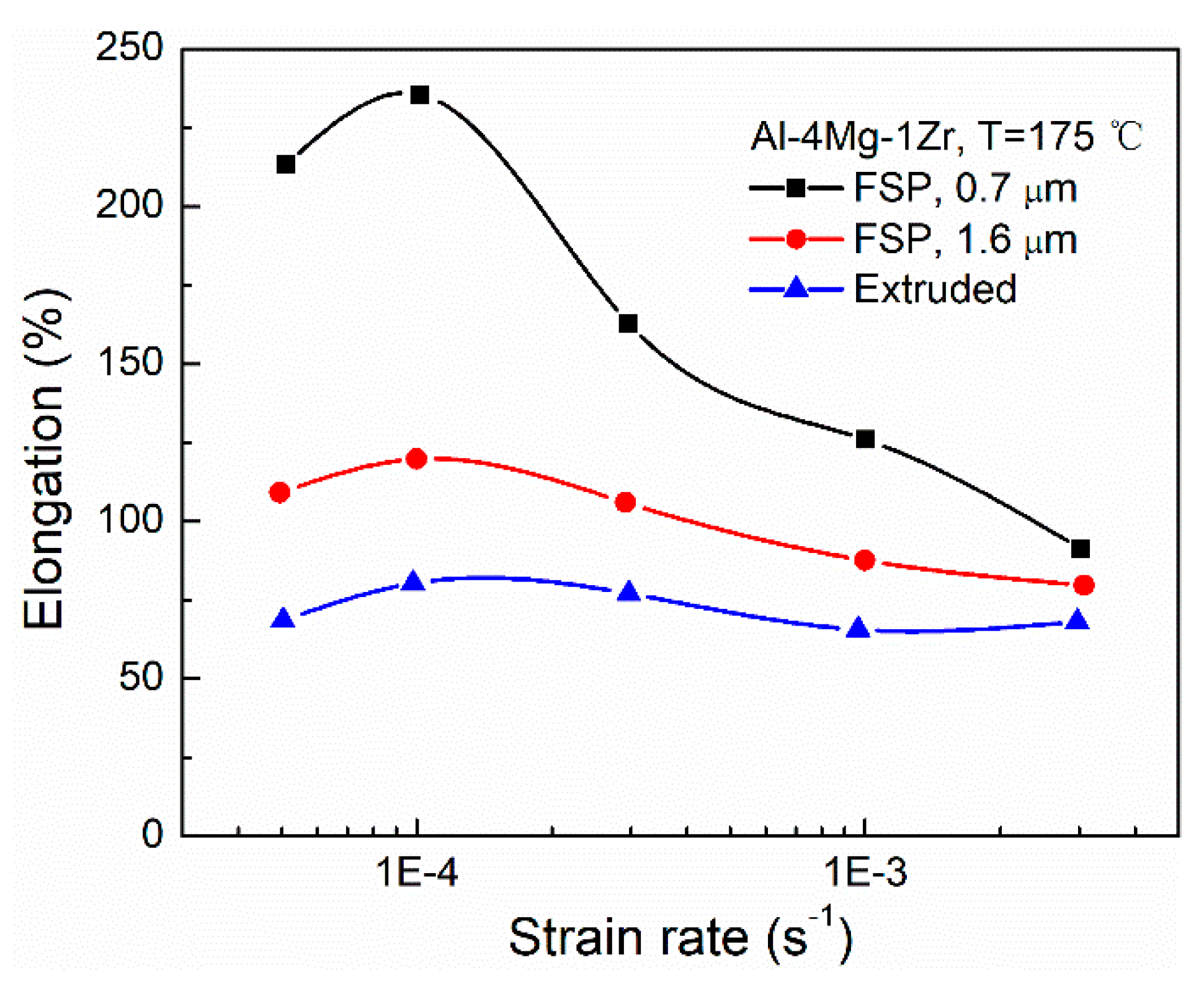
2.3. Effect of Strain Rate on the Superplasticity of Al Alloys
2.4. Effect of Strain Rate Sensitivity on the Superplasticity of Al Alloys
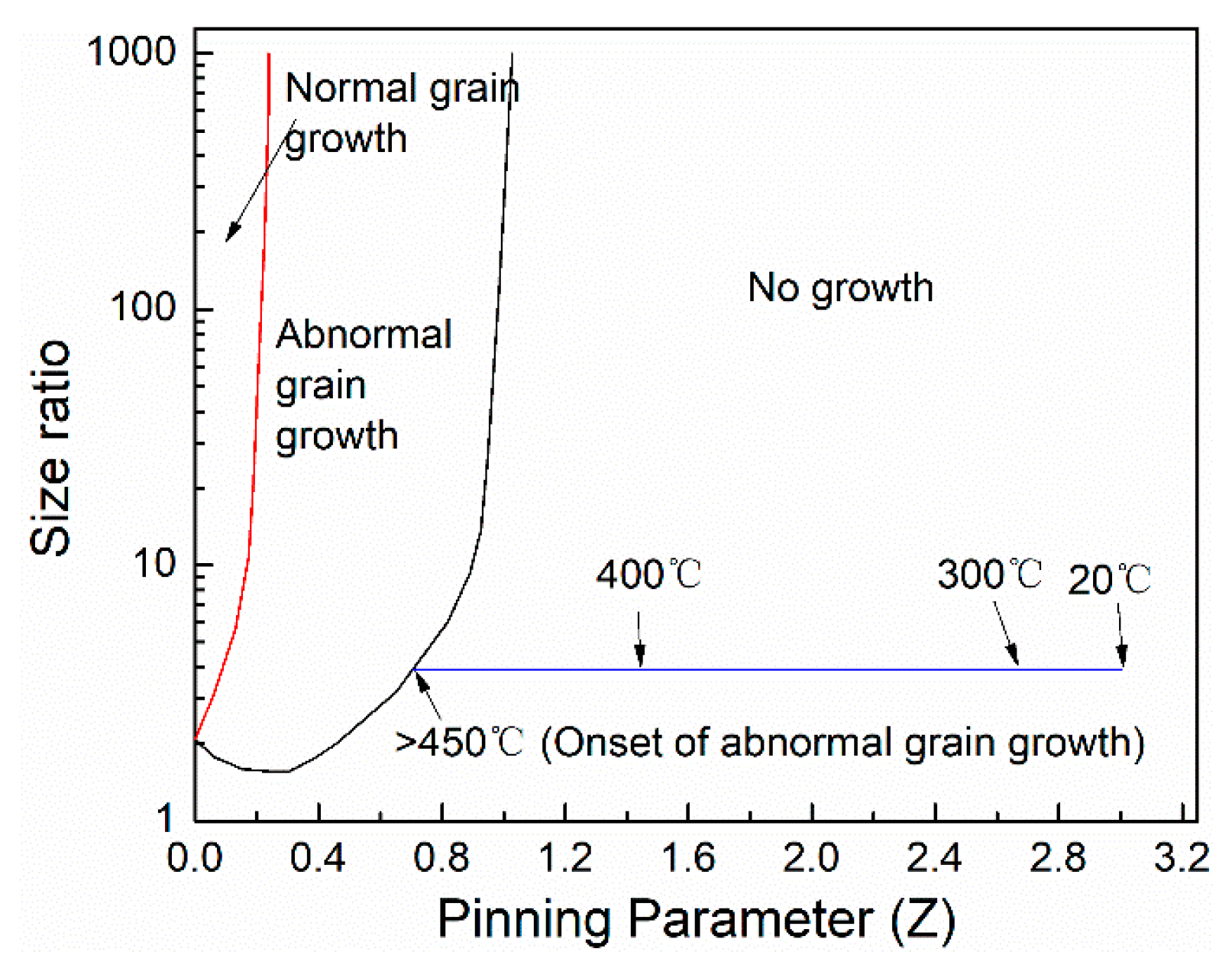
2.5. Effect of Microstructure Refinement Techniques on the Superplasticity of Al Alloys
2.6. Effect of Addition of Trace Elements in Alloys on the Superplasticity of Al Alloys
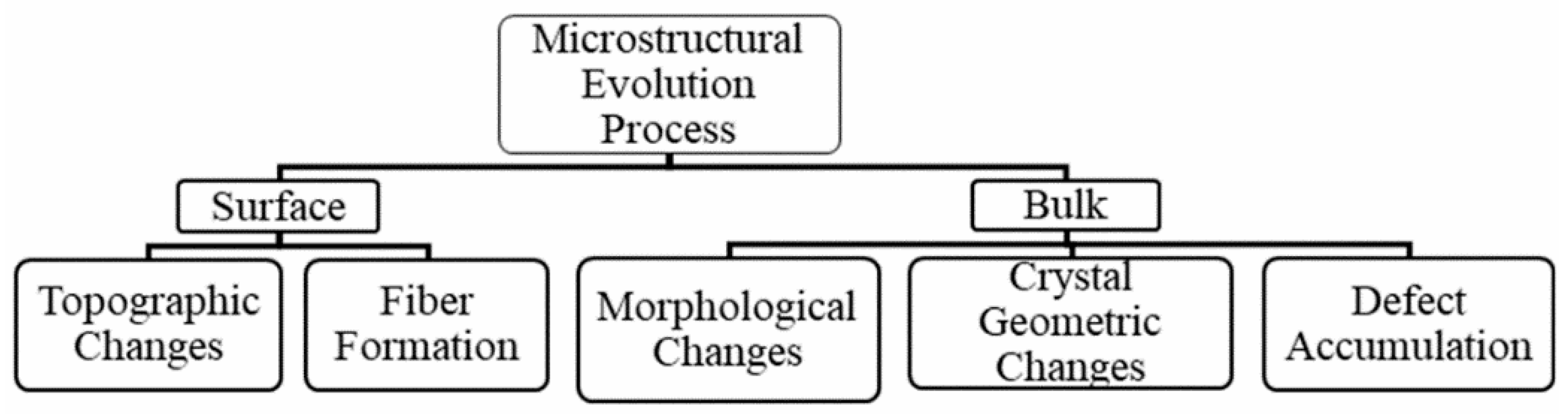
3. Superplastic Deformation Mechanism of Al Alloys
3.1. Superplastic Deformation Mechanisms
3.2. Constitutive Equations of Superplasticity
4. Summary and Prospect
Author Contributions
Funding
Conflicts of Interest
References
- Smolej, A.; Skaza, A.; Slacek, E. Superplasticity of the 5083 Aluminum alloy with the addition of Scandium. Mater. Technol. 2009, 14, 299–302. [Google Scholar]
- Edalati, K.; Masuda, T.; Arita, M.; Furui, M.; Sauvage, X.; Horita, Z.; Valiev, R.Z. Room-Temperature Superplasticity in an Ultrafine-Grained Magnesium Alloy. Sci. Rep. 2017, 7, 2662. [Google Scholar] [CrossRef] [PubMed]
- Kaibyshev, O.A. Superplasticity of Alloys, Intermetallides and Ceramics, 1st ed.; Springer: New York, NY, USA, 1992. [Google Scholar]
- Nieh, T.G.; Wadsworth, J.; Sherby, O.D. Superplasticity in Metals and Ceramics; Cambridge University Press: Cambridge, CA, USA, 1997. [Google Scholar]
- Smolej, A.; Skaza, B.; Markoli, B.; Klobčar, D.; Dragojević, V.; Slaček, E. Superplastic Behaviour of AA5083 Aluminium Alloy with Scandium and Zirconium. Mater. Sci. Forum 2012, 709, 395–401. [Google Scholar] [CrossRef]
- Li, Z.Q.; Zhu, H.L. Industrial applications of superplastic forming technology in China. Mater. Sci. Forum 2001, 357, 53–58. [Google Scholar] [CrossRef]
- Humphreys, F.J.; Lorimer, G.W. Thermomechanical Processing of Aluminium Alloys. Adv. Light Alloy. Compos. 1998, 59, 343–353. [Google Scholar]
- Pereira, P.H.R.; Huang, Y.; Kawasaki, M.; Langdon, T.G. An examination of the superplastic characteristics of Al–Mg–Sc alloys after processing. J. Mater. Res. 2017, 32, 4541–4553. [Google Scholar] [CrossRef]
- Valiev, R.Z.; Kuznetsov, R.I.; Kaibyshev, O.A.; Musalimov, R.S.; Tsenev, N.K. Low-temperature superplasticity of metallic materials. Sov. Phys. Dokl. 1988, 33, 626. [Google Scholar]
- Kapoor, R. Severe Plastic Deformation of Materials. In Materials under Extreme Conditions; Tyagi, A.K., Banerjee, S., Eds.; Elsevier: Mumbai, India, 2017; pp. 717–754. [Google Scholar]
- Zrnik, J.; Dobatkin, S.V.; Mamuzi, I. Processing of metals by severe plastic deformation (SPD)-Structure and mechanical properties respond. Metalurgija 2008, 47, 211–216. [Google Scholar]
- Kawasaki, M.; Langdon, T.G. Developing Superplasticity in Ultrafine-Grained Metals. Acta Phys. Pol. A 2015, 128, 470–478. [Google Scholar] [CrossRef]
- Azushima, A.; Kopp, R.; Korhonen, A.; Yang, D.Y.; Micari, F.; Lahoti, G.D.; Groche, P.; Yanagimoto, J.; Tsuji, N.; Rosochowski, A.; et al. Severe plastic deformation (SPD) processes for metals. Cirp Ann. 2008, 57, 716–735. [Google Scholar] [CrossRef]
- Furukawa, M.; Horita, Z.; Nemoto, M.; Langdon, T.G. Review Processing of metals by equal-channel angular pressing. J. Mater. Sci. 2001, 36, 2835–2843. [Google Scholar] [CrossRef]
- Valiev, R.Z.; Langdon, T.G. Principles of equal-channel angular pressing as a processing tool for grain refinement. Prog. Mater. Sci. 2006, 51, 881–981. [Google Scholar] [CrossRef]
- Zha, M.; Li, Y.; Mathiesen, R.H.; Baumgart, C. Influence of Mg content, grain size and strain rate on mechanical properties and DSA behavior of Al-Mg alloys processed by ECAP and annealing. Mater. Sci. Forum 2014, 794–796, 870–875. [Google Scholar] [CrossRef]
- Kapoor, R.; Chakravartty J, K. Deformation behavior of an ultrafine-grained Al–Mg alloy produced by equal-channel angular pressing. Acta Mater. 2007, 55, 5408–5418. [Google Scholar] [CrossRef]
- Huarte, B.; Luis, C.J.; Puertas, I.; Leon, J.; Luri, R. Optical and mechanical properties of an Al–Mg alloy processed by ECAE. J. Mater. Process. Technol. 2005, 162, 317–326. [Google Scholar] [CrossRef]
- Horita, Z.; Fujinami, T.; Nemoto, M.; Langdon, T.G. Equal-channel angular pressing of commercial aluminum alloys: Grain refinement, thermal stability and tensile properties. Metall. Mater. Trans. A 2000, 31, 691–701. [Google Scholar] [CrossRef]
- Chen, Y.C.; Huang, Y.Y.; Chang, C.P.; Kao, P.W. The effect of extrusion temperature on the development of deformation microstructures in 5052 aluminium alloy processed by equal channel angular extrusion. Acta Mater. 2003, 51, 2005–2015. [Google Scholar] [CrossRef]
- Thomas, W.M.; Nicholas, E.D.; Needham, J.C.; Murch, M.G.; Templesmith, P.; Dawes, C.J. Friction Stir Butt Welding. U.S. Patent PCT/GB92/02203, October 1995. [Google Scholar]
- García-Bernal, M.A.; Mishra, R.S.; Verma, R.; Hernández-Silva, D. Influence of friction stir processing tool design on microstructure and superplastic behavior of Al-Mg alloys. Mater. Sci. Eng. A 2016, 670, 9–16. [Google Scholar] [CrossRef]
- Eskandarzade, M.; Masoumi, A.; Faraji, G.; Mohammadpour, M.; Yan, X.S. A new designed incremental high pressure torsion process for producing long nanostructured rod samples. J. Alloys Compd. 2017, 695, 1539–1546. [Google Scholar] [CrossRef]
- Ivanisenko, Y.; Kulagin, R.; Fedorov, V.; Mazilkin, A.; Scherer, T.; Baretzky, B.; Hahn, H. High Pressure Torsion Extrusion as a new severe plastic deformation process. Mater. Sci. Eng. A 2016, 664, 247–256. [Google Scholar] [CrossRef]
- Edalati, K.; Lee, S.; Horita, Z. Continuous high-pressure torsion using wires. J. Mater. Sci. 2012, 47, 473–478. [Google Scholar] [CrossRef]
- Serra, D. Superplastic Forming Applications on Aero Engines. A Review of ITP Manufacturing Process. In Proceedings of the 6th EUROSPF Conference, Carcassonne, France, 3–5 September 2008; pp. 1–10. [Google Scholar]
- Xing, H.L.; Wang, C.W.; Zhang, K.F.; Wang, Z.R. Recent development in the mechanics of superplasticity and its applications. J. Mater. Process. Technol. 2004, 151, 196–202. [Google Scholar] [CrossRef]
- Zeng, Z.; Zhang, Y.; Zhou, Y.; Jin, Q. Superplastic forming of aluminum alloy car body panels. Mater. Sci. Forum 2005, 475, 3025–3028. [Google Scholar] [CrossRef]
- Avtokratova, E.; Sitdikov, O.; Markushev, M.; Mulyukov, R. Extraordinary high-strain rate superplasticity of severely deformed Al–Mg–Sc–Zr alloy. Mater. Sci. Eng. A 2012, 538, 386–390. [Google Scholar] [CrossRef]
- Kawasaki, M.; Langdon, T.G. Superplasticity in Ultrafine-Grained Materials. Rev. Adv. Mater. Sci. 2018, 54, 46–55. [Google Scholar] [CrossRef]
- Wang, G.; Jia, H.; Gu, Y.; Liu, Q. Research on Quick Superplastic Forming Technology of Industrial Aluminum Alloys for Rail Traffic. Defect Diffus. Forum 2018, 385, 468–473. [Google Scholar] [CrossRef]
- García-Infanta, J.M.; Zhilyaev, A.P.; Sharafutdinov, A.; Ruano, O.A.; Carreñoa, F. An evidence of high strain rate superplasticity at intermediate homologous temperatures in an Al–Zn–Mg–Cu alloy processed by high-pressure torsion. J. Alloys Compd. 2009, 473, 163–166. [Google Scholar] [CrossRef]
- Pearson, C.E. The viscous properties of extruded eutectic alloys of lead-tin and bismuth-tin. J. Inst. Met. 1934, 54, 111–123. [Google Scholar]
- Langdon, T.G. Seventy-five years of superplasticity: Historic developments and new opportunities. J. Mater. Sci. 2009, 44, 5998–6010. [Google Scholar] [CrossRef]
- Underwood, E.E. A review of Superplasticity. J. Met. 1962, 14, 914–919. [Google Scholar]
- Lee, D. The Nature of Superplastic Deformation in the MgAl Eutectic. Acta Metall. 1969, 17, 1057–1069. [Google Scholar] [CrossRef]
- Ishikawa, H.; Mohamed, F.A.; Langdon, T.G. The influence of strain rate on ductility in the superplastic Zn–22% Al eutectoid. Philos. Mag. 1975, 32, 1269–1271. [Google Scholar] [CrossRef]
- Grimes, R. Superplastic forming of advanced Metallic Materials. Woodhead Publ. 2011, 2011, 247–271. [Google Scholar]
- Horiuchi, R.; El-Sebai, A.B.; Otsuka, M. Superplasticity in the Ternary Eutectic Alloys, Al-33%Cu-7%Mg and Al-25%Cu-11%Mg. Scr. Metall. 1973, 7, 1101–1104. [Google Scholar] [CrossRef]
- Wadsworth, J.; Palmer, I.G.; Crooks, D.D. Superplasticity in Al-Li Based Alloys. Scr. Metall. 1983, 17, 347–352. [Google Scholar] [CrossRef]
- Higashi, K.; Ohnishi, T.; Nakatani, Y. Superplastic Behavior of Commercial Aluminum Bronze. Scr. Metall. 1985, 19, 821–823. [Google Scholar] [CrossRef]
- Wang, J.; Horita, Z.; Furukawa, M.; Nemoto, M.; Tsenev, N.K.; Valiev, R.Z.; MA, Y.; Langdon, T.G. An investigation of ductility and microstructural evolution in an Al-3% Mg alloy with submicron grain size. J. Mater. Res. 1993, 8, 2810–2818. [Google Scholar] [CrossRef]
- Shagiev, M.; Motohashi, Y.; Musin, F.; Kaibyshev, R.; Itoh, G. Superplastic Behavior in Al–Li–Mg–Cu–Sc Alloy Sheet. Mater. Trans. 2003, 44, 1694–1697. [Google Scholar] [CrossRef][Green Version]
- Haoyan, D.; Rehan, Q.; Tingting, W.; Shasha, Z.; Chaoli, M. Superplastic Behavior of Coarse-Grained Al-Mg Alloy. Int. J. Mod. Phys. Conf. Ser. 2012, 6, 401–406. [Google Scholar]
- Prasad, V.J.; Mohanarao, N.; Kamaluddin, S.; Bhattacharya, S.S. Development of superplasticity in an Al–Mg alloy through severe plastic deformation. Int. J. Adv. Manuf. Technol. 2018, 94, 2973–2979. [Google Scholar] [CrossRef]
- Luo, T.; Nia, D.R.; Xue, P.; Li, Y.Z.; Xiao, B.L.; Ma, Z.Y.; Fu, M.J.; Li, X.H.; Zeng, Y.S. Low-temperature superplasticity of nugget zone of friction stir welded Al-Mg alloy joint. Mater. Sci. Eng. A 2018, 727, 177–183. [Google Scholar] [CrossRef]
- Smolej, A.; Klobčar, D.; Skaza, B.; Nagode, A.; Slaček, E.; Dragojević, V.; Smolej, S. Superplasticity of the rolled and friction stir processed Al–4.5 Mg–0.35Sc–0.15Zr alloy. Mater. Sci. Eng. A 2014, 590, 239–245. [Google Scholar] [CrossRef]
- Xu, C.; Furukawa, M.; Horita, Z.; Langdon, T.G. Developing High Strain Rate Superplasticity in Aluminum Alloys. Mater. Sci. Forum 2015, 475, 2949–2954. [Google Scholar]
- Moguchevaa, A.; Yuzbekovab, D.; Kaibyshev, R. Superplasticity in a 5024 aluminium alloy subjected to ECAP and subsequent cold rolling. Mater. Sci. Forum 2016, 838, 428–433. [Google Scholar] [CrossRef]
- Valle, J.A.D.; Ruano, O.A. Influence of grain size fluctuations on ductility of superplastic magnesium alloys processed by severe plastic deformation. Mater. Sci. Technol. 2008, 24, 1238–1244. [Google Scholar] [CrossRef]
- Orozco-Caballero, A.; Álvarez, M.; Hidalgo-Manrique, P.; Cepeda-Jiménez, C.M.; Ruano, O.A.; Carreno, F. Grain size versus microstructural stability in the high strain rate superplastic response of a severely friction stir processed Al-Zn-Mg-Cu alloy. Mater. Sci. Eng. A 2017, 680, 329–337. [Google Scholar] [CrossRef]
- Asgharzadeh, H.; Mcqueen, H.J. Grain growth and stabilisation of nanostructured aluminium at high temperatures: Review. Mater. Sci. Technol. 2015, 31, 1016–1034. [Google Scholar] [CrossRef]
- Liew, K.M.; Tan, M.J.; Tan, H. Analysis of Grain Growth during Superplastic Deformation. Mech. Adv. Mater. Struct. 2007, 14, 541–547. [Google Scholar] [CrossRef]
- Masuda, H.; Kanazawa, T.; Tobe, H.; Sato, E. Dynamic anisotropic grain growth during superplasticity in Al–Mg–Mn alloy. Scr. Mater. 2018, 149, 84–87. [Google Scholar] [CrossRef]
- Ma, Z.; Mishra, R.S. (Eds.) High-Strain-Rate Superplasticity; Friction Stir Superplasticity for Unitized Structures: Oxford, UK, 2014; pp. 7–18. [Google Scholar]
- Mcnelley, T.R.; Oh-Ishi, K.; Zhilyaev, A.P.; Krajewski, P.E.; Swaminathan, S.; Taleff, E.M. Characteristics of the Transition from Grain-Boundary Sliding to Solute Drag Creep in Superplastic AA5083. Metall. Mater. Trans. A 2007, 39, 50–64. [Google Scholar] [CrossRef]
- Krauss, G. Deformation Processing and Structure. In Proceedings of the 1982 ASM Materials Science Seminar, St. Louis, MO, USA, 23–24 October 1982. [Google Scholar]
- Sherby, O.D.; Caligiuri, R.D.; Kayali, E.S.; White, R.A. Fundamentals of Superplasticity and Its Application; Bruke, J.J., Mehrabian, R., Weissm, V., Eds.; Advances in Metal Processing 1981; Springer: Boston, UK, 1981; pp. 133–171. [Google Scholar]
- Wadsworth, J.; Oyama, T.; Sherby, O.D. Advances in Materials Technology in the Americas. In Proceedings of the 6th Inter-American Conference on Materials Technology 1980, San Francisco, CA, USA, 12–15 August 1980. [Google Scholar]
- Tan, M.J.; Liew, K.M.; Tan, H. Cavitation and grain growth during superplastic forming. J. Achiev. Mater. Manuf. Eng. 2007, 24, 307–314. [Google Scholar]
- Li, S.; Huang, Z.; Jin, S. Superplastic Behavioral Characteristics of Fine-Grained 5A70 Aluminum Alloy. Metals 2019, 9, 62. [Google Scholar] [CrossRef]
- kh Hassan, A.A.; Norman, A.F.; Price, D.A.; Prangnell, P.B. Stability of nugget zone grain structures in high strength Al-alloy friction stir welds during solution treatment. Acta Mater. 2003, 51, 1923–1936. [Google Scholar] [CrossRef]
- Mishra, R.S.; Ma, Z.Y. Friction stir welding and processing. Mater. Sci. Eng. R 2005, 50, 1–78. [Google Scholar] [CrossRef]
- Charit, I.; Mishra, R.S. Abnormal grain growth in friction stir processed alloys. Scr. Mater. 2008, 58, 367–371. [Google Scholar] [CrossRef]
- Charit, I.; Mishra, R.S.; Mahoney, M.W. Multi-sheet structures in 7475 aluminum by friction stir welding in concert with post-weld superplastic forming. Scr. Mater. 2002, 47, 631–636. [Google Scholar] [CrossRef]
- Mahidharar, K. Effect of Grain Size on the Superplastic Behavior of a 7475 Aluminum Alloy. J. Mater. Eng. Perform. 1995, 4, 674–678. [Google Scholar] [CrossRef]
- Ma, Z.Y.; Mishra, R.S. Development of ultrafine-grained microstructure and low temperature (0.48 Tm) superplasticity in friction stir processed Al–Mg–Zr. Scr. Mater. 2005, 53, 75–80. [Google Scholar] [CrossRef]
- Orozco-Caballero, A.; Cepeda-Jiménez, C.M.; Hidalgo-Manrique, P.; Rey, P.; Gesto, D.; Verdera, D.; Ruano, O.A.; Carreño, F. Lowering the temperature for high strain rate superplasticity in an Al-Mg-Zn-Cu alloy via cooled friction stir processing. Mater. Chem. Phys. 2013, 142, 182–185. [Google Scholar] [CrossRef]
- Liu, F.C.; Ma, Z.Y.; Chen, L.Q. Low-temperature superplasticity of Al–Mg–Sc alloy produced by friction stir processing. Scr. Mater. 2009, 60, 968–971. [Google Scholar] [CrossRef]
- Nieh, T.G.; Hsiung, L.M.; Wadsworth, J.; Kaibyshev, R. High Strain Rate Superplasticity in a Continuously Recrystallized Al-6%Mg-0.3%Sc Alloy. Acta Mater. 1998, 46, 2789–2800. [Google Scholar] [CrossRef]
- Zhang, N.; Wang, Y.Q.; Hou, H.L.; Zhang, Y.L.; Dong, X.M.; Li, Z.Q. Superplastic deformation behavior of 7B04 Al alloy. J. Mater. Eng. 2017, 45, 27–33. [Google Scholar] [CrossRef]
- Alhamidi, A.; Horita, Z. Grain refinement and high strain rate superplasticity in aluminium 2024 alloy processed by high-pressure torsion. Mater. Sci. Eng. A 2015, 622, 139–145. [Google Scholar] [CrossRef]
- Li, F.; Roberts, W.T.; Bate, P.S. Superplasticity and the Development of Dislocation Structures in an Al-4.5%Mg Alloy. Acta Mater. 1996, 44, 217–233. [Google Scholar] [CrossRef]
- Noda, M.; Hirohashi, M.; Funami, K. Low Temperature Superplasticity and Its Deformation Mechanism in Grain Refinement of Al-Mg Alloy by Multi-Axial Alternative Forging. Mater. Trans. 2003, 44, 2288–2297. [Google Scholar] [CrossRef]
- Ota, S.; Akamatsu, H.; Neishi, K.; Furukawa, M.; Horita, Z.; Langdon, T.G. Low-Temperature Superplasticity in Aluminum Alloys Processed by Equal-Channel Angular Pressing. Mater. Trans. 2002, 43, 2364–2369. [Google Scholar] [CrossRef]
- Giuliano, G. Superplastic Forming of Advanced Metallic Materials; Woodhead Publishing: Cambridge, UK, 2011; pp. 3–33. [Google Scholar]
- Musin, F.; Kaibyshev, R.; Motohashi, Y.; Itoh, G. High strain rate superplasticity in a commercial Al–Mg–Sc alloy. Scr. Mater. 2004, 50, 511–516. [Google Scholar] [CrossRef]
- Xu, G.F.; Cao, X.W.; Zhang, T.; Duan, Y.L.; Peng, X.Y.; Deng, Y.; Yin, Z.M. Achieving high strain rate superplasticity of an Al-Mg-Sc-Zr alloy by a new asymmetrical rolling technology. Mater. Sci. Eng. A 2016, 672, 98–107. [Google Scholar] [CrossRef]
- Ma, Z.Y.; Mishra, R.S.; Mahoney, M.W.; Grimes, R. High strain rate superplasticity in friction stir processed Al-Mg-Zr alloy. Mater. Sci. Eng. A 2003, 351, 148–153. [Google Scholar] [CrossRef]
- Li, M.; Pan, Q.; Shi, Y.; Suna, X.; Xiang, H. High strain rate superplasticity in an Al–Mg–Sc–Zr alloy processed via simple rolling. Mater. Sci. Eng. A 2017, 687, 298–305. [Google Scholar] [CrossRef]
- Ye, L.; Zhang, X.; Zheng, D.; Liu, S.; Tang, J. Superplastic behavior of an Al–Mg–Li alloy. J. Alloys Compd. 2009, 487, 109–115. [Google Scholar] [CrossRef]
- Charit, I.; Mishra, R.S. Evaluation of microstructure and superplasticity in friction stir processed 5083 Al alloy. J. Mater. Res. 2004, 19, 3329–3342. [Google Scholar] [CrossRef]
- Lei, J.; Xiaolu, W.; Hui, L.; Yutao, Z.; Yonggang, Y.; Jianchao, C. High strain rate superplasticity of in situ Al3Zr/6063Al composites. Rare Metal Mat. Eng. 2016, 45, 2798–2803. [Google Scholar] [CrossRef]
- Mikhaylovskaya, A.V.; Yakovtseva, O.A.; Cheverikin, V.V.; Kotov, A.D.; Portnoy, V.K. Superplastic behaviour of Al-Mg-Zn-Zr-Sc-based alloys at high strain rates. Mater. Sci. Eng. A 2016, 659, 225–233. [Google Scholar] [CrossRef]
- Charit, I.; Mishra R, S. High strain rate superplasticity in a commercial 2024 Al alloy via friction stir processing. Mater. Sci. Eng. A 2003, 359, 290–296. [Google Scholar] [CrossRef]
- Straumal, B.B.; López, G.A.; Mittemeijer, E.J.; Gust, W.; Zhilyaev, A.P. Grain Boundary Phase Transitions in the Al–Mg System and Their Influence on High-Strain Rate Superplasticity. Defect Diffus. Forum 2003, 216, 307–312. [Google Scholar] [CrossRef]
- Hedworth, J.; Stowell, M.J. The Measurement of Strain-Rate Sensitivity in Superplastic Alloys. J. Mater. Sci. 1971, 6, 1061–1069. [Google Scholar] [CrossRef]
- Vairis, A. Superplasticity Effects and Strain Rate Dependency in A Material Joining Process. J. Eng. Sci. Technol. Rev. 2008, 1, 28–32. [Google Scholar] [CrossRef]
- Nazzal, M.A.; Khraisheh, M.K.; Abu-Farha, F.K. The effect of strain rate sensitivity evolution on deformation stability during superplastic forming. J. Mater. Process. Technol. 2007, 191, 189–192. [Google Scholar] [CrossRef]
- Arieli, A.; Mukherjee, A. Factors Affecting the Maximum Attainable Ductility in a Superplastic Titanium Alloy. Mater. Sci. Eng. 1980, 43, 47–54. [Google Scholar] [CrossRef]
- Friedman, P.A.; Copple, W.B. Superplastic response in Al-Mg sheet alloys. J. Mater. Eng. Perform. 2004, 13, 335–347. [Google Scholar] [CrossRef]
- Loucif, A.; Huang, Y.; Helbert, A.L.; Baudin, T.; Sabbaghianrad, S.; Langdon, T.G. Microtextural changes and superplasticity in an Al-7075 alloy processed by high-pressure torsion. Mater. Sci. Forum 2015, 838, 445–450. [Google Scholar] [CrossRef]
- Chentouf, S.M.; Belhadj, T.; Bombardier, N.; Brodusch, N.; Gauvin, R.; Jahazi, M. Influence of predeformation on microstructure evolution of superplastically formed Al 5083 alloy. Int. J. Adv. Manuf. Technol. 2017, 88, 2929–2937. [Google Scholar] [CrossRef]
- Wang, K.; Liu, F.C.; Ma, Z.Y.; Zhang, F.C. Realization of exceptionally high elongation at high strain rate in a friction stir processed Al–Zn–Mg–Cu alloy with the presence of liquid phase. Scr. Mater. 2011, 64, 572–575. [Google Scholar] [CrossRef]
- Park, K.T.; Lee, H.J.; Lee, C.S.; Shin, D.H. Effect of post-rolling after ECAP on deformation behavior of ECAPed commercial Al–Mg alloy at 723 K. Mater. Sci. Eng. A 2005, 393, 118–124. [Google Scholar] [CrossRef]
- Estrin, Y.; Vinogradov, A. Extreme grain refinement by severe plastic deformation: A wealth of challenging science. Acta Mater. 2013, 61, 782–817. [Google Scholar] [CrossRef]
- Segal, V.M. Engineering and commercialization of equal channel angular extrusion (ECAE). Mater. Sci. Eng. A 2004, 386, 269–276. [Google Scholar] [CrossRef]
- Mahoney, M.W.; Lynch, S.P. Friction-Stir Processing; Defense Technical Information Center: California, CA, USA, 2006. [Google Scholar]
- Mishra, R.S.; Mahoney, M.W. Friction stir processing: A new grain refinement technique to achieve high strain rate superplasticity in commercial alloys. Superplast. Adv. Mater. 2001, 357, 507–514. [Google Scholar] [CrossRef]
- Kawasaki, M.; Ahn, B.; Lee, H.; Zhilyaev, A.P.; Langdon, T.G. Using high-pressure torsion to process an aluminum–magnesium nanocomposite through diffusion bonding. J. Mater. Res. 2016, 31, 88–99. [Google Scholar] [CrossRef]
- Kulagina, R.; Beygelzimer, Y.; Ivanisenko, Y.; Mazilkin, A.; Hahn, H. Modelling of High Pressure Torsion using FEM. Procedia Eng. 2017, 207, 1445–1450. [Google Scholar] [CrossRef]
- Hallberg, H. Influence of process parameters on grain refinement in AA1050 aluminum during cold rolling. Int. J. Mech. Sci. 2013, 66, 260–272. [Google Scholar] [CrossRef]
- Karimi, M.; Toroghinejad, M.R.; Dutkiewicz, J. Nanostructure formation during accumulative roll bonding of commercial purity titanium. Mater. Charact. 2016, 122, 98–103. [Google Scholar] [CrossRef]
- Yu, H.L.; Su, L.; Lu, C.; Tieu, K.; Li, H.; Li, J.; Godbole, A.; Kong, C. Enhanced mechanical properties of ARB-processed aluminum alloy 6061 sheets by subsequent asymmetric cryorolling and ageing. Mater. Sci. Eng. A 2016, 674, 256–261. [Google Scholar] [CrossRef]
- Pesin, A.; Pustovoytov, D. Physical simulation of asymmetric sheet rolling process by multicycle shear-compression testing. Procedia Eng. 2017, 207, 1487–1492. [Google Scholar] [CrossRef]
- Wronski, S.; Bacroixb, B. Microstructure evolution and grain refinement in asymmetrically rolled aluminium. Acta Mater. 2014, 76, 404–412. [Google Scholar] [CrossRef]
- Yu, H.L.; Lu, C.; Tieu, K.; Liu, X.; Sun, Y.; Yu, Q.; Kong, C. Asymmetric cryorolling for fabrication of nanostructural aluminum sheets. Sci. Rep. 2012, 2, 772. [Google Scholar] [CrossRef]
- Anas, N.M.; Quah, W.L.; Zuhailawati, H.; Anasyida, A.S. Effect of immersion duration in liquid nitrogen for cryorolled A5052 aluminium sheet alloy. Procedia Chem. 2016, 19, 241–246. [Google Scholar] [CrossRef]
- Singh, D.; Rao, P.N.; Jayaganthan, R. Microstructures and impact toughness behavior of Al 5083 alloy processed by cryorolling and afterwards annealing. Int. J. Miner. Metall. Mater. 2013, 20, 759–769. [Google Scholar] [CrossRef]
- Yu, H.L.; Yan, M.; Li, J.; Godbole, A.; Lu, C.; Tieu, K.; Li, H.; Kong, C. Mechanical properties and microstructure of a Ti-6Al-4V alloy subjected to cold rolling, asymmetric rolling and asymmetric cryorolling. Mater. Sci. Eng. A 2018, 710, 10–16. [Google Scholar] [CrossRef]
- Kendig, K.L.; Miracle, D.B. Strengthening mechanisms of an Al-Mg-Sc-Zr alloy. Acta Mater. 2002, 50, 4165–4175. [Google Scholar] [CrossRef]
- Lee, S.; Utsunomiya, A.; Akamatsu, H.; Neishi, K.; Furukawa, M.; Horita, Z.; Langdon, T.G. Influence of scandium and zirconium on grain stability and superplastic ductilities in ultrafine-grained Al–Mg alloys. Acta Mater. 2002, 50, 553–564. [Google Scholar] [CrossRef]
- Sauvage, X.; Wilde, G.; Divinski, S.V.; Horita, Z.; Valiev, R.Z. Grain boundaries in ultrafine grained materials processed by severe plastic deformation and related phenomena. Mater. Sci. Eng. A 2012, 540, 1–12. [Google Scholar] [CrossRef]
- Henager, C.H.; Vetrano, J.S.; Gertsman, V.Y.; Bruemmer, S.M. Effect of Sn Additions on Superplasticity in Al-Mg-Mn-Sc Alloys. MRS Online Proc. Libr. Arch. (71026) 1999, 601, 31–36. [Google Scholar] [CrossRef]
- Kassner, M.E. Creep Fracture. In Fundamentals of Creep in Metals and Alloys 2015; Kassner, M.E., Ed.; Elsevier: Oxford, UK, 2015; pp. 233–260. [Google Scholar]
- Kishchik, A.A.; Mikhaylovskayaa, V.; Levchenkov, S.; Portnoyv, K. Formation of Microstructure and the Superplasticity of Al–Mg-Based Alloys. Phys. Met. Metallogr. 2017, 118, 96–103. [Google Scholar] [CrossRef]
- Kotov, A.D.; Mikhaylovskaya, A.V.; Portnoy, V.K. Effect of the solid-solution composition on the superplasticity characteristics of Al-Zn-Mg-Cu-Ni-Zr Alloys. Phys. Met. Metallogr. 2014, 115, 730–735. [Google Scholar] [CrossRef]
- Xiao, Y.Z.; Gao, C.R.; Ma, H.S.; Tian, S.P. Effect of Scandium on Superplasticity of Al-Mg alloys. Trans. Nonferrous Met. Soc. China 2001, 11, 235–238. [Google Scholar]
- Peng, Y.Y.; Yin, Z.M.; Bo, N.; Zhong, L. Effect of minor Sc and Zr on superplasticity of Al-Mg-Mn alloys. Trans. Nonferrous Met. Soc. China 2007, 17, 744–750. [Google Scholar] [CrossRef]
- Fujikawa, S.I. Scandium in pure aluminum and aluminum alloys—Its behavior and addition effects. J. Jpn. Inst. Light Met. 1999, 49, 128–144. [Google Scholar] [CrossRef]
- Shabestari, S.G.; Moemeni, H. Effect of copper and solidification conditions on the microstructure andmechanical properties of Al–Si–Mg alloys. J. Mater. Process. Technol. 2004, 153, 193–198. [Google Scholar] [CrossRef]
- Anyalebechi, P.N. Analysis of the effects of alloying elements on hydrogen solubility in liquid aluminum alloys. Scr. Metall. 1995, 33, 1209–1216. [Google Scholar] [CrossRef]
- Samuel, F.H.; Samuel, A.M.; Doty, H.W. Factors Controlling the Type and Morphology of Cu-Containing Phases in 319 Al Alloy (963–0). Trans. Am. Foundrymens Soc. 1996, 104, 893–902. [Google Scholar]
- Caceres, C.H.; Djurdjevic, M.B.; Stockwell, T.J.; Sokolowski, J.H. The effect of Cu content on the level of Microporosity in Al-Si-Cu-Mg Casting Alloys. Scr. Mater. 1999, 40, 631–637. [Google Scholar] [CrossRef]
- Hossain, A.; Kurny, A.S.W. Effects of Strain Rate on Tensile Properties and Fracture Behavior of Al-Si-Mg Cast Alloys with Cu Contents. Mater. Sci. Metall. Eng. 2013, 1, 27–30. [Google Scholar]
- Watanabe, H.; Ohori, K.; Takeuchi, Y. Superplastic behavior of Al-Mg-Cu alloys. Trans. Iron Steel Inst. Jpn. 1987, 27, 730–733. [Google Scholar] [CrossRef]
- Pushkareva, I.; Jin, H.O. Development of Al-Mg alloys with different levels of Mn and Fe for super-plastic forming. Mater. Sci. Forum 2015, 838–839, 202–207. [Google Scholar] [CrossRef]
- Ghosh, A.K.; Hamilton, C.H. Influences of Material Parameters and Microstructure on Superplastic Forming. Metall. Trans. A 1982, 13, 733–743. [Google Scholar] [CrossRef]
- Zelin, M.G. Processes of Microstructural Evolution during Superplastic Deformation. Mater. Charact. 1996, 37, 311–329. [Google Scholar] [CrossRef]
- Baudelet, B.; Lian, J. A composite model for superplasticity. J. Mater. Sci. 1995, 30, 1977–1981. [Google Scholar] [CrossRef]
- Tan, H.; Gao, P.; Lian, J. Microstructural modeling and numerical analysis for the superplastic forming process. Mater. Manuf. Process. 2001, 16, 331–340. [Google Scholar] [CrossRef]
- Zhang, J.-S. Mechanisms of Grain Boundary Sliding. In High Temperature Deformation and Fracture of Materials 2010; Zhang, J.-S., Ed.; Woodhead Publishing: Cambridge, UK, 2010; pp. 172–181. [Google Scholar]
- Langdon, T.G. Grain boundary sliding as a deformation mechanism during creep. Philos. Mag. 1970, 22, 689–700. [Google Scholar] [CrossRef]
- Lin, T.L.; Mclean, D. Changes Produced by Deformation in Grains and Grain Boundaries of Nickel. Met. Sci. J. 1968, 2, 108–113. [Google Scholar] [CrossRef]
- Crossman, F.W.; Ashby, M.F. The non-uniform flow of polycrystals by grain-boundary sliding accommodated by power-law creep. Acta Metall. 1975, 23, 425–440. [Google Scholar] [CrossRef]
- Ma, Z.; Mishra, R.S. Superplastic Deformation Mechanism. In Friction Stir Superplasticity for Unitized Structures 2014; Ma, Z., Mishra, R.S., Eds.; Elsevier: Oxford, UK, 2014; pp. 39–57. [Google Scholar]
- Ball, A.; Hutchison, M.M. Superplasticity in the Aluminium-Zinc Eutectoid. Met. Sci. J. 1969, 3, 1–7. [Google Scholar] [CrossRef]
- Mukherjee, A.K. The rate controlling mechanism in superplasticity. Mater. Sci. Eng. 1971, 8, 83–89. [Google Scholar] [CrossRef]
- Gifkins, R.C. Grain-Boundary Sliding and its Accommodation during Creep and Superplasticity. Metall. Trans. A 1976, 7, 1225–1232. [Google Scholar] [CrossRef]
- Perez-Prado, M.T.; Kassner, M.E. Superplasticity. In Fundamentals of Creep in Metals and Alloy; Kassner, M.E., Ed.; Butterworth-Heinemann: Oxford, UK, 2015; pp. 139–157. [Google Scholar]
- Lifshitz, I.M. On the Theory of Diffusion-viscous Flow of Polycrystalline Bodies. J. Exp. Theor. Phys. 1963, 17, 909. [Google Scholar]
- Rachinger, W.A. Relative Grain Translations in the Plastic Flow of Aluminum. J. Inst. Met. 1952, 81, 33. [Google Scholar]
- Langdon, T.G. A unified approach to grain boundary sliding in creep and superplasticity. Acta Metall. 1994, 42, 2437–2443. [Google Scholar] [CrossRef]
- Salama, A. Analysis of Grain Refinement and Superplasticity in Aluminum-Magnesium Alloys, in Department of Mechanical Engineering 1987; Naval Postgraduate School: Monterey, CA, USA, 1987; p. 166. [Google Scholar]
- Ashby, M.F.; Verrall, R.A. Diffusion-accommodated flow and superplasticity. Acta Metall. 1973, 21, 149–163. [Google Scholar] [CrossRef]
- Perez-Prado, M.T.; González-Doncel, G.; Ruano, O.A.; Mcnelley, T.R. Texture Analysis of the Transition from Slip to Grain Boundary Sliding in a Discontinuously Recrystallized Superplastic Aluminum Alloy. Acta Mater. 2001, 49, 2259–2268. [Google Scholar] [CrossRef]
- Hsiao, I.C.; Huang, J.C. Deformation Mechanisms during Low-and High-Temperature Superplasticity in 5083 Al-Mg Alloy. Metall. Mater. Trans. A 2002, 33, 1373–1384. [Google Scholar] [CrossRef]
- Kulas, M.-A.; Green, W.P.; Taleff, E.M.; Krajewski, P.E.; Mcnelley, T.R. Deformation Mechanisms in Superplastic AA5083 Materials. Metall. Mater. Trans. A 2005, 36, 1249–1261. [Google Scholar] [CrossRef]
- Duan, Y.-L.; Qian, J.J.; Xiao, D.; Cui, X.-M.; Xu, G.-F. Effect of Sc and Er additions on superplastic ductilities in Al-Mg-Mn-Zr alloy. J. Cent. South. Univ. 2016, 23, 1283–1292. [Google Scholar] [CrossRef]
- Stevens, R.N. Grain-boundary sliding and diffusion creep in polycrystalline solids. Philos. Mag. 1971, 23, 265–283. [Google Scholar] [CrossRef]
- Prabu, S.B.; Padmanabhan, K.A. Superplasticity in and Superplastic Forming of Aluminum–Lithium Alloys. In Aluminium-Lithium Alloys; Elsevier: Amsterdam, The Netherlands, 2014; pp. 221–258. [Google Scholar]
- Mukherjee, A.K.; Mishra, R.S. Superplasticity. In Encyclopedia of Materials: Science and Technology 2001; Jürgen Buschow, K.H., Cahn, R.W., Flemings, M.C., Ilschner, B., Kramer, E.J., Mahajan, S., Veyssière, P., Eds.; Elsevier: Amsterdam, The Netherlands, 2001; pp. 8977–8981. [Google Scholar]
- Sergueeva, A.; Mukherjee, A. Superplastic deformation in nanocrystalline metals and alloys. In Nanostructured Metals and Alloys 2011; Whang, S.H., Ed.; Woodhead Publishing: Cambridge, UK, 2011; pp. 542–593. [Google Scholar]
- Han, Y.B.; Li, Z.L.; Zhou, G.; Cao, F.R.; Ding, H.; Yu, F.X. Constitutive Equation of an Al-12.7Si-0.7Mg Alloy in Superplastic Deformation. Adv. Mater. Res. 2012, 415, 1147–1152. [Google Scholar] [CrossRef]
- Ruano, O.A.; Sherby, O.D. On constitutive equations for various diffusion-controlled creep mechanisms. Rev. de Phys. Appl. 1988, 23, 625–637. [Google Scholar] [CrossRef]
- Wang, X.G.; Li, Q.S.; Wu, R.R.; Zhang, X.Y.; Ma, L. A Review on Superplastic Formation Behavior of Al Alloys. Adv. Mater. Sci. Eng. 2018, 2018, 7606140. [Google Scholar] [CrossRef]
- Backofen, W.A.; Turner, I.R.; Avery, D.H. Superplasticity in an Al-Zn alloy. Trans. ASM 1964, 57, 980–990. [Google Scholar]
- Edington, J.W. Physical metallurgy of superplasticity. Met. Technol. 1976, 3, 138–153. [Google Scholar] [CrossRef]
- Maestas, T.A. Study of Processing and Microstructure of a Superplastic 5083 Aluminum Alloy 2002; Naval Postgraduate School: Monterey, CA, USA, 2002; pp. 1–77. [Google Scholar]
- Somekawa, H.; Mukai, T. Effect of dominant diffusion process on cavitation behavior in superplastic Mg–Al–Zn alloy. Scr. Mater. 2007, 57, 1008–1011. [Google Scholar] [CrossRef]
- Ma, Z.Y.; Mishra, R.S. Cavitation in superplastic 7075Al alloys prepared via friction stir processing. Acta Mater. 2003, 51, 3551–3569. [Google Scholar] [CrossRef]
- Nieh, T.G.; Wadsworth, J.; Imai, T. A Rheological view of High Strain Rate Superplasticity in Alloys and Metal Matrix Composites. Scr. Metall. 1992, 26, 703–708. [Google Scholar] [CrossRef]
- Rossard, C. Formation de la striction dans la déformation a chaud par traction. Rev. de Metall. 1966, 63, 225–236. [Google Scholar] [CrossRef]
- Song, Y.; Gao, B.; Wang, X. Semiviscosity flow equation with variable parameters under superplastic tension conditions. Sci. China Ser. E Technol. Sci. 1998, 41, 242–246. [Google Scholar] [CrossRef]
- Churyumov, A.Y.; Mikhailovskaya, A.V.; Kotov, A.D.; Bazlov, A.I.; Portnoi, V.K. Development of Mathematical Models of Superplasticity Properties as a Function of Parameters of Aluminum Alloys of Al–Mg–Si System. Phys. Met. Metallogr. 2013, 114, 272–278. [Google Scholar] [CrossRef]
- Mabuchi, M.; Higashi, K. Superplastic constitutive equation and rate-controlling process in aluminum matrix composites with discontinuous fiber and particle reinforcements. J. Mater. Res. 1998, 13, 640–648. [Google Scholar] [CrossRef]
- Khaleel, M.A.; Zbib, H.M.; Nyberg, E.A. Constitutive modeling of deformation and damage in superplastic materials. Int. J. Plast. 2001, 17, 277–296. [Google Scholar] [CrossRef]
- Guan, Z.; Ren, M.; Zhao, P.; Ma, P.; Wang, Q. Constitutive equations with varying parameters for superplastic flow behavior of Al–Zn–Mg–Zr alloy. Mater. Des. 2014, 54, 906–913. [Google Scholar] [CrossRef]













| Al alloys (wt.%) | Temp., Strain Rate (°C), (s−1) | Grain size (µm) | Process | Elongation (%) | Refs. |
|---|---|---|---|---|---|
| Al-4Mg-0.6Mn-0.3Sc | 550, 7.5 × 10−4 | 8–14 | Hot and cold rolling | 1400 | [1] |
| Al-5%Mg 0.2% Sc 0.15%Zr | 510, 1 × 10−2 | 5 | FSP | 1500 | [5] |
| Al–1.9Li–1.0Mg–1.7Cu–0.03Sc–0.08Zr | 450, 1.4 × 10−2 | 1–3 | Hot rolling | 415 | [43] |
| Al-2.35Mg-0.16Cr-0.03Mn | 425, 3.78 × 10−3 | 33 | Cold Rolling | 181 | [44] |
| Al–5%Mg–0.3%Sc | 550, 1.04 × 10−1 | 10 | Hot forging and cold rolling | 212 | [45] |
| 5083 Al | 300, 3 × 10−4 | 1.6–1.8 | FSW | 550–570 | [46] |
| Al-4.72Mg-0.35Sc-0.168Zr-0.016Fe-0.012Ti-0.007Si | 500, 1 × 10−2 | 1.3 | FSP | 1900 | [47] |
| 7034 Al | 400, 1 × 10−2 | 0.3 | ECAP | >1000 | [48] |
| AA 5024 | 450, 5.6 × 10−1 | 0.3 | ECAP | 3300 | [49] |
| Equations | Key Points | Ref. |
|---|---|---|
| It is used in the simple stress state without any strain effects | Backofen et al. [157] | |
| It involves the combined effect of the strain rate sensitivity (m) and the strain hardening (n) | Rossard et al. [163] | |
| It consists of equations with varying m based on Backofen equation | Song et al. [164] | |
| Backofen equation was modified to consider the effect of size and volume of particles of the second phase | Churyumov et al. [165] | |
| It describes the superplasticity in matrix composite (for aluminum matrix composite with Si3N4 whiskers (20%), n = p = 2) | Mabuchi and Higashi [166] | |
| Evaluates the deformation and internal damage of AA5083 alloy | Khaleel et al. [167] | |
| Phenomenological constitute equation for Al–Zn–Mg–Zr alloy | Guan et al. [168] |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bhatta, L.; Pesin, A.; Zhilyaev, A.P.; Tandon, P.; Kong, C.; Yu, H. Recent Development of Superplasticity in Aluminum Alloys: A Review. Metals 2020, 10, 77. https://doi.org/10.3390/met10010077
Bhatta L, Pesin A, Zhilyaev AP, Tandon P, Kong C, Yu H. Recent Development of Superplasticity in Aluminum Alloys: A Review. Metals. 2020; 10(1):77. https://doi.org/10.3390/met10010077
Chicago/Turabian StyleBhatta, Laxman, Alexander Pesin, Alexander P. Zhilyaev, Puneet Tandon, Charlie Kong, and Hailiang Yu. 2020. "Recent Development of Superplasticity in Aluminum Alloys: A Review" Metals 10, no. 1: 77. https://doi.org/10.3390/met10010077
APA StyleBhatta, L., Pesin, A., Zhilyaev, A. P., Tandon, P., Kong, C., & Yu, H. (2020). Recent Development of Superplasticity in Aluminum Alloys: A Review. Metals, 10(1), 77. https://doi.org/10.3390/met10010077






