Hot Deformation and Dynamic Recrystallization Behavior of CoCrNi and (CoCrNi)94Ti3Al3 Medium Entropy Alloys
Abstract
:1. Introduction
2. Materials and Methods
3. Results
3.1. Flow Behaviors
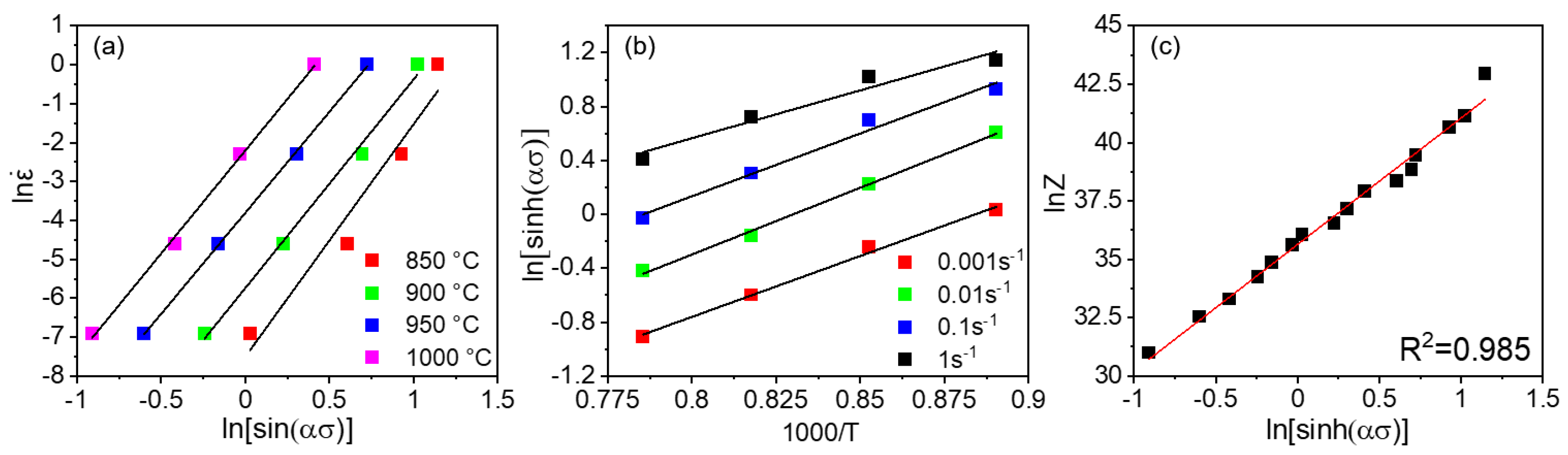
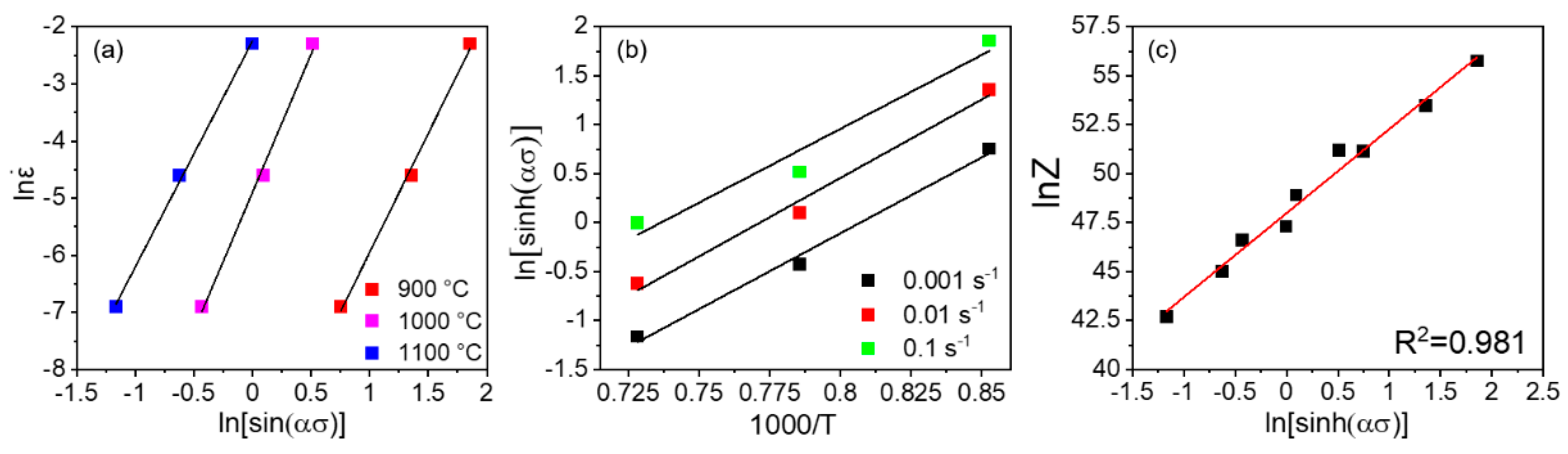
3.2. Calculation of Hot Deformation Parameters
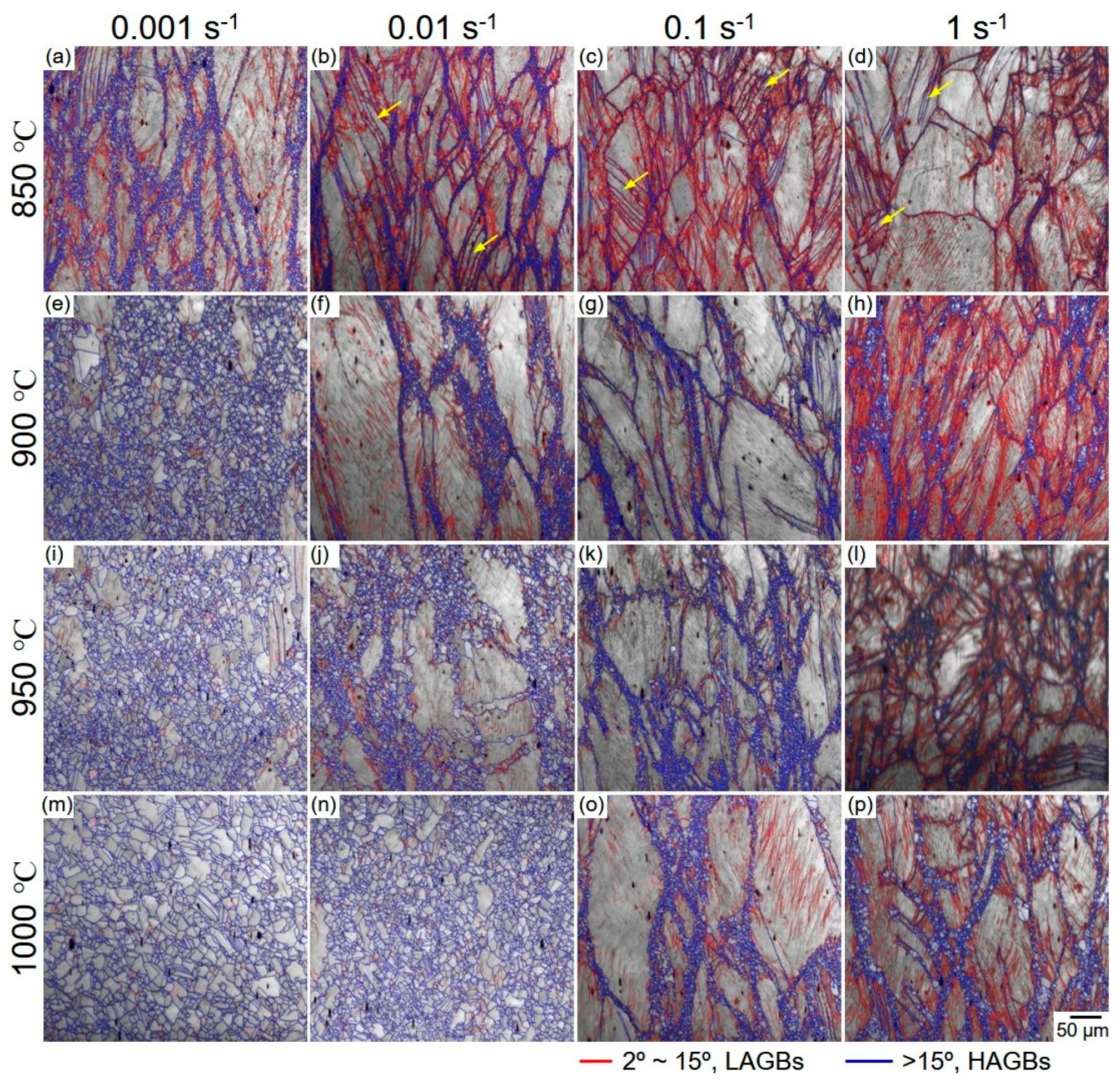
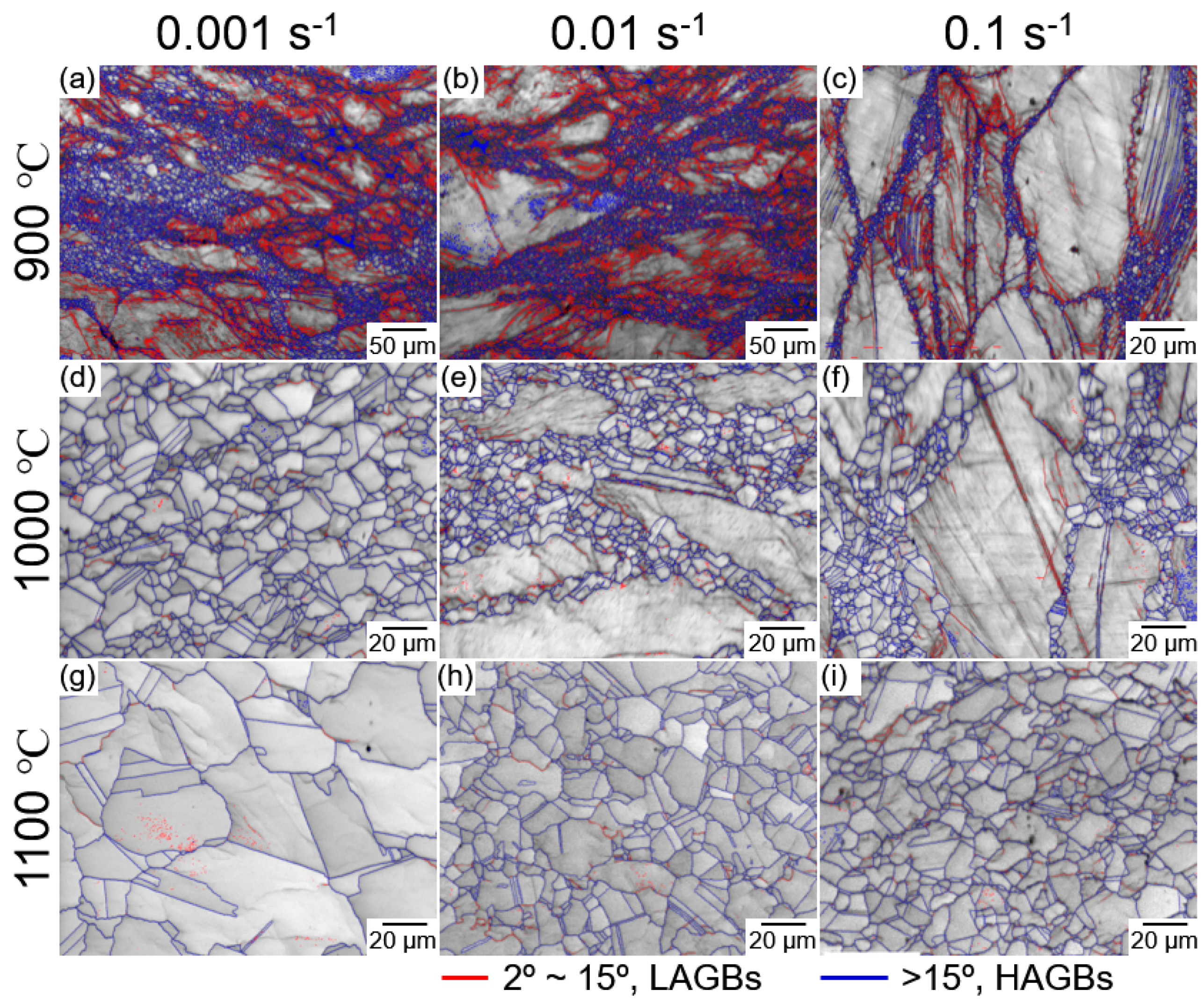
3.3. Microstructure After Hot Deformation
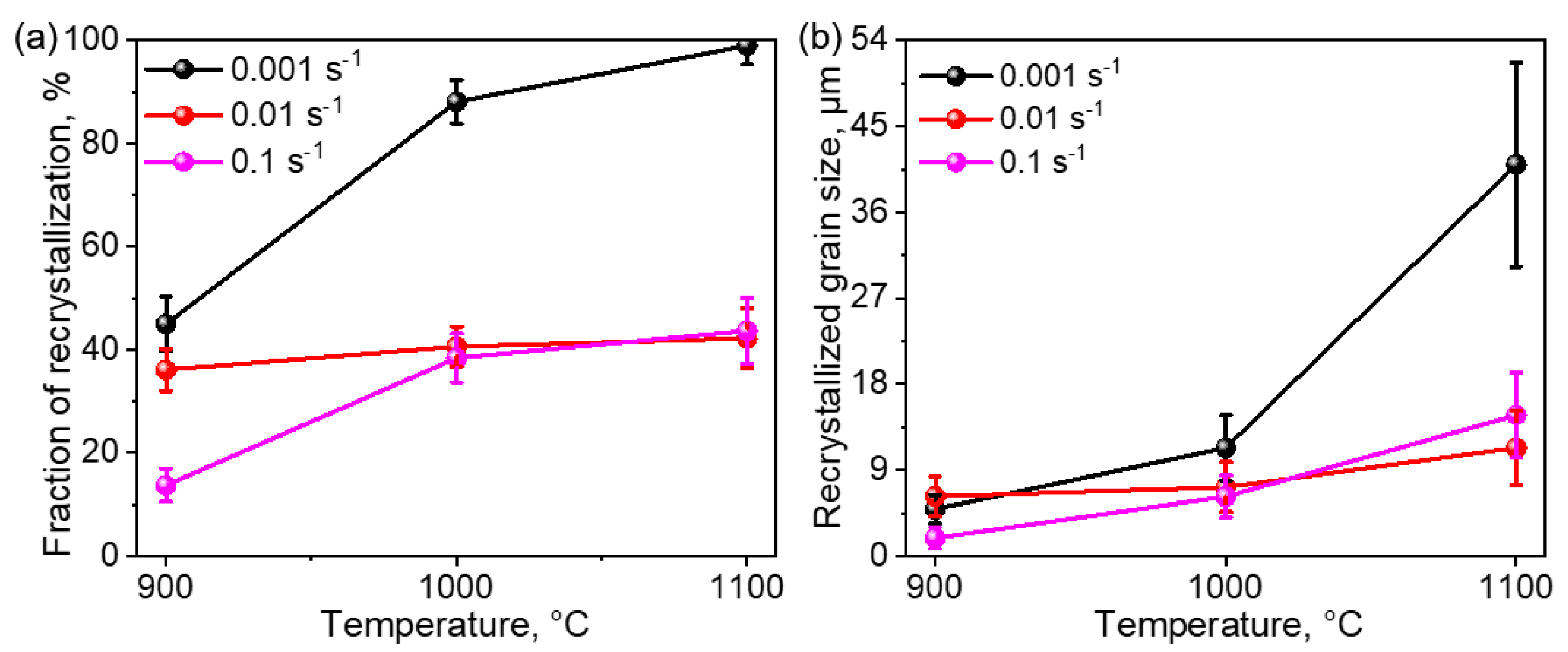
3.4. Statistics of Recrystallization
4. Discussion
4.1. Flow Behavior Analysis
4.2. Correlation Between Microstructure and Recrystallization
5. Conclusions
- Constitutive equations describing the relationship between flow stress and compression condition were obtained, where the hyperbolic-sine of the flow stress has a linear relationship with the Zener–Hollomon parameter in the two alloys. The apparent activation energy for the hot deformation was evaluated as 401.08 kJ/mol (CoCrNi) and 566.128 kJ/mol ((CoCrNi)94Ti3Al3), respectively, which is higher than that of CoCrFeMnNi high entropy alloys.
- In both alloys, the flow curves show peak stress followed by softening caused by the continuous dynamic recrystallization (cDRX) and discontinuous dynamic recrystallization (dDRX). According to the microstructure evolutions, the dDRX prefers to proceed at a small Zener–Hollomon parameter (high temperature and low strain rate).
- The temperature of 1000 °C and strain rate of 0.001 s−1 is the optimum condition for obtaining fully-recrystallized homogeneous grain structures, where the average grain size is 17 μm and 12 μm for the CoCrNi and (CoCrNi)94Ti3Al3 MEAs after compression to a true strain of 1.0. The recrystallized grains exhibited very weak textures, and the nucleation is without any preferential orientation selection.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Yeh, J.W.; Chen, S.K.; Lin, S.J.; Gan, J.Y.; Chin, T.S.; Shun, T.T.; Tsau, C.H.; Chang, S.Y. Nanostructured high-entropy alloys with multiple principal elements: Novel alloy design concepts and outcomes. Adv. Eng. Mater. 2004, 6, 299–303. [Google Scholar] [CrossRef]
- Cantor, B.; Chang, I.T.H.; Knight, P.; Vincent, A.J.B. Microstructural development in equiatomic multicomponent alloys. Mater. Sci. Eng. A 2004, 375–377, 213–218. [Google Scholar] [CrossRef]
- Gludovatz, B.; Hohenwarter, A.; Catoor, D.; Chang, E.H.; George, E.P.; Ritchie, R.O. A fracture-resistant high-entropy alloy for cryogenic applications. Science 2014, 345, 1153–1158. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Otto, F.; Dlouhý, A.; Somsen, C.; Bei, H.; Eggeler, G.; George, E.P. The influences of temperature and microstructure on the tensile properties of a CoCrFeMnNi high-entropy alloy. Acta Mater. 2013, 61, 5743–5755. [Google Scholar] [CrossRef] [Green Version]
- Gali, A.; George, E.P. Tensile properties of high- and medium-entropy alloys. Intermetallics 2013, 39, 74–78. [Google Scholar] [CrossRef] [Green Version]
- Li, Z.; Pradeep, K.G.; Deng, Y.; Raabe, D.; Tasan, C.C. Metastable high-entropy dual-phase alloys overcome the strength-ductility trade-off. Nat. Cell Biol. 2016, 534, 227–230. [Google Scholar] [CrossRef]
- Wei, D.; Li, X.; Heng, W.; Koizumi, Y.; He, F.; Choi, W.-M.; Lee, B.-J.; Kim, H.S.; Kato, H.; Chiba, A. Novel Co-rich high entropy alloys with superior tensile properties. Mater. Res. Lett. 2018, 7, 82–88. [Google Scholar] [CrossRef] [Green Version]
- Wei, D.; Li, X.; Jiang, J.; Heng, W.; Koizumi, Y.; Choi, W.-M.; Lee, B.-J.; Kim, H.S.; Kato, H.; Chiba, A. Novel Co-rich high performance twinning-induced plasticity (TWIP) and transformation-induced plasticity (TRIP) high-entropy alloys. Scr. Mater. 2019, 165, 39–43. [Google Scholar] [CrossRef]
- Wei, D.; Li, X.; Schönecker, S.; Jiang, J.; Choi, W.M.; Lee, B.J.; Kim, H.S.; Chiba, A.; Kato, H. Development of strong and ductile metastable face-centered cubic single-phase high-entropy alloys. Acta Mater. 2019, 181, 318–330. [Google Scholar] [CrossRef]
- De Cooman, B.C.; Estrin, Y.; Kim, S.K. Twinning-induced plasticity (TWIP) steels. Acta Mater. 2018, 142, 283–362. [Google Scholar] [CrossRef]
- Wei, D.; Koizumi, Y.; Takashima, T.; Nagasako, M.; Chiba, A. Fatigue improvement of electron beam melting-fabricated biomedical Co–Cr–Mo alloy by accessible heat treatment. Mater. Res. Lett. 2017, 6, 93–99. [Google Scholar] [CrossRef] [Green Version]
- Wei, D.-X.; Anniyaer, A.; Koizumi, Y.; Aoyagi, K.; Nagasako, M.; Kato, H.; Chiba, A. On microstructural homogenization and mechanical properties optimization of biomedical Co-Cr-Mo alloy additively manufactured by using electron beam melting. Addit. Manuf. 2019, 28, 215–227. [Google Scholar] [CrossRef]
- Liu, S.; Wu, Y.; Wang, H.; He, J.; Liu, J.; Chen, C.; Liu, X.; Lu, Z. Stacking fault energy of face-centered-cubic high entropy alloys. Intermetallics 2018, 93, 269–273. [Google Scholar] [CrossRef]
- Laplanche, G.; Kostka, A.; Reinhart, C.; Hunfeld, J.; Eggeler, G.; George, E.P. Reasons for the superior mechanical properties of medium-entropy CrCoNi compared to high-entropy CrMnFeCoNi. Acta Mater. 2017, 128, 292–303. [Google Scholar] [CrossRef]
- He, J.; Wang, H.; Huang, H.; Xu, X.; Chen, M.; Wu, Y.; Liu, X.; Nieh, T.; An, K.; Lu, Z. A precipitation-hardened high-entropy alloy with outstanding tensile properties. Acta Mater. 2016, 102, 187–196. [Google Scholar] [CrossRef] [Green Version]
- Zhao, Y.; Yang, T.; Tong, Y.; Wang, J.; Luan, J.; Jiao, Z.; Chen, D.; Yang, Y.; Hu, A.; Liu, C.; et al. Heterogeneous precipitation behavior and stacking-fault-mediated deformation in a CoCrNi-based medium-entropy alloy. Acta Mater. 2017, 138, 72–82. [Google Scholar] [CrossRef]
- Yang, T.; Zhao, Y.; Luan, J.; Han, B.; Wei, J.; Kai, J.; Liu, C. Nanoparticles-strengthened high-entropy alloys for cryogenic applications showing an exceptional strength-ductility synergy. Scr. Mater. 2019, 164, 30–35. [Google Scholar] [CrossRef]
- Zhao, Y.; Yang, T.; Han, B.; Luan, J.; Chen, D.; Kai, W.; Liu, C.T.; Kai, J.J. Exceptional nanostructure stability and its origins in the CoCrNi-based precipitation-strengthened medium-entropy alloy. Mater. Res. Lett. 2019, 7, 152–158. [Google Scholar] [CrossRef] [Green Version]
- Wei, D.-X.; Koizumi, Y.; Nagasako, M.; Chiba, A. Refinement of lamellar structures in Ti-Al alloy. Acta Mater. 2017, 125, 81–97. [Google Scholar] [CrossRef]
- Wei, D.-X.; Koizumi, Y.; Chiba, A. Discontinuous yielding and microstructural evolution of Ti-40 at.% Al alloy compressed in single α-hcp phase region. J. Alloy. Compd. 2017, 693, 1261–1276. [Google Scholar] [CrossRef]
- Wei, D.-X.; Koizumi, Y.; Li, Y.; Yamanak, K.; Chiba, A.; Yamanaka, K. Submicron lamellar porous structure formed by selective dissolution of Ti-Al alloy. Mater. Des. 2016, 98, 1–11. [Google Scholar] [CrossRef]
- Prasad, N.; Bibhanshu, N.; Nayan, N.; Avadhani, G.S.; Suwas, S. Hot deformation behavior of the high-entropy alloy CoCuFeMnNi. J. Mater. Res. 2019, 34, 744–755. [Google Scholar] [CrossRef]
- Reddy, S.R.; Bapari, S.; Bhattacharjee, P.P.; Chokshi, A.H. Superplastic-like flow in a fine-grained equiatomic CoCrFeMnNi high-entropy alloy. Mater. Res. Lett. 2017, 5, 408–414. [Google Scholar] [CrossRef] [Green Version]
- Eleti, R.R.; Bhattacharjee, T.; Zhao, L.; Bhattacharjee, P.P.; Tsuji, N. Hot deformation behavior of CoCrFeMnNi FCC high entropy alloy. Mater. Chem. Phys. 2018, 210, 176–186. [Google Scholar] [CrossRef]
- Stepanov, N.D.; Shaysultanov, D.G.; Yurchenko, N.Y.; Zherebtsov, S.V.; Ladygin, A.N.; Salishchev, G.A.; Tikhonovsky, M.A. High temperature deformation behavior and dynamic recrystallization in CoCrFeNiMn high entropy alloy. Mater. Sci. Eng. A 2015, 636, 188–195. [Google Scholar] [CrossRef]
- Li, J.; Gao, B.; Tang, S.; Liu, B.; Liu, Y.; Wang, Y.T.; Wang, J. High temperature deformation behavior of carbon-containing FeCoCrNiMn high entropy alloy. J. Alloy. Compd. 2018, 747, 571–579. [Google Scholar] [CrossRef]
- Sellars, C.M.; McTegart, W.J. On the mechanism of hot deformation. Acta Met. 1966, 14, 1136–1138. [Google Scholar] [CrossRef]
- Phaniraj, C.; Samantaray, D.; Mandal, S.; Bhaduri, A.K. A new relationship between the stress multipliers of Garofalo equation for constitutive analysis of hot deformation in modified 9Cr-1Mo (P91) steel. Mater. Sci. Eng. A 2011, 528, 6066–6071. [Google Scholar] [CrossRef]
- Sellars, C.M.; Tegart, W.J. Hot workability. Int. Metall. Rev. 1972, 17, 1–24. [Google Scholar] [CrossRef]
- Zener, C.; Hollomon, J.H. Effect of strain rate upon plastic flow of steel. J. Appl. Phys. 1944, 15, 22–32. [Google Scholar] [CrossRef]
- McQueen, H.J.; Jonas, J.J. Treatise on Materials Science and Technology, Vol.6: Plastic Deformation of Materials; Academic Press: New York, NY, USA, 1975; pp. 393–493. [Google Scholar]
- Eleti, R.R.; Bhattacharjee, T.; Shibata, A.; Tsuji, N. Unique deformation behavior and microstructure evolution in high temperature processing of HfNbTaTiZr refractory high entropy alloy. Acta Mater. 2019, 171, 132–145. [Google Scholar] [CrossRef]
- Senkov, O.N.; Scott, J.M.; Senkova, S.V.; Meisenkothen, F.; Miracle, D.B.; Woodward, C.F. Microstructure and elevated temperature properties of a refractory TaNbHfZrTi alloy. J. Mater. Sci. 2012, 47, 4062–4074. [Google Scholar] [CrossRef]
- Vinogradov, A.; Agletdinov, E.; Yasnikov, I.S.; Máthis, K.; Estrin, Y. A phenomenological model of twinning-mediated strain hardening. Mater. Sci. Eng. A 2020, 780, 139194. [Google Scholar] [CrossRef]
- Galiyev, A.; Kaibyshev, R.; Gottstein, G. Correlation of plastic deformation and dynamic recrystallization in magnesium alloy ZK60. Acta Mater. 2001, 49, 1199–1207. [Google Scholar] [CrossRef]
- Dudova, N.; Belyakov, A.; Sakai, T.; Kaibyshev, R. Dynamic recrystallization mechanisms operating in a Ni–20%Cr alloy under hot-to-warm working. Acta Mater. 2010, 58, 3624–3632. [Google Scholar] [CrossRef]
- Humphreys, F.J.; Hatherly, M. Recrystallization and Related Annealing Phenomena; Elsevier: Oxford, UK, 1995. [Google Scholar]
- Lu, S.H.; Wu, D.; Chen, R.S.; Han, E.H. Reasonable utilization of {10-12} twin for optimizing microstructure and improving mechanical property in a Mg-Gd-Y alloy. Mater. Des. 2020, 191, 108600. [Google Scholar] [CrossRef]
- Tikhonova, M.; Belyakov, A.; Kaibyshev, R. Strain-induced grain evolution in an austenitic stainless steel under warm multiple forging. Mater. Sci. Eng. A 2013, 564, 413–422. [Google Scholar] [CrossRef]

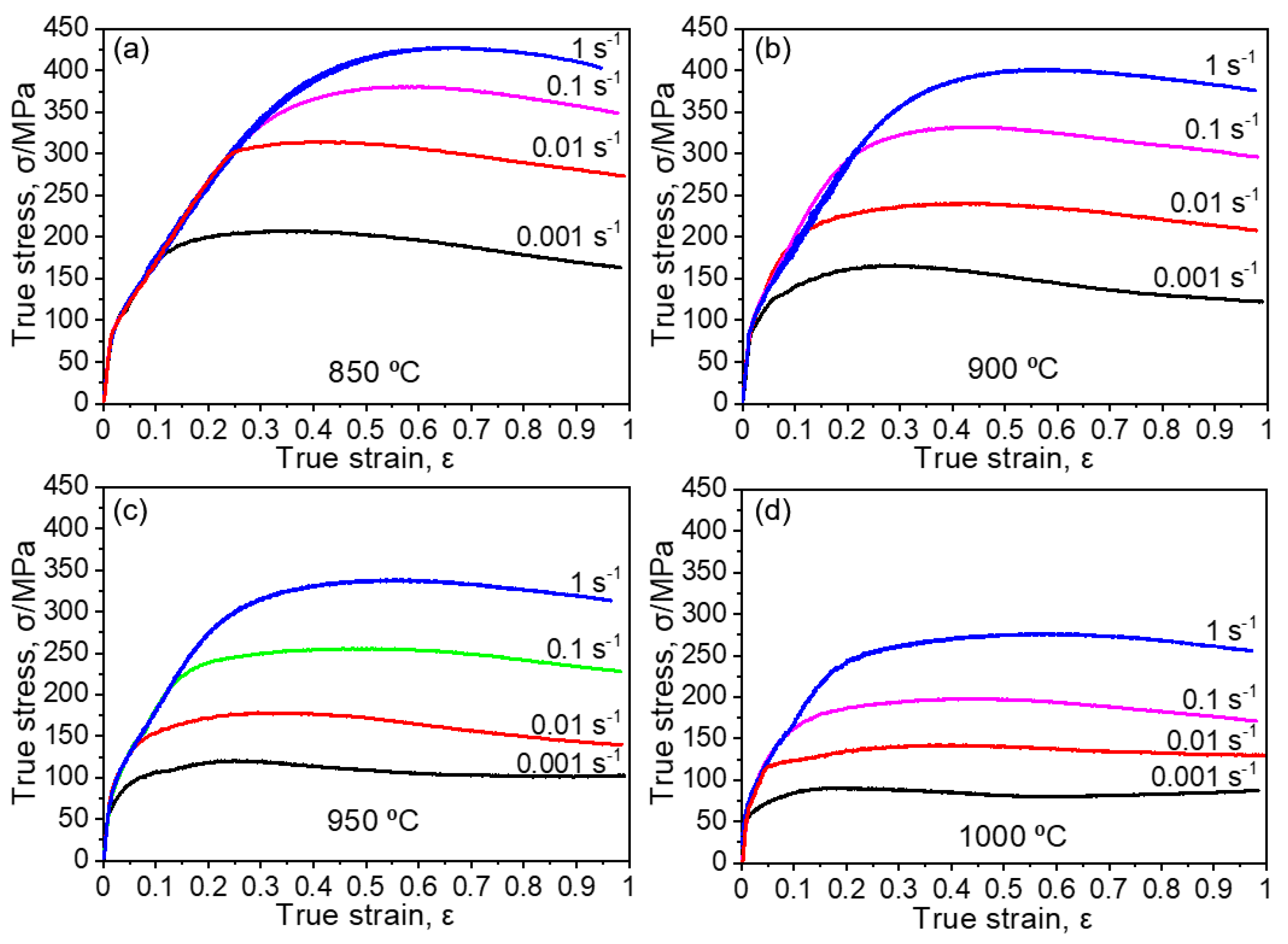
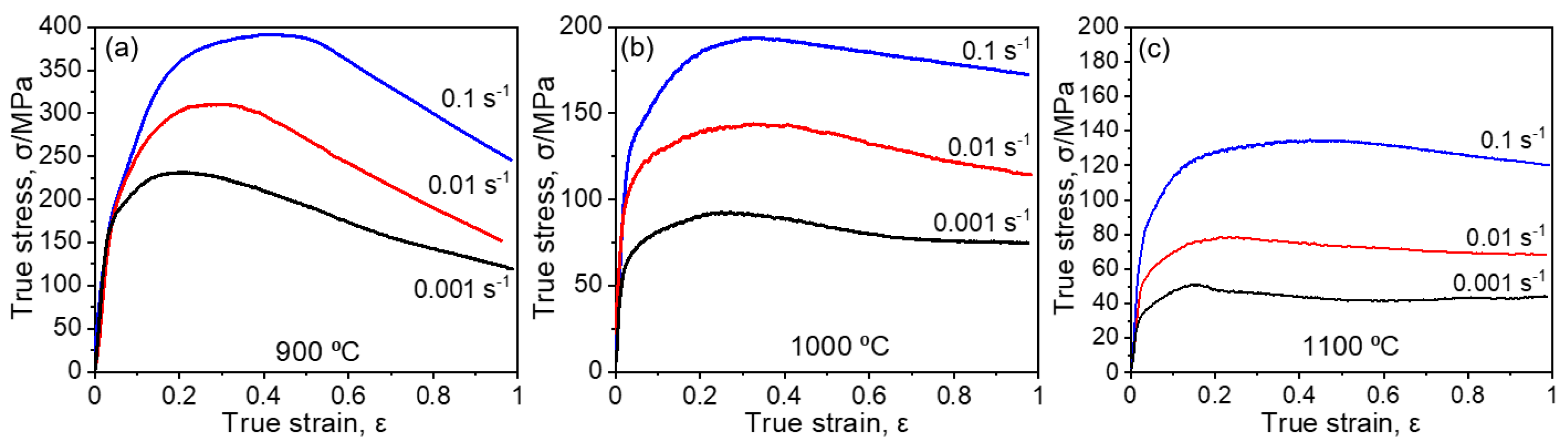



| Temperature | σmax (MPa) 1 s−1 | σmax (MPa) 0.1 s−1 | σmax (MPa) 0.01 s−1 | σmax (MPa) 0.001 s−1 |
|---|---|---|---|---|
| 850 °C | 427.6 | 380.1 | 313.9 | 206.8 |
| 900 °C | 410.4 | 331.9 | 239.8 | 165.3 |
| 950 °C | 336.2 | 254.0 | 178.2 | 119.9 |
| 1000 °C | 274.3 | 197.1 | 141.5 | 90.2 |
| Temperature | σmax (MPa) 0.1 s−1 | σmax (MPa) 0.01 s−1 | σmax (MPa) 0.001 s−1 |
|---|---|---|---|
| 900 °C | 390.6 | 310.6 | 231.5 |
| 1000 °C | 193.9 | 144.2 | 92.3 |
| 1100 °C | 134.3 | 78.1 | 51.0 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yi, H.-l.; Wei, D.; Wang, Y.; Wang, L.; Fang, M.-y.; Yang, K.; Kato, H. Hot Deformation and Dynamic Recrystallization Behavior of CoCrNi and (CoCrNi)94Ti3Al3 Medium Entropy Alloys. Metals 2020, 10, 1341. https://doi.org/10.3390/met10101341
Yi H-l, Wei D, Wang Y, Wang L, Fang M-y, Yang K, Kato H. Hot Deformation and Dynamic Recrystallization Behavior of CoCrNi and (CoCrNi)94Ti3Al3 Medium Entropy Alloys. Metals. 2020; 10(10):1341. https://doi.org/10.3390/met10101341
Chicago/Turabian StyleYi, Hai-long, Daixiu Wei, Yingchen Wang, Liqiang Wang, Ming-yang Fang, Kang Yang, and Hidemi Kato. 2020. "Hot Deformation and Dynamic Recrystallization Behavior of CoCrNi and (CoCrNi)94Ti3Al3 Medium Entropy Alloys" Metals 10, no. 10: 1341. https://doi.org/10.3390/met10101341
APA StyleYi, H.-l., Wei, D., Wang, Y., Wang, L., Fang, M.-y., Yang, K., & Kato, H. (2020). Hot Deformation and Dynamic Recrystallization Behavior of CoCrNi and (CoCrNi)94Ti3Al3 Medium Entropy Alloys. Metals, 10(10), 1341. https://doi.org/10.3390/met10101341






