Selective Recovery of Molybdenum over Rhenium from Molybdenite Flue Dust Leaching Solution Using PC88A Extractant
Abstract
:1. Introduction
2. Experimentation
2.1. Materials and Reagents
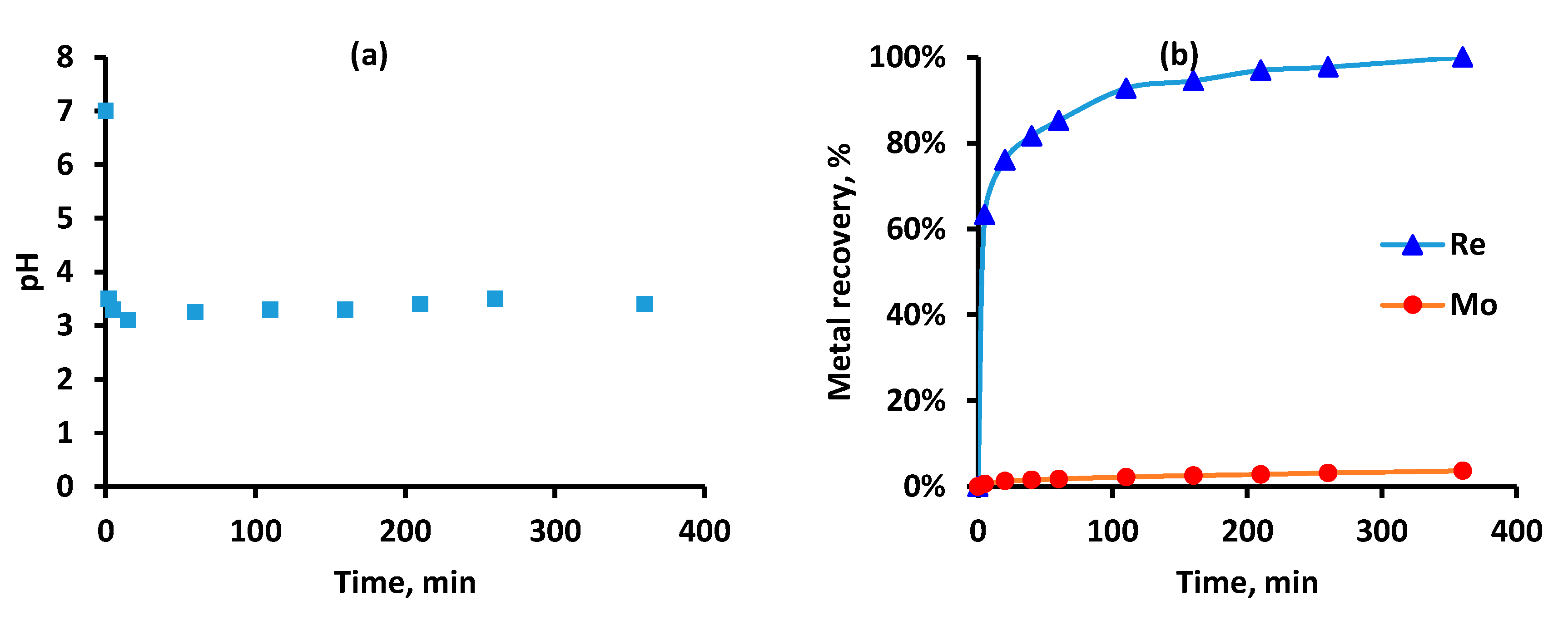
2.2. Flue Dust Leaching
2.3. Experimental Procedure
2.4. Chemical Analysis
2.5. Thermodynamic Analysis of Equilibria in Aqueous Solution
2.6. DFT Computational Details
3. Results and Discussion
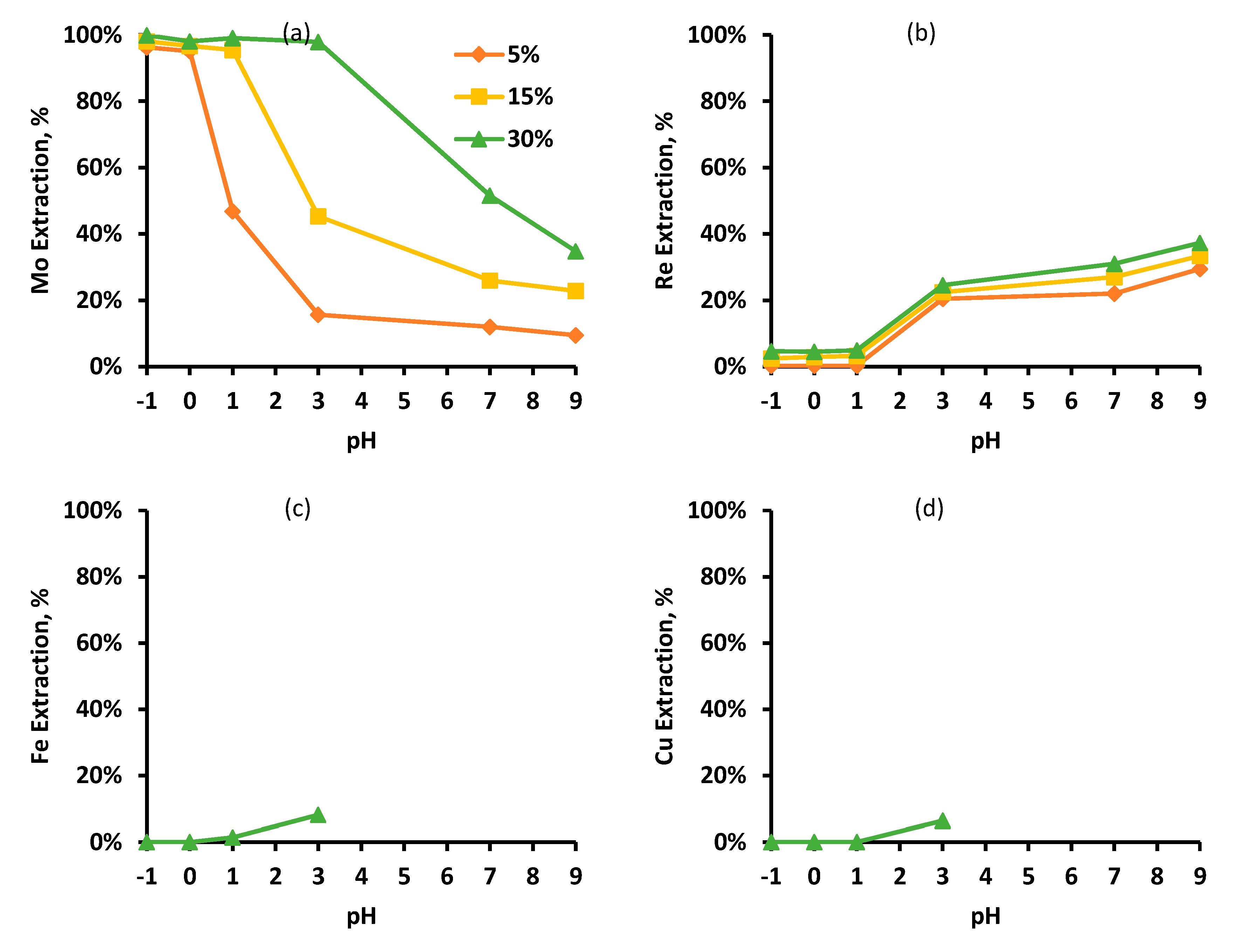
3.1. Effects of pH and Organic Phase Concentration
3.2. Effect of Extractant
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A. Appearance of the Leaching Solution

Appendix B. Details of the Thermodynamic Calculations
| Step i | Ki, mol/L | Reference |
|---|---|---|
| 1 | 1000 | [34] |
| 2 | 0.012 | [35] |

| + | |||||
| Initial state | 0 | 0 | |||
| Equilibrium state | x | x | |||
| + | |||||
| Initial state | x | 0 | x | ||
| Equilibrium state | x − y | y | x + y |
| PH | , mol/L | , mol/L | , mol/L | , mol/L |
|---|---|---|---|---|
| 2 | 2.94 × 10−8 | 0.00294 | 0.00353 | 0.00647 |
| 1 | 8.06 × 10−6 | 0.0806 | 0.00968 | 0.0903 |
| 0 | 0.000977 | 0.977 | 0.0117 | 0.989 |
| −1 | 0.0998 | 9.976 | 0.0119 | 10.088 |
| Ion | ai, Å |
|---|---|
| 4.5 | |
| 4.5 |
| pH | I, mol/L | , mol/L | , mol/L | ||
|---|---|---|---|---|---|
| 2 | 0.20288 | −0.138 | 0.00196 | −0.553 | 0.0263 |
| 1 | 0.299 | −0.155 | 0.00189 | −0.618 | 0.0226 |
| 0 | 1.096 | −0.214 | 0.00165 | −0.855 | 0.0131 |
| −1 | 10.201 | −0.285 | 0.00140 | −1.140 | 0.0068 |
References
- Habashi, F. Handbook of Extractive Metallurgy; Wiley-VCH: Weinheim/Heidelberg, Germany, 1997. [Google Scholar]
- Virolainen, S.; Laatikainen, M.; Sainio, T. Ion exchange recovery of rhenium from industrially relevant sulfate solutions: Single column separations and modeling. Hydrometallurgy 2015, 158, 74–82. [Google Scholar] [CrossRef]
- Srivastava, R.R.; Lee, J.-C.; Kim, M.-S. Complexation chemistry in liquid–liquid extraction of rhenium. J. Chem. Technol. Biotechnol. 2015, 90, 1752–1764. [Google Scholar] [CrossRef]
- Cheema, H.A.; Ilyas, S.; Masud, S.; Muhsan, M.A.; Mahmood, I.; Lee, J.-C. Selective recovery of rhenium from molybdenite flue-dust leach liquor using solvent extraction with TBP. Sep. Purif. Technol. 2018, 191, 116–121. [Google Scholar] [CrossRef]
- Nikolaychuk, P.A.; Tyurin, A.G. Utočnënnaâ diagramma Purbe dlâ molibdena. Butlerovskie Soobŝeniâ 2011, 24, 101–105. [Google Scholar]
- Khoshnevisan, A.; Yoozbashizadeh, H.; Mohammadi, M.; Abazarpoor, A.; Maarefvand, M. Separation of rhenium and molybdenum from molybdenite leach liquor by the solvent extraction method. Miner. Metall. Process. 2013, 30, 53–58. [Google Scholar] [CrossRef]
- Alamdari, E.K.; Darvishi, D.; Haghshenas, D.F.; Yousefi, N.; Sadrnezhaad, S.K. Separation of Re and Mo from roasting-dust leach-liquor using solvent extraction technique by TBP. Sep. Purif. Technol. 2012, 86, 143–148. [Google Scholar] [CrossRef]
- Cao, Z.F.; Zhong, H.; Jiang, T.; Liu, G.Y.; Wang, S. Selective electric-oxidation leaching and separation of Dexing molybdenite concentrates. Zhongguo Youse Jinshu Xuebao/Chin. J. Nonferrous Met. 2013, 23, 2290–2295. [Google Scholar]
- Zhan-fang, C.; Hong, Z.; Zhao-hui, Q. Solvent extraction of rhenium from molybdenum in alkaline solution. Hydrometallurgy 2009, 97, 153–157. [Google Scholar] [CrossRef]
- Kang, J.; Kim, Y.U.; Joo, S.H.; Yoon, H.S.; Kumar, J.R.; Park, K.H.; Shin, S.M. Behavior of Extraction, Stripping, and Separation Possibilities of Rhenium and Molybdenum from Molybdenite Roasting Dust Leaching Solution Using Amine Based Extractant Tri-Otyl-Amine (TOA). Mater. Trans. 2013, 54, 1209–1212. [Google Scholar] [CrossRef] [Green Version]
- Srivastava, R.R.; Kim, M.-S.; Lee, J.-C.; Ilyas, S. Liquid–liquid extraction of rhenium (VII) from an acidic chloride solution using Cyanex 923. Hydrometallurgy 2015, 157, 33–38. [Google Scholar] [CrossRef]
- Pathak, S.K.; Singh, S.; Mahtele, A.; Tripathi, S.C. Studies on extraction behaviour of molybdenum (VI) from acidic radioactive waste using 2 (ethylhexyl) phosphonic acids, mono 2 (ethylhexyl) ester (PC-88A)/n-dodecane. J. Radioanal. Nucl. Chem. 2010, 284, 597–603. [Google Scholar] [CrossRef]
- Wang, Y.; Jiang, K.; Zou, X.; Zhang, L.; Liu, S. Recovery of metals from molybdenite concentrate by hydrometallurgical technologies. In TT Chen Honorary Symposium on Hydrometallurgy, Electrometallurgy and Materials Characterization; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2012; pp. 315–322. [Google Scholar]
- Nguyen, T.H.; Lee, M.S. Separation of molybdenum (VI) and tungsten (VI) from sulfate solutions by solvent extraction with LIX 63 and PC 88A. Hydrometallurgy 2015, 155, 51–55. [Google Scholar] [CrossRef]
- Morís, M.A.A.; Díez, F.V.; Coca, J. Solvent extraction of molybdenum and tungsten by Alamine 336 and DEHPA in a rotating disc contactor. Sep. Purif. Technol. 1999, 17, 173–179. [Google Scholar] [CrossRef]
- Truong, H.T.; Lee, M.S. Separation of rhenium (VII), molybdenum (VI), and vanadium (V) from hydrochloric acid solution by solvent extraction with TBP. Geosyst. Eng. 2017, 20, 224–230. [Google Scholar] [CrossRef]
- Kim, H.S.; Park, J.S.; Seo, S.Y.; Tran, T.; Kim, M.J. Recovery of rhenium from a molybdenite roaster fume as high purity ammonium perrhenate. Hydrometallurgy 2015, 156, 158–164. [Google Scholar] [CrossRef]
- Xiao, C.; Zeng, L.; Xiao, L.; Zhang, G. Solvent Extraction of Molybdenum (VI) from Hydrochloric Acid Leach Solutions Using P507. Part I: Extraction and Mechanism. Solvent Extr. Ion Exch. 2017, 156, 130–144. [Google Scholar] [CrossRef]
- Entezari, A.; Karamoozian, M.; Eskandari Nasab, M. Investigation on selective rhenium leaching from molybdenite roasting flue dusts. J. Min. Environ. 2013, 4, 77–82. [Google Scholar]
- Entezari-Zarandi, A.; Larachi, F. Selective dissolution of rare-earth element carbonates in deep eutectic solvents. J. Rare Earths 2019, 37, 528–533. [Google Scholar] [CrossRef]
- Keshavarz Alamdari, E. Selective Leaching-Recovery of Re and Mo from Out-Gas Dust of Molybdenite Roasting Furnace. Trans. Indian Inst. Met. 2017, 70, 1995–1999. [Google Scholar] [CrossRef]
- Debye, P.; Hückel, E. Zur Theorie der Elektrolyte. I. Gefrierpunktserniedrigung und verwandte Erscheinungen. Phys. Z 1923, 24, 185–206. [Google Scholar]
- Kielland, J. Individual activity coefficients of ions in aqueous solutions. J. Am. Chem. Soc. 1937, 59, 1675–1678. [Google Scholar] [CrossRef]
- Coolidge, W. Dielektrische Untersuchungen und elektrische Drahtwellen. Ann. Phys. 1899, 305, 125–166. [Google Scholar] [CrossRef] [Green Version]
- Nikolaychuk, P. Das revidierte pourbaix-diagramm für schwefel. In Materialien Zum Wissenschaftlichen Seminar der Stipendiaten der Programme „Mikhail Lomonosov“ und „Immanuel Kant“; Deutscher Akademischer Austausch Dienst und Ministerium für Bildung und Wissenschaft der RF: Moscow, Russia, 2015; pp. 72–76. [Google Scholar]
- Wagman, D.D.; Evans, W.H.; Parker, V.B.; Schumm, R.H.; Halow, I. The NBS tables of chemical thermodynamic properties. Selected values for inorganic and C1 and C2 organic substances in SI units, National Standard Reference Data System. J. Phys. Chem. Ref. Data 1982, 11, 37–38. [Google Scholar]
- Azizi, D.; Larachi, F. Behavior of bifunctional phosphonium-based ionic liquids in solvent extraction of rare earth elements-quantum chemical study. J. Mol. Liq. 2018, 263, 96–108. [Google Scholar] [CrossRef]
- Palant, A.; Iatsenko, N.; Petrova, V. Solvent extraction of molybdenum (VI) by diisododecylamine from sulphuric acid solution. Hydrometallurgy 1998, 48, 83–90. [Google Scholar] [CrossRef]
- Loewenschuss, A.; Shamir, J.; Ardon, M. Vibrational spectra of binuclear molybdenum sulfate complexes of high bond order. Inorg. Chem. 1976, 15, 238–241. [Google Scholar] [CrossRef]
- Alamdari, E.K.; Sadrnezhaad, S.K. Thermodynamics of extraction of from aqueous sulfuric acid media with TBP dissolved in kerosene. Hydrometallurgy 2000, 55, 327–341. [Google Scholar] [CrossRef]
- Kholmogorov, A.G.; Kononova, O.N.; Panchenko, O.N. A Review of the Use of Ion Exchange for Molybdenum Recovery in Russia. Can. Metall. Q. 2004, 43, 297–304. [Google Scholar] [CrossRef]
- Shi, Q.; Zhang, Y.; Huang, J.; Liu, T.; Liu, H.; Wang, L. Synergistic solvent extraction of vanadium from leaching solution of stone coal using D2EHPA and PC88A. Sep. Purif. Technol. 2017, 181, 1–7. [Google Scholar] [CrossRef]
- Cheng, C.Y.; Barnard, K.R.; Zhang, W.; Robinson, D.J. Synergistic solvent extraction of nickel and cobalt: A review of recent developments. Solvent Extr. Ion Exch. 2011, 29, 719–754. [Google Scholar] [CrossRef]
- Sue, K.; Uchids, M.; Adschiri, T.; Arai, K. Determination of sulfuric acid first dissociation constants to 400 °C and 32 MPa by potentiometric pH measurements. J. Supercrit. Fluids 2004, 31, 295–299. [Google Scholar] [CrossRef]
- Wu, Y.C.; Feng, D. The second dissociation constant of sulfuric acid at various temperatures by the conductometric method. J. Solut. Chem. 1995, 24, 133–144. [Google Scholar] [CrossRef]




| Extractant/Diluent | Acidity | Selectivity | Ref. |
|---|---|---|---|
| N235 + TBP/kerosene | pH = 9 | Re over Mo 15 g/L Mo + 0.1 g/L Re 97.6%: 1.6% | [9] |
| N235/kerosene | pH = 0 | Re over Mo | [10] |
| Cyanex 923/kerosene | pH = 0 HCl | Re | [11] |
| N235 + isooctanol/kerosene | pH = 0 HNO3 | Re over Mo | [13] |
| LIX 63/kerosene | pH = 2–6 H2SO4 | Mo over W | [14] |
| D2EHPA/kerosene | pH = 3–4 H2SO4 | Mo over W | [15] |
| TBP/kerosene | pH = 2 pH = 0 H2SO4 | Mo over ReRe over Mo | [7] |
| TBP/kerosene | pH = 0 <3.0 M HCl | Re over Mo and V Re and Mo over V | [16] |
| TBP/kerosene | pH = 1.5 pH = −0.3 H2SO4 | Mo over Re Re over Mo | [1] |
| Alamine 304-1/Anysol-150 | pH = 2–3 H2SO4 | Re over Mo 260–280 mg/L Re + 80–90 mg/L Mo | [17] |
| PC88A/n-dodecane | 0.1–4.0 M HNO3 | Mo 0.01 mol/L | [12] |
| PC88A/Sulfonated kerosene | pH = −0.2 ~ 0.5 HCl | Mo | [18] |
| Component | As | Ca | Cu | Fe | Mg | Mo | Na | Pb | Re | S | Se | Zn |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Content | 785 | 3670 | 3450 | 1720 | 775 | 36.6% | 2380 | 1260 | 3980 | 25.2% | 6650 | 242 |
| Element | Mo | Re | Cu | Se | Fe |
|---|---|---|---|---|---|
| Concentration (ppm) | 9150 | 455 | 2.5 | 6.2 | 2000 |
| Extractant | pH | Org. Conc. | Mo Recovery (%) | Re Recovery (%) |
|---|---|---|---|---|
| PC88A | 1 | 15 | 95.48 | 3.19 |
| D2EHPA | 1 | 15 | 81.51 | 15.35 |
| PC88A | 3 | 15 | 45.32 | 22.45 |
| D2EHPA | 3 | 15 | 57.23 | 4.3 |
| PC88A | 7 | 15 | 25.94 | 27 |
| D2EHPA | 7 | 15 | 90.08 | 14.1 |
| Extractants | Active Group | Charge (e) | Average Charge (e) |
|---|---|---|---|
| D2EHPA | P = O | −0.551 | −0.552 |
| P − O | −0.553 | ||
| PC88A | P = O | −0.54 | −0.545 |
| P − O | −0.53 |
| Species | Charge e (Re or Mo) | Charge e (Re or Mo) | Changes (e) | Water Bond Length (Å) |
|---|---|---|---|---|
| Nonsolvated | Solvated | |||
| 0.83 | 0.61 | 0.22 | 2.3 | |
| 0.89 | 0.6 | 0.29 | 2.3 | |
| 0.74 | 0.4 | 0.34 | 2.2 |
| Species | PC88A | D2EHPA | ||
|---|---|---|---|---|
| Organic | Aqueous | Organic | Aqueous | |
| −178.1 | −138.1 | −321.3 | −237.5 | |
| −411.4 | −321.1 | −372.1 | −221.8 | |
| −189.1 | −155.3 | −195.3 | −157.1 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Entezari-Zarandi, A.; Azizi, D.; Nikolaychuk, P.A.; Larachi, F.; Pasquier, L.-C. Selective Recovery of Molybdenum over Rhenium from Molybdenite Flue Dust Leaching Solution Using PC88A Extractant. Metals 2020, 10, 1423. https://doi.org/10.3390/met10111423
Entezari-Zarandi A, Azizi D, Nikolaychuk PA, Larachi F, Pasquier L-C. Selective Recovery of Molybdenum over Rhenium from Molybdenite Flue Dust Leaching Solution Using PC88A Extractant. Metals. 2020; 10(11):1423. https://doi.org/10.3390/met10111423
Chicago/Turabian StyleEntezari-Zarandi, Ali, Dariush Azizi, Pavel Anatolyevich Nikolaychuk, Faïçal Larachi, and Louis-César Pasquier. 2020. "Selective Recovery of Molybdenum over Rhenium from Molybdenite Flue Dust Leaching Solution Using PC88A Extractant" Metals 10, no. 11: 1423. https://doi.org/10.3390/met10111423
APA StyleEntezari-Zarandi, A., Azizi, D., Nikolaychuk, P. A., Larachi, F., & Pasquier, L.-C. (2020). Selective Recovery of Molybdenum over Rhenium from Molybdenite Flue Dust Leaching Solution Using PC88A Extractant. Metals, 10(11), 1423. https://doi.org/10.3390/met10111423







