The Effect of Preliminary Thermomechanical Processing on the Kinetics of Localized Plasticity Autowaves in Trip Steel
Abstract
:1. Introduction
2. Materials and Methods
3. Results
3.1. Phase and Structure Evolution in the Material Depending on the Amount of Rolling Reduction
3.2. Nucleation and Propagation of Deformation Fronts in Tensile Trip Steel Specimens
4. Discussion
4.1. The Nature of Localized Deformation Fronts in TRIP Steel
4.2. The Autowave Nature of the Phase Transformation Fronts
5. Conclusions
- Preliminary heat treatment and warm rolling of chromium-nickel-molybdenum TRIP steel to 40–63% reduction significantly increases the stress of the onset of strain-induced phase transformation as well as changes the loading curve stages. After austenitization and rolling, the specimens demonstrate an S-shaped loading curve with a pseudo-plateau. Annealing leads to a decrease in strength and to a parabolic loading curve with a gradually decreasing hardening coefficient.
- The martensitic transformation in specimens with a pseudo-plateau begins with the nucleation and propagation of transformation bands similar to Lüders bands in materials with a dislocation deformation mechanism, whose moving boundaries (fronts) are switching autowaves of localized plasticity.
- Further plastic flow after annihilation of switching autowaves can occur by martensitic transformation in the form of propagating excitation autowaves, which are mobile PLC band fronts. Regardless of the presence of visible serrations in the stress-strain curve, the PLC band fronts repeatedly pass through the loaded specimen until the strain-induced phase transformation is fully completed.
- The interconversion of autowave modes was observed for the first time. It was found that the sources of excitation autowaves in the material are the nucleation or annihilation sites of switching autowaves. The sites divide the material into separate regions in which the strain-induced phase transformation processes proceed independently.
Author Contributions
Funding
Conflicts of Interest
References
- Fahr, D. Stress- and strain-induced formation of martensite and its effects on strength and ductility of metastable austenitic stainless steels. Metall. Trans. 1971, 2, 1883–1892. [Google Scholar] [CrossRef]
- Mumtaz, K.; Takahashi, S.; Echigoya, J.; Kamada, Y.; Zhang, F.; Kikuchi, H.; Ara, K.; Sato, M. Magnetic measurements of martensitic transformation in austenitic stainless steel after room temperature rolling. J. Mater. Sci. 2004, 39, 85–97. [Google Scholar] [CrossRef]
- Auricchio, F.; Bonetti, E.; Scalet, G.; Ubertini, F. Theoretical and numerical modeling of shape memory alloys accounting for multiple phase transformations and martensite reorientation. Int. J. Plast. 2014, 59, 30–54. [Google Scholar] [CrossRef]
- Luo, H.W.; Dong, H.; Huang, M.X. Effect of intercritical annealing on the Lüders strains of medium Mn transformation-induced plasticity steels. Mater. Des. 2015, 83, 42–48. [Google Scholar] [CrossRef]
- Blondé, R.; Jimenez-Melero, E.; Zhao, L.; Wright, J.P.; Brück, E.; van der Zwaag, S.; van Dijk, N.H. High-energy X-ray diffraction study on the temperature-dependent mechanical stability of retained austenite in low-alloyed TRIP steels. Acta Mater. 2012, 60, 565–577. [Google Scholar] [CrossRef]
- Scheil, E. Uber die Umwandlung des Austenits in Martensit in Eisen-Nickellegierungen unter Belastung. Z. Anorg. Allg. Chem. 1932, 207, 21–40. [Google Scholar] [CrossRef]
- Wechsler, M.S.; Lieberman, D.; Read, T. On the theory of the formation of martensite. Trans. AIME 1953, 197, 1503–1515. [Google Scholar]
- Bowles, J.; Mackenzie, J. The crystallography of martensite transformations I. Acta Metall. 1954, 2, 129–137. [Google Scholar] [CrossRef]
- Das, A.; Tarafder, S. Experimental investigation on martensitic transformation and fracture morphologies of austenitic stainless steel. Int. J. Plast. 2009, 25, 2222–2247. [Google Scholar] [CrossRef]
- De Meyer, M.; Vanderschueren, D.; De Cooman, B.C. The Influence of the Substitution of Si by Al on the Properties of Cold Rolled C-Mn-Si TRIP Steels. ISIJ Int. 1999, 39, 813–822. [Google Scholar] [CrossRef]
- Callahan, M.; Hubert, O.; Hild, F.; Perlade, A.; Schmitt, J.-H. Coincidence of strain-induced TRIP and propagative PLC bands in Medium Mn steels. Mater. Sci. Eng. 2017, 704, 391–400. [Google Scholar] [CrossRef] [Green Version]
- Wang, X.G.; Liu, C.H.; He, B.B.; Jiang, C.; Huang, M.X. Microscopic strain partitioning in Lüders band of an ultrafine-grained medium Mn steel. Mater. Sci. Eng. A. 2019, 761, 138050. [Google Scholar] [CrossRef]
- Koppenaal, T.J.; Gold, E. The effect of prior deformation on the strength and annealing of reverted austenite. Metall. Trans. B 1972, 3, 2965–2972. [Google Scholar] [CrossRef]
- Wang, Y.; Tomota, Y.; Ohmura, T.; Morooka, S.; Gong, W.; Harjoc, S. Real time observation of martensite transformation for a 0.4 C low alloyed steel by neutron diffraction. Acta Mater. 2020, 184, 30–40. [Google Scholar] [CrossRef]
- Van Dijk, N.H.; Butt, A.M.; Zhao, L.; Sietsma, J.; Offerman, S.E.; Wright, J.P.; Van Der Zwaag, S. Thermal stability of retained austenite in TRIP steels studied by synchrotron X-ray diffraction during cooling. Acta Mater. 2005, 53, 5439–5447. [Google Scholar] [CrossRef]
- Allain, S.Y.P.; Geandier, G.; Hell, J.-C.; Soler, M.; Danoix, F.; Gouné, M. Effects of Q&P processing conditions on austenite carbon enrichment studied by in situ high-energy X-ray diffraction experiments. Metals 2017, 7, 232. [Google Scholar] [CrossRef] [Green Version]
- Tan, X.; Ponge, D.; Lu, W.; Xu, Y.; He, H.; Yan, J.; Wu, D.; Raabe, D. Joint investigation of strain partitioning and chemical partitioning in ferrite-containing TRIP-assisted steels. Acta Mater. 2020, 186, 374–388. [Google Scholar] [CrossRef]
- Tasan, C.; Hoefnagels, J.; Geers, M. Microstructural banding effects clarified through micrographic digital image correlation. Scr. Mater. 2010, 62, 835–838. [Google Scholar] [CrossRef]
- Dutta, A.; Ponge, D.; Sandlöbes, S.; Raabe, D. Strain partitioning and strain localization in medium manganese steels measured by in situ microscopic digital image correlation. Materialia 2019, 5, 100252. [Google Scholar] [CrossRef]
- Egorov, S.A.; Volkov, A.E. Dependence of the enthalpy of the direct martensitic transformation in titanium nickelide on the stress. Tech. Phys. 2017, 62, 230–236. [Google Scholar] [CrossRef]
- Kim, K.; Daly, S. The effect of texture on stress-induced martensite formation in nickel–titanium. Smart Mater. Struct. 2013, 22, 075012. [Google Scholar] [CrossRef]
- Panin, V.E.; Grinyaev, Y.V.; Elsukova, T.F.; Ivanchin, A.G. Structural levels of deformation in solids. Sov. Phys. J. 1982, 25, 479–497. [Google Scholar] [CrossRef]
- Vladimirov, V.I. Collective effects in ensembles of defects. In Problems of the Theory of Defects in Crystals; Vonsovskii, S.V., Ed.; Nauka: Leningrad, Russia, 1987; pp. 43–57. [Google Scholar]
- Aifantis, E.C. Pattern formation in plasticity. Int. J. Eng. Sci. 1995, 33, 2161–2178. [Google Scholar] [CrossRef]
- Panin, V.E. Dynamic gauge theory of waves in elastoplastic media. In Physical Mesomechanics of Heterogeneous Media and Computer-Aided Design of Materials; Panin, V.E., Ed.; Cambridge International Science Publishing Ltd.: Great Abington, Cambridge, UK, 1998; pp. 97–117. [Google Scholar]
- Zbib, H.M.; de la Rubia, T.D. A multiscale model of plasticity. Int. J. Plasticity 2002, 18, 1133–1163. [Google Scholar] [CrossRef]
- Ohashi, T.; Kawamukai, M.; Zbib, H.M. A multiscale approach for modeling scale-dependent yield stress in polycrystalline metals. Int. J. Plasticity 2007, 23, 897–914. [Google Scholar] [CrossRef]
- Huvier, C.; Conforto, E.; El Alami, H.; Delafosse, D.; Feaugas, X. Some correlations between slip band and dislocation patterns. IOP Conf. Ser. Mater. Sci. Eng. 2009, 3, 012012–012018. [Google Scholar] [CrossRef]
- Seeger, A.; Frank, W. Structure formation by dissipative processes in crystals with high defect densities. In Non-Linear Wave Processes in Excitable Media; Kubin, L.P., Martin, G., Eds.; Trans. Tech. Publ.: New York, NY, USA, 1987; pp. 125–138. [Google Scholar] [CrossRef]
- Nicolis, G.; Prigogine, I. Exploring Complexity; Freeman: New York, NY, USA, 1989; p. 313. [Google Scholar]
- Davydov, V.A.; Zykov, V.S.; Mikhailov, A.S. Kinematics of autowave structures in excitable media. Phys.-Uspekhi 1991, 34, 665–684. [Google Scholar] [CrossRef]
- Zaiser, M.; Aifantis, E.C. Randomness and slip avalanches in gradient plasticity. Int. J. Plasticity 2006, 22, 1432–1455. [Google Scholar] [CrossRef]
- Lebyodkin, M.A.; Kobelev, N.P.; Bougherira, Y.; Entemeyer, D.; Fressengeas, C.; Gornakov, V.S.; Lebedkina, T.A.; Shashkov, I.V. On similarity of plastic flow processes during smooth and jerky flow: Statistical analysis. Acta Mater. 2012, 60, 3729–3740. [Google Scholar] [CrossRef]
- Zuev, L.B. and Barannikova, S.A. Autowave Physics of Material Plasticity. Crystals 2019, 9, 458. [Google Scholar] [CrossRef] [Green Version]
- Zuev, L.B. Using a crystal as a universal generator of localized plastic flow autowaves. Bull. Russ. Acad. Sci. Phys. 2014, 78, 957–964. [Google Scholar] [CrossRef]
- Zuev, L.B.; Danilov, V.I.; Barannikova, S.A.; Gorbatenko, V.V. Autowave model of localized plastic flow of solids. Phys. Wave Phenom. 2009, 17, 67–75. [Google Scholar] [CrossRef]
- Gorbatenko, V.V.; Danilov, V.I.; Zuev, L.B. Plastic flow instability: Chernov–Lüders bands and the Portevin—Le Chatelier effect. Tech. Phys. 2017, 62, 395–400. [Google Scholar] [CrossRef]
- Sutton, M.A. Digital image correlation for shape and deformation measurements. In Springer Handbook of Experimental Solid Mechanics; Sharpe, W.N., Jr., Ed.; Springer: Boston, MA, USA, 2008; pp. 565–600. [Google Scholar] [CrossRef]
- Terent’ev, V.F.; Slizov, A.K.; Sirotinkin, V.P.; Prosvirnin, D.V.; Kobeleva, L.I.; Eliseev, E.A.; Rybal’chenko, O.V.; Ashmarin, A.A. Effect of the removal of the surface layer of a TRIP steel sheet on its phase composition after static tension at various strain rates. Russ. Metall. 2016, 1, 34–38. [Google Scholar] [CrossRef]
- Terent’ev, V.F.; Ashmarin, A.A.; Titov, D.D.; Blinov, V.M.; Seval’neva, T.G.; Blinova, E.N.; Slizov, A.K. Mechanical properties and structure of a VNS9-sh steel as functions of the tempering temperature. Russ. Metall. 2019, 4, 403–408. [Google Scholar] [CrossRef]
- Danilov, V.I.; Gorbatenko, V.V.; Zuev, L.B.; Orlova, D.V. Kinetics and morphology of Lüders deformation in specimens with homogeneous structure and with a weld joint. J. Mater. Sci. Eng. A 2018, 714, 160–166. [Google Scholar] [CrossRef]
- Karaman, I.; Sehitoglu, H.; Maier, H.J.; Chumlyakov, Y.I. Competing mechanisms and modeling of deformation in austenitic stainless steel single crystals with and without nitrogen. Acta Met. 2001, 49, 3919–3933. [Google Scholar] [CrossRef]
- Mikhailov, A.S.; Loskutov, A.Y. Foundations of Synergetics II; Springer: Berlin/Heidelberg, Germany, 1991; p. 212. [Google Scholar] [CrossRef]
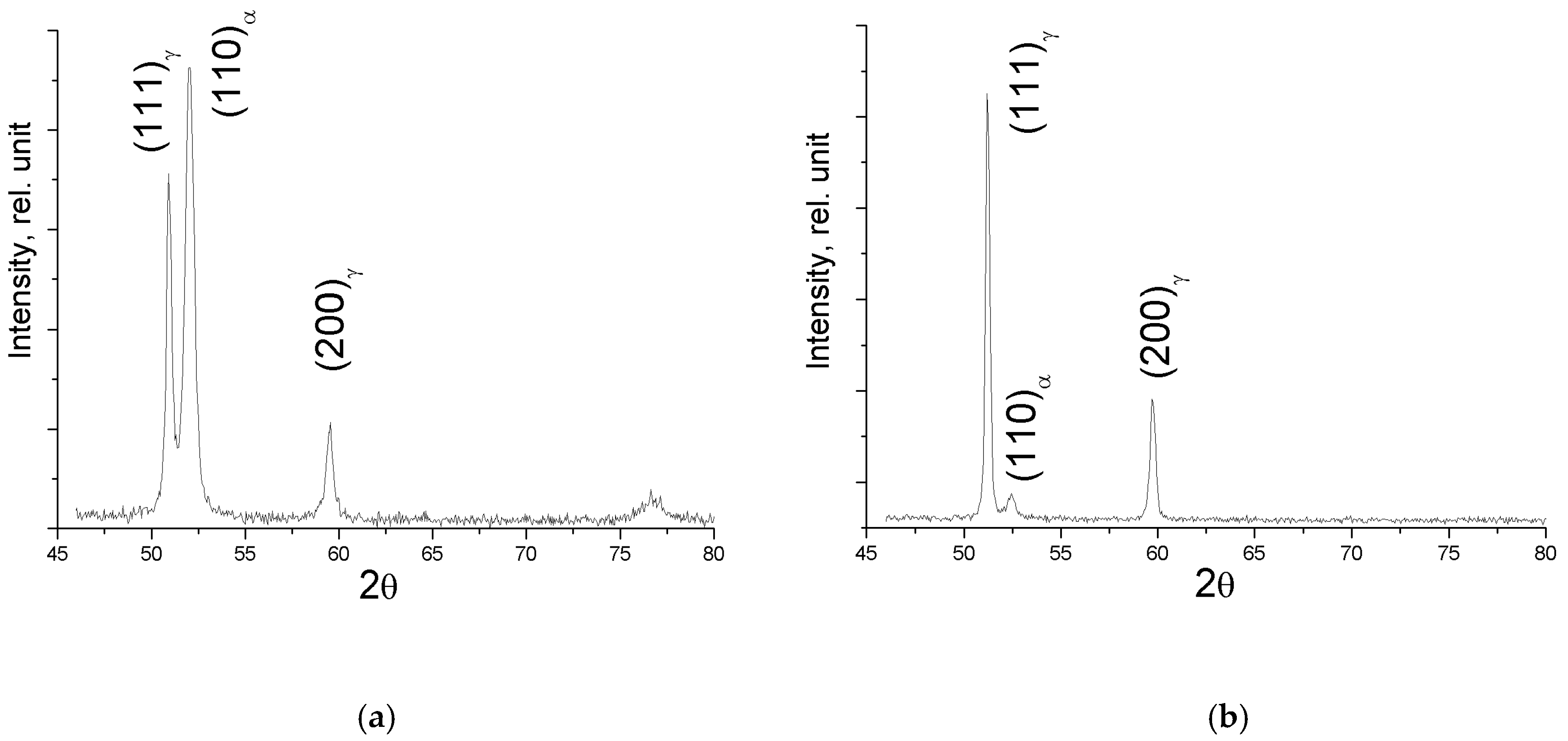
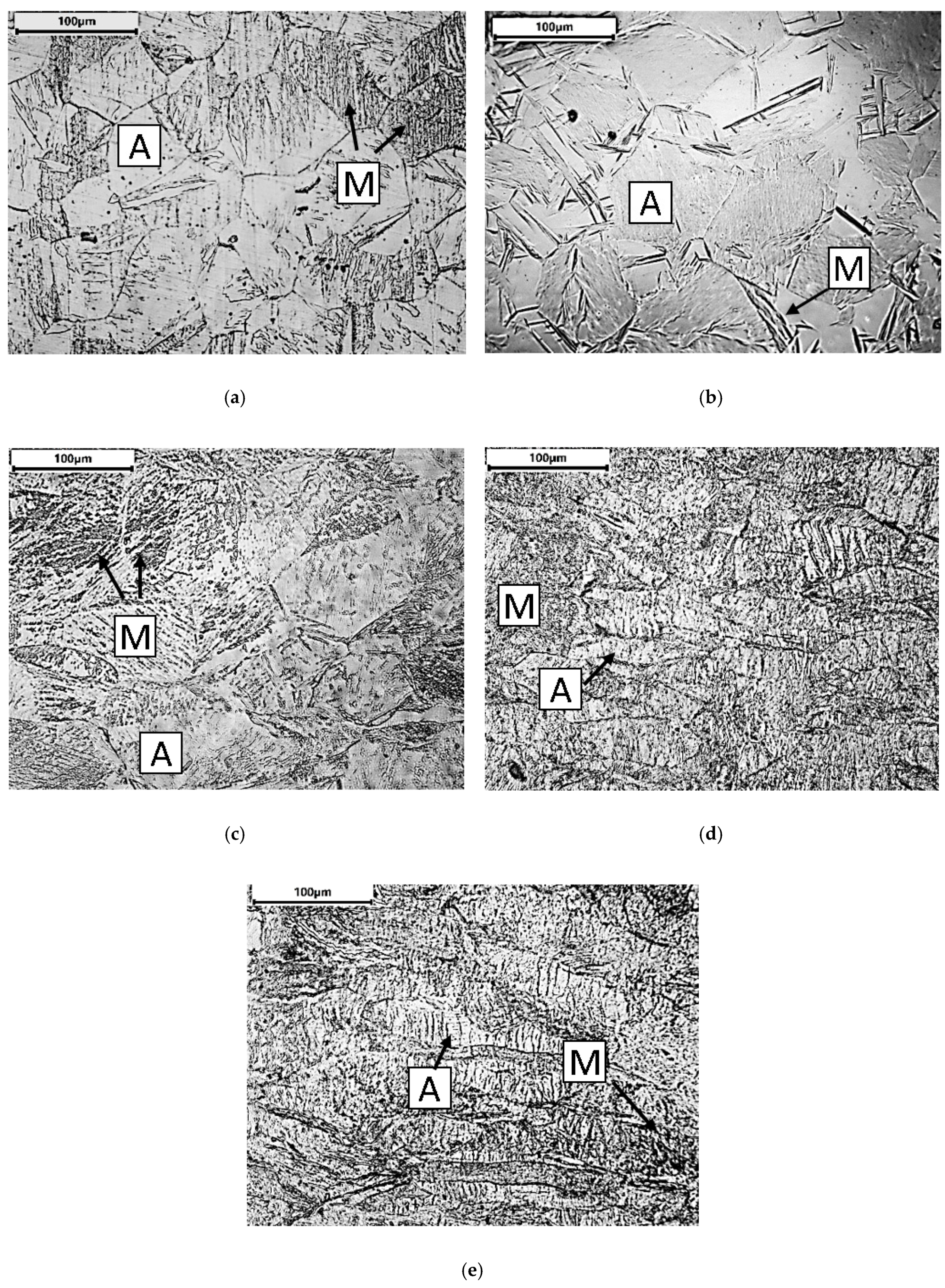

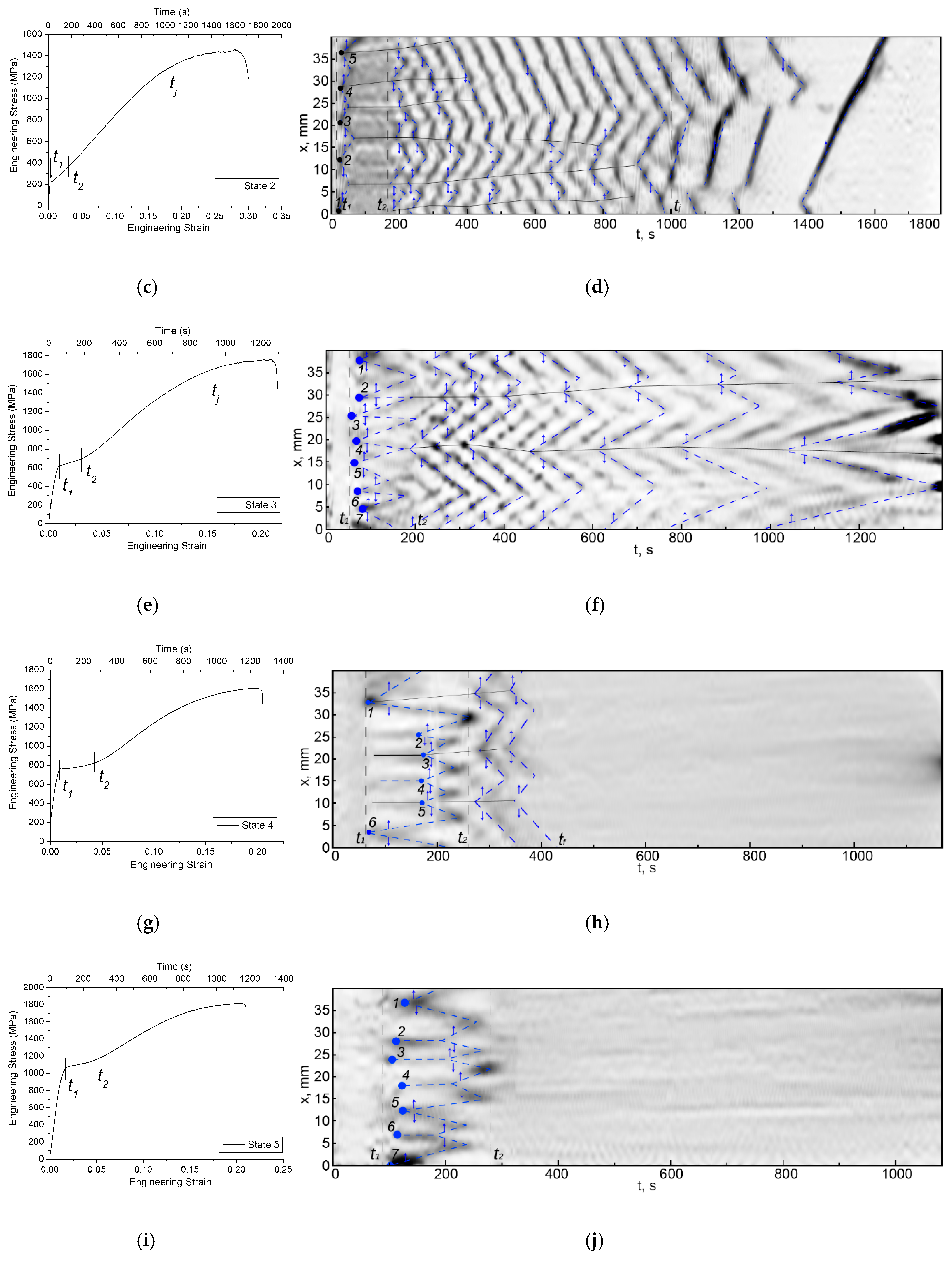
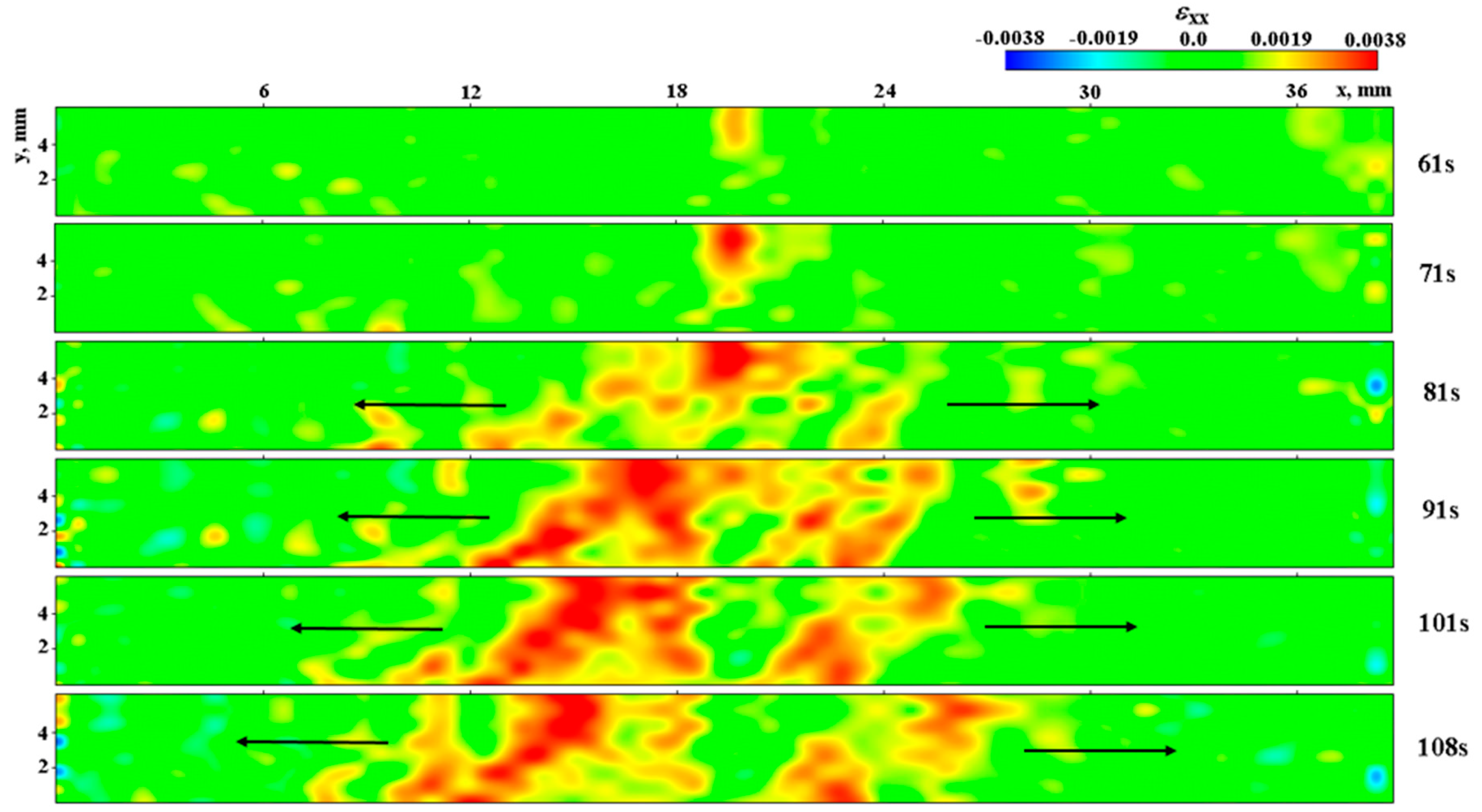
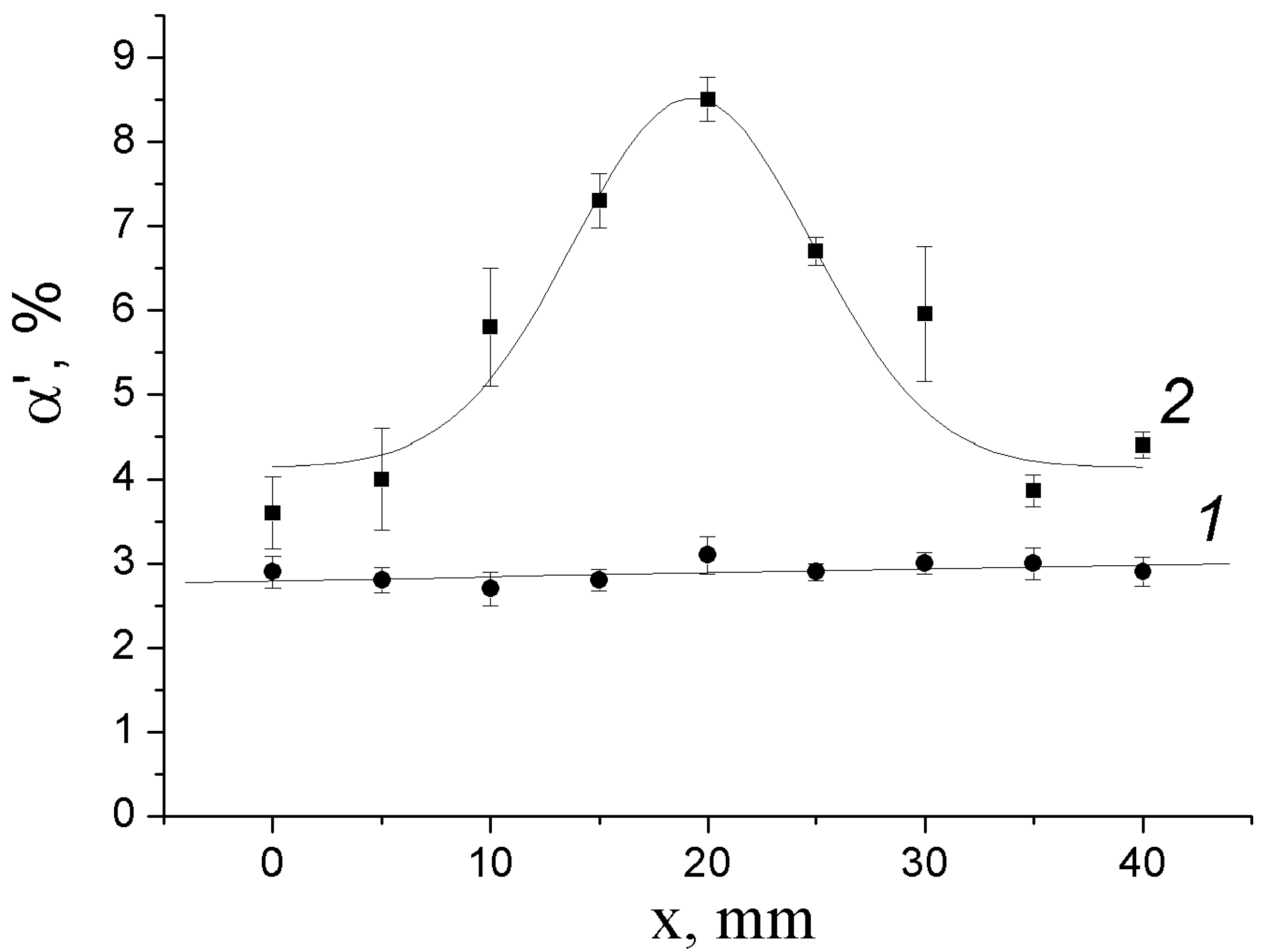
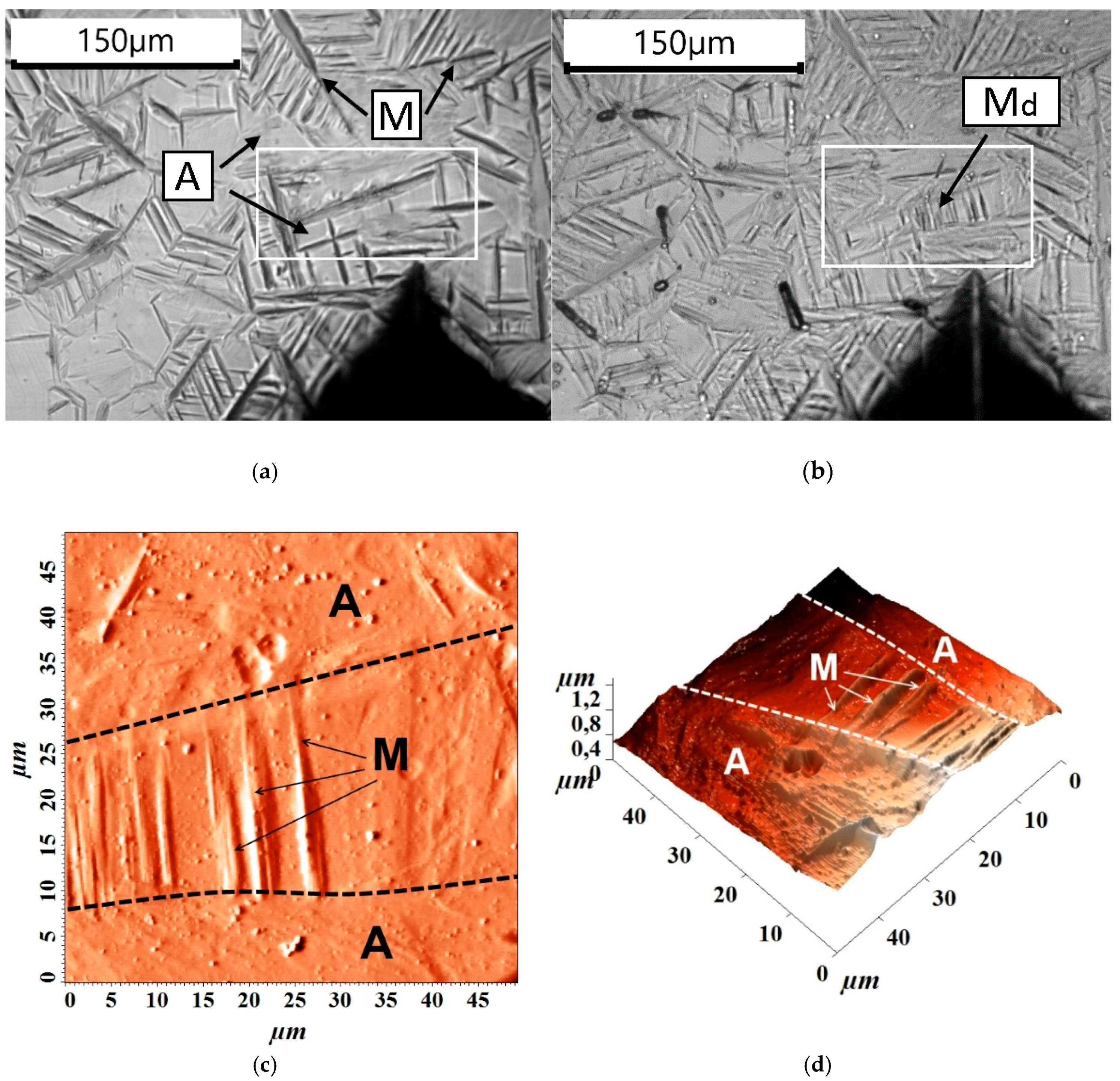
| Elements | C | Cr | Ni | Mo | N | Mn | Si | S | P |
|---|---|---|---|---|---|---|---|---|---|
| wt. % | 0.25 | 14.5–16.0 | 4.8–5.8 | 2.7–3.2 | 0.03–0.07 | ≤1 | ≤0.6 | ≤0.01 | ≤0.015 |
| Thermomechanical Processing Regimes | State | ||||
|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 5 | |
| Annealing, T = 875 K, τ = 1 h, furnace cooling | + | − | − | − | − |
| Austenitization (quenching), T = 1400 K, τ = 1 h, water cooling | − | + | + | + | + |
| Multi-pass warm rolling, T = 620 K | − | − | + | + | + |
| Reduction in rolling, % | − | − | 40 | 51 | 63 |
| Thickness, mm | 1 | 1 | 0.6 | 0.5 | 0.47 |
| State | 1 | 2 | 3 | 4 | 5 |
|---|---|---|---|---|---|
| Martensite content,% | ≤4 | ≤4 | 30 ± 1 | 17 ± 0.5 | 15 ± 0.4 |
| Austenite content,% | ≥96 | ≥96 | 70 ± 1 | 83 ± 0.5 | 85 ± 0.4 |
| State | Microhardness Hµ, MPa | Mean Grain Size, μm |
|---|---|---|
| 1 | 2370 ± 220 | 97 ± 29 |
| 2 | 2140 ± 260 | 120 ± 35 |
| 3 | 4580 ± 90 | 117 ± 39 |
| 4 | 5140 ± 140 | 141 ± 47 |
| 5 | 5450 ± 180 | 163 ± 45 |
| State | Yield Point, MPa | Tensile Strength, MPa | Strain at Break, % | Pseudo-Plateau | Parabolic Hardening | JERKY FLOW |
|---|---|---|---|---|---|---|
| 1 | 290 ± 8 | 1396 ± 2 | 40 ± 2 | - | 0.002 ≤ ε ≤ 0.42 | 0.29 ≤ ε ≤ 0.42 |
| 2 | 280 ± 40 | 1457 ± 10 | 33 ± 3 | 0.003 ≤ ε ≤ 0.03 | 0.03 ≤ ε ≤ 0.29 | 0.17 ≤ ε ≤ 0.29 |
| 3 | 598 ± 32 | 1766 ± 24 | 19 ± 1 | 0.009 ≤ ε ≤ 0.029 | 0.029 ≤ ε ≤ 0.20 | 0.14 ≤ ε ≤ 0.20 |
| 4 | 862 ± 60 | 1747 ± 56 | 20 ± 2 | 0.01 ≤ ε ≤ 0.043 | 0.043 ≤ ε ≤ 0.19 | - |
| 5 | 1060 ± 61 | 1752 ± 32 | 19 ± 1 | 0.015 ≤ ε ≤ 0.049 | 0.049 ≤ ε ≤ 0.19 | - |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Orlova, D.V.; Danilov, V.I.; Gorbatenko, V.V.; Danilova, L.V.; Shlyakhova, G.V.; Zuev, L.B. The Effect of Preliminary Thermomechanical Processing on the Kinetics of Localized Plasticity Autowaves in Trip Steel. Metals 2020, 10, 1494. https://doi.org/10.3390/met10111494
Orlova DV, Danilov VI, Gorbatenko VV, Danilova LV, Shlyakhova GV, Zuev LB. The Effect of Preliminary Thermomechanical Processing on the Kinetics of Localized Plasticity Autowaves in Trip Steel. Metals. 2020; 10(11):1494. https://doi.org/10.3390/met10111494
Chicago/Turabian StyleOrlova, Dina V., Vladimir I. Danilov, Vadim V. Gorbatenko, Lidiya V. Danilova, Galina V. Shlyakhova, and Lev B. Zuev. 2020. "The Effect of Preliminary Thermomechanical Processing on the Kinetics of Localized Plasticity Autowaves in Trip Steel" Metals 10, no. 11: 1494. https://doi.org/10.3390/met10111494
APA StyleOrlova, D. V., Danilov, V. I., Gorbatenko, V. V., Danilova, L. V., Shlyakhova, G. V., & Zuev, L. B. (2020). The Effect of Preliminary Thermomechanical Processing on the Kinetics of Localized Plasticity Autowaves in Trip Steel. Metals, 10(11), 1494. https://doi.org/10.3390/met10111494







