Numerical Investigation of Blast Furnace Operation with Scrap Charging
Abstract
1. Introduction
2. Simulation Conditions
3. Model Development
4. Results and Discussion
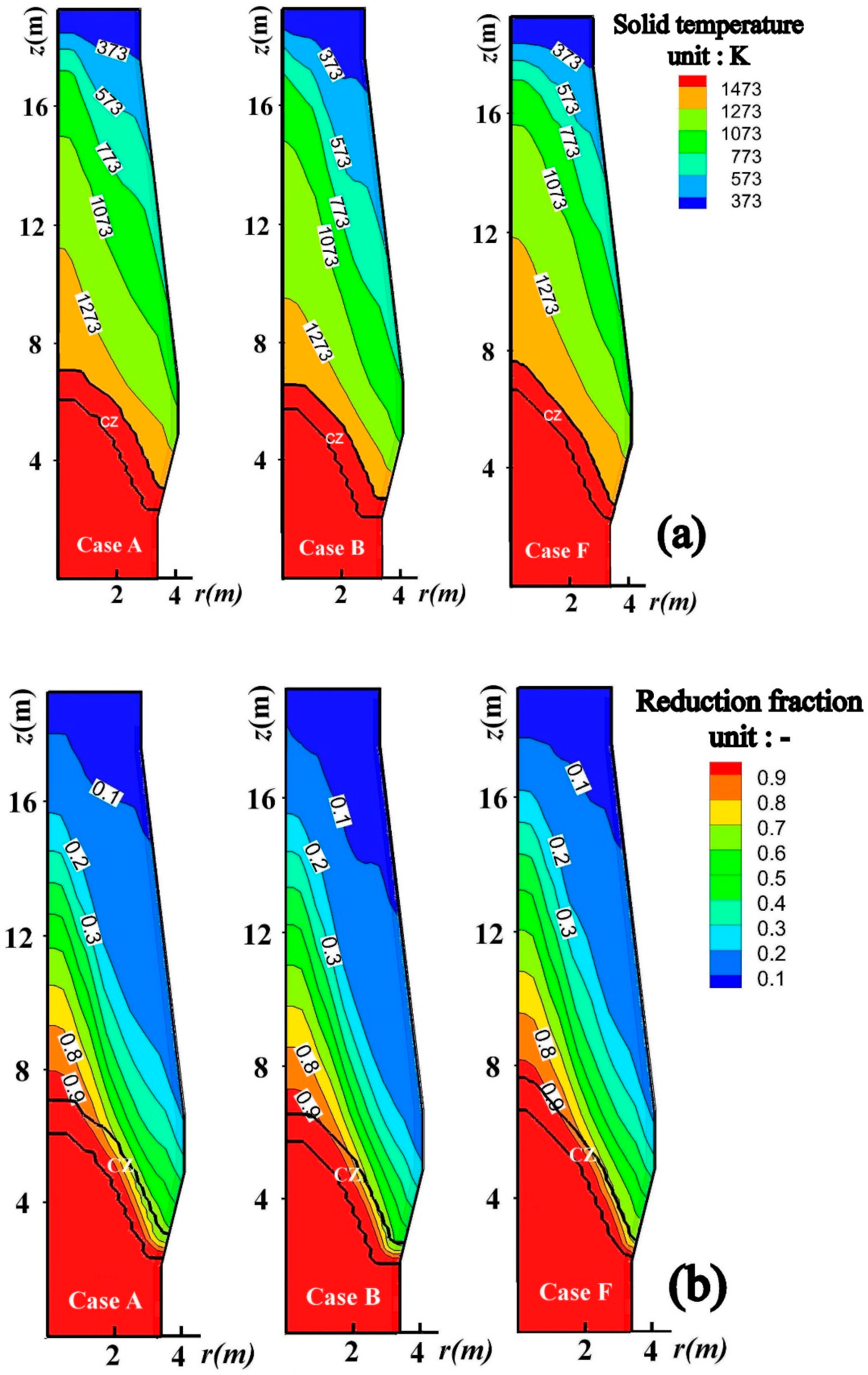
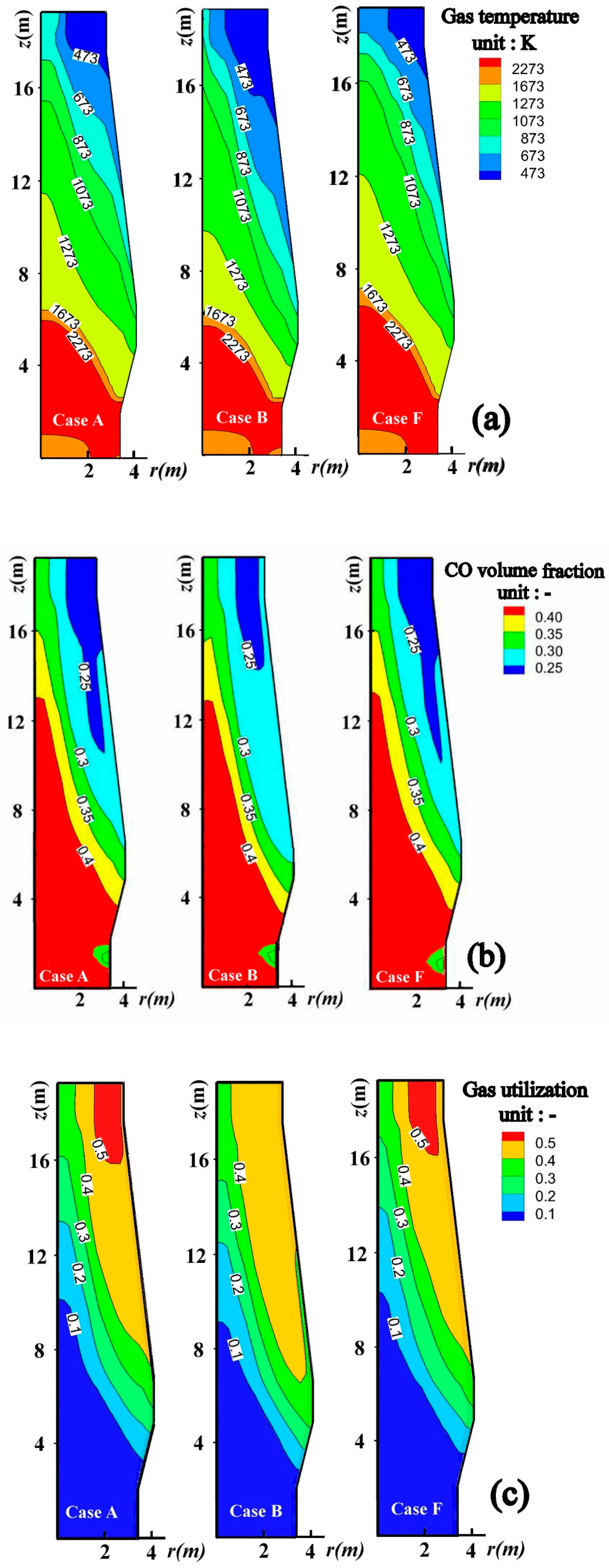
4.1. Effect of Charging Scrap on the BF Internal State
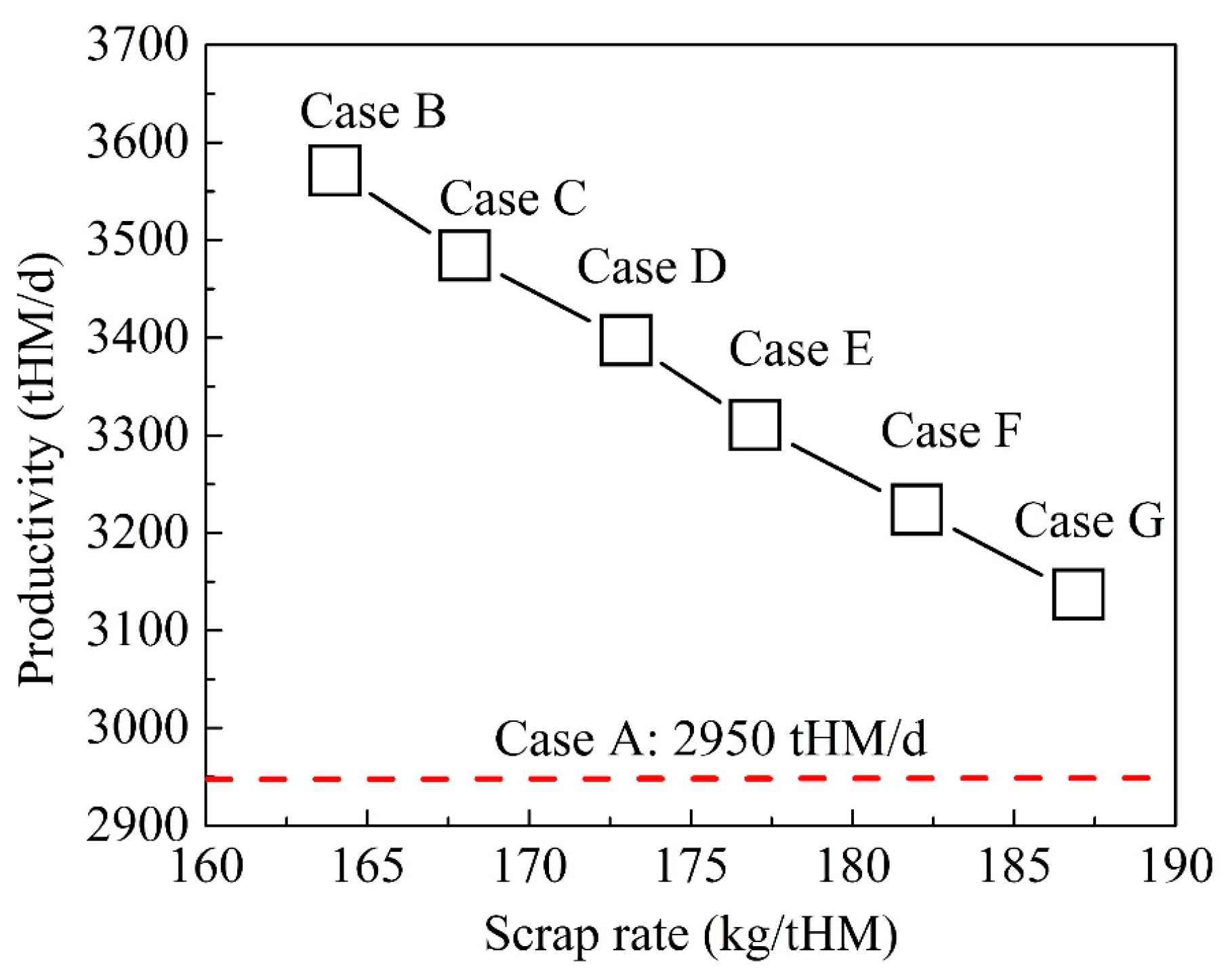
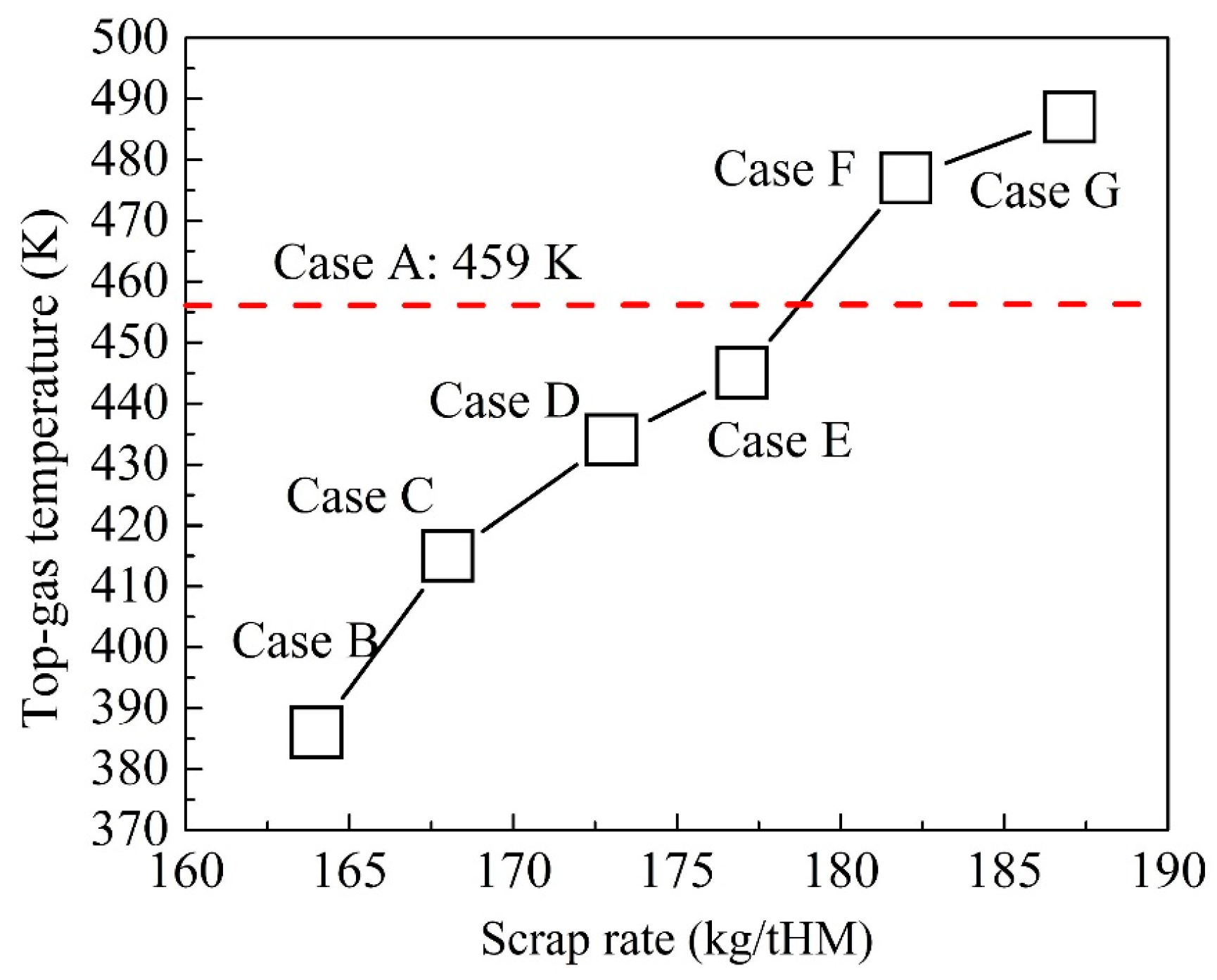
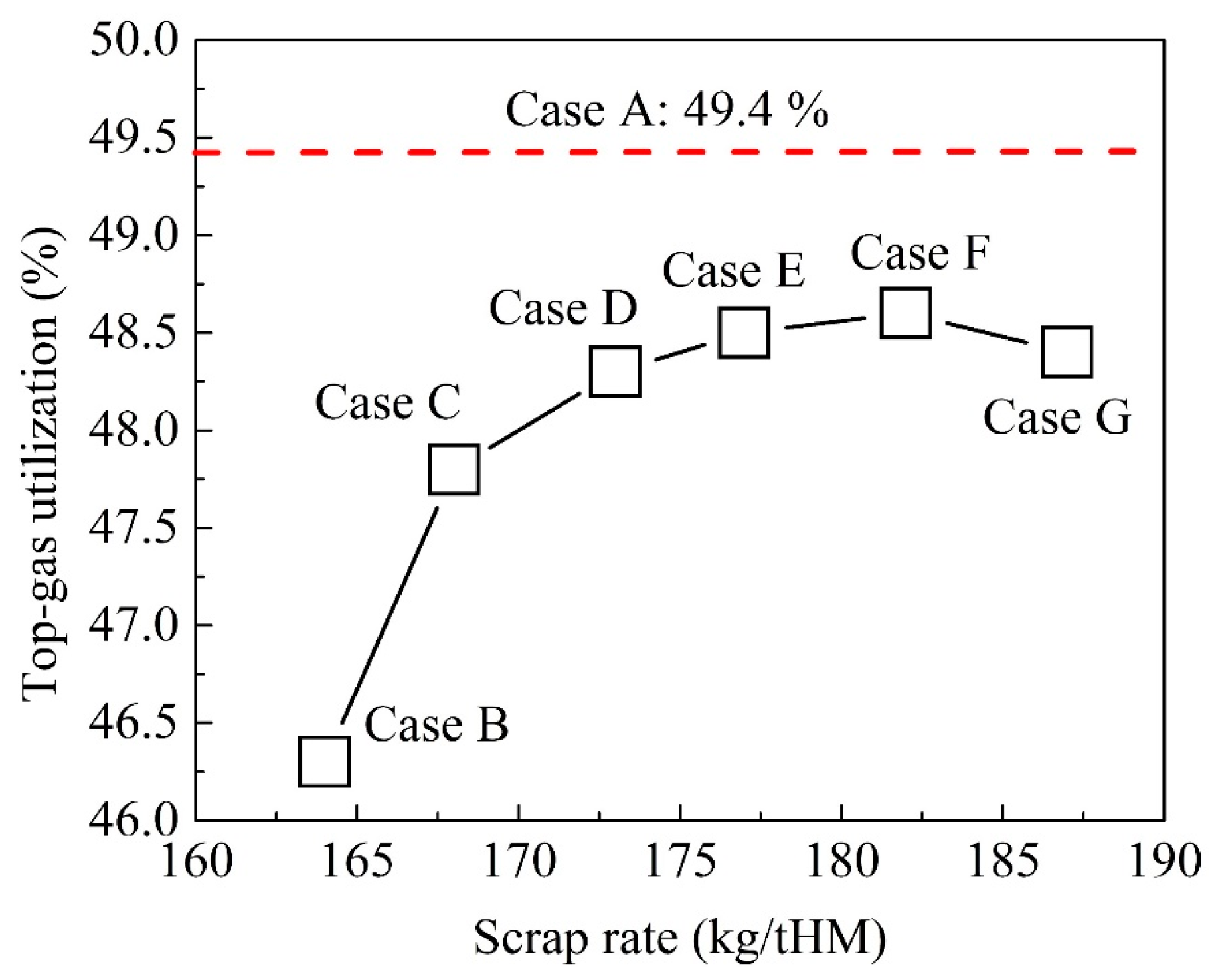
4.2. Optimization of BF Operation with Scrap Charging
4.3. Estimation on Energy Saving
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| Aface | cell face area, m2 |
| A | specific area, m2/m3 |
| ak | rate coefficient of interface k of the three-interface shrinking core model, m/s |
| Cp | heat capacity, J/(kg⋅K) |
| d | diameter, m |
| E | enthalpy source, J/(m3·s) |
| H | total enthalpy, J/kg |
| ΔHi | reaction heat of reaction i, J/kmol |
| k | reaction rate constant/mass transfer coefficient, 1/s, m/s |
| M | molar weight (kg/kmol) |
| m | mass supply/consumption rate of the given element, kg/s |
| P | pressure, Pa |
| Pr | Prandtal number, - |
| Ri | reaction rate of reaction i, kmol/(m3⋅s) |
| Re | Reynolds number, - |
| S | source term, units vary |
| Sc | Schmidt number, - |
| T | temperature, K |
| VCell | volume of cell, m3 |
| y | mass fraction, - |
| Vector | |
| gas flow resistance, N/m3 | |
| normal unit vector on the cell face | |
| gas superficial velocity vector, m/s | |
| solid physical velocity vector, m/s | |
| Greek symbol | |
| ϕ, φ | general dependent variable |
| Γ | general diffusion coefficient |
| α | volume fraction, - |
| ⍴ | density, bulk density of solid phase and its species or element, kg/m3 |
| η | fraction of the liquid phase, - |
| λ | thermal conductivity, W/(m⋅K) |
| ε | porosity, - |
| μ | fluid viscosity, kg/(m⋅s) |
| Subscript | |
| s | solid variable |
| g | gas variable |
| l | liquid variable |
| coke | coke variable |
| ore | ore variable |
| scrap | scrap variable |
| species or element name variable of assigned species or element | |
References
- World Steel Association. Steel’s Contribution to a Low Carbon Future and Climate Resilient Societies—World Steel Position Paper; World Steel Association: Brussels, Belgium, 2020; ISBN 978-2-930069-83-8. Available online: https://www.worldsteel.org/en/dam/jcr:7ec64bc1-c51c-439b-84b8-94496686b8c6/Position_paper_climate_2020_vfinal.pdf (accessed on 5 October 2020).
- Holappa, L. A general vision for reduction of energy consumption and CO2 emissions from the steel industry. Metals 2020, 10, 1117. [Google Scholar] [CrossRef]
- Wang, C.; Ryman, C.; Dahl, J. Potential CO2 emission reduction for BF–BOF steelmaking based on optimised use of ferrous burden materials. Int. J. Greenh. Gas Control 2009, 3, 29–38. [Google Scholar] [CrossRef]
- Jaimes, W.; Maroufi, S. Sustainability in steelmaking. Curr. Opin. Green Sustain. Chem. 2020, 24, 42–47. [Google Scholar] [CrossRef]
- Yamamoto, T.; Ujisawa, Y.; Ishida, H.; Takatani, K. Operation and design of scrap melting process of the packed bed type. ISIJ Int. 1999, 39, 705–714. [Google Scholar] [CrossRef]
- Janke, D.; Savov, L.; Weddige, H.J.; Schulz, E. Scrap-based steel production and recycling of steel. Mater. Tehnol. 2000, 34, 387. [Google Scholar]
- Oda, J.; Akimoto, K.; Tomoda, T. Long-term global availability of steel scrap. Resour. Conserv. Recyl. 2013, 81, 81–91. [Google Scholar] [CrossRef]
- Vercammen, S.; Chalabyan, A.; Ramsbottom, O.; Ma, J.; Tsai, C.; The Growing Importance of Steep Scrap in China. McKinsey Company 2017. Available online: https://www.mckinsey.com/~/media/mckinsey/industries/metals%20and%20mining/our%20insights/the%20growing%20importance%20of%20steel%20scrap%20in%20china/the-growing-importance-of-steel-scrap-in-china.pdf (accessed on 5 October 2020).
- Huang, J.; Lin, C. Analysis of Cost Benefit Change Brought by Scrap Ratio Increase in Crude Steel Industry; Chinese Logistics and Purchasing: Beijing, China, 2019; pp. 49–50. (In Chinese) [Google Scholar]
- Liu, K.; Xu, D.; Mei, Z.; Wan, J.; Zhu, Z. Technological measures for increasing scrap ratio. China Steel Focus 2020, 9, 1–2. (In Chinese) [Google Scholar]
- Austin, P.R.; Nogami, H.; Yagi, J. Computational investigation of scrap charging to the blast furnace. ISIJ Int. 1998, 38, 697–703. [Google Scholar] [CrossRef]
- Ryman, C.; Larsson, M. Reduction of CO2 emissions from integrated steelmaking by optimised scrap strategies: Application of process integration models on the BF–BOF system. ISIJ Int. 2006, 46, 1752–1758. [Google Scholar] [CrossRef][Green Version]
- Deng, Y.; Zhang, J.; Liu, R.; Jiao, K.; Yan, B. Smelting practice of scrap addition in blast furnace and theoretical analysis of cost saving. J. Iron Steel Res. Int. 2020, 27, 1005–1010. [Google Scholar] [CrossRef]
- Li, H.; Mo, C.; Tang, Z.; Wang, Z.; Pan, J. Analysis and practice of cost reduction based on addition of scrap steel in Liusteel 5# blast furnace. Ind. Furn. 2018, 40, 39–42. (In Chinese) [Google Scholar]
- Liu, L.; Chen, S.; Chen, Y. Experiment of increasing output based on addition of scrap steel in Shaosteel 6# blast furnace. Ironmaking 2019, 38, 37–39. (In Chinese) [Google Scholar]
- Chu, M.; Yang, X.; Shen, F.; Yagi, J.; Nogami, H. Numerical simulation of innovative operation of blast furnace based on multi-fluid model. J. Iron Steel Res. Int. 2006, 13, 8–15. [Google Scholar] [CrossRef]
- Okosun, T.; Silaen, A.K.; Zhou, C.Q. Review on computational modeling and visualization of the ironmaking blast furnace at Purdue University Northwest. Steel Res. Int. 2019, 90, 1900046. [Google Scholar] [CrossRef]
- Shen, Y.; Guo, B.; Chew, S.; Austin, P.; Yu, A. Three-dimensional modeling of flow and thermochemical behavior in a blast furnace. Metall. Mater. Trans. B 2015, 46, 432–448. [Google Scholar] [CrossRef]
- Tang, H.; Rong, T.; Fan, K. Numerical investigation of applying high-carbon metallic briquette in blast furnace ironmaking. ISIJ Int. 2019, 59, 810–819. [Google Scholar] [CrossRef]
- Austin, P.R.; Nogami, H.; Yagi, I.J. A mathematical model of four phase motion and heat transfer in the blast furnace. ISIJ Int. 2019, 37, 458–467. [Google Scholar] [CrossRef]
- Gupta, G.S.; Rudolph, V. Comparison of blast furnace raceway size with theory. ISIJ Int. 2006, 46, 195–201. [Google Scholar] [CrossRef]
- Mathieson, J.G.; Truelove, J.S.; Rogers, H. Toward an understanding of coal combustion in blast furnace tuyere injection. Fuel 2005, 84, 1229–1237. [Google Scholar] [CrossRef]
- Chen, J.; Akiyama, T.; Nogami, H.; Yagi, I.J.; Takahashi, H. Modeling of solid flow in moving beds. ISIJ Int. 1993, 33, 664–671. [Google Scholar] [CrossRef]
- Austin, P.R.; Nogami, H.; Yagi, J.I. A mathematical model for blast furnace reaction analysis based on the four fluid model. ISIJ Int. 1997, 37, 748–755. [Google Scholar] [CrossRef]
- CHAM. PHOENICS User Document; CHAM Ltd.: London, UK, 2000. [Google Scholar]
- Tokuda, M.; Yoshikoshi, H.Y.; Ohtani, M. Kinetics of the reduction of iron ore. Trans. ISIJ. 1973, 13, 351–363. [Google Scholar] [CrossRef]
- Ueda, S.; Kon, T.; Kurosawa, H.; Natsui, S.; Ariyama, T.; Nogami, H.J.I.I. Influence of shape of cohesive zone on gas flow and permeability in the blast furnace analyzed by DEM-CFD model. ISIJ Int. 2015, 55, 1232–1236. [Google Scholar] [CrossRef]
- Nath, N.K. Simulation of gas flow in blast furnace for different burden distribution and cohesive zone shape. Mater. Manuf. Process. 2002, 17, 671–681. [Google Scholar] [CrossRef]
- Wang, J.; Guo, Z.; Wang, Z. Application and practice of fully dry cloth-bag dust removing technology of No. 4 BF in baogang. Energy Metall. Ind. 2007, 1, 5–11. (In Chinese) [Google Scholar]
- Li, H.; Tao, W.; Feng, X.; Sun, Z. Application of the dry cloth-bag dedusting technology in baosteel no. 1 blast furnace. Baosteel Technol. 2010, 5, 15–19. (In Chinese) [Google Scholar]
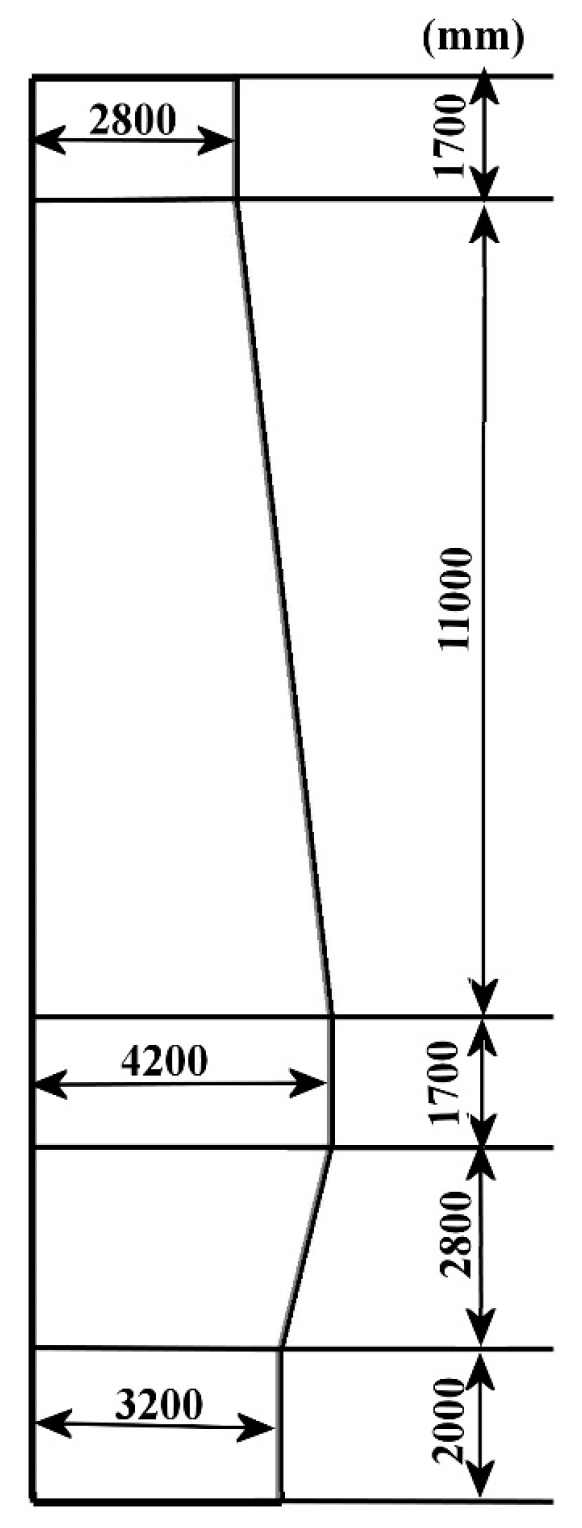
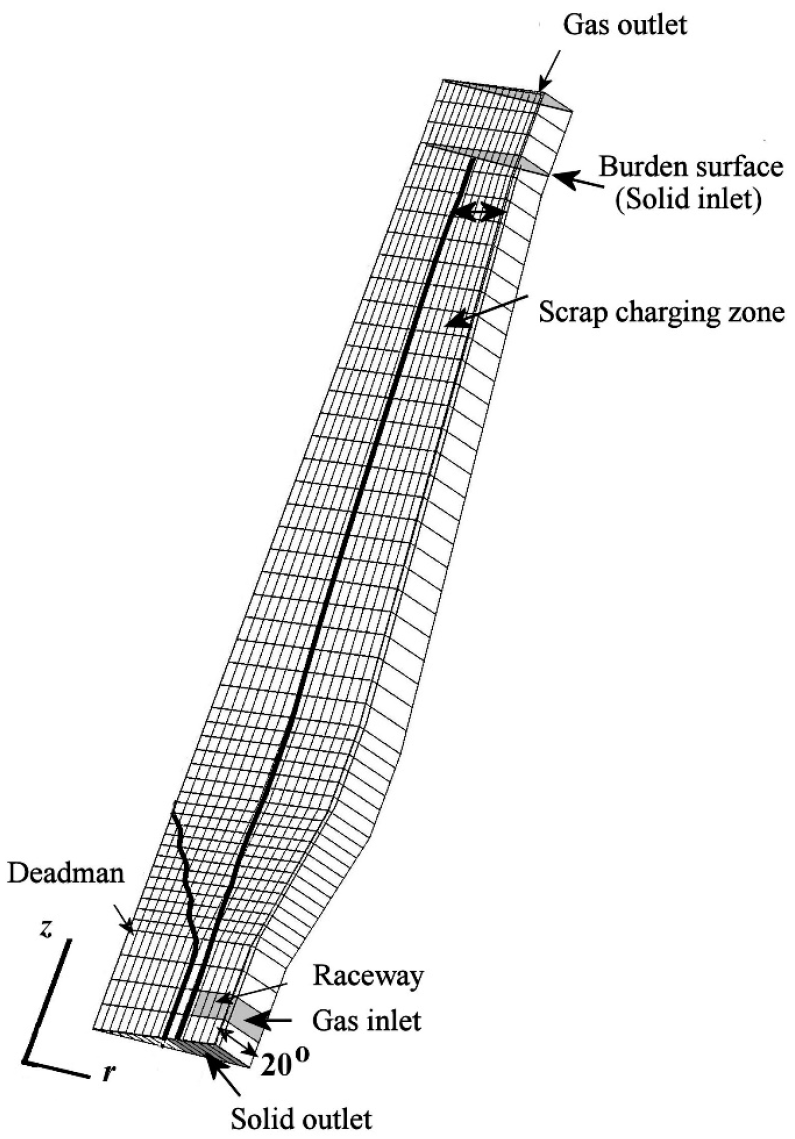
| Parameter | Value |
|---|---|
| Output (t/d) | 2950 |
| Blast temperature (K) | 1423 |
| Blast flow rate (Nm3/min) | 2100 |
| Blast oxygen enrichment (%) | 4.5 |
| BF top pressure (Pa) | 2.0 × 105 |
| PC injection rate (kg/s) | 5.12 |
| Weight of ore batch (t) | 27.0 |
| Weight of coke batch (t) | 2.9 |
| Solid temperature (K) | 300 |
| Properties of ore burden (78% sinter, 14% pellet, and 8% lump ore) | Composition: TFe: 56.3 wt%, FeO: 7.19 wt%, CaO: 8.60 wt%, SiO2: 7.07 wt%, Al2O3: 2.19 wt%; Porosity: 0.35; Bulk density: 1750 kg/m3; Average size: 20 mm; Ore rate: 1698 kg/tHM. |
| Properties of coke | Composition: Fixed carbon: 88.0 wt%, ash: 12.0 wt%; porosity: 0.50; Bulk density: 500 kg/m3; Average size: 40 mm; Coke rate: 385 kg/tHM. |
| Properties of pulverized coal (PC) | Composition C: 80.0 wt%, H: 4.0 wt%, O: 3.5 wt%, N: 2.0 wt%, and S: 0.32 wt%; H2O: 4.0 wt%, ash: 7.0 wt%; Average size: 90 µm; PC rate: 150 kg/tHM. |
| Properties of molten iron and molten slag | Molten iron: [% C]: 5.0 wt%, Temperature: 1773 K, Average heat capacity: 1000 J/kg; Slag rate: 360 kg/tHM |
| Average Size (mm) | Bulk Density (kg/m3) | Porosity (-) | Chemical Composition (wt%) | ||||
|---|---|---|---|---|---|---|---|
| C | Si | Mn | P | S | |||
| 12 | 5320.0 | 0.3 | 0.24 | 0.31 | 1.39 | 0.024 | 0.027 |
| Case | Weight of Ore Batch (t) | Sinter Weight in Ore Batch (t) | Scrap Weight in Ore Batch (t) | Decrease of Sinter in Ore Batch (t) |
|---|---|---|---|---|
| A (base case) | 27.0 | 27.0 | 0 | 0 |
| B | 30.2 | 27.0 | 3.2 | 0 |
| C | 29.4 | 26.2 | 3.2 | 0.8 |
| D | 28.6 | 25.4 | 3.2 | 1.6 |
| E | 27.8 | 24.6 | 3.2 | 2.4 |
| F | 27.0 | 23.8 | 3.2 | 3.2 |
| G | 26.2 | 23.0 | 3.2 | 4.0 |
| No. | Reaction | Reaction Rate, kmol/(m3⋅s) | Expression |
|---|---|---|---|
| 1 | 3Fe2O3(s) + CO(g) = 2Fe3O4(s) + CO2(g) | R1 | |
| 2 | Fe3O4(s) + CO(g) = 3FeO(s) + CO2(g) | R2 | |
| 3 | FeO(s) + CO(g) = Fe(s) + CO2(g) | R3 | |
| 4 | C(coke) + 1/2O2(g) = CO(g) | R4 | , , , . For reaction (4), , . For reaction (5), , . |
| 5 | C(coke) + CO2(g) = 2CO(g) | R5 | |
| 6 | Fe(s) = Fe(l) | R6 | . for reactions (6–8); and for reaction (9). |
| 7 | FeO(s) = FeO(l) | R7 | |
| 8 | Gangue(s) = Slag(l) | R8 | |
| 9 | Fe(scrap,s) = Fe(l) | R9 | |
| 10 | FeO(l) + C(s) = Fe(l) + CO(g) | R10 |
| Equation | |||
|---|---|---|---|
| Mass | 1 | 0 | |
| Momentum | |||
| Enthalpy | |||
| Species | 0 | ||
| Equation | |||
|---|---|---|---|
| Continuity | 1 | 0 | |
| Momentum | |||
| Enthalpy | |||
| Species | 0 | 0 | |
| 0 | |||
| 0 | |||
| 0 | |||
| 0 | |||
| 0 | |||
| 0 |
| Condition | Variables | Case A | Case B | Case C | Case D | Case E | Case F | Case G |
|---|---|---|---|---|---|---|---|---|
| Solid inlet conditions | Ore supply rate (kg/s) | 2.90 | 2.90 | 2.81 | 2.73 | 2.64 | 2.56 | 2.47 |
| Scrap supply rate (kg/s) | 0 | 0.34 | 0.34 | 0.34 | 0.34 | 0.34 | 0.34 | |
| Coke supply rate (kg/s) | 0.657 | - | - | - | - | - | - | |
| Solid temperature (K) | 300 | |||||||
| Gas inlet conditions | Gas supply rate (kg/s) | 2.53 | ||||||
| Gas composition (mass fraction) | CO: 0.18, O2: 0.16, and N2: 0.66 | |||||||
| Gas temperature (K) | 2441 | |||||||
| Case | A | B | C | D | E | F | G |
|---|---|---|---|---|---|---|---|
| Scrap rate (kg/tHM) | 0 | 165 | 169 | 174 | 178 | 183 | 188 |
| Ore rate (kg/tHM) | 1698 | 1403 | 1396 | 1388 | 1380 | 1371 | 1362 |
| Reducing Agent | Item | Case A (Base) (kg/tHM) | Case E (kg/tHM)) | |
|---|---|---|---|---|
| PC | I | Combustion at raceway | 150 | 133.70 |
| - | Total | 150 | 133.70 | |
| Coke | I | Combustion at raceway | 196.0 | 175.0 |
| II | Solution loss in upper BF | 36.76 | 29.09 | |
| III | Direct reduction of molten FeO in lower BF | 75.64 | 64.50 | |
| IV | Carburization of molten iron | 57.00 | 57.00 | |
| V | Other (SiO2, and MnO reduction, etc.) | 19.60 | 19.60 | |
| - | Total | 385.00 | 345.20 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Z.; Yu, Z.; She, X.; Tang, H.; Xue, Q. Numerical Investigation of Blast Furnace Operation with Scrap Charging. Metals 2020, 10, 1666. https://doi.org/10.3390/met10121666
Liu Z, Yu Z, She X, Tang H, Xue Q. Numerical Investigation of Blast Furnace Operation with Scrap Charging. Metals. 2020; 10(12):1666. https://doi.org/10.3390/met10121666
Chicago/Turabian StyleLiu, Zhu, Zi Yu, Xuefeng She, Huiqing Tang, and Qingguo Xue. 2020. "Numerical Investigation of Blast Furnace Operation with Scrap Charging" Metals 10, no. 12: 1666. https://doi.org/10.3390/met10121666
APA StyleLiu, Z., Yu, Z., She, X., Tang, H., & Xue, Q. (2020). Numerical Investigation of Blast Furnace Operation with Scrap Charging. Metals, 10(12), 1666. https://doi.org/10.3390/met10121666






