Specific Features of Structure Transformation and Properties of Amorphous-Nanocrystalline Alloys
Abstract
:1. Introduction
2. Structural Changes Occurring during Heating and Deformation
3. Formation of Nanoglasses
4. Processes of Crystallization of Amorphous Alloys
5. Heterogeneous Nucleation
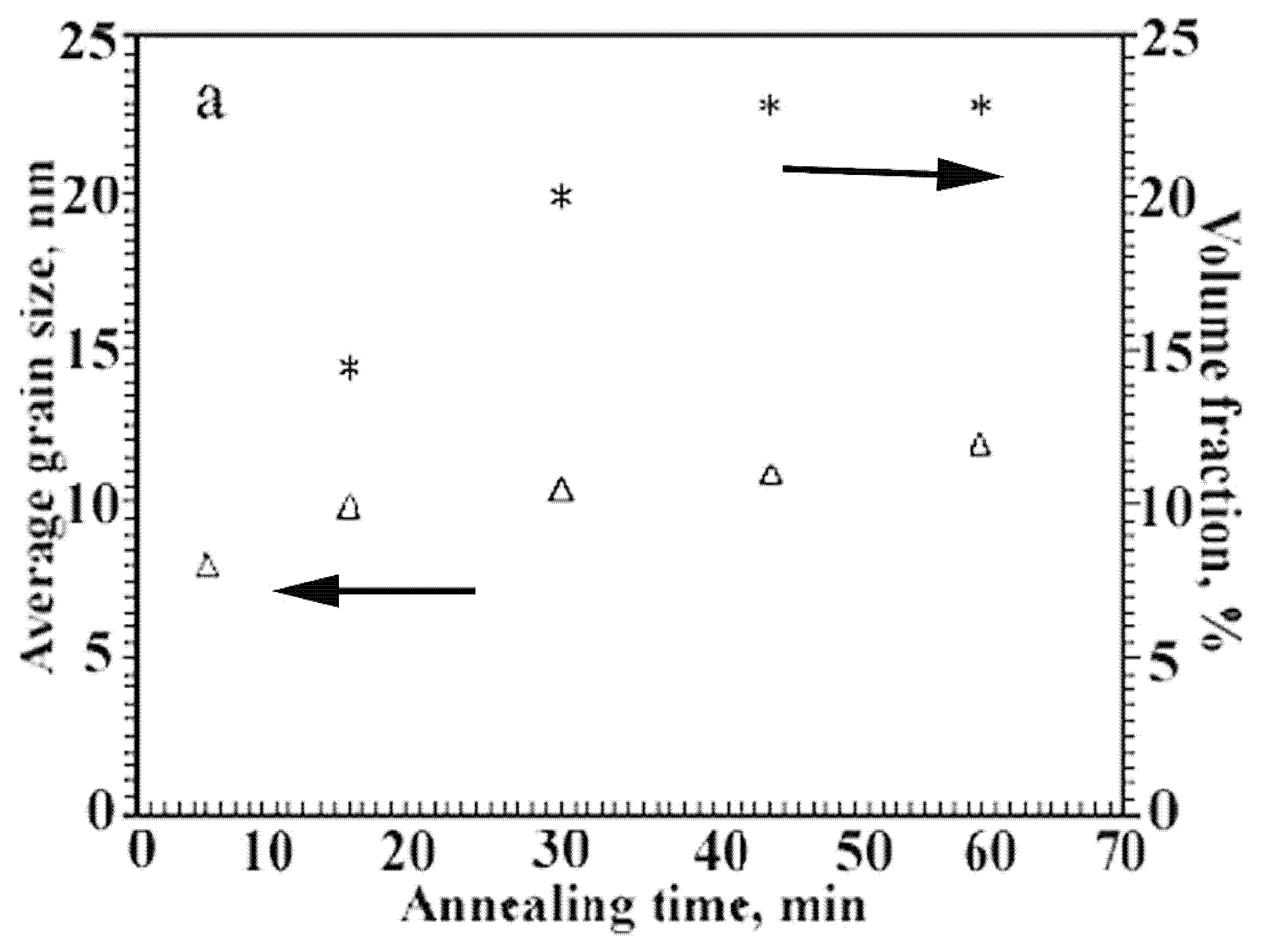
- the absence of small crystals in the distribution under exposure for 15 min;
- a significant decrease in the region of small sizes under exposure for 5 min;
- a gradual decrease in the large particle fraction (the right branch of the distribution), which is typical for the nonstationary rate of nucleation of the nanocrystals (with the latent period).
6. Some Features of Nanocrystal Formation (Free Volume)
- using elastic deformation;
- by viscous flow of an amorphous matrix;
- by plastic deformation of the crystalline phases;
- due to diffusion escape of excess volume with formation of pores in the reaction front and/or diffusion of the elementary carriers of free volume to the surface and their annihilation on it;
- by disruption.
6.1. Dependence of the Sequence of Phase Formation and Crystal Morphology on the Bulk Effect Demonstration in Alloys of Fe-B, Fe-Co-Si-B Systems
6.2. Formation of a Nanocrystalline Structure as Demonstration of the Bulk Effect
6.3. Compensation of Structural Mismatch by Pore Fformation and Nanocrystal Formation in the Shear Bands
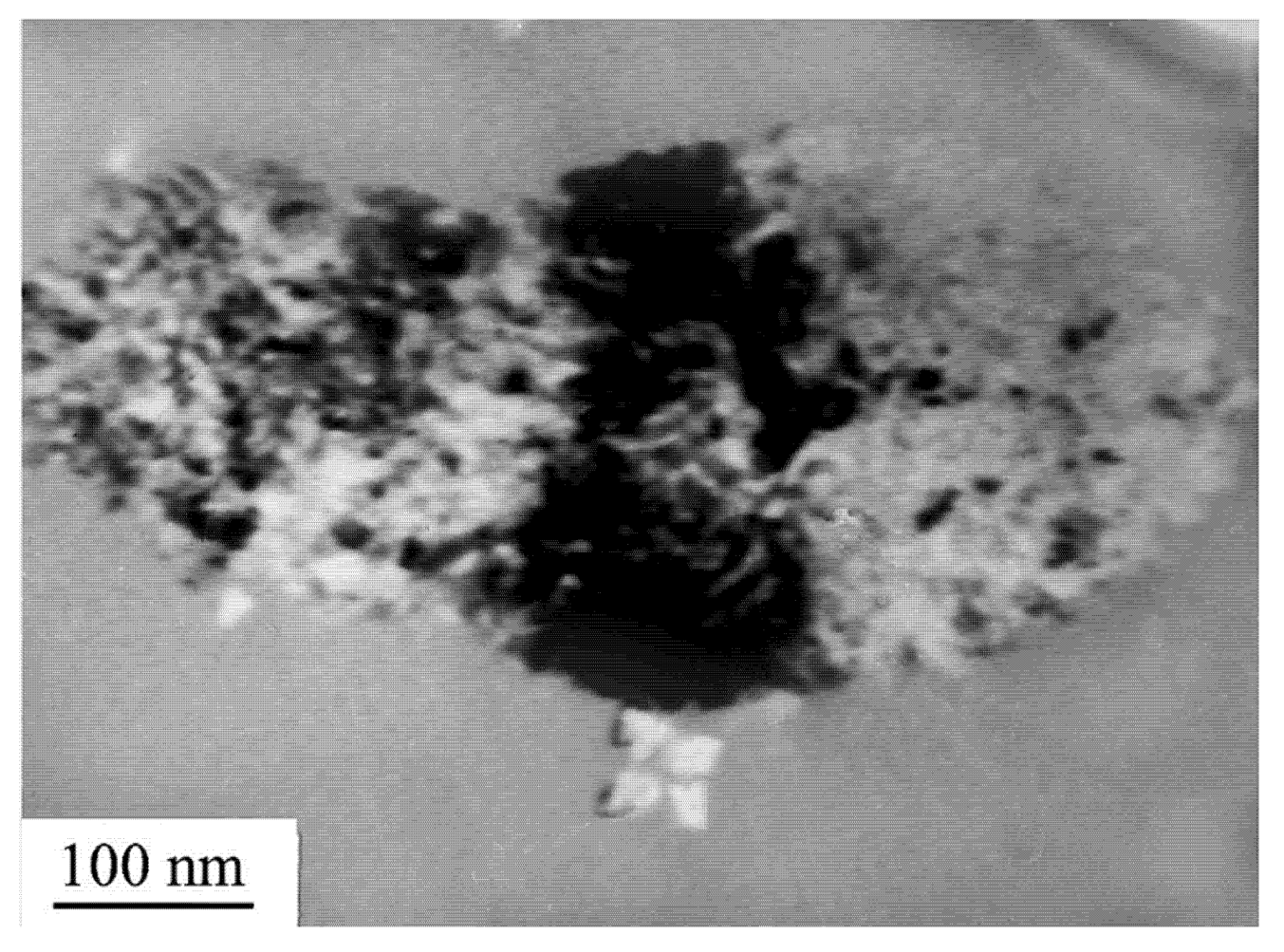
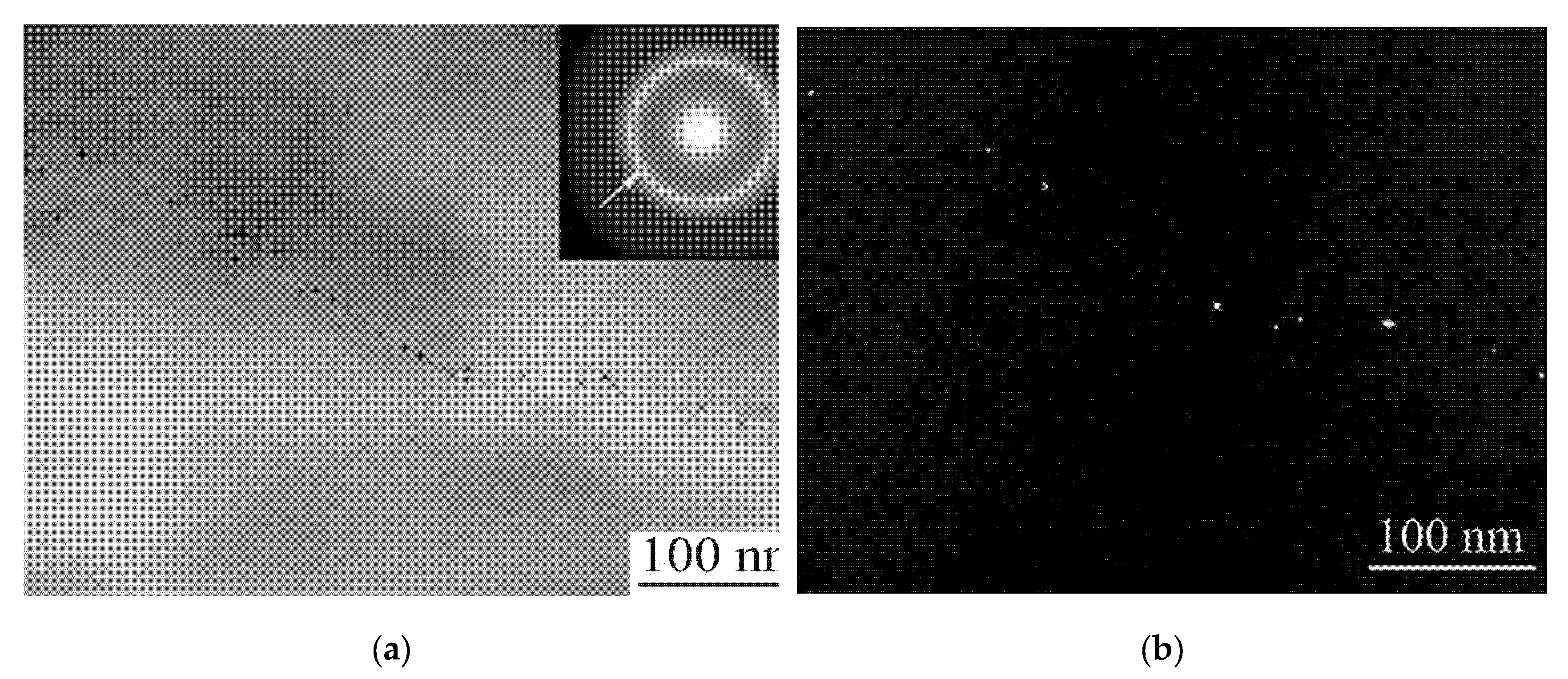
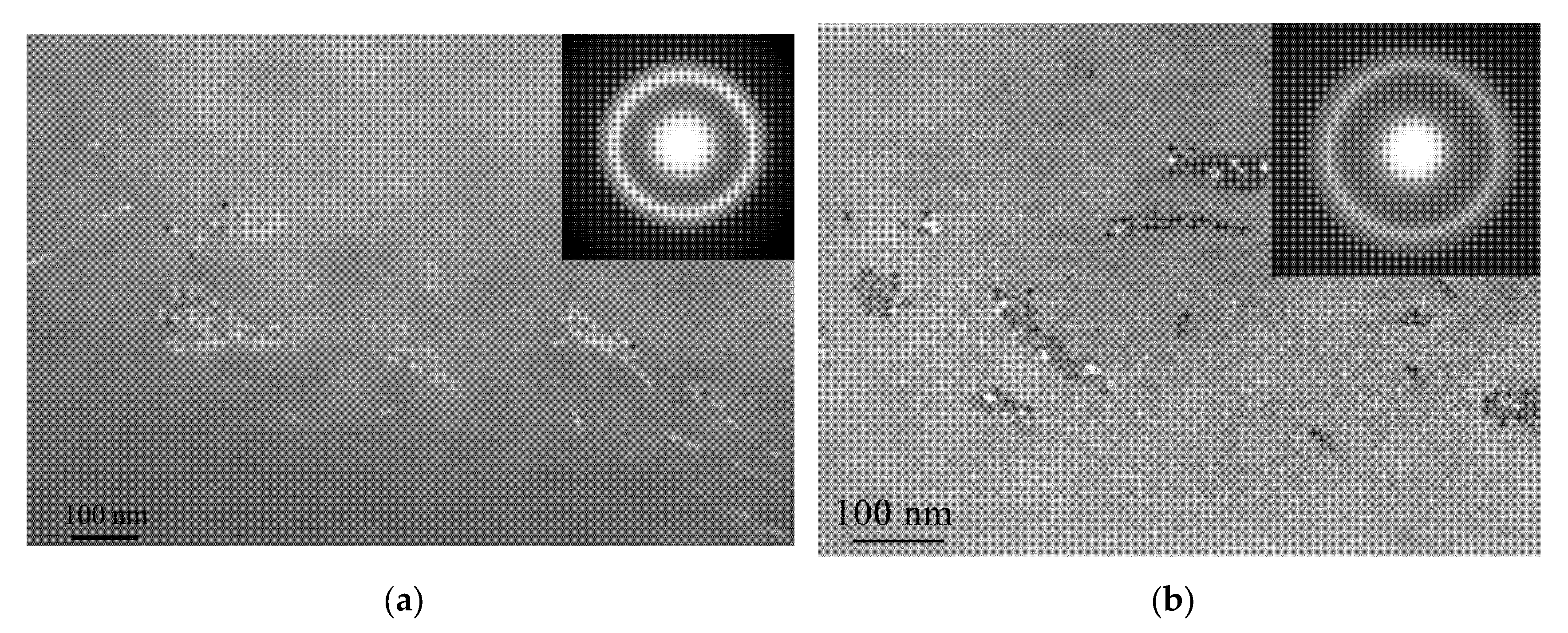
7. Nanocrystal Formation in Amorphous Phase
8. Nanocrystal Formation under Heating and Deformation
9. Mechanical Properties of Metallic Glasses near Shear Bands
10. Amorphous Structure Rejuvenation
11. Possibility of Controlling the Structure of Fully or Partially Crystallized Samples
12. Conclusions
- The transformation of a homogeneous amorphous phase into a heterogeneous phase, including the scale of inhomogeneities and the effect of external influences, are considered.
- The influence of amorphous structure changes on the forming crystalline structure is shown.
- The crystallization processes of the amorphous phase, such as homogeneous and heterogeneous nucleation of crystals, are considered.
- Possible way of compensation for volume mismatch (changing the sequence of formation of crystalline phases, crystal morphology, formation of nanocrystals or pores) is analyzed.
- Nanocrystal formation is described.
- The effect of plastic deformation on the formation of nanocrystals and mechanical properties of the amorphous phase near the shear bands are shown.
- The possibility of restoring the amorphous structure is discussed.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Willens, R.H.; Klement, W.; Duwez, P. Continuous series of metastable solid solutions in silver-copper alloys. J. Appl. Phys. 1960, 31, 1136–1137. [Google Scholar] [CrossRef]
- He, Y.; Poon, J.F.; Shiflet, G.Y. Synthesis and properties of metallic glasses that contain aluminum. Science 1988, 241, 1640. [Google Scholar] [CrossRef]
- Gleiter, H. Nanocrystalline materials. Prog. Mater. Sci. 1989, 33, 223. [Google Scholar] [CrossRef] [Green Version]
- Birringer, R. Nanocrystalline materials. Mater. Sci. Eng. A 1989, 117, 33–43. [Google Scholar] [CrossRef]
- Abrosimova, G.E.; Aronin, A.S.; Kir’janov, Y.V.; Gloriant, T.F.; Greer, A.L. Nanostructure and microhardness of Al86Ni11Yb3 nanocrystalline alloy. NanoStructured Mater. 1999, 12, 617–620. [Google Scholar] [CrossRef]
- Inoue, A.; Ochiai, T.; Horio, Y.; Masumoto, T. Formation and mechanical properties of amorphous Al-Ni-Nd alloys. Mater. Sci. Eng. A 1994, 179, 649. [Google Scholar] [CrossRef]
- Kim, Y.H.; Inoue, A.; Masumoto, T. Increase in mechanical strength of Al–Y–Ni amorphous alloys by dispersion of nanoscale fcc-Al particles. Mater. Trans. JIM 1991, 32, 331–338. [Google Scholar] [CrossRef] [Green Version]
- Chou, C.-P.; Turnbull, D. Transformation behavior of Pd-Au-Si metallic glasses. J. Non Cryst. Solids 1975, 17, 169–188. [Google Scholar] [CrossRef]
- Abrosimova, G.E.; Aronin, A.S. Phase segregation in the Fe90Zr10 amorphous alloy under heating. Phys. Solid State 1998, 40, 1603–1606. [Google Scholar] [CrossRef]
- Chen, H.S.; Turnbull, D. Formation, stability and structure of palladium-silicon based alloy glasses. Acta Metal. 1969, 17, 1021. [Google Scholar] [CrossRef]
- Osamura, K. SAXS study on the structure and crystallization of amorphous metallic alloys. Colloid. Polym. Sci. 1981, 259, 677. [Google Scholar] [CrossRef]
- Terauchi, H. Heterogeneous structure of amorphous materials. J. Phys. Soc. Jap. 1983, 52, 3454–3459. [Google Scholar] [CrossRef]
- Osamura, K. Structure and mechanical properties of a Fe90Zr10 amorphous alloy. J. Mater. Sci. 1984, 19, 1917. [Google Scholar] [CrossRef]
- Mak, A.; Samwer, K.; Johnson, W.L. Evidence for two distinct amorphous phases in (Zr0.667Ni0.333)1−xBx alloys. Phys. Lett. A 1983, 98, 353. [Google Scholar] [CrossRef]
- Mehra, M.; Schulz, R.; Johnson, W.L. Structural studies and relaxation behavior of (Mo0.6Ru0.4)100-xBxmetallic glasses. J. Non Crystall. Solids 1984, 61, 859–864. [Google Scholar] [CrossRef]
- Nagarajan, T.; Asari, U.C.; Srinivasan, S.; Sridharan, V.; Narayanasamy, A. Amorphous phase separation in METGLAS 2605CO. Hyperfine Interact. 1987, 34, 491. [Google Scholar] [CrossRef]
- Abrosimova, G.E.; Aronin, A.S.; Ignat’eva, E.Y.; Molokanov, V.V. Phase decomposition and nanocrystallization in amorphous Ni70Mo10P20 alloy. J. Magn. Magn. Mater. 1999, 203, 169–171. [Google Scholar] [CrossRef]
- Abrosimova, G.E.; Aronin, A.S. Evolution of the amorphous-phase structure in metal-metal type metallic glasses. J. Surf. Investig. 2015, 9, 887–893. [Google Scholar] [CrossRef]
- Marcus, M.A. Phases separation and crystallization in amorphous Pd-Si-Sb. J. Non Cryst. Solids 1979, 30, 317–335. [Google Scholar] [CrossRef]
- Yavari, A.R. New amorphous Pb60Pd40 with split first X-ray halo and possible unmixing. Inter. J. Rapid Solidif. 1986, 2, 47–54. [Google Scholar]
- Inoue, A.; Bizen, Y.; Kimura, H.M.; Yamamoto, Y.; Tsai, A.P. Development of compositional short-range ordering in an Al50Ge40Mn10 amorphous alloy upon annealing. J. Mater. Sci. Lett. 1987, 6, 811–814. [Google Scholar] [CrossRef]
- Inoue, A.; Yamamoto, M.; Kimura, H.M.; Masomoto, T. Ductile aluminum-based amorphous alloys with two separated phases. J. Mater. Sci. Lett. 1987, 6, 194–196. [Google Scholar] [CrossRef]
- Yavari, A.R. On the structure of metallic glasses with double diffraction halos. Acta Metall. 1988, 36, 1863–1872. [Google Scholar] [CrossRef]
- Kűndig, A.A.; Ohnuma, M.; Ping, D.H.; Ohkubo, T.; Hono, K. In situ formed two-phase metallic glass with surface fractal microstructure. Acta Mater. 2004, 52, 2441–2448. [Google Scholar] [CrossRef]
- Mattern, N.; Kühn, U.; Gebert, A.; Gemming, T.; Zinkevich, M.; Wendrock, H.; Schultz, L. Microstructure and thermal behavior of two-phase amorphous Ni–Nb–Y alloy. Scr. Mater. 2005, 53, 271–274. [Google Scholar] [CrossRef]
- Han, J.H.; Mattern, N.; Vainio, U.; Shariq, A.; Sohn, S.W.; Kim, D.H.; Eckert, J. Phase separation in Zr56-xGdxCo28Al16 metallic glasses (0 <x <20). Acta Mater. 2014, 66, 262–272. [Google Scholar] [CrossRef]
- Masumoto, T.; Maddin, R. Structural stability and mechanical properties of amorphous metals. Mater. Sci. Eng. 1975, 19, 1–24. [Google Scholar] [CrossRef]
- Wang, X.D.; Bednarcik, J.; Saksi, K.; Franz, H.; Cao, Q.P.; Jiang, J.Z. Tensile behavior of bulk metallic glasses by in situ x-ray diffraction. Appl. Phys. Lett. 2007, 91, 081913. [Google Scholar] [CrossRef] [Green Version]
- Stoica, M.; Das, J.; Bednarcik, J.; Franz, H.; Mattern, N.; Wang, W.H.; Eckert, J. Strain distribution in Zr64.13Cu15.75Ni10.12Al10 bulk metallic glass investigated by in situ tensile tests under synchrotron radiation. J. Appl. Phys. 2008, 104, 013522. [Google Scholar] [CrossRef] [Green Version]
- Wang, X.D.; Bednarcik, J.; Franz, H.; Lou, H.B.; He, Z.H.; Cao, Q.P.; Jiang, J.A. Local strain behavior of bulk metallic glasses under tension studied by in situ x-ray diffraction. Appl. Phys. Lett. 2009, 94, 011911. [Google Scholar] [CrossRef] [Green Version]
- Abrosimova, G.; Aronin, A.; Afonikova, N.; Kobelev, N. Influence of deformation on the structural transformation of the Pd40Ni40P20 amorphous phase. Phys. Solid State 2010, 52, 1892–1898. [Google Scholar] [CrossRef]
- Suzuki, C.K.; Doi, K.; Kohra, K. Small Angle and Vary Small Angle X-ray Scattreing from Amorphous Pd80Si20 before and after Cold Work. Jpn. J. Appl. Phys. 1981, 20, L271–L274. [Google Scholar] [CrossRef]
- Walter, J.; Legrand, D.G.; Luborsky, F.E. Small Angle X-ray Scattering from the Amorphous Alloy Fe40Ni40P14B6. Mater. Sci. Eng. 1977, 29, 161–167. [Google Scholar] [CrossRef]
- Liebermann, H.H.; Graham, C.D.; Flanders, P., Jr. Changes in curie temperature, physical dimensions, and magnetic anisotropy during annealing of amorphous magnetic alloys. IEEE Trans. Magn. 1977, 13, 1541–1543. [Google Scholar] [CrossRef]
- Abrosimova, G.E.; Serebeyakov, A.V.; Sokolovskaya, Z.D. Change of structure and magnetic-properties of amorphous iron base-metal—metalloid alloys during heat-treatment. Fiz. Met. I Metalloved. 1988, 66, 727–730. [Google Scholar]
- Chen, H.S. Ductile-brittle transition in metallic glasses. Mater. Sci. Eng. 1976, 26, 79–82. [Google Scholar] [CrossRef]
- Naka, M.; Masumoto, T.; Chen, H.S. Effect of metalloidal elements on strength and thermal stability of Fe-base glasses. J. De Phys. C 1980, 41, C8-839–C8-842. [Google Scholar]
- Gleiter, H. Nanoglasses: A new kind of noncrystalline materials. Beilstein J. Nanotechnol. 2013, 4, 517–533. [Google Scholar] [CrossRef] [Green Version]
- Aronin, A.S.; Abrosimova, G.E.; Gurov, A.F.; Kir’janov, Y.V.; Molokanov, V.V. Nanocrystallization of bulk Zr–Cu–Ti metallic glass. Mater. Sci. Eng. A 2001, 304, 375–379. [Google Scholar] [CrossRef]
- Abrosimova, G.; Aronin, A.; Ignatieva, E. Decomposition of amorphous phase in Ni70Mo10B20 alloy above glass transition temperature. Mater. Sci. Eng. A 2007, 449, 485–488. [Google Scholar] [CrossRef]
- Doi, K.; Kayano, H.; Masumoto, T. Small-angle scattering from neutron-irradiated amorphous Pd80Si20. J. Appl. Cryst. 1978, 11, 605. [Google Scholar] [CrossRef]
- Fang, J.X.; Vainio, U.; Puff, W.; Würschum, R.; Wang, X.L.; Wang, D.; Ghafari, M.; Jiang, F.; Sun, J.; Hahn, H.; et al. Atomic structure and structural stability of Sc75Fe25 nanoglasses. Nano Lett. 2012, 12, 458–463. [Google Scholar] [CrossRef] [PubMed]
- Hebert, R.J. Deformation-induced synthesis of Al-based heterogeneous nanoscale microstructures. Rev. Adv. Mater. Sci. 2004, 6, 120–130. [Google Scholar]
- Gleiter, H. Are there ways to synthesize materials beyond the limits of today? Metall. Mater. Trans. A 2009, 40, 1499–1509. [Google Scholar] [CrossRef]
- Ritter, Y.; Şopu, D.; Gleiter, H.; Albe, K. Structure, stability and mechanical properties of internal interfaces in Cu64Zr36 nanoglasses studied by MD simulations. Acta Mater. 2011, 59, 6588–6593. [Google Scholar] [CrossRef]
- Adjaoud, O.; Albe, K. Microstructure formation of metallic nanoglasses: Insights from molecular dynamic simulations. Acta Mater. 2018, 145, 322–330. [Google Scholar] [CrossRef]
- Cheng, B.; Trelewicz, J.R. Controlling interface structure in nanoglasses produced through hydrostatic compression of amorphous nanoparticles. Phys. Rev. Mater. 2019, 3, 035602. [Google Scholar] [CrossRef]
- Aronin, A.S.; Abrosimova, G.E.; Zver’kova, I.I.; Kir’janov, Y.V.; Molokanov, V.V.; Petrzhik, M.I. The structure of nanocrystalline Ni58.5Mo31.5B10 and structure evolution at heat treatment. Mater. Sci. Eng. A 1997, 226, 536–540. [Google Scholar] [CrossRef]
- Zhang, Y.; Hono, K.; Inoue, A.; Sakurai, T. APFIM studies of nanocrystalline microstructural evolution in Fe-2%B(-Cu) amorphous alloys. Mater. Sci. Eng. A 1996, 217, 407–413. [Google Scholar] [CrossRef]
- Hono, K.; Ping, D.H.; Ohnuma, M.; Onodera, H. Cu clustering and Si partitioning in the early crystallization stage of an Fe73.5Si13.5B9Nb3Cu1amorphous alloy. Acta Mater. 1999, 47, 997–1006. [Google Scholar] [CrossRef]
- Abrosimova, G.E.; Aronin, A.S.; Zver’kova, I.I.; Kir’janov, Y.V.; Shekhtman, V.S. Nanocrystalline Ni58.5Mo31.5B10 and Ni63Mo27B10 alloys: Microstructure and its evolution at annealling. J. Surf. Investig. X Ray Synchrotron Neutron Tech. 1998, 4, 31–39. (In Russian) [Google Scholar]
- Abrosimova, G.E.; Aronin, A.S.; Zver’kova, I.I.; Kir’janov, Y.V.; Gurov, A.F. Production, structure and microhardness of nanocrystalline Ni-Mo-B alloys. Phys. Solid State 1998, 40, 8–13. [Google Scholar] [CrossRef]
- Abrosimova, G.E.; Aronin, A.S.; Kir’janov, Y.V.; Zver’kova, I.I.; Molokanov, V.V.; Alves, H.; Köster, U. The formation, structure and properties of nanocrystalline Ni-Mo-B alloys. J. Mater. Sci. 1999, 34, 1611–1618. [Google Scholar] [CrossRef]
- Hansen, M.; Anderko, K. Constitution of Binary Alloys, 2nd ed.; McGraw-Hill Book Company, Inc.: New York, NY, USA; Toronto, ON, Canada; London, UK, 1958. [Google Scholar]
- Nakarato, K.; Kawamura, Y.; Tsai, A.P.; Inoue, A. On the growth of nanocrystalline grains in an aluminum-based amorphous alloy. Appl. Phys. Lett. 1993, 63, 2644–2646. [Google Scholar] [CrossRef]
- Greer, A.L. Nanostructured Materials: Science & Technology; Chow, G.M., Ed.; NATO ASI Series; Kluwer Academical Publisher: Dordrecht, The Netherlands; London, UK, 1998; p. 457. [Google Scholar]
- Inoue, A.; Tomioke, H.; Masumoto, T. Mechanical properties of ductile Fe-Ni-Zr and Fe-Ni-Zr (Nb or Ta) amorphous alloys containing fine crystalline particles. J. Mater. Sci. 1983, 18, 153–160. [Google Scholar] [CrossRef]
- Köster, U.; Herold, U. Glassy Metals II. Atomic Structure and Dynamics, Electronicv Structure, Magnetic Propertie; Beck, H., Gűntherodt, H.-J., Eds.; Springer: Berlin, Germany, 1983. [Google Scholar]
- Abrosimova, G.E.; Aronin, A.S.; Kir’janov, Y.V. Formation and structure of nanocrystals in an Al86Ni11Yb3 alloy. Phys. Solid State 2001, 43, 2003–2011. [Google Scholar] [CrossRef]
- Köster, U.; Schunemann, M. Rapidly Solidified Alloys; Liebermann, H.H., Ed.; Marcel Dekker Inc.: New York, NY, USA, 1993; p. 303. [Google Scholar]
- Christian, J.W. The Theory of Transformation in Metals and Alloys; Part 1; Pergamon Press: Oxford, UK, 1978; ISBN 9780080542775. [Google Scholar]
- Kantrowitz, A. Nucleation in very rapid vapor expansions. J. Chem. Phys. 1951, 19, 1097–1100. [Google Scholar] [CrossRef]
- Greenwood, G.W. The Mechanism of Phase Transformations in Crystalline Solids; Inst of Metals: London, UK, 1969; p. 103. [Google Scholar]
- Galagher, P.C.J. The influence of alloying, temperature, and related effects on the stacking fault energy. Metall. Trans. 1970, 1, 2429–2461. [Google Scholar]
- Foley, J.C.; Allen, D.R.; Perepezko, J.H. Analysis of nanocrystal development in Al-Y-Fe and Al-Sm glasses. Scr. Mater. 1996, 35, 655–669. [Google Scholar] [CrossRef]
- Hono, K.; Zhang, Y.; Inoue, A.; Sakurai, T. Atom probe studies of nanocrystalline microstructural evolution in some amorphous alloys. Mater. Trans. JIM 1995, 36, 909–917. [Google Scholar] [CrossRef] [Green Version]
- Ham, F.S. Theory of diffusion-limited precipitation. J. Phys. Chem. Solids. 1958, 6, 335–351. [Google Scholar] [CrossRef]
- Allen, D.R.; Folley, J.C.; Perepezko, J.H. Nanocrystal development during primary crystallization of amorphous alloys. Acta Mater. 1998, 46, 431–440. [Google Scholar] [CrossRef]
- Howie, A.; Swann, P.R. Direct measurement of stacking-fault energies from observations of dislocation nodes. Phil. Mag. 1961, 6, 1215–1226. [Google Scholar] [CrossRef]
- Ardell, A.Y. The effect of volume fraction on particle coarsening: Theoretical considerations. Acta Metal. 1972, 20, 61–71. [Google Scholar] [CrossRef]
- Abrosimova, G.E.; Aronin, A.S.; Barkalov, O.I.; Dement’eva, M.M. Formation of the nanostructure in amorphous alloys of Al-Ni-Y system. Phys. Solid State 2013, 55, 1773–1778. [Google Scholar] [CrossRef]
- Kosevich, V.M.; Sokol, A.A. Crystal Growth from Amorphous Phase; International School on Crystal Growth: Suzdal, Russia, 1980; p. 161. (In Russian) [Google Scholar]
- Walter, J.L.; Bartram, S.R.; Mella, I. Formation and crystallization of alloys with two amorphous phases. Mat. Sci. Eng. 1978, 36, 193–205. [Google Scholar] [CrossRef]
- Vincze, I.; Kemeny, I.; Schaafsma, A.; Lovas, A.; van der Woode, F. Chemical and Topological Short-Range Order in Metallic Glasses; KFKI-90 Hungarian Academy of Sciences: Budapest, Hungary, 1980; pp. 11–15. [Google Scholar]
- O`Handley, R.C.; Hasegava, R.; Ray, R.; Chou, C.P. Ferromagnetic properties of some new metallic glasses. Appl. Phys. Lett. 1976, 29, 330–332. [Google Scholar] [CrossRef]
- Barkalov, O.I.; Aronin, A.S.; Abrosimova, G.E.; Ponyatovsky, E.G. Formation and structure evolution of the bilk amorpgous Al32Ge68 alloy on heating. J. Non Cryst. Solids 1996, 202, 266–271. [Google Scholar] [CrossRef]
- Barkalov, O.I.; Belash, I.T.; Degtyareva, V.F.; Ponyatovsky, E.G. Crystalline and amorphous state of high pressure-treated Al-Ge alloys. Fiz. Tverd. Tela 1987, 29, 1975–1978. [Google Scholar]
- Greer, A.L.; Cheng, Y.Q.; Ma, E. Shear bands in metallic glasses. Mater. Sci. Eng. R 2013, 74, 71–132. [Google Scholar] [CrossRef]
- Li, J.; Wang, Z.L.; Hufnagel, T.C. Characterization of nanometer-scale defects in metallic glasses by quantitative high-resolution transmission electron microscopy. Phys. Rev. B 2002, 65, 144201. [Google Scholar] [CrossRef] [Green Version]
- Glezer, A.; Plotnikova, M.R.; Shalimova, A.V.; Dobatkin, S.V. Megaplastic deformation of amorphous alloys. I. Structure and mechanical properties. Proc. RAS Phys. Ser. 2009, 73, 1302–1309. (In Russian) [Google Scholar]
- Stolpe, M.J.; Kruzic, J.; Busch, R. Evolution of shear bands, free volume and hardness during cold rolling of a Zr-based bulk metallic glass. Acta Mater. 2014, 64, 231–240. [Google Scholar] [CrossRef]
- Spaepen, F. A microscopic mechanism for steady state inhomogeneous flow in metallic glasses. Acta Metall. 1977, 25, 407–415. [Google Scholar] [CrossRef]
- Wang, K.; Fujita, T.; Zeng, Y.Q.; Nishiyama, N.; Inoue, A.; Chen, M.W. Micromechanisms of serrated flow in a Ni50Pd30P20 bulk metallic glass with a large compression plasticity. Acta Mater. 2008, 56, 2834–2842. [Google Scholar] [CrossRef]
- Aronin, A.; Abrosimova, G.; Matveev, D.; Rybchenko, O. Structure and Properties Of Nanocrystalline Alloys Prepared By High Pressure Torsion. Rev. Adv. Mater. Sci. 2010, 25, 52–57. [Google Scholar]
- Abrosimova, G.E.; Aronin, A.S.; Dobatkin, S.V.; Kaloshkin, S.D.; Matveev, D.V.; Rybchenko, O.G.; Tatiyanin, E.V.; Zverkova, I.I. The formation of nanocrystalline structure in amorphous Fe-Si-B alloy by severe plastic deformation. J. Metastable Nanocrystalline Mater. Ser. 2005, 24, 69–72. [Google Scholar] [CrossRef]
- Aronin, A.S.; Louzguine-Luzgin, D.V. On nanovoids formation in shear bands of an amorphous Al-based alloy. Mech. Mater. 2017, 113, 19–23. [Google Scholar] [CrossRef]
- Hebert, R.J.; Boucharat, N.; Perepezko, J.H.; Rösner, H.; Wilde, G. Calorimetric and microstructural analysis of deformation induced crystallization reactions in amorphous Al88Y7Fe5 alloy. J. Alloy. Compd. 2007, 434, 18–21. [Google Scholar] [CrossRef]
- Wilde, G.; Rösner, H. Nanocrystallization in a shear band: An in situ investigation. Appl. Phys. Lett. 2011, 98, 251904. [Google Scholar] [CrossRef]
- Abrosimova, G.; Aronin, A.; Barkalov, O.; Matveev, D.; Rybchenko, O.; Maslov, V.; Tkatch, V. Structural transformations in the Al85Ni6.1Co2Gd6Si0.9 amorphous alloy during multiple rolling. Phys. Solid State 2011, 53, 229–233. [Google Scholar] [CrossRef]
- Mazzone, G.; Montone, A.; Antisari, M.V. Effect of plastic flow on the kinetics of amorphous phase growth by solid-state reaction in the Ni-Zr system. Phys. Rev. Lett. 1990, 65, 2019–2023. [Google Scholar] [CrossRef] [PubMed]
- Yoshizawa, Y.; Oguma, S.; Yamauchi, K. New Fe-based soft magnetic alloys composed of ultrafine grain structure. J. Appl. Phys. 1988, 64, 6044–6046. [Google Scholar] [CrossRef]
- Allia, P.; Baricco, M.; Knobel, M.; Tiberto, P.; Vinai, F. Nanocrystalline Fe73.5 Cu1Nb3Si13.5B9 obtained by direct-current Joule heating. Magnetic and mechanical properties. Philos. Mag. B Phys. Condens. Matter. 1993, 68, 853–860. [Google Scholar] [CrossRef]
- Ito, N.; Suzuki, K. Improvement of magnetic softness in nanocrystalline soft magnetic materials by rotating magnetic field annealing. J. Appl. Phys. 2005, 97, 10F503. [Google Scholar] [CrossRef]
- Gheiratmand, T.H.; Hosseini, R.M. Finemet nanocrystalline soft magnetic alloy: Investigation of glass forming ability, crystallization mechanism, production techniques, magnetic softness and the effect of replacing the main constituents by other elements. J. Magn. Magn. Mater. 2016, 408, 177–192. [Google Scholar] [CrossRef]
- Takenaka, K.; Setyawanm, A.D.; Sharma, P.; Nishiyama, N.; Makino, A. Industrialization of nanocrystalline Fe–Si–B–P–Cu alloys for high magnetic flux density cores. J. Magn. Magn. Mater. 2016, 401, 479–483. [Google Scholar] [CrossRef]
- Shuvaeva, E.; Kaloshkin, S.; Churyukanova, M.; Perminov, A.; Khriplivets, I.; Mitra, A.; Panda, A.K.; Roy, R.K.; Premkumar, V.; Zhukova, A. The impact of bending stress on magnetic properties of Finemet type microwires and ribbons. J. Alloy. Compd. 2018, 743, 388–393. [Google Scholar] [CrossRef]
- Mikhalitsyna, E.A.; Kataev, V.A.; Larrañaga, A.; Lepalovskij, V.N.; Kurlyandskaya, G.V. Nanocrystallization in FINEMET-Type Fe73.5Nb3Cu1Si13.5B9 and Fe72.5Nb1.5Mo2Cu1.1Si14.2B8.7 thin films. Materials 2020, 13, 348. [Google Scholar] [CrossRef] [Green Version]
- Louzguine, D.V.; Inoue, A. Comparative study of the effect of cold rolling on the structure of Al–RE–Ni–Co (RE = rare-earth metals) amorphous and glassy alloys. J. Non Cryst. Solids 2006, 352, 3903–3909. [Google Scholar] [CrossRef]
- Kovács, Z.; Henits, P.; Hobor, S.; Révész, A. Nanocrystallization process in amorphous alloys during severe plastic deformation and thermal treatments. Rev. Adv. Mater. Sci. 2008, 18, 593–596. [Google Scholar]
- Tkach, V.I.; Rassolov, S.G.; Popov, V.V.; Maksimov, V.V.; Maslov, V.V.; Nosenko, V.K.; Aronin, A.S.; Abrosimova, G.E.; Rybchenko, O.G. Complex crystallization mode of amorphous/nanocrystalline composite Al86Ni2Co5.8Gd5.7Si0.5. J. Non Cryst. Solids 2011, 357, 1628–1631. [Google Scholar] [CrossRef]
- Louzguine-Luzgin, D.V.; Bazlov, A.I.; Ketov, S.V.; Inoue, A. Crystallization behavior of Fe- and Co-based bulk metallic glasses and their glass-forming ability. Mater. Chem. Phys. 2015, 162, 197–206. [Google Scholar] [CrossRef]
- Herold, U.; Kőster, U. Metastabile Phasen in extreme schnell erstarrten Eisen-Bor-Legierungen. Z Metallk. 1978, 69, 326–332. [Google Scholar]
- Khan, Y.; Sostarich, M. Dynamic temperature X-ray diffraction analysis of amorphous Fe80B20. Z Metallk. 1981, 72, 266–268. [Google Scholar]
- Abrosimova, G.E.; Aronin, A.S.; Gantmakher, V.F.; Levin, Y.B.; Osherov, M.V. Variation of the electric resistivity of the amorphous Ni-Zr alloy in the initial-stages of crystallization. Fiz. Tverd. Tela 1988, 30, 1424–1430. [Google Scholar]
- Abrosimova, G.E.; Aronin, A.S. Phase transformation in Fe-B alloys at heating. Metallofizika 1988, 10, 47–52. (In Russian) [Google Scholar]
- Abrosimova, G.; Matveev, D.; Pershina, E.; Aronin, A. Effect of treatment conditions on parameters of nanocrystalline structure in Al-based alloys. Mater. Lett. 2016, 183, 131–134. [Google Scholar] [CrossRef]
- Aronin, A.; Matveev, D.; Pershina, E.; Tkatch, V.; Abrosimova, G. The effect of changes in Al-based amorphous phase structure on structure forming upon crystallization. J. Alloy. Compd. 2017, 715, 176–183. [Google Scholar] [CrossRef]
- Aronin, A.; Budchenko, A.; Matveev, D.; Pershina, E.; Tkatch, V.; Abrosimova, G. Nanocrystal formation in light metallic glasses at heating and deformation. Rev. Adv. Mater. Sci. 2016, 46, 53–69. [Google Scholar]
- Boucharat, N.; Hebert, R.; Rösner, H.; Valiev, R.; Wilde, G. Nanocrystallization of amorphous Al88Y7Fe5 alloy induced by plastic deformation. Scr. Mater. 2005, 53, 823–828. [Google Scholar] [CrossRef]
- Valiev, R.Z.; Alexandrov, I.V. Bulk Nanocrystalline Metallic Materials; Akademkniga: Moscow, Russia, 2007; p. 398. (In Russian) [Google Scholar]
- Kovacs, Z.; Henits, P.; Zhilyaev, A.P.; Revesz, A. Deformation induced primary crystallization in a thermally non-primary crystallizing amorphous Al85Ce8Ni5Co2 alloy. Scr. Mater. 2006, 54, 1733–1737. [Google Scholar] [CrossRef]
- Boucharat, N.; Hebert, R.; Rösner, H.; Valiev, R.; Wilde, G. Synthesis routes for controlling the microstructure in nanostructured Al88Y7Fe5 alloys. J. Alloy. Compd. 2007, 434, 252–254. [Google Scholar] [CrossRef]
- Aronin, A.; Abrosimova, G.; Matveev, D.; Pershina, E. Nanocrystal formation, structure and magnetic properties of Fe-Si-B amorphous alloy after deformation. Mater. Lett. 2013, 97, 15–17. [Google Scholar] [CrossRef]
- Lewandowski, J.J.; Greer, A.L. Temperature rise at shear bands in metallic glasses. Nat. Mater. 2006, 5, 15–18. [Google Scholar] [CrossRef]
- Csontos, A.A.; Shiflet, G.J. Formation and chemistry of nanocrystalline phases formed during deformation in aluminum-rich metallic glasses. Nano Struct. Mater. 1997, 9, 281–289. [Google Scholar] [CrossRef]
- Georgarakis, K.; Aljerf, M.; Li, Y.; LeMoulec, A.; Charlot, F.; Yavari, A.R.; Chornokhvostenko, K.; Tabachnikova, E.; Evangelakis, G.A.; Miracle, D.B.; et al. Shear band melting and serrated flow in metallic glasses. Appl. Phys. Lett. 2008, 93, 031907. [Google Scholar] [CrossRef]
- Hartley, K.A.; Duffy, J.; Hawley, R.H. Measurement of the temperature profile during shear band formation in steels deforming at high strain rates. J. Mech. Solids. 1987, 35, 283–301. [Google Scholar] [CrossRef] [Green Version]
- Li, J.G.; Umemoto, M.; Todaka, Y.; Fujisaku, K.; Tsuchiya, K. The dynamic phase transformation and formation of nanocrystalline structure in SUS304 austenitic stainless steel subjected to high pressure torsion. Rev. Adv. Mater. Sci. 2008, 18, 577–582. [Google Scholar]
- Jiang, W.H.; Atzmon, M. The effect of compression and tension on shear-band structure and nanocrystallization in amorphous Al90Fe5Gd5: A high-resolution transmission electron microscopy study. Acta Mater. 2003, 51, 4095–4105. [Google Scholar] [CrossRef]
- Kim, J.J.; Choi, Y.; Suresh, S.; Argon, A.S. Nanocrystallization during nanoindentation of a bulk Amorphous metal alloy at room temperature. Science 2002, 295, 654–656. [Google Scholar] [CrossRef] [PubMed]
- Rösner, H.; Peterlechler, M.; Kűbel, C.; Schmidt, V.; Wilde, G. Density changes in shear bands of a metallic glass determined by correlative analytical transmission electron microscopy. Ultramicroscopy 2014, 142, 1–9. [Google Scholar] [CrossRef] [PubMed]
- Schmidt, V.; Rösner, H.; Peterlechler, M.; Wilde, G. Quantitative measurement of density in a shear band of metallic glass monitored along its propagation direction. Phys. Rev. Lett. 2015, 115, 035501. [Google Scholar] [CrossRef] [PubMed]
- Slaughter, S.K.; Kertis, F.; Deda, E.; Gu, X.; Wright, W.J.; Hufnagel, T.C. Shear bands in metallic glasses are not necessarily hot. APL Mater. 2014, 2, 096110. [Google Scholar] [CrossRef] [Green Version]
- Abrosimova, G.; Aronin, A. Nanocrystal formation in Al- and Ti-based amorphous alloys at Deformation. J. Alloy. Compd. 2018, 747, 26–30. [Google Scholar] [CrossRef]
- Yoo, B.G.; Kim, Y.J.; Oh, J.H.; Ramamurty, U.; Jang, J. On the hardness of shear bands in amorphous alloys. Scr. Mater. 2009, 61, 951–954. [Google Scholar] [CrossRef]
- Pan, J.; Chen, Q.; Liu, L.; Li, Y. Softening and dilatation in a single shear band. Acta Mater. 2011, 59, 5146–5158. [Google Scholar] [CrossRef]
- Maaß, R.; Samwer, K.; Arnold, W.; Volkert, C.A. A single shear band in a metallic glass: Local core and wide soft one. Appl. Phys. Lett. 2014, 10, 17190. [Google Scholar] [CrossRef] [Green Version]
- Abrosimova, G.; Aronin, A.; Fokin, D.; Orlova, N.; Postnova, E. The decrease of Young’s modulus in shear bands of amorphous Al87Ni8Gd5 alloy. Mater. Lett. 2019, 252, 114–116. [Google Scholar] [CrossRef]
- Perepezko, J.H. Nucleation-controlled reactions and metastable structures. Prog. Mater. Sci. 2004, 49, 263–284. [Google Scholar] [CrossRef]
- Mu, J.; Fu, H.; Zhu, Z.; Wang, A.; Li, H.; Hu, Z.Q.; Zhang, H. Synthesis and properties of Al-Ni-La bulk metallic glass. Adv. Eng. Mater. 2009, 11, 530–532. [Google Scholar] [CrossRef]
- Yang, B.J.; Yao, J.H.; Chao, Y.S.; Wang, J.Q.; Ma, E. Developing aluminum-based bulk metallic glasses. Phil. Mag. 2010, 90, 3215–3231. [Google Scholar] [CrossRef]
- Du, S.Z.; Li, C.C.; Pang, S.Y.; Leng, J.F.; Geng, H.R. Influences of melt superheat treatment on glass forming ability and properties of Al84Ni10La6 alloy. Mater. Des. 2013, 47, 358–364. [Google Scholar] [CrossRef]
- Chunchu, V.J.; Markandeyulu, G. Magnetoimpedance studies in as quenched Fe73.5Si13.5B8CuV3-xAlNbx nanocrystalline ribbons. Appl. Phys. 2013, 113, 17A321. [Google Scholar] [CrossRef]
- Xiang, R.; Zhou, S.; Dong, B.; Zhang, G.; Li, Z.; Wang, Y.; Chang, C. Effect of Co addition on crystallization and magnetic properties of FeSiBPC alloys. Progr. Nat. Sci. Mater. Intern. 2014, 24, 649–654. [Google Scholar] [CrossRef] [Green Version]
- Meng, F.; Tsuchija, K.; Yokoyama, Y. Reversible transition of deformation mode by structural rejuvenation and relaxation in bulk metallic glass. Appl. Phys. Lett. 2012, 101, 121914. [Google Scholar] [CrossRef]
- Tong, Y.; Iwashita, T.; Dmowski, W.; Bei, H.; Yokoyama, Y.; Egami, T. Structural rejuvenation in bulk metallic glasses. Acta Mater. 2015, 86, 240–246. [Google Scholar] [CrossRef] [Green Version]
- Dmowski, W.; Yokoyama, Y.; Chuang, A.; Ren, Y.; Umemoto, M.; Tsuchiya, K.; Inoue, A.; Egami, T. Structural rejuvenation in a bulk metallic glass induced by severe plastic deformation. Acta Mater. 2010, 58, 429–438. [Google Scholar] [CrossRef]
- Tong, Y.; Dmowski, W.; Bei, H.; Yokoyama, Y.; Egami, T. Mechanical rejuvenation in bulk metallic glass induced by thermo-mechanical creep. Acta Mater. 2018, 148, 384–390. [Google Scholar] [CrossRef]
- Guo, W.; Yamada, R.; Saida, J. Rejuvenation and plasticization of metallic glass by deep cryogenic cycling. Intermetallics 2018, 93, 141–147. [Google Scholar] [CrossRef]
- Ketov, S.V.; Sun, Y.H.; Nachum, S.; Lu, Z.; Checchi, A.; Beraldin, A.R.; Bai, H.Y.; Wang, W.H.; Louzguine-Luzgin, D.V.; Carpenter, M.A.; et al. Rejuvenation of metallic glasses by non-affine thermal strain. Nature 2015, 524, 200–203. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hufnagel, T.C. Cryogenic rejuvenation. Nat. Mater. 2015, 14, 87–868. [Google Scholar] [CrossRef] [PubMed]
- Bian, X.; Wang, G.; Wang, Q.; Sun, B.A.; Hussain, I.; Zhai, Q.J.; Mattern, N.; Bednarcik, J.; Eckert, J. Cryogenic-temperature-induced structural transformation of a metallic glass. Mater. Res. Lett. 2017, 5, 284–291. [Google Scholar] [CrossRef] [Green Version]
- Guo, W.; Shao, Y.M.; Saida, J.; Zhao, M.; Lu, S.L.; Wu, S.S. Rejuvenation and plasticization of Zr-based bulk metallic glass with various Ta content upon deep cryogenic cycling. J. Alloy. Compd. 2019, 795, 314–318. [Google Scholar] [CrossRef]
- Guo, W.; Saida, J.; Zhao, M.; Lu, S.; Wu, S. Rejuvenation of Zr-based bulk metallic glass matrix composite upon deep cryogenic cycling. Mater. Lett. 2019, 247, 135–138. [Google Scholar] [CrossRef]
- Kang, S.J.; Cao, Q.P.; Liu, J.; Tang, Y.; Wang, X.D.; Zhang, D.X.; Ahn, I.S.; Caron, A.; Jiang, J.Z. Intermediate structural state for maximizing the rejuvenation effect inmetallic glass via thermo-cycling treatment. J. Alloy. Compd. 2019, 795, 493–500. [Google Scholar] [CrossRef]
- Abrosimova, G.; Volkov, N.; Van Tuan, T.; Pershina, E.A.; Aronin, A.S. Cryogenic rejuvenation of Al-based amorphous-nanocrystalline alloys. Mater. Lett. 2019, 240, 150–152. [Google Scholar] [CrossRef]
- Abrosimova, G.; Volkov, N.; Van Tuan, T.; Pershina, E.A.; Aronin, A.S. Amorphous structure rejuvenation under cryogenic treatment of Al-based amorphous-nanocrystalline alloys. J. Non Cryst. Solids 2020, 528, 119751. [Google Scholar] [CrossRef]
- Abrosimova, G.E.; Aronin, A.S. Reversible structure changes in amorphous Fe-B alloys. Int. J. Rapid Solidif. 1991, 6, 29–40. [Google Scholar]
- Abrosimova, G.; Aronin, A.; Ignatieva, E. Phase transformation in the Ni70Mo10P20 amorphous alloy at heating. Phys. Met. Metallogr. 2003, 95, 569–574. [Google Scholar]
- Abrosimova, G.; Aronin, A.; Ignatieva, E. A metastable phase forming during crystallization of an amorphous Ni70Mo10P20 alloy. Phys. Solid State 2006, 48, 122–128. [Google Scholar] [CrossRef]
- Abrosimova, G.; Aronin, A.; Ignatieva, E. Mechanism of crystallization of the Ni70Mo10B20 alloy above the glass transition temperature. Phys. Solid State 2006, 48, 563–569. [Google Scholar] [CrossRef]
- Abrosimova, G.; Aronin, A.; Kabanov, Y.P.; Matveev, D.V.; Molokanov, V.V. Magnetic structure and properties of a bulk Fe72Al5P10Ga2C6B4Si1 alloy in the amorphous and nanocrystalline states. Phys. Solid State 2004, 46, 885–890. [Google Scholar] [CrossRef]
- Abrosimova, G.; Aronin, A.; Kabanov, Y.P.; Matveev, D.V.; Molokanov, V.V.; Rybchenko, O.G. Effect of thermal treatment on the microstructure and magnetic properties of a bulk amorphous Fe72Al5P10Ga2C6B4Si1 alloy. Phys. Solid State 2004, 46, 2232–2237. [Google Scholar] [CrossRef]
- Masumoto, T.; Waseda, Y.; Kimura, H.; Inoue, A. Thermal instability and crystallization characteristics of amorphous metal-metalloid system. Sci. Rep. Res. Inst. Tohoku Univ. 1976, 26, 21–35. [Google Scholar]
- Aronin, A.; Ivanov, A.S.; Yakshin, A.E. Increase Of The Near-Surface Crystallization Temperature In A Fe-B-P Amorphous Alloy. Phys. Solid State 1991, 33, 2527–2532. [Google Scholar]
















| A Structure before the Crystallization Onset | After the First Stage of | Crystallization |
|---|---|---|
| D, nm | f, % | |
| Al87Ni8Y5 | - | |
| The uniform amorphous phase | 23 | 18 |
| The non-uniform amorphous phase after heat treatment | 15 | 20 |
| The non-uniform amorphous phase after rolling deformation | 13 | 25 |
| Al87Ni8La5 | - | |
| The uniform amorphous phase | 32 | 12 |
| The non-uniform amorphous phase after heat treatment | 30 | 13 |
| The non-uniform amorphous phase after rolling deformation | 26 | 14 |
| Al87Ni8Gd5 | - | |
| The uniform amorphous phase | 26 | 23 |
| The non-uniform amorphous phase after heat treatment | 24 | - |
| The non-uniform amorphous phase after rolling deformation | 21 | - |
| HPT 1 rev | 6 | 22 |
| HPT 5 rev | 6 | 25 |
| Al88Ni10Y2 | - | |
| The uniform amorphous phase | 19 | 28 |
| The non-uniform amorphous phase after rolling deformation | 18 | 35 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Aronin, A.; Abrosimova, G. Specific Features of Structure Transformation and Properties of Amorphous-Nanocrystalline Alloys. Metals 2020, 10, 358. https://doi.org/10.3390/met10030358
Aronin A, Abrosimova G. Specific Features of Structure Transformation and Properties of Amorphous-Nanocrystalline Alloys. Metals. 2020; 10(3):358. https://doi.org/10.3390/met10030358
Chicago/Turabian StyleAronin, Alexandr, and Galina Abrosimova. 2020. "Specific Features of Structure Transformation and Properties of Amorphous-Nanocrystalline Alloys" Metals 10, no. 3: 358. https://doi.org/10.3390/met10030358
APA StyleAronin, A., & Abrosimova, G. (2020). Specific Features of Structure Transformation and Properties of Amorphous-Nanocrystalline Alloys. Metals, 10(3), 358. https://doi.org/10.3390/met10030358





