Effect of Sulfur Content on the Composition of Inclusions and MnS Precipitation Behavior in Bearing Steel
Abstract
:1. Introduction
2. Materials and Methods
3. Results and Discussion
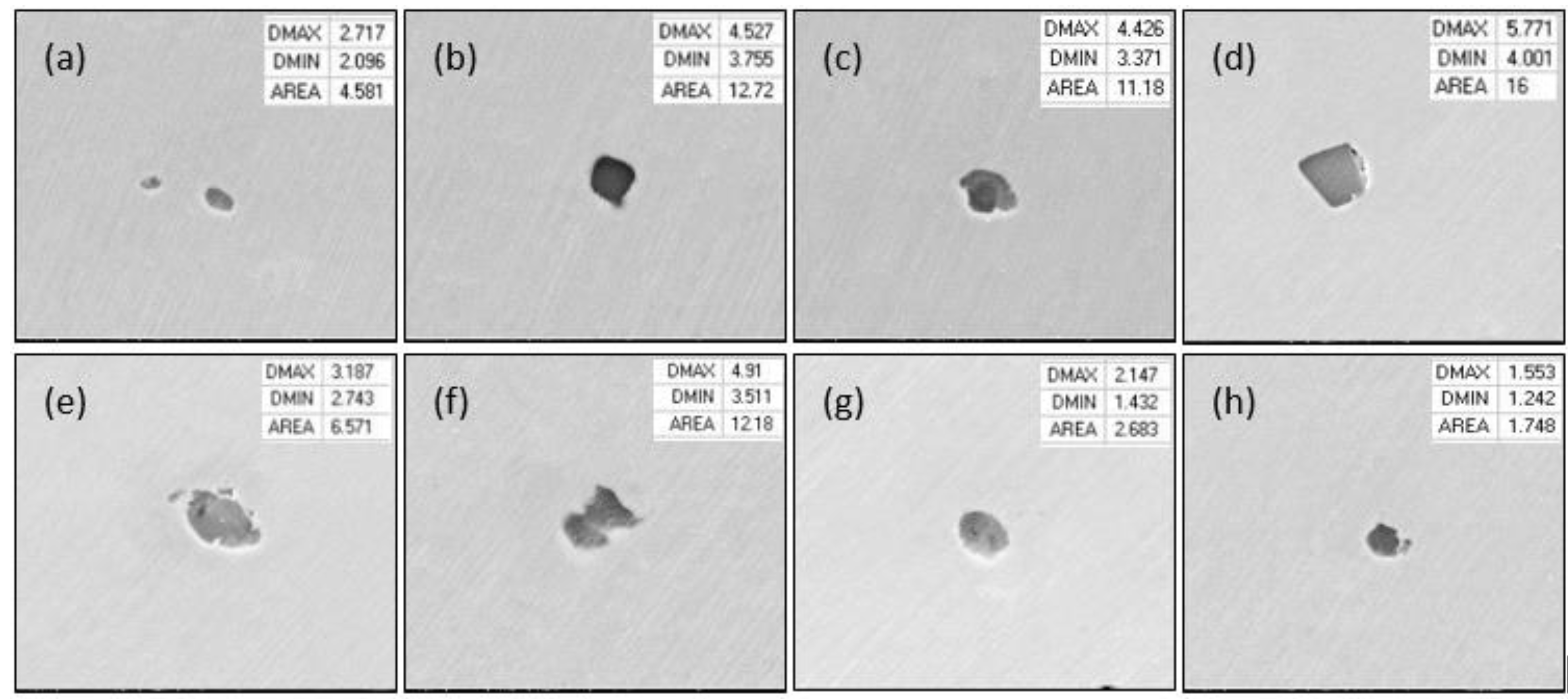
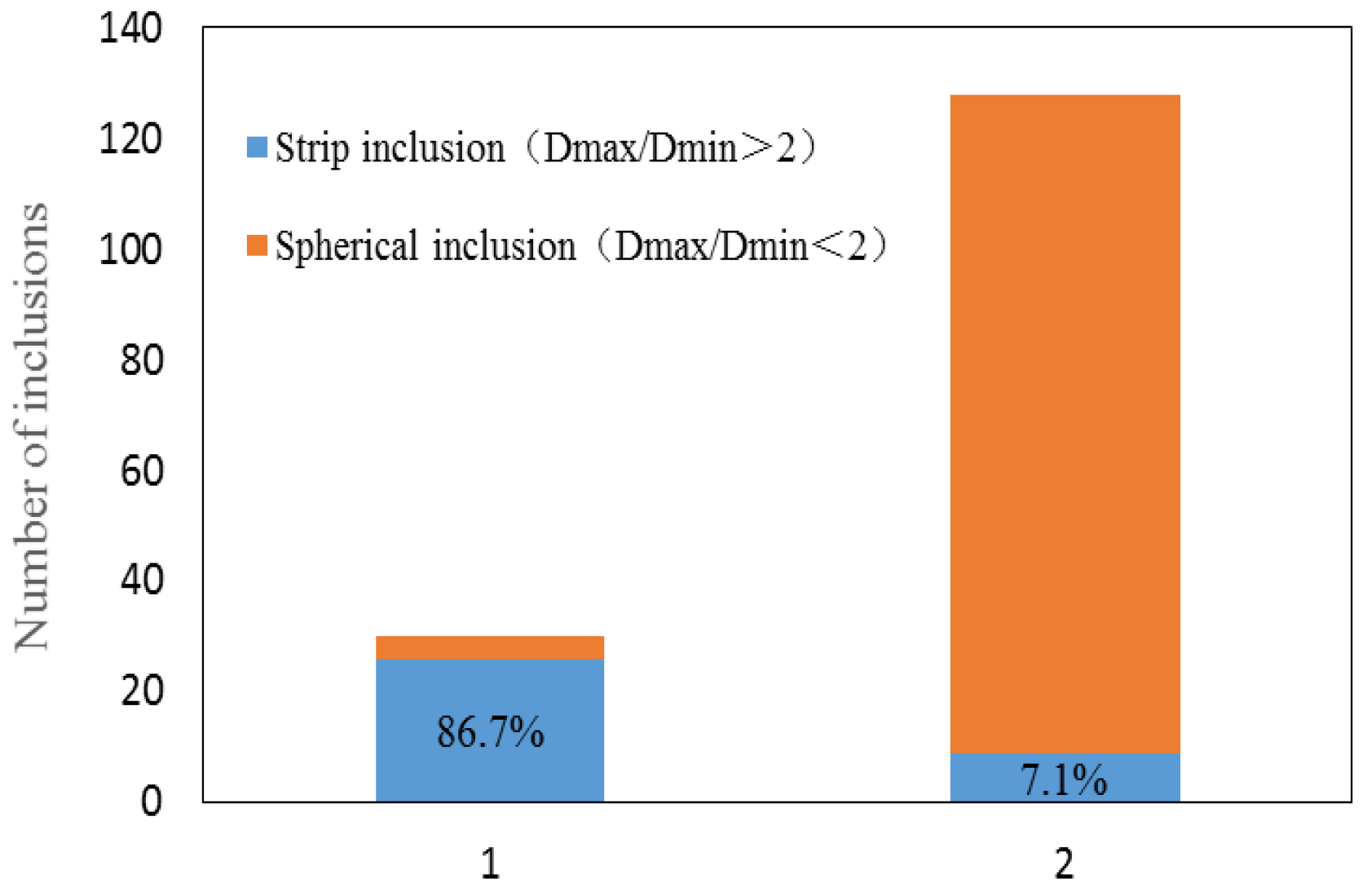
3.1. Morphology of Inclusions in Bearing Steel with Different Sulfur Contents
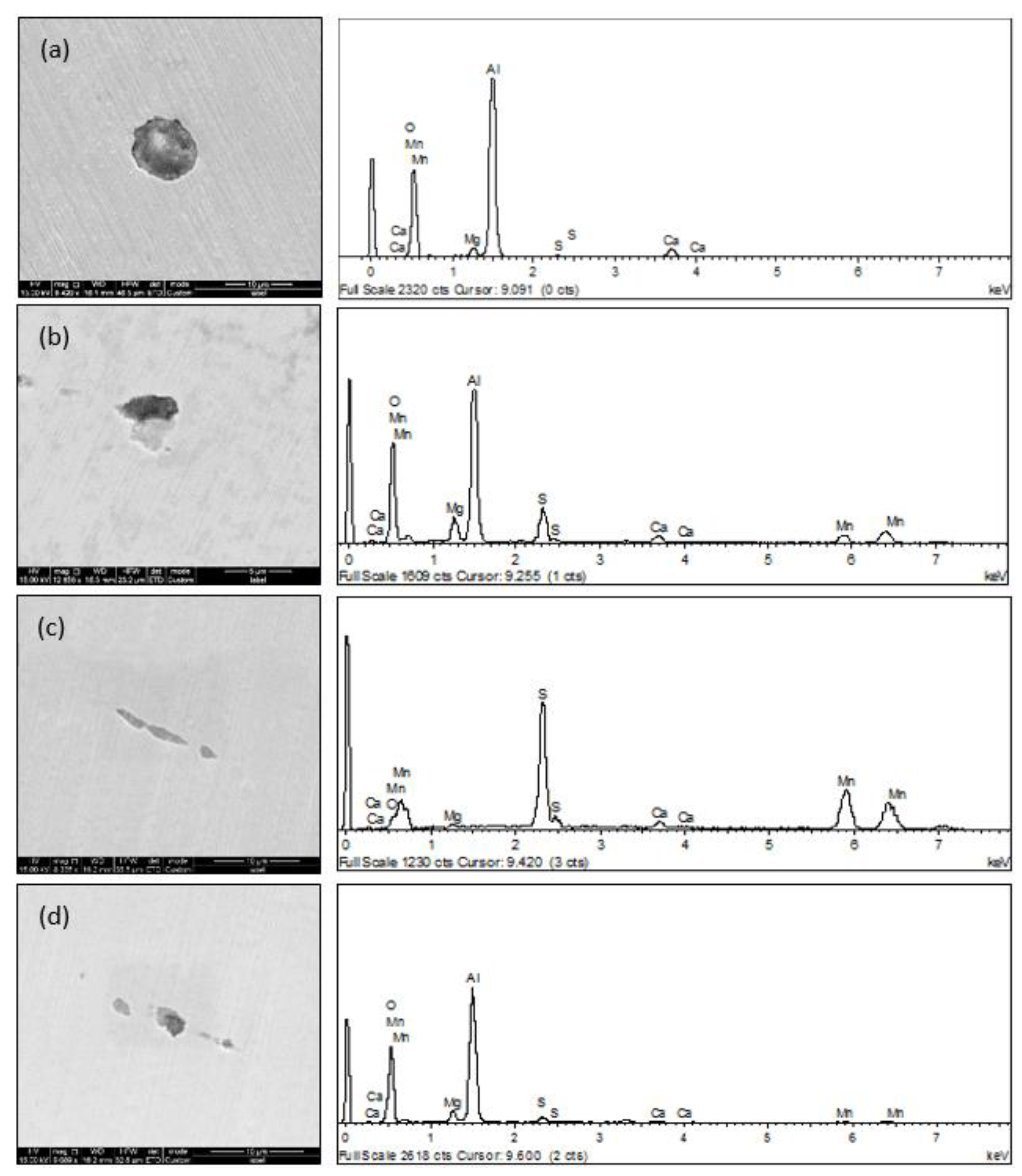
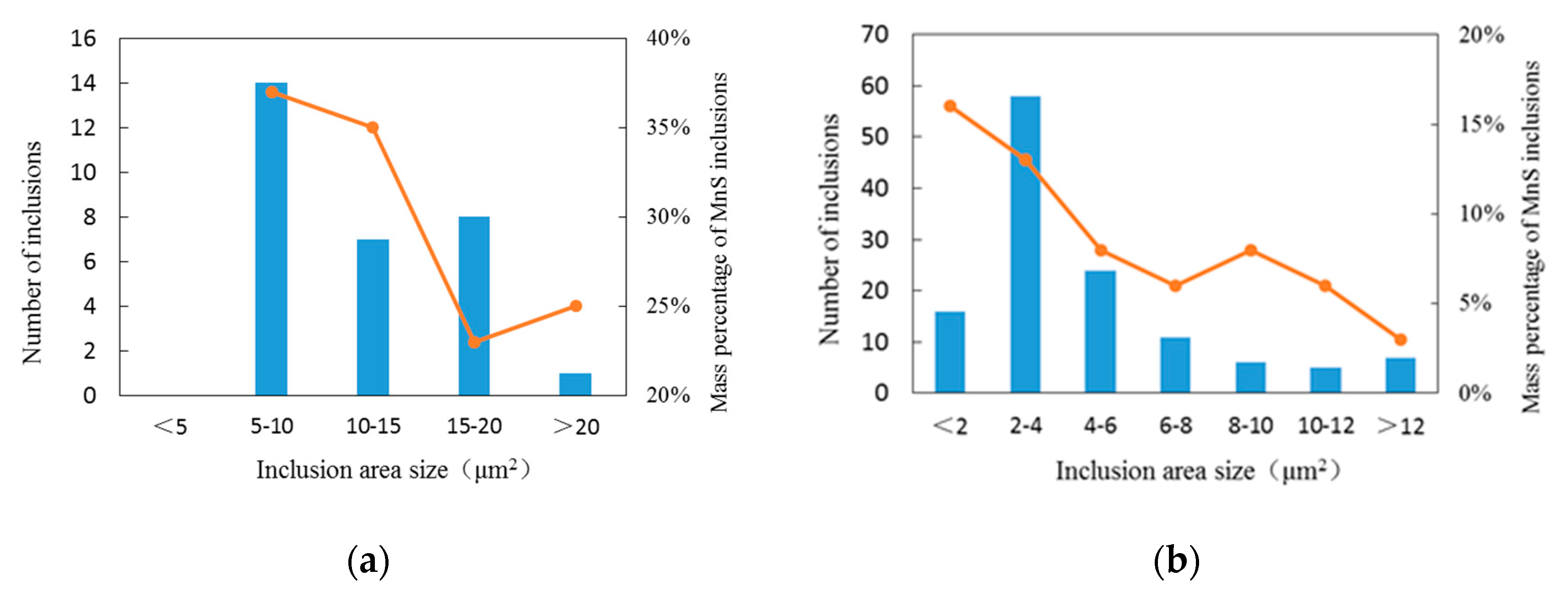
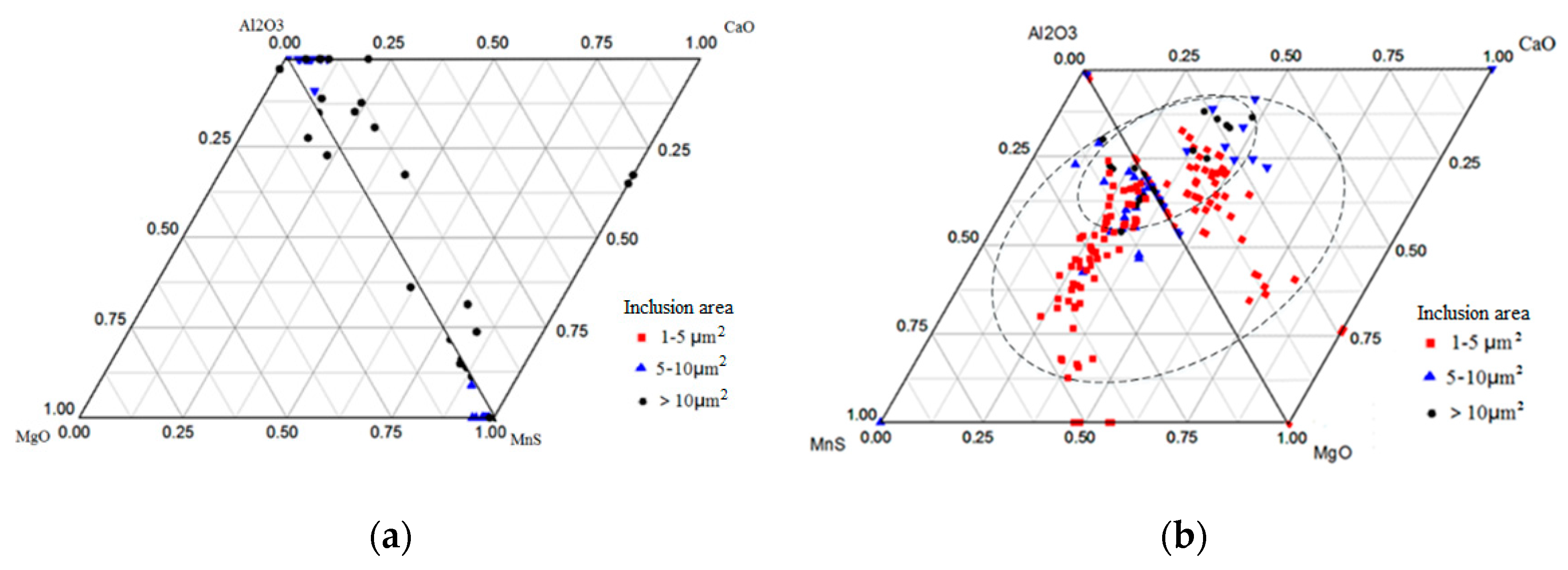
3.2. Relationship between Size and Composition of Inclusions in Bearing Steel
3.3. Thermodynamic Calculation of Al2O3–CaO–MgO–MnS with Different MnS Contents
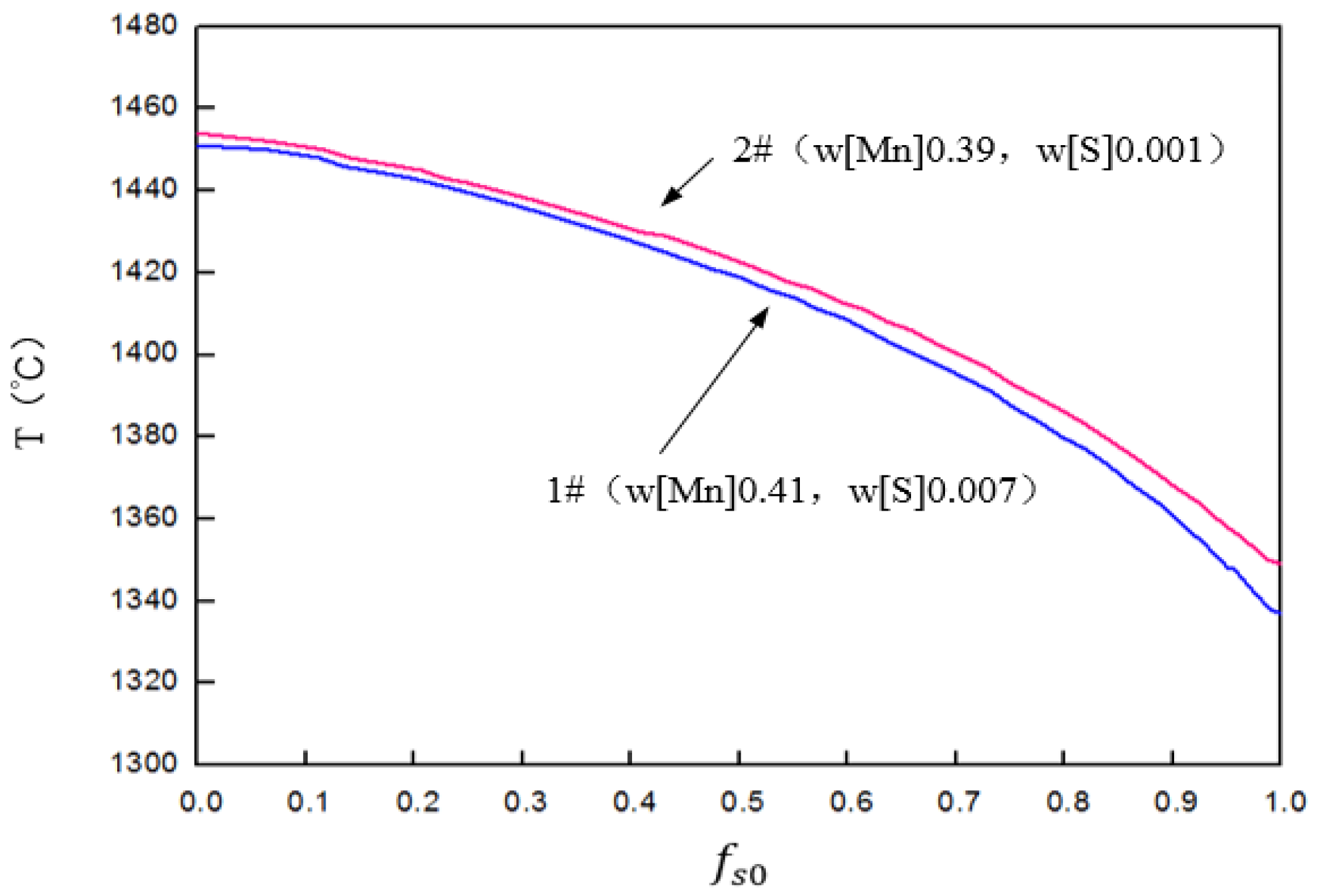
3.4. Thermodynamic Analysis of MnS Precipitation Behavior of Bearing Steel
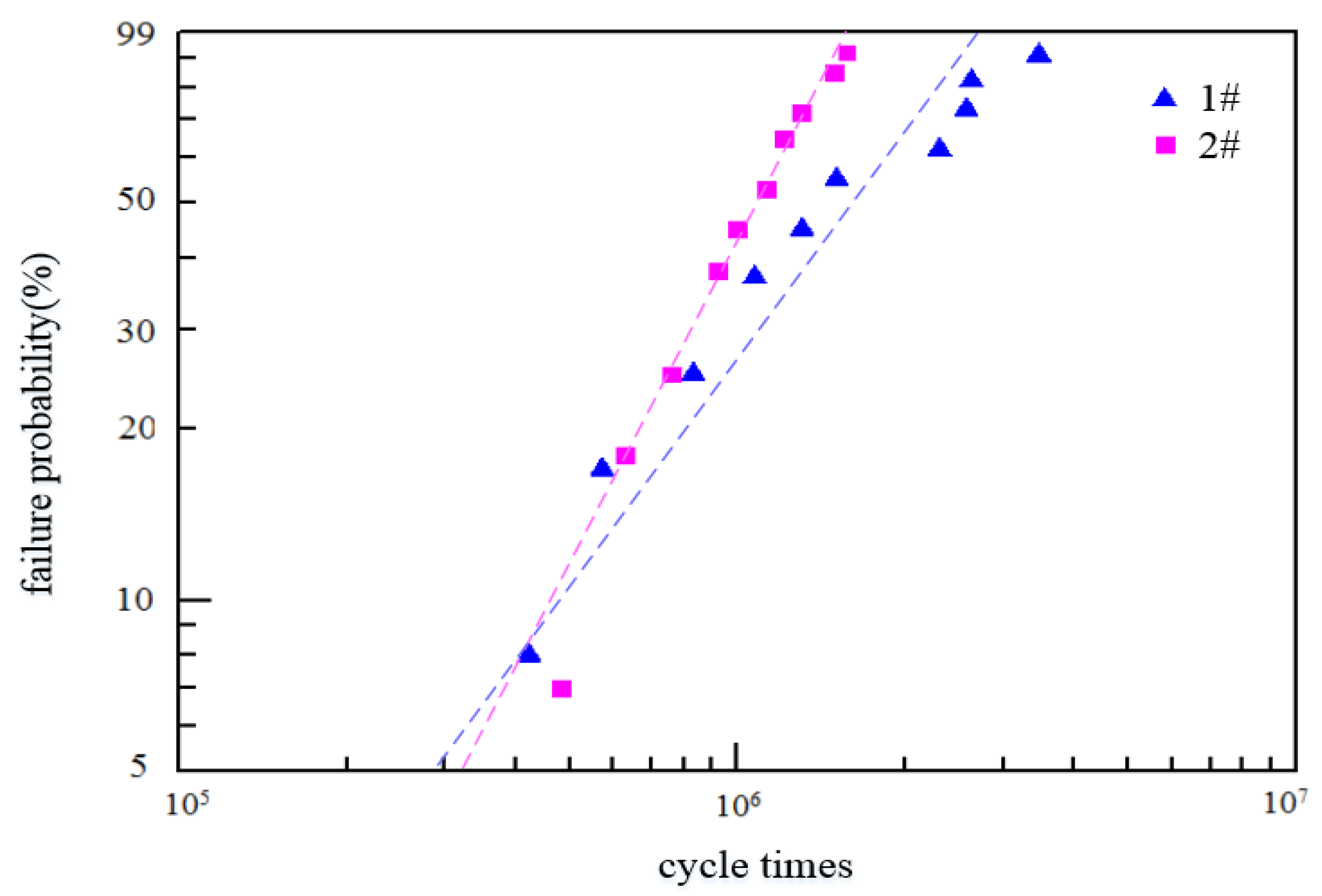
3.5. Fatigue Properties of Bearing Steel with Different Sulfur Contents
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- E Silva, A.C. Non-metallic inclusions in steels–origin and control. J. Mater. Res. Technol. 2018, 3, 283–299. [Google Scholar] [CrossRef]
- Ånmark, N.; Karasev, A.; Jönsson, P.G. The effect of different non-metallic inclusions on the machinability of steels. Materials 2015, 8, 751–783. [Google Scholar] [CrossRef] [PubMed]
- Xiao, G.H.; Dong, H.; Wang, M.Q.; Hui, W.J. Effect of sulfur content and sulfide shape on fracture ductility in case hardening steel. J. Iron Steel Res. Int. 2011, 18, 58–64. [Google Scholar] [CrossRef]
- Nakayama, T.; Honjou, N.; Minaga, T.; Yashiki, H. Effects of manganese and sulfur contents and slab reheating temperatures on the magnetic properties of non-oriented semi-processed electrical steel sheet. J. Magn. Magn. Mater. 2001, 234, 55–61. [Google Scholar] [CrossRef]
- Domizzi, G.; Anteri, G.; Ovejero-Garcıa, J. Influence of sulphur content and inclusion distribution on the hydrogen induced blister cracking in pressure vessel and pipeline steels. Corros. Sci. 2001, 43, 325–339. [Google Scholar] [CrossRef]
- Lijie, Y.; Longmei, W.; Jinsheng, H. Effects of rare earth on inclusions and corrosion resistance of 10PCuRE weathering steel. J. Rare Earths 2010, 28, 952–956. [Google Scholar]
- Lin, S.-G.; Yang, H.-H.; Su, Y.-H.; Chang, K.-L.; Yang, C.-H.; Lin, S.-K. CALPHAD-assisted morphology control of manganese sulfide inclusions in free-cutting steels. J. Alloy. Compd. 2019, 779, 844–855. [Google Scholar] [CrossRef]
- Nagels, E.; Duflou, J.R.; Van Humbeeck, J. The influence of sulphur content on the quality of laser cutting of steel. J. Mater. Process. Technol. 2007, 194, 159–162. [Google Scholar] [CrossRef]
- Hashimoto, K.; Fujimatsu, T.; Tsunekage, N.; Hiraoka, K.; Kida, K.; Santos, E.C. Study of rolling contact fatigue of bearing steels in relation to various oxide inclusions. Mater. Des. 2011, 32, 1605–1611. [Google Scholar] [CrossRef]
- Kim, D.; Han, K.; Lee, B.; Han, I.; Park, J.H.; Lee, C. Oxide formation mechanisms in high manganese steel welds. Metall. Mater. Trans. A 2014, 45, 2046–2054. [Google Scholar] [CrossRef]
- Xiao, W.; Wang, M.; Bao, Y. The Research of Low-Oxygen Control and Oxygen Behavior during RH Process in Silicon-Deoxidization Bearing Steel. Metals 2019, 9, 812. [Google Scholar] [CrossRef] [Green Version]
- Gu, C.; Lian, J.; Bao, Y.; Xie, Q.; Münstermann, S. Microstructure-based fatigue modelling with residual stresses: Prediction of the fatigue life for various inclusion sizes. Int. J. Fatigue 2019, 129, 105158. [Google Scholar] [CrossRef]
- Sadeghi, F.; Jalalahmadi, B.; Slack, T.S.; Raje, N.; Arakere, N.K. A Review of Rolling Contact Fatigue. J. Tribol. 2009, 131, 041403. [Google Scholar] [CrossRef]
- Gu, C.; Wang, M.; Bao, Y.; Wang, F.-M.; Lian, J. Quantitative analysis of inclusion engineering on the fatigue property improvement of bearing steel. Metals 2019, 9, 476. [Google Scholar] [CrossRef] [Green Version]
- Zhang, X.; Yang, S.; Li, J.; Wu, J. Transformation of oxide inclusions in stainless steel containing yttrium during isothermal heating at 1473 K. Metals 2019, 9, 961. [Google Scholar] [CrossRef] [Green Version]
- Wang, F.; Guo, H.; Liu, W.; Yang, S.; Zhang, S.; Li, J. Control of MnS inclusions in high-and low-sulfur steel by tellurium treatment. Materials 2019, 12, 1034. [Google Scholar] [CrossRef] [Green Version]
- Shin, J.H.; Park, J.H. Formation mechanism of oxide-sulfide complex inclusions in high-sulfur-containing steel melts. Metall. Mater. Trans. B 2018, 49, 311–324. [Google Scholar] [CrossRef]
- Doostmohammadi, H.; Jönsson, P.; Komenda, J.; Hagman, S. Inclusion characteristics of bearing steel in a runner after ingot casting. Steel Res. Int. 2010, 81, 142–149. [Google Scholar] [CrossRef]
- You, D.; Michelic, S.; Bernhard, C.; Loder, D.; Wieser, G. Modeling of inclusion formation during the solidification of steel. ISIJ Int. 2016, 56, 1770–1778. [Google Scholar] [CrossRef] [Green Version]
- Chen, P.-J.; Zhu, C.-Y.; Li, G.; Dong, Y.-W.; Zhang, Z.-C. Effect of sulphur concentration on precipitation behaviors of MnS-containing inclusions in GCr15 bearing steels after LF refining. ISIJ Int. 2017, 57, 1019–1028. [Google Scholar] [CrossRef] [Green Version]
- Drar, H. Metallographic and fractographic examination of fatigue loaded PM-steel with and without MnS additive. Mater. Charact. 2000, 45, 211–220. [Google Scholar] [CrossRef]
- Scurria, M.; Emre, S.; Möller, B.; Wagener, R.; Melz, T. Evaluation of the influence of MnS in forged steel 38MnVS6 on fatigue life. SAE Int. J. Engines 2017, 10, 366–372. [Google Scholar] [CrossRef]
- Wang, J.; Ren, Q.; Luo, Y.; Zhang, L. Effect of non-metallic precipitates and grain size on core loss of non-oriented electrical silicon steels. J. Magn. Magn. Mater. 2018, 451, 454–462. [Google Scholar] [CrossRef]
- Bhadeshia, H.K.D.H. Steels for bearings. Prog. Mater. Sci. 2012, 57, 268–435. [Google Scholar] [CrossRef]
- Tian, C.; Liu, J.-H.; Lu, H.-C.; Dong, H. Estimation of maximum inclusion by statistics of extreme values method in bearing steel. J. Iron Steel Res. Int. 2017, 24, 1131–1136. [Google Scholar] [CrossRef]
- Zhang, J.; Wang, F.; Li, C. Thermodynamic analysis of the compositional control of inclusions in cutting-wire steel. Int. J. Miner. Metall. Mater. 2014, 21, 647–653. [Google Scholar] [CrossRef]
- Ghosh, A. Thermodynamic evaluation of formation of oxide–sulfide duplex inclusions in steel. ISIJ Int. 2008, 48, 1552–1559. [Google Scholar]
- Huang, X.H. The Principle of Ferrous Metallurgy, 4th ed.; Metallurgical Industry Press: Beijing, China, 2005; p. 181. [Google Scholar]
- Choudhary, S.K.; Ghosh, A. Fundamentals of high temperature processes-mathematical model for prediction of composition of inclusions formed during solidification of liquid steel. ISIJ Int. 2009, 49, 1819. [Google Scholar] [CrossRef] [Green Version]
- Gao, Y.; Han, H.; Zhang, X. Measurement of P-S-N curve of contact fatigue of specially strengthened GCr15 steel ball. Bearings 2005, 8, 27–28. [Google Scholar]
- Gao, S.; Wang, G.; Qu, S. Research on contact fatigue performance of bearing steel. Mech. Eng. Autom. 2014, 184, 105–107. [Google Scholar]
- Yang, C.; Luan, Y.; Li, D.; Li, Y. Very high cycle fatigue properties of bearing steel with different aluminum and sulfur content. Int. J. Fatigue 2018, 116, 396–408. [Google Scholar] [CrossRef]
- Yang, C.; Liu, P.; Luan, Y.; Li, D.; Li, Y. Study on transverse-longitudinal fatigue properties and their effective-inclusion-size mechanism of hot rolled bearing steel with rare earth addition. Int. J. Fatigue 2019, 128, 105193. [Google Scholar] [CrossRef]






| Elements | C | Si | Mn | P | S | Cr | Mo | Ti | Al | O |
|---|---|---|---|---|---|---|---|---|---|---|
| 1# | 0.99 | 0.25 | 0.41 | 0.01 | 0.007 | 1.35 | 0.019 | 0.001 | 0.015 | 0.0002 |
| 2# | 0.96 | 0.27 | 0.39 | 0.009 | 0.001 | 1.57 | 0.01 | 0.002 | 0.014 | 0.0006 |
| Inclusion Composition | TiN | MnS | Complex Inclusion with MnS | No MnS Complex Inclusion | Total Number of Inclusions | Percentage of MnS Inclusions |
|---|---|---|---|---|---|---|
| 1# | 0 | 7 | 22 | 1 | 30 | 96.7% |
| 2# | 2 | 0 | 104 | 22 | 128 | 81.3% |
| C | Si | Mn | P | S | Cr | Mo | Al | O | Ti | |
|---|---|---|---|---|---|---|---|---|---|---|
| Mn | −0.07 | 0 | 0 | −0.0035 | −0.048 | 0 | 0 | 0 | −0.083 | 0 |
| S | 0.11 | 0.063 | −0.0026 | 0.029 | −0.028 | −0.011 | 0.0027 | 0.035 | −0.27 | −0.072 |
| Element | ||
|---|---|---|
| Mn | 0.055Exp(−249366/RT) | 0.785 |
| S | 2.4Exp(−223426/RT) | 0.035 |
| Sample | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 |
|---|---|---|---|---|---|---|---|---|---|---|
| 1# | 3.45 × 106 | 2.94 × 106 | 2.65 × 106 | 2.68 × 106 | 0.83 × 106 | 1.07 × 106 | 1.34 × 106 | 0.57 × 106 | 1.52 × 106 | 0.42 × 106 |
| 2# | 1.63 × 106 | 1.12 × 106 | 0.98 × 106 | 1.24 × 106 | 1.09 × 106 | 1.83 × 106 | 0.69 × 106 | 0.48 × 106 | 0.75 × 106 | 1.29 × 106 |
| Sample | L10 | k |
|---|---|---|
| SUJ2 | 0.685 × 106 | 2.214 |
| GCr15 | 0.543 × 106 | 3.269 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, H.; Feng, G.; Liu, X.; Wang, B.; Liu, X. Effect of Sulfur Content on the Composition of Inclusions and MnS Precipitation Behavior in Bearing Steel. Metals 2020, 10, 570. https://doi.org/10.3390/met10050570
Zhang H, Feng G, Liu X, Wang B, Liu X. Effect of Sulfur Content on the Composition of Inclusions and MnS Precipitation Behavior in Bearing Steel. Metals. 2020; 10(5):570. https://doi.org/10.3390/met10050570
Chicago/Turabian StyleZhang, Hongliang, Guanghong Feng, Xin Liu, Baoshan Wang, and Xuming Liu. 2020. "Effect of Sulfur Content on the Composition of Inclusions and MnS Precipitation Behavior in Bearing Steel" Metals 10, no. 5: 570. https://doi.org/10.3390/met10050570
APA StyleZhang, H., Feng, G., Liu, X., Wang, B., & Liu, X. (2020). Effect of Sulfur Content on the Composition of Inclusions and MnS Precipitation Behavior in Bearing Steel. Metals, 10(5), 570. https://doi.org/10.3390/met10050570




