Influence of Structural Defects on the Properties of Metamagnetic Shape Memory Alloys
Abstract
:1. Introduction
2. Materials and Methods
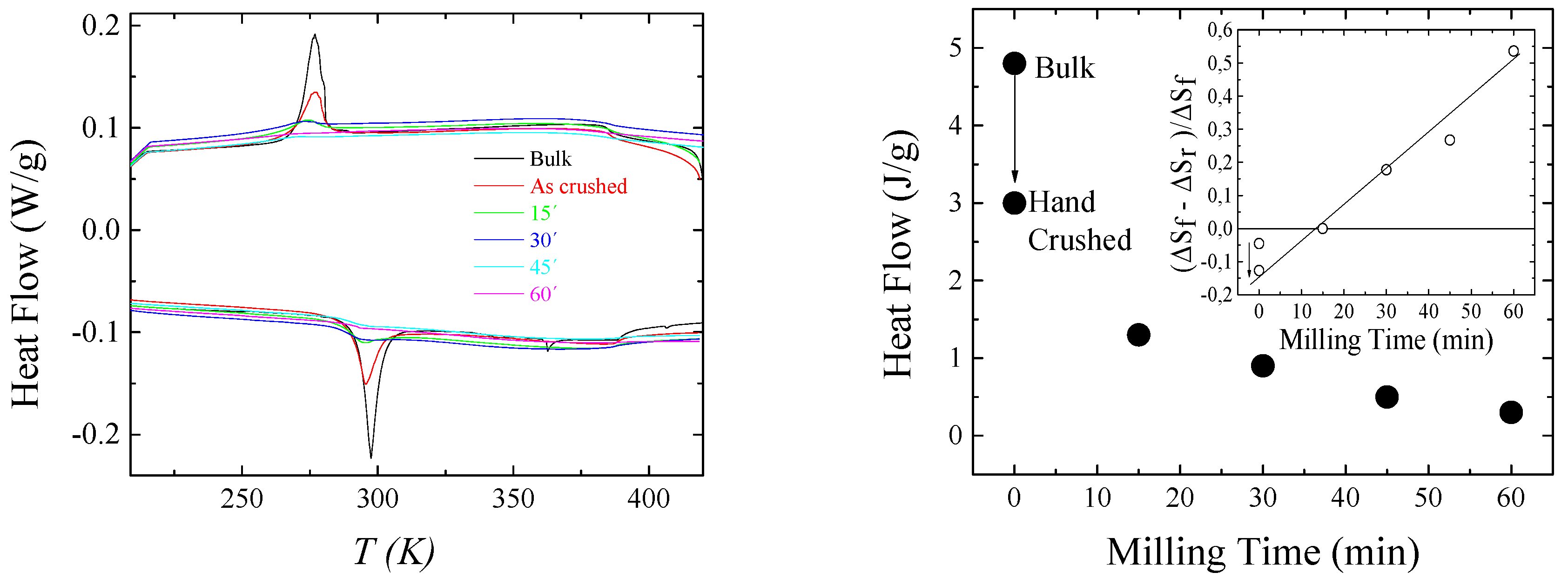
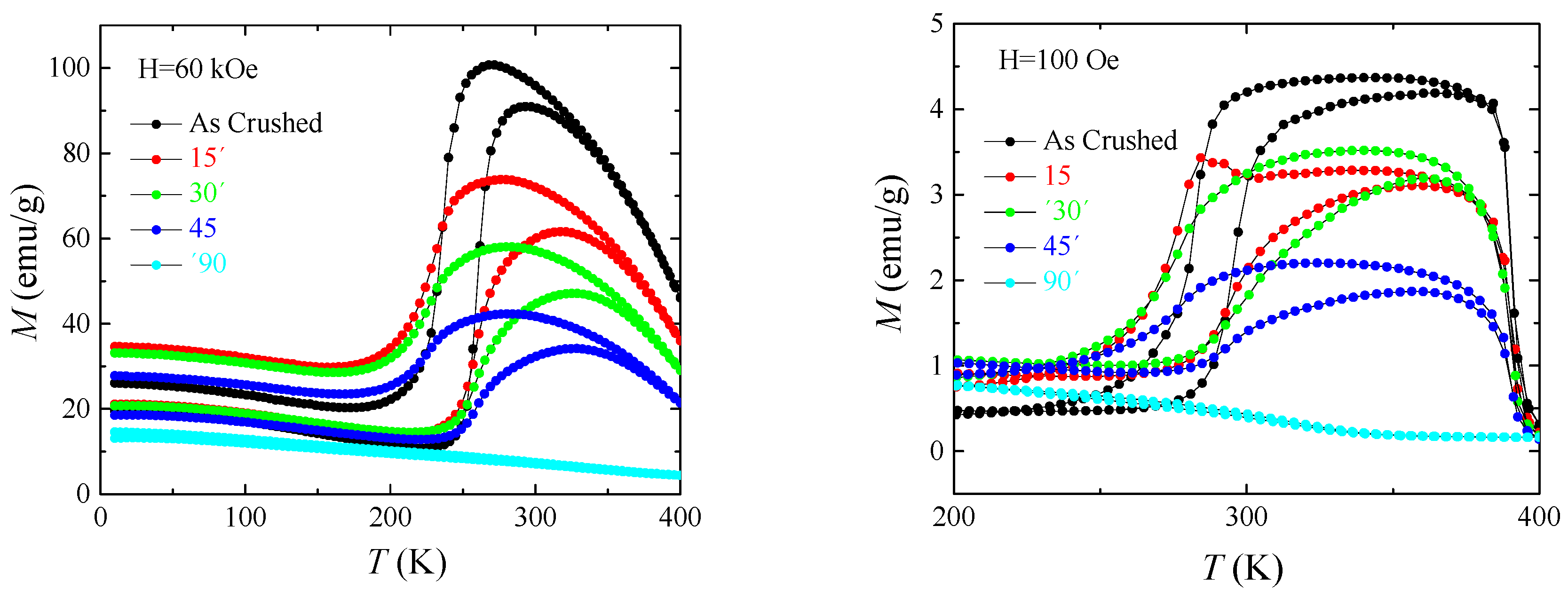
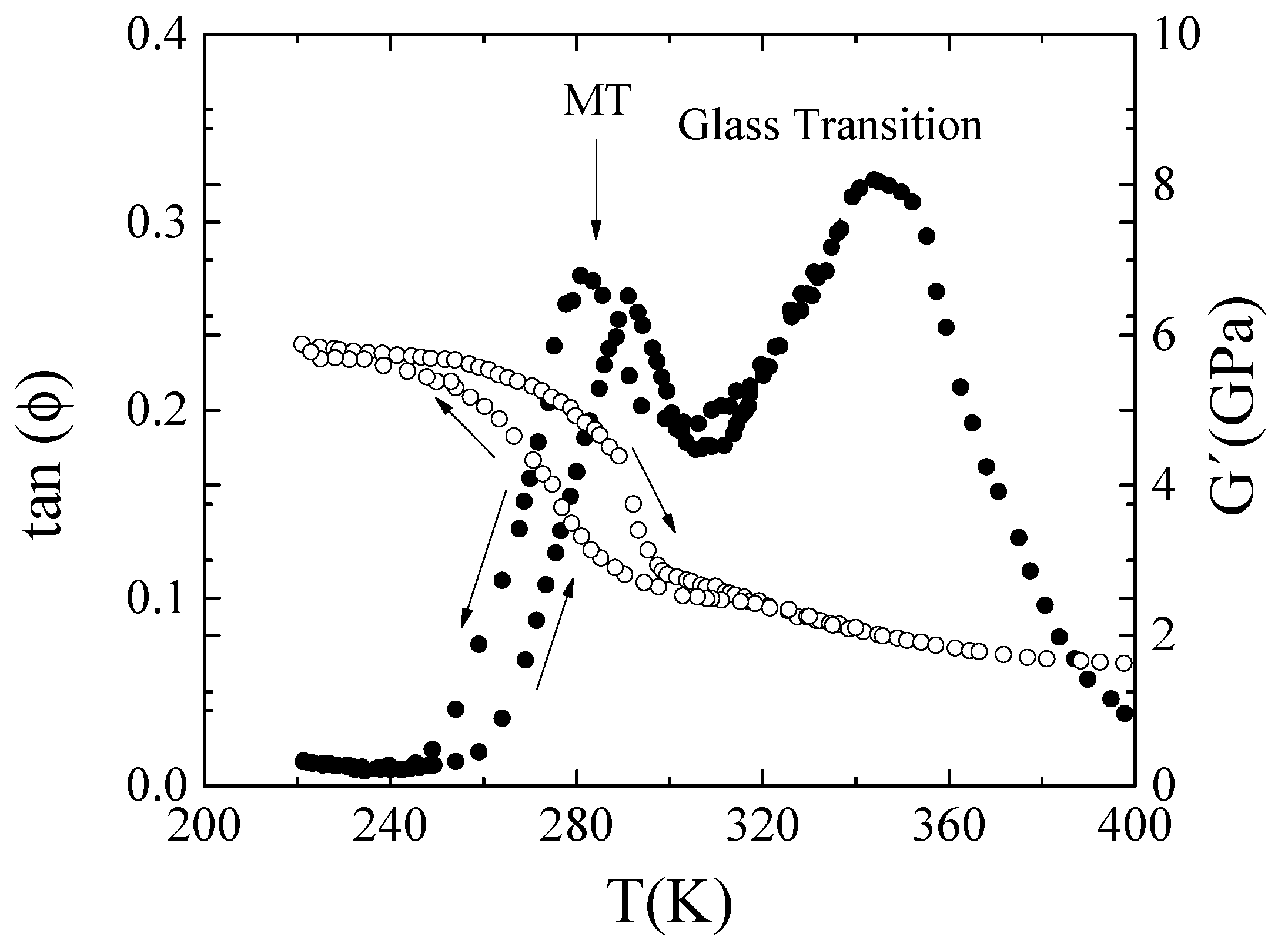
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Kainuma, R.; Imano, Y.; Ito, W.; Sutou, Y.; Morito, H.; Okamoto, S.; Kitakami, O.; Oikawa, K.; Fujita, A.; Kanomata, T.; et al. Magnetic-field-induced shape recovery by reverse phase transformation. Nature 2006, 439, 957–960. [Google Scholar] [CrossRef] [PubMed]
- Koyama, K.; Watanabe, K.; Kanomata, T.; Kainuma, R.; Oikawa, K.; Ishida, K. Observation of field-induced reverse transformation in ferromagnetic shape memory alloy Ni50Mn36Sn14. Appl. Phys. Lett. 2006, 88, 132505. [Google Scholar] [CrossRef]
- Oikawa, K.; Ito, W.; Imano, Y.; Sutou, Y.; Kainuma, R.; Ishida, K.; Okamoto, S.; Kitakami, O.; Kanomata, T. Effect of magnetic field on martensitic transition of Ni46Mn41In13 Heusler alloy. Appl. Phys. Lett. 2006, 88, 122507. [Google Scholar] [CrossRef]
- Acet, M.; Mañosa, L.; Planes, A. Chapter Four—Magnetic-Field-Induced Effects in Martensitic Heusler-Based Magnetic Shape Memory Alloys; Buschow, K.H.J., Ed.; Elsevier: Amsterdam, The Netherlands, 2011; Volume 19. [Google Scholar]
- Şaşıoğlu, E.; Sandratskii, L.M.; Bruno, P. First-principles calculation of the intersublattice exchange interactions and Curie temperatures of the full Heusler alloys Ni2MnX (X=Ga, In, Sn). Phys. Rev. B 2004, 70, 24427. [Google Scholar] [CrossRef] [Green Version]
- Şaşıoğlu, E.; Sandratskii, L.M.; Bruno, P. Role of conduction electrons in mediating exchange interactions in Mn-based Heusler alloys. Phys. Rev. B 2008, 77, 64417. [Google Scholar] [CrossRef] [Green Version]
- Buchelnikov, V.D.; Entel, P.; Taskaev, S.V.; Sokolovskiy, V.V.; Hucht, A.; Ogura, M.; Akai, H.; Gruner, M.E.; Nayak, S.K. Monte Carlo study of the influence of antiferromagnetic exchange interactions on the phase transitions of ferromagnetic Ni-Mn-X alloys (X=In, Sn, Sb). Phys. Rev. B 2008, 78, 184427. [Google Scholar] [CrossRef]
- Kainuma, R.; Ito, W.; Umetsu, R.Y.; Khovaylo, V.V.; Kanomata, T. Metamagnetic shape memory effect and magnetic properties of Ni-Mn based Heusler alloys. Mater. Sci. Forum 2011, 684, 139–150. [Google Scholar] [CrossRef]
- Yu, S.Y.; Liu, Z.H.; Liu, G.D.; Chen, J.L.; Cao, Z.X.; Wu, G.H.; Zhang, B.; Zhang, X.X. Large magnetoresistance in single-crystalline Ni50Mn50−xInx alloys (x=14–16) upon martensitic transformation. Appl. Phys. Lett. 2006, 89, 162503. [Google Scholar] [CrossRef]
- Sharma, V.K.; Chattopadhyay, M.K.; Shaeb, K.H.B.; Chouhan, A.; Roy, S.B. Large magnetoresistance in Ni50Mn34In16 alloy. Appl. Phys. Lett. 2006, 89, 222509. [Google Scholar] [CrossRef]
- Krenke, T.; Duman, E.; Acet, M.; Wassermann, E.F.; Moya, X.; Mañosa, L.; Planes, A. Inverse magnetocaloric effect in ferromagnetic Ni-Mn-Sn alloys. Nat. Mater. 2005, 4, 450–454. [Google Scholar] [CrossRef] [Green Version]
- Krenke, T.; Duman, E.; Acet, M.; Wassermann, E.F.; Moya, X.; Mañosa, L.; Planes, A.; Suard, E.; Ouladdiaf, B. Magnetic superelasticity and inverse magnetocaloric effect in Ni-Mn-In. Phys. Rev. B 2007, 75, 104414. [Google Scholar] [CrossRef] [Green Version]
- Planes, A.; Mañosa, L.; Acet, M. Magnetocaloric effect and its relation to shape-memory properties in ferromagnetic Heusler alloys. J. Phys. Condens. Matter 2009, 21, 233201. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Liu, J.; Gottschall, T.; Skokov, K.P.; Moore, J.D.; Gutfleisch, O. Giant magnetocaloric effect driven by structural transitions. Nat. Mater. 2012, 11, 620–626. [Google Scholar] [CrossRef] [PubMed]
- Pérez-Landazábal, J.I.; Lambri, O.A.; Bonifacich, F.G.; Sánchez-Alarcos, V.; Recarte, V.; Tarditti, F. Influence of defects on the irreversible phase transition in Fe–Pd ferromagnetic shape memory alloys. Acta Mater. 2015, 86, 110–117. [Google Scholar] [CrossRef]
- Bonifacich, F.G.; Lambri, O.A.; Pérez-Landazábal, J.I.; Gargicevich, D.; Recarte, V.; Sánchez-Alarcos, V. Mobility of Twin Boundaries in Fe-Pd-Based Ferromagnetic Shape Memory Alloys. Mater. Trans. 2016, 57, 1837–1844. [Google Scholar] [CrossRef] [Green Version]
- Sutou, Y.; Imano, Y.; Koeda, N.; Omori, T.; Kainuma, R.; Ishida, K.; Oikawa, K. Magnetic and martensitic transformations of NiMnX(X=In,Sn,Sb) ferromagnetic shape memory alloys. Appl. Phys. Lett. 2004, 85, 4358–4360. [Google Scholar] [CrossRef]
- Krenke, T.; Acet, M.; Wassermann, E.F.; Moya, X.; Mañosa, L.; Planes, A. Martensitic transitions and the nature of ferromagnetism in the austenitic and martensitic states of Ni-Mn-Sn alloys. Phys. Rev. B 2005, 72, 14412. [Google Scholar] [CrossRef] [Green Version]
- Krenke, T.; Acet, M.; Wassermann, E.F.; Moya, X.; Mañosa, L.; Planes, A. Ferromagnetism in the austenitic and martensitic states of Ni-Mn-In alloys. Phys. Rev. B 2006, 73, 174413. [Google Scholar] [CrossRef] [Green Version]
- Khan, M.; Dubenko, I.; Stadler, S.; Ali, N. Magnetostructural phase transitions in Ni50Mn25+xSb25−xHeusler alloys. J. Phys. Condens. Matter 2008, 20, 235204. [Google Scholar] [CrossRef]
- Recarte, V.; Pérez-Landazábal, J.I.; Sánchez-Alarcos, V.; Rodríguez-Velamazán, J.A. Dependence of the martensitic transformation and magnetic transition on the atomic order in Ni–Mn–In metamagnetic shape memory alloys. Acta Mater. 2012, 60, 1937–1945. [Google Scholar] [CrossRef]
- Recarte, V.; Pérez-Landazábal, J.I.; Sánchez-Alarcos, V. Dependence of the relative stability between austenite and martensite phases on the atomic order in a Ni–Mn–In Metamagnetic Shape Memory Alloy. J. Alloys Compd. 2012, 536, S308–S311. [Google Scholar] [CrossRef]
- Sánchez-Alarcos, V.; Recarte, V.; Pérez-Landazábal, J.; Cesari, E.; Rodríguez-Velamazán, J. Long-Range Atomic Order and Entropy Change at the Martensitic Transformation in a Ni-Mn-In-Co Metamagnetic Shape Memory Alloy. Entropy 2014, 16, 2756–2767. [Google Scholar] [CrossRef] [Green Version]
- Sánchez-Alarcos, V.; Pérez-Landazábal, J.I.; Recarte, V.; Lucia, I.; Vélez, J.; Rodríguez-Velamazán, J.A. Effect of high-temperature quenching on the magnetostructural transformations and the long-range atomic order of Ni–Mn–Sn and Ni–Mn–Sb metamagnetic shape memory alloys. Acta Mater. 2013, 61, 4676–4682. [Google Scholar] [CrossRef]
- Kainuma, R.; Imano, Y.; Ito, W.; Morito, H.; Sutou, Y.; Oikawa, K.; Fujita, A.; Ishida, K.; Okamoto, S.; Kitakami, O.; et al. Metamagnetic shape memory effect in a Heusler-type Ni43Co7Mn39Sn11 polycrystalline alloy. Appl. Phys. Lett. 2006, 88, 192513. [Google Scholar] [CrossRef]
- Nayak, A.K.; Suresh, K.G.; Nigam, A.K. Giant inverse magnetocaloric effect near room temperature in Co substituted NiMnSb Heusler alloys. J. Phys. D. Appl. Phys. 2009, 42, 35009. [Google Scholar] [CrossRef] [Green Version]
- Huang, L.; Cong, D.; Suo, H.; Wang, Y. Giant magnetic refrigeration capacity near room temperature in Ni40Co10Mn40Sn10 multifunctional alloy. Appl. Phys. Lett. 2014, 104, 132407. [Google Scholar] [CrossRef]
- Umetsu, R.Y.; Sheikh, A.; Ito, W.; Ouladdiaf, B.; Ziebeck, K.R.A.; Kanomata, T.; Kainuma, R. The effect of Co substitution on the magnetic properties of the Heusler alloy Ni50Mn33Sn17. Appl. Phys. Lett. 2011, 98, 42507. [Google Scholar] [CrossRef]
- Lahelin, M.; Aaltio, I.; Heczko, O.; Söderberg, O.; Ge, Y.; Löfgren, B.; Hannula, S.-P.; Seppälä, J. DMA testing of Ni–Mn–Ga/polymer composites. Compos. Part A Appl. Sci. Manuf. 2009, 40, 125–129. [Google Scholar] [CrossRef]
- Scheerbaum, N.; Hinz, D.; Gutfleisch, O.; Müller, K.-H.; Schultz, L. Textured polymer bonded composites with Ni–Mn–Ga magnetic shape memory particles. Acta Mater. 2007, 55, 2707–2713. [Google Scholar] [CrossRef]
- Feuchtwanger, J.; Richard, M.L.; Tang, Y.J.; Berkowitz, A.E.; O’Handley, R.C.; Allen, S.M. Large energy absorption in Ni–Mn–Ga/polymer composites. J. Appl. Phys. 2005, 97, 10M319. [Google Scholar] [CrossRef] [Green Version]
- Liu, J.; Scheerbaum, N.; Kauffmann-Weiss, S.; Gutfleisch, O. NiMn-Based Alloys and Composites for Magnetically Controlled Dampers and Actuators. Adv. Eng. Mater. 2012, 14, 653–667. [Google Scholar] [CrossRef]
- Tian, B.; Chen, F.; Tong, Y.; Li, L.; Zheng, Y. Magnetic field induced strain and damping behavior of Ni–Mn–Ga particles/epoxy resin composite. J. Alloys Compd. 2014, 604, 137–141. [Google Scholar] [CrossRef]
- Alves, A.L.; Passamani, E.C.; Nascimento, V.P.; Takeuchi, A.Y.; Larica, C. Influence of grain refinement and induced crystal defects on the magnetic properties of Ni50Mn36Sn14Heusler alloy. J. Phys. D Appl. Phys. 2010, 43, 345001. [Google Scholar] [CrossRef]
- Passamani, E.; Nascimento, V.; Larica, C.; Takeuchi, A.; Alves, A.; Capua Proveti, J.; Pereira, M.; Fabris, J. The influence of chemical disorder enhancement on the martensitic transformation of the Ni 50Mn 36Sn 14 Heusler-type alloy. J. Alloy. Compd. 2011, 509, 7826–7832. [Google Scholar] [CrossRef]
- Ghotbi Varzaneh, A.; Kameli, P.; Zahedi, V.R.; Karimzadeh, F.; Salamati, H. Effect of heat treatment on martensitic transformation of Ni47Mn40Sn13 ferromagnetic shape memory alloy prepared by mechanical alloying. Met. Mater. Int. 2015, 21, 758–764. [Google Scholar] [CrossRef]
- Czaja, P.; Przewoźnik, J.; Fitta, M.; Bałanda, M.; Chrobak, A.; Kania, B.; Zackiewicz, P.; Wójcik, A.; Szlezynger, M.; Maziarz, W. Effect of ball milling and thermal treatment on exchange bias and magnetocaloric properties of Ni48Mn39.5Sn10.5Al2 ribbons. J. Magn. Magn. Mater. 2016, 401, 223–230. [Google Scholar] [CrossRef]
- Wang, X.; Sun, F.; Wang, J.; Yu, Q.; Wu, Y.; Hua, H.; Jiang, C. Influence of annealing temperatures on the magnetostructural transition and magnetocaloric effect of Ni40Co10Mn40Sn10 powders. J. Alloys Compd. 2017, 691, 215–219. [Google Scholar] [CrossRef]
- Unzueta, I.; López-García, J.; Sánchez-Alarcos, V.; Recarte, V.; Pérez-Landazábal, J.I.; Rodríguez-Velamazán, J.A.; Garitaonandia, J.S.; García, J.A.; Plazaola, F. 119Sn Mössbauer spectroscopy for assessing the local stress and defect state towards the tuning of Ni-Mn-Sn alloys. Appl. Phys. Lett. 2017, 110, 181908. [Google Scholar] [CrossRef] [Green Version]
- Murakami, Y.; Yanagisawa, K.; Niitsu, K.; Park, H.S.; Matsuda, T.; Kainuma, R.; Shindo, D.; Tonomura, A. Determination of magnetic flux density at the nanometer-scale antiphase boundary in Heusler alloy Ni50Mn25Al12.5Ga12.5. Acta Mater. 2013, 61, 2095–2101. [Google Scholar] [CrossRef]
- Fauth, F.; Peral, I.; Popescu, C.; Knapp, M. The new Material Science Powder Diffraction beamline at ALBA Synchrotron. Powder Diffr. 2013, 28, S360–S370. [Google Scholar] [CrossRef]
- Peral, I.; McKinlay, J.; Knapp, M.; Ferrer, S. Design and construction of multicrystal analyser detectors using Rowland circles: Application to MAD26 at ALBA. J. Synchrotron Radiat. 2011, 18, 842–850. [Google Scholar] [CrossRef] [PubMed]
- Rodríguez-Carvajal, J. Recent advances in magnetic structure determination by neutron powder diffraction. Phys. B Condens. Matter 1993, 192, 55–69. [Google Scholar] [CrossRef]
- Recarte, V.; Pérez-Landazábal, J.I.; Sánchez-Alarcos, V.; Zablotskii, V.; Cesari, E.; Kustov, S. Entropy change linked to the martensitic transformation in metamagnetic shape memory alloys. Acta Mater. 2012, 60, 3168–3175. [Google Scholar] [CrossRef]
- Pérez-Landazábal, J.I.; Recarte, V.; Sánchez-Alarcos, V.; Ruiz, M.J.; Cesari, E. Outstanding role of the magnetic entropy in arrested austenite in an ordered Ni45Mn36.7In13.3Co5 metamagnetic shape memory alloy. Scr. Mater. 2019, 168, 91–95. [Google Scholar] [CrossRef]
- López-García, J.; Unzueta, I.; Sánchez-Alarcos, V.; Recarte, V.; Pérez-Landazábal, J.I.; Rodríguez-Velamazán, J.A.; García, J.A.; Plazaola, F. Correlation between defects and magneto-structural properties in Ni-Mn-Sn metamagnetic shape memory alloys. Intermetallics 2018, 94, 133–137. [Google Scholar] [CrossRef]
- Li, G.T.; Liu, Z.H.; Ma, X.Q.; Yu, S.Y.; Liu, Y. Grinding-induced martensite stabilization in Mn50Ni33.5Sn8Co8.5 alloy. Mater. Lett. 2013, 107, 239–242. [Google Scholar] [CrossRef]
- Singh, S.; Kushwaha, P.; Scheibel, F.; Liermann, H.-P.; Barman, S.R.; Acet, M.; Felser, C.; Pandey, D. Residual stress induced stabilization of martensite phase and its effect on the magnetostructural transition in Mn-rich Ni-Mn-In/Ga magnetic shape-memory alloys. Phys. Rev. B 2015, 92, 20105. [Google Scholar] [CrossRef] [Green Version]
- Chulist, R.; Czaja, P.; Tokarski, T.; Faryna, M. Martensite stabilisation in single crystalline Ni-Mn-Ga and Ni-Mn-Sn magnetic shape memory alloys. Mater. Lett. 2018, 230, 266–269. [Google Scholar] [CrossRef]
- Umetsu, R.Y.; Fujita, A.; Ito, W.; Kanomata, T.; Kainuma, R. Determination of the magnetic ground state in the martensite phase of Ni–Mn–Z (Z= In, Sn and Sb) off-stoichiometric Heusler alloys by nonlinear AC susceptibility. J. Phys. Condens. Matter 2011, 23, 326001. [Google Scholar] [CrossRef]
- Larumbe, S.; Unzueta, I.; Sánchez-Alarcos, V.; Pérez-Landazábal, J.I.; Recarte, V.; García, J.A.; Plazaola, F. Low temperature magnetic properties of a Ni50Mn34In16 ball-milled metamagnetic shape memory alloy. J. Non. Cryst. Solids 2016, 447, 16–20. [Google Scholar] [CrossRef]
- Sánchez-Alarcos, V.; Recarte, V.; Pérez-Landazábal, J.I.; Larumbe, S.; Caballero-Flores, R.; Unzueta, I.; García, J.A.; Plazaola, F.; Rodríguez-Velamazán, J.A. Mechanically induced disorder and crystallization process in Ni-Mn-In ball-milled alloys. J. Alloys Compd. 2016, 689, 983–991. [Google Scholar] [CrossRef]
- Takahashi, S.; Chiba, A.; Takahashi, E. The influence of plastic deformation on the spin-glass in Fe-37.0 at.% Al compound. Phys. Lett. A 1995, 197, 350–352. [Google Scholar] [CrossRef]
- L’vov, V.A.; Kosogor, A.; Barandiaran, J.M.; Chernenko, V.A. Theoretical description of magnetocaloric effect in the shape memory alloy exhibiting metamagnetic behavior. J. Appl. Phys. 2016, 119, 13902. [Google Scholar] [CrossRef]
- Kosogor, A.; L’vov, V.; Lázpita, P.; Seguí, C.; Cesari, E. Magnetocaloric Effect Caused by Paramagnetic Austenite–Ferromagnetic Martensite Phase Transformation. Metals 2018, 9, 11. [Google Scholar] [CrossRef] [Green Version]




© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pérez-Landazábal, J.I.; Sánchez-Alarcos, V.; Recarte, V.; Lambri, O.A.; Bonifacich, F.G.; Khanna, D.L.R.; Unzueta, I.; García, J.A.; Plazaola, F.; López-García, J.; et al. Influence of Structural Defects on the Properties of Metamagnetic Shape Memory Alloys. Metals 2020, 10, 1131. https://doi.org/10.3390/met10091131
Pérez-Landazábal JI, Sánchez-Alarcos V, Recarte V, Lambri OA, Bonifacich FG, Khanna DLR, Unzueta I, García JA, Plazaola F, López-García J, et al. Influence of Structural Defects on the Properties of Metamagnetic Shape Memory Alloys. Metals. 2020; 10(9):1131. https://doi.org/10.3390/met10091131
Chicago/Turabian StylePérez-Landazábal, J. I., V. Sánchez-Alarcos, V. Recarte, O. A. Lambri, F. G. Bonifacich, D. L.R. Khanna, I. Unzueta, J.A. García, F. Plazaola, J. López-García, and et al. 2020. "Influence of Structural Defects on the Properties of Metamagnetic Shape Memory Alloys" Metals 10, no. 9: 1131. https://doi.org/10.3390/met10091131
APA StylePérez-Landazábal, J. I., Sánchez-Alarcos, V., Recarte, V., Lambri, O. A., Bonifacich, F. G., Khanna, D. L. R., Unzueta, I., García, J. A., Plazaola, F., López-García, J., Jimenez Ruiz, M., Rodríguez-Velamazán, J. A., & Cesari, E. (2020). Influence of Structural Defects on the Properties of Metamagnetic Shape Memory Alloys. Metals, 10(9), 1131. https://doi.org/10.3390/met10091131






