Vertical–Horizontal Coupling Vibration of Hot Rolling Mill Rolls under Multi-Piecewise Nonlinear Constraints
Abstract
1. Introduction
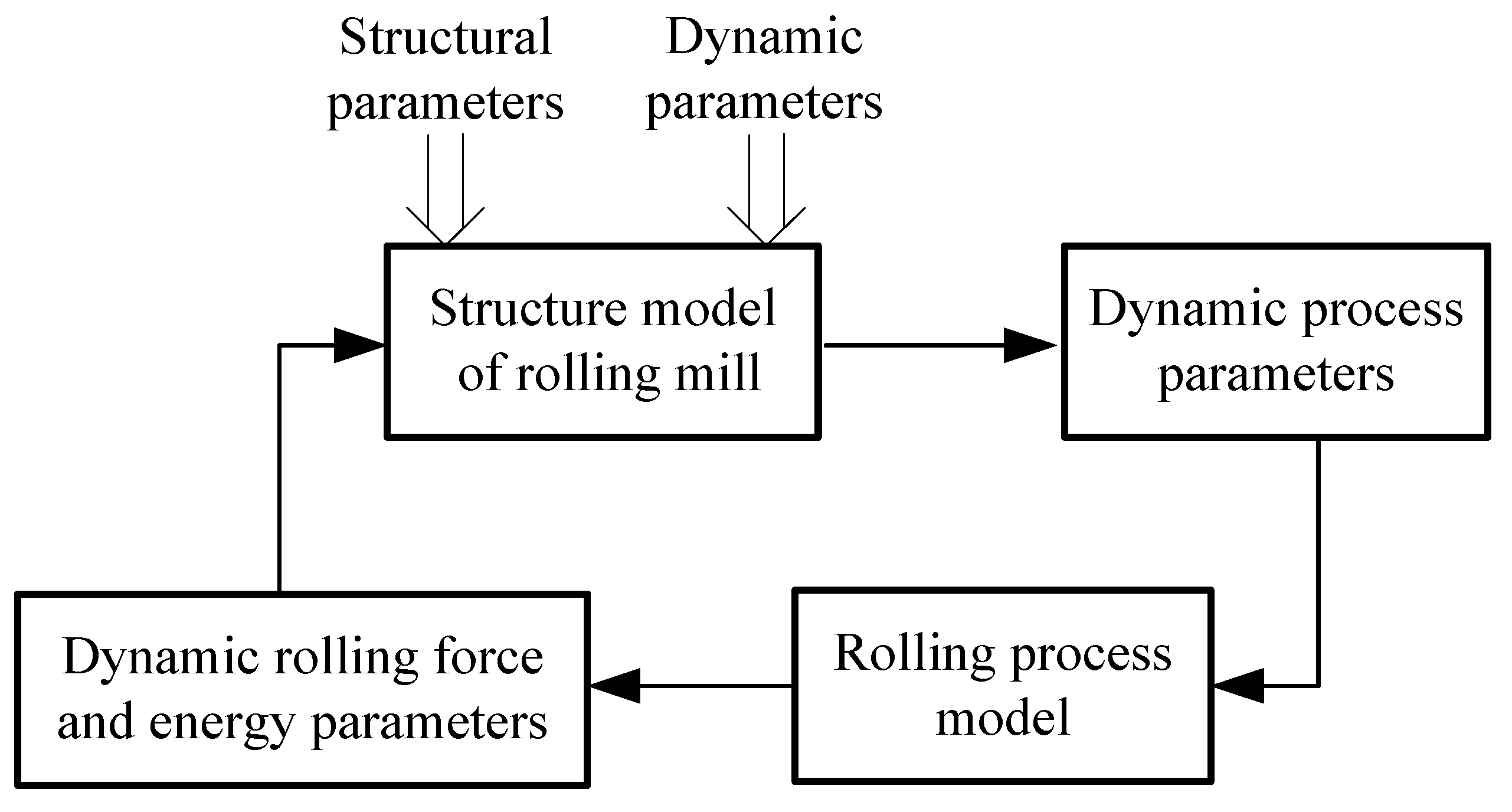
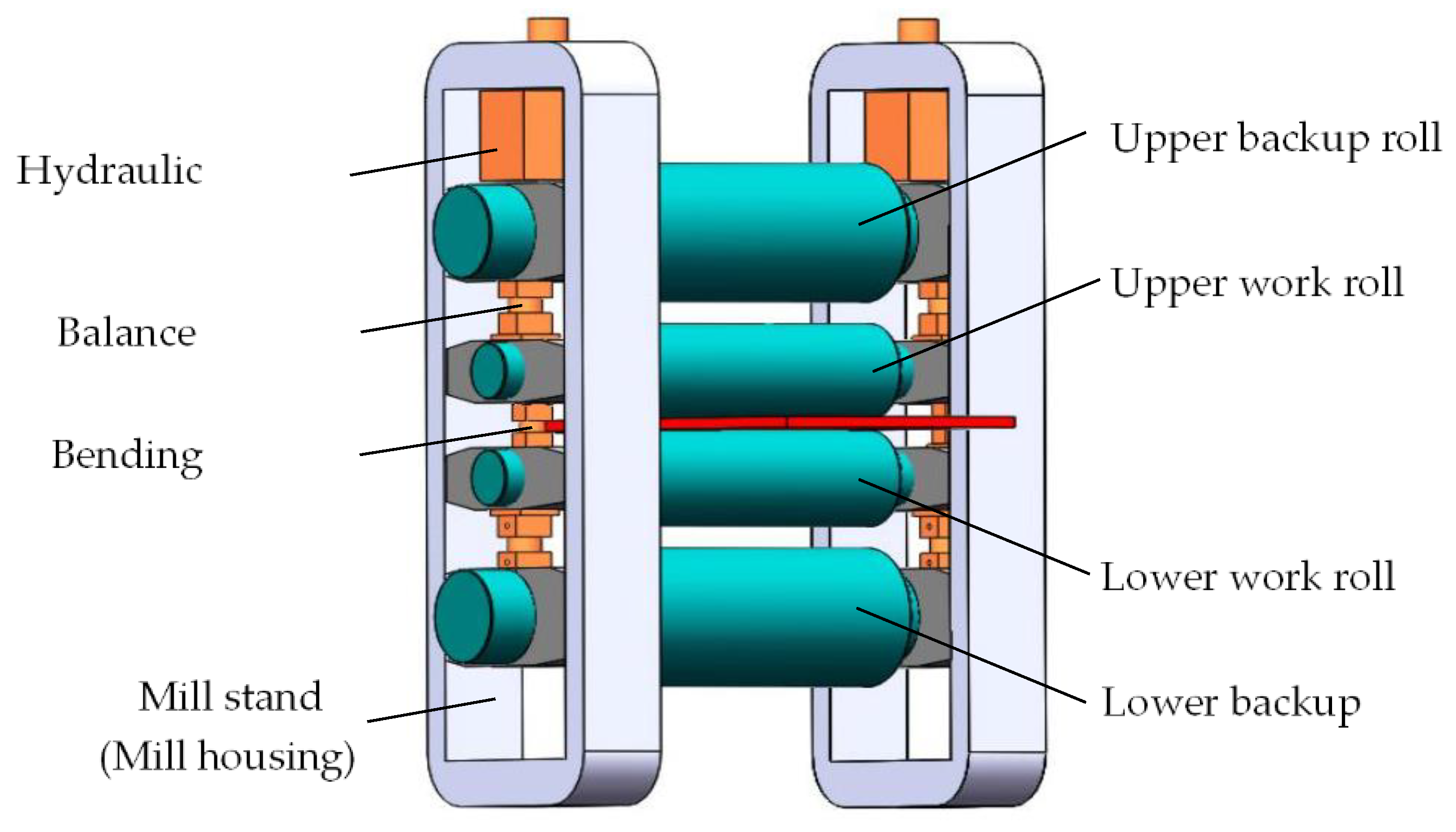
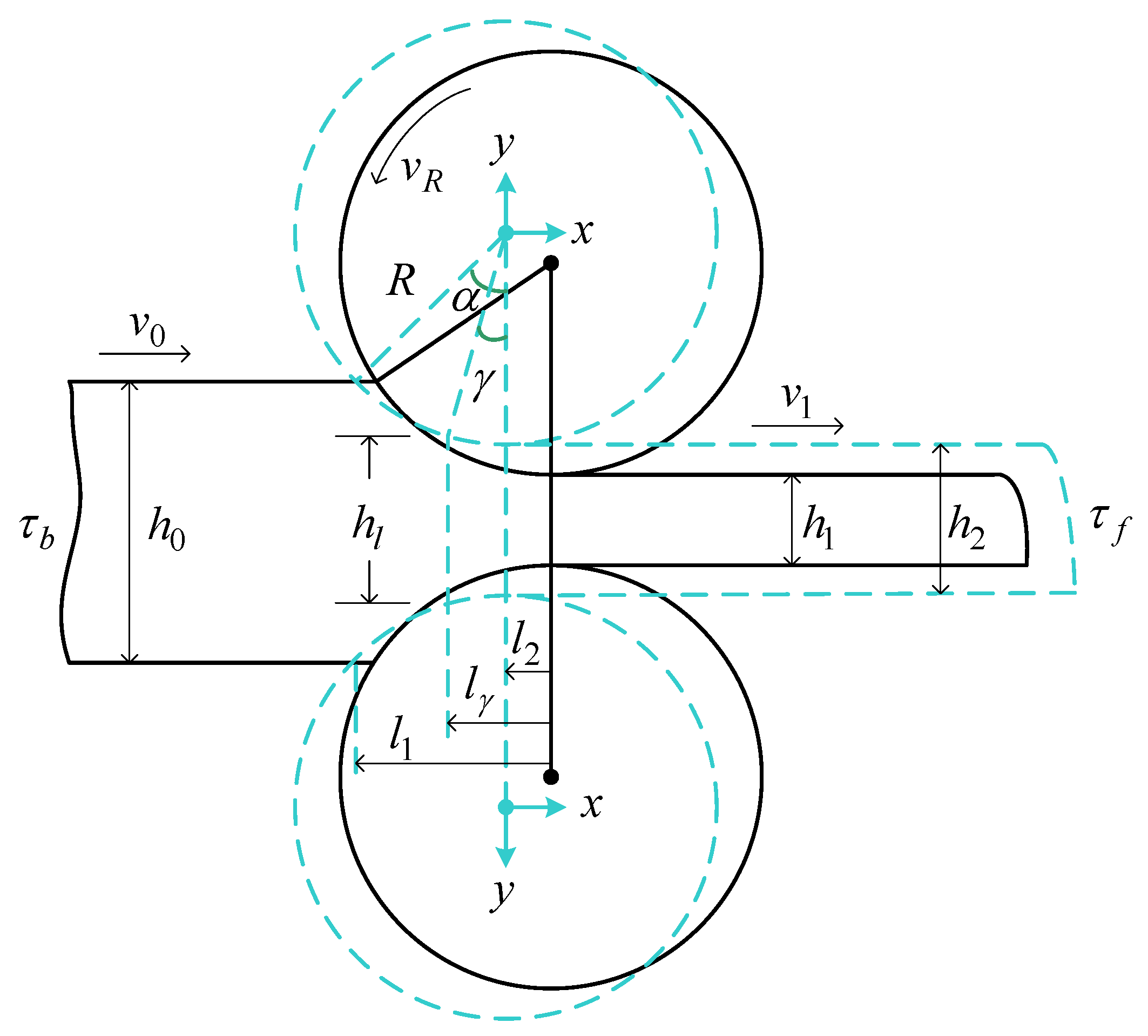
2. Multi-Piecewise Nonlinear Constraint Modeling of Hot Rolling Mill Rolls
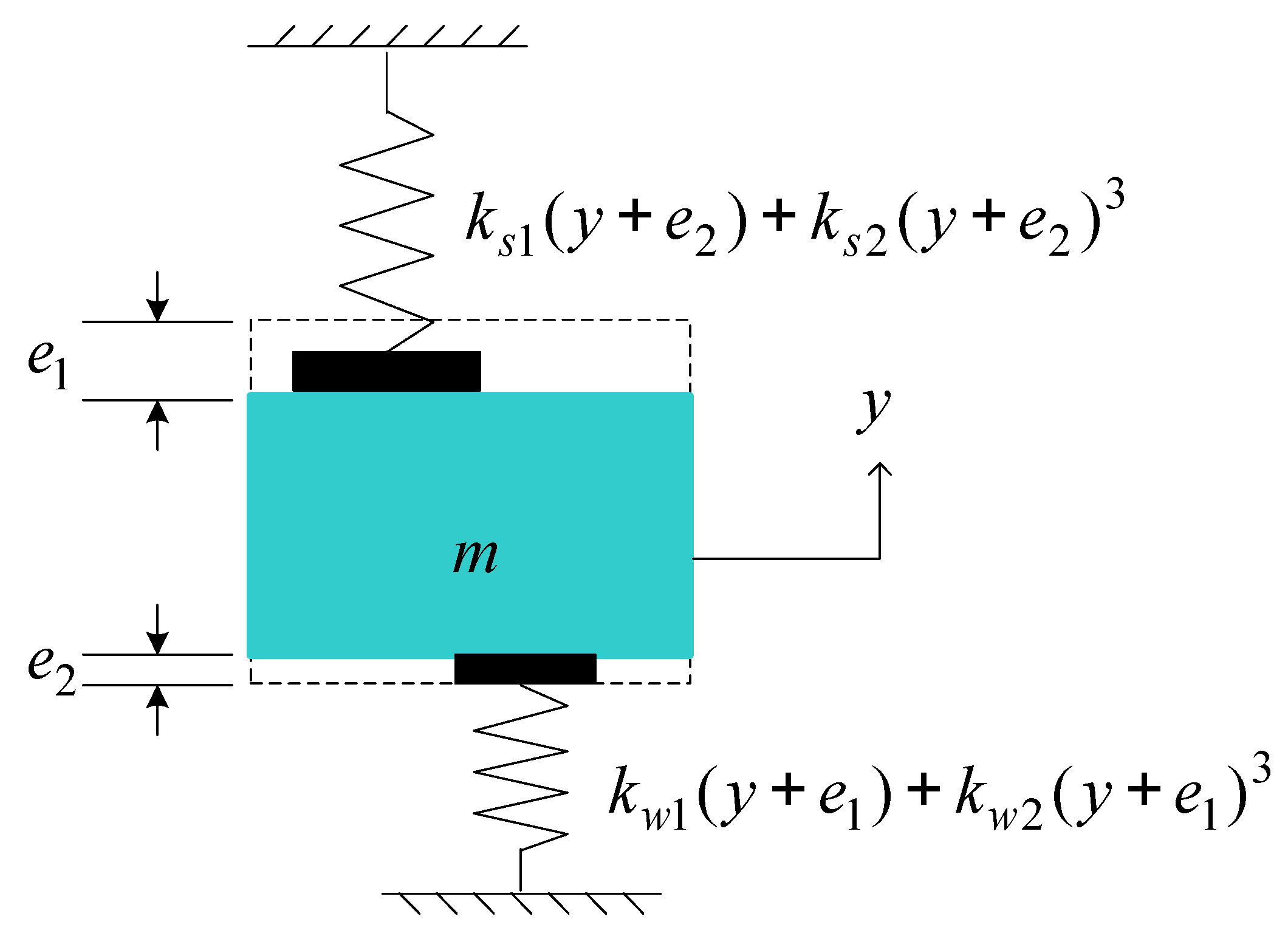
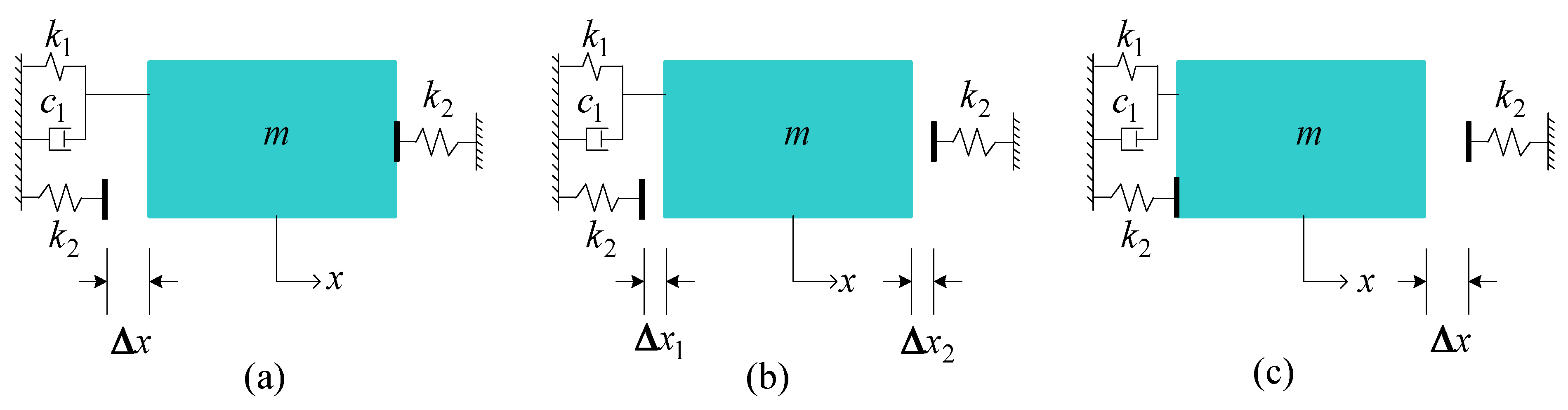
2.1. Piecewise Nonlinear Spring Force Model
2.2. Piecewise Nonlinear Friction Force Model
2.3. Piecewise Stiffness Model
3. Dynamic Rolling Force Model of Hot Rolling Mill Rolls
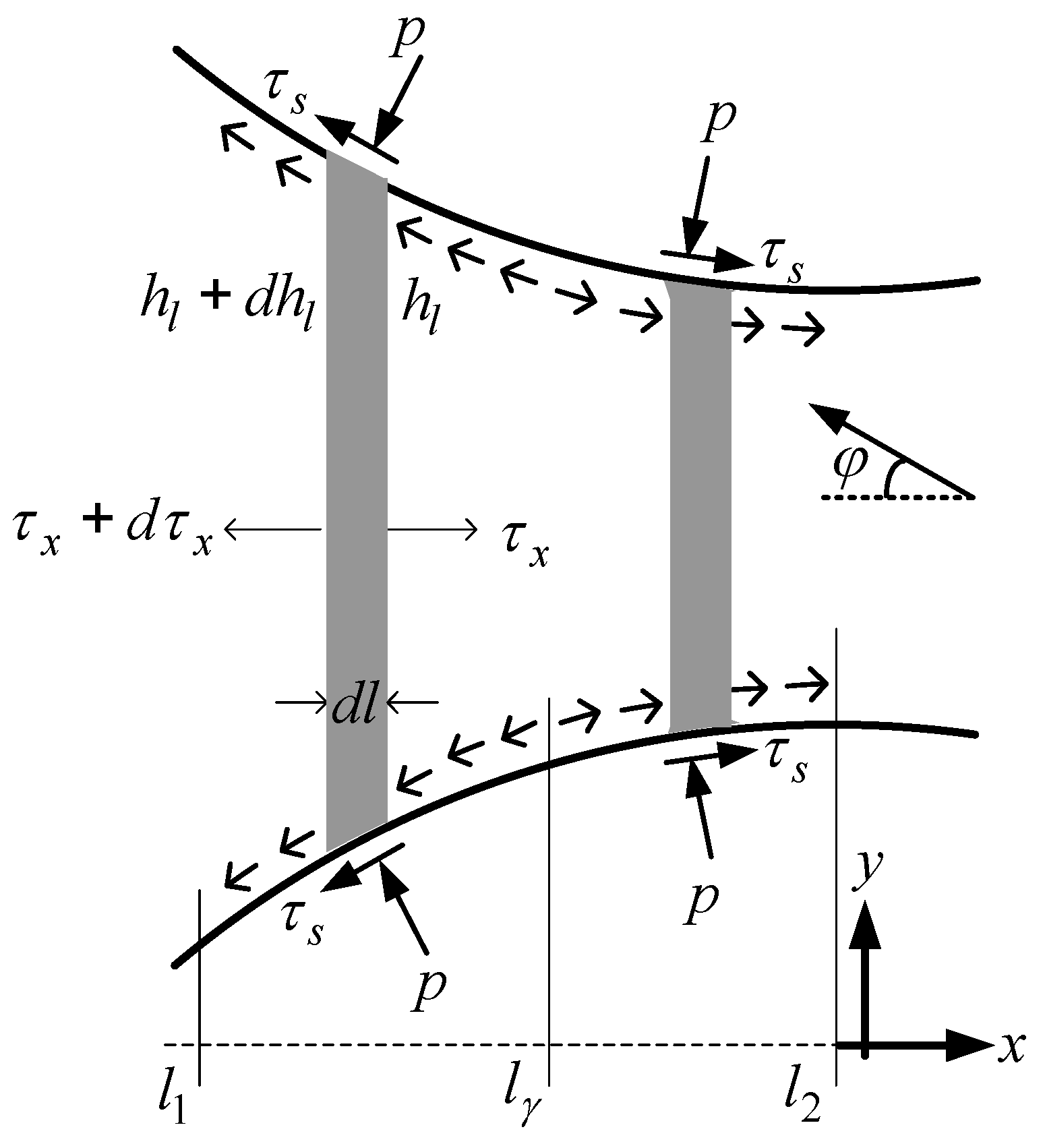
3.1. Determination of Rolling Parameters in Deformation Zone
3.2. Determination of Vertical–Horizontal Coupling Dynamic Rolling Force
4. Coupling Vibration Modeling and System Response Solution of Hot Rolling Mill Rolls
5. Analysis of Vibration Characteristics of Coupling System of Hot Rolling Mill Rolls
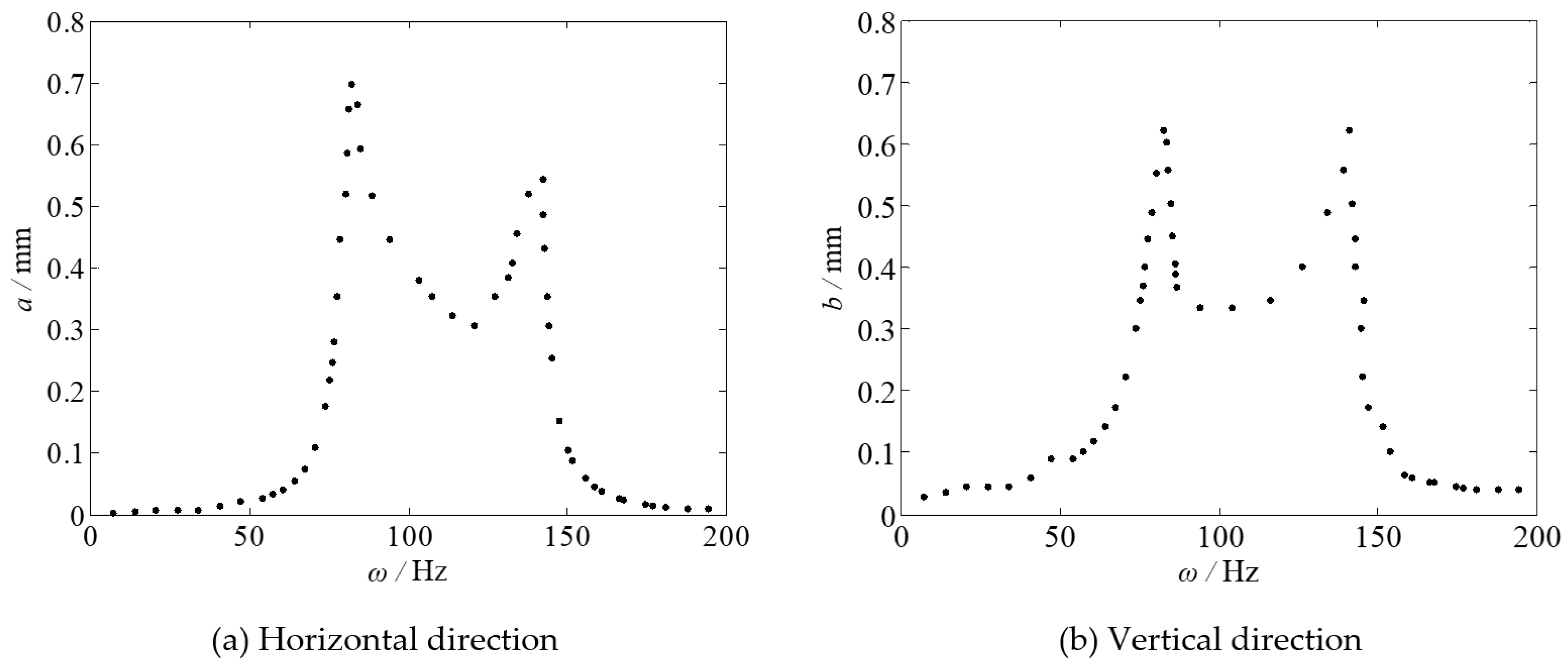
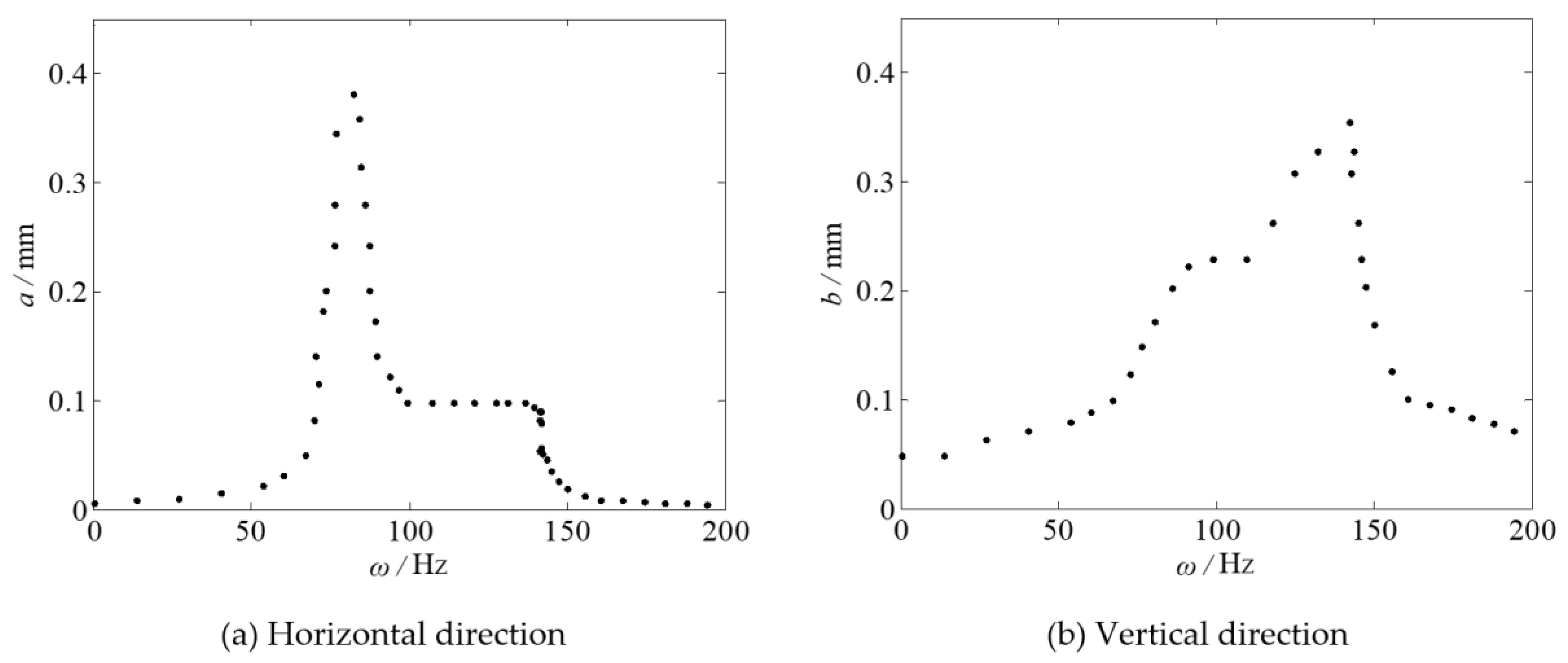
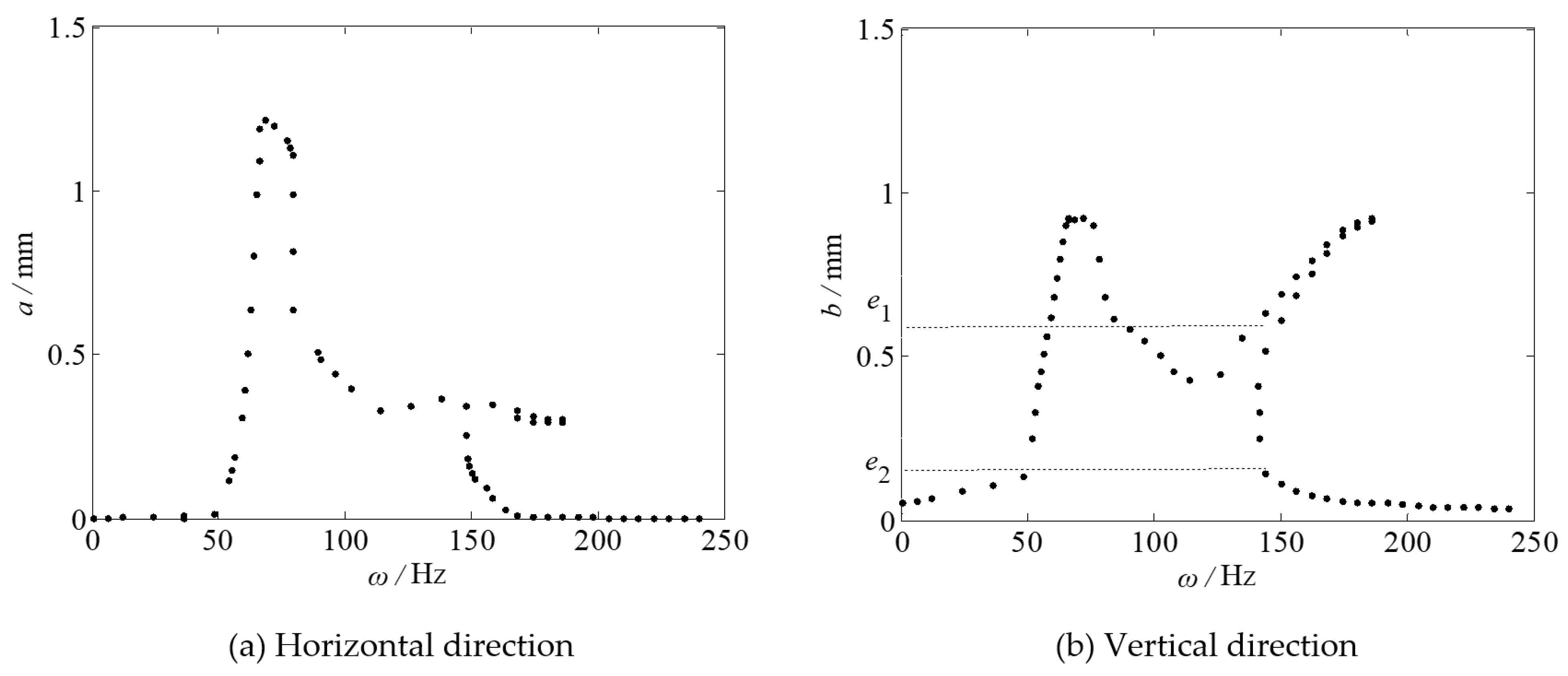
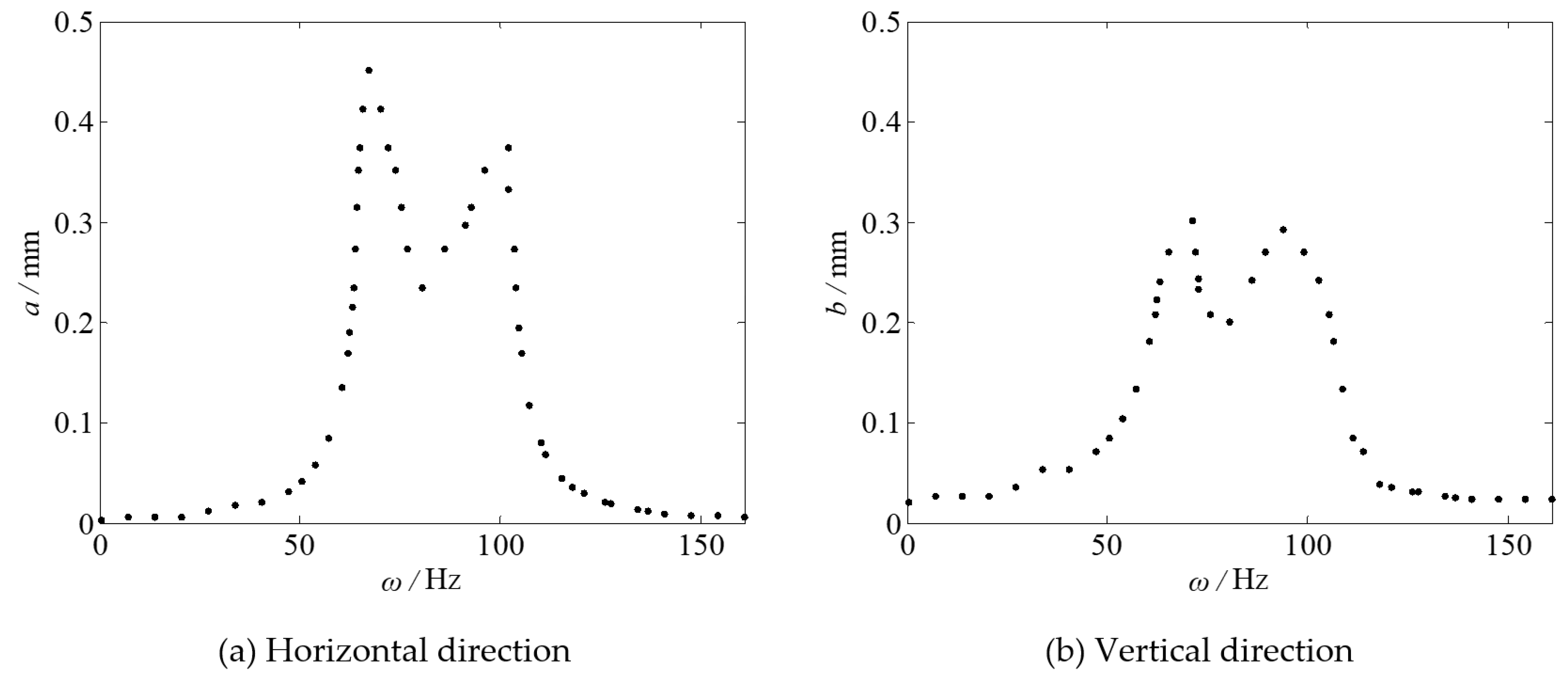
5.1. Analysis of Amplitude–Frequency Characteristics
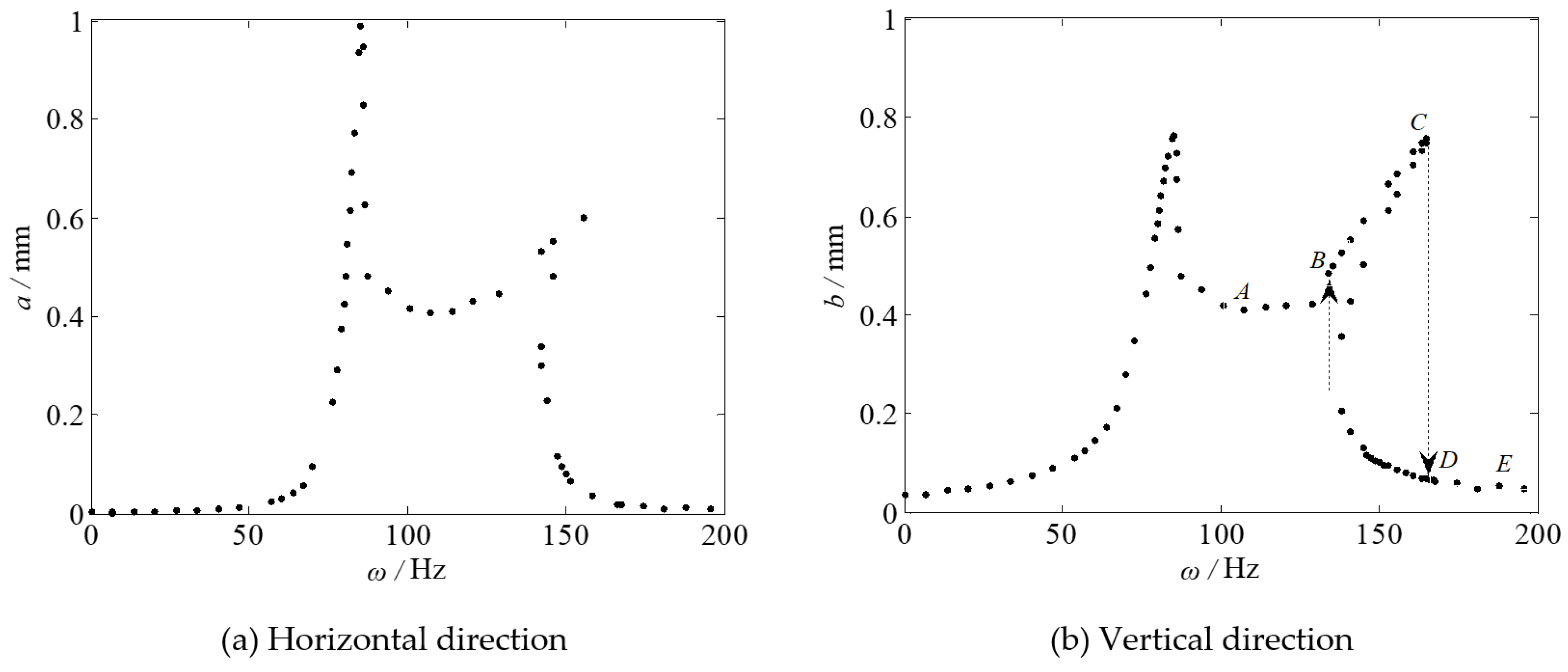
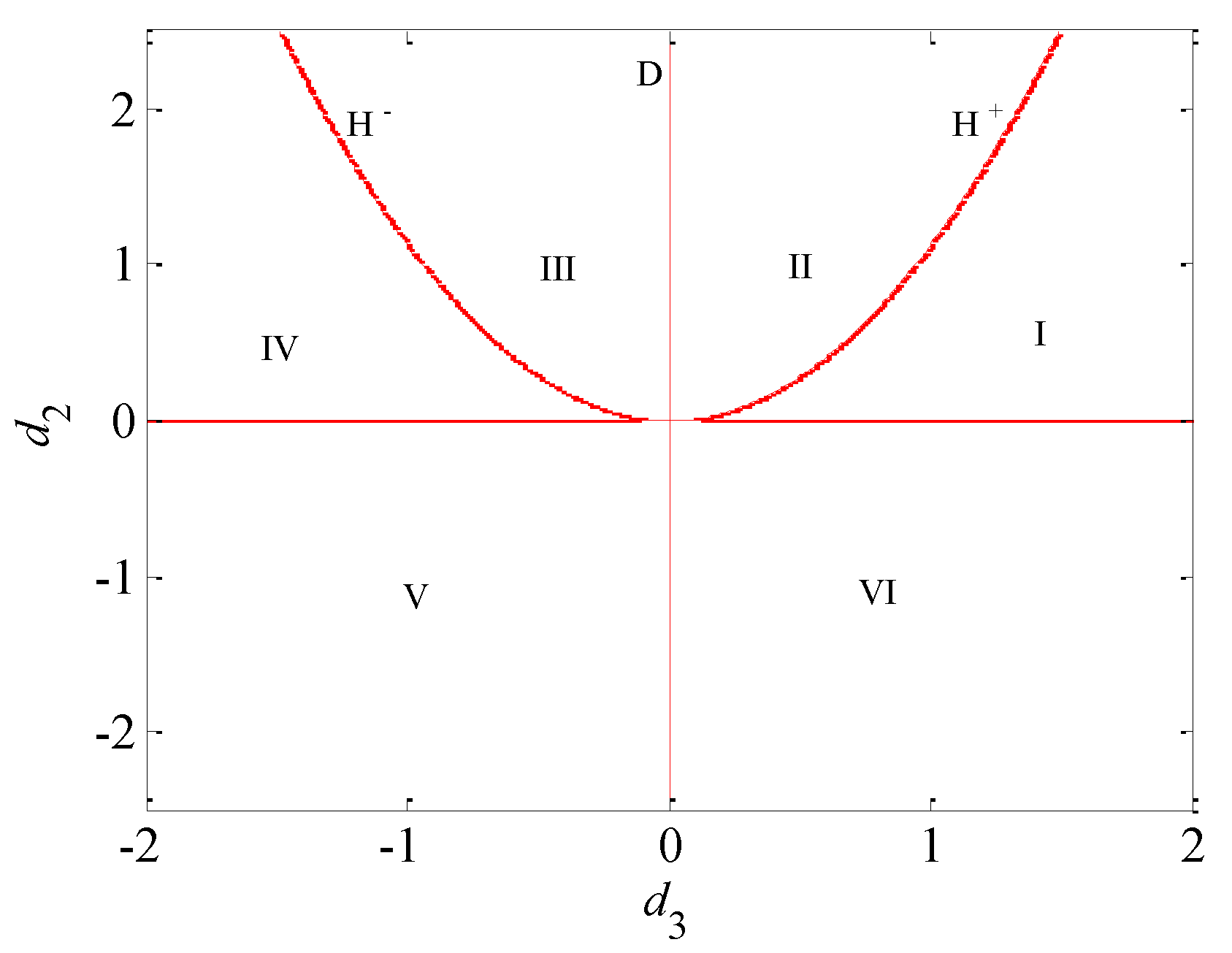
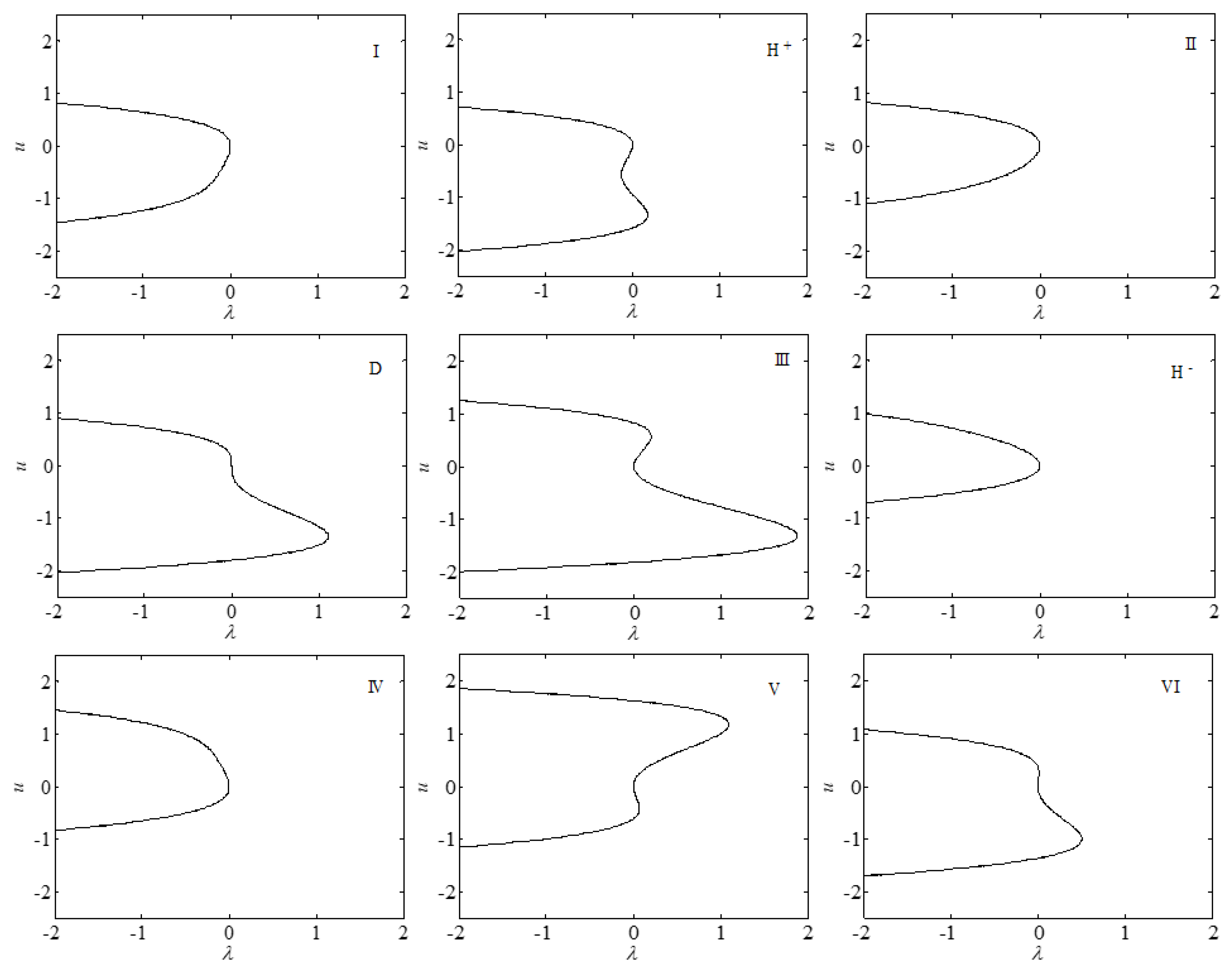
5.2. Analysis of Bifurcation Characteristics
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
| First Order Parameter of Taylor Expansion: | ||||||||
| Parameter item | x | ẋ | y | ẏ | ||||
| Proportion value | 0.0033 | 0.0000 | 3.6610 | 0.0028 | ||||
| Second Order Parameter of Taylor Expansion: | ||||||||
| parameter item | x2 | xẋ | xy | xẏ | ẋ2 | |||
| Proportion value | 0.0000 | 0.0000 | 0.0000 | 0.0000 | 0.0000 | |||
| parameter item | ẋy | ẋẏ | y2 | yẏ | ẏ2 | |||
| Proportion value | 0.0002 | 0.0201 | 1.6583 | 0.0005 | 0.0002 | |||
| Cubic Order Parameter of Taylor Expansion: | ||||||||
| parameter item | ẋ2ẏ | ẋ3 | xẋ2 | ẋẏ2 | ẏ3 | x2y | x3 | ẋ2y |
| Proportion value | 0.0000 | 0.0000 | 0.0000 | 0.0000 | 0.0000 | 0.0000 | 0.0000 | 0.0000 |
| parameter item | xẋẏ | xyẏ | y3 | ẋyẏ | y2ẏ | yẏ2 | ẋy2 | xy2 |
| Proportion value | 0.0000 | 0.0000 | 0.1169 | 0.0000 | 0.0000 | 0.0000 | 0.0000 | 0.0013 |
| First Order Parameter of Taylor Expansion: | ||||||||
| Parameter item | x | ẋ | y | ẏ | ||||
| Proportion value | 0.0229 | 0.0000 | 0.0000 | 0.0344 | ||||
| Second Order Parameter of Taylor Expansion: | ||||||||
| parameter item | x2 | xẋ | xy | xẏ | ẋ2 | |||
| Proportion value | 0.0013 | 0.0000 | 0.0532 | 0.0001 | 0.0029 | |||
| parameter item | ẋy | ẋẏ | y2 | yẏ | ẏ2 | |||
| Proportion value | 0.0001 | 0.0001 | 2.9445 | −0.0000 | −0.0003 | |||
| Cubic Order Parameter of Taylor Expansion: | ||||||||
| parameter item | ẋ2ẏ | ẋ3 | xẋ2 | ẋẏ2 | ẏ3 | x2y | x3 | ẋ2y |
| Proportion value | 0.0000 | 0.0000 | 0.0000 | 0.0000 | 0.0000 | 0.0002 | 0.0000 | 0.0000 |
| parameter item | xẋẏ | xyẏ | y3 | ẋyẏ | y2ẏ | yẏ2 | ẋy2 | xy2 |
| Proportion value | 0.0000 | 0.0000 | 1.2374 | 0.0000 | 0.0004 | 0.0003 | 0.0001 | 0.0000 |
References
- Ubici, E.; Borda, M.; Klempnow, A.; Pieyro, J. Identification and countermeasures to resolve hot strip mill chatter. AISE Steel Technol. 2001, 6, 48–52. [Google Scholar]
- Yan, X.-Q.; Sun, Z.-H.; Chen, W. Vibration control in thin slab hot strip mills. Ironmak. Steelmak. 2011, 38, 309–313. [Google Scholar] [CrossRef]
- Younes, M.A.; Shahtout, M.; Damir, M.A. A parameters design approach to improve quality and equipment performance in hot rolling. J. Mater. Process. Technol. 2006, 171, 83–92. [Google Scholar] [CrossRef]
- Panjković, V.; Gloss, R.; Steward, J.; Dilks, S.; Steward, R.; Fraser, G. Causes of chatter in hot strip mill: Observation, qualitative analysers and mathematical modelling. J. Mater. Process. Technol. 2012, 212, 954–961. [Google Scholar] [CrossRef]
- Johnson, R.; Qi, Q. Chatter dynamics in sheet rolling. Int. J. Mech. Sci. 1994, 36, 617–630. [Google Scholar] [CrossRef]
- Jan, R.; Peter, M.; Jozef, S.; Vladimir, R.; Norbert, S. The research of the rolling speed influence on the mechanism of strip breaks in the steel rolling process. Meterials 2020, 13, 3509. [Google Scholar]
- Swiatoniowski, A.; Bar, A. Parametrical excitement vibration in tandem mills—mathematical model and its analysis. J. Mater. Process. Technol. 2003, 134, 214–224. [Google Scholar] [CrossRef]
- Fan, X.B.; Zang, Y.; Sun, Y.K.; Wang, P.A. Impact analysis of roller system stability for four-high mill horizontal vibration. Shock Vib. 2016, 2016, 5693584. [Google Scholar] [CrossRef]
- Shi, P.M.; Li, J.Z.; Jiang, J.S.; Liu, B.; Han, D.Y. Nonlinear dynamics of torsional vibration for rolling mill’s main drive system under parametric excitation. J. Iron. Steel. Res. Int. 2013, 20, 7–12. [Google Scholar] [CrossRef]
- Tang, H.P.; Wang, D.Y.; Zhong, J. Investigation into the electronmechanical coupling unstability of a rolling mill. J. Mater. Process. Technol. 2002, 129, 294–298. [Google Scholar] [CrossRef]
- Liu, S.; Wang, Z.L.; Wang, J.J.; Li, H.B. Sliding bifurcation research of a horzontal-torsional coupled main drive system of rolling mill. Nonlinear. Dynam. 2016, 83, 441–455. [Google Scholar] [CrossRef]
- Liu, S.; Ai, H.L.; Pang, Z.F.; Lin, Z.J.; Zhao, D.X. Hopf bifurcation control of nonlinear electromechanical coupling main drive system of rolling mill. Eur. Phys. J. Plus 2020, 135, 365. [Google Scholar] [CrossRef]
- Peng, Y.; Zhang, Y.; Sun, J.L.; Zang, Y. Tandem strip mill’s multi-parameter coupling dynamic modeling based on the thickness control. Chin. J. Mech. Eng. 2015, 28, 353–362. [Google Scholar] [CrossRef]
- Wang, Q.Y.; Jiang, Z.Y.; Zhao, J.W.; Fang, M. Multi-factor coupling system characteristic of the dynamic roll gap in the high-speed rolling mill during the unsteady lubrication process. Tribol. Int. 2013, 67, 174–181. [Google Scholar] [CrossRef][Green Version]
- Xu, H.; Cui, L.L.; Shang, D.G. A study of nonlinear coupling dynamic characteristics of the cold rolling mill system under different rolling parameters. Adv. Mech. Eng. 2017, 9, 1–15. [Google Scholar] [CrossRef]
- Liu, B.; Jiang, J.H.; Liu, F.; Liu, H.R.; Li, P. Nonlinear vibration characteristic of strip mill under the coupling effect of roll-rolled piece. J. Vibroeng. 2016, 18, 492–5505. [Google Scholar] [CrossRef]
- Liu, Z.L.; Jiang, J.H.; Li, P.; Pan, G.X.; Liu, B. Dynamic analysis and control of strip mill vibration under the coupling effect of roll and rolled piece. J. Vibroeng. 2017, 19, 6079–6093. [Google Scholar] [CrossRef]
- Qian, C.; Zhang, L.L.; Hua, C.C.; Bai, Z.H. Adaptive fuzzy vertical vibration suppression control of the mechanical-hydraulic coupling rolling mill system with input dead-zone and output constraints. IEEE Access 2020, 8, 85793. [Google Scholar] [CrossRef]
- Younghae, D.; Sang, D.K.; Phil, S.K. Stability of fixed points placed on the border in the piecewise linear systems. Chaos. Soliton. Fract. 2008, 38, 391–399. [Google Scholar]
- Wang, K.Q.; Yu, P.; Wu, Z.Q. Bifurcation analysis on a self-excited hysteretic system. Int. J. Bifurcat. Chaos 2004, 14, 2825–2842. [Google Scholar]
- Zhu, Y.; Qian, P.F.; Tang, S.N.; Jiang, W.L.; Li, W.; Zhao, J.H. Amplitude-frequency characteristics analysis for vertical vibration of hydraulic AGC system under nonlinear action. Aip. Adv. 2019, 9, 035019. [Google Scholar] [CrossRef]
- Lorinc, M.; Szabolcs, F.; Nariman, S. A practical method for friction identification in hydraulic actuators. Mechatronics 2011, 21, 350–356. [Google Scholar]
- Peng, R.R.; Zhang, X.Z.; Shi, P.M. Coupled vibration behavior of hot rolling mill rolls under multinonlinear effects. Shock. Vib. 2020, 2020, 6104028. [Google Scholar] [CrossRef]
- Tran, X.B.; Hafizah, N.; Yanada, H. Modeling of dynamic friction behaviors of hydraulic cylinders. Mechatronics 2012, 22, 65–75. [Google Scholar] [CrossRef]
- Hou, D.X.; Peng, R.R.; Liu, H.R. Analysis of vertical-horizontal coupling vibration characteristics of rolling mill rolls based on strip dynamic deformation process. Shock. Vib. 2014, 2014, 543793. [Google Scholar] [CrossRef]
- Sims, R.B. The calculation of roll force and torque in hot rolling mills. Proc. Inst. Mech. Eng. 1954, 168, 191–200. [Google Scholar] [CrossRef]
- Yang, X.; Tong, C.N.; Yue, G.F.; Meng, J.J. Coupling dynamic model of chatter for cold rolling. J. Iron. Steel Res. Int. 2010, 17, 30–34. [Google Scholar] [CrossRef]
- Hu, P.H. Stability and Chatter in Rolling; Northwestern University: Evanston, IL, USA, 1998. [Google Scholar]
- Raffa, F.A.; Vatta, F. The dynamic stiffness method for linear rotor-bearing systems. J. Vib. Acoust. 1996, 118, 332–339. [Google Scholar] [CrossRef]
- Hu, P.H.; Ehmann, K.F. A dynamic model of the rolling process. Part 1: Homogenous model; Part 2: Inhomogeneous model. Int. J. Mach. Tool. Manuf. 2000, 40, 1–31. [Google Scholar] [CrossRef]
- Roberts, W.L. Fourth mill stand chatter of the fifth-octave mode. Iron. Steel. Eng. 1978, 55, 206–216. [Google Scholar]




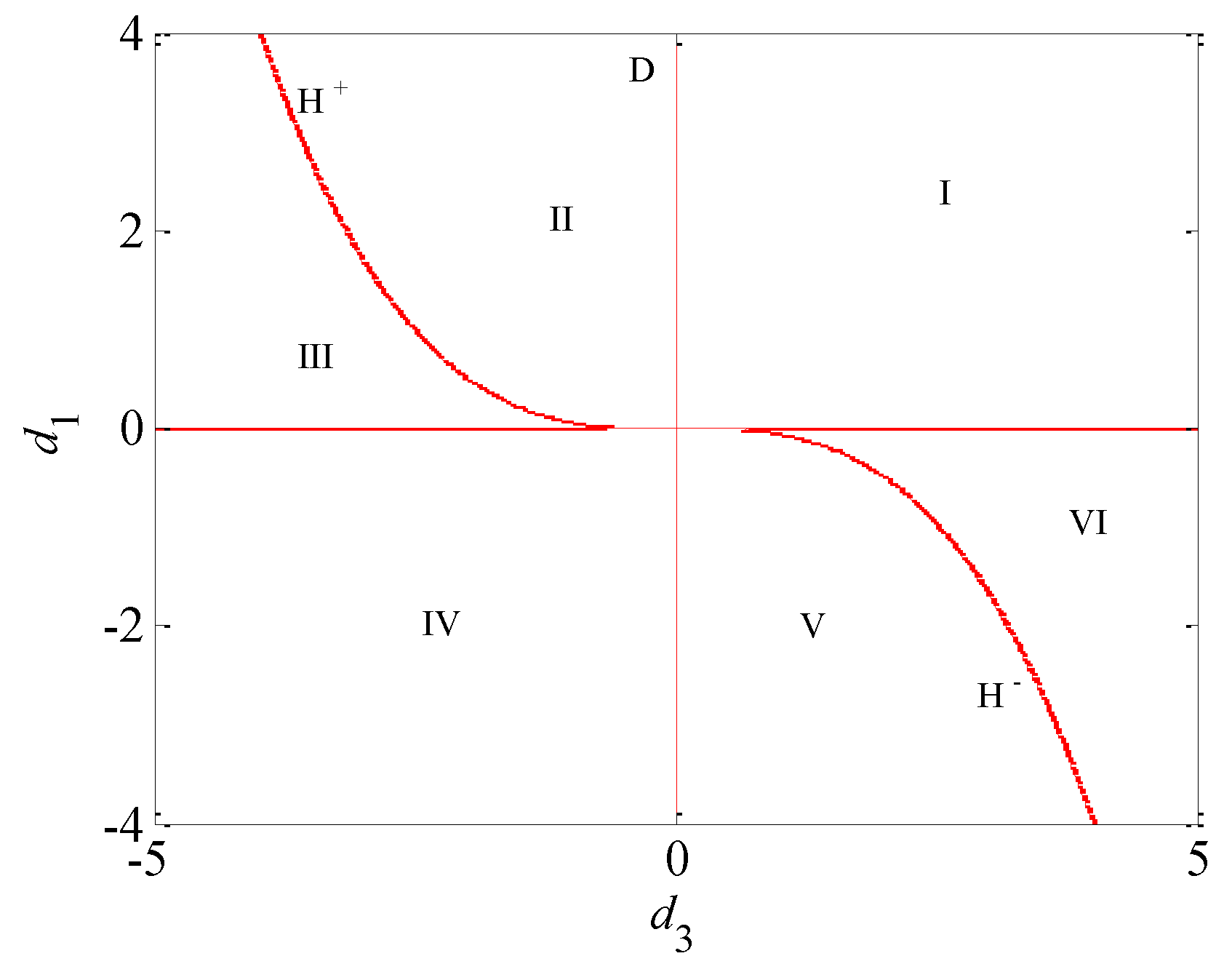



| Parameters | Value | Parameters | Value | Parameters | Value |
|---|---|---|---|---|---|
| h0 (m) | 0.0141 | k1 (N/m) | 7.31 × 109 | e1 (mm) | 0.6 |
| h1 (m) | 0.0082 | k2 (N/m) | 1.68 × 1010 | e2 (mm) | −0.2 |
| R (m) | 0.42 | k3 (N/m) | 1.52 × 1011 | vm (m/s) | 0.01 |
| v0 (m/s) | 2.5 | c0 (N·s/m) | 8.85 × 105 | pf (MN) | 0.04 |
| τb (MPa) | 5.5 | c1 (N·s/m) | 5.20 × 103 | μx | 0.26 |
| τf (MPa) | 3.8 | c3 (N·s/m) | 7.83 × 105 | μs | 0.02 |
| m (Kg) | 1.44 × 105 | ks1 (N/m) | 4.09 × 1010 | μm | 0.01 |
| k0 (N/m) | 2.35 × 1010 | kw1 (N/m) | 2.08 × 1010 | Δx (m) | 1 × 10−3 |
| Parameters | Value | Parameters | Value | Parameters | Value |
|---|---|---|---|---|---|
| a1 (N/m) | 5.8 × 106 | a5 (N·s2/m2) | 3613.40 | b3 (N/m2) | 1.70 × 1010 |
| a2 (N/m) | 1.46 × 108 | a6 (N/m3) | −7.47 × 1011 | b4 (N·s2/m2) | −152.85 |
| a3 (N·s/m) | −1131.23 | b1 (N/m) | 6.98 × 107 | b5 (N/m2) | 4.7 × 1011 |
| a4 (N/m2) | 2.65 × 1010 | b2 (N·s/m) | 1.37 × 104 | b6 (N/m3) | −7.90 × 1013 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Peng, R.; Zhang, X.; Shi, P. Vertical–Horizontal Coupling Vibration of Hot Rolling Mill Rolls under Multi-Piecewise Nonlinear Constraints. Metals 2021, 11, 170. https://doi.org/10.3390/met11010170
Peng R, Zhang X, Shi P. Vertical–Horizontal Coupling Vibration of Hot Rolling Mill Rolls under Multi-Piecewise Nonlinear Constraints. Metals. 2021; 11(1):170. https://doi.org/10.3390/met11010170
Chicago/Turabian StylePeng, Rongrong, Xingzhong Zhang, and Peiming Shi. 2021. "Vertical–Horizontal Coupling Vibration of Hot Rolling Mill Rolls under Multi-Piecewise Nonlinear Constraints" Metals 11, no. 1: 170. https://doi.org/10.3390/met11010170
APA StylePeng, R., Zhang, X., & Shi, P. (2021). Vertical–Horizontal Coupling Vibration of Hot Rolling Mill Rolls under Multi-Piecewise Nonlinear Constraints. Metals, 11(1), 170. https://doi.org/10.3390/met11010170






