Optimization of Manganese Recovery from a Solution Based on Lithium-Ion Batteries by Solvent Extraction with D2EHPA
Abstract
1. Introduction
2. Materials and Methods
Experimental Design
3. Results and Discussion
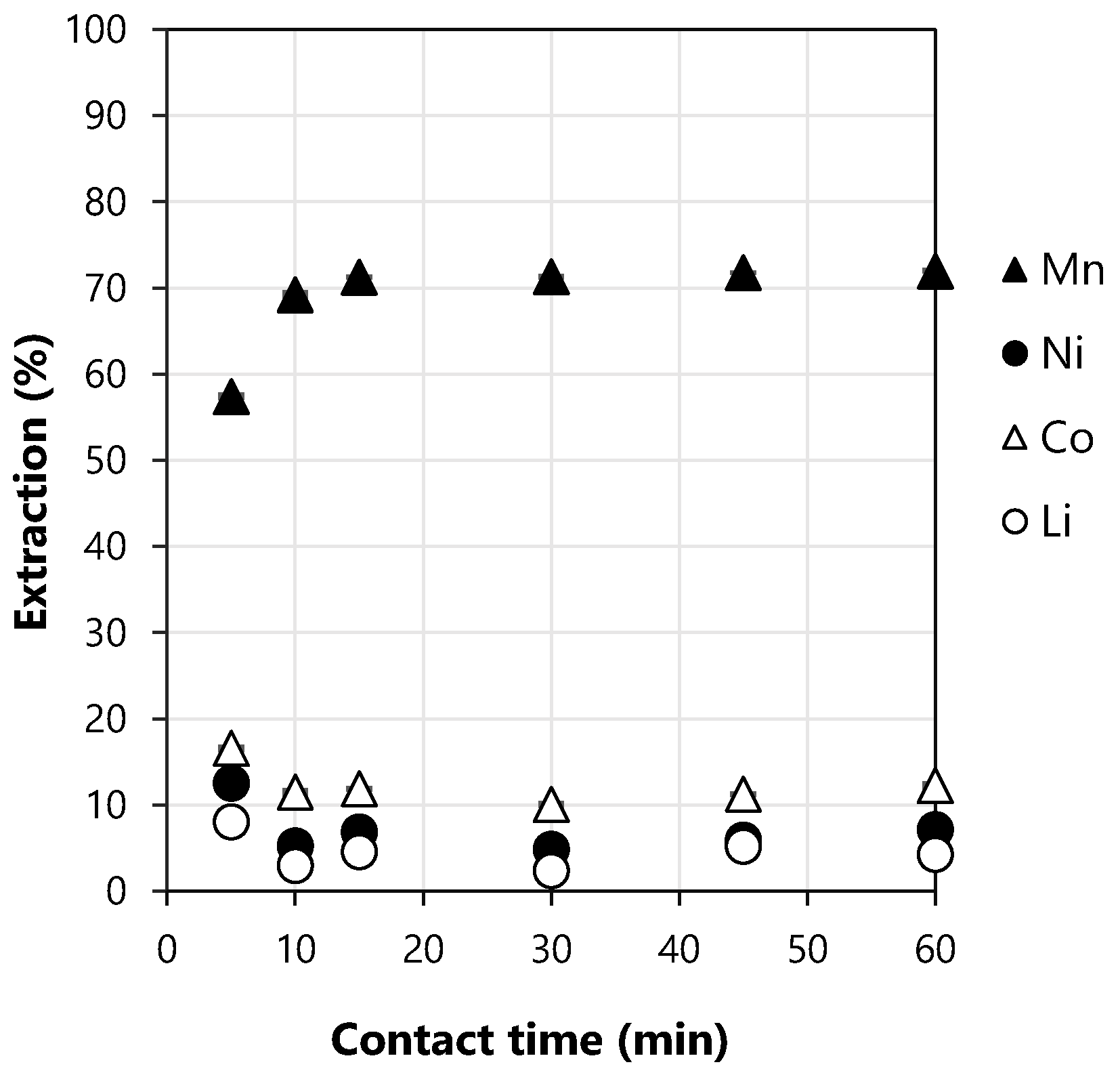
3.1. Preliminary Tests of Extraction
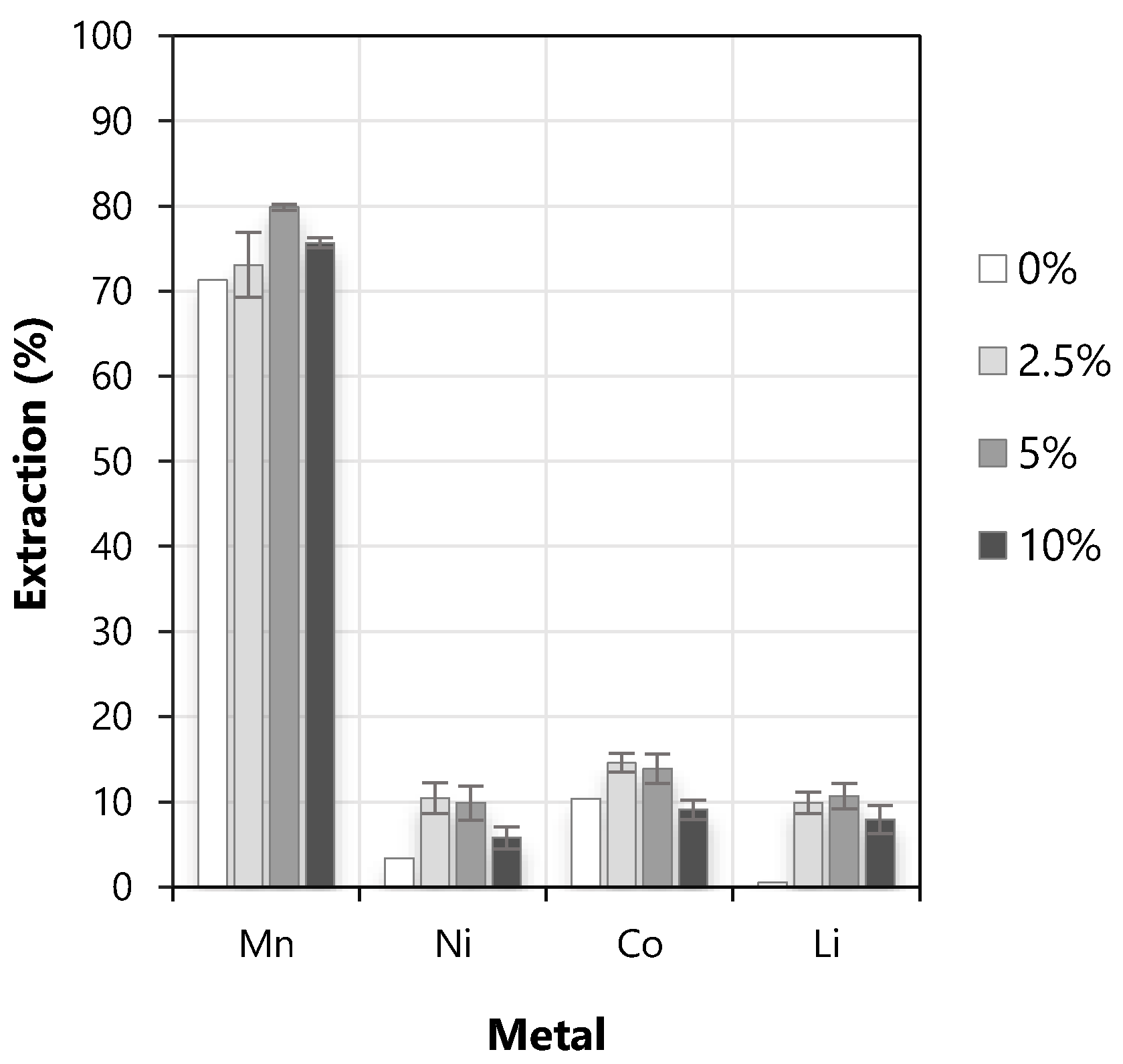
3.2. Effect of the Concentration of Modifier (% Volume of TBP)
3.3. Effect of the pH on the Extraction of Metals
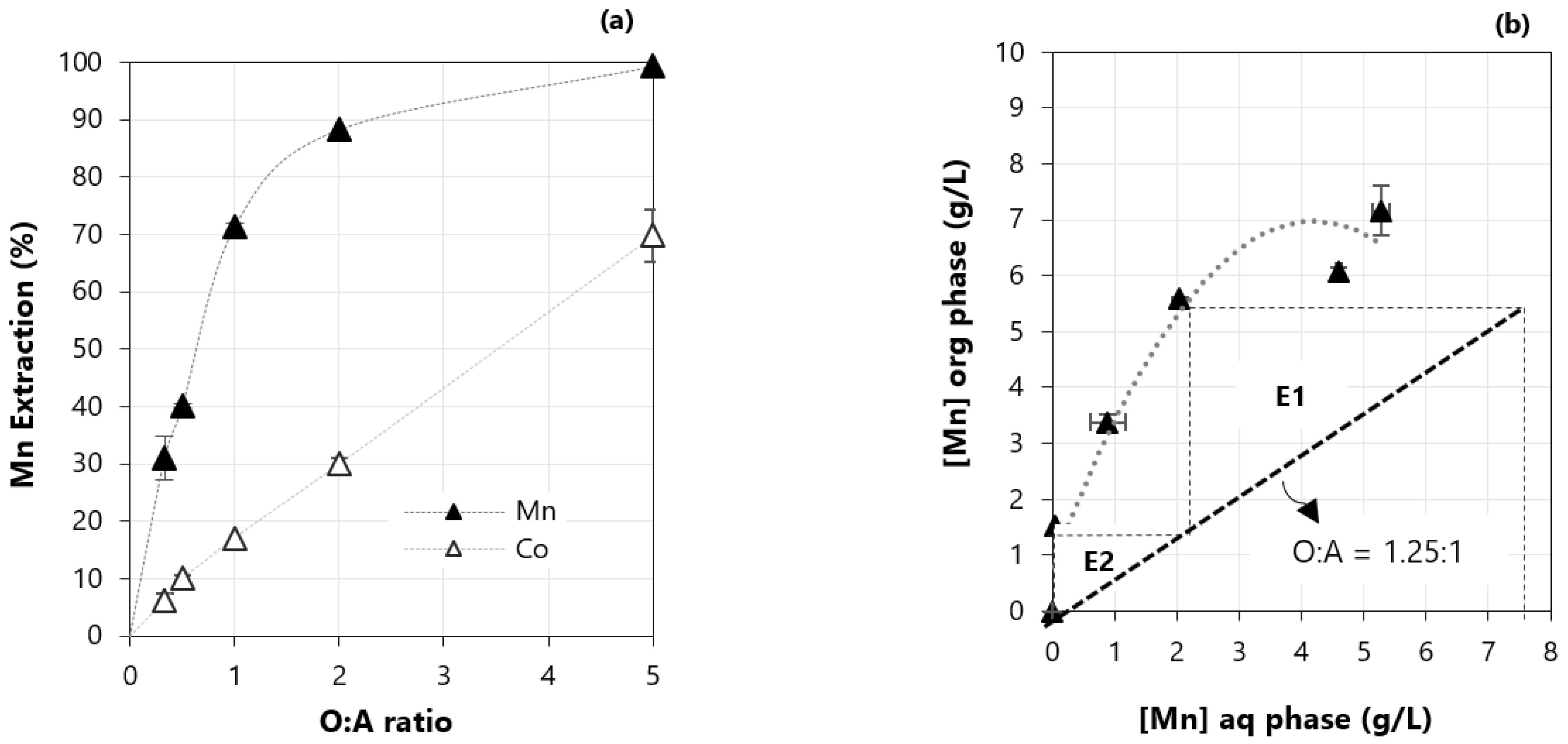
3.4. Effect of the Organic to Aqueous Ratio (O:A)
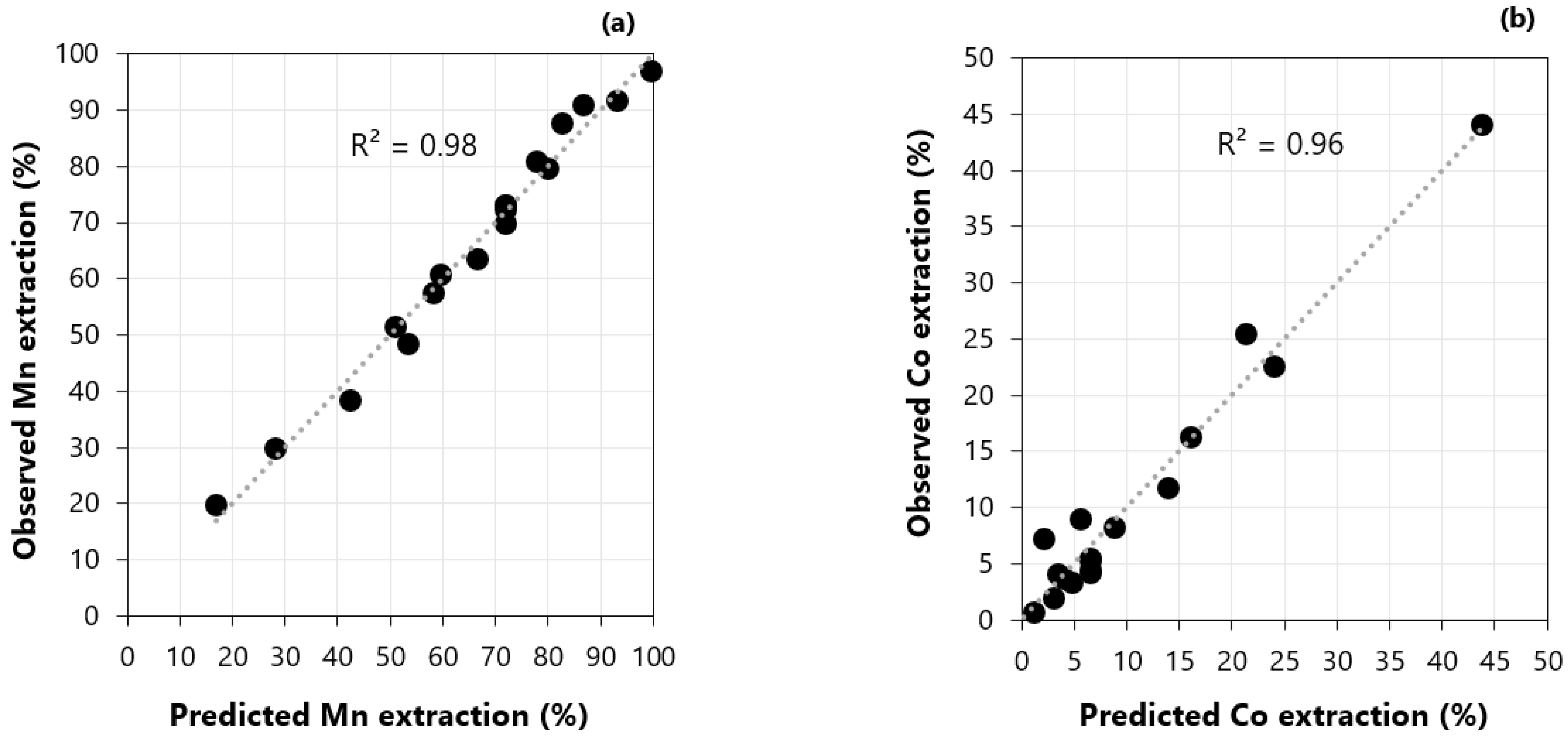
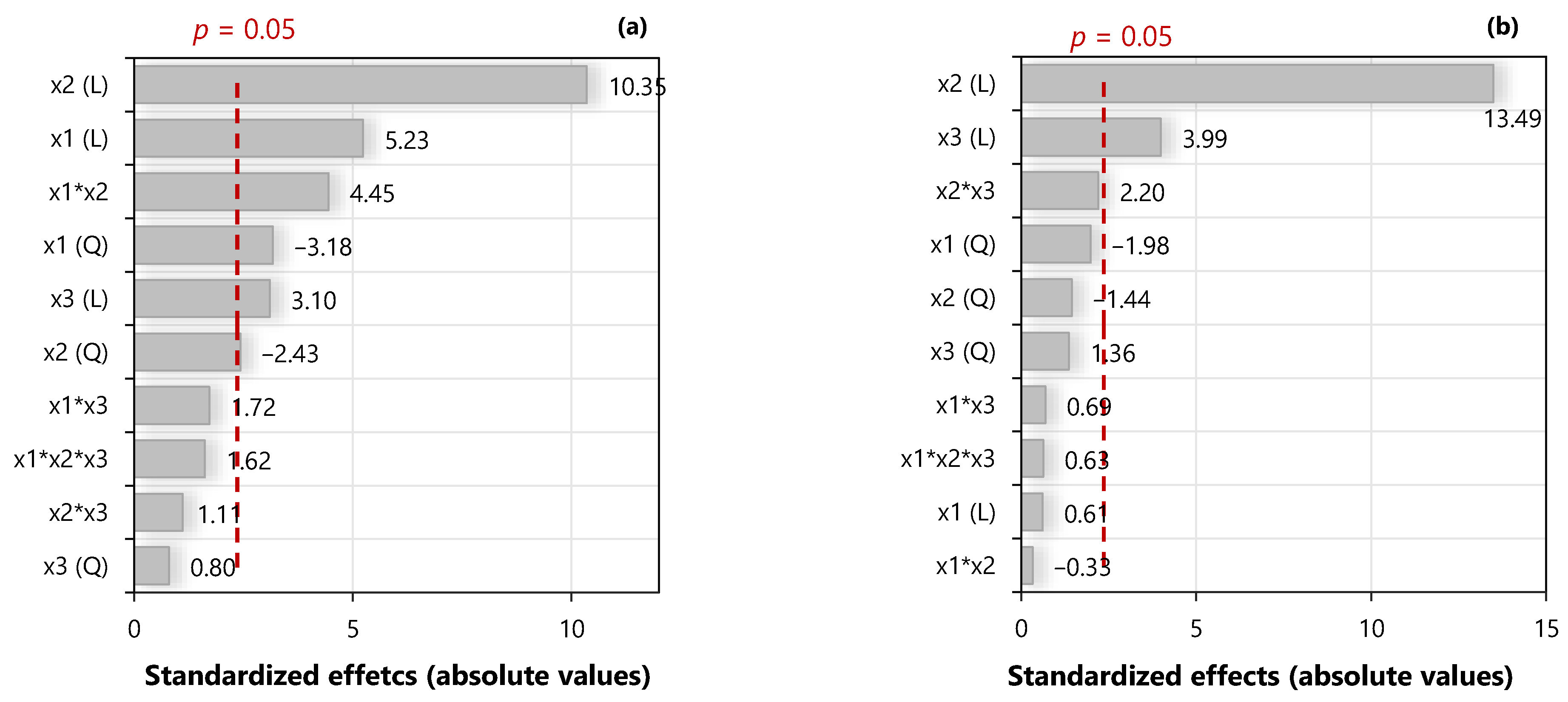
3.5. Extraction Stage: Factorial Design of Experiments and Regression Model
3.6. Response Surfaces: Extraction of Manganese and Cobalt
3.7. Scrubbing of the Loaded Organic
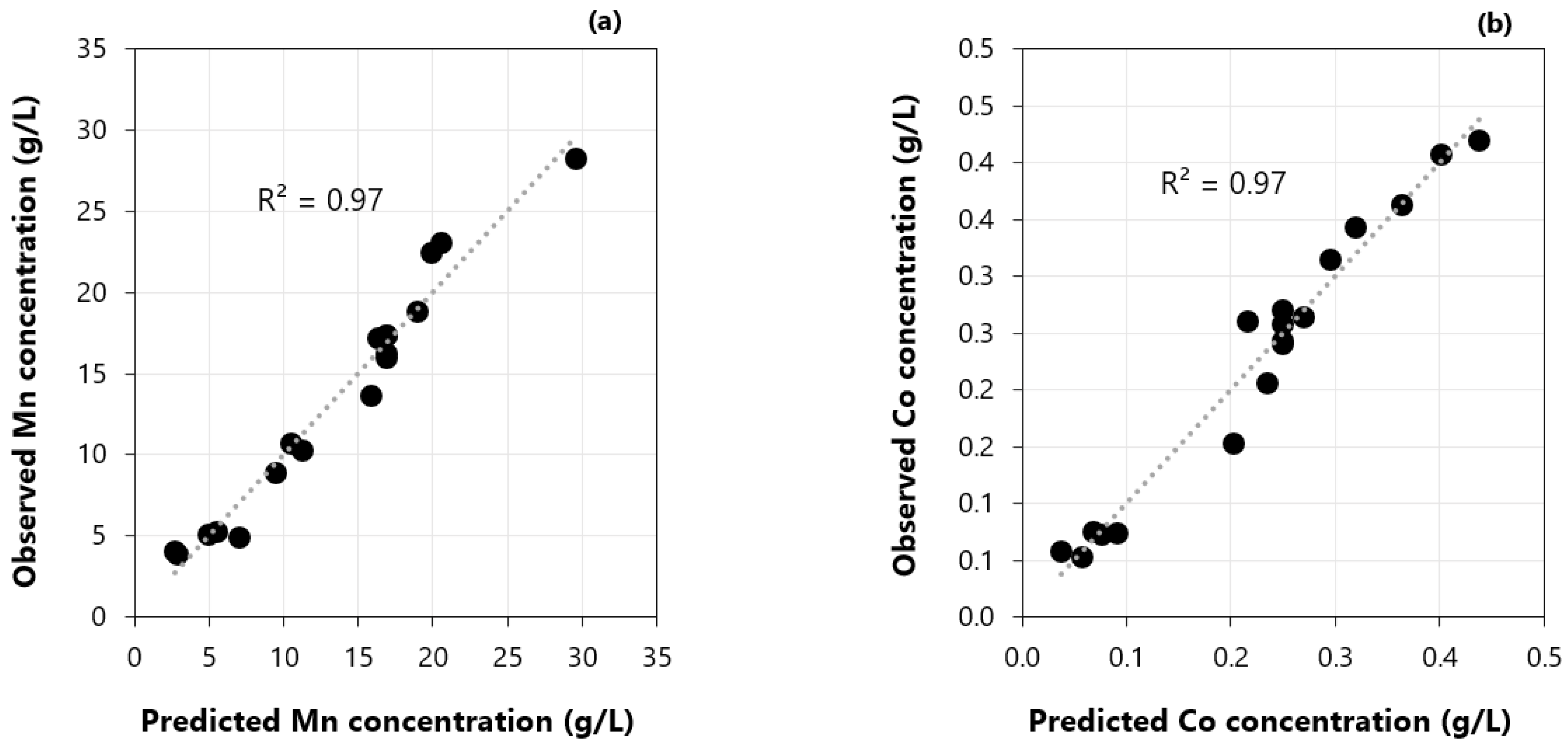
3.8. Stripping Stage: Factorial Design of Experiments and Regression Model
3.9. Response Surfaces: Stripping of Manganese and Cobalt
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- The Royal Society of Chemistry, Manganese. Available online: https://www.rsc.org/periodic-table/element/25/manganese (accessed on 21 November 2020).
- Corathers, L.A.; U.S. Geological Survey. Manganese. In Mineral Commodity Summaries; 2020; p. 105. Available online: https://pubs.usgs.gov/periodicals/mcs2020/mcs2020.pdf (accessed on 10 October 2020).
- South Australia, Government of South Australia, Department of Energy and Mining, Manganese. Available online: https://energymining.sa.gov.au/minerals/mineral_commodities/manganese (accessed on 21 November 2020).
- Cardarelli, F. Materials Handbook: A Concise Desktop Reference, 2nd ed.; Springer: London, UK, 2008; ISBN 978-1-84628-668-1. [Google Scholar]
- United States Government Printing Office. Strategic and Critical Materials. Hearings before a Subcommittee of the Committee on Military Affairs, United States Senate, Seventy-Seventh Congress, First Session, Relative to Strategic and Critical Materials and Minerals; Printed for the use of the Committee on Military Affairs, Washington: Fairfax, VA, USA, 1941. [Google Scholar]
- Manganese Reserves and Resources of the World and Their Industrial Implications; National Academies Press: Washington, DC, USA, 1981; ISBN 978-0-309-32998-9.
- Maynard, J.B. Manganese Rocks and Ores. In Isotope Geochemistry: The Origin and Formation of Manganese Rocks; Elsevier: Amsterdam, The Netherlands, 2017; ISBN 9780128031650. [Google Scholar]
- Thackeray, M.M.; Croy, J.R.; Lee, E.; Gutierrez, A.; He, M.; Park, J.S.; Yonemoto, B.T.; Long, B.R.; Blauwkamp, J.D.; Johnson, C.S.; et al. The quest for manganese-rich electrodes for lithium batteries: Strategic design and electrochemical behavior. Sustain. Energy Fuels 2018, 2, 1375–1397. [Google Scholar] [CrossRef]
- Olivetti, E.A.; Ceder, G.; Gaustad, G.G.; Fu, X. Lithium-Ion Battery Supply Chain Considerations: Analysis of Potential Bottlenecks in Critical Metals. Joule 2017, 1, 229–243. [Google Scholar] [CrossRef]
- Cannon, W.F.; Kimball, B.E.; Corathers, L.A. Manganese; Schulz, K.J., DeYoung John, H., Jr., Seal, R.R., II, Bradley, D.C., Eds.; The U.S. Geological Survey: Reston, VA, USA, 2017; series number 1802; ISBN 978-1-4113-3991-0. Available online: https://pubs.er.usgs.gov/publication/pp1802L (accessed on 21 November 2020).
- Statista, Projected Size of the Global Lithium-Ion Battery Market from 2020 to 2025. Available online: https://www.statista.com/statistics/1011187/projected-global-lithium-ion-battery-market-size/ (accessed on 21 November 2020).
- Roskill Manganese Outlook to 2030, 16th ed; Available online: https://roskill.com/market-report/manganese/ (accessed on 25 November 2020).
- Chow, N. Manganese ore for lithium batteries. Metal Powder Rep. 2012, 67, 34–36. [Google Scholar] [CrossRef]
- Zhang, W.; Cheng, C.Y. Manganese metallurgy review. Part I: Leaching of ores/secondary materials and recovery of electrolytic/chemical manganese dioxide. Hydrometallurgy 2007, 89, 137–159. [Google Scholar] [CrossRef]
- Biswal, A.; Chandra Tripathy, B.; Sanjay, K.; Subbaiah, T.; Minakshi, M. Electrolytic manganese dioxide (EMD): A perspective on worldwide production, reserves and its role in electrochemistry. RSC Adv. 2015, 5, 58255–58283. [Google Scholar] [CrossRef]
- Moore Stephans. Manganese. Is It the Forgotten Battery Mineral? Snapshot Worldwide Manganese Ore Prices. Available online: https://www.moorestephens.com.au/MediaLibsAndFiles/media/australia.moorestephens.com/Images/Profile%20Photos%20(Contact%20boxes)%20110w%20x%20110h%20px/Western%20Australia/Manganese-Moore-Stephens-Report.pdf (accessed on 25 November 2020).
- Jones, T.S. Manganese Recycling in the United States in 1998; US Department of the Interior, US Geological Survey: Reston, VA, USA, 2001. [Google Scholar] [CrossRef]
- Bullis, L.H.; Mielke, J.E. Strategic and Critical Materials; Routledge: New York, NY, USA, 2019; ISBN 9780367288822. [Google Scholar]
- Granata, G.; Pagnanelli, F.; Moscardini, E.; Takacova, Z.; Havlik, T.; Toro, L. Simultaneous recycling of nickel metal hydride, lithium ion and primary lithium batteries: Accomplishment of European Guidelines by optimizing mechanical pre-treatment and solvent extraction operations. J. Power Sources 2012, 212, 205–211. [Google Scholar] [CrossRef]
- Peng, C.; Chang, C.; Wang, Z.; Wilson, B.P.; Liu, F.; Lundström, M. Recovery of High-Purity MnO2 from the Acid Leaching Solution of Spent Li-Ion Batteries. JOM 2020, 72, 790–799. [Google Scholar] [CrossRef]
- Yang, Y.; Xu, S.; He, Y. Lithium recycling and cathode material regeneration from acid leach liquor of spent lithium-ion battery via facile co-extraction and co-precipitation processes. Waste Manag. 2017, 64, 219–227. [Google Scholar] [CrossRef] [PubMed]
- Wang, F.; Sun, R.; Xu, J.; Chen, Z.; Kang, M. Recovery of cobalt from spent lithium ion batteries using sulphuric acid leaching followed by solid–liquid separation and solvent extraction. RSC Adv. 2016, 6, 85303–85311. [Google Scholar] [CrossRef]
- Joo, S.-H.; Shin, S.M.; Shin, D.; Oh, C.; Wang, J.-P. Extractive separation studies of manganese from spent lithium battery leachate using mixture of PC88A and Versatic 10 acid in kerosene. Hydrometallurgy 2015, 156, 136–141. [Google Scholar] [CrossRef]
- Chen, X.; Chen, Y.; Zhou, T.; Liu, D.; Hu, H.; Fan, S. Hydrometallurgical recovery of metal values from sulfuric acid leaching liquor of spent lithium-ion batteries. Waste Manag. 2015, 38, 349–356. [Google Scholar] [CrossRef] [PubMed]
- Pagnanelli, F.; Moscardini, E.; Altimari, P.; Abo Atia, T.; Toro, L. Cobalt products from real waste fractions of end of life lithium ion batteries. Waste Manag. 2016, 51, 214–221. [Google Scholar] [CrossRef] [PubMed]
- Li, J.; Yang, X.; Yin, Z. Recovery of manganese from sulfuric acid leaching liquor of spent lithium-ion batteries and synthesis of lithium ion-sieve. J. Environ. Chem. Eng. 2018, 6, 6407–6413. [Google Scholar] [CrossRef]
- Devi, N.B.; Nathsarma, K.C.; Chakravortty, V. Separation of divalent manganese and cobalt ions from sulphate solutions using sodium salts of D2EHPA, PC 88A and Cyanex 272. Hydrometallurgy 2000, 54, 117–131. [Google Scholar] [CrossRef]
- Hossain, M.R.; Nash, S.; Rose, G.; Alam, S. Cobalt loaded D2EHPA for selective separation of manganese from cobalt electrolyte solution. Hydrometallurgy 2011, 107, 137–140. [Google Scholar] [CrossRef]
- Cheng, C.Y. Purification of synthetic laterite leach solution by solvent extraction using D2EHPA. Hydrometallurgy 2000, 56, 369–386. [Google Scholar] [CrossRef]
- Chen, X.; Zhou, T.; Kong, J.; Fang, H.; Chen, Y. Separation and recovery of metal values from leach liquor of waste lithium nickel cobalt manganese oxide based cathodes. Sep. Purif. Technol. 2015, 141, 76–83. [Google Scholar] [CrossRef]
- Ahan, S.C.; Jeon, B.K.; Kim, B.E.; Lee, M.J.; Sonu, C.H. Method for Recovering Valuable Metals from Lithium Secondary Battery Wastes; 2010. Available online: https://patents.google.com/patent/WO2012050317A3/en (accessed on 25 November 2020).
- Jouni, P.; Erkki, P. Recovery of Manganese from Mixed Metal Solutions by Solvent Extraction with Organophosphorus Acid Extractants; Saint-Petersburg Mining University: St Petersburg, Russia, 2008. [Google Scholar]
- Feather, A.; Sole, K.C.; Dreisinger, D.B. Pilot-plant evaluation of manganese removal and cobalt purification by solvent extraction. In Proceedings of the ISEC’99; Bercelona, Spain, 1999, Society of Chemical Industry (SCI): London, UK, 2001; Volume 2, ISBN 0-901001-83-8. Available online: http://www.solventextract.org/documents/1999/ISEC-1999-Proceedings-Vol2.pdf (accessed on 25 November 2020).
- Cole, P.M. The introduction of solvent-extraction steps during upgrading of a cobalt refinery. Hydrometallurgy 2002, 64, 69–77. [Google Scholar] [CrossRef]
- Montgomery, D.C. Design and Analysis of Experiments, 8th ed; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2012; ISBN 978-1-118-14692-7. [Google Scholar]
- Ritcey, G.M.; Ashbrook, A.W. Solvent Extraction: Principles and Applications to Process Metallurgy; Elsevier: Amsterdam, The Netherlands, 1984; ISBN 0444417702. [Google Scholar]




| Extractant | Saponification | Modifier | O:A | Optimum pH | Temperature (°C) | Contact Time (min) | Feed | Initial Composition (g/L) | %E (Mn) | Reference | |||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Mn | Co | Ni | Li | ||||||||||
| 0.4 M D2EHPA | - | - | 2:1 | 3.2 | 25 | 15 | Leach solution produced from spent LIBs (acid leaching with H2SO4 and H2O2) | 3.66 | 19.33 | 5.19 | 3.58 | - | [20] |
| 15% D2EHPA | 60% (with 0.5 M ammonia) | 5% TBP | 1:1 | 2.25 | 25 | 5 | Leaching liquor of spent LIBs | 5.91 | 24.79 | 6.24 | 6.68 | 99.9 | [26] |
| 4 M (D2EHPA/Mn molar) | 65% (with NaOH 5 M) | 10% TBP | 1:1 | 3.8 | room | 10 | Electrodic LIB powder pre-leached with H2SO4 | 4.6 | 21.8 | 2.7 | 3.2 | ~90 | [25] |
| 0.05 M NaD2EHPA (best results) | - | 5% TBP | 9:8 | 2.7 | 30 | 5 | Stock solution with Mn and Co (0.01 M) | 0.01 M | 0.01 M | - | - | 99.94% | [27] |
| 15% Cobalt loaded D2EHPA | 70–75% (with NaOH 10 M) | 5% TBP | 1:1 | 3.2 | 25 | 5 | Sulfuric acid leaching liquor of mixed types of cathode materials (real sample) waste cathode materials | 6.31 | 6.45 | 6.89 | 1.6 | 99% | [24] |
| 20% PC88A/25% Versatic 10 | - | - | 1:1 | 4.5 | room | 5 | Leaching solution from spent LIBs | 11.7 | 11.4 | 12.2 | 5.3 | 99.5% | [23] |
| 25% Cobalt loaded D2EHPA | - | 1-decanol | 1:1 | 3.5 | 25 | 5 | Cobalt electrolyte solution | 0.8 | 55.7 | - | - | 100% (70% in one stage) | [28] |
| 10% D2EHPA | - | 5%TBP | 1:1 | 3.5 | 40 | 10 | Synthetic laterite solution containing Ni, Co, Mn, Mg, Zn, and Cu | 2 | 0.3 | 3 | - | 99% | [29] |
| 30% D2EHPA | 20% (with NaOH 10 M) | 5% TBP | 1:1 | 2.6–2.7 | room | 15 | Leaching solution from spent LIBs, treated with H2SO4 and H2O2 | 2 | 0.3 | 3 | - | Removal of Mn and Cu | [22] |
| 40% D2EHPA | - | - | 1:1 | 3.5 | room | 10 | Leaching acid solution from cathode material | 9.18 | 11.32 | 11.51 | 1.76 | ~100 | [21] |
| 20% D2EHPA (0.6 M) | 70–75% (with NaOH 10 M) | - | 1:2 | 4–5 | 25 | 5 | Co, Ni, and Li were removed by precipitation | 5.27 | 5.84 | 4.93 | 1.25 | 97% | [30] |
| D2EHPA | - | - | - | 2.5–3.5 | n.i. | n.i. | Leach liquor from LIBs | 5–30 | 5 to 45 | 5 to 30 | 1 to 10 | 100% | [31] |
| 25% D2EHPA (Cyanex 272 was also tested) | - | - | 1:1.5 | 2.7 | 5 and 25 | n.i. | Synthetic sulfuric acid solutions (Ca, Mn, Na, and Mg) | 0.58–5.3 | - | - | - | 65% | [32] |
| D2EHPA | - | - | 1:1–1:5 | 2.2–2.3 | 40 | continuous | Kakanda tailings (Cu and Co recovery in RDC) | 1.3 | 3 | - | - | 70–90% | [33] |
| 20% D2EHPA | - | - | 1:1 | 2.2–2.3 | n.i. | continuous | Cobalt bearing feed from a cobalt refinery in South Africa. Fe and Cu were first precipitated | 0.1 | 5.5 | - | - | 100% | [34] |
| Stage | Factors | Unit | Levels | ||
|---|---|---|---|---|---|
| Low (−1) | Standard (0) | High (+1) | |||
| Extraction | Equilibrium pH (x1) * | dimensionless | 2.5 | 3.25 | 4.0 |
| Organic to aqueous phase, O:A (x2) | dimensionless | 0.5 | 1.25 | 2 | |
| Concentration of D2EHPA (x3) | M | 0.4 | 0.5 | 0.6 | |
| Stripping | Concentration of H2SO4 (x1) | M | 0.05 | 1.025 | 2 |
| Organic to aqueous phase, O:A (x2) | dimensionless | 1 | 4.5 | 8 | |
| Stripping time (x3) | min | 2 | 13.5 | 25 | |
| Run Order | Std Order | Coded Variables | Real Variables | Response (Extraction) | |||||
|---|---|---|---|---|---|---|---|---|---|
| x1 | x2 | x3 | pH | O:A | D2EHPA | Mn (%) | Co (%) | ||
| 6 | 1 | −1 | −1 | −1 | 2.5 | 0.5 | 0.4 | 20 | 2 |
| 11 | 2 | 1 | −1 | −1 | 4 | 0.5 | 0.4 | 51 | 12 |
| 10 | 3 | −1 | 1 | −1 | 2.5 | 2 | 0.4 | 61 | 4 |
| 4 | 4 | 1 | 1 | −1 | 4 | 2 | 0.4 | 92 | 23 |
| 14 | 5 | −1 | −1 | 1 | 2.5 | 0.5 | 0.6 | 30 | 4 |
| 5 | 6 | 1 | −1 | 1 | 4 | 0.5 | 0.6 | 57 | 8 |
| 12 | 7 | −1 | 1 | 1 | 2.5 | 2 | 0.6 | 79 | 1 |
| 13 | 8 | 1 | 1 | 1 | 4 | 2 | 0.6 | 97 | 44 |
| 18 | 9 | 0 | 0 | 0 | 3.25 | 1.25 | 0.5 | 72 | 4 |
| 8 | 10 | 0 | 0 | 0 | 3.25 | 1.25 | 0.5 | 73 | 5 |
| 7 | 11 | 0 | 0 | 0 | 3.25 | 1.25 | 0.5 | 73 | 5 |
| 9 | 12 | 0 | 0 | 0 | 3.25 | 1.25 | 0.5 | 70 | 4 |
| 15 | 13 | −1 | 0 | 0 | 2.5 | 1.25 | 0.5 | 48 | 1 |
| 16 | 14 | 1 | 0 | 0 | 4 | 1.25 | 0.5 | 88 | 25 |
| 2 | 15 | 0 | −1 | 0 | 3.25 | 0.5 | 0.5 | 38 | 9 |
| 1 | 16 | 0 | 1 | 0 | 3.25 | 2 | 0.5 | 91 | 16 |
| 17 | 17 | 0 | 0 | −1 | 3.25 | 1.25 | 0.4 | 63 | 7 |
| 3 | 18 | 0 | 0 | 1 | 3.25 | 1.25 | 0.6 | 81 | 3 |
| Response Source | Degree of Freedom | Sum of Squares | Mean Square | F-Value | p-Value | |
|---|---|---|---|---|---|---|
| Manganese extraction | Regression | 10 | 7964.8 | 796.5 | 43.6 | 2.4 × 10−5 |
| Residual | 7 | 127.9 | 18.3 | - | - | |
| Lack of fit | 4 | 120.4 | 30.1 | 12.2 | 3.4 × 10−2 | |
| Pure error | 3 | 7.4 | 2.5 | - | - | |
| Totals | 17 | 8092.7 | - | - | - | |
| Cobalt extraction | Regression | 10 | 1988.3 | 198.8 | 17.6 | 4.9 × 10−4 |
| Residual | 7 | 79.2 | 11.3 | - | ||
| Lack of fit | 4 | 78.0 | 19.5 | 49.2 | 4.6 × 10−3 | |
| Pure error | 3 | 1.2 | 0.4 | - | - | |
| Totals | 17 | 2067.5 | - | - | - | |
| Solution | Concentration (g/L) | |||
|---|---|---|---|---|
| Mn | Co | Ni | Li | |
| Feed solution | 7.4 | 18.7 | 7.2 | 1.1 |
| Aqueous phase (after extraction) | 2.1 | 18.0 | 7.0 | 1.0 |
| Scrubbing solution 1 (aqueous phase) | 0.8 | 3.0 | 0.3 | 0.1 |
| Scrubbing solution 2 (aqueous phase) | 2.1 | 1.9 | <0.1 | <0.1 |
| Organic phase | 4.7 | 0.1 | 0.1 | <0.1 |
| Random Order | Std Order | Coded Variables | Real Variables | Response | |||||
|---|---|---|---|---|---|---|---|---|---|
| x1 | x2 | x3 | [H2SO4] | O:A | Time | Mn (g/L) | Co (g/L) | ||
| 9 | 1 | −1 | −1 | −1 | 0.05 | 1 | 2 | 4 | 0.06 |
| 14 | 2 | 1 | −1 | −1 | 2 | 1 | 2 | 4 | 0.05 |
| 4 | 3 | −1 | 1 | −1 | 0.05 | 8 | 2 | 11 | 0.31 |
| 2 | 4 | 1 | 1 | −1 | 2 | 8 | 2 | 19 | 0.26 |
| 15 | 5 | −1 | −1 | 1 | 0.05 | 1 | 25 | 5 | 0.08 |
| 11 | 6 | 1 | −1 | 1 | 2 | 1 | 25 | 5 | 0.07 |
| 3 | 7 | −1 | 1 | 1 | 0.05 | 8 | 25 | 10 | 0.41 |
| 8 | 8 | 1 | 1 | 1 | 2 | 8 | 25 | 28 | 0.42 |
| 16 | 9 | 0 | 0 | 0 | 1.025 | 4.5 | 13.5 | 17 | 0.26 |
| 5 | 10 | 0 | 0 | 0 | 1.025 | 4.5 | 13.5 | 16 | 0.24 |
| 7 | 11 | 0 | 0 | 0 | 1.025 | 4.5 | 13.5 | 16 | 0.24 |
| 1 | 12 | 0 | 0 | 0 | 1.025 | 4.5 | 13.5 | 17 | 0.27 |
| 18 | 13 | −1 | 0 | 0 | 0.05 | 4.5 | 13.5 | 9 | 0.15 |
| 12 | 14 | 1 | 0 | 0 | 2 | 4.5 | 13.5 | 17 | 0.26 |
| 10 | 15 | 0 | −1 | 0 | 1.025 | 1 | 13.5 | 5 | 0.07 |
| 6 | 16 | 0 | 1 | 0 | 1.025 | 8 | 13.5 | 23 | 0.36 |
| 13 | 17 | 0 | 0 | −1 | 1.025 | 4.5 | 2 | 14 | 0.21 |
| 17 | 18 | 0 | 0 | 1 | 1.025 | 4.5 | 25 | 22 | 0.34 |
| Response Source | Degree of Freedom | Sum of Squares | Mean Square | F-Value | p-Value | |
|---|---|---|---|---|---|---|
| Concentration of manganese | Regression | 10 | 880.2 | 88.0 | 20.4 | 3.0 × 10−4 |
| Residual | 7 | 30.2 | 4.3 | - | - | |
| Lack of fit | 4 | 28.7 | 7.2 | 13.9 | 2.8 × 10−2 | |
| Pure error | 3 | 1.5 | 0.5 | - | - | |
| Totals | 17 | 910.4 | - | - | - | |
| Concentration of cobalt | Regression | 10 | 0.2 | 2.4 × 10−2 | 21.4 | 2.6 × 10−4 |
| Residual | 7 | 7.93 × 10−3 | 1.1 × 10−3 | - | - | |
| Lack of fit | 4 | 7.33 × 10−3 | 1.8 × 10−3 | 9.0 | 5.1 × 10−2 | |
| Pure error | 3 | 6.08 × 10−4 | 2.0 × 10−4 | - | - | |
| Totals | 17 | 0.2 | - | - | - | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Vieceli, N.; Reinhardt, N.; Ekberg, C.; Petranikova, M. Optimization of Manganese Recovery from a Solution Based on Lithium-Ion Batteries by Solvent Extraction with D2EHPA. Metals 2021, 11, 54. https://doi.org/10.3390/met11010054
Vieceli N, Reinhardt N, Ekberg C, Petranikova M. Optimization of Manganese Recovery from a Solution Based on Lithium-Ion Batteries by Solvent Extraction with D2EHPA. Metals. 2021; 11(1):54. https://doi.org/10.3390/met11010054
Chicago/Turabian StyleVieceli, Nathália, Niclas Reinhardt, Christian Ekberg, and Martina Petranikova. 2021. "Optimization of Manganese Recovery from a Solution Based on Lithium-Ion Batteries by Solvent Extraction with D2EHPA" Metals 11, no. 1: 54. https://doi.org/10.3390/met11010054
APA StyleVieceli, N., Reinhardt, N., Ekberg, C., & Petranikova, M. (2021). Optimization of Manganese Recovery from a Solution Based on Lithium-Ion Batteries by Solvent Extraction with D2EHPA. Metals, 11(1), 54. https://doi.org/10.3390/met11010054





