Recrystallization Behavior of a Pure Cu Connection Interface with Ultrasonic Welding
Abstract
:1. Introduction
2. Experimental and Finite Element Model
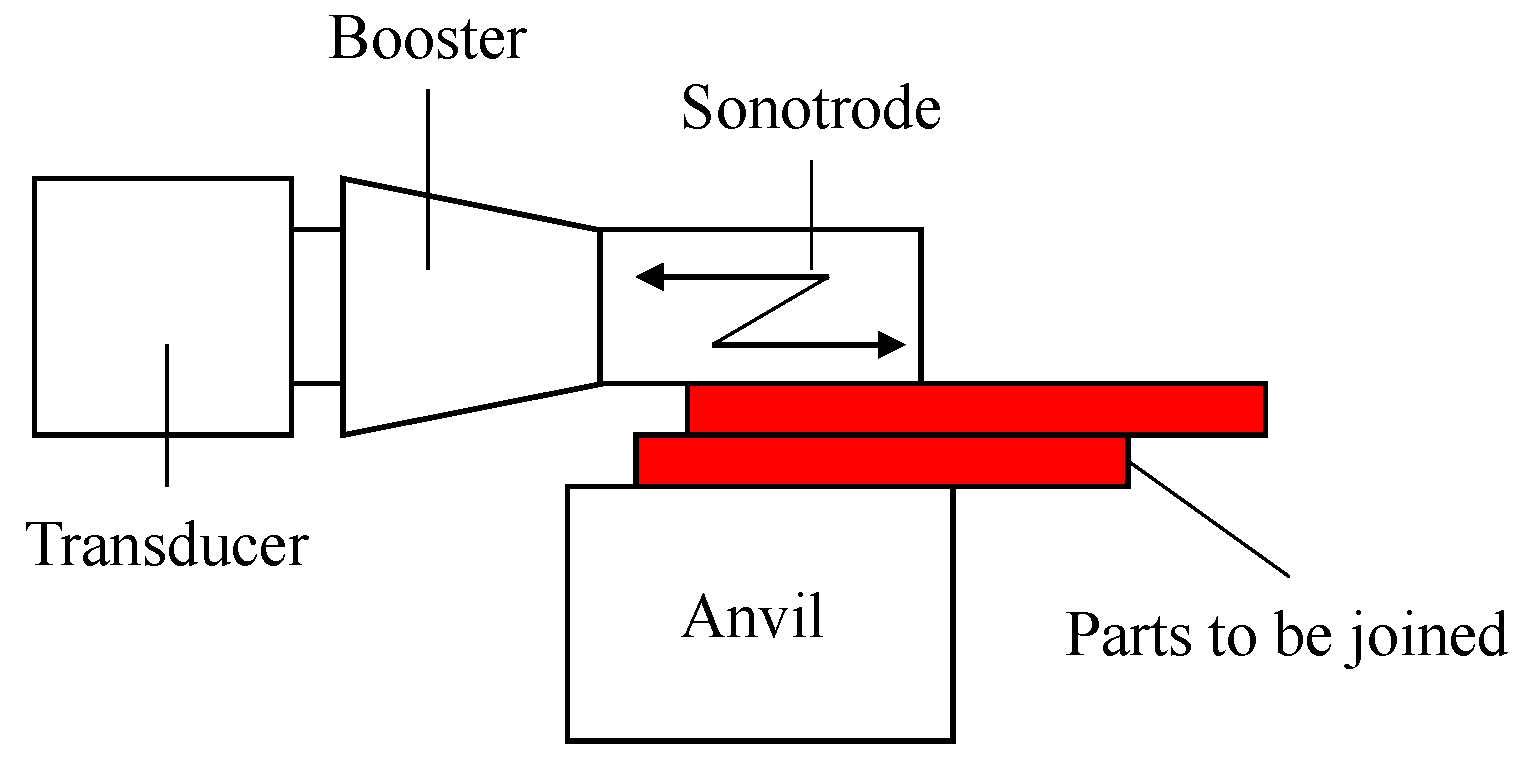
2.1. Experimental Materials and Methods
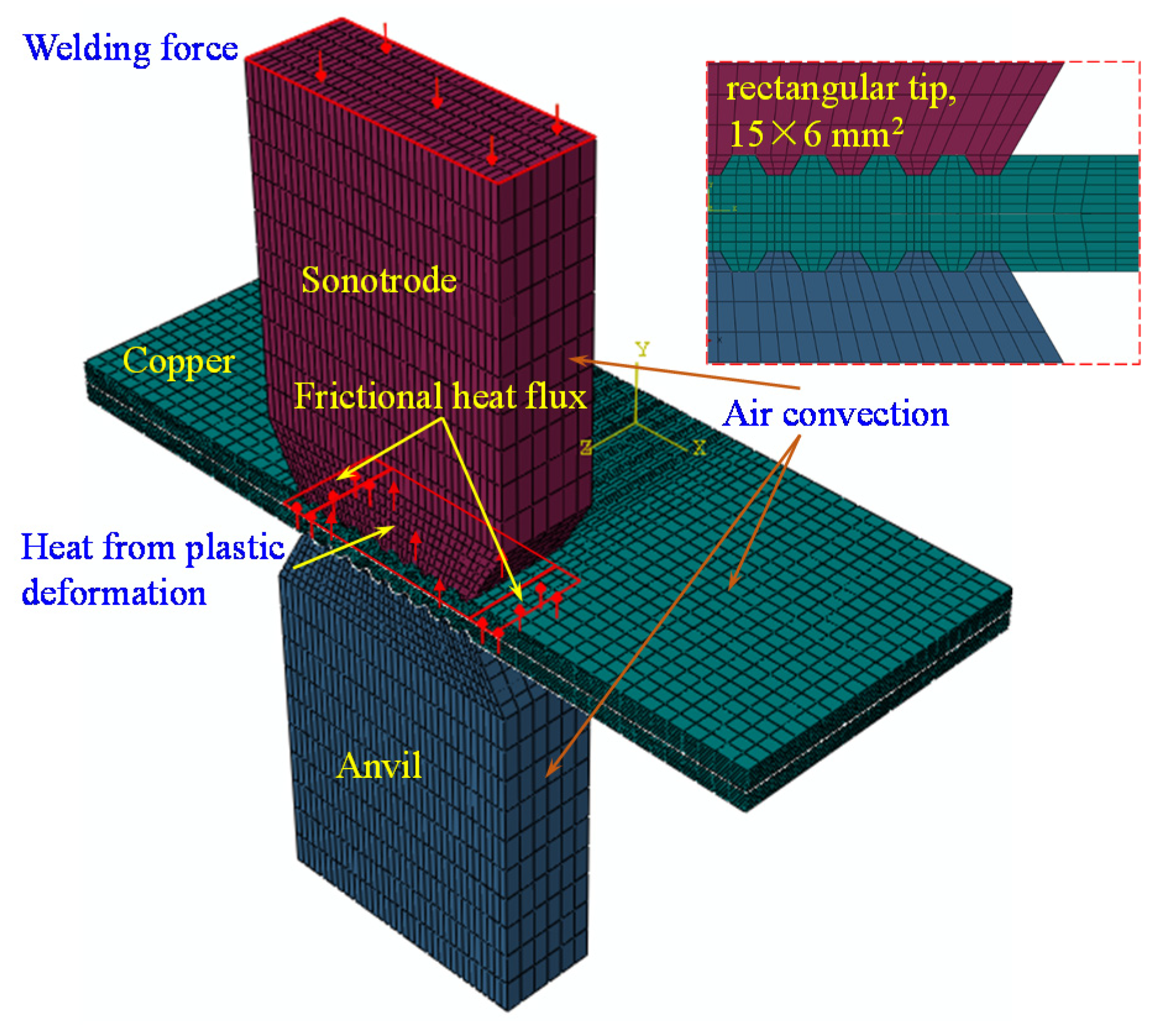
2.2. Finite Element Model
3. Results and Discussion
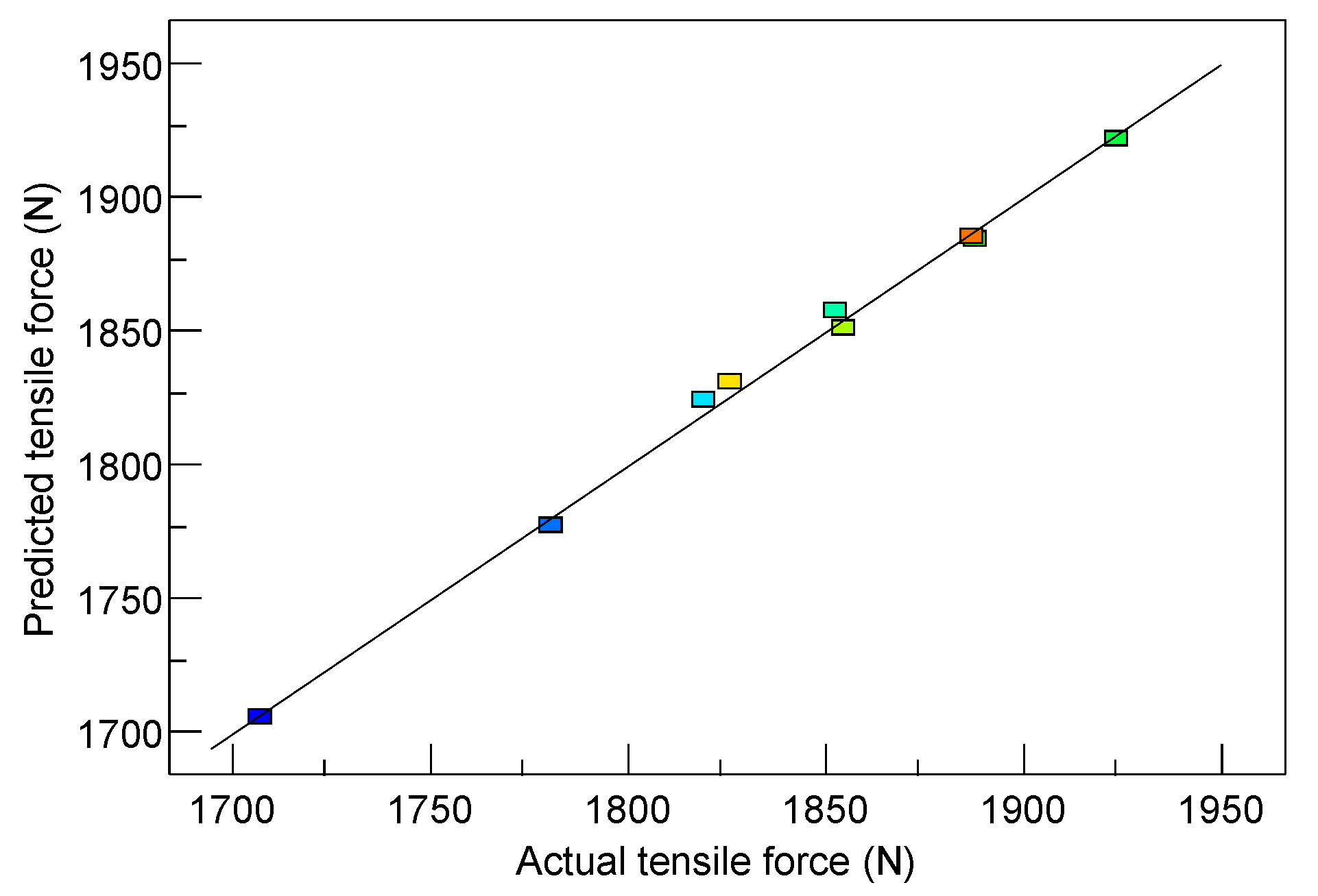
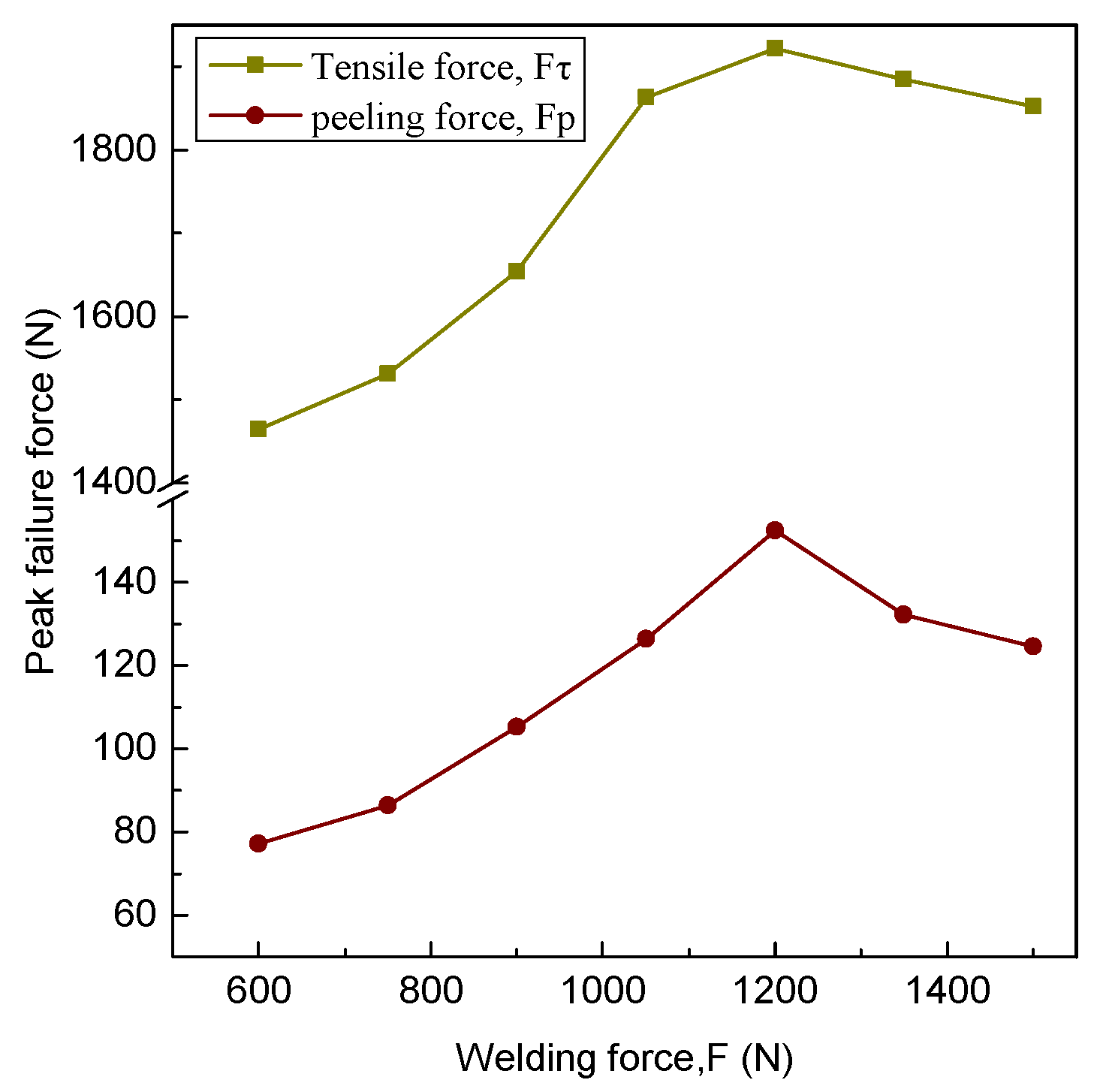
3.1. Effect of the Process Parameters on the Response Value
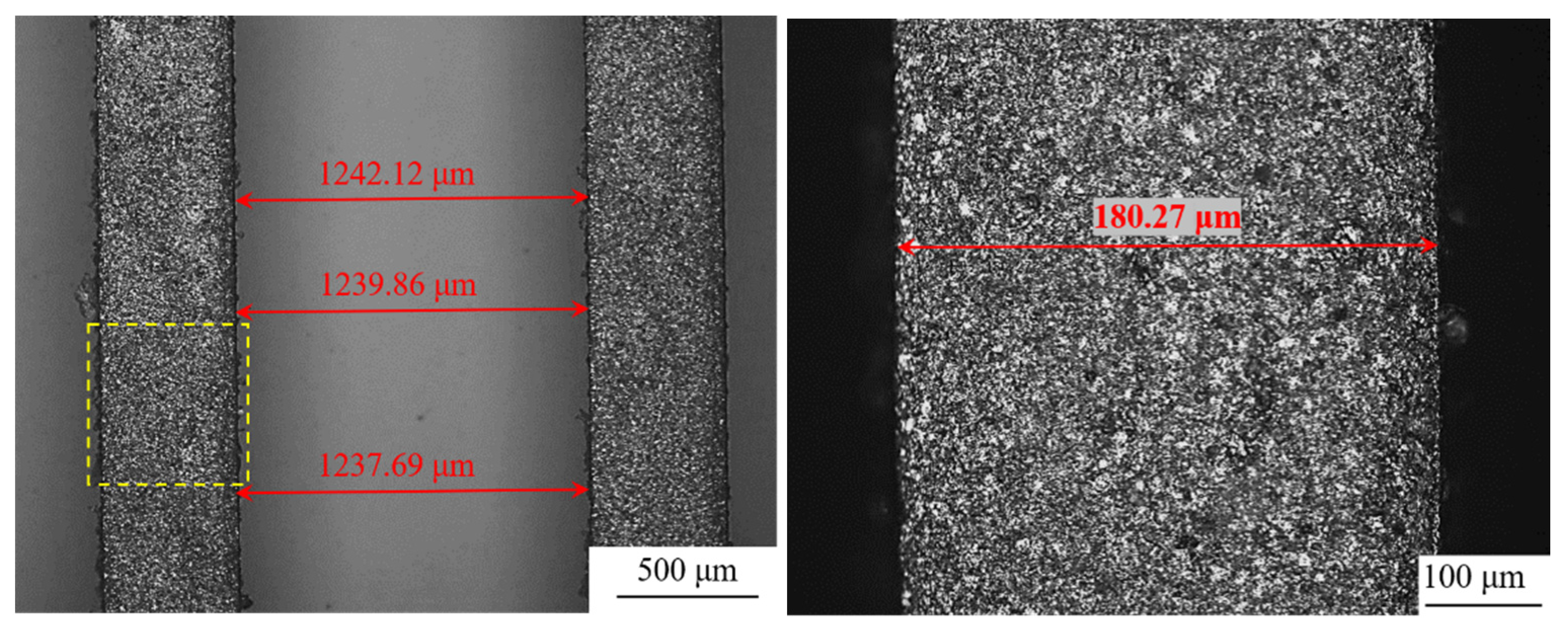
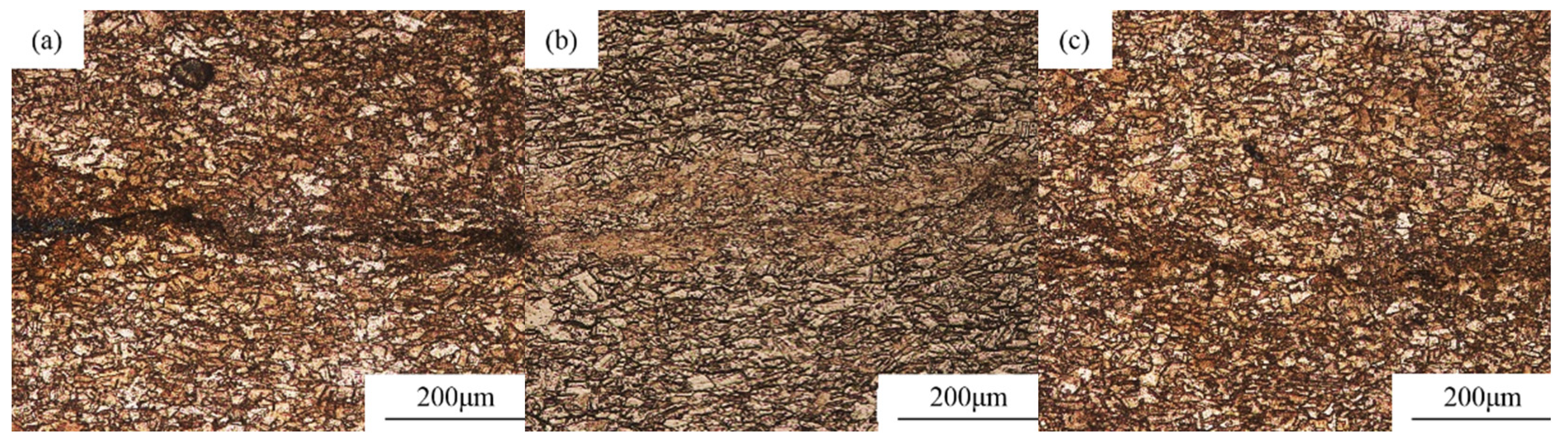
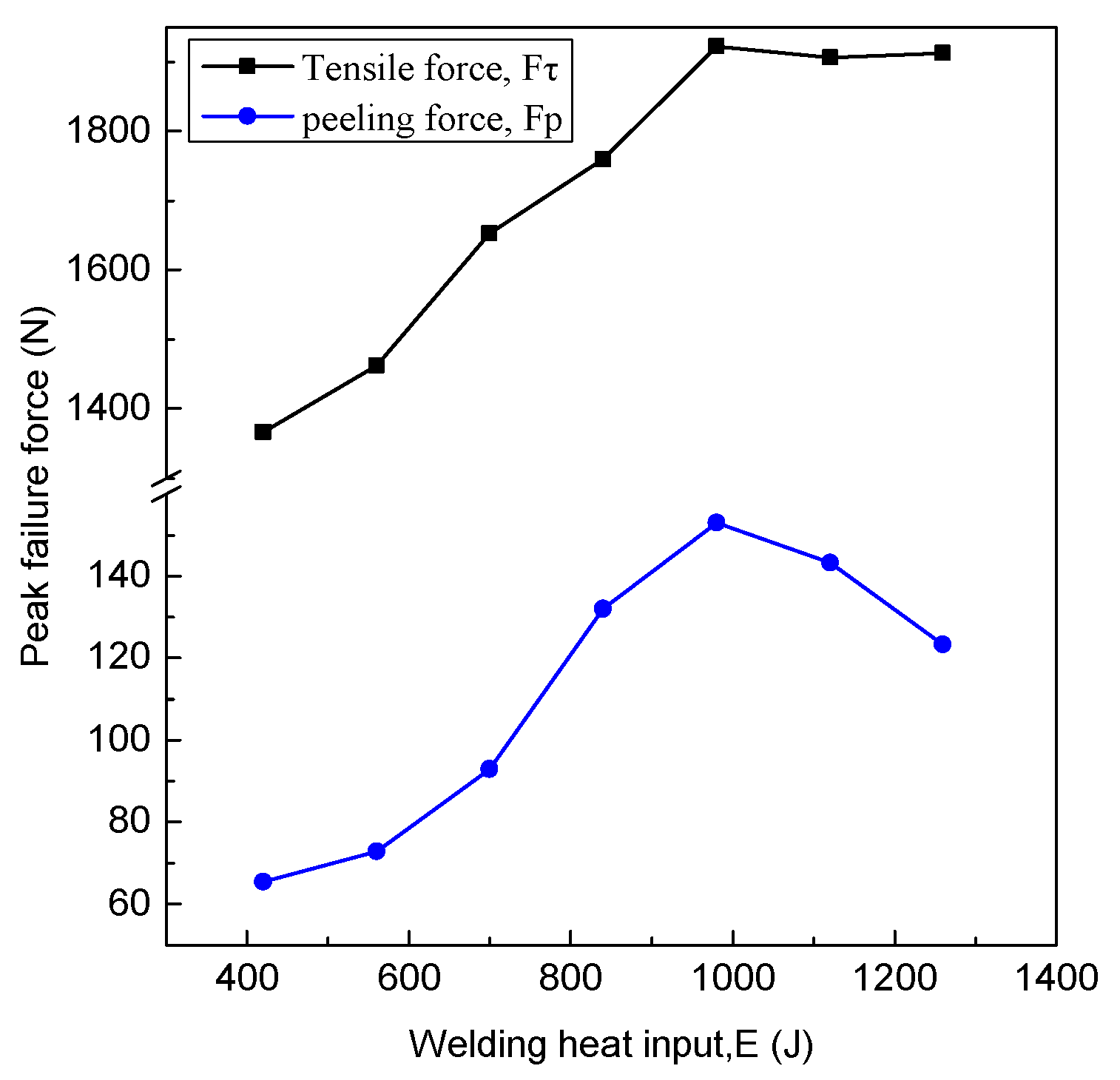
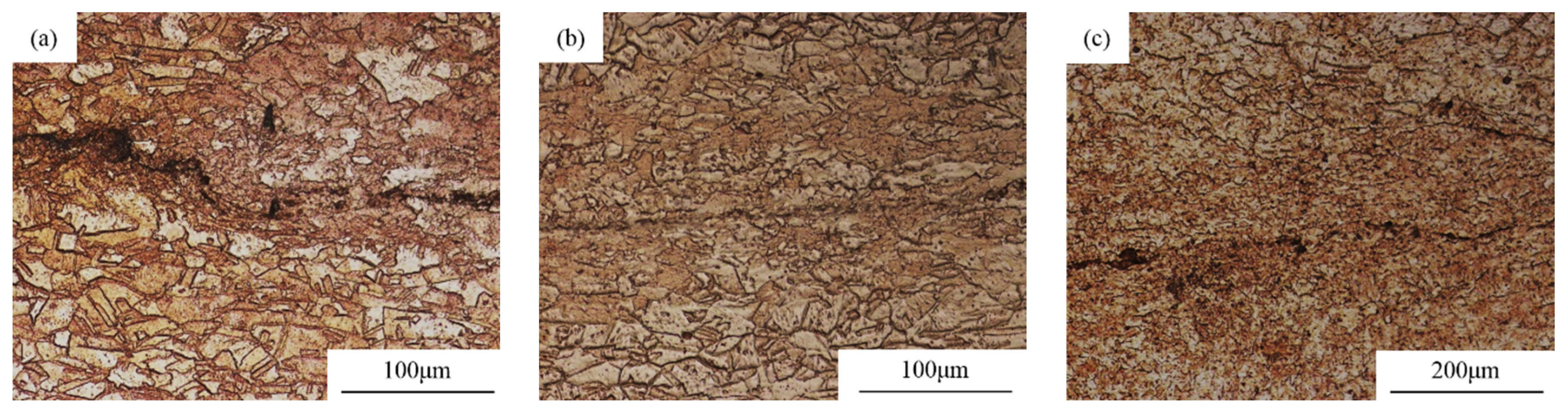
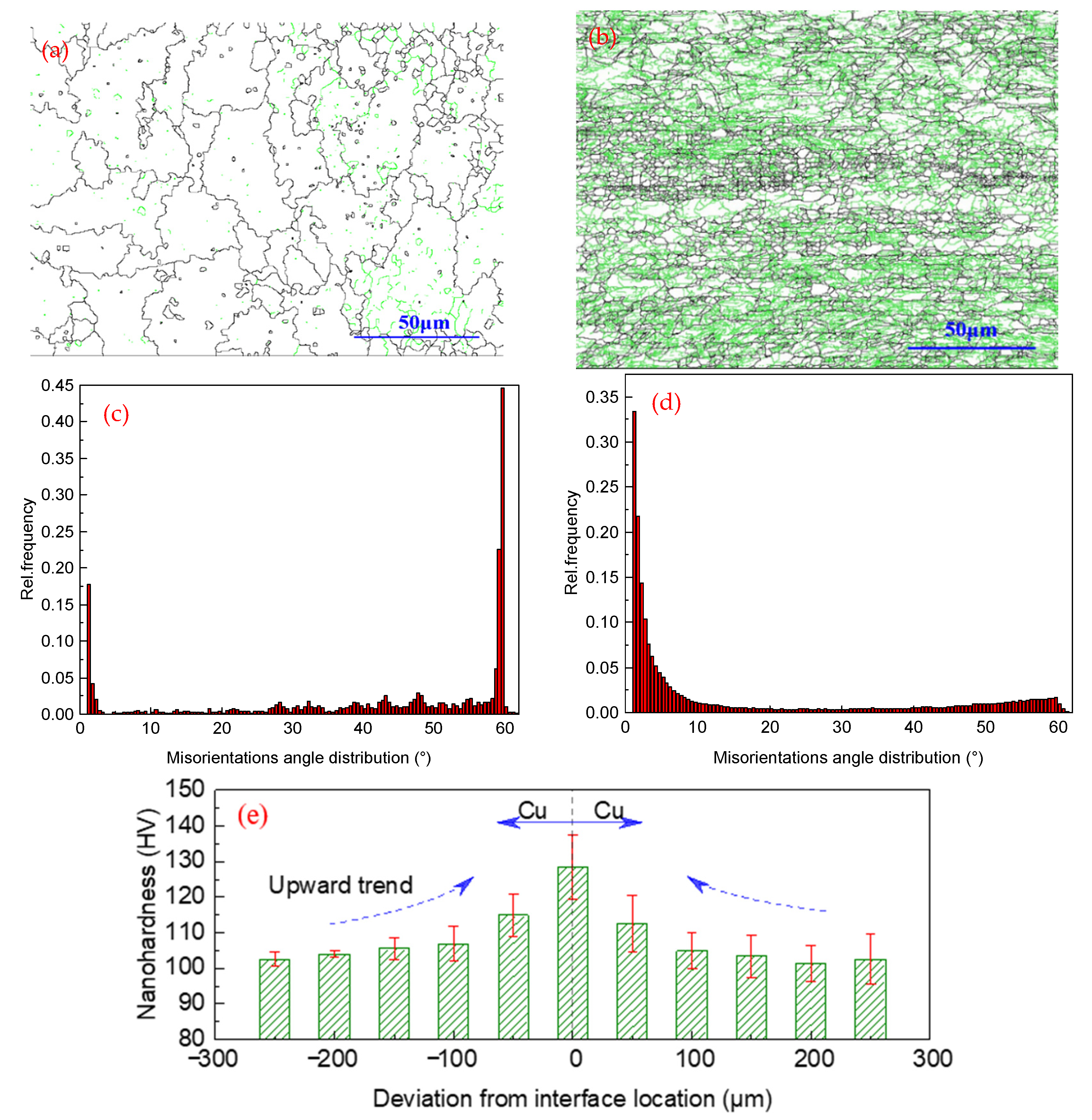
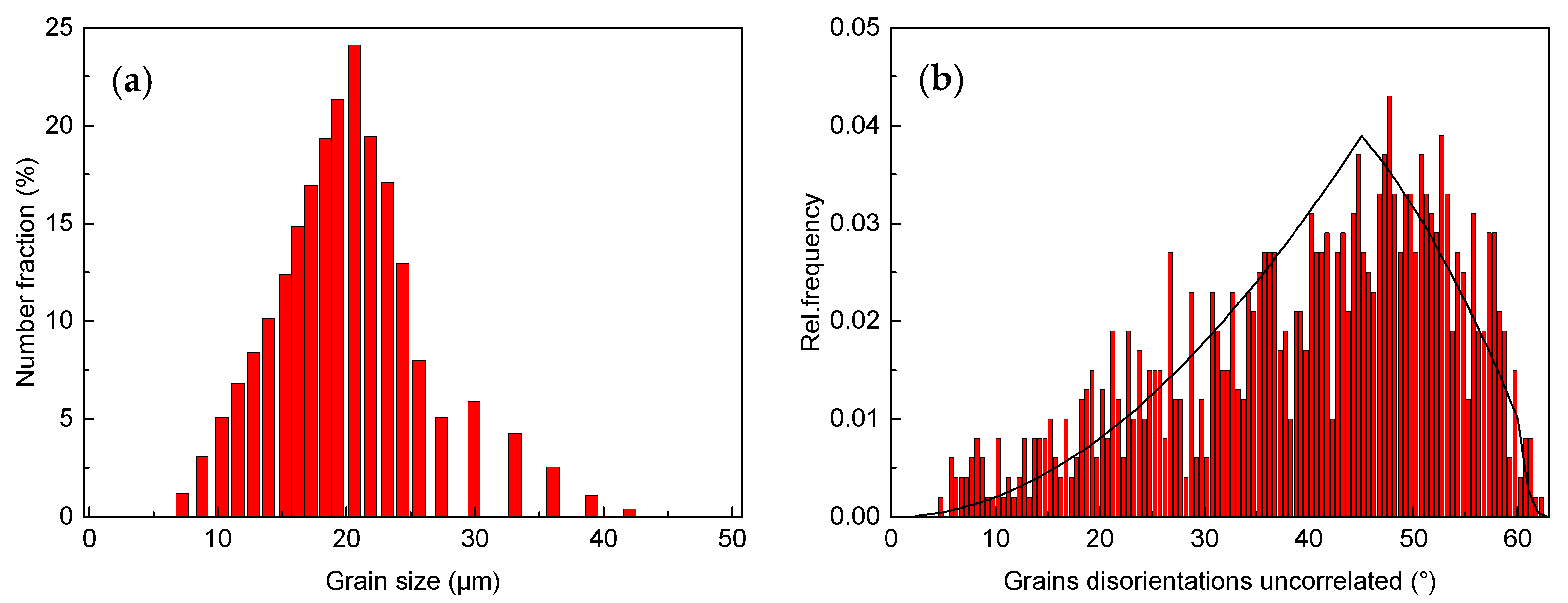
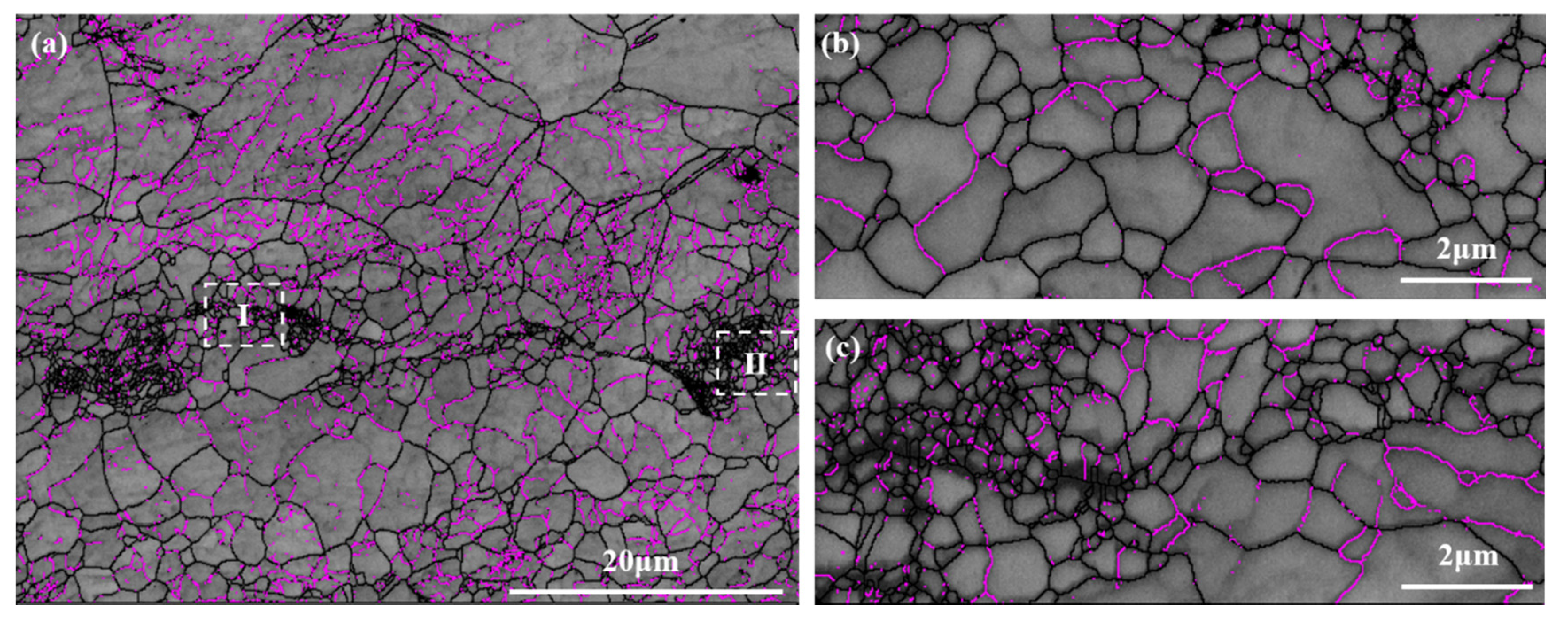
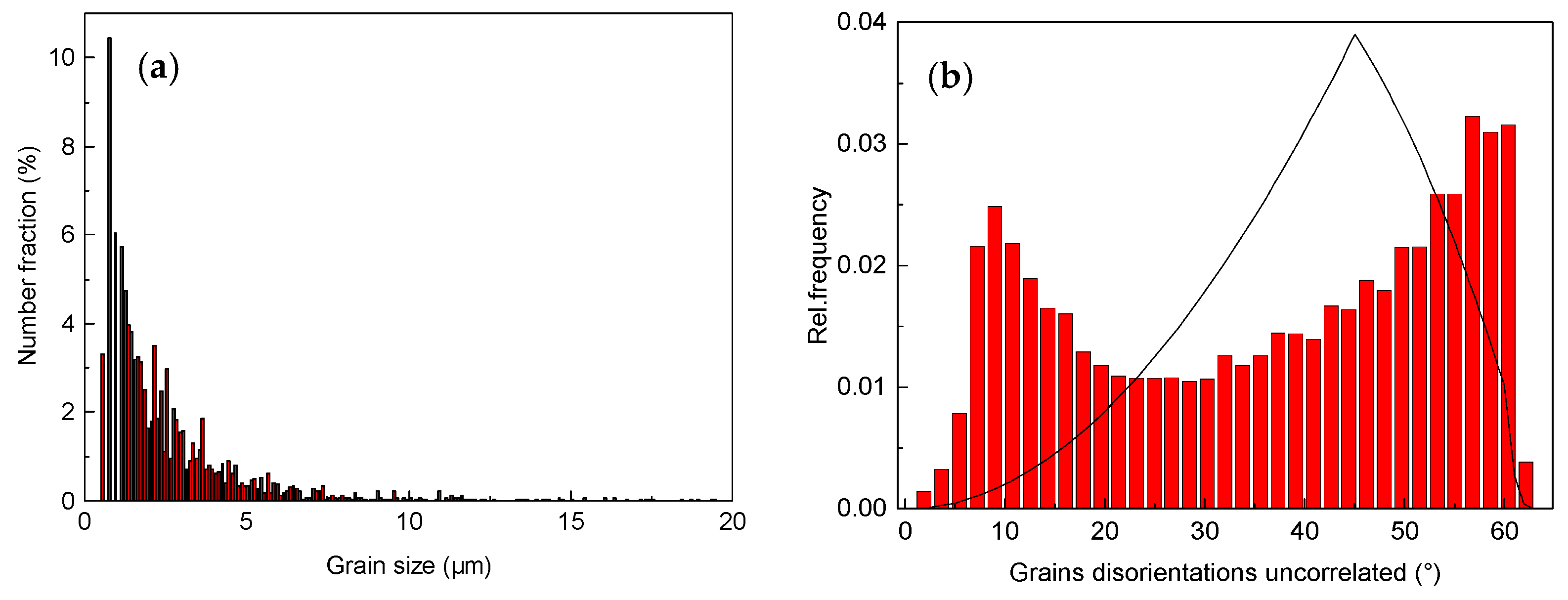
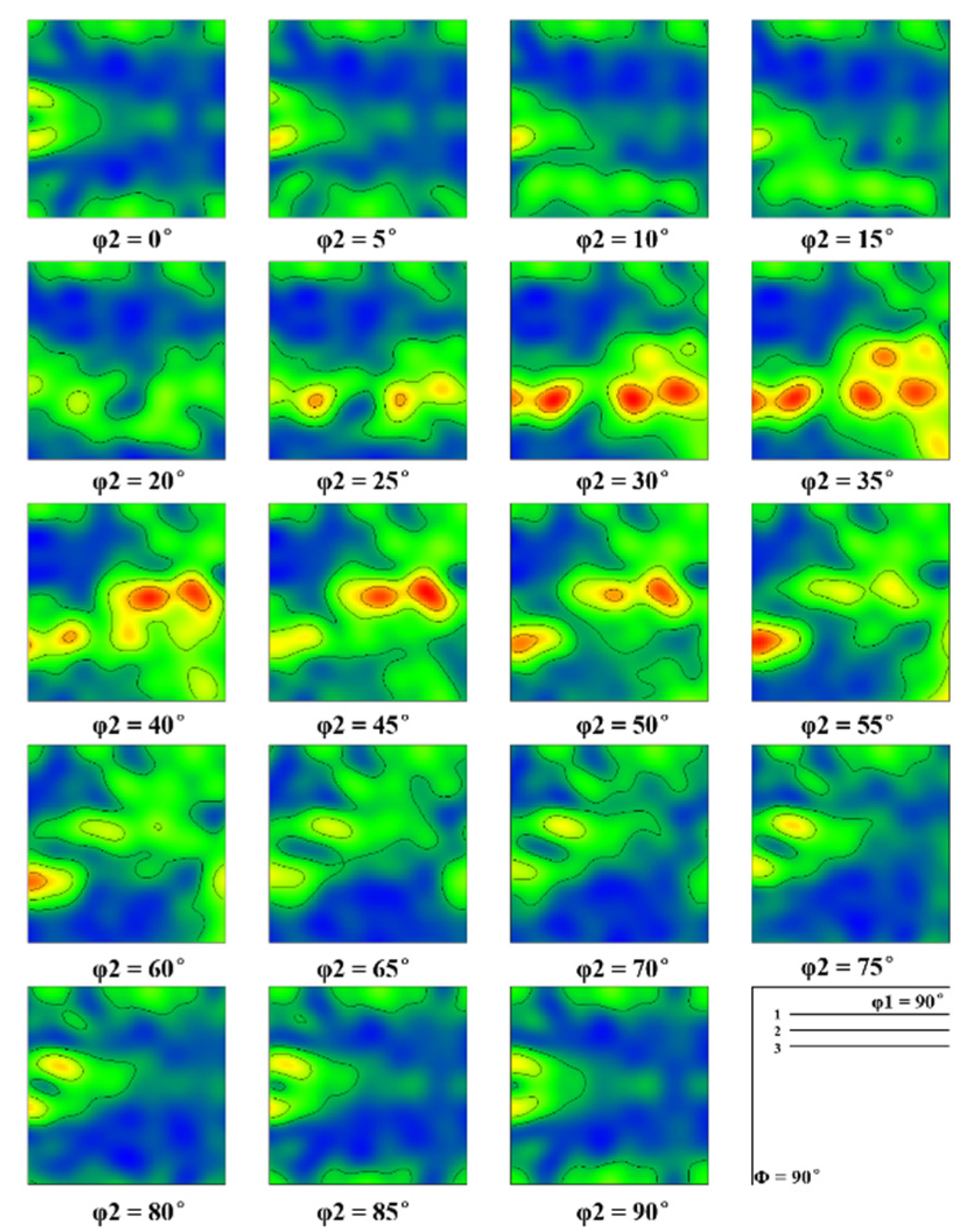
3.2. Analysis of Interface Recrystallization Behavior
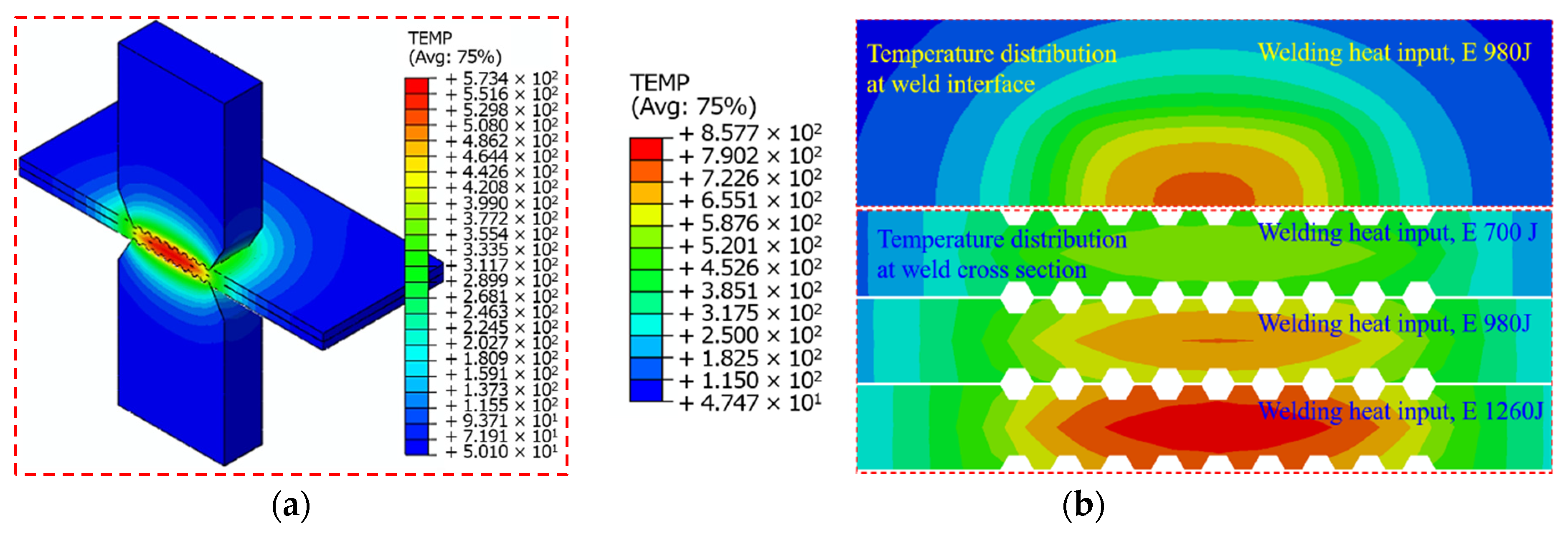
3.3. Numerical Simulation Results and Analysis
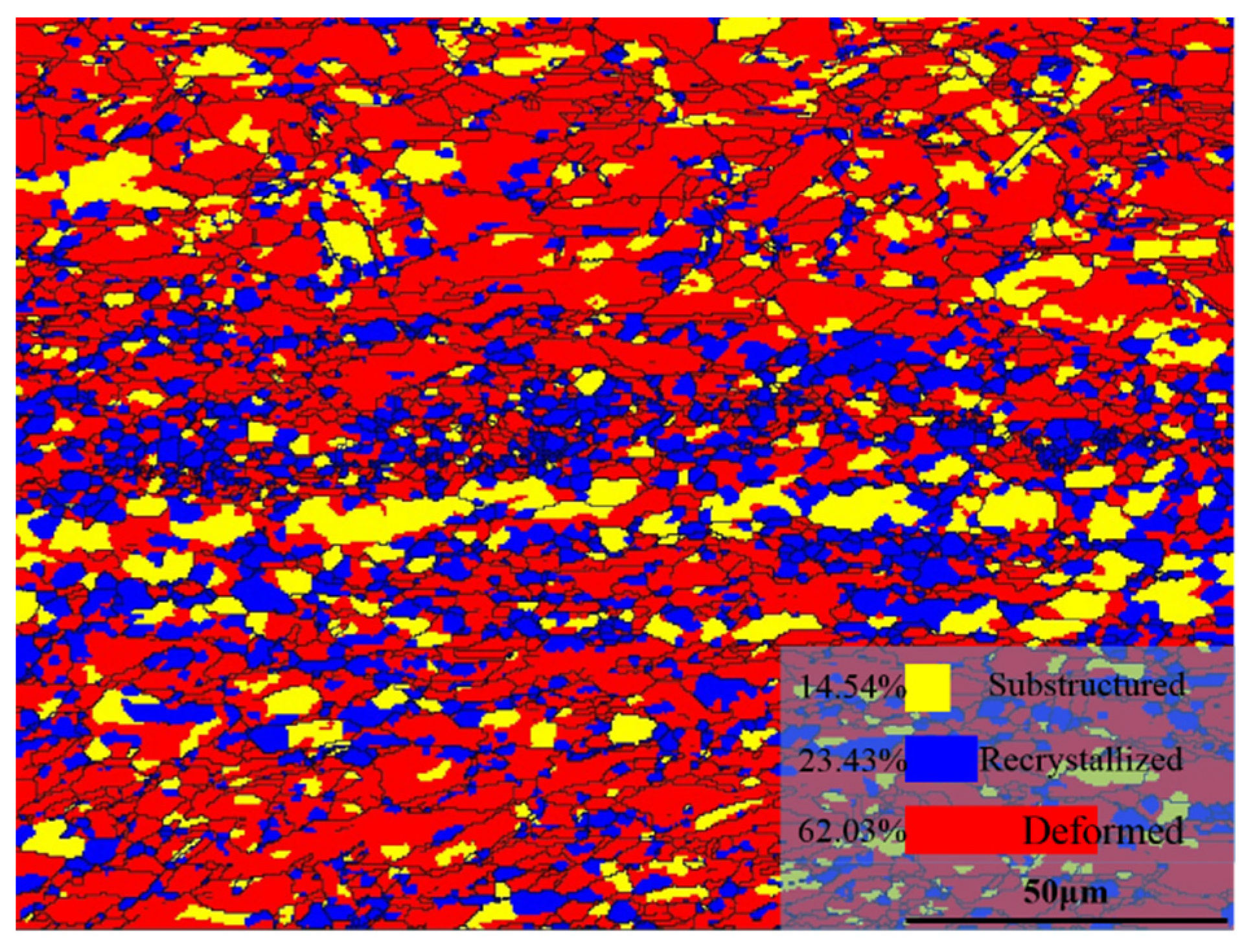
3.4. Analysis of DRV and DRX
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Shan, W.; Yang, J.; Shi, S.; Yao, Q.; Zuo, Y.; Lin, Z.; Chen, S.; Zhang, X.; Duan, W.; Cao, A.; et al. Development of Superconducting Spectroscopic Array Receiver: A Multibeam 2SB SIS Receiver for Millimeter-Wave Radio Astronomy. IEEE Trans. Terahertz Sci. Technol. 2012, 2, 593–604. [Google Scholar] [CrossRef]
- Groppi, C.; Kawamura, J.H. Coherent Detector Arrays for Terahertz Astrophysics Applications. IEEE Trans. Terahertz Sci. Technol. 2011, 1, 85–96. [Google Scholar] [CrossRef]
- Wang, Y.; Yang, Q.; Liu, X.; Liu, Y.; Liu, B.; Misra, R.D.K.; Xu, H.; Bai, P. Microstructure and mechanical properties of amorphous strip/aluminum laminated composites fabricated by ultrasonic additive consolidation. Mater. Sci. Eng. A 2019, 749, 74–78. [Google Scholar] [CrossRef]
- Friel, R.J.; Harris, R.A. Ultrasonic Additive Manufacturing–A Hybrid Production Process for Novel Functional Products. Procedia CIRP 2013, 6, 35–40. [Google Scholar] [CrossRef] [Green Version]
- Kelly, G.S.; Just, M.S.; Advani, S.; Gillespie, J.W. Energy and bond strength development during ultrasonic consolidation. J. Mater. Process. Technol. 2014, 214, 1665–1672. [Google Scholar] [CrossRef]
- Kelly, G.S.; Advani, S.; Gillespie, J.W.; Bogetti, T.A. A model to characterize acoustic softening during ultrasonic consolidation. J. Mater. Process. Technol. 2013, 213, 1835–1845. [Google Scholar] [CrossRef]
- Kelly, G.S.; Advani, S.G.; Gillespie, J.W. A model to describe stick–slip transition time during ultrasonic consolidation. Int. J. Adv. Manuf. Technol. 2015, 79, 1931–1937. [Google Scholar] [CrossRef]
- Zhang, L.; Tong, H.; Li, Y. Precision machining of micro tool electrodes in micro EDM for drilling array micro holes. Precis. Eng. 2015, 39, 100–106. [Google Scholar] [CrossRef]
- Dehoff, R.; Babu, S. Characterization of interfacial microstructures in 3003 aluminum alloy blocks fabricated by ultrasonic additive manufacturing. Acta Mater. 2010, 58, 4305–4315. [Google Scholar] [CrossRef]
- Sridharan, N.; Norfolk, M.; Babu, S.S. Characterization of Steel-Ta Dissimilar Metal Builds Made Using Very High Power Ultrasonic Additive Manufacturing (VHP-UAM). Met. Mater. Trans. A 2016, 47, 2517–2528. [Google Scholar] [CrossRef]
- Gao, S.; Wu, C.; Padhy, G.; Shi, L. Evaluation of local strain distribution in ultrasonic enhanced Al 6061-T6 friction stir weld nugget by EBSD analysis. Mater. Des. 2016, 99, 135–144. [Google Scholar] [CrossRef]
- Ward, A.A.; French, M.R.; Leonard, D.N.; Cordero, Z.C. Grain growth during ultrasonic welding of nanocrystalline alloys. J. Mater. Process. Technol. 2018, 254, 373–382. [Google Scholar] [CrossRef]
- Lin, J.-Y.; Nambu, S.; Koseki, T. Evolution of Bonding Interface during Ultrasonic Welding between Ni and Steels with Various Microstructure. ISIJ Int. 2020, 60, 330–336. [Google Scholar] [CrossRef] [Green Version]
- Siddiq, A.; El Sayed, T.; Siddiq, A. A thermomechanical crystal plasticity constitutive model for ultrasonic consolidation. Comput. Mater. Sci. 2012, 51, 241–251. [Google Scholar] [CrossRef]
- Fujii, H.; Endo, H.; Sato, Y.; Kokawa, H. Interfacial microstructure evolution and weld formation during ultrasonic welding of Al alloy to Cu. Mater. Charact. 2018, 139, 233–240. [Google Scholar] [CrossRef]
- Nambu, S.; Seto, K.; Lin, J.-Y.; Koseki, T. Development of a bonding interface between steel/steel and steel/Ni by ultrasonic welding. Sci. Technol. Weld. Join. 2018, 23, 687–692. [Google Scholar] [CrossRef]
- Sriraman, M.; Babu, S.; Short, M. Bonding characteristics during very high power ultrasonic additive manufacturing of copper. Scr. Mater. 2010, 62, 560–563. [Google Scholar] [CrossRef]
- Xie, J.; Zhu, Y.; Bian, F.; Liu, C. Dynamic recovery and recrystallization mechanisms during ultrasonic spot welding of Al-Cu-Mg alloy. Mater. Charact. 2017, 132, 145–155. [Google Scholar] [CrossRef]
- Fujii, H.T.; Goto, Y.; Sato, Y.S.; Kokawa, H. Microstructure and lap shear strength of the weld interface in ultrasonic welding of Al alloy to stainless steel. Scr. Mater. 2016, 116, 135–138. [Google Scholar] [CrossRef]
- Haddadi, F.; Tsivoulas, D. Grain structure, texture and mechanical property evolution of automotive aluminium sheet during high power ultrasonic welding. Mater. Charact. 2016, 118, 340–351. [Google Scholar] [CrossRef]
- Srinivasan, V.; Balamurugan, S.; Balakarthick, B.; Darshan, S.D.; Prabhu, A.D. Experimental investigation on ultrasonic metal welding of copper sheet with copper wire using Taguchi method. Mater. Today Proc. 2020. [Google Scholar] [CrossRef]
- Elangovan, S.; Prakasan, K.; Jaiganesh, V. Optimization of ultrasonic welding parameters for copper to copper joints using design of experiments. Int. J. Adv. Manuf. Technol. 2010, 51, 163–171. [Google Scholar] [CrossRef]
- Li, H.; Cao, B.; Liu, J.; Yang, J. Modeling of high-power ultrasonic welding of Cu/Al joint. Int. J. Adv. Manuf. Technol. 2018, 97, 833–844. [Google Scholar] [CrossRef]
- Jedrasiak, P.; Shercliff, H.; Chen, Y.C.; Wang, L.; Prangnell, P.; Robson, J.D. Modeling of the Thermal Field in Dissimilar Alloy Ultrasonic Welding. J. Mater. Eng. Perform. 2014, 24, 799–807. [Google Scholar] [CrossRef] [Green Version]
- Elangovan, S.; Semeer, S.; Prakasan, K. Temperature and stress distribution in ultrasonic metal welding—An FEA-based study. J. Mater. Process. Technol. 2009, 209, 1143–1150. [Google Scholar] [CrossRef]
- Yang, J.; Zhang, J.; Qiao, J. Molecular Dynamics Simulations of Atomic Diffusion during the Al–Cu Ultrasonic Welding Process. Materials 2019, 12, 2306. [Google Scholar] [CrossRef] [Green Version]
- Shen, N.; Samanta, A.; Ding, H.; Cai, W.W. Simulating microstructure evolution of battery tabs during ultrasonic welding. J. Manuf. Process. 2016, 23, 306–314. [Google Scholar] [CrossRef] [Green Version]
- Mariani, E.; Ghassemieh, E. Microstructure evolution of 6061 O Al alloy during ultrasonic consolidation: An insight from electron backscatter diffraction. Acta Mater. 2010, 58, 2492–2503. [Google Scholar] [CrossRef]
- Zeng, X.; Xue, P.; Wu, L.; Ni, D.; Xiao, B.; Wang, K.; Ma, Z. Microstructural evolution of aluminum alloy during friction stir welding under different tool rotation rates and cooling conditions. J. Mater. Sci. Technol. 2019, 35, 972–981. [Google Scholar] [CrossRef]
- Fujii, H.T.; Sriraman, M.R.; Babu, S.S. Quantitative Evaluation of Bulk and Interface Microstructures in Al-3003 Alloy Builds Made by Very High Power Ultrasonic Additive Manufacturing. Met. Mater. Trans. A 2011, 42, 4045–4055. [Google Scholar] [CrossRef]

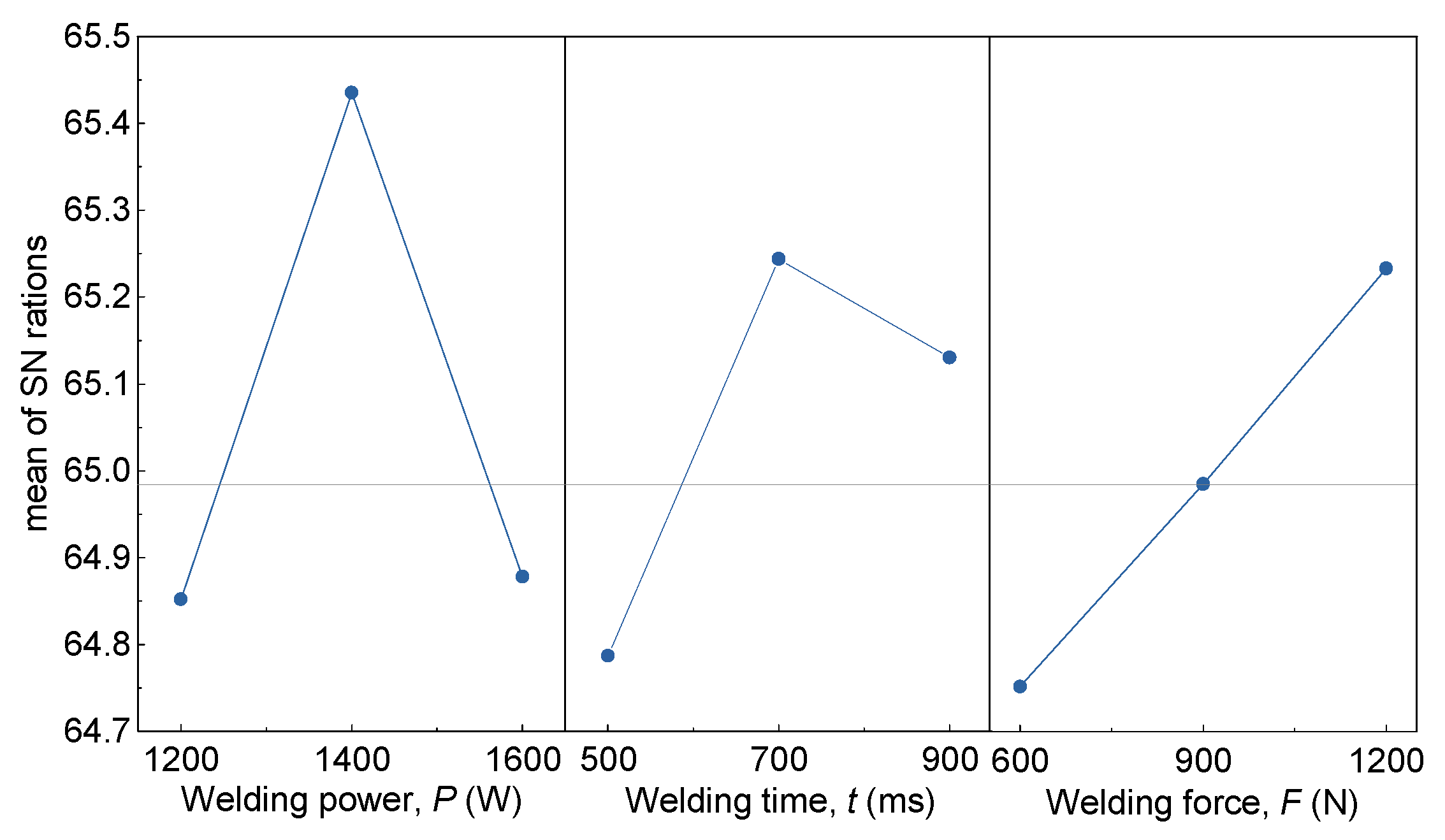


| Level Coding | Factor | ||
|---|---|---|---|
| Welding Power, P (W) | Welding Time, t (ms) | Welding Force, F (N) | |
| − | 1200 | 500 | 600 |
| 0 | 1400 | 700 | 900 |
| + | 1600 | 900 | 1200 |
| Experiment No. | Mode | P (W) | t (ms) | F (N) | Tensile Force, Fτ (N) |
|---|---|---|---|---|---|
| 1 | −−− | 1200 | 500 | 600 | 1707.0 |
| 2 | −00 | 1200 | 700 | 900 | 1780.5 |
| 3 | −++ | 1200 | 900 | 1200 | 1819.1 |
| 4 | 0−0 | 1400 | 500 | 900 | 1852.4 |
| 5 | 00+ | 1400 | 700 | 1200 | 1923.4 |
| 6 | 0+− | 1400 | 900 | 600 | 1887.7 |
| 7 | +−+ | 1600 | 500 | 1200 | 1854.4 |
| 8 | +0− | 1600 | 700 | 600 | 1825.8 |
| 9 | ++0 | 1600 | 900 | 900 | 1886.8 |
| Temperature (°C) | Elastic Modulus (GPa) | Density (kg/m3) | Poisson’s Ratio | Linear Expansion Coefficient (10−5/°C) | Thermal Conductivity (W/m·K) | Specific Heat Capacity (J/kg·K) |
|---|---|---|---|---|---|---|
| 20 | 212 | 7800 | 0.29 | 1.48 | 34 | 460 |
| 300 | 192 | 7800 | 0.29 | 1.51 | 36.2 | 513 |
| 500 | 156 | 7800 | 0.29 | 1.58 | 38.1 | 532 |
| 800 | 139 | 7800 | 0.29 | 1.65 | 39.5 | 560 |
| 1200 | 107 | 7800 | 0.29 | 1.72 | 40.7 | 584 |
| 1500 | 83 | 7800 | 0.29 | 1.75 | 41.2 | 607 |
| Temperature (°C) | Elastic Modulus (GPa) | Density (kg/m3) | Yield Strength (MPa) | Poisson’s Ratio | Linear Expansion Coefficient (10−5/°C) | Thermal Conductivity (W/m·K) | Specific Heat Capacity (J/kg·K) |
|---|---|---|---|---|---|---|---|
| 20 | 109 | 8969 | 804 | 0.35 | 1.64 | 390 | 385.2 |
| 100 | 105 | 8969 | 620 | 0.35 | 1.68 | 382 | 406 |
| 200 | 102 | 8969 | 638 | 0.35 | 1.72 | 371 | 406 |
| 300 | 98 | 8969 | 552 | 0.35 | 1.75 | 352 | 410 |
| 400 | 92 | 8969 | 455 | 0.35 | 1.79 | 348 | 412 |
| 500 | 90 | 8969 | 377 | 0.35 | 1.79 | 343 | 416 |
| 600 | 87 | 8969 | 303 | 0.35 | 1.81 | 339 | 418 |
| df | Sum of Squares | Mean Square | F Value | ∆Prob > ∆F | |
|---|---|---|---|---|---|
| Intercept | 6 | 33,473.28 | 5578.88 | 94.27 | 0.0105 |
| P | 2 | 22,722.00 | 11,361.00 | 191.97 | 0.0052 |
| t | 2 | 5538.23 | 2769.11 | 46.79 | 0.0209 |
| F | 2 | 5213.05 | 2606.52 | 44.04 | 0.0222 |
| Residual | 2 | 118.36 | 59.18 | ||
| Cor Total | 8 | 33,591.64 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Su, Z.; Zhu, Z.; Zhang, Y.; Zhang, H.; Xiao, Q. Recrystallization Behavior of a Pure Cu Connection Interface with Ultrasonic Welding. Metals 2021, 11, 61. https://doi.org/10.3390/met11010061
Su Z, Zhu Z, Zhang Y, Zhang H, Xiao Q. Recrystallization Behavior of a Pure Cu Connection Interface with Ultrasonic Welding. Metals. 2021; 11(1):61. https://doi.org/10.3390/met11010061
Chicago/Turabian StyleSu, Zhanzhan, Zhengqiang Zhu, Yifu Zhang, Hua Zhang, and Qiankun Xiao. 2021. "Recrystallization Behavior of a Pure Cu Connection Interface with Ultrasonic Welding" Metals 11, no. 1: 61. https://doi.org/10.3390/met11010061
APA StyleSu, Z., Zhu, Z., Zhang, Y., Zhang, H., & Xiao, Q. (2021). Recrystallization Behavior of a Pure Cu Connection Interface with Ultrasonic Welding. Metals, 11(1), 61. https://doi.org/10.3390/met11010061





