Three-Point Bending Fatigue Behavior of Aluminum Foam Sandwich Panels with Different Density Core Material
Abstract
:1. Introduction
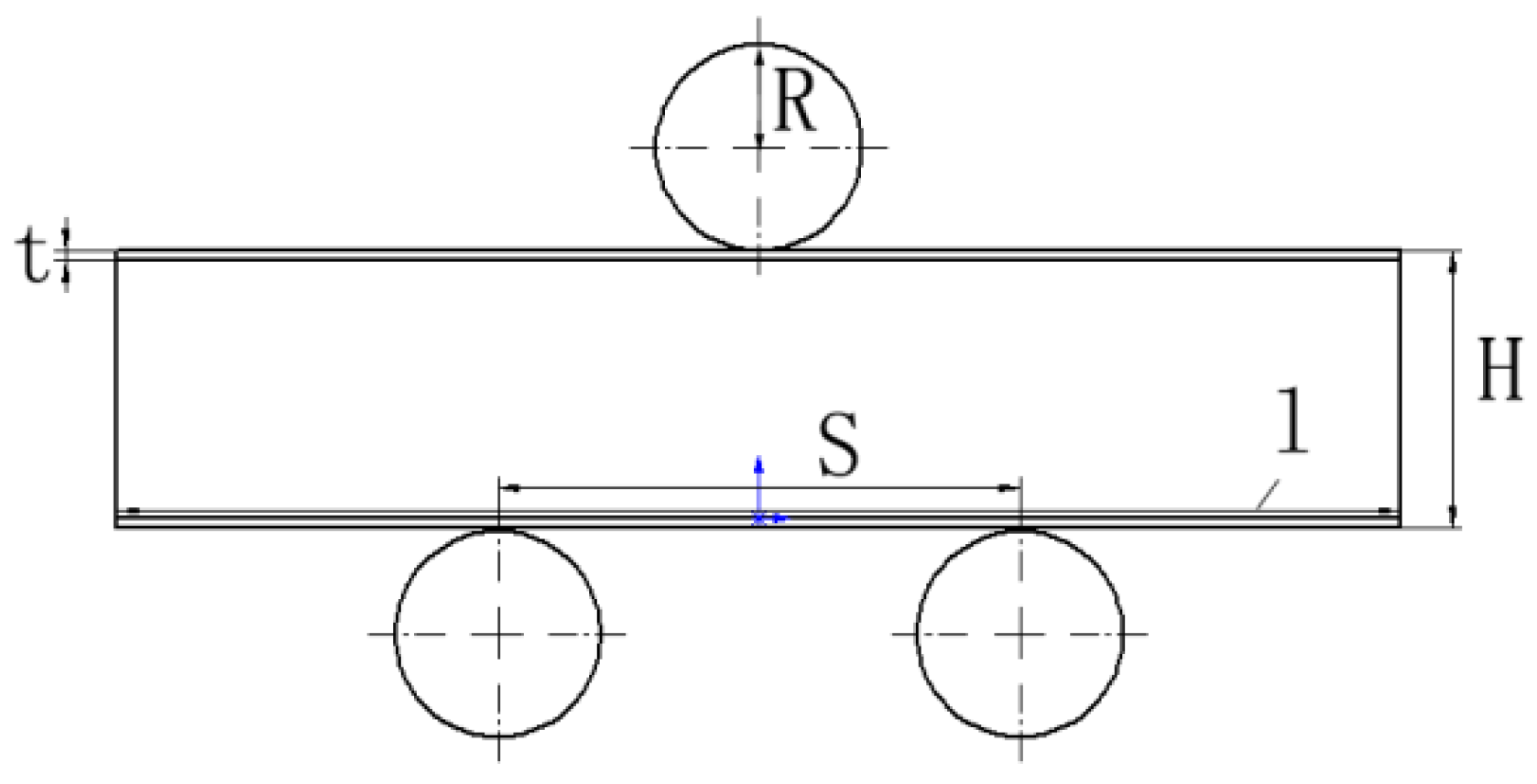
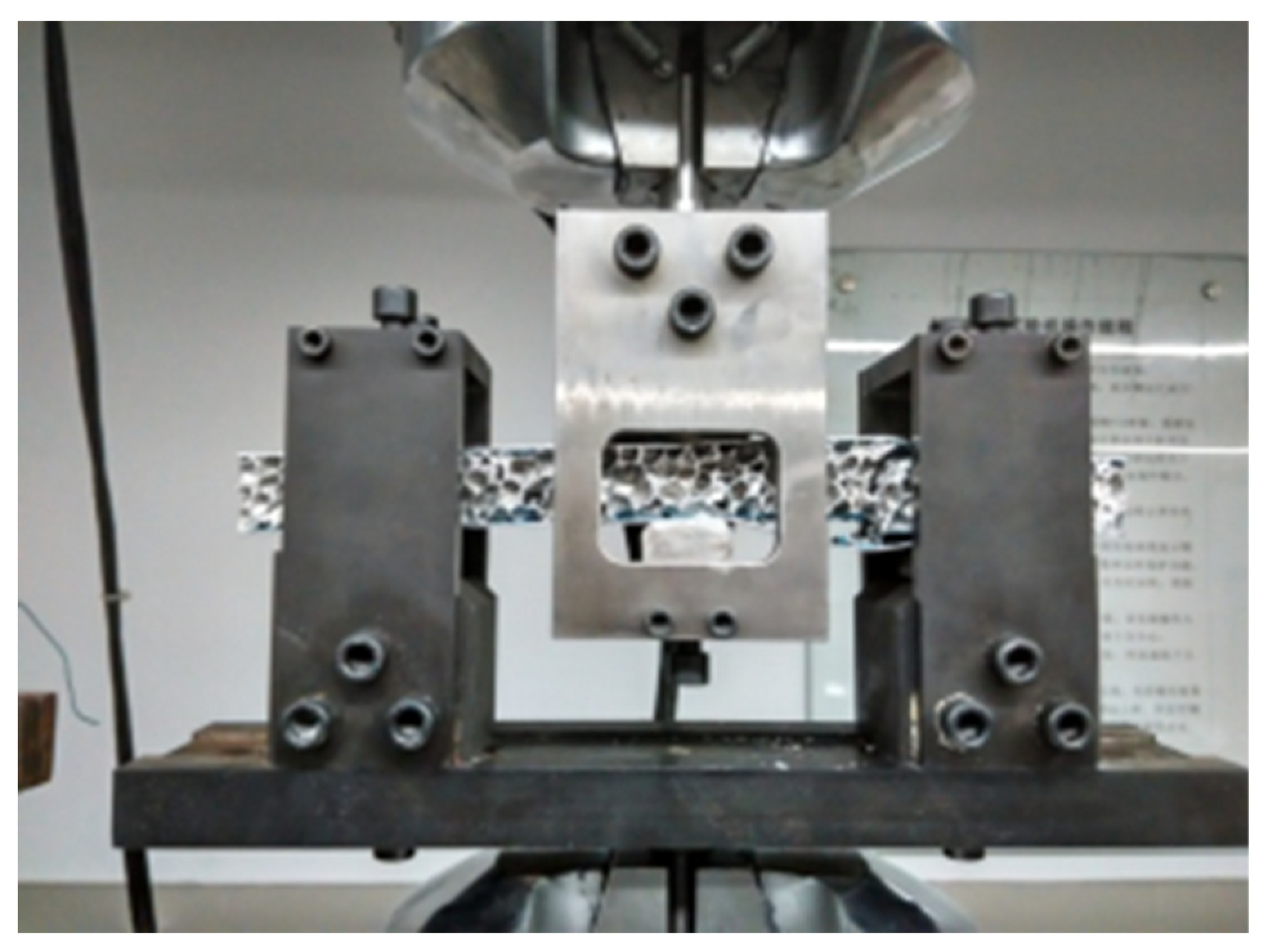
2. Materials and Methods
3. Results
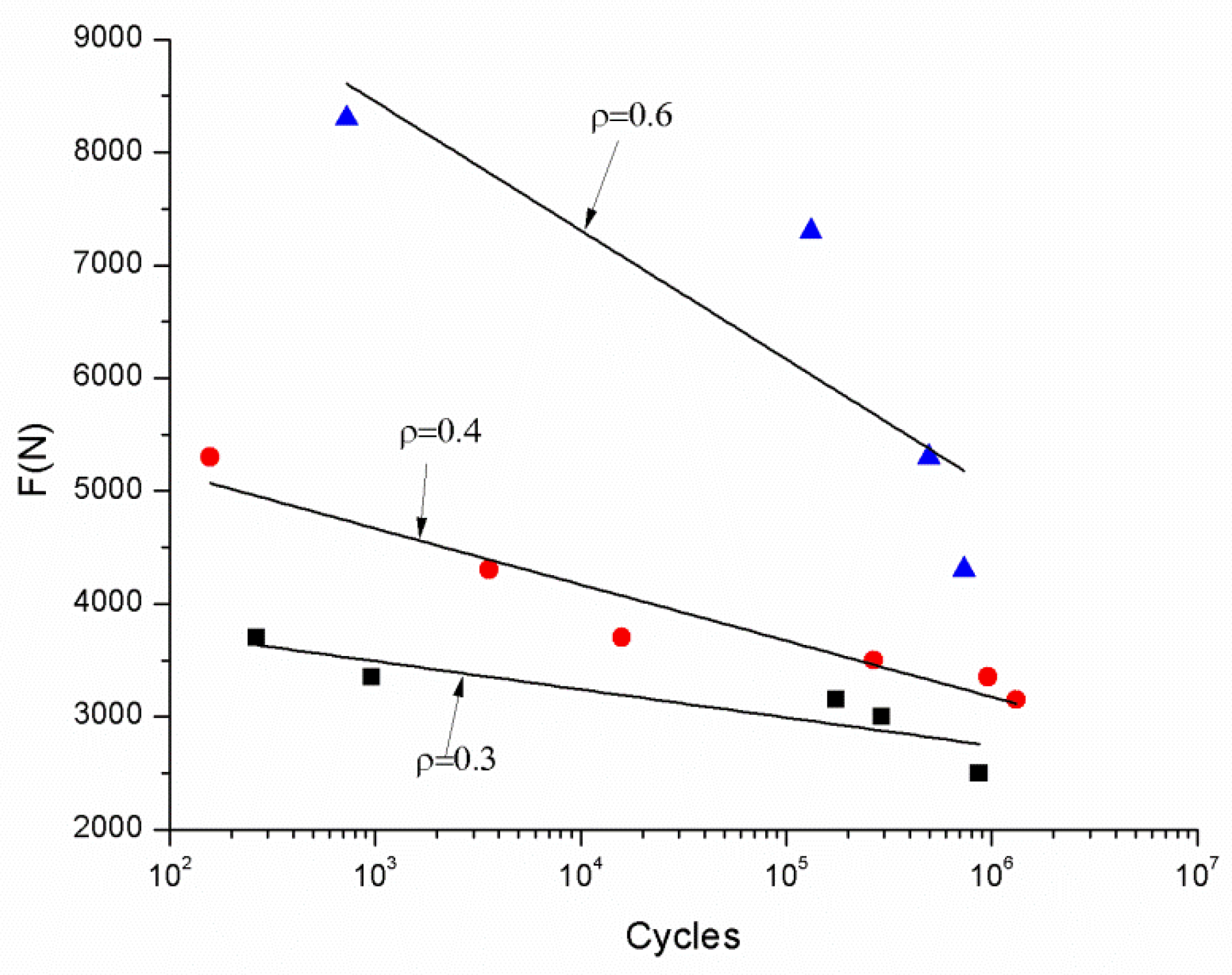
3.1. Force Cycle Curves
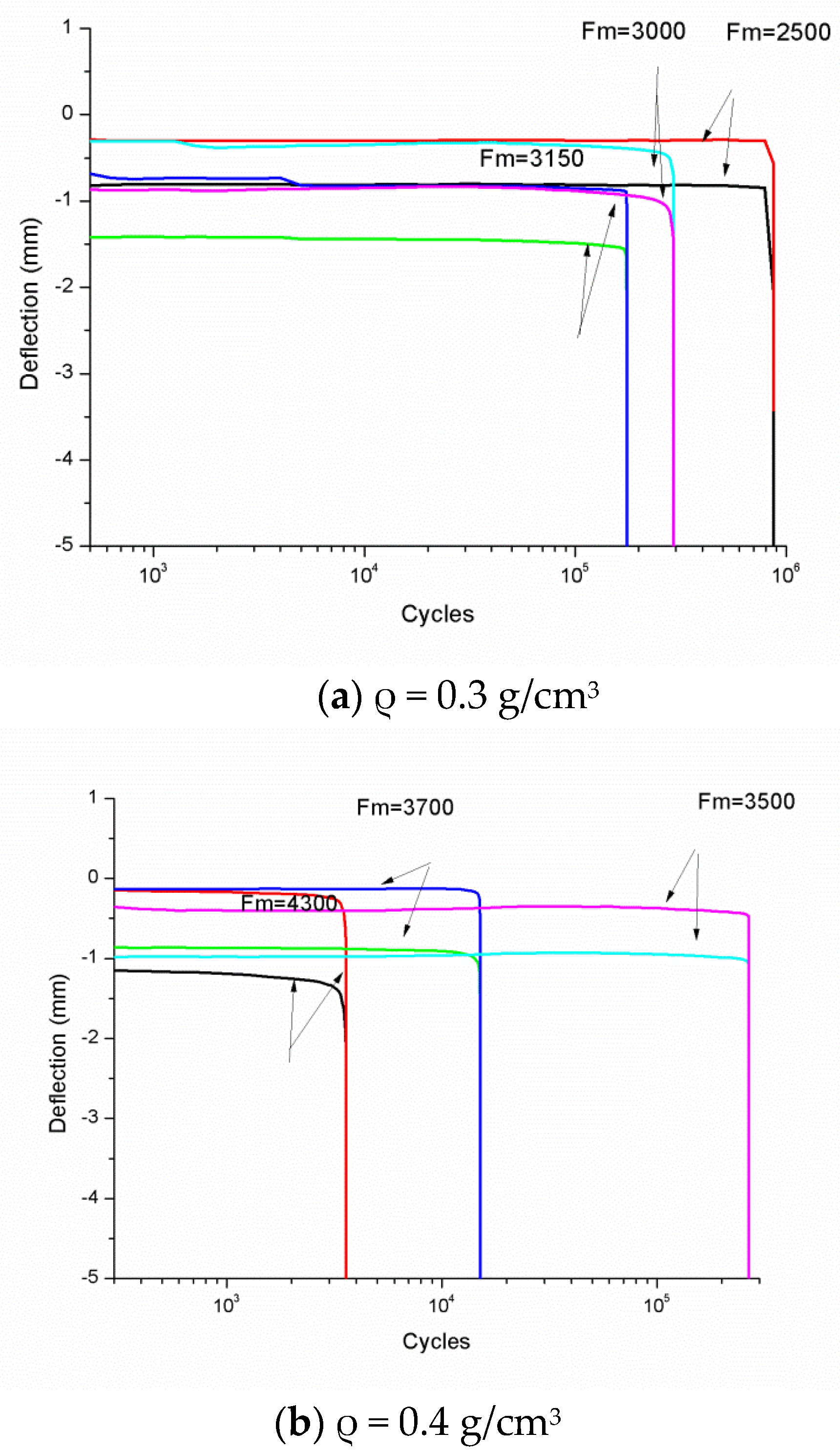
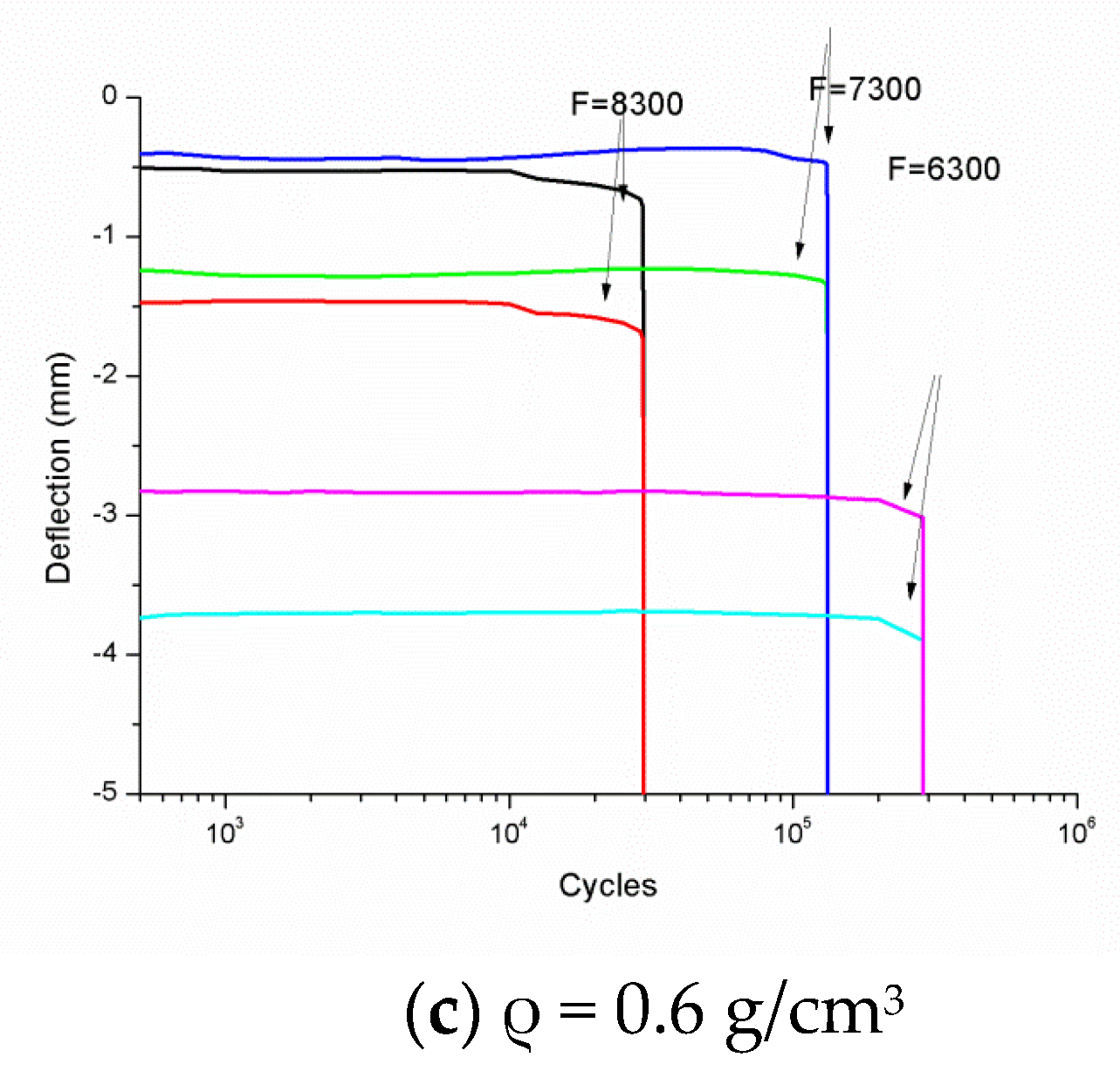
3.2. Deflection
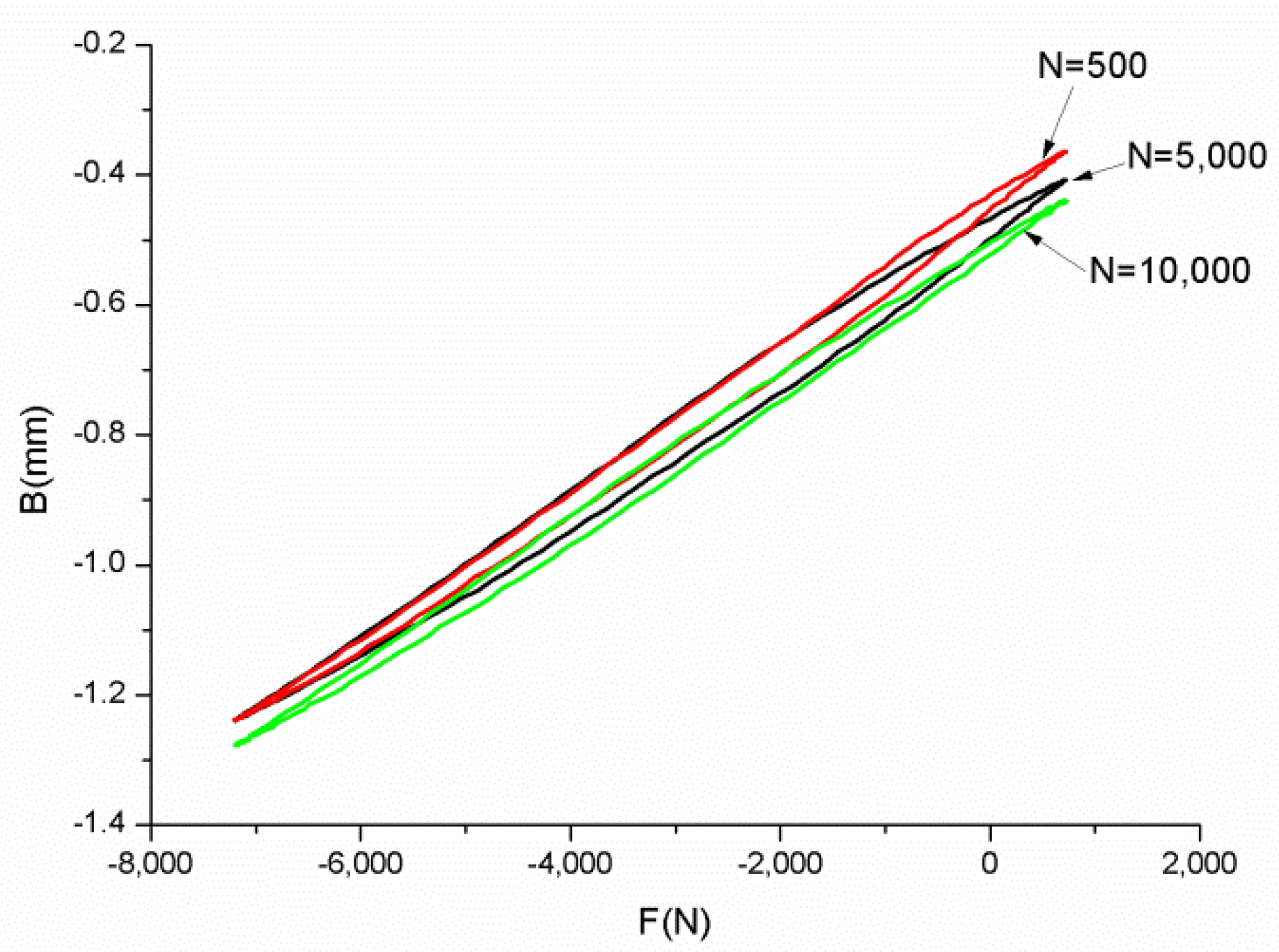
3.3. Hysteretic Curves
3.4. Fatigue Fracture Morphology
3.5. Stiffness Degradation
- (1)
- The first stage is the initial loading stage, during which the stiffness attenuation is rapid. The first stage is very short, for the initial loading stage is in the adaptation stage. At the same time, initial defects in the aluminum foam sandwich panels occur due to the porous characteristics of aluminum foam;
- (2)
- The second stage is the stable stage of stiffness degradation, which accounts for the majority of the fatigue life. The fatigue damage accumulates slowly with the number of cycles, and the stiffness degrades relatively slowly;
- (3)
- The third stage is a stage of rapid stiffness degradation. After the slow accumulation of fatigue damage in the second stage, the internal fatigue damage reaches a critical value. The stiffness decreases rapidly, and the samples come to failure in the end.
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ashby, M.F.; Lu, T.J. Metals foam: A survey. Sci. China 2003, 46, 521–532. [Google Scholar] [CrossRef]
- Davies, G.J.; Zhen, S. Metallic foams: Their production, properties and applications. J. Mater. Sci. 1983, 18, 1899–1911. [Google Scholar] [CrossRef]
- Alvandi-Tabrizi, Y.; Whisler, D.A.; Kim, H.; Rabiei, A. High strain rate behavior of composite metal foams. Mater. Sci. Eng. 2015, A631, 248–257. [Google Scholar] [CrossRef]
- Gui, M.C.; Wang, D.B.; Wu, J.J.; Yuan, G.J.; Li, C.G. Deformation and damping behaviors of foamed Al-Si-SiCp composite. Mater. Sci. Eng. 2000, A286, 282–288. [Google Scholar] [CrossRef]
- Gibson, L.J.; Ashby, M.F. Cellular Solids: Structure & Properties; Cambridge University Press: Cambridge, UK, 1997; p. 510. [Google Scholar]
- Katona, B.; Szebényi, G.; Orbulov, I.N. Fatigue properties of ceramic hollow sphere filled aluminium matrix syntactic foams. Mater. Sci. Eng. 2017, A679, 350–357. [Google Scholar] [CrossRef] [Green Version]
- Katona, B.; Szebényi, A.T.; Orbulov, I.N. Compressive characteristics and low frequency damping of aluminium matrix syntactic foams. Mater. Sci. Eng. 2019, A739, 140–148. [Google Scholar] [CrossRef]
- Banhart, J. Manufacture, Characterization and Application of Cellular Metals And Metal Foams. Prog. Mater. Sci. 2001, 46, 559–632. [Google Scholar] [CrossRef]
- Banhart, J.; Seeliger, H.W. Aluminium Foam Sandwich Panels: Manufacture, Metallurgy and Applications. Adv. Eng. Mater. 2008, 10, 793–802. [Google Scholar] [CrossRef]
- Garcia-Moreno, F. Commercial Applications of Metal Foams: Their Properties and Production. Materials 2018, 9, 85. [Google Scholar] [CrossRef]
- Crupi, V.; Epasto, G.; Guglielmino, E. Comparison of aluminium sandwiches for lightweight ship structures: Honeycomb vs. foam. Mar. Struct. 2013, 30, 74–96. [Google Scholar] [CrossRef]
- Golovin, I.S.; Sinning, H.R. Damping in some cellular metallic materials. J. Alloys Compd. 2003, 355, 2–9. [Google Scholar] [CrossRef]
- Yang, D.H.; Yang, S.R.; Wang, H.; Ma, A.B.; Jiang, J.H.; Chen, J.Q.; Wang, D.L. Compressive properties of cellular Mg foams fabricated by melt-foaming method. Mater. Sci. Eng. 2010, A527, 5405–5409. [Google Scholar] [CrossRef]
- Huang, Z.; Qin, Z.; Chu, F. Damping mechanism of elastic-viscoelastic-elastic sandwich structures. Compos. Struct. 2016, 153, 96–107. [Google Scholar] [CrossRef]
- Yu, G.C.; Feng, L.J.; Wu, L.Z. Thermal and mechanical properties of a multifunctional composite square honeycomb sandwich structure. Mater. Des. 2016, 102, 238–246. [Google Scholar] [CrossRef]
- Harte, A.M.; Fleck, N.A.; Ashby, M.F. Sandwich panel design using Aluminum alloy foam. Adv. Eng. Mater. 2000, 2, 219–222. [Google Scholar] [CrossRef]
- Burman, M.; Zenkert, D. Fatigue of foam core sandwich beams—1: Undamaged specimens. Int. J. Fatigue 1997, 19, 551–561. [Google Scholar] [CrossRef]
- Harte, A.M.; Fleck, N.A.; Ashby, M.F. The fatigue strength of sandwich beams with an aluminium alloy foam core. Int. J. Fatigue 2001, 23, 499–507. [Google Scholar] [CrossRef]
- Chen, C.; Harte, A.M.; Fleck, N.; Ashby, A. The plastic collapse of sandwich beams with a metallic foam. Int. J. Mech. Sci. 2001, 43, 1483–1506. [Google Scholar] [CrossRef]
- Vendra, L.; Neville, B.; Rabiei, A. Fatigue in aluminum–steel and steel–steel composite foams. Mater. Sci. Eng. A 2009, 517, 146–153. [Google Scholar] [CrossRef]
- Yang, F.P.; Lin, Q.Y.; Jiang, J.J. Experimental study on fatigue failure and damage of sandwich structure with PMI foam core. Fatigue Fract. Eng. Mater. Struct. 2015, 38, 456–465. [Google Scholar] [CrossRef]
- Shapovalov, O.S.; Kodzhaspirov, G.E.; Hantelmann, K.; Mikhailov, V.G. Effect of a Welding Method on the Fatigue Strength and Fracture of Foam-Aluminum Sandwiches. Russ. Metall. 2019, 10, 1063–1066. [Google Scholar] [CrossRef]
- Wang, Z.G.; Li, Z.D.; Xiong, W. Experimental investigation on bending behavior of honeycomb sandwich panel with ceramic tile face-sheet. Compost. Part B-Eng. 2019, 164, 280–286. [Google Scholar] [CrossRef]
- Yao, C.; Hu, Z.F.; Mo, F.; Wang, Y. Fabrication and Fatigue Behavior of Aluminum Foam Sandwich Panel via Liquid Diffusion Welding Method. Metals 2019, 9, 582. [Google Scholar] [CrossRef] [Green Version]
- Duart, I.; Teixeir-Dias, F.; Graça, A.; Ferreira, A.J.M. Failure Modes and Influence of the Quasi-static Deformation Rate on the Mechanical Behavior of Sandwich Panels with Aluminum Foam Cores. Mech. Adv. Mater. Struct. 2010, 17, 335–342. [Google Scholar] [CrossRef]
- Zhao, J.; Cai, G.; Larbi, A.S.; Zhang, Y.; Dun, H.; Degée, H.; Vandoren, B. Hysteretic behaviour of steel fibre RC coupled shear walls under cyclic loads: Experimental study and modeling. Eng. Struct. 2018, 156, 92–104. [Google Scholar] [CrossRef]
- Zhang, Z.; Bai, Y.; He, X.; Jin, L.; Zhu, L. Cyclic performance of bonded sleeve beam-column connections for FRP tubular sections. Compos. Part B 2018, 142, 172–182. [Google Scholar] [CrossRef]
- ASTM_C393-C393M-06 Standard Test Method for Core Shear Properties of Sandwich Constructions by Beam Flexure; ASTM International: West Conshohocken, PA, USA, 2006.
- Roosen, D. Fatigue behavior of sandwich foam core materials comparison of different core materials. J. Adv. Mater. 2005, 37, 16–20. [Google Scholar]
- El Mahi, A.; Farooq, M.K.; Sahraoui, S.; Bezazi, A. Modelling the flexural behaviour of sandwich composite materials under cyclic fatigue. Mater. Des. 2004, 25, 199–208. [Google Scholar] [CrossRef]


| Number | Density (g/cm3) | Relative Density (ρ/ρs) | Cell Size (mm) |
|---|---|---|---|
| 1 | 0.3 | 0.11 | 10 |
| 2 | 0.4 | 0.15 | 7 |
| 3 | 0.6 | 0.22 | 4 |
| Project | a | l | S | c | b | t | H | R |
|---|---|---|---|---|---|---|---|---|
| Length (mm) | 300 | 50 | 200 | 25 | 50 | 1 | 27 | 5 |
| ρ = 0.3 | F(N) | 3700 | 3350 | 3150 | 3000 | 2500 | |
| Cycles | 267 | 965 | 176,088 | 292,371 | 871,419 | ||
| ρ = 0.4 | F(N) | 5300 | 4300 | 3700 | 3500 | 3350 | 3150 |
| Cycles | 157 | 3592 | 15,861 | 267,345 | 958,252 | 1,320,034 | |
| ρ = 0.6 | F(N) | 8300 | 7300 | 6300 | 5300 | ||
| Cycles | 838 | 131,727 | 287,251 | 987,570 |
| Number | Density (g/cm3) | Fatigue Life Limit (N, 5 × 106 Cycles) |
|---|---|---|
| 1 | 0.3 | 2501.8 |
| 2 | 0.4 | 2829.3 |
| 3 | 0.6 | 4047.1 |
| Density (g/cm3) | Fm (N) | Chosen Cycle of the Hysteresis Curve | First Cycle Area (N·mm) | Half Cycle Area (N·mm) | Last Cycle Area (N·mm) |
|---|---|---|---|---|---|
| 0.3 | 2500 | 500, 400,000, 800,000 | 3403 | 3316 | 3381 |
| 3000 | 500, 100,000, 200,000 | 2215 | 2248 | 2435 | |
| 3150 | 500, 50,000, 100,000 | 7612 | 8156 | 8271 | |
| 0.4 | 3500 | 500, 100,000, 200,000 | 5119 | 5276 | 5444 |
| 3700 | 500, 5000, 10,000 | 4007 | 4090 | 4175 | |
| 4300 | 500, 2000, 3000 | 6417 | 6496 | 7602 | |
| 0.6 | 6300 | 500, 100,000, 200,000 | 9347 | 9402 | 9915 |
| 7300 | 500, 50,000, 100,000 | 12,865 | 13,128 | 13,670 | |
| 8300 | 500, 10,000, 20,000 | 17,836 | 18,215 | 19,964 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yao, C.; Hu, Z.; Mo, F. Three-Point Bending Fatigue Behavior of Aluminum Foam Sandwich Panels with Different Density Core Material. Metals 2021, 11, 1542. https://doi.org/10.3390/met11101542
Yao C, Hu Z, Mo F. Three-Point Bending Fatigue Behavior of Aluminum Foam Sandwich Panels with Different Density Core Material. Metals. 2021; 11(10):1542. https://doi.org/10.3390/met11101542
Chicago/Turabian StyleYao, Cheng, Zhengfei Hu, and Fan Mo. 2021. "Three-Point Bending Fatigue Behavior of Aluminum Foam Sandwich Panels with Different Density Core Material" Metals 11, no. 10: 1542. https://doi.org/10.3390/met11101542
APA StyleYao, C., Hu, Z., & Mo, F. (2021). Three-Point Bending Fatigue Behavior of Aluminum Foam Sandwich Panels with Different Density Core Material. Metals, 11(10), 1542. https://doi.org/10.3390/met11101542






