Structural-Phase State, Mechanical Properties, Acoustic and Magnetic Characteristics in the Sustainable Deformation Localization Zones of Power Equipment Made of Structural and Heat Resistant Steels
Abstract
:1. Introduction
2. Materials and Methods
3. Results
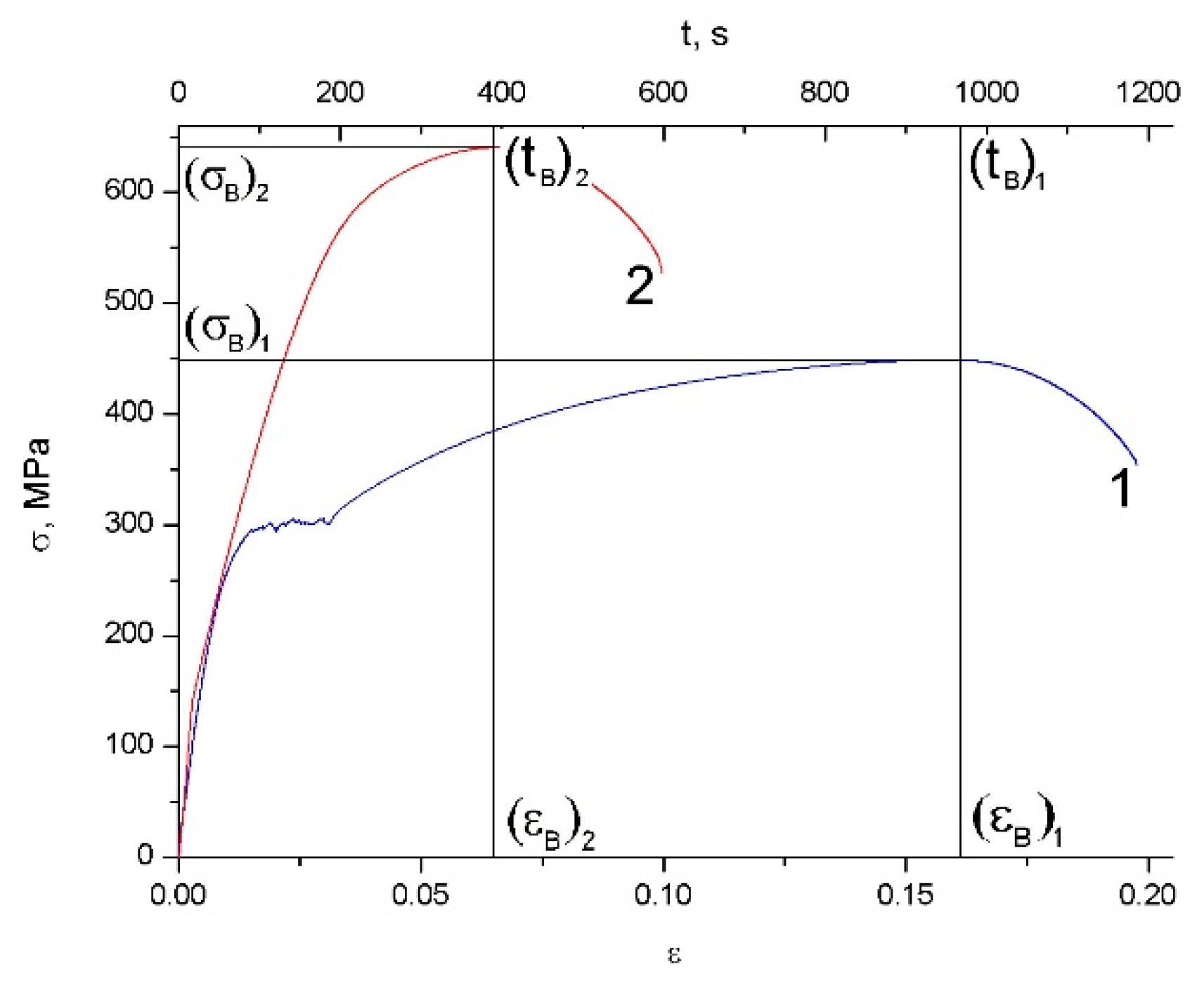
3.1. Mechanical Test Results of Samples of Power Equipment Made of Structural and Heat Resistant Steels
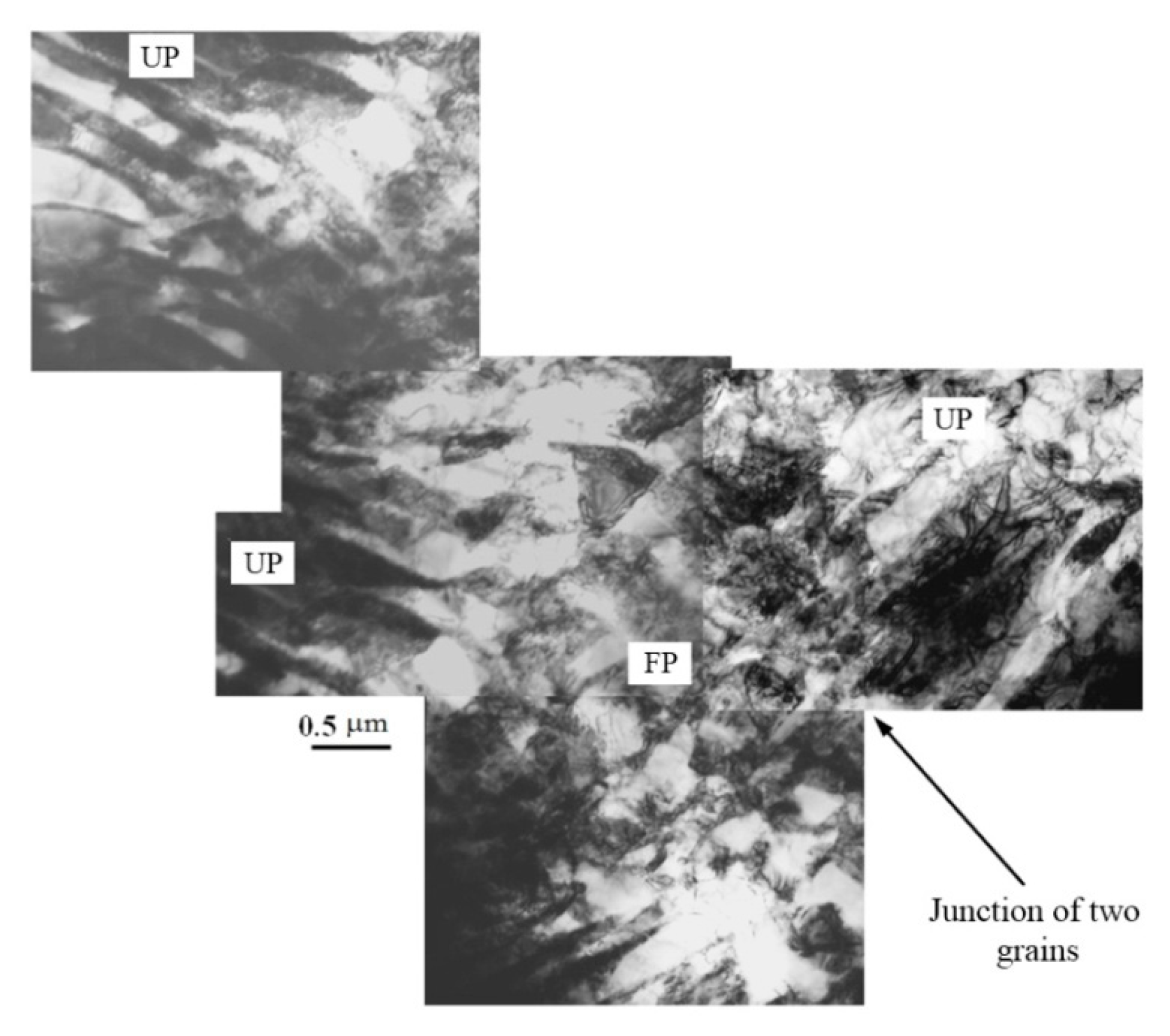
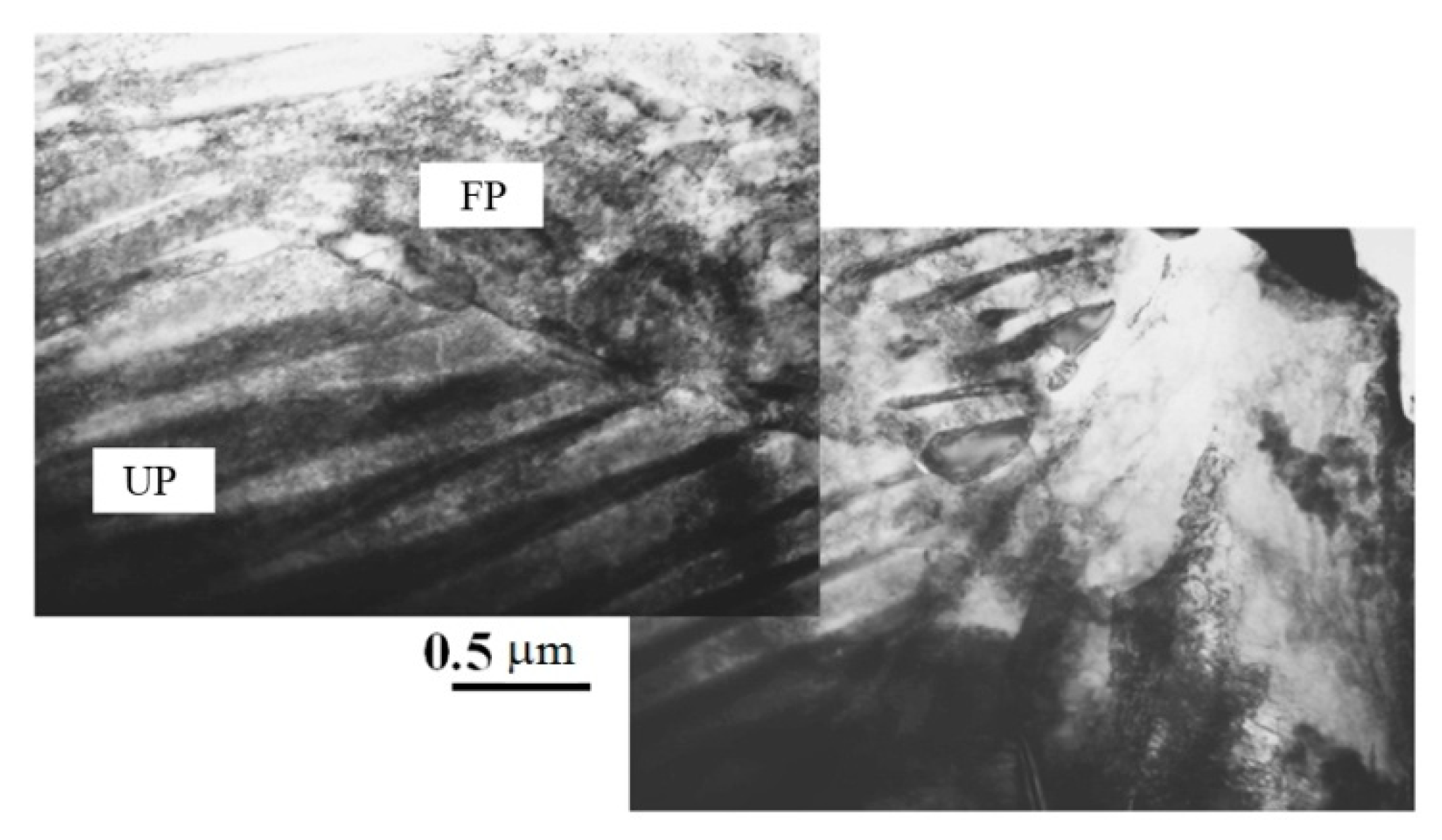
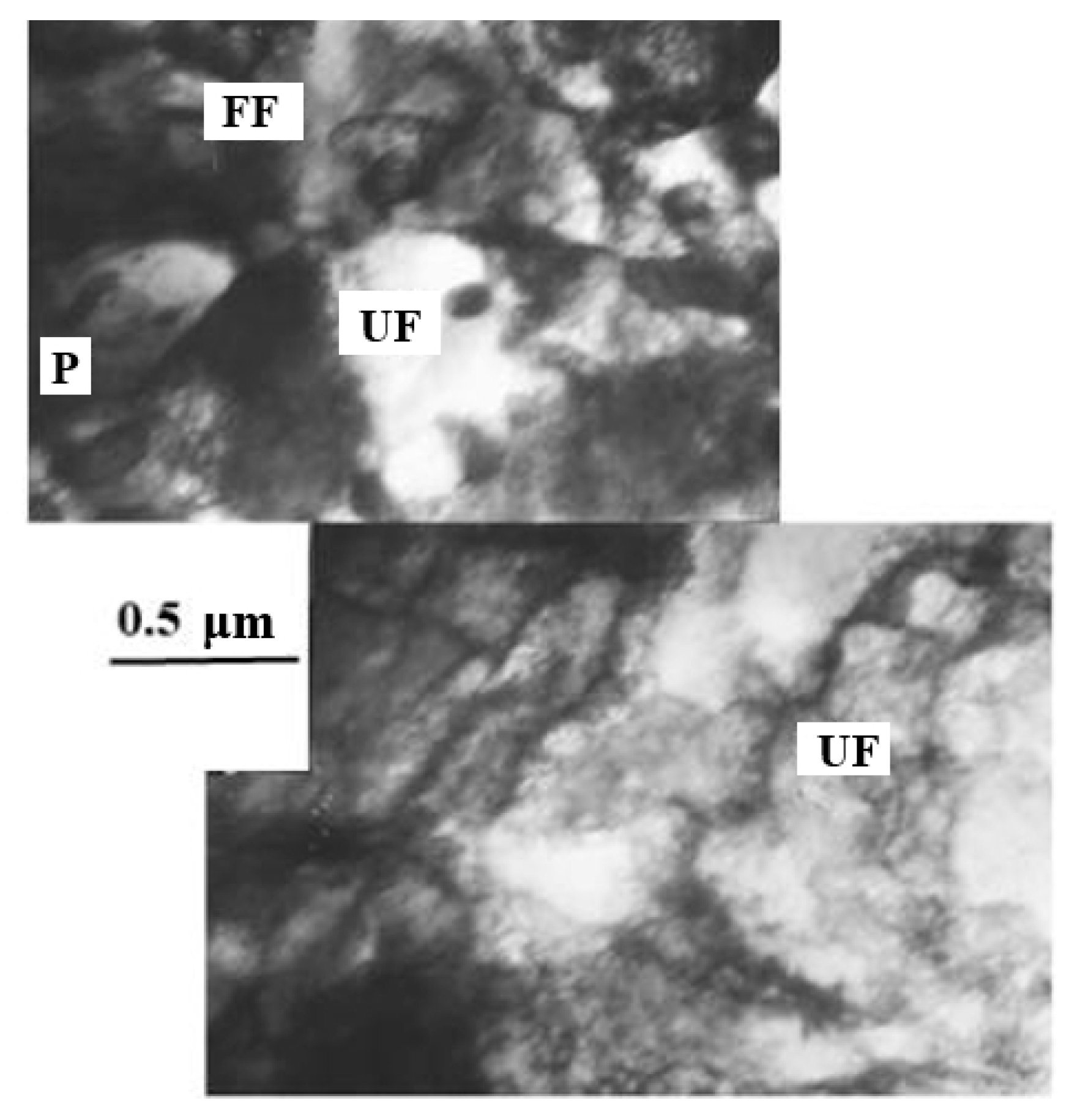
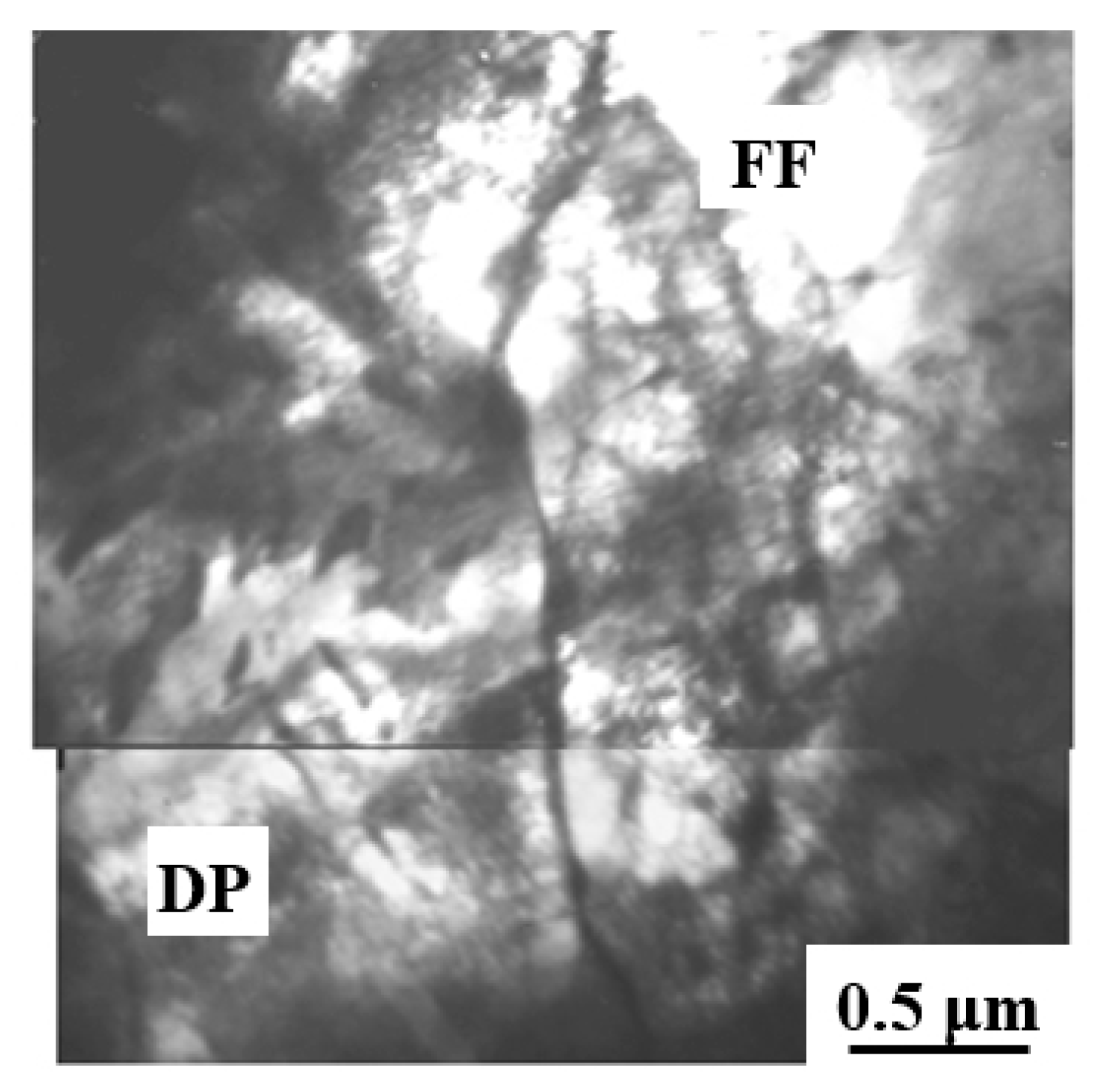
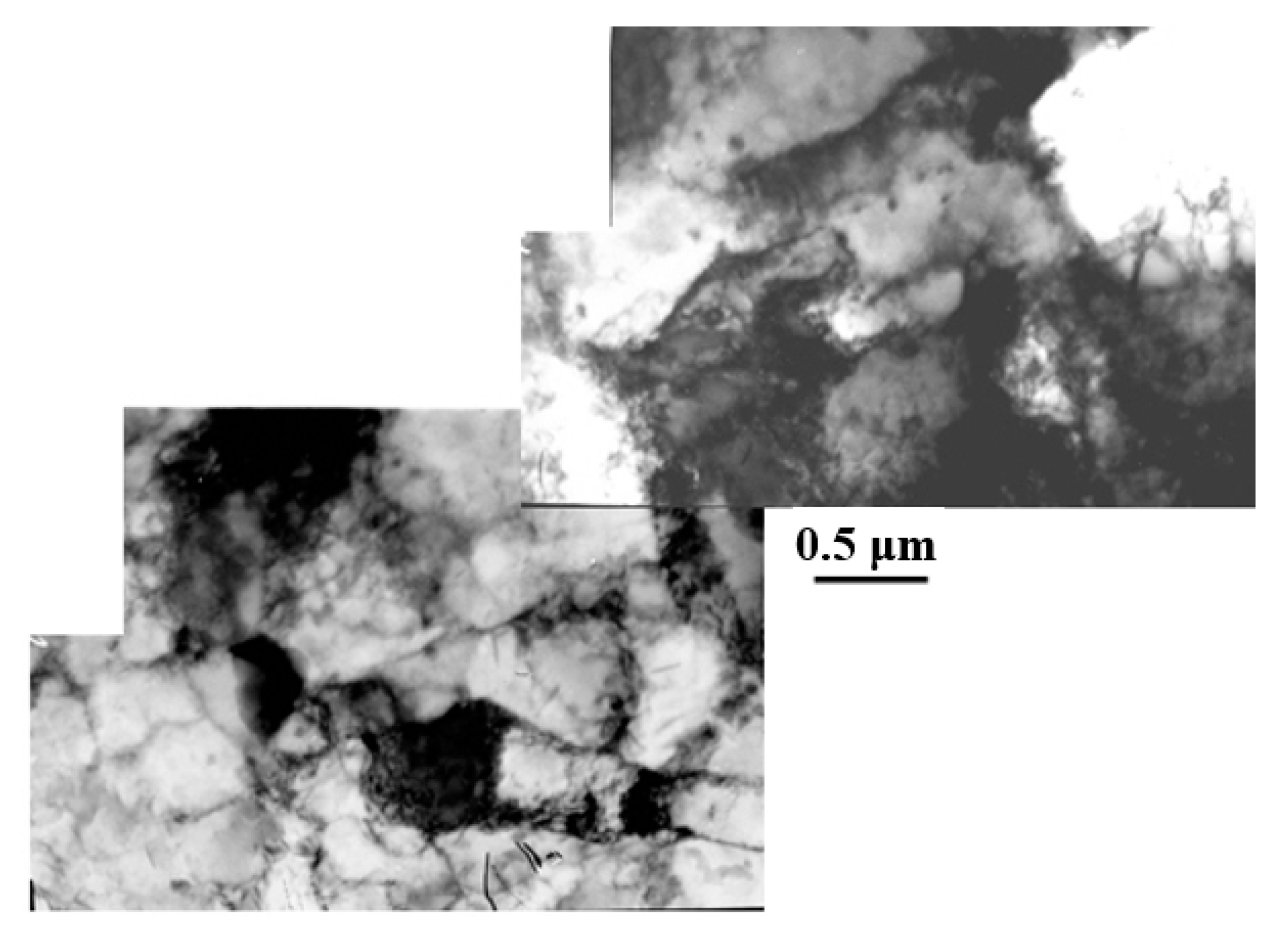
3.2. Structural-Phase State of Samples of Power Equipment Made of Structural and Heat-Resistant Steels before Deformation and in Zones of Stable Localization of Deformation
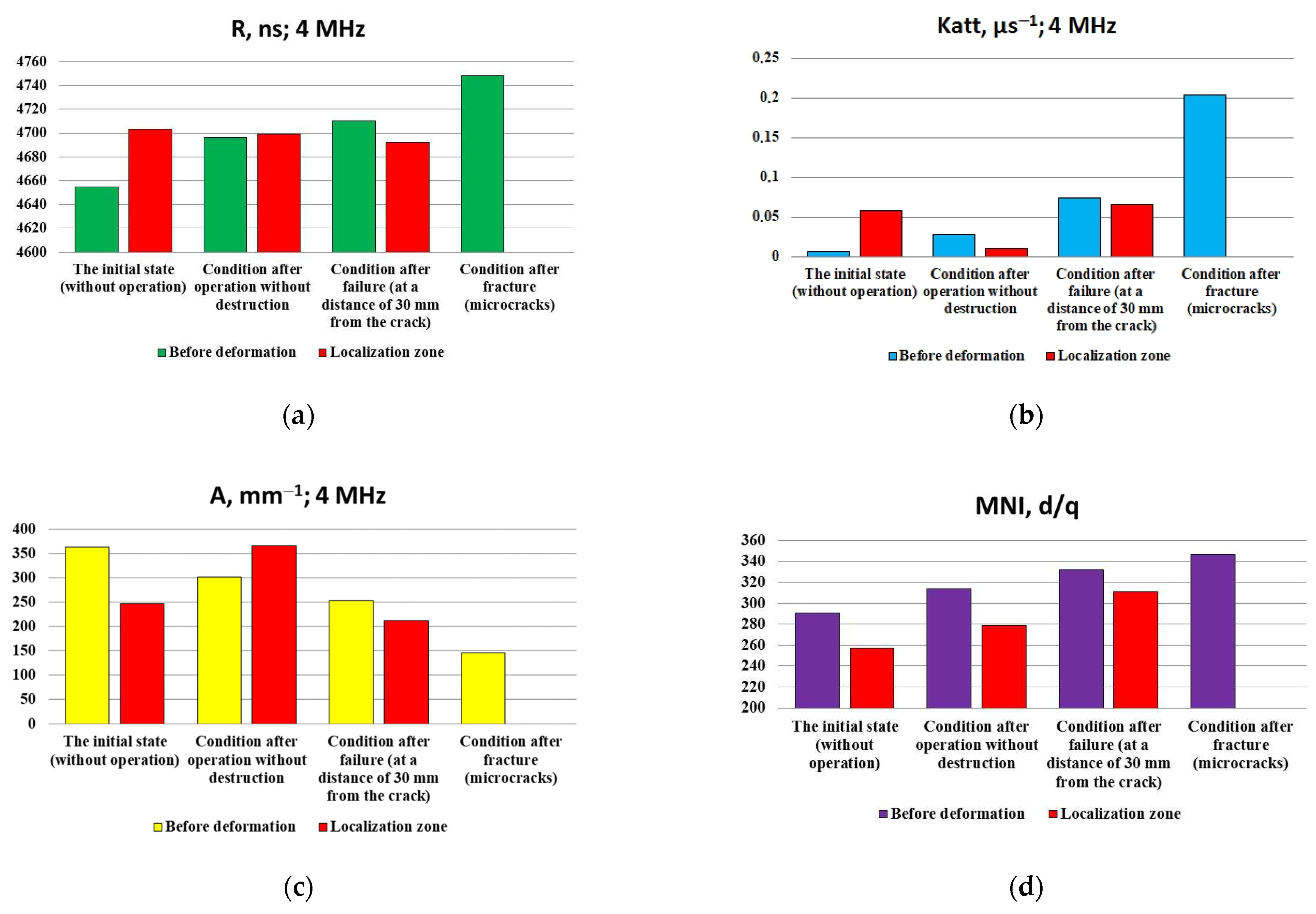
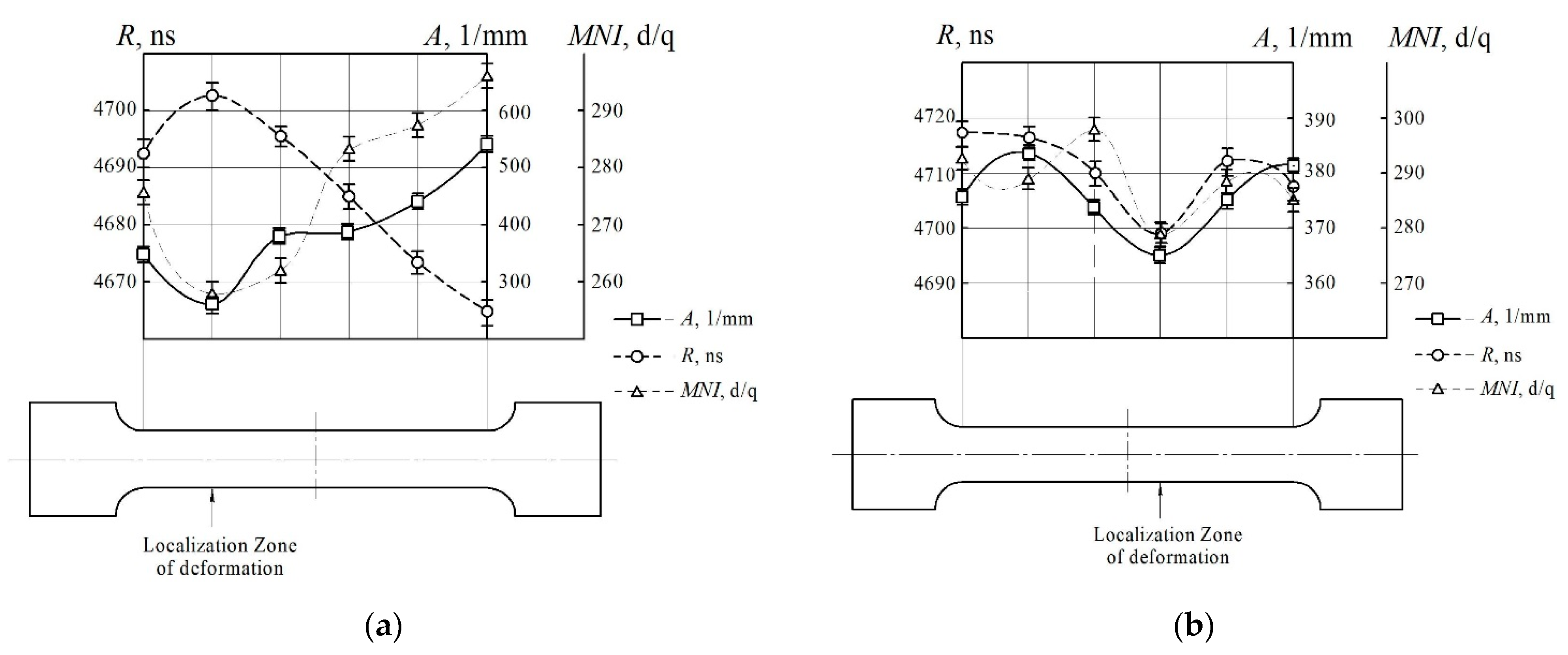
3.3. Acoustic and Magnetic Characteristics of Metal Samples of Power Equipment Made of Structural and Heat-Resistant Steels before Deformation and in Zones of Stable Deformation Localization
4. Discussion
4.1. Discussion of the Results of Mechanical Tests of Samples of Power Equipment Made of Structural and Heat-Resistant Steels
4.2. Discussion of the Results of Studying the Structural-Phase State of Samples of Power Equipment Made of Structural and Heat-Resistant Steels before Deformation and in Zones of Stable Localization of Deformation
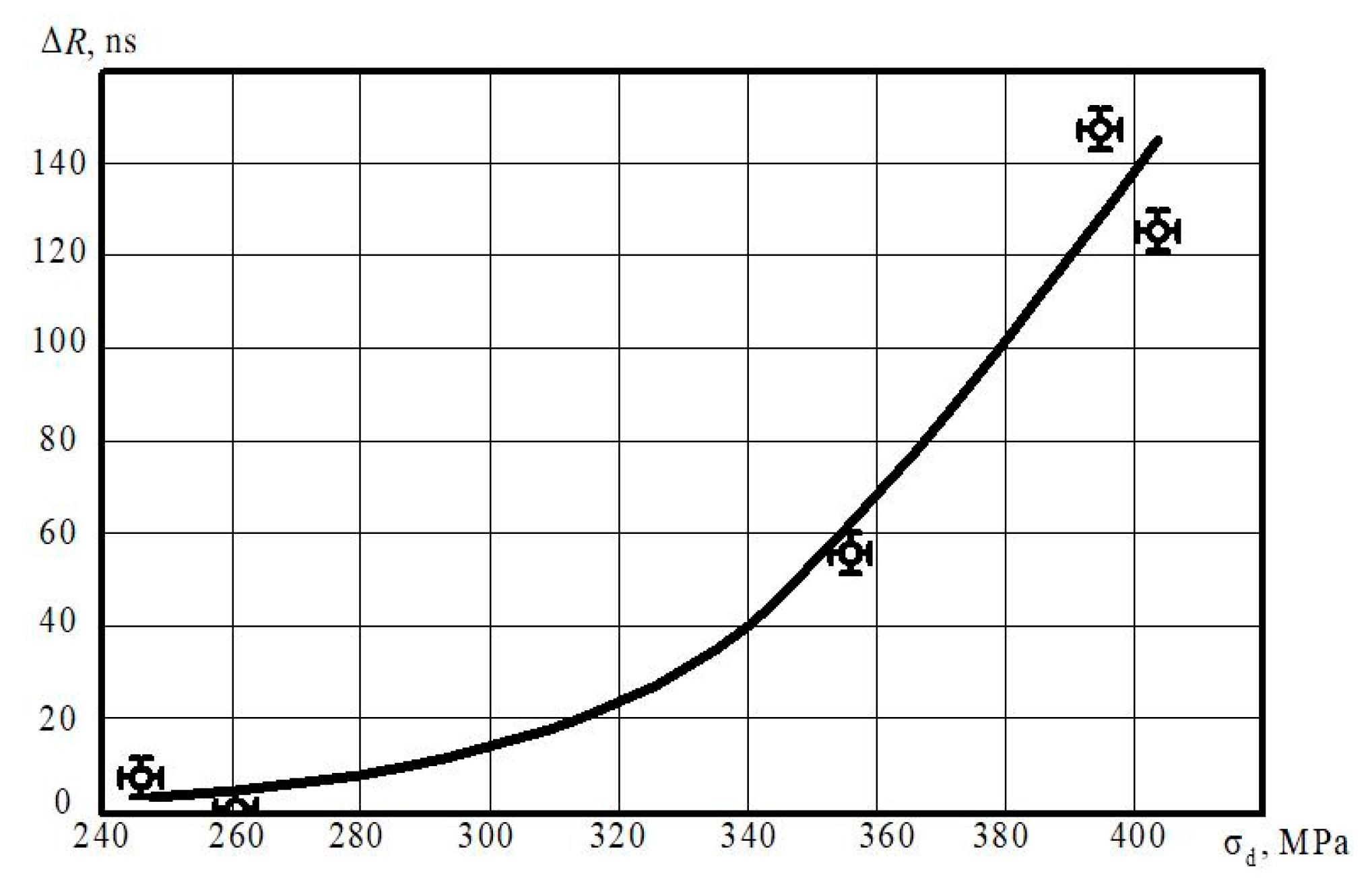
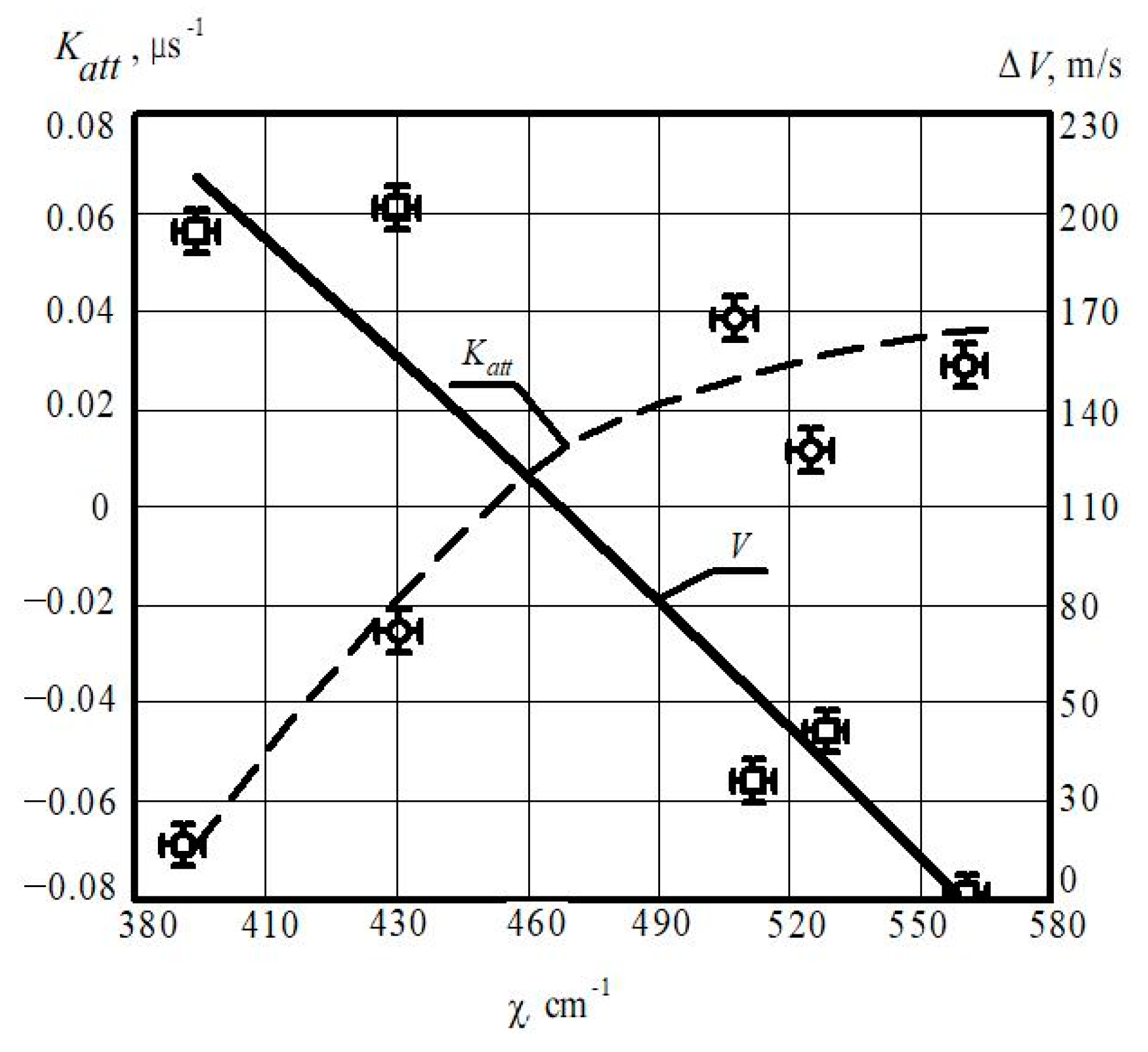
4.3. Discussion of the Results of Measuring the Acoustic and Magnetic Characteristics of the Metal of Samples of Power Equipment Made of Structural and Heat-Resistant Steels before Deformation and in Zones of Stable Localization of Deformation
4.4. Statistical Processing of the Results
5. Conclusions
- The studies of the structural-phase state of the metal of the samples were carried out without operation, after operation without destruction and with destruction from structural and heat resistant steels in the zone of stable localization of macrodeformations. A connection between the processes of localization of macrodeformations and the evolution of the substructural state for structural and heat resistant steels is established. On the basis of the presented results and their discussion, on the one hand, it can be argued that information on the localization of macrodeformation, together with the data of the spectral-acoustic method, can be used to assess the residual life of a long-term operating power equipment.
- The structure of the metal of all specimens made of structural and heat-resistant steels after deformation before the formation of zones of stable localization of deformations consists of a ferrite-pearlite mixture, and for specimens after operation before fracture only of unfragmented and fragmented ferrite. Ferrite, which occupies the bulk of the material, is present both unfragmented and fragmented. For all samples, the ratios ρ > ρ±, χ = χpl, σL > σd were calculated, which indicate whether there is a danger of the initiation of microcracks in metal samples. For specimens without operation and after operation without damage in zones of stable localization of deformations, these conditions are met, and for specimens after operation until destruction, they are not met.
- The structure of the metal in the zones of localization of deformations directly affects the characteristics of non-destructive tests. The characteristics of non-destructive tests for all investigated states of samples made of structural and heat-resistant steels in the zones of localization of deformation had similar values: the delay time of the surface acoustic wave was 4700 ± 5 ns; attenuation coefficient of the surface acoustic wave 0.006 ± 0.003 μs−1 and 0.004 ± 0.002 μs−1; the amplitude range of the received signal of the surface acoustic wave is 227 ± 12 mm−1 and 302 ± 14 mm−1 and the intensity of magnetic noise is 275 ± 31 units and 400 ± 19 units for structural and heat-resistant steels, respectively. The possibility of detecting zones of localized deformation by the spectral-acoustic control method in structural and heat-resistant steels has been found.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Rezinskikh, V.F.; Zlepko, V.F.; Shtromberg, Y.Y. The main requirements for the control of metal heat power equipment. Therm. Eng. 1999, 46, 8–20. [Google Scholar]
- Chrysochoos, A.; Louche, H. An infrared image processing to analyse the calorific effects accompanying strain localization. Int. J. Eng. Sci. 2000, 38, 1759–1788. [Google Scholar] [CrossRef]
- Sun, H.B.; Yoshida, F.; Ohmori, M.; Ma, X. Effect of strain rate on Lüders band propagating velocity and Lüders strain for annealed mild steel under uniaxial tension. Mater. Lett. 2003, 57, 4535–4539. [Google Scholar] [CrossRef]
- Avril, S.; Pierron, F.; Sutton, M.A.; Yan, J. Identification of elasto-visco-plastic parameters and characterization of Lüders behavior using digital image correlation and the virtual field method. Mech. Mater. 2008, 40, 729–742. [Google Scholar] [CrossRef] [Green Version]
- Makhutov, N.A. Strength, service life, and safety of machine systems. J. Mach. Manuf. Reliab. 2014, 43, 217–232. [Google Scholar] [CrossRef]
- Tyutin, M.R.; Botvina, L.R.; Sinev, I.O. Changes in the physical properties and the damage of low- and medium-carbon steels during tension. Russ. Metall. 2018, 2018, 521–528. [Google Scholar] [CrossRef]
- Klevtsov, G.V.; Botvina, L.R. Microscopic and macroscopic plastic deformation as a criterion of the limiting state of a material during fracture. Strength Mater. 1984, 16, 473–479. [Google Scholar] [CrossRef]
- Syromyatnikova, A.S.; Gulyaeva, E.M.; Alekseeva, K.I. Estimation of the degree of plastic deformation and the remaining life of gas main pipes having operated for a long time. Russ. Metall. 2016, 2016, 996–998. [Google Scholar] [CrossRef]
- Korsunsky, A.M.; Botvina, L.R.; Kim, K. Defect population statistics near and far from critical event. Int. J. Fract. 2004, 128, 139–145. [Google Scholar] [CrossRef]
- Smirnov, A.N.; Ozhiganov, E.A.; Danilov, V.I.; Gorbatenko, V.V.; Murav’ev, V.V. The dependence of local deformations and internal stress fields on welding technique for grade VSt3Sp structural steel: I. The influence of welding technique on the mechanical characteristics and acoustic emission parameters of grade VSt3Sp steel. Russ. J. Nondestruct. Test. 2015, 51, 705–712. [Google Scholar] [CrossRef]
- Barnett, M.R.; Nave, M.D.; Ghaderi, A. Yield point elongation due to twinning in a magnesium alloy. Acta Mater. 2012, 60, 1433–1443. [Google Scholar] [CrossRef]
- Wang, X.G.; Wang, L.; Huang, M.X. Kinematic and thermal characteristics of Lüders and Portevin-Le Châtelier bands in a medium Mn transformation-induced plasticity steel. Acta Mater. 2017, 124, 17–29. [Google Scholar] [CrossRef] [Green Version]
- Shklyarov, M.; Osmakov, V.; Edachev, G. Some features of selection of structurally free graphite in welded connections of steam pipes from carbon steel. Therm. Eng. 1999, 5, 360–365. [Google Scholar]
- Grote, K.; Antonsson, E. Handbook of Mechanical Engineering; Springer: New York, NY, USA, 2008. [Google Scholar]
- Kreith, F. The CRC Handbook of Thermal Engineering; CRC Press: Boca Raton, FL, USA, 2000. [Google Scholar]
- Segal, V.M.; Noskova, N.I.; Mulyukov, R.R.; Kopylov, V.I.; Chuvil’deev, V.N.; Nazarov, A.A.; Levit, V.; Smirnova, N.; Han, B.Q.; Mohamed, F.A.; et al. Severe Plastic Deformation: Toward Bulk Production of Nanostructured Materials; Nova Science Publishers, Inc.: New York, NY, USA, 2006. [Google Scholar]
- Zharkova, N.A.; Botvina, L.R.; Tyutin, M.R. Damage accumulation stages in a low-carbon steel during uniaxial tension. Russ. Metall. 2007, 2007, 223–229. [Google Scholar] [CrossRef]
- Tyutin, M.R.; Botvina, L.R.; Zharkova, N.A.; Petersen, T.B.; Hudson, J.A. Evolution of damage accumulation in low-carbon steel in tension condition. Strength Fract. Complex. 2005, 3, 73–80. [Google Scholar]
- Botvina, L.R.; Tyutin, M.R.; Levin, V.P.; Soldatenkov, A.P.; Prosvirnin, D.V.; Petersen, T.B. Residual strength, microhardness, and acoustic properties of low-carbon steel after cyclic loading. J. Mach. Manuf. Reliab. 2018, 47, 516–524. [Google Scholar] [CrossRef]
- Information Bulletin of the Federal Service for Environmental, Technological and Nuclear Supervision; No. 106; Federal Service for Environmental, Technological and Nuclear Supervision: Moscow, Russia, 2020; Volume 1, pp. 22–24.
- Khlybov, A.A. Studying the effect of microscopic medium inhomogeneity on the propagation of surface waves. Russ. J. Nondestruct. Test. 2018, 54, 385–393. [Google Scholar] [CrossRef]
- Mishakin, V.V.; Klyushnikov, V.A.; Kassina, N.V. Research on the fracture process of steels by the acoustic method and the pitch net method. J. Mach. Manuf. Reliab. 2014, 38, 443–447. [Google Scholar] [CrossRef]
- Mishakin, V.V.; Serebryany, V.N. Aplication of the acoustoelastic effect in the precise evaluation of the plastic strain value. Acoust. Lett. 1994, 17, 123. [Google Scholar]
- Ser’eznov, A.N.; Murav’ev, V.V.; Stepanova, L.N.; Barabanova, S.B.; Kozhemyakin, V.L.; Kabanov, S.I. Localizing acoustic-emission signals in metal structures. Russ. J. Nondestruct. Test. 1997, 33, 726–730. [Google Scholar]
- Muravyev, V.V.; Muravyeva, O.V.; Strizhak, V.A.; Pryakhin, A.V.; Balobanov, E.N.; Volkova, L.V. Evaluation of residual stresses in rims of wagon wheels using the electromagnetic-acoustic method. Russ. J. Nondestruct. Test. 2011, 54, 16–28. [Google Scholar] [CrossRef]
- Smirnov, A.N.; Ababkov, N.V.; Muravev, V.V.; Folmer, S.V. Criteria for the evaluation of the technical state of the long-lived metal of hpp equipment based on acoustic structuroscopy. Russ. J. Nondestruct. Test. 2015, 58, 94–100. [Google Scholar] [CrossRef]
- Danilov, V.I.; Orlova, D.V.; Zuev, L.B. On the kinetics of localized plasticity domains emergent at the pre-failure stage of deformation process. Mater. Des. 2011, 32, 1554–1558. [Google Scholar] [CrossRef]
- Gorbatenko, V.V.; Danilov, V.I.; Zuev, L.B. Elastoplastic transition in the material with sharp yield point. AIP Conf. Proc. 2015, 1683, 020058. [Google Scholar]
- Campbell, F. Elements of Metallurgy and Engineering Alloys; ASM International: Novelty, OH, USA, 2008. [Google Scholar]
- Brent, F.; James, H. Transmission Electron Microscopy and Diffractometry of Materials; Springer: New York, NY, USA, 2013. [Google Scholar]
- Vorob’ev, G.M.; Popova, V.I. Stereometric metallography in the-interpretation of polar figures for compressed iron. Sov. Phys. J. 1965, 8, 29–32. [Google Scholar]
- Gromov, V.E.; Morozov, K.V.; Alsaraeva, K.V.; Yur’ev, A.B.; Ivanov, Y.F. Structure, phase composition, and defect substructure of differentially quenched rail. Steel Transl. 2014, 44, 883–885. [Google Scholar] [CrossRef]
- Kozlov, E.V.; Popova, N.A.; Ignatenko, L.N.; Teplyakova, L.A.; Klopotov, A.A.; Koneva, N.A. Influence of the Substructure Type on the Carbon Redistribution in Martensitic Steel in the Course of Plastic Deformation. Russ. Phys. J. 2002, 45, 285–302. [Google Scholar] [CrossRef]
- Kozlov, E.V.; Popova, N.A.; Pekarskaya, E.E.; Koneva, N.A.; Zhdanov, A.N. Subgrain structure and internal stress fields in ufg materials: Problem of Hall-Petch relation. Mater. Sci. Eng. 2004, 387–389, 789–794. [Google Scholar] [CrossRef]
- Makhutov, N.A. A criterion base for assessment of strength, lifetime, reliability, survivability, and security of machines and man-machine systems. J. Mach. Manuf. Reliab. 2013, 42, 364–373. [Google Scholar] [CrossRef]
- Makhutov, N.A.; Abrosimov, N.V.; Gadenin, M.M. Provision of safety—The priority in the sphere of fundamental and applied research. Econ. Soc. Chang. Facts Trends Forecast 2013, 27, 39–61. [Google Scholar]
- Makhutov, N.A. Generalized regularities of deformation and fracture processes. Her. Russ. Acad. Sci. 2017, 87, 217–228. [Google Scholar] [CrossRef]
- Makhutov, N.A.; Fomin, A.V.; Ivanov, V.I.; Permyakov, V.N.; Vasil’ev, I.E. Integrated diagnostics of limit states and early warning of emergency conditions of structures. J. Mach. Manuf. Reliab. 2013, 42, 109–113. [Google Scholar] [CrossRef]
- Danilov, V.; Gorbatenko, V.; Zuev, L.; Orlova, D. Localized plastic deformation autowaves in materials with unstable phase structure. AIP Conf. Proc. 2018, 2051, 020059. [Google Scholar]
- Zuev, L.B. Chernov–Luders and Portevin–Le Chatelier deformations in active deformable media of different nature. J. Appl. Mech. Tech. Phys. 2017, 58, 328–334. [Google Scholar] [CrossRef]
- Danilov, V.I.; Gorbatenko, V.V.; Orlova, D.V.; Danilova, L.V.; Smirnov, A.N. Luders deformation in weld joints. Steel Transl. 2018, 48, 87–92. [Google Scholar] [CrossRef]
- Ababkov, N.V.; Smirnov, A.N.; Danilov, V.I.; Gorbatenko, V.V. Non-destructive testing and diagnostics of the material after long-term operation in thermal power equipment. AIP Conf. Proc. 2018, 2051, 020001. [Google Scholar]
- Botvina, L.R.; Tyutin, M.R. New acoustic parameter characterizing loading history effects. Eng. Fract. Mechanics. 2018, 202, 358–366. [Google Scholar] [CrossRef]
- Botvina, L.R.; Soldatenkov, A.P.; Levin, V.P.; Tyutin, M.R.; Demina, Y.A.; Petersen, T.B.; Dubov, A.A.; Semashko, N.A. Assessment of mild steel damage characteristics by physical methods. Russ. Metall. 2016, 2016, 23–33. [Google Scholar] [CrossRef]
- Botvina, L.R.; Soldatenkov, A.P.; Tyutin, M.R.; Petersen, T.B. Time dependences of acoustic signal characteristics during fracture of metal samples. Dokl. Earth Sci. 2015, 462, 475–478. [Google Scholar] [CrossRef]
- Wu, K.T.; Jen, C.K.; Kobayashi, M.; Blouin, A. Integrated piezoelectric ultrasonic receivers for laser ultrasound in non-destructive testing of metals. J. Nondestruct. Eval. 2012, 30, 1–8. [Google Scholar] [CrossRef]
- Labergere, C.; Rassineux, A.; Saanouni, K. Numerical simulation of continuous damage and fracture in metal-forming processes with 2D mesh adaptive methodology. Finite Elem. Anal. Des. 2014, 82, 46–61. [Google Scholar] [CrossRef]
- Yang, J.; Wang, G.Z.; Xuan, F.Z.; Tu, S.T.; Liu, C.J. Out-of-plane constraint effect on local fracture resistance of a dissimilar metal welded joint. Mater. Des. 2014, 55, 542–550. [Google Scholar] [CrossRef]
- Osovski, S.; Nachmany, Y.; Rittel, D.; Landau, P.; Venkert, A. On the dynamic character of localized failure. Scr. Mater. 2012, 67, 693–695. [Google Scholar] [CrossRef]
- Khokhlov, M.; Fischer, A.; Rittel, D. Multi-scale stereo-photogrammetry system for fractographic analysis using the scanning elec-tron microscope. Exp. Mech. 2012, 52, 975–991. [Google Scholar] [CrossRef]
- Pokluda, J.; Pippan, R.; Vojtek, T.; Hohenwarter, A. Near-threshold behaviour of shear-mode fatigue cracks in metallic materials. Fatigue Fract. Eng. Mater. Struct. 2014, 37, 232–254. [Google Scholar] [CrossRef]
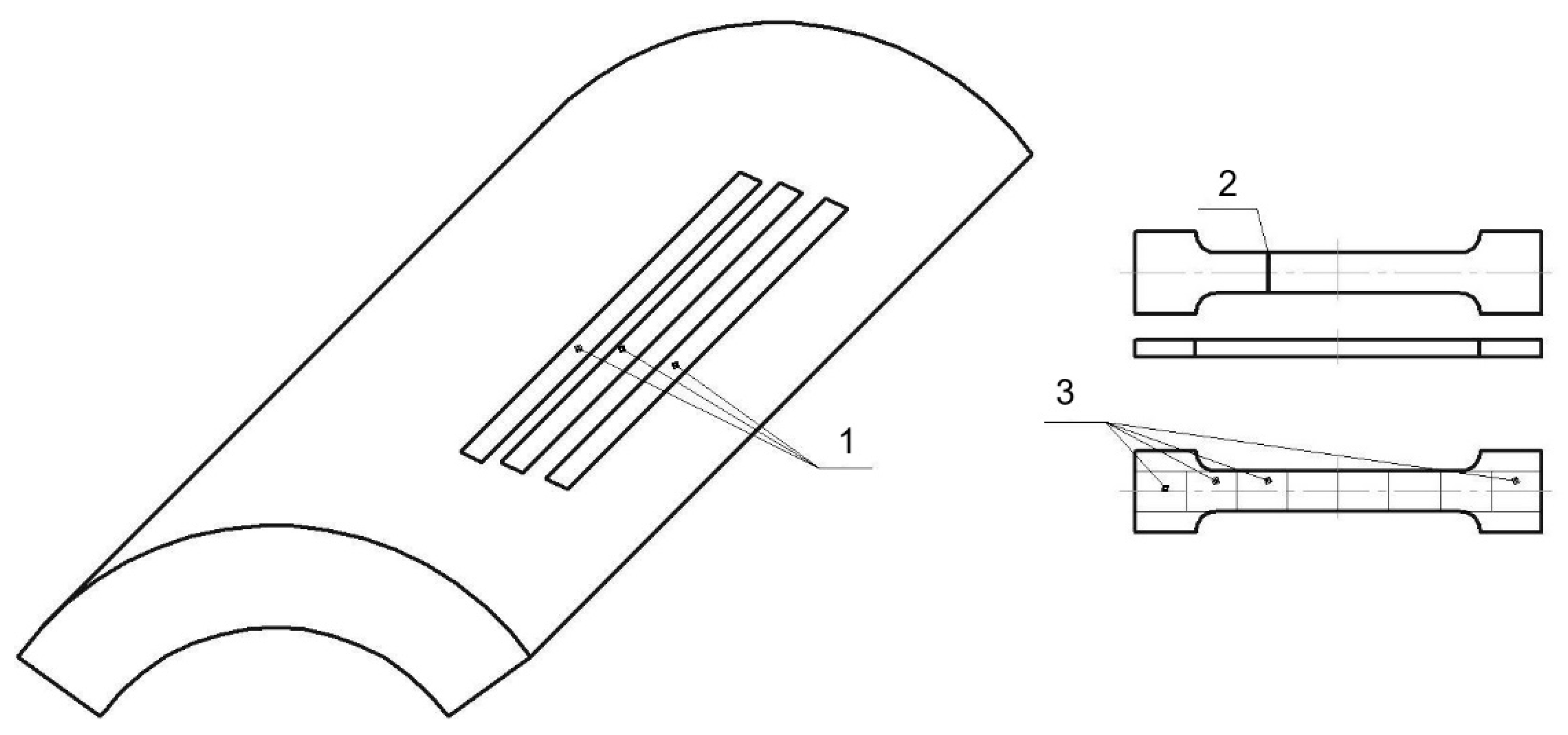






| Steel | Mass Fraction of Elements, % | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| C | Si | Mn | Cr | Ni | Mo | S | P | Va | Fe | |
| 0.2 C steel | 0.17–0.24 | 0.17–0.37 | 0.35–0.65 | till 0.25 | till 0.25 | till 0.25 | till 0.04 | till 0.035 | till 0.08 | ~98 |
| 0.12C-1Cr-1Mo-1V | 0.08–0.15 | 0.17–0.37 | 0.4–0.7 | 0.9–1.2 | till 0.3 | 0.25–0.35 | till 0.025 | till 0.03 | 0.15–0.3 | ~96 |
| The Initial State | After Operation | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| No. | 1 | 2 | 3 | 4 | Average | 1 | 2 | 3 | 4 | 5 | Average |
| tloc, s | 770 | 1070 | 780 | 1070 | 922 ± 170 | 186 | 300 | 168 | 168 | 312 | 227 ± 73 |
| tuts, s | 960 | 1367 | 951 | 1173 | 1112 ± 198 | 354 | 468 | 420 | 366 | 414 | 404 ± 46 |
| tcrac, s | 1342 | 1655 | 1187 | 1376 | 1390 ± 195 | 590 | 793 | 709 | 651 | 599 | 688 ± 84 |
| a1 | 0.52 | 0.67 | 0.70 | 0.82 | 0.69 ± 0.09 | 0.68 | 0.43 | 0.28 | 0.29 | 0.67 | 0.47 ± 0.20 |
| a2 | 0.80 | 0.78 | 0.82 | 0.90 | 0.82 ± 0.05 | 0.52 | 0.64 | 0.40 | 0.46 | 0.75 | 0.51 ± 0.10 |
| Sample Status | Unfragmented Perlite | Fragmented Perlite | Unfragmented Ferrite | Partially Fragmented Ferrite | In General, the Material | ||
|---|---|---|---|---|---|---|---|
| Dislocation Fragments | Micro-Grain | Nano-Grain | |||||
| Initial | 2.50 | 2.65 | - | - | 2.72 | 2.45 | 2.60 |
| Undestroyed | 2.28 | 2.20 | - | - | 2.50 | 2.25 | 2.24 |
| Destroyed | 1.88 | 1.91 | 0.91 | 0 | - | 1.93 | 1.90 |
| Sample Status | Unfragmented Perlite | Fragmented Perlite | Unfragmented Ferrite | Partially Fragmented Ferrite | In General, the Material | ||
|---|---|---|---|---|---|---|---|
| Dislocation Fragments | Micro-Grain | Nano-Grain | |||||
| Initial | 315 | 325 | - | - | 330 | 315 | 320 |
| Undestroyed | 300 | 295 | - | - | 315 | 300 | 295 |
| Destroyed | 275 | 275 | 190 | 0 | - | 275 | 250 |
| Condition | Microstructure Parameters | ||||
|---|---|---|---|---|---|
| ρ, cm−2 | ρ±, cm−2 | χ, cm−1 | σL, MPa | σd, MPa | |
| Initial state | 3.41 × 1010 | 2.24 × 1010 | 560 | 368 | 298 |
| Condition after operation (260 thousand hours) without destruction | 3.0 × 1010 | 2.52 × 1010 | 630 | 330 | 405 |
| Condition after operation (263 thousand hours) and destruction | 2.07 × 1010 | 1.95 × 1010 | 572 | 277 | 427 |
| 0.2 C Steel | 0.12C-1Cr-1Mo-1V Steel |
|---|---|
| ρ = 2.7597 + 0.0003 A − 5.7679 × 10−6 A2 | ρ = −871.11 + 0.3777 R − 4.0809 × 10−5 R2 |
| ρ± = −363.25 + 0.2004 V − 2.7421 × 10−5 V2 | ρ± = 10.81 − 0.00237432 V |
| χ = −3.3745 × 10−5 + 179.9686 V − 0.0239 V2 | χ = 27452.28 − 13.13 V + 0.0016 V2 |
| σL = −2371.44 + 0.7080649 V | σL = −28663.05 + 12.7609 R − 0.0014 R2 |
| σd = 535.56 − 0.744254329 A | σd = −2.276 × 10−5 + 95.7175 R − 0.01 R2 |
| a1 = 2.04 − 0.0049163803 MNI | a1 = 0.52 − 3.1536 Katt − 64.692 Katt2, if Katt < 0; a1 = 0.78 − 2.46726 × Katt, if Katt > 0 |
| a2 = 2.25 − 0.005016609 MNI | a2 = 0.75 − 4.355 Katt − 95.4879 Katt2, if Katt < 0; a2 = 0.94 − 3.341855 × Katt, if Katt > 0 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ababkov, N.; Smirnov, A.; Danilov, V.; Zuev, L.; Popova, N.; Nikonenko, E. Structural-Phase State, Mechanical Properties, Acoustic and Magnetic Characteristics in the Sustainable Deformation Localization Zones of Power Equipment Made of Structural and Heat Resistant Steels. Metals 2021, 11, 1638. https://doi.org/10.3390/met11101638
Ababkov N, Smirnov A, Danilov V, Zuev L, Popova N, Nikonenko E. Structural-Phase State, Mechanical Properties, Acoustic and Magnetic Characteristics in the Sustainable Deformation Localization Zones of Power Equipment Made of Structural and Heat Resistant Steels. Metals. 2021; 11(10):1638. https://doi.org/10.3390/met11101638
Chicago/Turabian StyleAbabkov, Nikolay, Alexandr Smirnov, Vladimir Danilov, Lev Zuev, Natalya Popova, and Elena Nikonenko. 2021. "Structural-Phase State, Mechanical Properties, Acoustic and Magnetic Characteristics in the Sustainable Deformation Localization Zones of Power Equipment Made of Structural and Heat Resistant Steels" Metals 11, no. 10: 1638. https://doi.org/10.3390/met11101638
APA StyleAbabkov, N., Smirnov, A., Danilov, V., Zuev, L., Popova, N., & Nikonenko, E. (2021). Structural-Phase State, Mechanical Properties, Acoustic and Magnetic Characteristics in the Sustainable Deformation Localization Zones of Power Equipment Made of Structural and Heat Resistant Steels. Metals, 11(10), 1638. https://doi.org/10.3390/met11101638








