Deformation Mechanisms of FCC-Structured Metallic Nanocrystal with Incoherent Twin Boundary
Abstract
:1. Introduction
2. Simulation Method
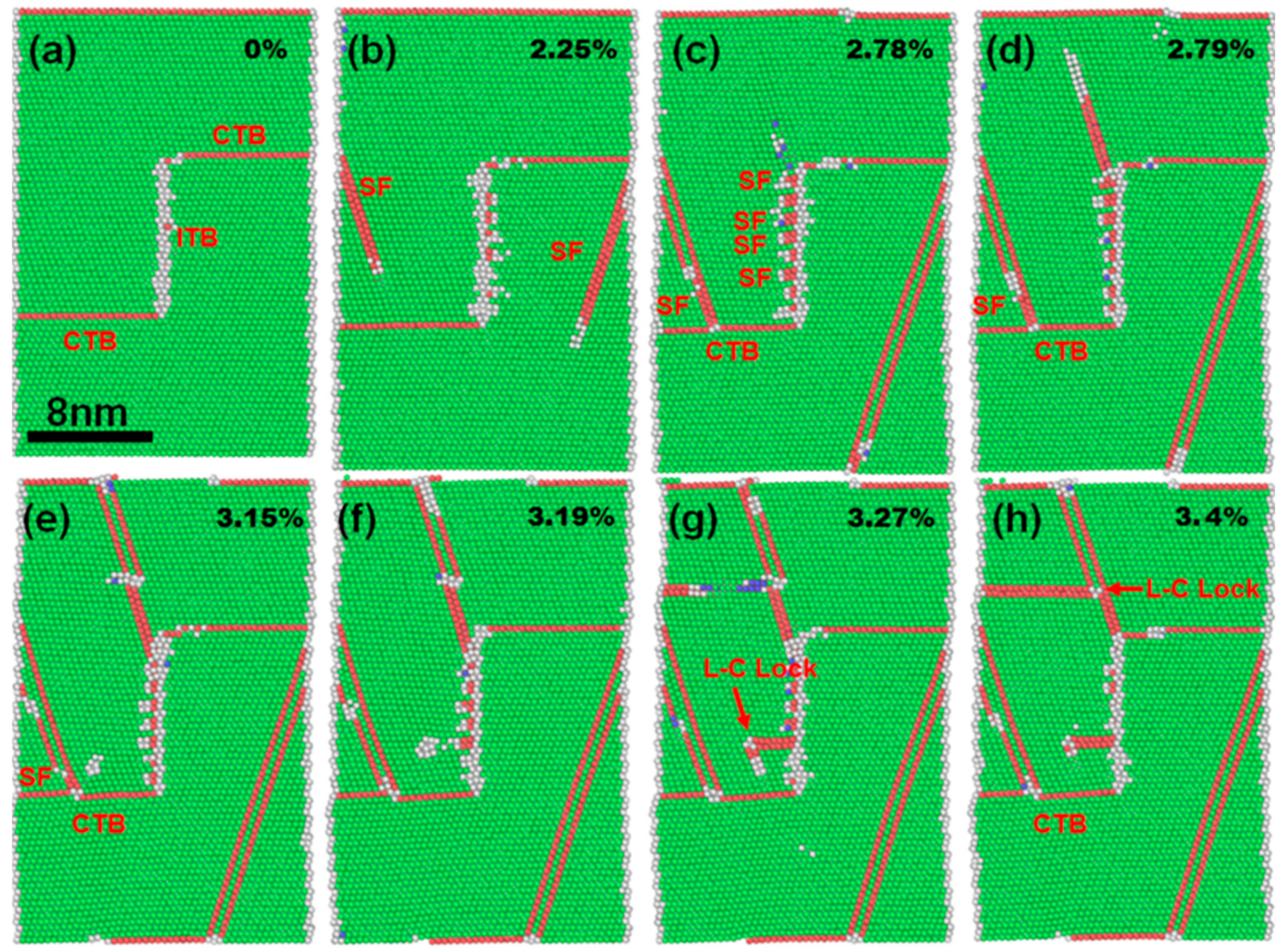
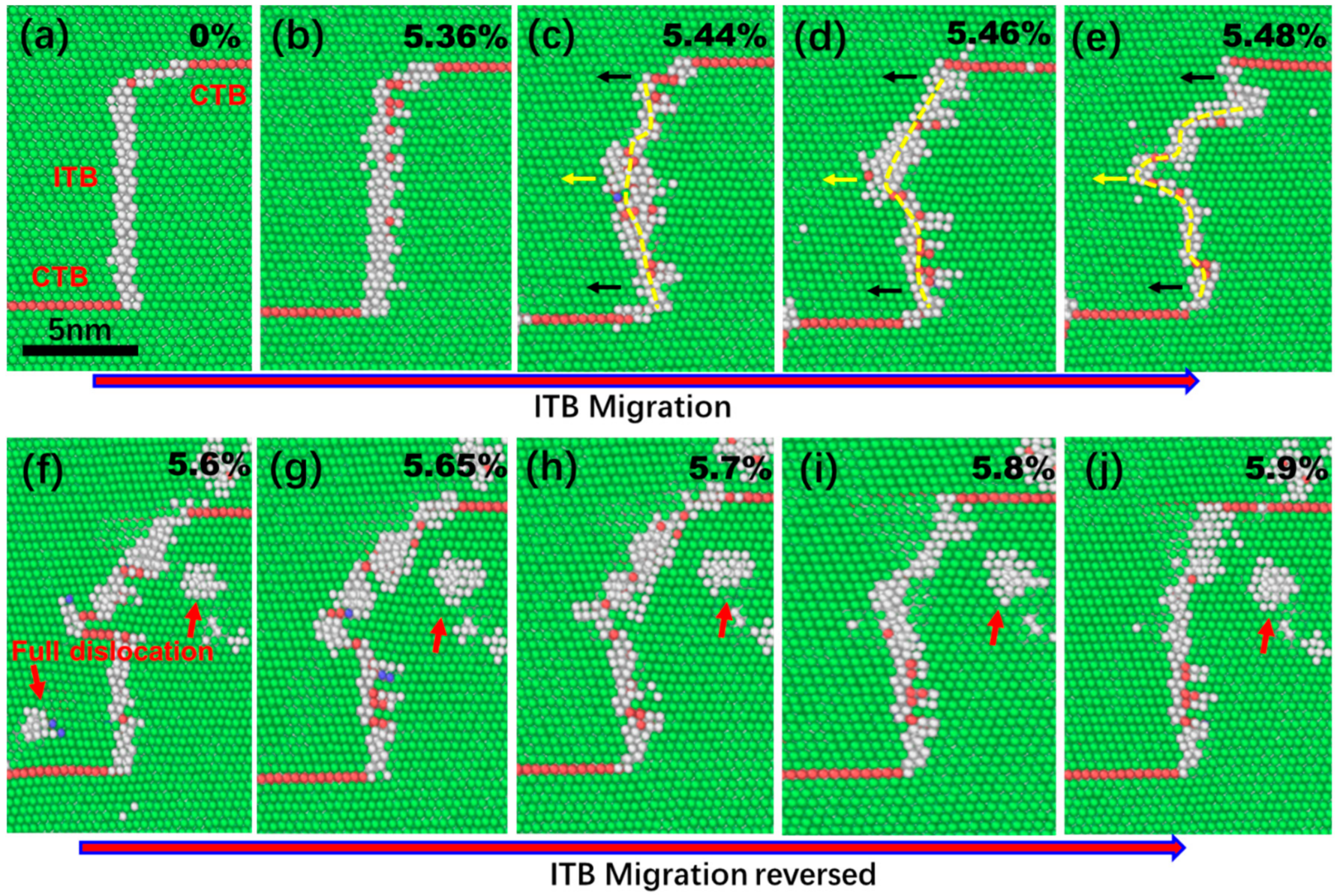
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Lu, K.; Lu, L.; Suresh, S. Strengthening materials by engineering coherent internal boundaries at the nanoscale. Science 2009, 324, 349–352. [Google Scholar] [CrossRef] [Green Version]
- Jang, D.C.; Li, X.Y.; Gao, H.J.; Greer, J.R. Deformation mechanisms in nanotwinned metal nanopillars. Nat. Nanotechnol. 2012, 7, 594–601. [Google Scholar] [CrossRef]
- Wang, J.W.; Zeng, Z.; Weinberger, C.R.; Zhang, Z.; Zhu, T.; Mao, S.X. In situ atomic-scale observation of twinning-dominated deformation in nanoscale body-centred cubic tungsten. Nat. Mater. 2015, 14, 594–600. [Google Scholar] [CrossRef]
- Pan, Q.S.; Zhou, H.F.; Lu, Q.H.; Gao, H.J.; Lu, L. History-independent cyclic response of nanotwinned metals. Nature 2017, 551, 214–217. [Google Scholar] [CrossRef]
- Cheng, Z.; Zhou, H.F.; Lu, Q.H.; Gao, H.J.; Lu, L. Extra strengthening and work hardening in gradient nanotwinned metals. Science 2018, 362, eaau1925. [Google Scholar] [CrossRef] [Green Version]
- Wang, L.H.; Du, K.; Yang, C.P.; Teng, J.; Fu, L.B.; Guo, Y.Z.; Zhang, Z.; Han, X.D. In situ atomic-scale observation of grain size and twin thickness effect limit in twin-structural nanocrystalline platinum. Nat. Commun. 2020, 11, 1167. [Google Scholar] [CrossRef] [Green Version]
- Lu, K. Stabilizing nanostructures in metals using grain and twin boundary architectures. Nat. Rev. Mater. 2016, 1, 16019. [Google Scholar] [CrossRef]
- Lu, N.; Du, K.; Lu, L.; Ye, H.Q. Transition of dislocation nucleation induced by local stress concentration in nanotwinned copper. Nat. Commun. 2015, 6, 7648. [Google Scholar] [CrossRef] [Green Version]
- Zhu, Y.T.; Liao, X.Z.; Wu, X.L. Deformation twinning in nanocrystalline materials. Prog. Mater. Sci. 2012, 57, 1–62. [Google Scholar] [CrossRef] [Green Version]
- Ovid’ko, I.A.; Valiev, R.Z.; Zhu, Y.T. Review on superior strength and enhanced ductility of metallic nanomaterial. Prog. Mater. Sci. 2018, 94, 462–540. [Google Scholar] [CrossRef]
- Wang, L.H.; Han, X.D.; Zhang, Z. In situ experimental mechanics of nanomaterials at the atomic scale. NPG Asia Mater. 2013, 5, e40. [Google Scholar] [CrossRef] [Green Version]
- Lu, Y.; Sun, S.; Zeng, Y.; Deng, Q.; Han, X. Atomistic mechanism of nucleation and growth of a face-centered orthogonal phase in small-sized single-crystalline mo. Mater. Res. Lett. 2020, 8, 348–355. [Google Scholar] [CrossRef]
- Oliveira, J.P.; Curado, T.M.; Zeng, Z.; Lopes, J.G.; Rossinyol, E.; Park, J.M.; Schell, N.; Fernandes, F.M.B.; Kim, H.S. Gas tungsten arc welding of as-rolled CrMnFeCoNi high entropy alloy. Mater. Des. 2020, 189, 108505. [Google Scholar] [CrossRef]
- Oliveira, J.P.; Shen, J.J.; Zeng, Z.; Park, J.M.; Choi, Y.T.; Schell, N.; Maawad, E.; Zhou, N.; Kim, H.S. Dissimilar laser welding of a CoCrFeMnNi high entropy alloy to 316 stainless steel. Scr. Mater. 2022, 206, 114219. [Google Scholar] [CrossRef]
- Lu, Y.; Shu, X.Y.; Li, Z.P.; Long, H.B.; Kong, D.L.; Sun, S.D.; Deng, Q.S.; Chen, Y.H.; Hu, Z.W.; Wang, L.H.; et al. In situ investigation of synchronized dislocation array nucleation and phase transformation at mode I-II cracks of single-crystalline mo. J. Alloys Compd. 2019, 806, 283–291. [Google Scholar] [CrossRef]
- Beyerlein, I.J.; Zhang, X.H.; Misra, A. Growth Twins and Deformation Twins in Metals. Annu. Rev. Mater. Res. 2014, 44, 329–363. [Google Scholar] [CrossRef]
- Zeng, Z.; Li, X.Y.; Lu, L.; Zhu, T. Fracture in a thin film of nanotwinned copper. Acta Mater. 2015, 98, 313–317. [Google Scholar] [CrossRef] [Green Version]
- Lee, S.; Im, J.; Yoo, Y.; Bitzek, E.; Kiener, D.; Richter, G.; Kim, B.; Oh, S.H. Reversible cyclic deformation mechanism of gold nanowires by twinning–detwinning transition evidenced from in situ TEM. Nat. Commun. 2014, 5, 3033. [Google Scholar] [CrossRef] [Green Version]
- Xiong, L.; You, Z.S.; Lu, L. Fracture behavior of an austenitic stainless steel with nanoscale deformation twins. Scr. Mater. 2017, 127, 173–177. [Google Scholar] [CrossRef] [Green Version]
- Sun, J.P.; Li, C.; Han, J.; Shao, X.Y.; Yang, X.W.; Liu, H.; Song, D.; Ma, A.B. Size effect and deformation mechanism in twinned copper nanowires. Metals 2017, 7, 438. [Google Scholar] [CrossRef] [Green Version]
- Han, J.; Sun, J.P.; Han, Y.; Zhu, H.; Fang, L. Strengthening versus softening of nanotwinned copper depending on prestress and twin spacing. Metals 2018, 8, 344. [Google Scholar] [CrossRef] [Green Version]
- An, X.L.; Ni, S.; Song, M.; Liao, X.Z. Deformation twinning and detwinning in face-centered cubic metallic materials. Adv. Eng. Mater. 2020, 22, 1900479. [Google Scholar] [CrossRef]
- Jiang, Y.Q.; Wang, B.J.; Xu, C.; Zhang, J.G. Atomistic simulation of the strain driven phase transition in pure iron thin films containing twin boundaries. Metals 2020, 10, 953. [Google Scholar] [CrossRef]
- You, Z.; Lu, L. Effect of strain rate on tensile ductility and fracture behavior of bulk nanotwinned copper. Adv. Eng. Mater. 2016, 17, 1754–1759. [Google Scholar] [CrossRef]
- Li, X.Y.; Lu, L.; Li, J.G.; Zhang, X.; Gao, H.J. Mechanical properties and deformation mechanisms of gradient nanostructured metals and alloys. Nat. Rev. Mater. 2020, 5, 706–723. [Google Scholar] [CrossRef]
- Cao, Y.; Wang, Y.B.; An, X.H.; Liao, X.Z.; Kawasaki, M.; Ringer, S.P.; Langdon, T.G.; Zhu, Y.T. Grain boundary formation by remnant dislocations from the de-twinning of thin nano-twins. Scr. Mater. 2015, 100, 98–101. [Google Scholar] [CrossRef]
- Sun, L.G.; He, X.Q.; Lu, J. Nanotwinned and hierarchical nanotwinned metals: A review of experimental, computational and theoretical efforts. npj Comput. Mater. 2018, 4, 6. [Google Scholar] [CrossRef]
- You, Z.S.; Lu, L. Deformation and fracture mechanisms of nanotwinned metals. Natl. Sci. Rev. 2017, 4, 519–521. [Google Scholar] [CrossRef] [Green Version]
- Ni, S.; Wang, Y.B.; Liao, X.Z.; Figueiredo, R.B.; Li, H.Q.; Ringer, S.P.; Langdon, T.G.; Zhu, Y.T. The effect of dislocation density on the interactions between dislocations and twin boundaries in nanocrystalline materials. Acta Mater. 2012, 60, 3181–3189. [Google Scholar] [CrossRef]
- Li, N.; Wang, J.; Huang, J.Y.; Misra, A.; Zhang, X. Influence of slip transmission on the migration of incoherent twin boundaries in epitaxial nanotwinned Cu. Scr. Mater. 2011, 64, 149–152. [Google Scholar] [CrossRef]
- Wang, J.; Li, N.; Anderoglu, O.; Zhang, X.; Misra, A.; Huang, J.Y.; Hirth, J.P. Detwinning mechanisms for growth twins in face-centered cubic metals. Acta Mater. 2010, 58, 2262–2270. [Google Scholar] [CrossRef]
- Wang, L.H.; Teng, J.; Kong, D.L.; Yu, G.H.; Zou, J.; Zhang, Z.; Han, X.D. In situ atomistic deformation mechanisms of twin-structured nanocrystal Pt. Scr. Mater. 2018, 147, 103–107. [Google Scholar] [CrossRef]
- Lu, Y. Shu, X., Liao, X. Size effect for achieving high mechanical performance body-centered cubic metals and alloys. Sci. China Mater. 2018, 61, 1495–1516. [Google Scholar] [CrossRef] [Green Version]
- Cao, Y.; Wang, Y.B.; Chen, Z.B.; Liao, X.Z.; Kawasaki, M.; Ringer, S.P.; Langdon, T.G.; Zhu, Y.T. Detwinning via secondary twinning in face-centered cubic alloys. Mater. Sci. Eng. A 2013, 578, 110–114. [Google Scholar] [CrossRef]
- Li, N.; Wang, J.; Misra, A.; Hang, Z.X.; Hirth, J.P. Twinning dislocation multiplication at a coherent twin boundary. Acta Mater. 2011, 59, 5989–5996. [Google Scholar] [CrossRef]
- Bufford, D.C.; Wang, Y.M.; Liu, Y.; Lu, L. Synthesis and microstructure of electrodeposited and sputtered nanotwinned face-centered-cubic metals. MRS Bull. 2016, 41, 286–291. [Google Scholar] [CrossRef] [Green Version]
- Medlin, D.L.; Campbell, G.H.; Carter, C.B. Stacking defects in the 9R phase at an incoherent twin boundary in copper. Acta Mater. 1998, 46, 5135–5142. [Google Scholar] [CrossRef]
- Mishin, Y.; Mehl, M.J.; Papaconstantopoulos, D.A.; Voter, A.F.; Kress, J.D. Structural stability and lattice defects in copper: Ab initio, tight-binding, and embedded-atom calculations. Phys. Rev. B 2001, 63, 224106. [Google Scholar] [CrossRef] [Green Version]
- Plimpton, S. Fast parallel algorithms for short-range molecular-dynamics. J. Comput. Phys. 1995, 117, 1–19. [Google Scholar] [CrossRef] [Green Version]
- Mishin, Y.; Farkas, D.; Mehl, M.J.; Papaconstantopoulos, D.A. Interatomic potentials for monoatomic metals from experimental data and ab initio calculations. Phys. Rev. B 1999, 59, 3393. [Google Scholar] [CrossRef] [Green Version]
- Ackland, G.J.; Tichy, G.; Vitek, V.; Finnis, M.W. Simple n-body potentials for the noble-metals and nickel. Philos. Mag. A 1987, 56, 735–756. [Google Scholar] [CrossRef]
- Grochola, G.; Russo, S.P.; Snook, I.K. On fitting a gold embedded atom method potential using the force matching method. J. Chem. Phys. 2005, 123, 204719. [Google Scholar] [CrossRef]
- Ackland, G.J.; Jones, A.P. Applications of local crystal structure measures in experiment and simulation. Phys. Rev. B 2006, 73, 4104. [Google Scholar] [CrossRef]
- Stukowski, A. Visualization and analysis of atomistic simulation data with OVITO-the Open Visualization Tool. Model. Simul. Mater. Sci. Eng. 2010, 1, 2154–2162. [Google Scholar] [CrossRef]
- Lu, Y.; Xiang, S.S.; Xiao, L.R.; Wang, L.H.; Deng, Q.S.; Zhang, Z.; Han, X.D. Dislocation “bubble-like-effect” and the ambient temperature super-plastic elongation of body-centred cubic single crystalline molybdenum. Sci. Rep. 2016, 6, 22937. [Google Scholar] [CrossRef] [Green Version]
- Fu, L.B.; Yang, C.P.; Wei, R.J.; Pei, X.F.; Teng, J.; Kong, D.L.; Lu, Y.; Guo, Y.Z.; Liu, T.F.; Hu, Y.L.; et al. In situ atomic-scale observation of AuCu alloy nanowire with superplasticity and high strength at room temperature. Mater. Today Nano 2021, 15, 100123. [Google Scholar] [CrossRef]
- Shin, Y.A.; Yin, S.; Li, X. Nanotwin-governed toughening mechanism in hierarchically structured biological materials. Nat. Commun. 2016, 7, 10772. [Google Scholar] [CrossRef]
- Lu, Q.H.; You, Z.S.; Huang, X.X.; Hansen, N.; Lu, L. Dependence of dislocation structure on orientation and slip systems in highly oriented nanotwinned Cu. Acta Mater. 2017, 127, 85–97. [Google Scholar] [CrossRef]
- Wang, Y.M.; Sansoz, F.; Lagrange, T.; Ott, R.T.; Marian, J.T.W.B., Jr.; Hamza, A.V. Defective twin boundaries in nanotwinned metals. Nat. Mater. 2013, 12, 697–702. [Google Scholar] [CrossRef]
- Zhou, H.; Li, X.; Qu, S.; Yang, W.; Gao, H. A jogged dislocation governed strengthening mechanism in nanotwinned metals. Nano Lett. 2014, 14, 5075–5080. [Google Scholar] [CrossRef]
- Wu, X.L.; Zhu, Y.T.; Wei, Y.G.; Wei, Q. Strong Strain Hardening in Nanocrystalline Nickel. Phys. Rev. Lett. 2009, 103, 205504. [Google Scholar] [CrossRef]
- Bulatov, V.V.; Abraham, F.F.; Kubin, L.P.; Devincre, B.; Yip, S. Connecting atomistic and mesoscale simulations of crystal plasticity. Nature 1998, 391, 669–672. [Google Scholar] [CrossRef]
- Madec, R.; Devincre, B.; Kubin, L.; Hoc, T.; Rodney, D. The Role of Collinear Interaction in Dislocation-Induced Hardening. Science 2003, 301, 1879–1882. [Google Scholar] [CrossRef]
- Lee, J.H.; Holland, T.B.; Mukherjee, A.K.; Zhang, X.H.; Wang, H.Y. Direct observation of Lomer-Cottrell locks during strain hardening in nanocrystalline nickel by in situ TEM. Sci. Rep. 2013, 3, 1061. [Google Scholar] [CrossRef] [Green Version]
- Wang, L.H.; Teng, J.; Sha, X.C.; Zou, J.; Zhang, Z.; Han, X.D. Plastic deformation through dislocation saturation in ultrasmall Pt nanocrystals and its in situ atomistic mechanisms. Nano Lett. 2017, 17, 4733–4739. [Google Scholar] [CrossRef]
- Fu, L.B.; Kong, D.L.; Yang, C.P.; Teng, J.; Lu, Y.; Guo, Y.Z.; Yang, G.; Yan, X.; Liu, P.; Chen, M.W.; et al. Ultra-high strength yet superplasticity in a hetero-grain-sized nanocrystalline Au nanowire. J. Mater. Sci. Technol. 2022, 101, 95–106. [Google Scholar] [CrossRef]
- Fu, L.B.; Yang, C.P.; Lu, Y.; Teng, J.; Kong, D.L.; Guo, Y.Z.; Zhang, Z.; Wang, L.H.; Han, X.D. In situ atomistic mechanisms of detwinning in nanocrystalline AuAg Alloy. Sci. China Mater. 2021. [Google Scholar] [CrossRef]
- Wang, J.; Misra, A.; Hirth, J.P. Shear response of Σ3{112} twin boundaries in face-centered-cubic metals. Phys. Rev. B 2011, 83, 064106. [Google Scholar] [CrossRef]
- Liu, L.; Wang, J.; Gong, S.K.; Mao, S.X. High resolution transmission electron microscope observation of zero-strain deformation twinning mechanisms in Ag. Phys. Rev. Lett. 2011, 106, 175504. [Google Scholar] [CrossRef]
- Wang, R.; Chen, X.; Huang, Z.Y.; Yang, J.L.; Liu, F.S.; Chu, M.H.; Liu, T.C.; Wang, C.Q.; Zhu, W.M.; Li, S.K.; et al. Twin boundary defect engineering improves lithium-ion diffusion for fast-charging spinel cathode materials. Nat. Commun. 2021, 12, 3085. [Google Scholar] [CrossRef]
- Moriwake, H.; Kuwabara, A.; Fisher, C.A.J.; Huang, R.; Hitosugi, T.; Ikuhara, Y.H.; Oki, H.; Ikuhara, Y. First-principles calculations of lithium-ion migration at a coherent grain boundary in a cathode material, LiCoO2. Adv. Mater. 2013, 25, 618–622. [Google Scholar] [CrossRef]
- Hristu, R.; Stanciu, S.G.; Tranca, D.E.; Matei, A.; Stanciu, G.A. Nonlinear optical imaging of defects in cubic silicon carbide epilayers. Sci. Rep. 2014, 4, 5258. [Google Scholar] [CrossRef] [Green Version]
- Giannazzo, F.; Greco, G.; Franco, S.D.; Fiorenza, P.; Deretzis, I.; Magna, A.L.; Bongiorno, C.; Zimbone, M.; Via, F.L.; Zielinski, M.; et al. Impact of Stacking Faults and Domain Boundaries on the Electronic Transport in Cubic Silicon Carbide Probed by Conductive Atomic Force Microscopy. Adv. Electron. Mater. 2021, 6, 1901171. [Google Scholar] [CrossRef] [Green Version]
- Cleri, F.; Rosato, V. Tight binding potentials for transition metal alloys. Phys. Rev. B 1993, 48, 22–33. [Google Scholar] [CrossRef]
- Mishin, Y.; Farkas, D.; Mehl, M.J.; Papaconstantopoulos, D.A. Interatomic Potentials for Al and Ni From Experimental Data and ab Initio Calculations. MRS Proc. 1998, 538, 535–540. [Google Scholar] [CrossRef]
- Zimmerman, J.A.; Abraham, F.F.; Gao, H.J. Generalized stacking fault energies for embedded atom FCC metals. Model. Simul. Mater. Sci. Eng. 2000, 2, 103–115. [Google Scholar] [CrossRef]
- Swygenhoven, H.V.; Derlet, P.M.; Frøseth, A.G. Stacking fault energies and slip in nanocrystalline metals. Nat. Mater. 2004, 6, 399–403. [Google Scholar] [CrossRef]
- Bernstein, N.; Tadmor, E.B. Tight-binding calculations of stacking energies and twinnability in FCC metals. Phys. Rev. B 2004, 9, 50–55. [Google Scholar] [CrossRef]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tao, Y.; Zhao, Y.; Wang, Z.; Fu, L.; Wang, L. Deformation Mechanisms of FCC-Structured Metallic Nanocrystal with Incoherent Twin Boundary. Metals 2021, 11, 1672. https://doi.org/10.3390/met11111672
Tao Y, Zhao Y, Wang Z, Fu L, Wang L. Deformation Mechanisms of FCC-Structured Metallic Nanocrystal with Incoherent Twin Boundary. Metals. 2021; 11(11):1672. https://doi.org/10.3390/met11111672
Chicago/Turabian StyleTao, Yang, Yufeng Zhao, Zhanxin Wang, Libo Fu, and Lihua Wang. 2021. "Deformation Mechanisms of FCC-Structured Metallic Nanocrystal with Incoherent Twin Boundary" Metals 11, no. 11: 1672. https://doi.org/10.3390/met11111672
APA StyleTao, Y., Zhao, Y., Wang, Z., Fu, L., & Wang, L. (2021). Deformation Mechanisms of FCC-Structured Metallic Nanocrystal with Incoherent Twin Boundary. Metals, 11(11), 1672. https://doi.org/10.3390/met11111672





