Digitized Stress Function-Based Feed Rate Scheduling for Prevention of Mesoscale Tool Breakage during Milling Hardened Steel
Abstract
:1. Introduction
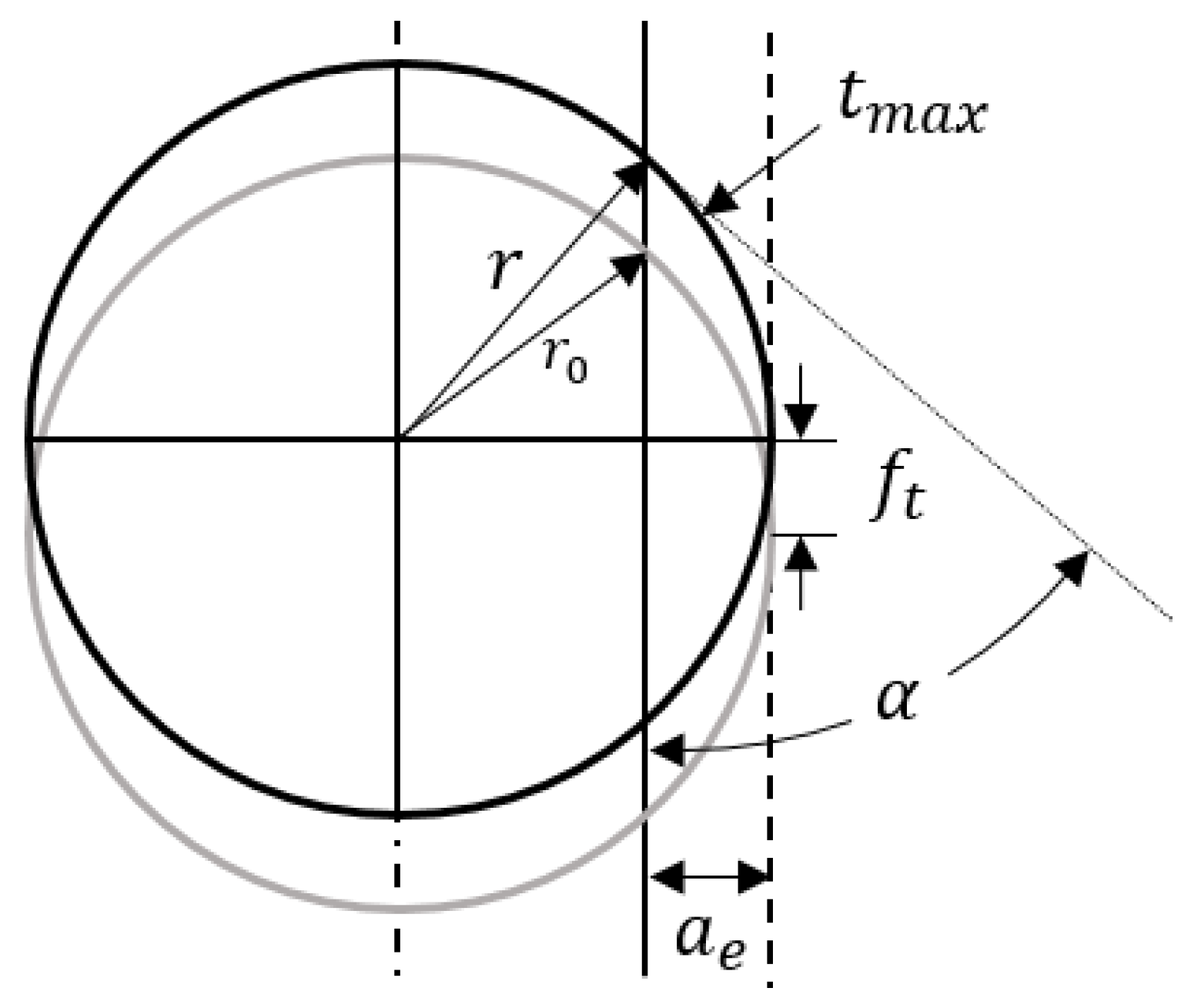
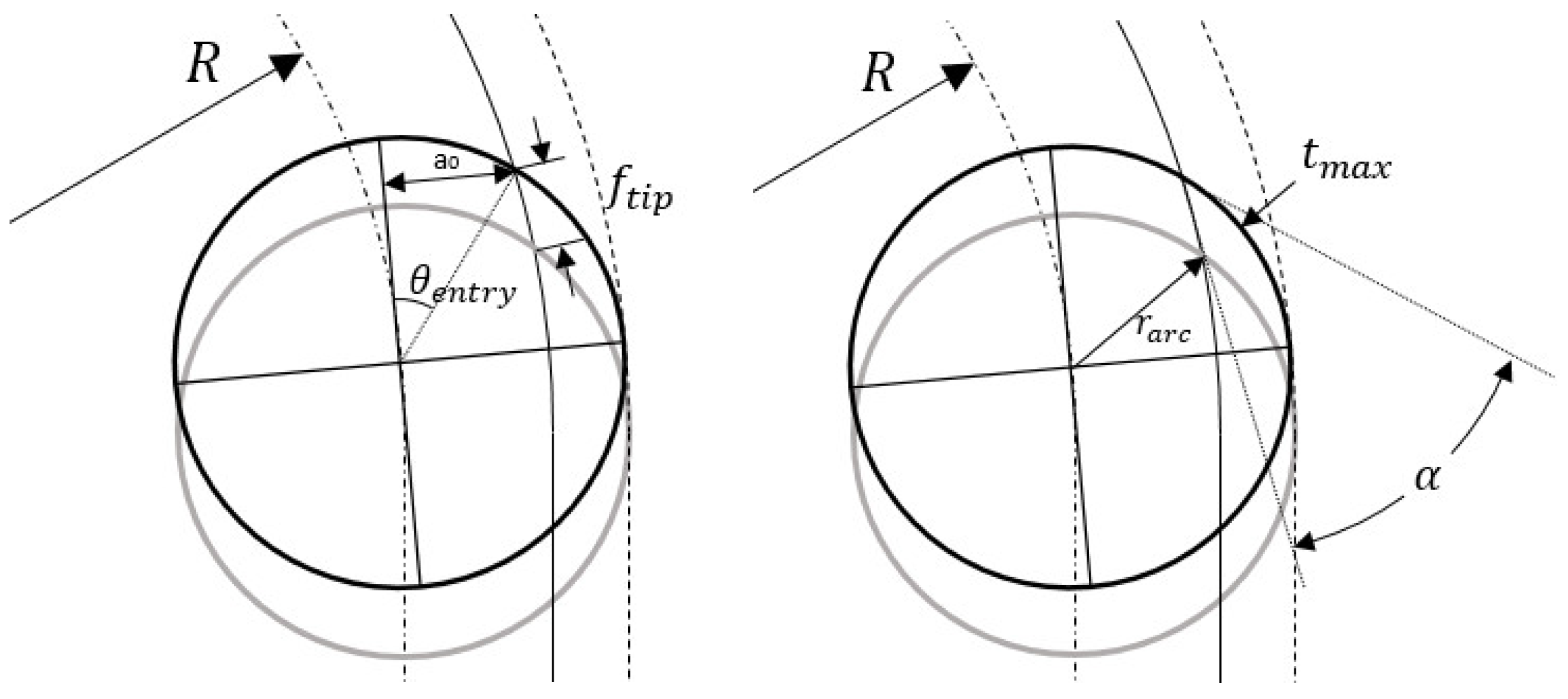
2. Evaluation of Maximum Thickness and Engaging Angle of the Uncut Chip for Tool Breakage Prediction
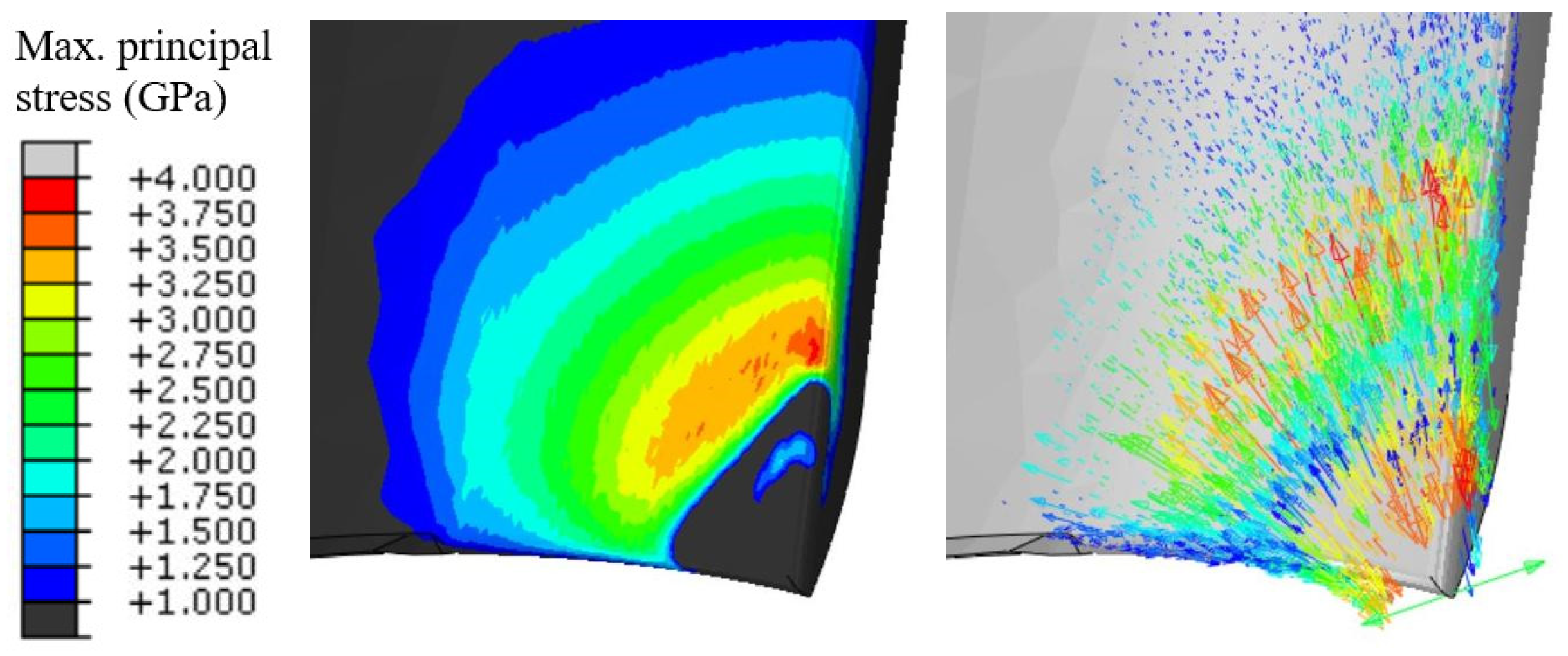
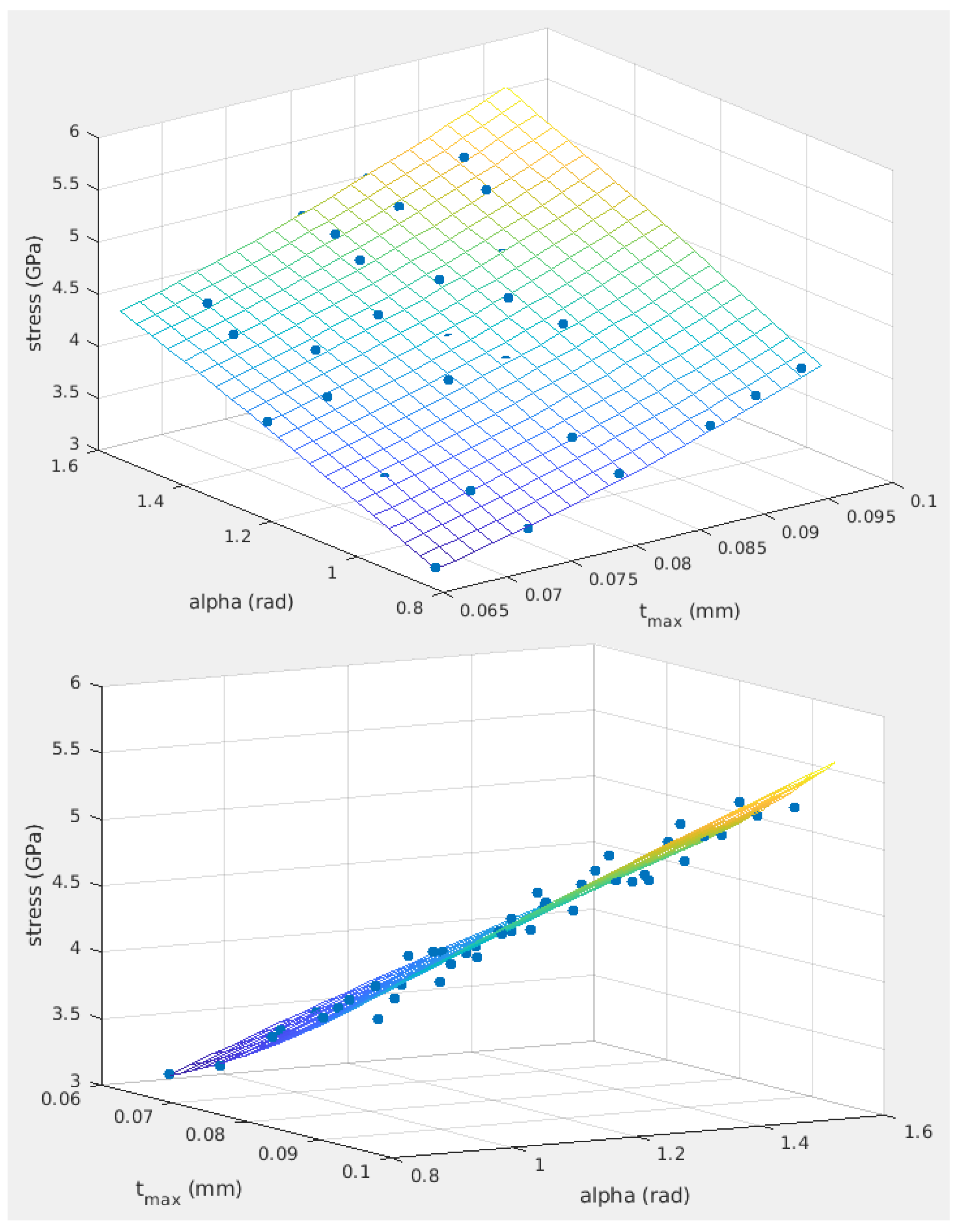
3. Prediction of the Maximum Tensile Stress in the Cutting Tool
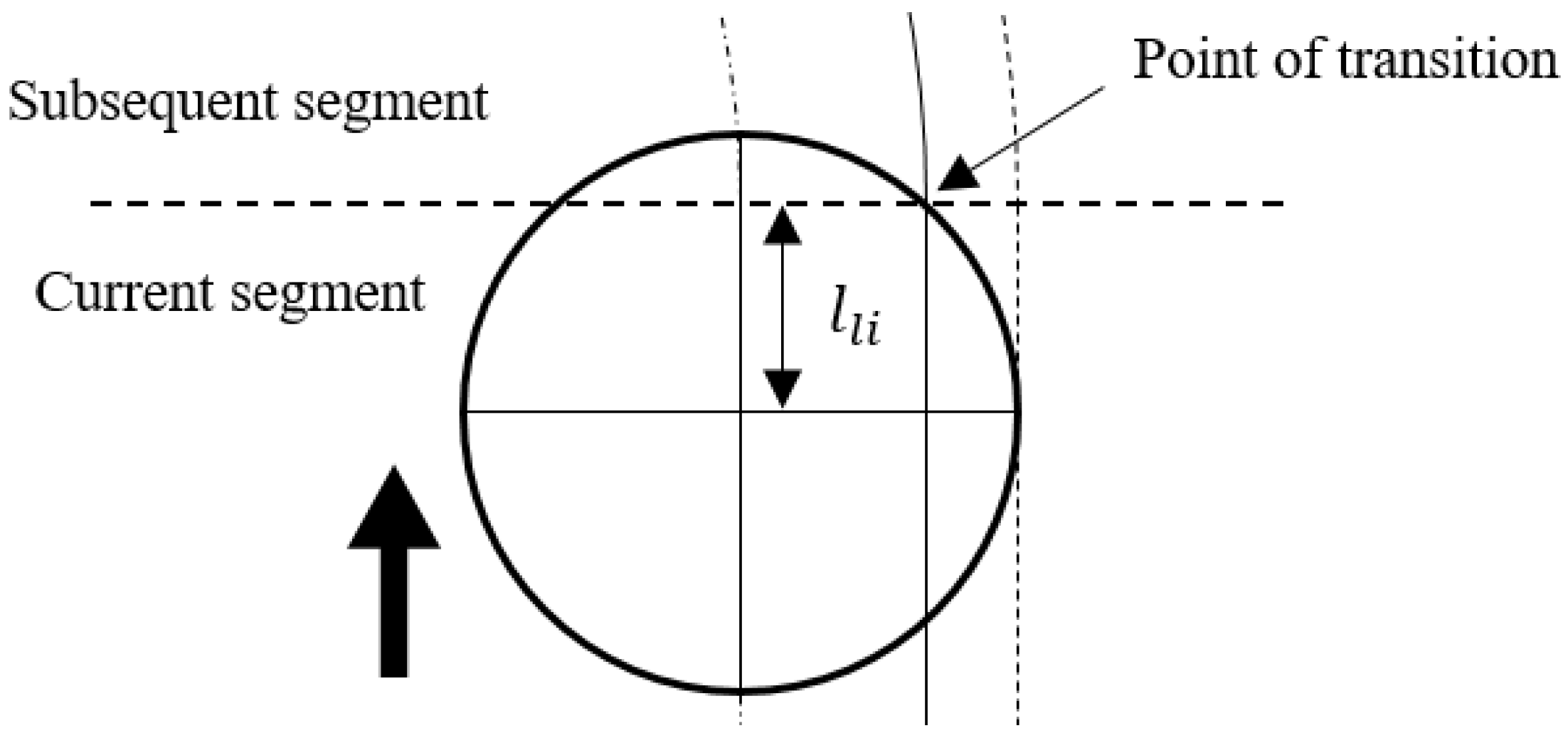
4. Feed Rate Scheduling Based on Digitized Maximum Tensile Stress Function and Experimental Validation
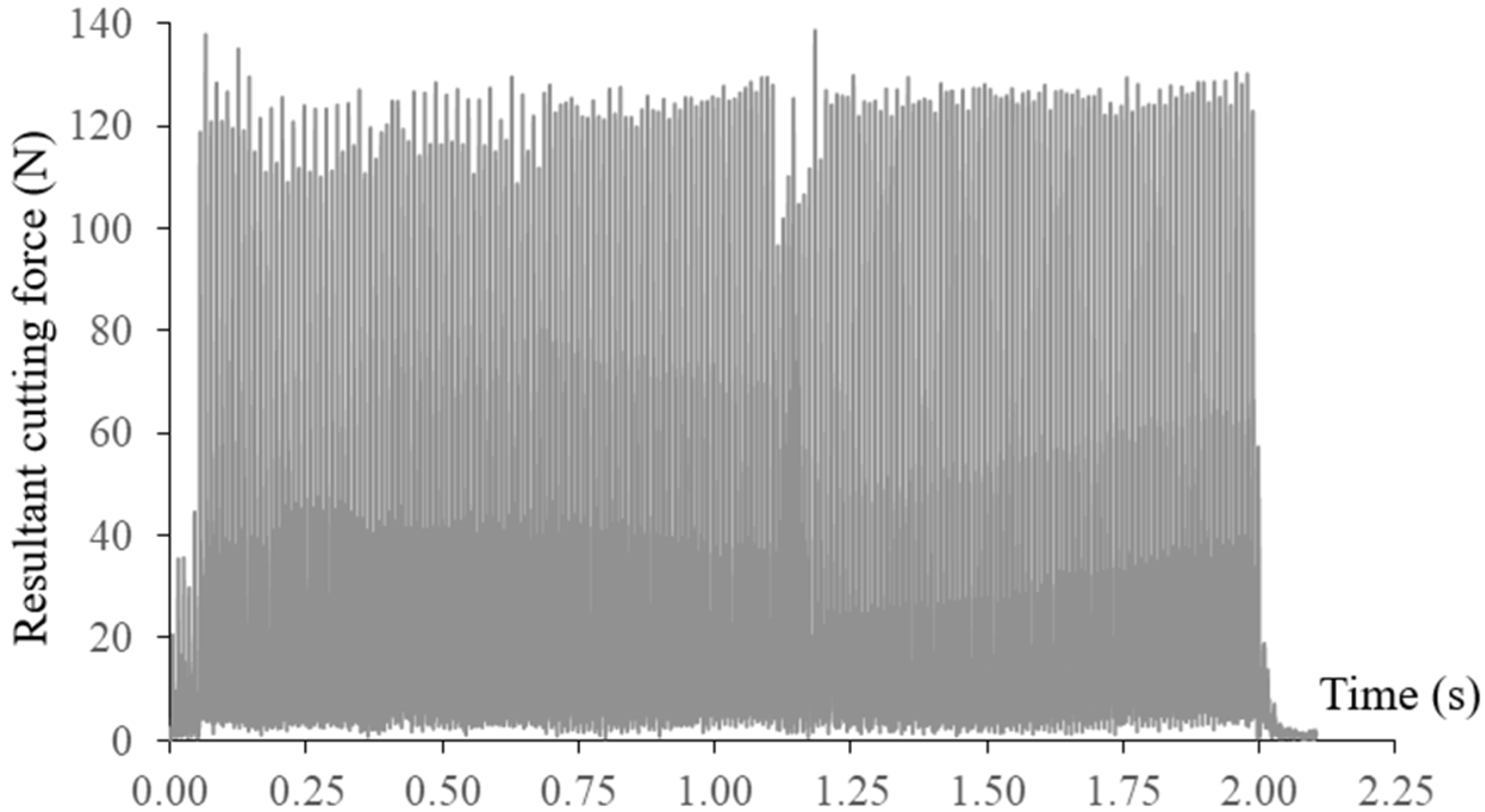
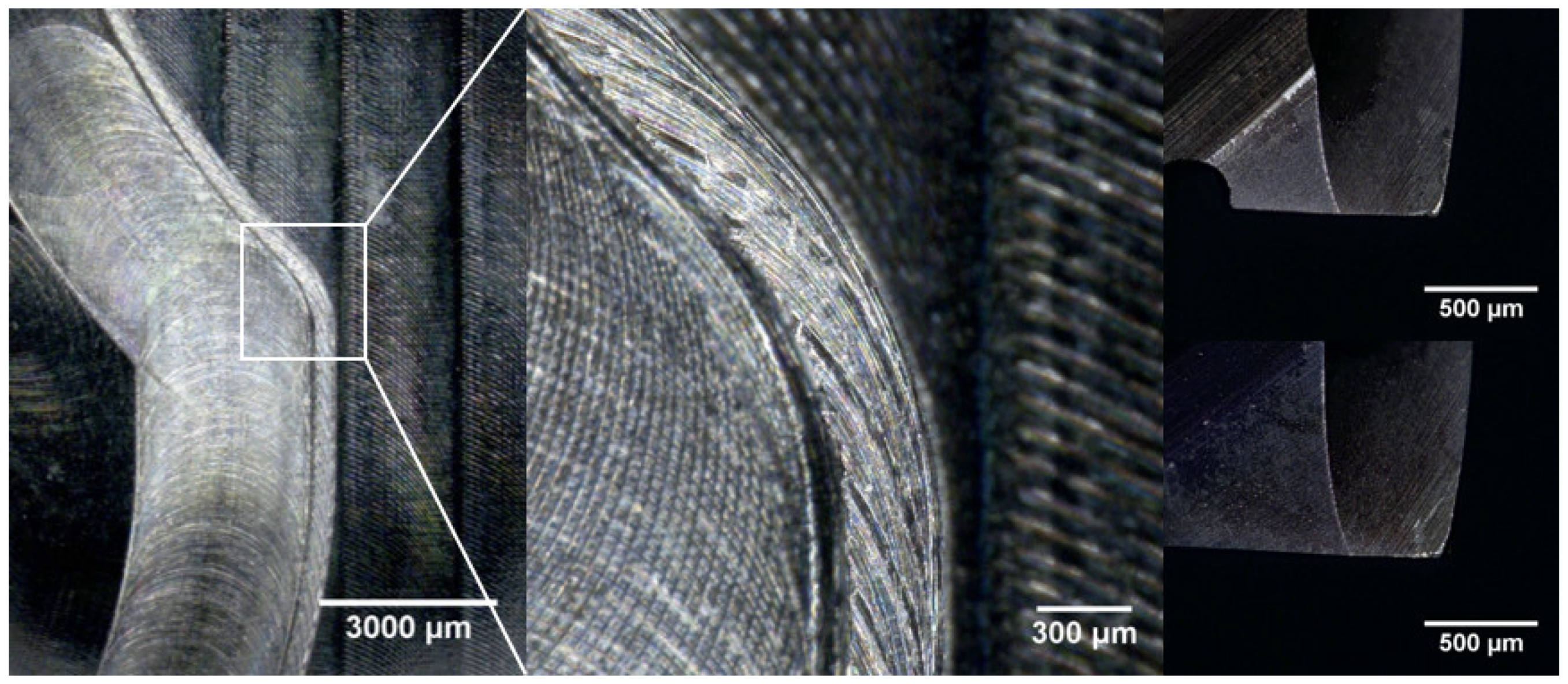
4.1. 1st Tool Path with Concave Curvature and Small Radial Cutting Depth
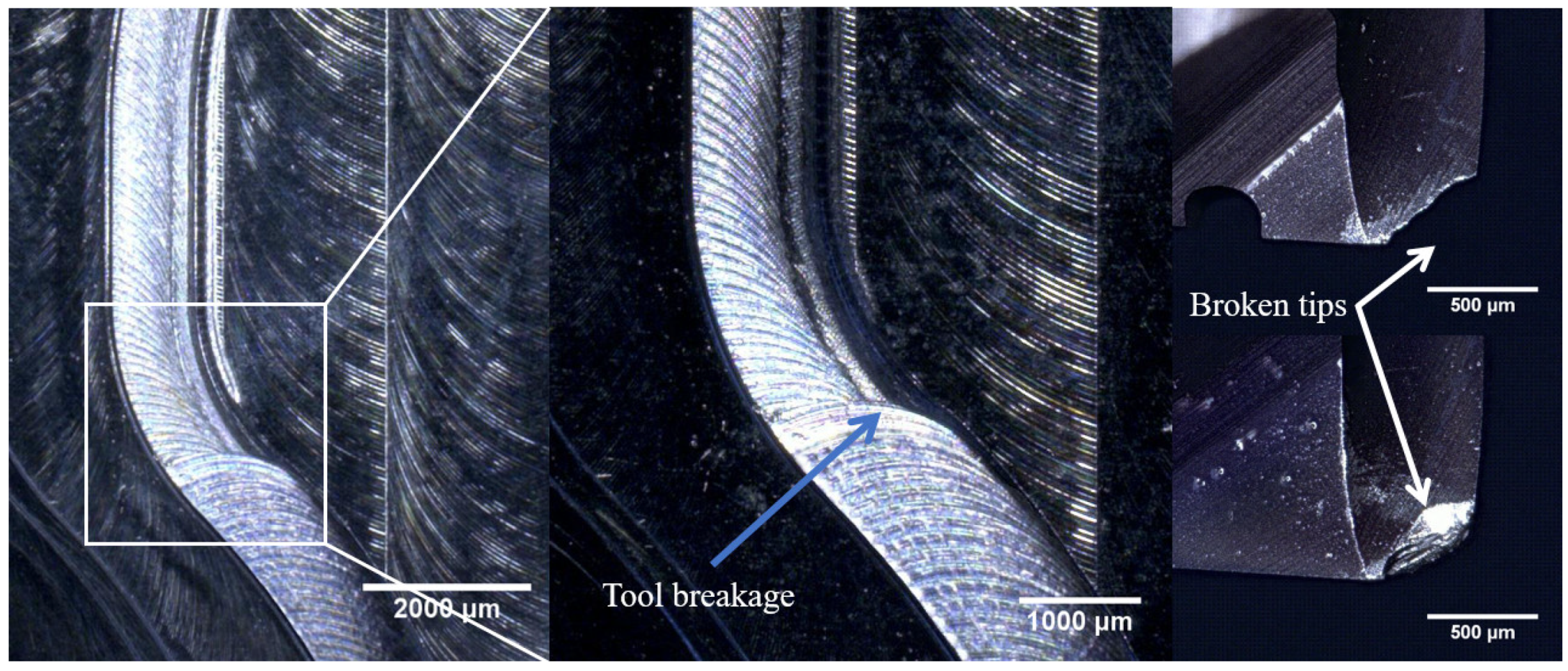
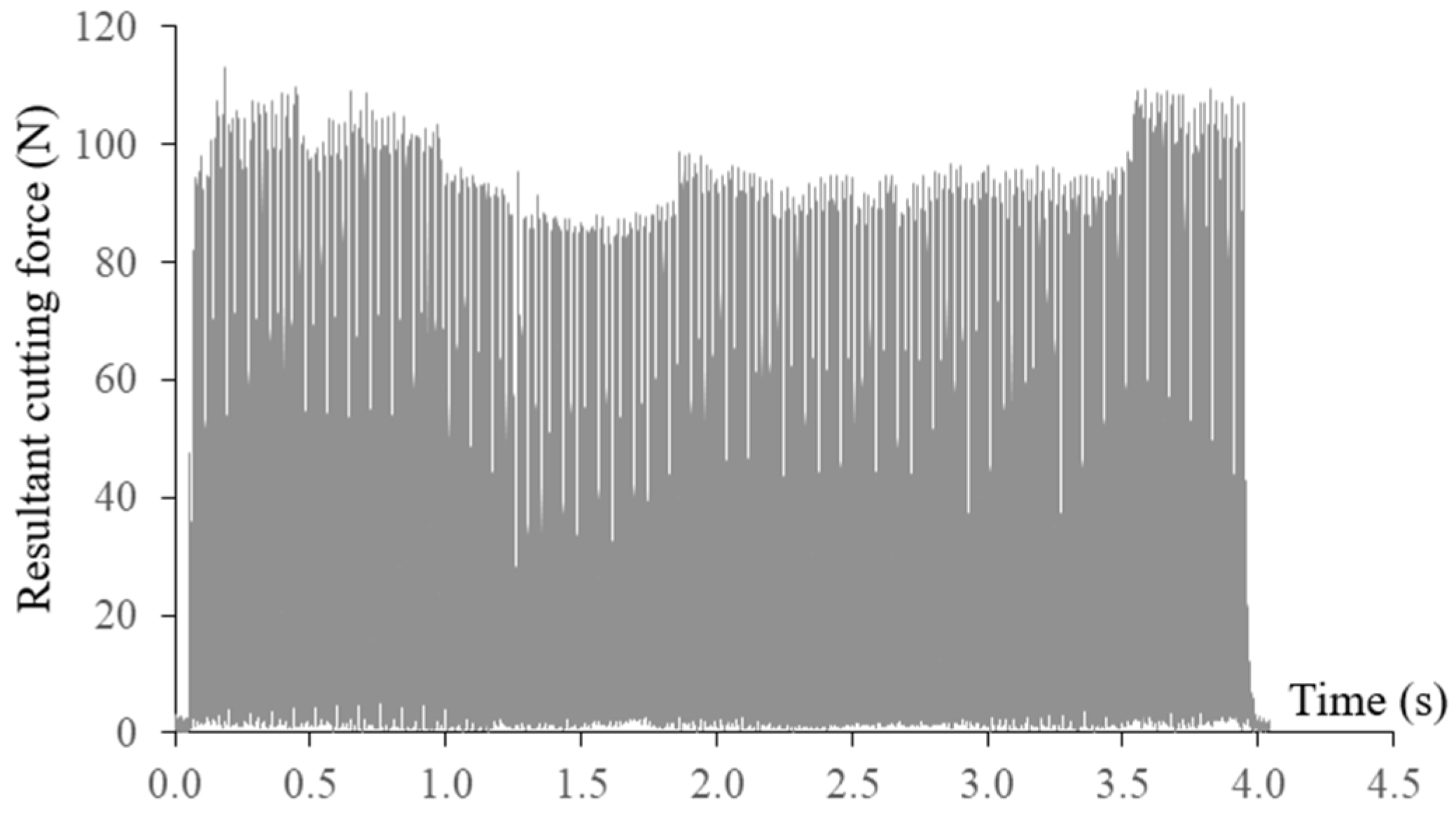
4.2. 2nd Tool Path with Convex Curvature and Large Radial Cutting Depth
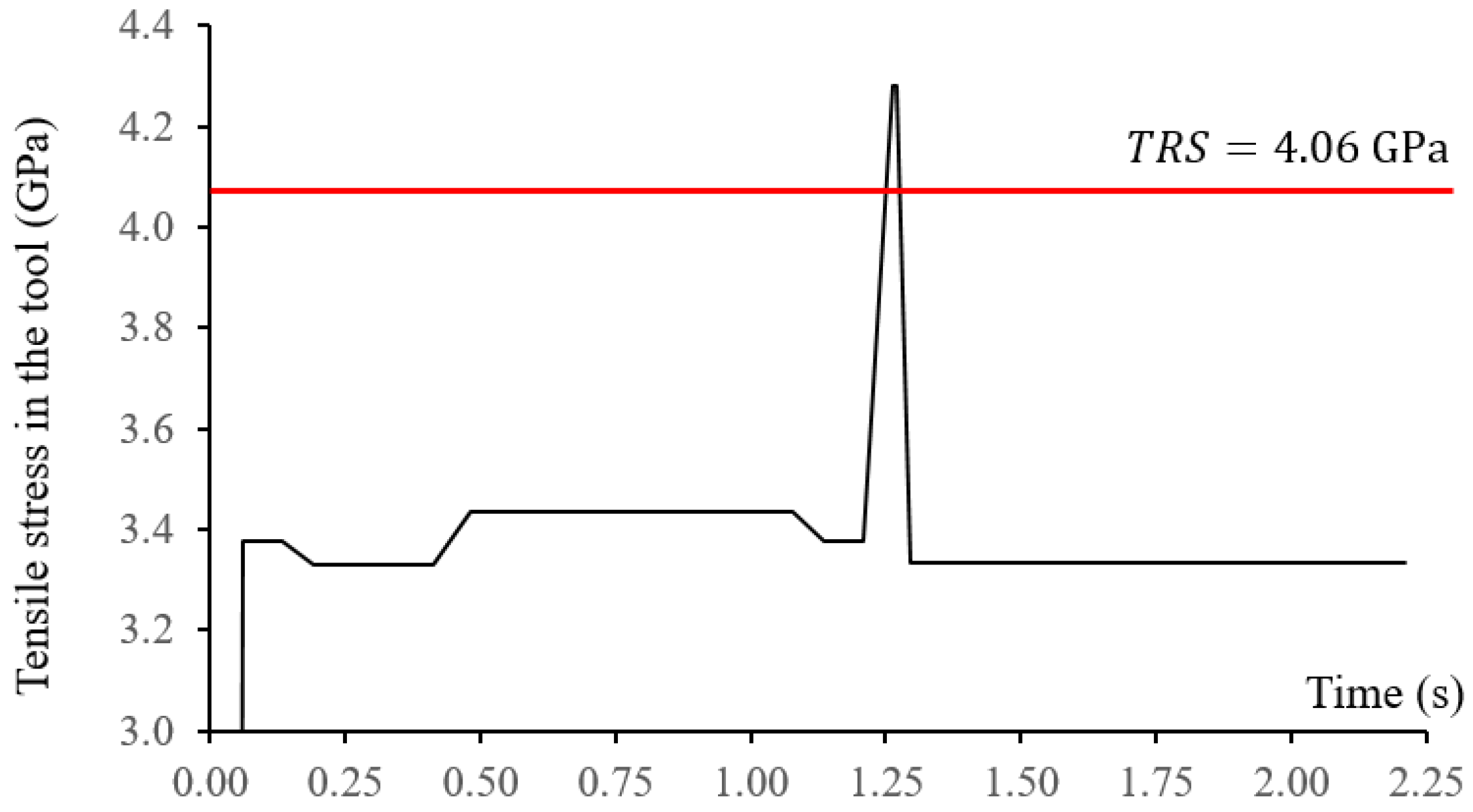
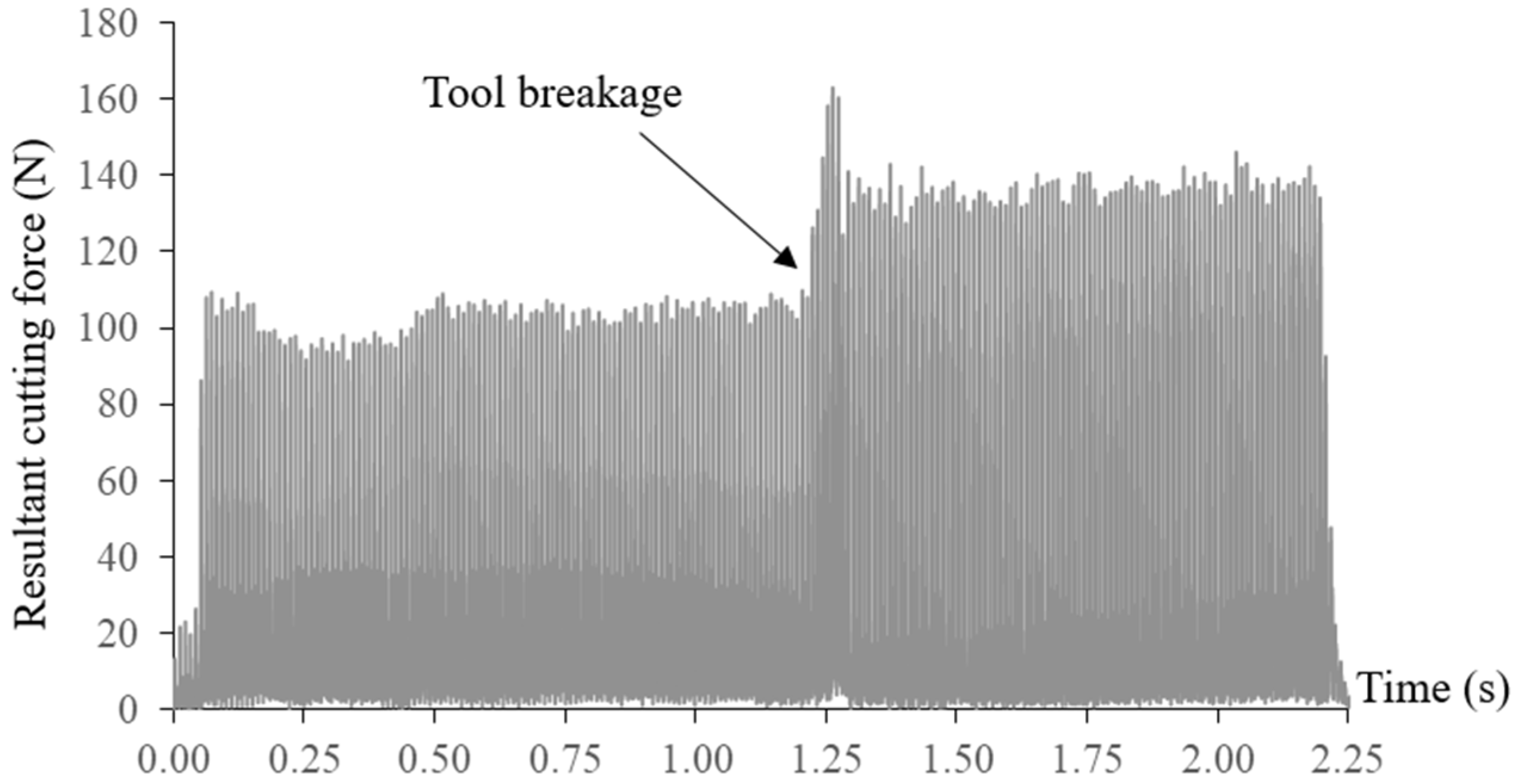
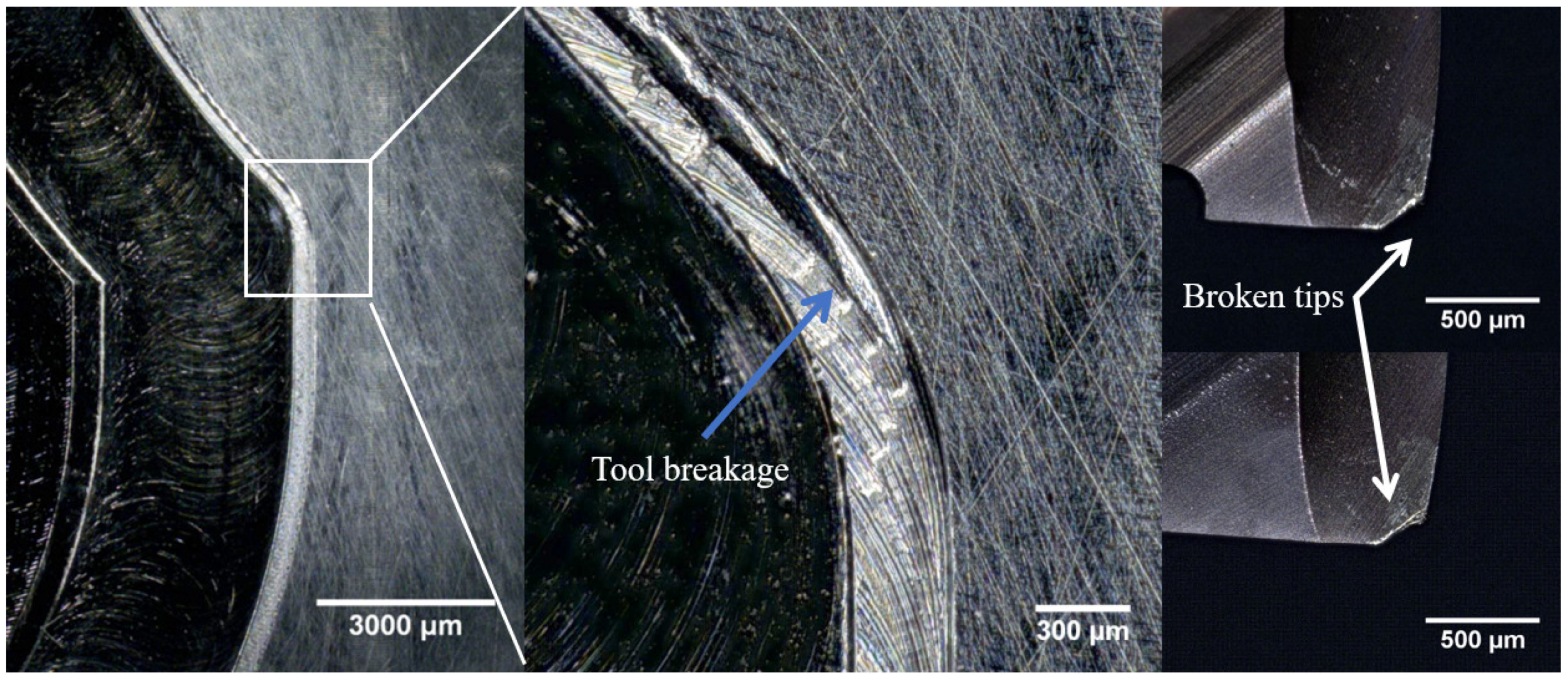
5. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| FEM | finite element method |
| CEL | coupled Eulerian Lagrangian |
| MRR | material removal rate |
| radius of end mill | |
| feed per tooth | |
| uncut chip thickness | |
| angular position of end mill | |
| axial cutting depth | |
| radial cutting depth | |
| spindle speed | |
| transverse rupture strength | |
| uncut chip engaging angle | |
| maximum uncut chip thickness | |
| tool entry angle respect to instantaneous feed direction | |
| actual feed per tooth experienced by the tip of the end mill | |
| maximum tensile stress in the cutting tool | |
| scaling factor for tool stress | |
| feed rate | |
| length of transition for linear interpolation | |
| length of transition for arc interpolation |
References
- Wang, W.P. Solid modeling for optimizing metal removal of three-dimensional NC end milling. J. Manuf. Syst. 1988, 7, 57–65. [Google Scholar] [CrossRef]
- Karunakaran, K.; Shringi, R. A solid model-based off-line adaptive controller for feed rate scheduling for milling process. J. Mater. Process. Technol. 2008, 204, 384–396. [Google Scholar] [CrossRef]
- Li, Z.; Zheng, M.; Zheng, L.; Wu, Z.; Liu, D. A solid model-based milling process simulation and optimization system integrated with CAD/CAM. J. Mater. Process. Technol. 2003, 138, 513–517. [Google Scholar] [CrossRef]
- Ridwan, F.; Xu, X.; Ho, F.C.L. Adaptive execution of an NC program with feed rate optimization. Int. J. Adv. Manuf. Technol. 2012, 63, 1117–1130. [Google Scholar] [CrossRef]
- Spence, A.; Altintas, Y. A solid modeller based milling process simulation and planning system. J. Eng. Ind. 1994, 116, 61–69. [Google Scholar] [CrossRef]
- Lee, H.U.; Cho, D.W. Development of a reference cutting force model for rough milling feedrate scheduling using FEM analysis. Int. J. Mach. Tools Manuf. 2007, 47, 158–167. [Google Scholar] [CrossRef]
- Ko, J.H.; Cho, D.-W. Feed rate scheduling model considering transverse rupture strength of a tool for 3D ball-end milling. Int. J. Mach. Tools Manuf. 2004, 44, 1047–1059. [Google Scholar] [CrossRef]
- Ko, J.H.; Yun, W.S.; Cho, D.-W. Off-line feed rate scheduling using virtual CNC based on an evaluation of cutting performance. Comput. Aided Des. 2003, 35, 383–393. [Google Scholar] [CrossRef]
- Lee, H.U.; Cho, D.-W. An intelligent feedrate scheduling based on virtual machining. Int. J. Adv. Manuf. Technol. 2003, 22, 873–882. [Google Scholar] [CrossRef]
- Mamedov, A.; Lazoglu, I. Micro ball-end milling of freeform titanium parts. Adv. Manuf. 2015, 3, 263–268. [Google Scholar] [CrossRef]
- Erdim, H.; Lazoglu, I. Offline force control and feedrate scheduling for complex free form surfaces in 5-axis milling. Procedia CIRP 2012, 1, 96–101. [Google Scholar]
- Özel, T.; Liu, X. Investigations on mechanics-based process planning of micro-end milling in machining mold cavities. Mater. Manuf. Process. 2009, 24, 1274–1281. [Google Scholar] [CrossRef]
- Liang, Y.; Ren, J.; Zhang, D.; Li, X.; Zhou, J. Mechanics-based feedrate scheduling for multi-axis plunge milling. Int. J. Adv. Manuf. Technol. 2015, 79, 123–133. [Google Scholar] [CrossRef]
- Ferry, W.; Altintas, Y. Virtual five-axis flank milling of jet engine impellers—Part II: Feed rate optimization of five-axis flank milling. J. Manuf. Sci. Eng. 2008, 130, 011013. [Google Scholar] [CrossRef]
- Li, Z.; Zhang, Z.; Zheng, L. Feedrate optimization for variant milling process based on cutting force prediction. Int. J. Adv. Manuf. Technol. 2004, 24, 541–552. [Google Scholar] [CrossRef]
- Fussell, B.; Jerard, R.; Hemmett, J. Robust feedrate selection for 3-axis NC machining using discrete models. J. Manuf. Sci. Eng. 2000, 123, 214–224. [Google Scholar] [CrossRef]
- Guzel, B.; Lazoglu, I. Increasing productivity in sculpture surface machining via off-line piecewise variable feedrate scheduling based on the force system model. Int. J. Mach. Tool. Manuf. 2004, 44, 21–28. [Google Scholar] [CrossRef]
- Erdim, H.; Lazoglu, I.; Ozturk, B. Feedrate scheduling strategies for free-form surfaces. Int. J. Mach. Tools Manuf. 2006, 46, 747–757. [Google Scholar] [CrossRef]
- Zhang, L.; Feng, J.; Wang, Y.; Chen, M. Feedrate scheduling strategy for free-form surface machining through an integrated geometric and mechanistic model. Int. J. Adv. Manuf. Technol. 2009, 40, 1191–1201. [Google Scholar] [CrossRef]
- Tikhon, M.; Ko, T.J.; Lee, S.H.; Kim, H.S. NURBS interpolator for constant material removal rate in open NC machine tools. Int. J. Mach. Tools Manuf. 2004, 44, 237–245. [Google Scholar] [CrossRef]
- Gao, Y.; Ko, J.H.; Lee, H.P. Meso-scale tool breakage prediction based on finite element stress analysis for shoulder milling of hardened steel. J. Manuf. Process. 2020, 55, 31–40. [Google Scholar] [CrossRef]
- Gao, Y.; Ko, J.H.; Lee, H.P. 3D coupled Eulerian-Lagrangian finite element analysis of end milling. Int. J. Adv. Manuf. Technol. 2018, 98, 849–857. [Google Scholar] [CrossRef]
- Burgoyne, H.A.; Daraio, C. Strain-rate-dependent model for the dynamic compression of elastoplastic spheres. Phys. Rev. E 2014, 89, 032203. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Thamizhmanii, S.; Bin Omar, B.; Saparudin, S.; Hasan, S. Surface roughness analyses on hard martensitic stainless steel by turning. J. Achiev. Mater. Manuf. Eng. 2008, 26, 139–142. [Google Scholar]
- Freedman, D.A. Statistical Models: Theory and Practice; Cambridge University Press: Cambridge, UK, 2009; p. 26. [Google Scholar]





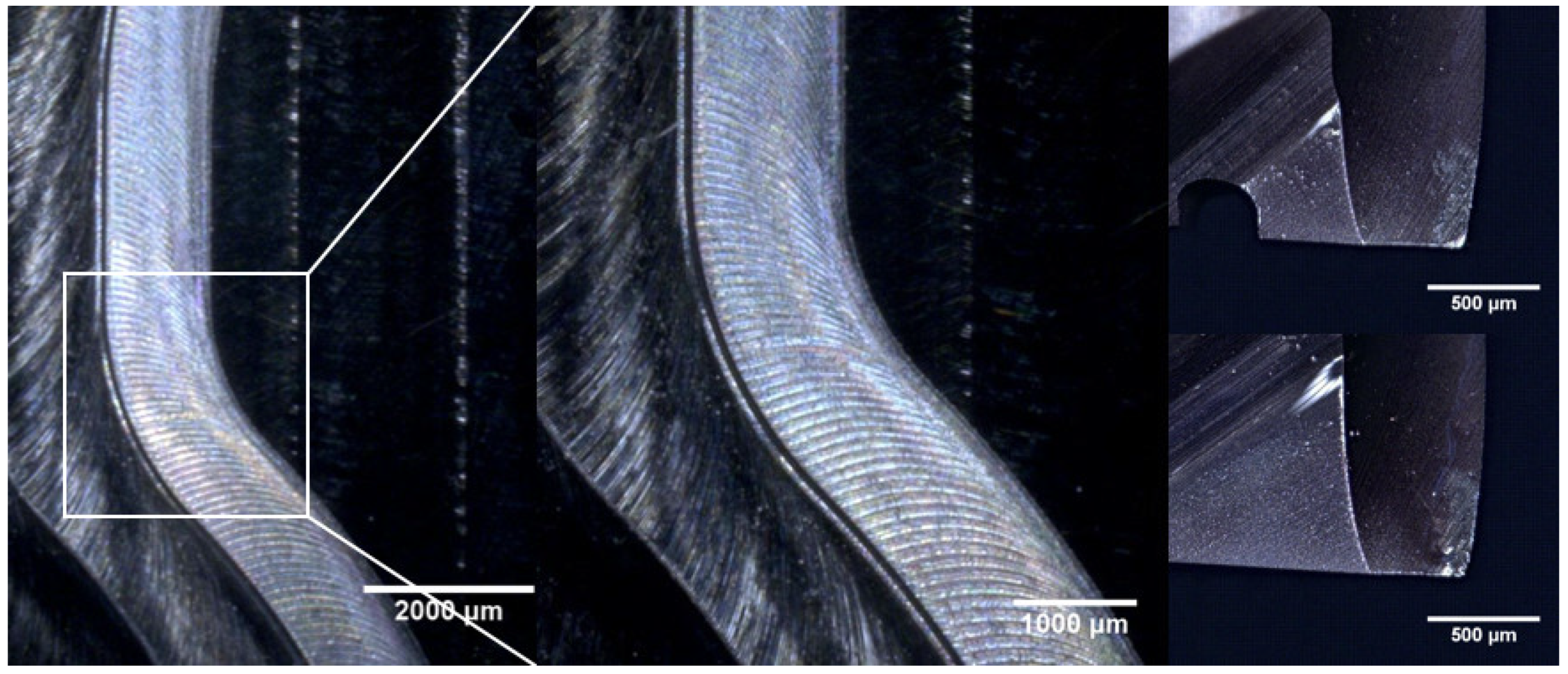
| Diameter | 3.0 mm | Radius of curvature of clearance face | 1.40 mm |
| Helix angle | 30° | Rake angle of bottom cutting edge | 5° |
| Rake angle | 16° | Clearance angle of bottom cutting edge | 8° |
| Radius of curvature of rake face | 0.57 mm | Cutting edge radius | 10 μm |
| Clearance angle | 10° |
| No. | (mm) | (mm) | (mm) | (°) | Tensile Stress (GPa) |
|---|---|---|---|---|---|
| 1 | 0.090 | 0.500 | 0.066 | 48.2 | 3.14 |
| 2 | 0.100 | 0.500 | 0.073 | 48.2 | 3.31 |
| 3 | 0.110 | 0.500 | 0.080 | 48.2 | 3.62 |
| 4 | 0.120 | 0.500 | 0.087 | 48.2 | 3.87 |
| 5 | 0.125 | 0.500 | 0.091 | 48.2 | 4.05 |
| 6 | 0.130 | 0.500 | 0.094 | 48.2 | 4.20 |
| 7 | 0.090 | 0.625 | 0.072 | 54.3 | 3.52 |
| 8 | 0.100 | 0.625 | 0.080 | 54.3 | 3.79 |
| 9 | 0.110 | 0.625 | 0.088 | 54.3 | 3.97 |
| 10 | 0.120 | 0.625 | 0.096 | 54.3 | 4.34 |
| 11 | 0.080 | 0.750 | 0.069 | 60.0 | 3.57 |
| 12 | 0.090 | 0.750 | 0.077 | 60.0 | 3.63 |
| 13 | 0.100 | 0.750 | 0.086 | 60.0 | 4.03 |
| 14 | 0.110 | 0.750 | 0.094 | 60.0 | 4.51 |
| 15 | 0.080 | 0.875 | 0.072 | 65.4 | 3.78 |
| 16 | 0.085 | 0.875 | 0.077 | 65.4 | 4.10 |
| 17 | 0.090 | 0.875 | 0.081 | 65.4 | 4.15 |
| 18 | 0.095 | 0.875 | 0.086 | 65.4 | 4.37 |
| 19 | 0.100 | 0.875 | 0.090 | 65.4 | 4.44 |
| 20 | 0.070 | 1.000 | 0.066 | 70.5 | 3.87 |
| 21 | 0.075 | 1.000 | 0.070 | 70.5 | 3.97 |
| 22 | 0.080 | 1.000 | 0.075 | 70.5 | 3.99 |
| 23 | 0.085 | 1.000 | 0.080 | 70.5 | 4.26 |
| 24 | 0.090 | 1.000 | 0.085 | 70.5 | 4.51 |
| 25 | 0.070 | 1.125 | 0.068 | 75.5 | 3.93 |
| 26 | 0.075 | 1.125 | 0.072 | 75.5 | 4.21 |
| 27 | 0.080 | 1.125 | 0.077 | 75.5 | 4.41 |
| 28 | 0.085 | 1.125 | 0.082 | 75.5 | 4.61 |
| 29 | 0.090 | 1.125 | 0.087 | 75.5 | 4.71 |
| 30 | 0.070 | 1.250 | 0.069 | 80.4 | 4.33 |
| 31 | 0.075 | 1.250 | 0.074 | 80.4 | 4.26 |
| 32 | 0.080 | 1.250 | 0.079 | 80.4 | 4.74 |
| 33 | 0.085 | 1.250 | 0.084 | 80.4 | 4.67 |
| 34 | 0.090 | 1.250 | 0.089 | 80.4 | 5.12 |
| 35 | 0.070 | 1.375 | 0.070 | 85.2 | 4.46 |
| 36 | 0.075 | 1.375 | 0.075 | 85.2 | 4.45 |
| 37 | 0.080 | 1.375 | 0.080 | 85.2 | 4.83 |
| 38 | 0.085 | 1.375 | 0.085 | 85.2 | 4.94 |
| 39 | 0.090 | 1.375 | 0.090 | 85.2 | 5.27 |
| 40 | 0.070 | 1.500 | 0.070 | 90.0 | 4.36 |
| 41 | 0.075 | 1.500 | 0.075 | 90.0 | 4.57 |
| 42 | 0.080 | 1.500 | 0.080 | 90.0 | 4.84 |
| 43 | 0.085 | 1.500 | 0.085 | 90.0 | 5.06 |
| 44 | 0.090 | 1.500 | 0.090 | 90.0 | 5.19 |
| A | B | C | D | E | F |
|---|---|---|---|---|---|
| 373.7649 | −0.2070 | 9.0942 | −32.6897 | 1.5759 | 1.9833 |
| 01. | G90 G00 G54 X-1.5 Y-10. M03 S3000 |
| 02. | Z10. M07 |
| 03. | G01 Z-0.3 F900 |
| 04. | G01 Y2. F991 |
| 05. | G17 G02 X0.950 Y3.090 I5.5 F1081 |
| 06. | G02 X1.611 Y5.889 I4.550 J-3.090 F965 |
| 07. | G03 X5.272 Y14.728 I-8.839 J8.839 F965 |
| 08. | G01 Y15.828 F991 |
| 09. | G01 Y16.728 F760 |
| 10. | G03 X5.126 Y17.081 I-0.5 F760 |
| 11. | G02 X0. Y29.456 I12.374 J12.37 F1008 |
| 12. | G01 X-20. |
| 13. | M09 |
| 14. | G00 Z50. M05 |
| 15. | M30 |
| 01. | G90 G00 G54 X-1.5 Y-10. M03 S3000 |
| 02. | Z10. M07 |
| 03. | G01 Z-0.3 F365 |
| 04. | G01 Y0. |
| 05. | G17 G03 X-1.699 Y5.263 I-9. F365 |
| 06. | G03 X-2.636 Y6.364 I-7.301 J-5.263 F321 |
| 07. | G02 X-3.808 Y9.192 I2.828 J2.828 F321 |
| 08. | G01 Y9.722 F350 |
| 09. | G01 Y11.192 F337 |
| 10. | G02 X-1.025 Y17.910 I9.5 F337 |
| 11. | G03 X0. Y22.385 I-2.475 J2.475 F393 |
| 12. | G01 X-20. |
| 13. | M09 |
| 14. | G00 Z50. M05 |
| 15. | M30 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gao, Y.; Ko, J.H.; Lee, H.P. Digitized Stress Function-Based Feed Rate Scheduling for Prevention of Mesoscale Tool Breakage during Milling Hardened Steel. Metals 2021, 11, 215. https://doi.org/10.3390/met11020215
Gao Y, Ko JH, Lee HP. Digitized Stress Function-Based Feed Rate Scheduling for Prevention of Mesoscale Tool Breakage during Milling Hardened Steel. Metals. 2021; 11(2):215. https://doi.org/10.3390/met11020215
Chicago/Turabian StyleGao, Yifan, Jeong Hoon Ko, and Heow Pueh Lee. 2021. "Digitized Stress Function-Based Feed Rate Scheduling for Prevention of Mesoscale Tool Breakage during Milling Hardened Steel" Metals 11, no. 2: 215. https://doi.org/10.3390/met11020215
APA StyleGao, Y., Ko, J. H., & Lee, H. P. (2021). Digitized Stress Function-Based Feed Rate Scheduling for Prevention of Mesoscale Tool Breakage during Milling Hardened Steel. Metals, 11(2), 215. https://doi.org/10.3390/met11020215








