High-Temperature Deformation of Naturally Aged 7010 Aluminum Alloy
Abstract
:1. Introduction
2. Materials and Methods
3. Results
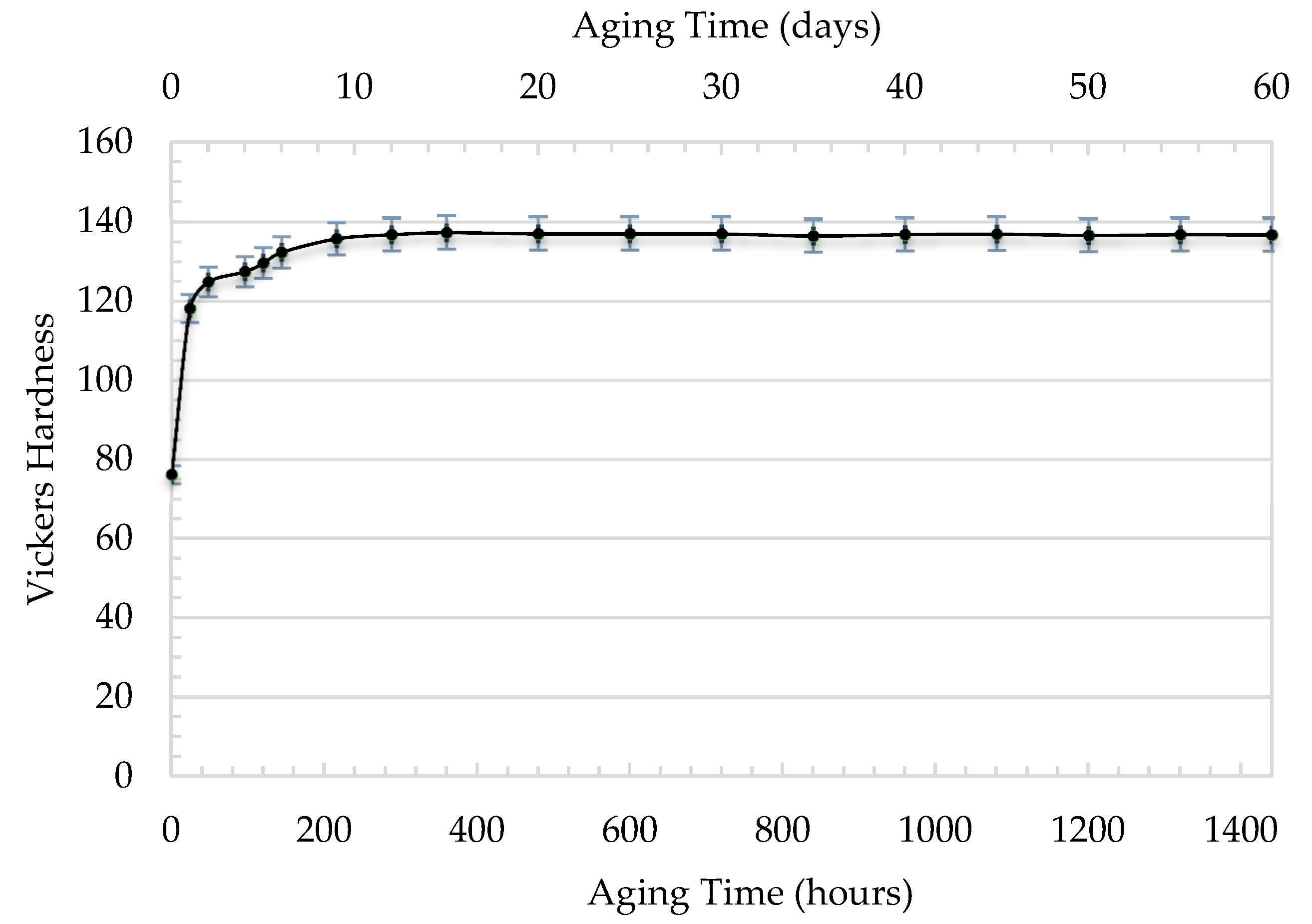
3.1. Hardness
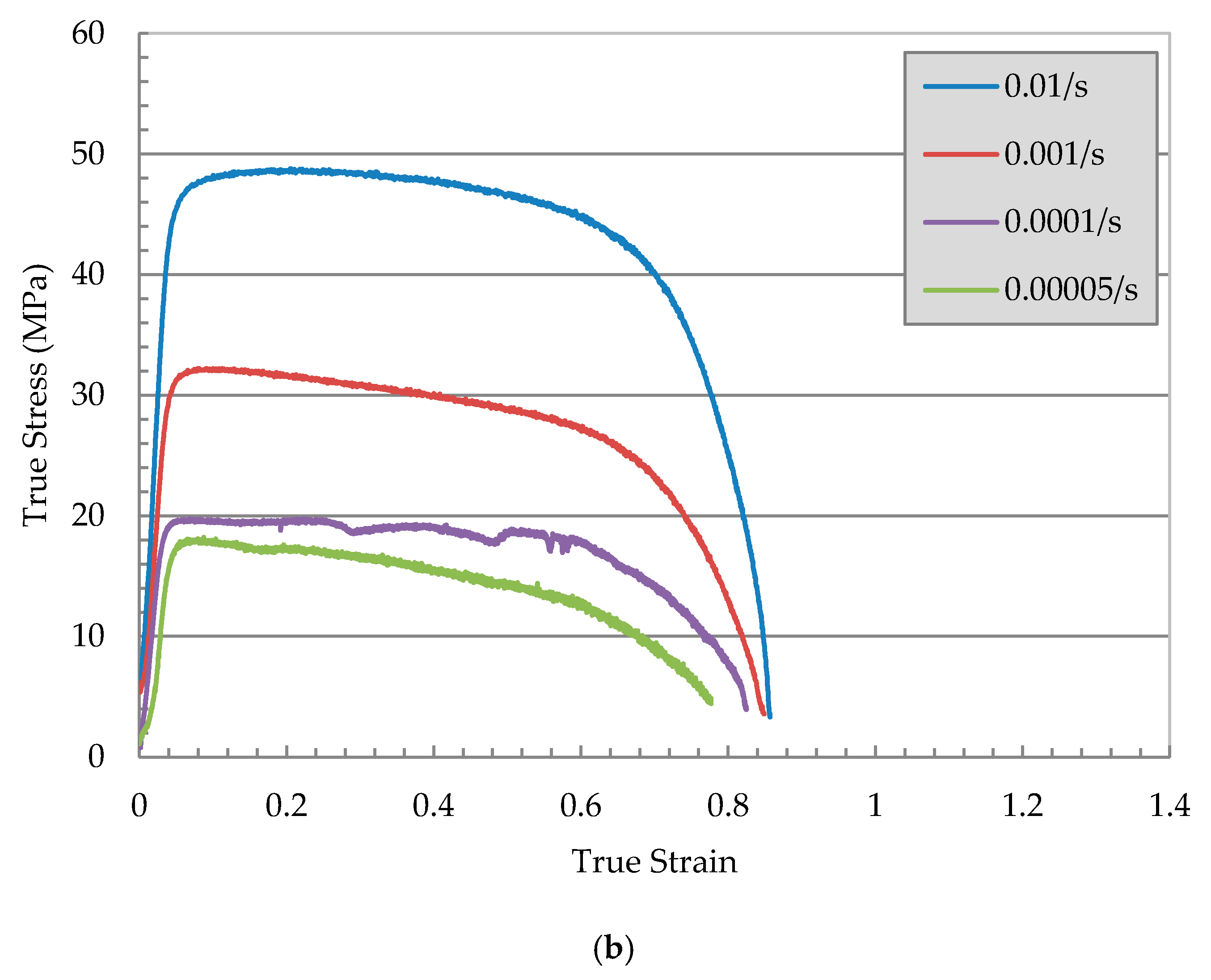
3.2. True Stress-Strain Rate
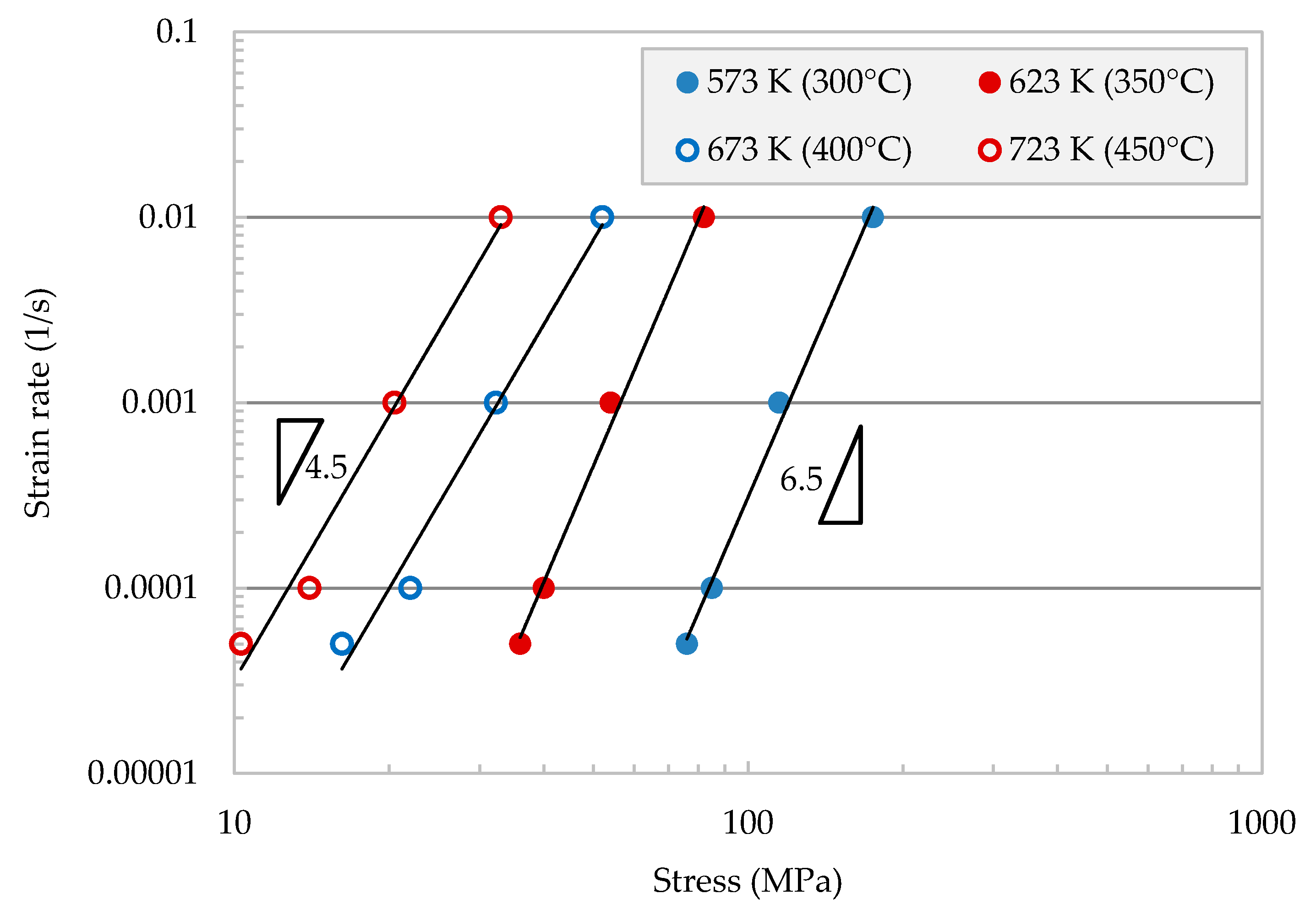
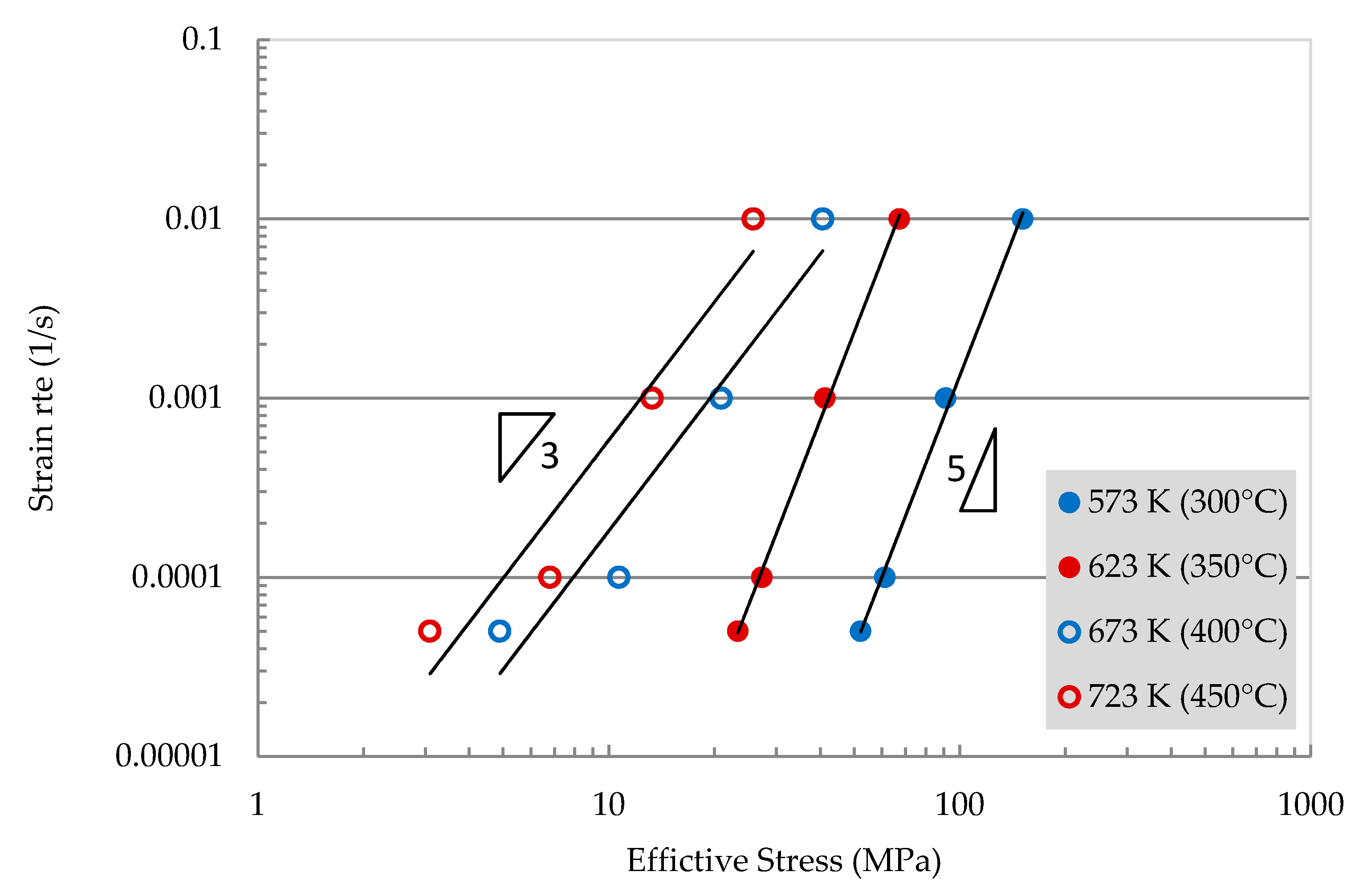
3.3. Stress Dependence on Strain Rate
3.4. Ductility
3.5. Apparent Activation Energy
4. Discussion
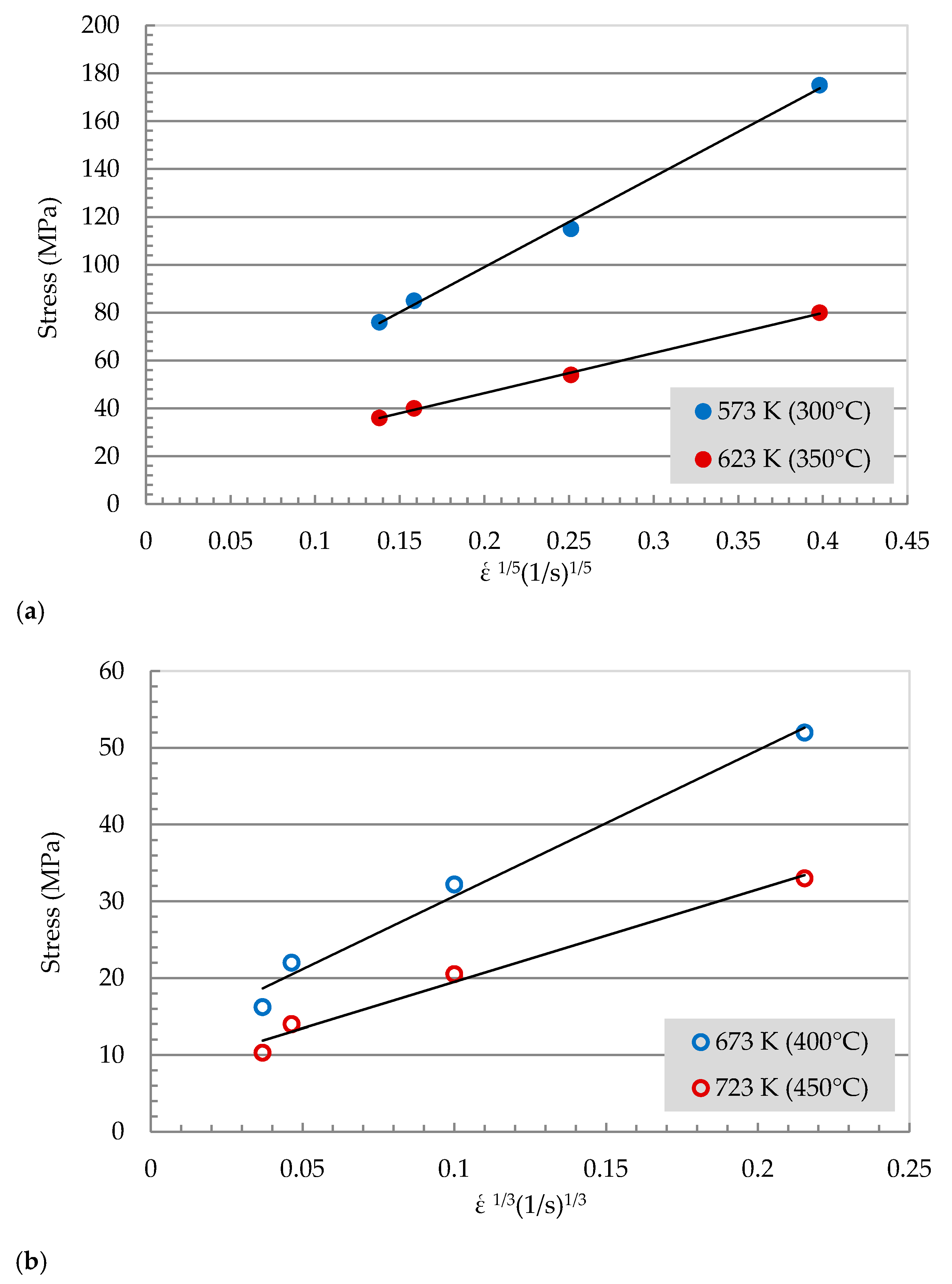
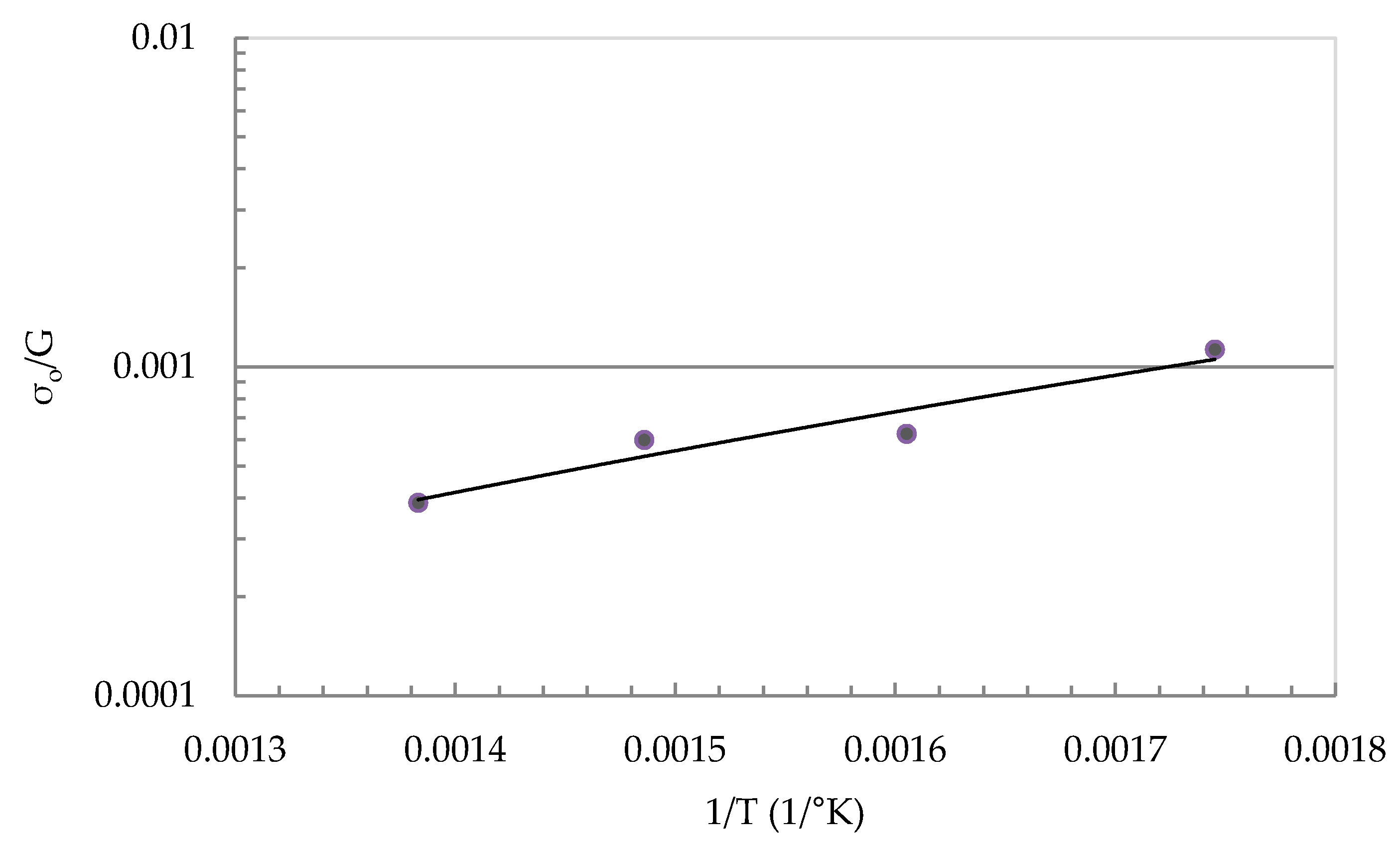
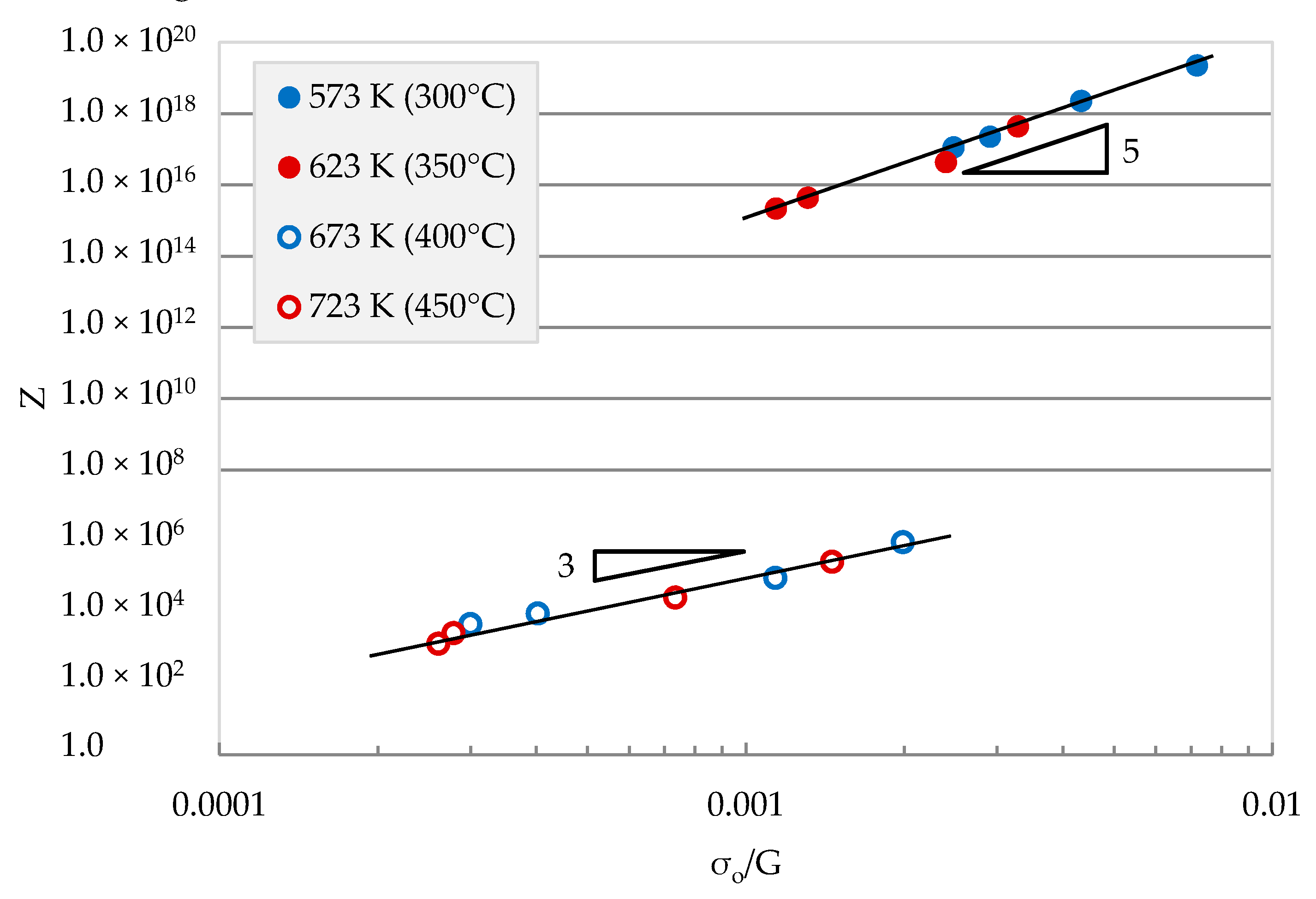
4.1. Threshold Stress
4.2. True Activation Energy
5. Conclusions
Funding
Institutional Review Board Statement
Informed Con sent Statement
Data Availability Statement
Conflicts of Interest
References
- McQueen, H.; Jones, J. Recovery and Recrystallization during High Temperature Deformation. In Plastic Deformation of Materials; Arsenault, R., Ed.; Academic Press: New York, NY, USA, 1975; Volume 6, pp. 393–493. [Google Scholar]
- Kaibyshev, R.; Sitdikov, O.; Mazurina, I.; Lesuer, D. Deformation Behavior of a 2219 Al Alloy. Mater. Sci. Eng. A 2002, 334, 104. [Google Scholar] [CrossRef]
- Marquis, E.; Seidman, D.; Dunand, D. Effect of Mg Addition on the Creep and Yield Behavior of an Al-Sc Alloy. Acta Mater. 2003, 51, 4751–4760. [Google Scholar] [CrossRef]
- He, Z.; Wang, Z.; Lin, Y.; Fan, X. Hot Deformation Behavior of a 2024 Aluminum Alloy Sheet and its Modeling by Fields-Backofen Model Considering Strain Rate Evolution. Metals 2019, 9, 243. [Google Scholar] [CrossRef] [Green Version]
- McQueen, H.; Kassner, M. Elevated temperature deformation: Hot working amplifies creep. Mater. Sci. Eng. A 2005, 410–411, 58–61. [Google Scholar] [CrossRef]
- Seung-Baek, Y.; Mok-Soon, K. Microstructure and High Temperature Deformation of Extruded Al-12Si-3Cu-Based Alloy. Metals 2016, 6, 32. [Google Scholar]
- Mrowka-Nowotnik, G.; Sieniawski, J. Influence of heat treatment on the microstructure and mechanical properties of 6005 and 6082 aluminium alloys. J. Mater. Process. Technol. 2005, 162, 367–372. [Google Scholar] [CrossRef]
- Kassner, M.; Perez-Prado, M. Five-Power-Law Creep in SinglePhase Metals and Alloys. Prog. Mater. Sci. 2000, 45, 1–102. [Google Scholar] [CrossRef]
- Soliman, M.; Mohamed, F. Correlation between Creep Behavior and Substructure in Al-3% Mg Solid-Solution Alloy. Mat. Sci. Eng. 1982, 55, 111–119. [Google Scholar] [CrossRef]
- Sajadifar, S.; Scharifi, E.; Weidig, U.; Steinhoff, K.; Niendorf, T. Performance of Thermo-Mechanically Processed AA7075 Alloy at Elevated Temperatures—From Microstructure to Mechanical Properties. Metals 2020, 10, 884. [Google Scholar] [CrossRef]
- Schindler, I.; Kawulok, P.; Očenášek, V.; Opěla, P.; Kawulok, R.; Rusz, S. Flow Stress and Hot Deformation Activation Energy of 6082 Aluminium Alloy Influenced by Initial Structural State. Metals 2019, 9, 1248. [Google Scholar] [CrossRef] [Green Version]
- Mohamed, F.; Langdon, T. The Transition from Dislocation Climb to Viscous Glide in Creep of Solid Solution Alloys. Acta Metall. 1974, 22, 779–788. [Google Scholar] [CrossRef]
- Yavari, P.; Mohamed, F.; Langdon, T. Creep and Substructure Formation in an Al-5% Mg Solid Solution Alloy. Acta Metall. 1981, 29, 1495–1507. [Google Scholar] [CrossRef]
- El-Danaf, E.; Almajid, A.; Soliman, M. Hot deformation of AA6082-T4 aluminum alloy. J. Mat. Sci. 2008, 43, 6324–6330. [Google Scholar] [CrossRef]
- Almajid, A. High temperature deformation of solution treated7010 Al-alloy. J. King Saud Univ. Eng. Sci. 2011, 23, 21–25. [Google Scholar]
- Abdu, M.; Soliman, M.; El-Danaf, E.; Almajid, A.; Mohamed, F. Creep characteristics and Microstructure in nanoparticle strengthened AA6082. Mater. Sci. Eng. A 2012, 531, 35–44. [Google Scholar] [CrossRef]
- Oikawa, H.; Honda, K.; Ito, S. Experimental Study on the Stress Range of Class I Behavior in the Creep of Al-Mg Alloys. Mat. Sci. Eng. 1984, 64, 237–245. [Google Scholar] [CrossRef]
- Oikawa, H.; Sato, H.; Maruyama, K. Influence of Temperature on the Transition of Deformation Characteristics of Al-1Mg Alloy in the Power Law Creep Regime. Mat. Sci. Eng. 1985, 75, 21–28. [Google Scholar] [CrossRef]
- Sato, H.; Oikawa, H. Further Experimental Study of Deformation Characteristics of Al-Mg Alloys in the Power-Law Creep Regime. Scr. Metall. 1988, 22, 87–92. [Google Scholar] [CrossRef]
- Soliman, M. Effect of Cu Concentration on the Creep Behavior of Al-Cu Solid-Solution Alloys. Mat. Sci. Eng. A 1995, 201, 111–117. [Google Scholar] [CrossRef]
- Soliman, M.; Mohamed, F. Creep Transitions in an Al-Zn Alloy. Metall. Trans. A 1984, 15, 1893–1904. [Google Scholar] [CrossRef]
- Mohamed, F. Incorporation of the Suzuki and the Fisher Interactions in the Analysis of Creep Behavior of Solid Solution Alloys. Mat. Sci. Eng. 1983, 61, 149–165. [Google Scholar] [CrossRef]
- Mohamed, F. Correlation between Creep Behavior in Al-based Solid Solution Alloys and Powder Metallurgy Al Alloys. Mat. Sci. Eng. A 1998, 245, 242–256. [Google Scholar] [CrossRef]
- Evangelista, E.; Spigarelli, S. Constitutive Equations for Creep and Plasticity of Aluminum Alloys Produced by Powder Metallurgy and Aluminum-Based Metal Matrix Composites. Metall. Mater. Trans. A 2002, 33, 373–381. [Google Scholar] [CrossRef]
- Spigarelli, S.; Evangelista, E.; Cucchieri, S. Analysis of the creep response of an Al–17Si–4Cu–0.55Mg alloy. Mat. Sci. Eng. A 2004, 387–389, 702–705. [Google Scholar] [CrossRef]
- Godard, D.; Archambault, P.; Aeby-Gautier, E.; Lapasset, G. Precipitation sequences during quenching of the AA 7010Alloy. Acta Mater. 2002, 50, 2319–2329. [Google Scholar] [CrossRef]
- Hamerskt, M.; Lukac, P.; Trojanova, Z.; Pink, E. Creep of Al-3wt% Mg as measured with the incremental loading method. Mat. Sci. Eng. A 1991, 148, 7–14. [Google Scholar] [CrossRef]


| Element | Zn | Mg | Cu | Si | Fe | Mn | Al |
|---|---|---|---|---|---|---|---|
| AA 7010 wt% | 5.7 | 2.4 | 1.84 | 0.29 | 0.15 | 0.03 | Bal |
| Temperature Range (K) | Strain Rate | Activation Energy, Qa (kJ/mol) | Average Activation Energy, Qa (kJ/mol) |
|---|---|---|---|
| 573–623 | 10−2 | 292 | 290 |
| 10−3 | 291 | ||
| 10−4 | 290 | ||
| 5 × 10−5 | 288 | ||
| 673–723 | 10−2 | 165 | 165 |
| 10−3 | 165 | ||
| 10−4 | 164 | ||
| 5 × 10−5 | 164 |
| Temperature Range (K) | Strain Rate | True Activation Energy, Qt (kJ/mol) | Average True ActivationEnergy, Qt (kJ/mol) |
|---|---|---|---|
| 573–623 (n = 5) | 10−2 | 235 | 234 |
| 10−3 | 230 | ||
| 10−4 | 235 | ||
| 5 × 10−5 | 234 | ||
| 673–723 (n = 3) | 10−2 | 102 | 102 |
| 10−3 | 101 | ||
| 10−4 | 102 | ||
| 5 × 10−5 | 103 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Almajid, A.A. High-Temperature Deformation of Naturally Aged 7010 Aluminum Alloy. Metals 2021, 11, 581. https://doi.org/10.3390/met11040581
Almajid AA. High-Temperature Deformation of Naturally Aged 7010 Aluminum Alloy. Metals. 2021; 11(4):581. https://doi.org/10.3390/met11040581
Chicago/Turabian StyleAlmajid, Abdulhakim A. 2021. "High-Temperature Deformation of Naturally Aged 7010 Aluminum Alloy" Metals 11, no. 4: 581. https://doi.org/10.3390/met11040581
APA StyleAlmajid, A. A. (2021). High-Temperature Deformation of Naturally Aged 7010 Aluminum Alloy. Metals, 11(4), 581. https://doi.org/10.3390/met11040581






