Shape Memory Effect and Superelasticity of [001]-Oriented FeNiCoAlNb Single Crystals Aged under and without Stress

Abstract

The two-step ageing of Fe-28Ni-17Co-11.5Al-2.5Nb (at.%) single crystals under and without stress, leads to the precipitation of the γ′- and β-phase particles. Research has shown that γ–α′ thermoelastic martensitic transformation (MT), with shape memory effect (SME) and superelasticity (SE), develops in the [001]-oriented crystals under tension. SE was observed within the range from the temperature of the start of MT upon cooling M_s, to the temperature of the end of the reverse MT upon heating A_f, and at temperatures from A_f to 323–373 K. It was found that at γ–α′ MT in the [001]-oriented crystals, with γ′- and β-phase particles, a high level of elastic energy, ΔG_el, is generated, which significantly exceeds the energy dissipation, ΔG_dis. As a result, the temperature of the start of the reverse MT, while heating A_s, became lower than the temperature M_s. The development of γ–α′ MT under stress occurs with high values of the transformation hardening coefficient, Θ = dσ/dε from 2 to 8 GPa and low values of mechanical Δσ and thermal ΔT_h hysteresis. The reasons for an increase in ΔG_el during the development of γ–α′ MT under stress are discussed.

1. Introduction

FeNiCoAlX alloys (X = Ta, Nb, Ti), undergoing thermoelastic martensitic transformation (MT) from the fcc (γ)-phase to bct (α′)-martensite upon cooling/heating and under stress, are characterized by high values of superelasticity (SE) from 5 to 13.5% and values of shape memory effect (SME) of up to 8%; these alloys can compete with TiNi-based alloys [1,2,3,4,5,6,7,8,9,10,11,12,13,14,15,16,17,18,19,20,21,22,23,24,25,26,27,28,29,30,31,32,33,34]. The necessary conditions for the development of thermoelastic γ–α′ MT are achieved: (i) due to the precipitation of nanosized γ′-phase particles, with L1₂-type ordering of between 3 and 8 nm in size, which have coherent lattice conjugation with high-temperature γ-phase and retain coherence during MT in α′-martensite [1,2,3,4,28]; (ii) particles of the γ′-phase strengthen the high-temperature phase, reduce the transformation strain by comparison with the single-phase state [1,5] and contribute to an increase in the tetragonality of martensite c/a to 1.1–1.23 [1,28]. This, in turn, decreases the value of the shear strain, g, at γ–α′ MT and the Burgers vector, b, of twinning dislocations a/6<211>(111), arising during MT [1,2,3,4,5,18].

In the FeNiCoAlX polycrystals (X = Ta, Nb, Ti) during ageing at high temperatures, T ~ 873–973 K, the precipitation of β-phase particles with B2-type ordering at the grain boundaries occurs simultaneously with the precipitation of γ′-phase particles in the grain body, leading to a catastrophic decrease in plasticity [5,31,32]. Alloying with boron to 0.05 at.% suppresses the precipitation of β-phase particles along the grain boundaries, which contributes to the manifestation of SME and SE in the polycrystals of these alloys [5,31,32]. In addition, an increase in the grain size to 100–200 µm and the creation of a sharp <001>{035} texture in polycrystals, as well as the production of oligocrystals, leads to the appearance of SE from 5 to 13.5% in these alloys [5,6,31,32].

Studies of γ–α′ MT on the FeNiCoAlX single crystals (X = Ta, Nb, Ti) have a number of advantages over polycrystals. Firstly, single crystals make it possible to study the orientation dependence and asymmetry under the tension and compression of SME and SE [11,12,13,14,15,17,27]. These results are necessary for the development of micromechanical models in the development of a γ–α′ MT under stress in polycrystals with different textures. Secondly, earlier studies of the FeNiCoAlX single crystals (X = Ta, Nb, Ti) showed that the precipitation of β-phase particles in single crystals, in contrast with the polycrystals, does not lead to a loss of plasticity [5,25,31,32,33,34]. Thirdly, nanosized β-phase particles, which are precipitated in single crystals at 973 K, with an ageing time of t ≥ 7 h, in relation to one- and two-step ageing, lead to an increase in MT temperatures, the retention of SME and SE from 3 to 8%, the appearance of rubber like elasticity and the development of SE in the temperature range from M_s to A_f [13,19,33,34].

In the present paper, on the Fe-28Ni-17Co-11.5Al-2.5Nb (FeNiCoAlNb) (at.%) single crystals oriented along the [001] direction, the task was to investigate the temperature dependence of the stresses, σ_cr(T), required for the onset of stress-induced γ–α′ MT, SE and its temperature range, SME and the value of thermal ΔT_h and mechanical Δσ hysteresis, using ageing methods under and without stress under tension. The choice of ageing under and without stress is due to the following. In alloys with coherent non-equiaxial particles, ageing under stress leads to the predominant growth of one variant of particles and the appearance of the internal oriented stresses, which are absent during ageing without stress [35,36,37]. In the case of precipitation of non-coherent and non-equiaxed particles after ageing without stress, internal stresses do not arise due to the formation of dislocations at the “particle-matrix” boundaries [29,30]. The effect of ageing under stress on the precipitation of non-coherent β-phase particles in the FeNiCoAlX alloys (X = Ta, Nb, Ti) has not yet been studied. In the present paper, such experiments using FeNiCoAlNb single crystals oriented along the [001] direction are presented for the first time.

2. Materials and Methods

Ingots of the Fe-28Ni-17Co-11.5Al-2.5Nb (FeNiCoAlNb) (at.%) alloy were melted from pure elements in a resistance furnace (InterSELT, St. Petersburg, Russia) in a helium atmosphere. To achieve a homogeneous distribution of the elements in the bulk of the ingots, they were remelted three times. Single crystals of the FeNiCoAlNb alloy were grown by the Bridgman method in a helium atmosphere, on a Russian-made Redmet installation (Firm “Kristallooptika”, Tomsk, Russia). To determine the orientation of the crystals, the diffractometric method was used by means of a DRON-3M X-ray diffractometer (Bourevestnik, St. Petersburg, Russia) with monochromatic Fe Kα radiation. Dog-bone-shaped tension samples had a gauge length of 12 mm and a cross section of 2 × 1.5 mm². Samples were cut using wire electrical discharge machining ARTA-5.9 (DELTA-TEST, Fryazino, Moscow region, Russia). The damaged surface layer was ground off mechanically, and then electrically polished in 200 mL of an H₃PO₄ + 50 g CrO₃ (phosphoric acid with chromium trioxide) electrolyte at room temperature. All samples were initially homogenized at 1573 K for 24 h in a quartz tube, in a helium atmosphere, followed by water quenching. Two-step ageing was used to precipitate γ′- and β-phase particles. The first step of ageing was carried out at a temperature of 973 K for 5 h without stress, in an MRU-STF15180-01_301 resistance furnace (Carbolite Gero, Hope Valley, UK) within a quartz tube, in a helium atmosphere with water quenching. The second step of ageing was performed in a vacuum at 873 K, for 2 and 4 h in a special device (Firm “Kristallooptika”, Tomsk, Russia) with a vacuum chamber, able to apply tensile stresses of 120 MPa during ageing, in the following way. Two samples were simultaneously used during each ageing treatment in the second step, with their axes in vertical and horizontal positions. The temperature was monitored by a thermocouple, attached to the vertical sample. The device was pre-heated to 473 K, subsequently, a constant tensile stress of 120 MPa was applied to the vertical sample, whereas the horizontal sample remained in a stress-free condition. Next, the device was heated to 873 K at a rate of 20 K/min and kept for 2 h and 4 h at 873 K. A controlled stress of 120 MPa, applied to the vertical sample, was maintained during all the ageing treatment. The simultaneous ageing of two samples (under and without applied stress) allows for a better comparison of these, to ascertain the effect of the applied external stress, as the samples adhere to exactly the same temperature evolutions during ageing. After completion of the ageing process under stress, the device was slowly cooled to 373 K over 1.5 h and unloaded. Start M_s and finish M_f temperatures of the forward γ–α′ MT during cooling and start A_s and finish A_f temperatures of the reverse γ–α′ MT during heating, were determined by the intersection of tangents on the temperature dependence of the electrical resistance ρ(T) on a Russian-manufactured installation (Firm “Kristallooptika”, Tomsk, Russia). The MT temperatures under stress were determined in terms of deviation from the linear dependence in the “transformation strain-temperature” (ε_tr(T)) curves. The value of thermal ΔT_h and mechanical Δσ hysteresis was determined in the middle of the ε_tr(T) and “stress-strain” (σ(ε)) hysteresis loops, respectively. Mechanical properties and SE were investigated using an Instron 5969 universal testing machine (Instron, Norwood, MA, USA) at the deformation rate of 4∙10⁻⁴ s⁻¹. Tests at room temperature were carried out in air. Tests within the temperature range of 185–350 K were carried out in a special cooling/heating chamber, which is included in the equipment of the Instron 5969 universal testing machine (Instron, Nowood, MA, USA). The heating/cooling rate of the chamber was 2 K/min. The sample was inserted into grips, heated/cooled together with the chamber, kept at each temperature for 30 min before testing, and then deformed. SME at a constant tensile stress in the cycle, was studied using a Russian-made dilatometer (Firm “Kristallooptika”, Tomsk, Russia) during cooling and heating within a temperature range of 77–400 K, with a heating/cooling rate of 10 K/min. Temperature variations at ~10 K/min were achieved by liquid nitrogen, flowing in copper tubes, coiled around the grips and by induction heating of the grips. The sample temperature was measured by a thermocouple, fixed to the sample gauge section. The lowest sample temperature, attainable by the dilatometer, was ~77 K. A miniature extensometer, attached to the sample, was used for the strain measurements. The crystal structure after ageing was investigated using a JEOL-2010 transmission electron microscope (TEM) (JEOL, Tokyo, Japan) at an accelerating voltage of 200 kV. The thin foils were prepared using double-jet electropolishing (TenuPol-5, “Struers”, Ballerup, Denmark), with an electrolyte containing 20% sulfuric acid in methyl alcohol at room temperature, with 12.5 V applied voltage. In this paper, the following denotations are adopted for heat treatments: Crystal I—ageing without stress at 973 K for 5 h and then at 873 K for 2 h without stress and with slow cooling; Crystal II—ageing without stress at 973 K for 5 h and then ageing under stress 120 MPa at 873 K for 2 h with slow cooling; Crystal III—ageing at 973 K for 5 h, then at 873 K for 4 h without stress and with slow cooling; and Crystal IV—ageing without stress at 973 K for 5 h and then ageing under stress 120 MPa at 873 K for 4 h with slow cooling. For each ageing type, namely, Crystals I–IV, SE and SME were investigated on separate samples after determining the MT temperatures.

3. Results and Discussion

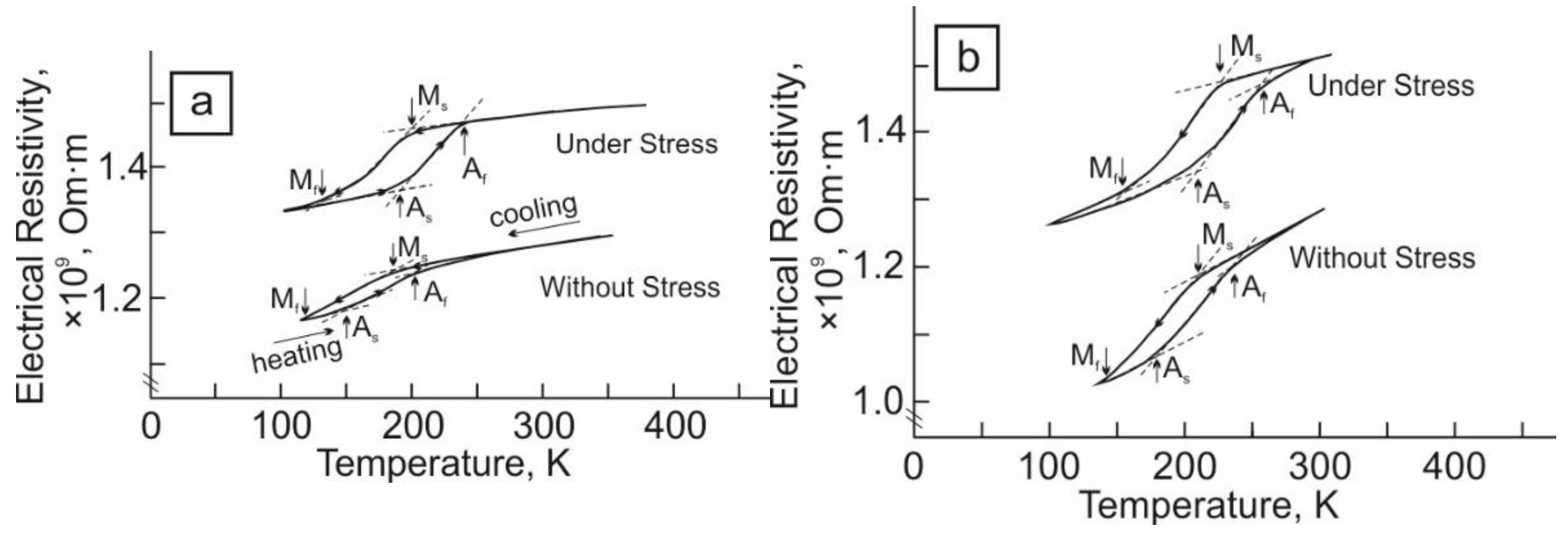
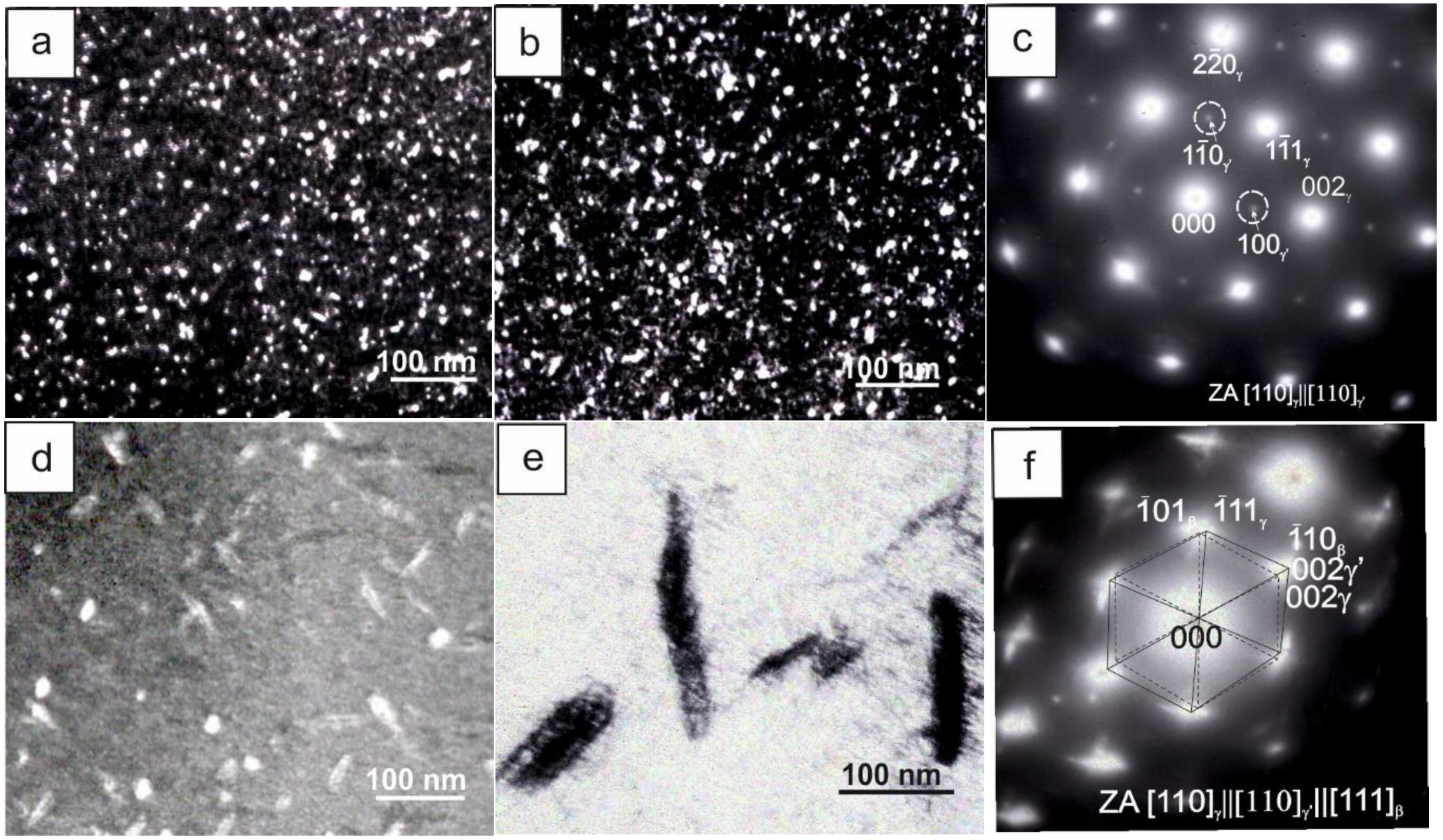
3.1. Effect of Ageing under and without Stress on Temperatures of γ–α′ MT and the Precipitation of γ′- and β-Phase Particles

The temperatures of γ–α′ MT, depending on the ageing condition under and without stress, and determined from the temperature dependence of the electrical resistivity ρ(T), are presented in Figure 1 and

Table 1. Characteristics of γ–α′ MT in the FeNiCoAlNb single crystals after ageing under and without stress, obtained from the analysis of the temperature dependence of the electrical resistivity ρ(T): ΔT_h = A_f − M_s is the thermal hysteresis; ΔM = M_s − M_f is overcooling; ΔA = A_f − A_s is overheating; ΔG_el is the stored elastic energy; ΔG_dis is the dissipated energy.

Crystals	Ms, K	Mf, K	As, K	Af, K	ΔTh, K	ΔM, K	ΔA, K	ΔGel/ΔGdis
Crystal I	185	120	150	203	18	65	53	6.6
Crystal II	200	130	190	240	40	70	50	3
Crystal III	214	140	180	235	21	74	55	6.5
Crystal IV	225	155	210	260	35	70	50	3.7

. It may be observed that in Crystals III with regard to two-step ageing, an increase in the ageing time at the second step at 873 K from 2 to 4 h without stress leads to an increase in the M_s temperature by 29 K compared to Crystals I. In Crystals II and IV, ageing under stress at the second step leads to an increase in the M_s temperature by 15 and 11 K, respectively, relative to Crystals I and III.

TEM studies (Figure 2) showed that in Crystals I–IV, the γ′- and β-phase particles are precipitated. The γ′-phase particles of the equiaxed shape, with L1₂-type ordering, which is confirmed by the presence of superstructural reflections (Figure 2c), have the chemical composition, (FeNiCo)₃AlNb [5,13,19,31,32]. Equiaxial γ′-phase particles have a size d~8–12 nm and coherent conjugation with the high-temperature γ-phase. These data are consistent with previously published results in [5,13,19,31,32]. TEM studies have shown that the particle size of the γ′-phase depends weakly on the ageing time at the second step and the external, applied stresses during ageing (Figure 2a,b).

The β-phase particles of the non-equiaxial shape with B2-type ordering have the chemical composition, (FeNiCo)(AlNb) [31,32] and are non-coherent with the high-temperature γ-phase (Figure 2d,e). Due to the significant difference in the lattice parameters (a_γ = 0.36 nm, a_β = 0.28 nm), the β-phase particles lose coherence at small sizes, d < 5 nm [35]. Similar lattice parameters of the γ- and β-phases were obtained in the FeNiCoAlTaB polycrystals (a_γ = 0.3604 nm, a_β = 0.2880 nm) [5,31,32]. In the FeNiCoAlTaB polycrystals, β-phase particles are precipitated at the grain boundaries with the formation of regions free from γ′-phase particles. In these polycrystals, the nucleation of β-phase particles was also found on the Ta particles, with a lattice parameter close to the β-phase. The Ta particles decrease the energy barrier, associated mainly with the generation of elastic energy, for the β-phase nucleation [31,38]. In the FeNiCoAlNb single crystals, β-phase particles are precipitated in the volume of the crystal, and zones free from γ′-phase particles are not observed. During ageing without and under stress at the second step, four crystallographically equivalent variants of the β-phase particles, with an orientation relationship (111)_γ||(110)_β, $[1\overline{1}0]$_γ||$[1\overline{1}1]$_β, are formed (Figure 2d–f) [29,38]. With an increase in the ageing time at the second step, the volume fraction and particle size of the β-phase increase from f = 0.1, d = 10–15 nm and l = 35–40 nm at 2 h, to f = 0.15, d = 22–35 nm and l = 100–135 nm at 4 h (Figure 2d,e). Ageing under a tensile stress of 120 MPa does not lead to a notable difference in the morphology of the particles of the γ′-and β-phases compared to ageing without stress. In crystals aged under stress, an increased dislocation density was observed at the “matrix–β-phase” interface (Figure 2e), which was less pronounced during ageing without stress.

TEM studies facilitate an explanation of the dependence of the M_s temperature on the ageing conditions [2]. Firstly, in Crystals I–IV, the volume fraction and particle size of the γ′-phase change insignificantly (Figure 2a,b) and, therefore, the M_s temperature change is not associated with this phase. Secondly, in Crystals I–IV, the volume fraction and particle size of the β-phase change in relation to the ageing time at the second step. The volume fraction and size of β-phase particles are larger in Crystals III and IV, than in Crystals I and II. This leads to a decrease in the nickel concentration in the matrix and explains the increase in the M_s temperature in Crystals III and IV, in comparison with Crystals I and II [1,2,3,4,5,6,38]. Thirdly, the differences in the sizes and volume fractions of β-phase particles, as a consequence of the ageing conditions under and without stress, were not found in the study of the crystal structure. Consequently, an increase in the M_s temperature in Crystals II and IV compared to Crystals I and III, respectively, cannot be explained from the standpoint of changes in the concentration of alloying elements in the matrix, and in the morphology of the particles of the γ′- and β-phases. The increase in the M_s temperature in Crystals II and IV in comparison with Crystals I and III is due to the formation of internal oriented stress fields <σ_G> from the β-phase particles. A qualitative confirmation of the <σ_G> appearance is the formation of dislocations at the “matrix-β-phase” boundaries (Figure 2e), as was previously observed in TiNi crystals, aged under stress and containing partially non-coherent Ti₃Ni₄ precipitates [29,30,39,40].

As can be seen in Table 1, γ–α′ MT during heating-cooling in a free state, is associated with thermoelastic MT of the second type, in which A_s ≤ M_s [29,30,41]. Another feature is the large value of overcooling ΔM = M_s − M_f, with a forward γ–α′ MT and overheating of ΔA = A_f − A_s with a reverse α′–γ MT, varying from 50 to 74 K in combination with low values of thermal hysteresis, ΔT_h = A_f − M_s = 18–40 K. According to the results of thermodynamic analysis for the thermoelastic MT of the second type [41,42,43,44,45], the ratio of the stored elastic energy, ΔG_el, is equal to or exceeds the doubled value of the dissipated energy, 2ΔG_dis, ΔG_el ≥ 2ΔG_dis. The values of ΔG_el and ΔG_dis are estimated from the relationships obtained in [33,41,42,43,44,45]:

$$\mathsf{\Delta }{\mathrm{G}}_{\mathrm{el}}=\frac{\mathsf{\Delta }{\mathrm{S}}_{\mathrm{ch}}}{2}\left({\mathrm{M}}_{\mathrm{s}}-{\mathrm{M}}_{\mathrm{f}}\right)+\frac{\mathsf{\Delta }{\mathrm{S}}_{\mathrm{ch}}}{2}\left({\mathrm{A}}_{\mathrm{f}}-{\mathrm{A}}_{\mathrm{s}}\right)$$

(1)

$$\mathsf{\Delta }{\mathrm{G}}_{\mathrm{dis}}=\frac{\mathsf{\Delta }{\mathrm{S}}_{\mathrm{ch}}}{2}\left({\mathrm{A}}_{\mathrm{f}}-{\mathrm{M}}_{\mathrm{s}}\right)$$

(2)

M_s, M_f and A_s, A_f are, respectively, the temperatures of the start and finish of the forward γ–α′ MT during cooling and the reverse during the heating of the α′–γ MT; ΔS_ch is the change in entropy under MT. Estimates show that ΔG_el/ΔG_dis varies from 3 to 6.6 (Table 1). High values of ΔG_el, as will be shown below, determine the temperature range of SE in Crystals I–IV.

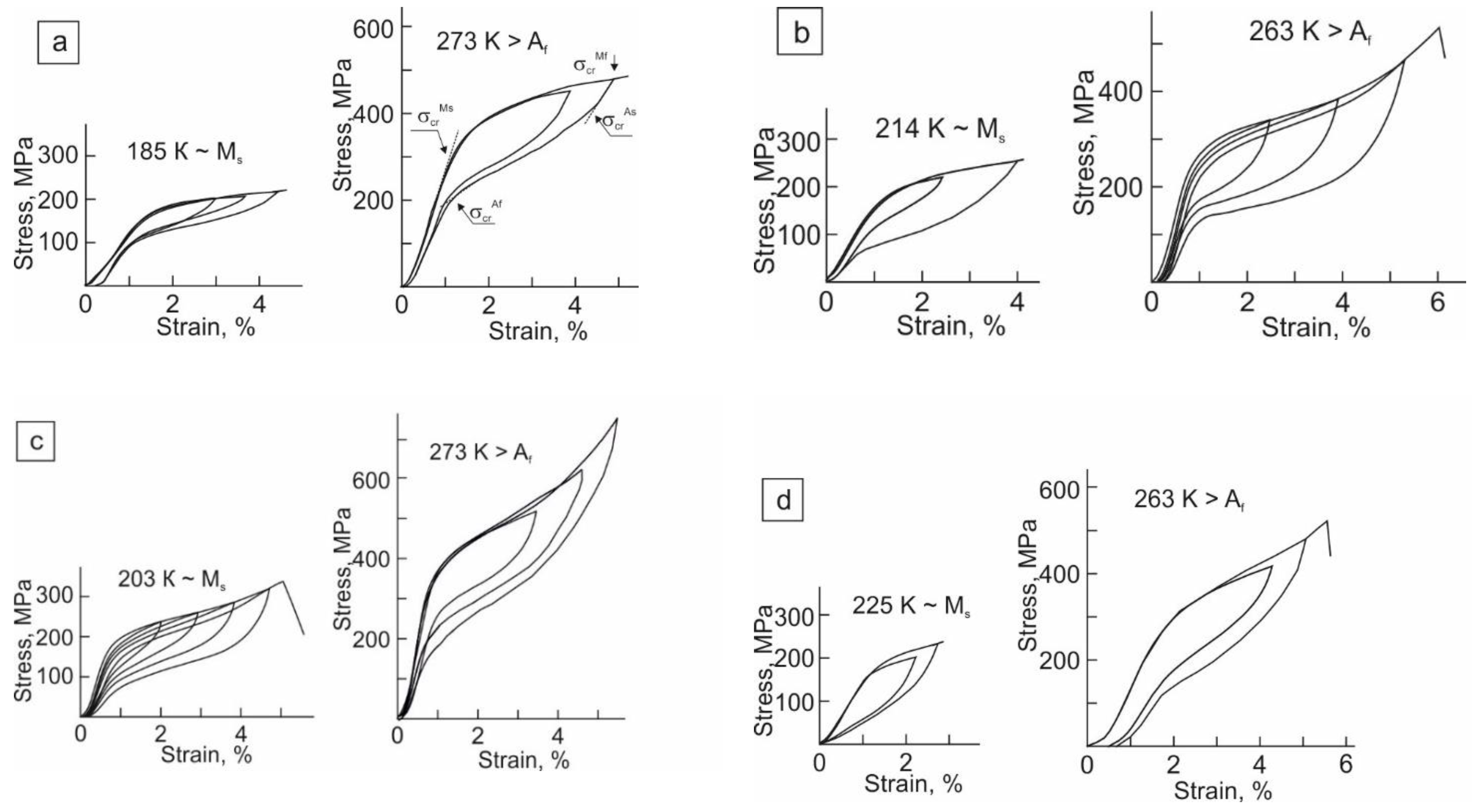
3.2. SE and SME in the [001]-Oriented FeNiCoAlNb Crystals Aged under and without Stress

SE in Crystals I–IV was found within a broad temperature range (Figure 3). A comparison of the γ–α′ MT temperatures, obtained from the ρ(T) dependence (Figure 1) and the SE temperature range (Figure 3), shows that SE in Crystals I–IV takes place at M_s temperature, within the temperature range M_s < T < A_f and above the A_f temperature. According to well-known experimental and theoretical studies, the conditions for SE are achieved at T ≥ A_f, when martensite becomes thermodynamically unstable, when the load is removed [29,30]. SE within the temperature range from M_s to A_f was observed in FeNiCoAlNb single crystals [33], FeNiCoAlTaB [31,32] and FeNiCoTi polycrystals [9]. Reversible deformation within the temperature range between 77 K and M_s is not associated with the development of γ–α′ MT under stress, but is caused by the reversible motion of “austenite-martensite” interphase and twin boundaries of a cooling α′-martensite in “load–unloading” cycles. It was previously found in FeNiCoAlNb single crystals [33] and FeNiCoAlTaB polycrystals [31,32]. This was defined as having rubber-like behavior [33]. In this paper, the reversible deformation at T < M_s was not investigated.

It may be observed that σ_cr^Ms for the onset of stress-induced γ–α′ MT within the temperature range from M_s to 373 K increases, with an increase in the test temperature, and the dependence, σ_cr^Ms(T), turns out to be characteristic of alloys, undergoing MT under stress at T > M_s (Figure 3 and Figure 4). This dependence is described by the Clapeyron–Clausius relationship [29,30,45]:

$$\frac{{\mathrm{d}\mathsf{\sigma }}_{\mathrm{cr}}^{\mathrm{Ms}}}{\mathrm{dT}}=-\frac{\mathsf{\Delta }{\mathrm{S}}_{\mathrm{ch}}}{{\mathsf{\epsilon }}_{\mathrm{tr}}}=-\frac{\mathsf{\Delta }{\mathrm{H}}_{\mathrm{ch}}}{{\mathsf{\epsilon }}_{\mathrm{tr}}{\mathrm{T}}_0}=\mathsf{\alpha }$$

(3)

ΔH_ch and ΔS_ch are the enthalpy and entropy changes, respectively, at MT; T₀ is the temperature of the chemical equilibrium of phases and ε_tr is the transformation strain at MT. Figure 4 demonstrates that the value α = dσ_cr^Ms/dT varies from 3.2 to 3.8 MPa/K in Crystals I–IV. In the case of Crystals II and IV, α = dσ_cr^Ms/dT is greater than for Crystals I and III. A comparison of the α = dσ_cr^Ms/dT values for Crystals I–IV with the known literature data, obtained on the [001]-oriented FeNiCoAlX crystals (X = Ta, Nb, Ti) under tensile strain and containing only γ′-phase particles, 3–8 nm in size and particles of the γ′- and β-phases [25,27,33,34], showed that these values, α = dσ_cr^Ms/dT, are in close proximity to one other.

Using relationship (3) and the results of an increase in the M_s temperature during ageing under stress, ΔM_s = M_s^σ − M_s⁰ (M_s^σ and M_s⁰ are temperatures, respectively, in crystals aged under and without stress), one can estimate the level of internal stresses <σ_G> in accordance with relationship (4):

<σ_G> = (ΔM_s^G/ε_tr) ΔS_ch; ΔS_ch/ε_tr = α = dσ_cr/dT

(4)

In Crystals II and IV, the value of <σ_G> calculated by relationship (4) is 35–57 MPa at the ΔM_s = 11–15 K and α = (3.2–3.8) MPa/K.

SE loops with a sequential increase in ε_tr in the “load–unloading” cycle until fracture at M_s and the temperature above A_f of Crystals I–IV, are presented in Figure 5. The results obtained for Crystals I are close to those obtained earlier in [33]. In relation to Crystals I, at M_s temperature, the maximum SE was 3.3%. With an increase in the transformation strain, ε_tr, in the “load–unloading” cycle, an increase in the mechanical hysteresis, Δσ, is observed, and rather high values of the transformation hardening coefficient, Θ = dσ/dε, equal to 2 GPa, are found. At 273 K, the SE was 3%, the mechanical hysteresis, Δσ, increased three times relative to the M_s temperature and became equal to 170 MPa and Θ = 4.3 MPa. Among Crystals II, at an M_s temperature, the SE was 3.5%, Δσ increased to 135 MPa and Θ = dσ/dε, up to 3.2 GPa, compared to Crystals I at this temperature. At 273 K, the SE was 4%, Δσ = 190 MPa and Θ = 8 GPa. Thus, ageing under stress leads to a significant increase in Θ = dσ/dε and Δσ, compared to ageing without stress.

In Crystals III and IV, an increase in the ageing time at the second step to 4 h at 873 K, as shown above, increases the volume fraction of β-phase particles in comparison with Crystals I and II. As a result, the SE decreases, while Δσ and Θ, on the contrary, increase and an irreversible strain appears of up to 0.5% at temperatures above A_f (Figure 5).

An analysis of the SE loops for Crystals I–IV in the temperature range from M_s to 373 K, shows that in all studied crystals, the stresses, σ_cr^Ms, for the onset of stress-induced γ–α′ MT, are lower than the stresses, σ_cr^As, of the start of the reverse α′–γ MT, when unloading (Figure 3 and Figure 5). Using the results of the thermodynamic analysis of SE loops [33,42,43,44,45], the contribution of ΔG_el and ΔG_dis can be determined from the values of σ_cr^Ms, σ_cr^Mf and σ_cr^As, σ_cr^Af:

ΔG_el = (σ_cr^Mf(T) − σ_cr^Ms(T))ε_tr

(5)

ΔG_dis = ½(σ_cr^Mf(T) − σ_cr^As (T))ε_tr

(6)

σ_cr^Ms and σ_cr^Mf are the stresses at the start and end of the forward γ–α′ MT under load, and σ_cr^As and σ_cr^Af are the stresses at the start and end of the reverse α′–γ MT, when the load is removed. The estimate of the ratio ΔG_el/ΔG_dis will depend on ε_tr, therefore, its calculations for Crystals I–IV for different temperatures, were tested in relation to the close values of ε_tr = 2% (Figure 3). Estimates showed that ΔG_el/ΔG_dis vary from 5.5 to 6.3, within the range between M_s and A_f, and at T > A_f.

The condition for the appearance of a closed loop at T ≥ M_s was obtained in [33] according to the assumption that σ_cr^Af(T) > 0, and is written as follows:

ΔG_ch(T) + ΔG_el > ΔG_dis

(7)

$\mathsf{\Delta }{\mathrm{G}}_{\mathrm{ch}}\left(\mathrm{T}\right)=\left(\mathrm{T}-{\mathrm{T}}_0\right)\mathsf{\Delta }{\mathrm{S}}_{\mathrm{ch}}$ is the chemical energy change at MT. At T ≥ M_s, condition (7) is satisfied since ΔG_el = (5.5–6.3)ΔG_dis and the reverse α′–γ MT occurs, due to the large stored elastic energy. A high level of ΔG_el determines high values of the transformation hardening coefficient Θ = dσ/dε at T ≥ M_s. This is due to the fact that β-phase particles have a non-coherent conjugation of the lattice with the high-temperature phase. These particles are stable and their atomic structure locks the MT inside the β-phase. Consequently, in the case of a reversible γ–α′ MT, the β-phase particles are only deformed elastically and can assist to the predominant nucleation of α′-martensite near the “β-phase–high-temperature phase” interphase surfaces, as was previously observed in the TiNi-based composites, containing TiC particles [46,47]. High values of Θ = dσ/dε at a low level of strain ε ≤ 5%, were observed in fcc poly- and single crystals, containing non-coherent, plastically undeformed particles during slip deformation in Cu-SiO₂, Cu-Al₂O₃ [48,49] and upon twinning deformation in Cu-Al-Co with non-coherent CoAl particles [50]. In this case, an increase in ΔG_el and, accordingly, <σ_G>, depending on the level of plastic deformation ε_pl by slip, twinning and γ–α′ MT in the absence of relaxation processes, is written as [48,49]:

$$<{\mathsf{\sigma }}_{\mathrm{G}}>=2{\mathsf{\gamma }\mathrm{Gf}\mathsf{\epsilon }}_{\mathrm{pl}}\frac{1}{\mathrm{m}}$$

(8)

$$\mathsf{\theta }\approx \frac{{\mathrm{d}\mathsf{\sigma }}_{\mathrm{G}}}{{\mathrm{d}\mathsf{\epsilon }}_{\mathrm{pl}}}=2\mathsf{\gamma }\mathrm{fG}\frac{1}{\mathrm{m}}$$

(9)

$\mathsf{\gamma }=\frac{5-7\mathsf{\nu }}{15\left(1-\mathsf{\nu }\right)}$ = 0.28, where ν = 0.3 is Poisson’s coefficient for martensite, G = 72 GPa is the shear modulus of martensite, f is a volume fraction of β-phase particles, which is estimated as being between 10–15%, ε_pl is plastic deformation and m is the Schmid factor for twinning in the [001] orientation in martensite, which is equal to 0.41 at tensile strain [33]. The experimental Θ = dσ/dε values for Crystals I–IV, which ranged from 2 to 8 GPa, were close to the calculated Θ = dσ/dε values according to relationship (9). It is interesting that in the study of SE of the [001]-oriented FeNiCoAlX crystals (X = Ta, Nb, Ti), containing only the nanosized γ′-phase particles, the value of Θ = dσ/dε was equal to 2–3 GPa, σ_cr^Ms > σ_cr^As, M_s < A_s. Therefore, MTs are transformations of the first type, according to the Tong–Wayman classification [11,12,18,21,27].

SME studies during cooling/heating, under the constant stresses of Crystals I–IV are shown in Figure 6 and Figure 7. The values of SME ε_SME, overcooling ΔM = M_s − M_f and overheating ΔA = A_f − A_s, as well as thermal hysteresis ΔT_h = A_f − M_s, depend on the level of applied stresses, σ_cr, in the “cooling–heating” cycle and heat treatment (Figure 6). In the studied Crystals I–IV, the M_s^σ temperature of the onset of the γ–α′ MT under stress increases with an increase in the level of applied stresses, σ_cr, and coincides with the M_s^σ temperature obtained in experiments on the SE study (Figure 3 and Figure 4). The values of ΔM, ΔA, ΔT_h and ε_tr also increase with increasing level of applied stresses, σ_cr. Figure 4 displays the stresses of the finish of the reverse MT, σ_cr^Af, when the load is removed, which like the stresses for the onset of the stress-induced MT σ_cr^Ms, were obtained from the results of studying the SE temperature range (Figure 3) and the SME under stress (Figure 6). It can be seen that similar values of σ_cr^Af and σ_cr^Ms were obtained for Crystals I–IV in both experiments. The σ_cr^Af curves for Crystals I and II are parallel to the σ_cr^Ms curves, which indicates a weak dependence of Δσ on the test temperature and thermal hysteresis ΔT_h on the level of external stresses. In Crystals III and IV, Δσ and ΔT_h are greater than in Crystals I and II and increase with growth of the test temperature and external stresses, respectively. In this case, the σ_cr^Ms and σ_cr^Af curves are not parallel to each other. Therefore, in Crystals III and IV, Δσ and ΔT_h depend, respectively, on the test temperature and external stresses. The physical reason for the stronger dependence of the Δσ and ΔT_h, respectively, on the test temperature and external stresses in Crystals III and IV as compared to Crystals I and II requires additional structural studies.

A comparison of Crystals I and III with Crystals II and IV reveals a number of differences. Firstly, the values of ε_tr and dε_tr/dσ in Crystals III and IV differ significantly (Figure 6 and Figure 7). At a stress level of 300 MPa, in case of Crystals IV, ε_tr reaches 3.3%, whereas ε_tr reaches 1.4% in Crystals III. The value of ε_tr in these crystals is limited by their destruction. In the [001]-oriented FeNiCoAlX crystals (X = Ta, Nb, Ti), containing only the γ′-phase particles, ε_tr = 5.5–8.7% in experiments relating to cooling/heating under constant stress [12,18] and up to 13.5% in experiments on the SE study [5,27]. Similar results were obtained for Crystals I and II (Figure 6 and Figure 7). Therefore, as studies of the SE and SME in single crystals containing particles of the γ′- and β-phases have shown, the particles of the β-phase lead to a decrease in the plasticity associated with the stress induced γ–α′ MT.

Secondly, among Crystals I–IV at the level of constant stress of σ_cr = 300–350 MPa, high values of ΔM and ΔA were found, which reached 150 and 190 K; 110 and 140 K; 150 and 190 K; and 125 and 200 K, respectively, for Crystals I, II, III and IV. At the maximum level of constant stress of σ_cr = 300–350 MPa, the values of thermal hysteresis, ΔT_h, in Crystals I, II, III and IV are equal to 150 K, 50 K, 50 K and 100 K, respectively. In all studied crystals on the ε_tr(T) curves under constant stress, the A_s temperature is lower than the M_s temperature (Figure 6). Therefore, such MTs under constant stress are related to the thermoelastic MT of the second type [41]. This indicates that in the [001]-oriented FeNiCoAlNb crystals, with particles of the γ′- and β-phases, a high level of ΔG_el accumulates at the γ–α′ MT under stress. These results confirm the aforementioned conclusions regarding the second type of γ–α′ MT, obtained in the study of the temperature dependence of ρ(T) (Figure 1) and SE (Figure 3 and Figure 5).

Thirdly, ageing under stress leads to the formation of internal oriented stress fields <σ_G>, which is confirmed by an increase in the M_s temperature in Crystals II and IV as compared to Crystals I and III with the same ageing time at the second step. The source of these internal stress fields <σ_G> = 35–57 MPa are dislocations arising at the “matrix–β-phase” interface during ageing under stress (Figure 2e). Earlier, in the [001]-oriented crystals of another Fe–28Ni–17Co–11.5Al–2.5(TiNb) (at.%) (FeNiCoAlNbTi) alloys, after ageing under stress, as in Crystals II and also containing particles of γ′- and β-phases, similar values of ΔM_s = 15 K and <σ_G> = 65 MPa were found [51].

Thus, the development of thermoelastic γ–α′ MT in the [001]-oriented FeNiCoAlX crystals (X = Ta, Nb, Ti) occurs in a disordered fcc high-temperature phase, in which the nanosized γ′-phase particles with a size d = 3–12 nm are precipitated. The fundamental role of the γ′-phase particles is that they act as “memory elements”, which determine the restoration of the initial orientation of austenite and the realization of the fact that reverse α′–γ MT is “exactly backward” [1,2,4,5,28].

The γ′-phase particles have coherent conjugation with the high-temperature phase and retain coherence with the α′-martensite. With d > d_cr at γ–α′ MT, there is a loss of coherence of the γ′-phase with α′-martensite and relaxation of the elastic energy, ΔG_el, stored during the MT. As a result, the transformation becomes non-thermoelastic [1,2]. In relation to Crystals I–IV, containing particles of γ′- and β-phases, the γ–α′ MT is not suppressed by the presence of the β-phase particles. In contrast to the γ′-phase particles, the β-phase particles have non-coherent conjugation with the γ-phase and with α′-martensite. This leads to a decrease in plasticity and, accordingly, to a decrease in the value of SE and SME at MT under stress. Non-coherent β-phase particles act as preferential sites for the nucleation of α′-martensite, which grows in the regions between the β-phase. The high values of Θ = dσ/dε, due to MT in these crystals, which are characteristic of the development of deformation by slip and twinning [48,49,50], indicate an increase in ΔG_el with an increase in the volume fraction of α′-martensite.

The combination of a high level of ΔGel and small values of ΔGdis creates conditions for the development of SE within the temperature range from Ms to Af, which are not usually observed during the development of thermoelastic MTs. Ageing under stress at the second step leads to an increase in Ms temperature, compared to ageing without stress, which is due to the appearance of the internal oriented stress fields, <σG>. To elucidate the mechanism of <σG> formation, additional studies are required, in which it will be necessary to change the ageing conditions at the second step, in order to amend the size and volume fraction of the β-phase particles. This will facilitate the creation of unique composite materials, with a simultaneous precipitation of γ′- and β-phase particles, high transformation strain values and the development interval of the γ–α′ MT within the high-temperature region due to a decrease in nickel in the fcc high-temperature phase, as a result of the precipitation of the β-phase particles.

In the present paper, it is shown that the maximum values of the transformation strain, ε_tr, obtained in experiments when studying the SE and SME during cooling/heating under constant stress, are equal to 4 and 3.4%, respectively. Theoretical estimates of ε_tr, carried out in [5,12], show that the value of ε_tr for the [001]-oriented crystals under tension decreases from 8.3 to 5.5% with an increase in the tetragonality of martensite c/a from 1.1 to 1.15%. This estimate assumes that the γ–α′ MT occurs in the alloy without the γ′-phase particles or when these particles undergo elastic deformation together with the fcc matrix. The experimental values of the SME and SE under tension along the [001] direction vary from 14.5 to 5.5% for the γ′-phase particle sizes of 3 nm and 8–12 nm [5,12,18,26,27]. The non-coherent β-phase particles, the sizes of which are much larger than the γ′-phase particles, cannot accommodate transformation strain in the matrix due to elastic deformation and contribute to the generation of multiple variants of martensite from the “matrix-β-phase” interface [46,47]. As a result, ε_tr significantly decreases to between 3.4 and 4% and the determination of the total resource of ε_tr is limited by the low plasticity of Crystals I–IV due to the effect of the β-phase on transformation plasticity.

4. Conclusions

The studies of development of the thermoelastic γ–α′ MT under stress using [001]-oriented FeNiCoAlNb single crystals, containing non-coherent β-phase particles after ageing under and without stress, showed that the effect of the β-phase particles on SME and SE in single crystals and polycrystals of FeNiCoAlX alloys (X = Ta, Nb, Ti) is fundamentally different. Based on the results of the SME and SE studies, crystal structure after ageing under and without stresses and their analysis, the following conclusions can be summarized as the main findings:

• A new two-step ageing has been proposed for the FeNiCoAlNb single crystals, oriented along the [001]-direction for tensile strain, including ageing at the first step at 973 K for 5 h without stress and at the second step, at 873 K for 2 and 4 h without and under tensile stress at 120 MPa. At the first step of ageing at 973 K for 5 h, particles of the γ′-phase are precipitated, and at the second low-temperature step at 873 K for 2 and 4 h, a structure, consisting of particles of the γ′- and β-phases is formed.
• An increase in the ageing time at the second low-temperature step from 2 to 4 h leads to an increase in the M_s temperature, which is associated with a decrease in the nickel concentration in the matrix due to an increase in the volume fraction of the nickel-rich β-phase particles with the composition, (FeNiCo)(AlNb).
• Ageing under a tensile stress of 120 MPa at the second low-temperature step for 2 and 4 h leads to an increase in the M_s temperature, in comparison with ageing without stress by 11–15 K, which is associated with the generation of internal oriented tensile stresses during ageing under stress.
• In a structure containing particles of the γ′- and β-phases, conditions are created for observing SE within the temperature range from M_s to A_f due to the formation of a high level of accumulated elastic energy, ΔG_el, which significantly exceeds the value of the dissipated energy, ΔG_dis.