Numerical Study of Coupled Electrical-Thermal-Mechanical-Wear Behavior in Electrical Contacts
Abstract
1. Introduction
2. Electrical-Thermal-Mechanical-Wear Coupling Model
2.1. Current Conservation Model
2.2. Transient Heat Transfer Model
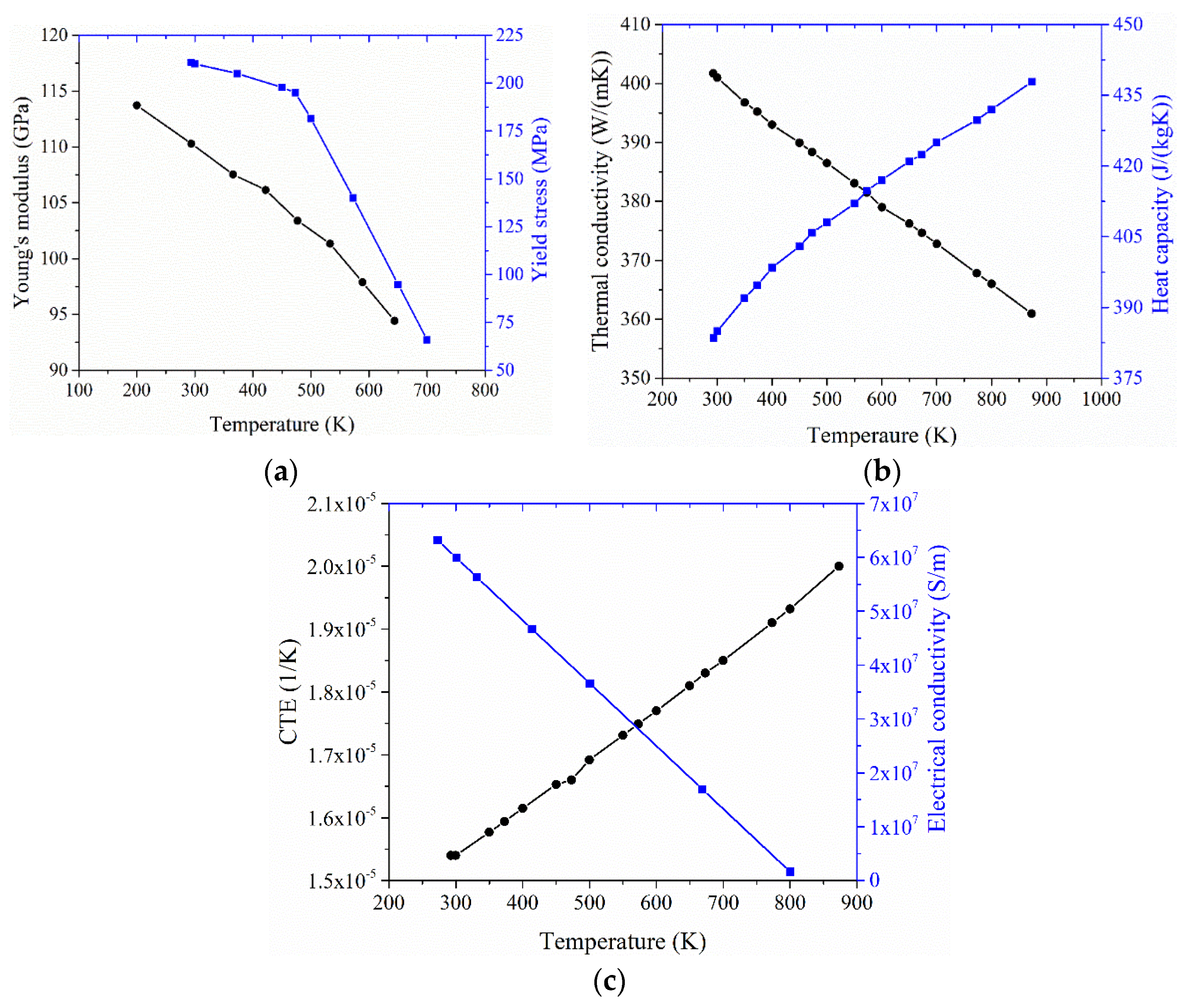
2.3. Material Constitutive Model
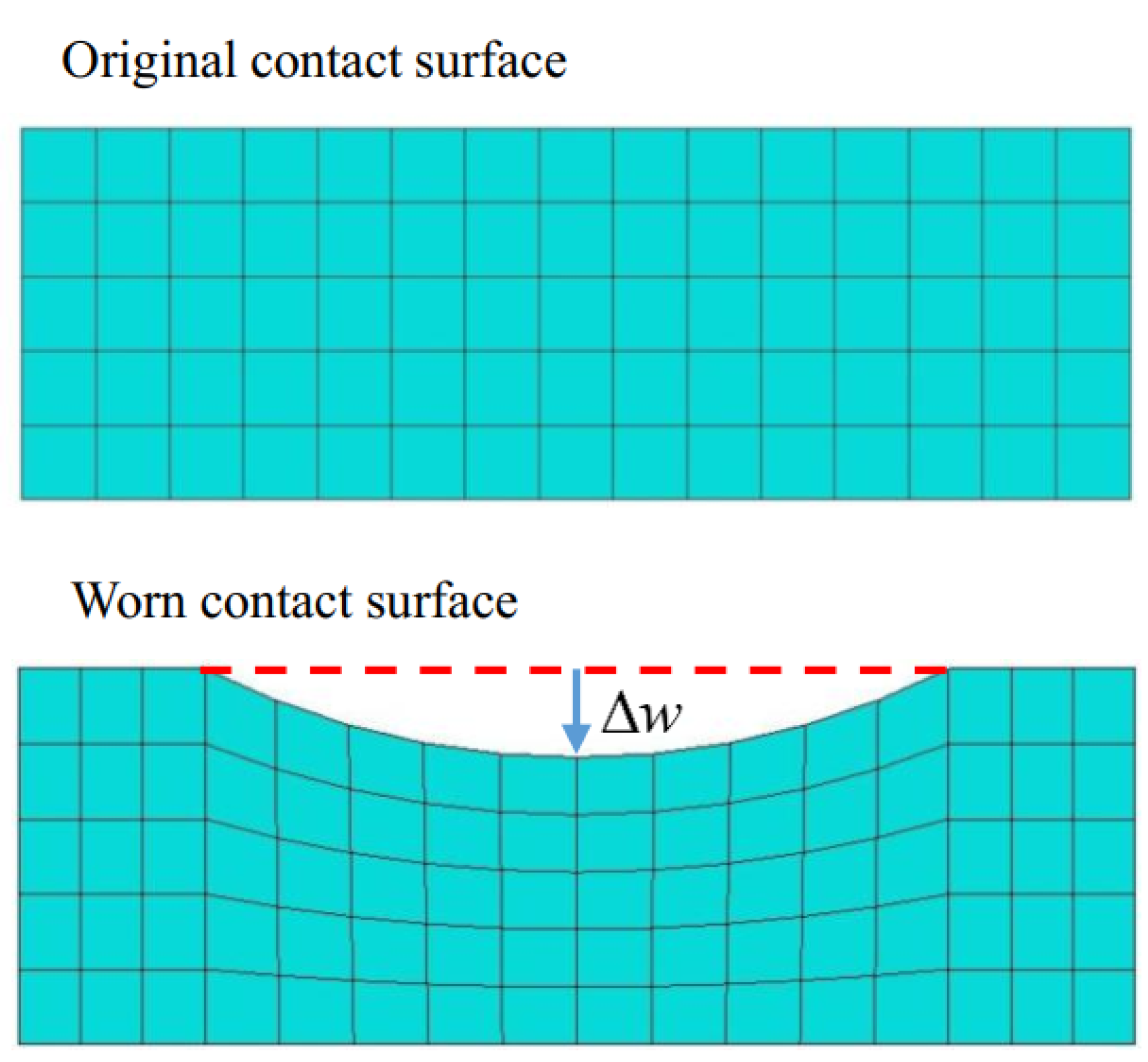
2.4. Frictional Energy-Based Wear Model
3. Computational Method
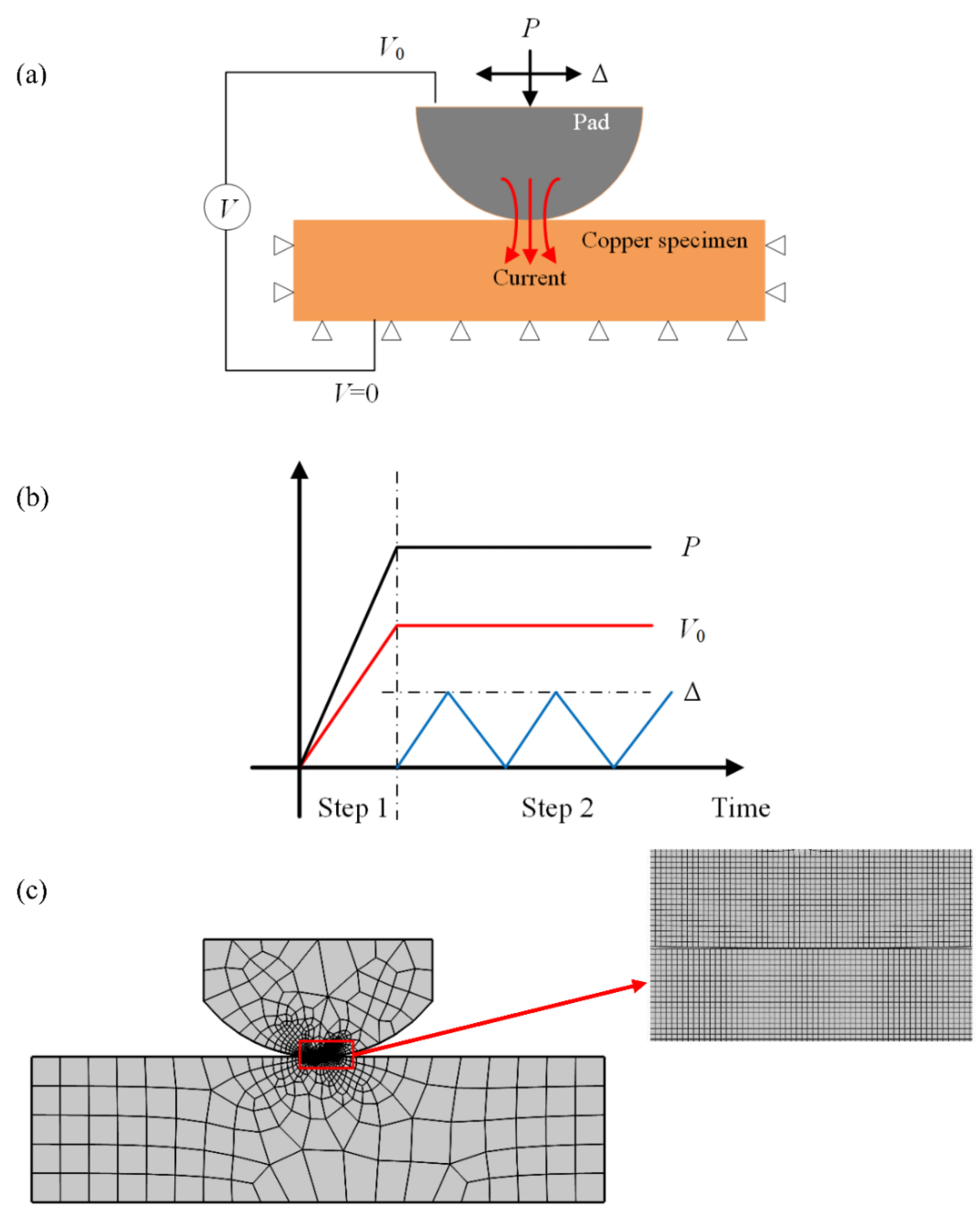
3.1. Fully Coupled Algorithm
3.2. Finite Element Modeling of Electrical Contact
4. Results and Discussion
4.1. Model Validation
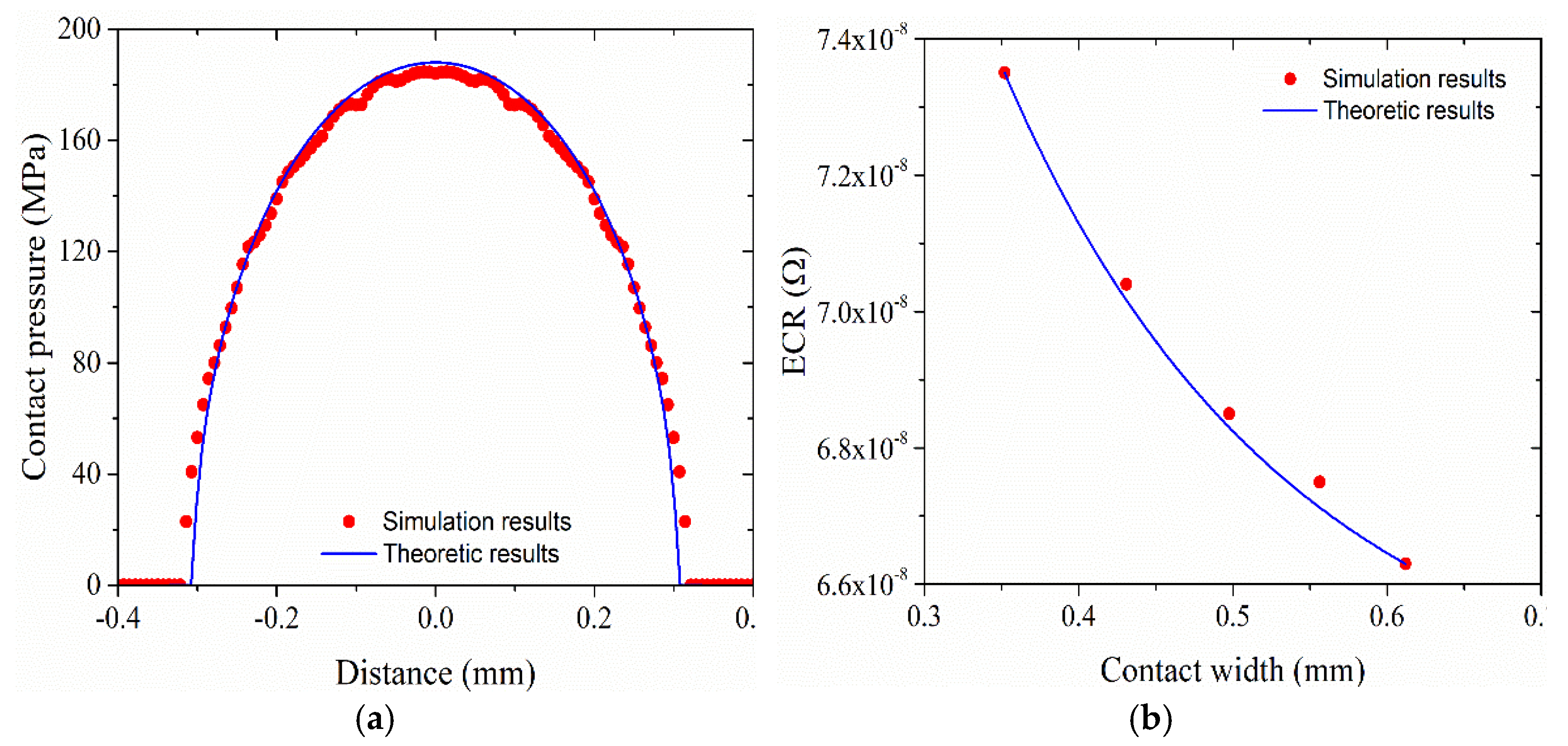
4.1.1. Comparison with Theoretic Results of Contact Pressure and ECR
4.1.2. Comparison with Experimental Fretting Wear Profile
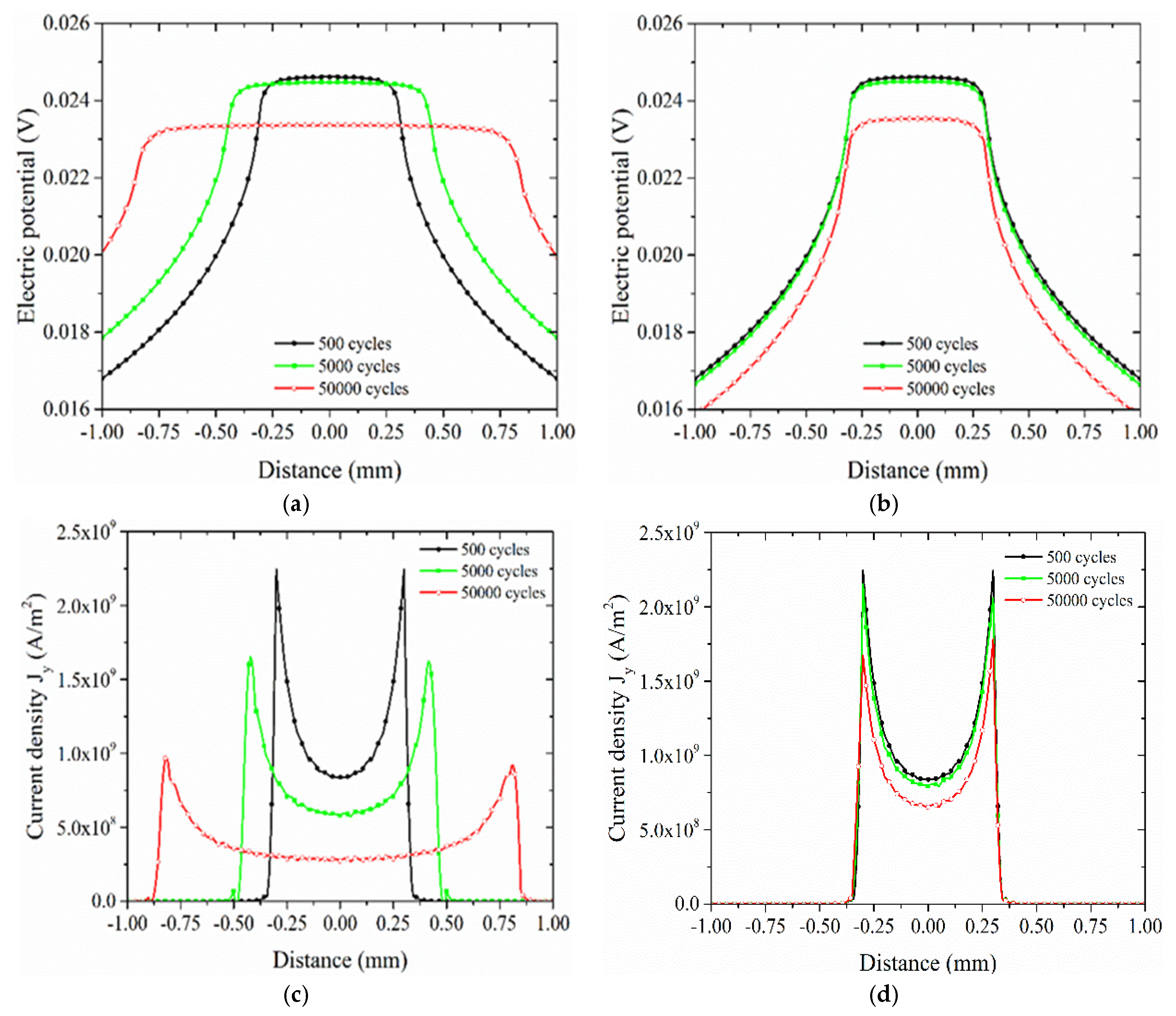
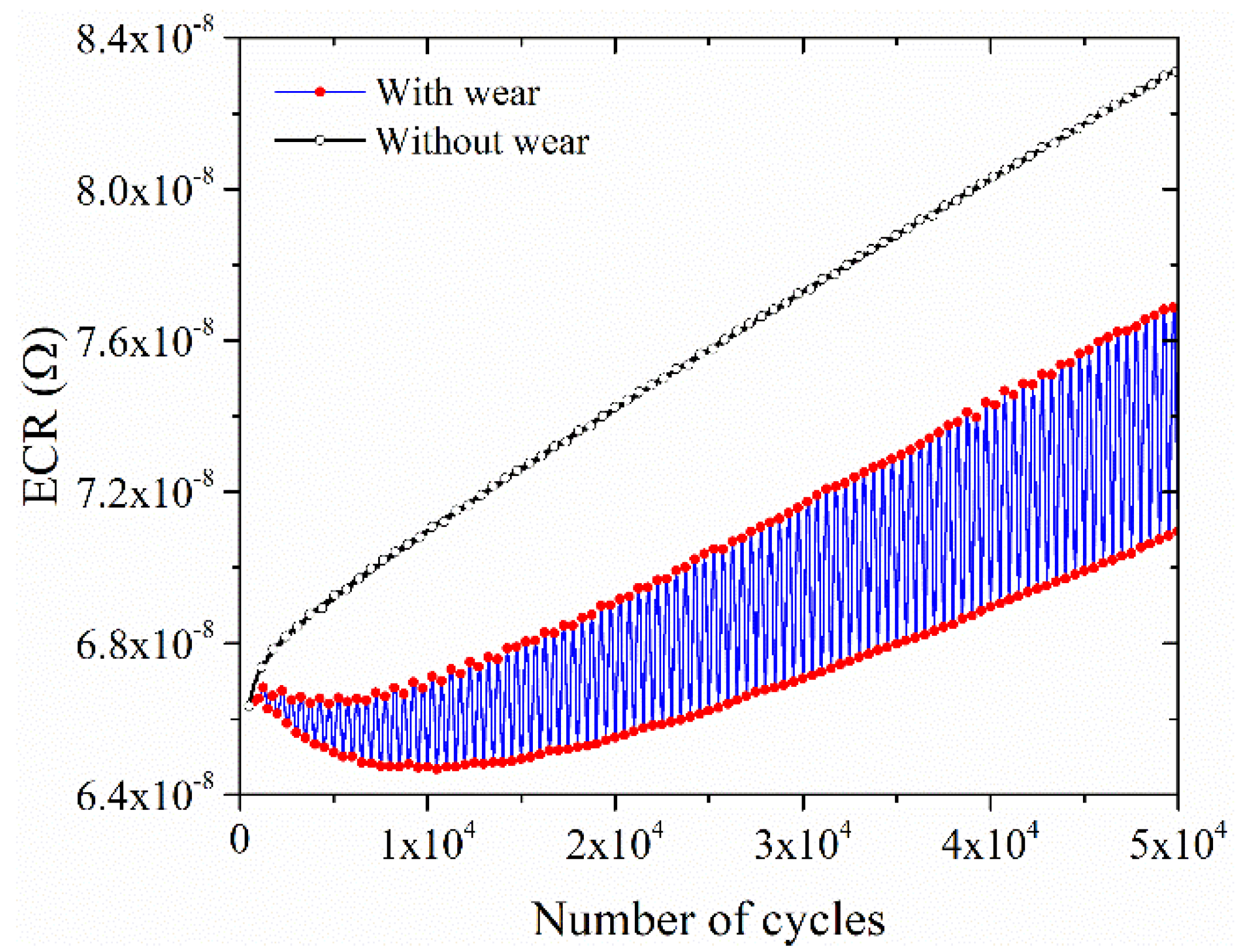
4.2. Wear Profile, Contact Pressure, Temperature, and Electric Potential
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Holm, R. Electric Contacts: Theory and Application; Springer Science & Business Media: New York, NY, USA, 2013. [Google Scholar]
- Zuev, L.B.; Danilov, V.I.; Konovalov, S.V.; Filip’ev, R.A.; Gromov, V.E. Influence of contact potential difference and electric potential on the microhardness of metals. Phys. Solid State 2009, 51, 1137–1141. [Google Scholar] [CrossRef]
- Orlova, D.V.; Danilov, V.I.; Zuev, L.B.; Staskevich, O.S. Effect of low electrical potentials on the microhardness of metallic materials. Phys. Solid State 2016, 58, 9–11. [Google Scholar] [CrossRef]
- Slade, P.G. Electrical Contacts: Principles and Applications; CRC Press: London, UK, 2017. [Google Scholar]
- Hannel, S.; Fouvry, S.; Kapsa, P.; Vincent, L. The fretting sliding transition as a criterion for electrical contact performance. Wear 2001, 249, 761–770. [Google Scholar] [CrossRef]
- Maruschak, P.O.; Panin, S.V.; Zakiev, I.M.; Poltaranin, M.A.; Sotnikov, A.L. Scale levels of damage to the raceway of a spherical roller bearing. Eng. Fail. Anal. 2016, 59, 69–78. [Google Scholar] [CrossRef]
- Ito, T.; Sawada, S.; Hattori, Y.; Saitoh, Y.; Tamai, T.; Iida, K. Microscopy study of fretting corrosion caused by the tin plating thickness. In Proceedings of the 2008 54th IEEE Holm Conference on Electrical Contacts, Orlando, FL, USA, 27–29 October 2008; pp. 294–300. [Google Scholar]
- Gagnon, D.; Braunovic, M.; Masounave, J. Effect of fretting slip amplitude on the friction behaviour of electrical contact materials. In Proceedings of the Fifty-First IEEE Holm Conference on Electrical Contacts, Chicago, IL, USA, 26–28 September 2005; pp. 186–195. [Google Scholar]
- Heaton, C.E.; McCarthy, S.L. High cycle fretting corrosion studies on tin-coated contact materials. In Proceedings of the Forth-Seventh IEEE Holm Conference on Electrical Contacts (IEEE Cat. No. 01CH37192), Montreal, QC, Canada, 12 September 2001; pp. 209–214. [Google Scholar]
- Liu, X.-L.; Cai, Z.-B.; Cui, Y.; Liu, S.-B.; Xu, X.-J.; Zhu, M.-H. Effect of different atmospheres on the electrical contact performance of electronic components under fretting wear. J. Phys. D Appl. Phys. 2018, 51. [Google Scholar] [CrossRef]
- Liu, X.-L.; Cai, Z.-b.; He, J.-f.; Peng, J.-f.; Zhu, M.-h. Effect of elevated temperature on fretting wear under electric contact. Wear 2017, 376–377, 643–655. [Google Scholar] [CrossRef]
- Wang, P.; Yue, W.; Lu, Z.; Zhang, G.; Zhu, L. Friction and wear properties of MoS2-based coatings sliding against Cu and Al under electric current. Tribol. Int. 2018, 127, 379–388. [Google Scholar] [CrossRef]
- Fouvry, S.; Jedrzejczyk, P.; Chalandon, P. Introduction of an exponential formulation to quantify the electrical endurance of micro-contacts enduring fretting wear: Application to Sn, Ag and Au coatings. Wear 2011, 271, 1524–1534. [Google Scholar] [CrossRef]
- Noh, H.J.; Kim, J.W.; Lee, S.M.; Jang, H. Effect of grain size on the electrical failure of copper contacts in fretting motion. Tribol. Int. 2017, 111, 39–45. [Google Scholar] [CrossRef]
- Ren, W.; Wang, P.; Song, J.; Zhai, G. Effects of current load on wear and fretting corrosion of gold-plated electrical contacts. Tribol. Int. 2014, 70, 75–82. [Google Scholar] [CrossRef]
- He, L.-P.; Cai, Z.-B.; Peng, J.-F.; Deng, W.-L.; Li, Y.; Yang, L.-Y.; Zhu, M.-H. Effects of oxidation layer and roughness on the fretting wear behavior of copper under electrical contact. Mater. Res. Express 2020, 6. [Google Scholar] [CrossRef]
- Weißenfels, C.; Wriggers, P. Numerical modeling of electrical contacts. Comput. Mech. 2009, 46, 301–314. [Google Scholar] [CrossRef]
- Ren, W.; Wei, J.; Meng, X.; Zhi, H. Numerical Simulation of Temperature–Voltage Relation in Electrical Contacts and Correction of Classical Kohlrausch’s Equation. IEEE Trans. Electron Devices 2016, 63, 1217–1224. [Google Scholar] [CrossRef]
- Terhorst, M.; Ozhoga-Maslovskaja, O.; Trauth, D.; Shirobokov, A.; Mattfeld, P.; Solf, M.; Klocke, F. Electro-thermo-mechanical contact model for bulk metal forming under application of electrical resistance heating. Int. J. Adv. Manuf. Technol. 2016, 89, 3601–3618. [Google Scholar] [CrossRef]
- Jiang, X.; Pan, F.; Shao, G.; Huang, J.; Hong, J.; Zhou, A. Prediction of electrical contact endurance subject to micro-slip wear using friction energy dissipation approach. Friction 2018, 7, 537–550. [Google Scholar] [CrossRef]
- Archard, J.F. Contact and Rubbing of Flat Surfaces. J. Appl. Phys. 1953, 24, 981–988. [Google Scholar] [CrossRef]
- McColl, I.R.; Ding, J.; Leen, S.B. Finite element simulation and experimental validation of fretting wear. Wear 2004, 256, 1114–1127. [Google Scholar] [CrossRef]
- Ding, J.; McColl, I.R.; Leen, S.B.; Shipway, P.H. A finite element based approach to simulating the effects of debris on fretting wear. Wear 2007, 263, 481–491. [Google Scholar] [CrossRef]
- Madge, J.J.; Leen, S.B.; Shipway, P.H. The critical role of fretting wear in the analysis of fretting fatigue. Wear 2007, 263, 542–551. [Google Scholar] [CrossRef]
- Paulin, C.; Fouvry, S.; Meunier, C. Finite element modelling of fretting wear surface evolution: Application to a Ti–6A1–4V contact. Wear 2008, 264, 26–36. [Google Scholar] [CrossRef]
- Fouvry, S.; Paulin, C.; Liskiewicz, T. Application of an energy wear approach to quantify fretting contact durability: Introduction of a wear energy capacity concept. Tribol. Int. 2007, 40, 1428–1440. [Google Scholar] [CrossRef]
- Arnaud, P.; Fouvry, S. A dynamical FEA fretting wear modeling taking into account the evolution of debris layer. Wear 2018, 412–413, 92–108. [Google Scholar] [CrossRef]
- Zhang, T.; McHugh, P.E.; Leen, S.B. Computational study on the effect of contact geometry on fretting behaviour. Wear 2011, 271, 1462–1480. [Google Scholar] [CrossRef]
- Shen, F.; Hu, W.; Meng, Q. A damage mechanics approach to fretting fatigue life prediction with consideration of elastic–plastic damage model and wear. Tribol. Int. 2015, 82, 176–190. [Google Scholar] [CrossRef]
- Shen, F.; Hu, W.; Voyiadjis, G.Z.; Meng, Q. Effects of fatigue damage and wear on fretting fatigue under partial slip condition. Wear 2015, 338–339, 394–405. [Google Scholar] [CrossRef]
- Shen, F.; Hu, W.; Meng, Q. A non-local approach based on the hypothesis of damage dissipation potential equivalence to the effect of stress gradient in fretting fatigue. Int. J. Fatigue 2016, 90, 125–138. [Google Scholar] [CrossRef]
- Cooper, M.G.; Mikic, B.B.; Yovanovich, M.M. Thermal contact conductance. Int. J. Heat Mass Transf. 1969, 12, 279–300. [Google Scholar] [CrossRef]
- Shen, F.; Zhou, K. Investigation on thermal response in fretting sliding with the consideration of plastic dissipation, surface roughness and wear. Int. J. Mech. Sci. 2018, 148, 94–102. [Google Scholar] [CrossRef]
- Paulin, C.; Fouvry, S.; Deyber, S. Wear kinetics of Ti–6Al–4V under constant and variable fretting sliding conditions. Wear 2005, 259, 292–299. [Google Scholar] [CrossRef]
- Fallahnezhad, K.; Oskouei, R.H.; Taylor, M. Development of a fretting corrosion model for metallic interfaces using adaptive finite element analysis. Finite Elem. Anal. Des. 2018, 148, 38–47. [Google Scholar] [CrossRef]
- El-Tayeb, N. The variation of hardness and wear coefficient in sliding wear of copper and aluminum alloys. Wear 1994, 174, 63–69. [Google Scholar] [CrossRef]
- Davis, J.R. Copper and Copper Alloys; ASM International: Novelty, OH, USA, 2001. [Google Scholar]
- Aichi, H. Analysis on the constriction resistance of the electric contact by the contact model using the electrolyte bath. In Proceedings of the 17th International Conference on Electrical Contacts, Nagoya, Japan, 4–9 July 1994; pp. 1–6. [Google Scholar]
- Liu, X.-L.; Cai, Z.-B.; Xiao, Q.; Shen, M.-X.; Yang, W.-B.; Chen, D.-Y. Fretting wear behavior of brass/copper-graphite composites as a contactor material under electrical contact. Int. J. Mech. Sci. 2020, 184. [Google Scholar] [CrossRef]
- Grandin, M.; Wiklund, U. Wear phenomena and tribofilm formation of copper/copper-graphite sliding electrical contact materials. Wear 2018, 398–399, 227–235. [Google Scholar] [CrossRef]
- Sung, I.H.; Kim, J.W.; Noh, H.J.; Jang, H. Effect of displacement and humidity on contact resistance of copper electrical contacts. Tribol. Int. 2016, 95, 256–261. [Google Scholar] [CrossRef]
- Zhao, H.; Feng, Y.; Zhou, Z.; Qian, G.; Zhang, J.; Huang, X.; Zhang, X. Effect of electrical current density, apparent contact pressure, and sliding velocity on the electrical sliding wear behavior of Cu–Ti3AlC2 composites. Wear 2020, 444–445. [Google Scholar] [CrossRef]







| Damage Type | Possible Causes and Typical Characteristics |
|---|---|
| Abrasive wear | Formation of grooves caused by plowing as hard asperities slide across a softer surface |
| Adhesive wear | Plastic deformation, material detachment and transfer between contacting parts |
| Fatigue cracks | Fatigue cracks initiation and propagation on the contact surfaces due to the reciprocating motion |
| Chemical corrosion | Oxidation and electrochemical corrosion on the metallic material surfaces |
| Flash temperature | High interfacial temperature due to the electrical contact resistance and the friction energy |
| Arc erosion | Arc erosion holes and a splash of molten droplets caused by high arc temperature under the high-voltage condition |
| Parameter | Value |
|---|---|
| Cyclic horizontal displacement, (μm) | 100 |
| Normal force, (N/mm) | 30, 45, 60, 75 and 90 |
| Electric potential, (mV) | 50 |
| Coefficient of friction | 0.75 |
| Average height of asperities, (μm) | 3.67 |
| Average slope of asperities, | 0.4 |
| Wear coefficient, (MPa−1) | |
| Ambient temperature, (K) | 293 |
| Heat convection coefficient, (W/(m2K)) | 10 |
| Normal Force, (N/mm) | Contact Width, (mm) | Purpose | |
|---|---|---|---|
| 30 | 0.352 | 0.0735 | |
| 90 | 0.612 | 0.0663 | |
| 45 | 0.431 | 0.0704 | Compare with theoretical results |
| 60 | 0.498 | 0.0685 | |
| 75 | 0.556 | 0.0675 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shen, F.; Ke, L.-L. Numerical Study of Coupled Electrical-Thermal-Mechanical-Wear Behavior in Electrical Contacts. Metals 2021, 11, 955. https://doi.org/10.3390/met11060955
Shen F, Ke L-L. Numerical Study of Coupled Electrical-Thermal-Mechanical-Wear Behavior in Electrical Contacts. Metals. 2021; 11(6):955. https://doi.org/10.3390/met11060955
Chicago/Turabian StyleShen, Fei, and Liao-Liang Ke. 2021. "Numerical Study of Coupled Electrical-Thermal-Mechanical-Wear Behavior in Electrical Contacts" Metals 11, no. 6: 955. https://doi.org/10.3390/met11060955
APA StyleShen, F., & Ke, L.-L. (2021). Numerical Study of Coupled Electrical-Thermal-Mechanical-Wear Behavior in Electrical Contacts. Metals, 11(6), 955. https://doi.org/10.3390/met11060955





