Experimental and Numerical Study on the Protective Behavior of Weldox 900 E Steel Plates Impacted by Blunt-Nosed Projectiles
Abstract
:1. Introduction
2. Experimental Overview and Results
2.1. Experimental Set-Up
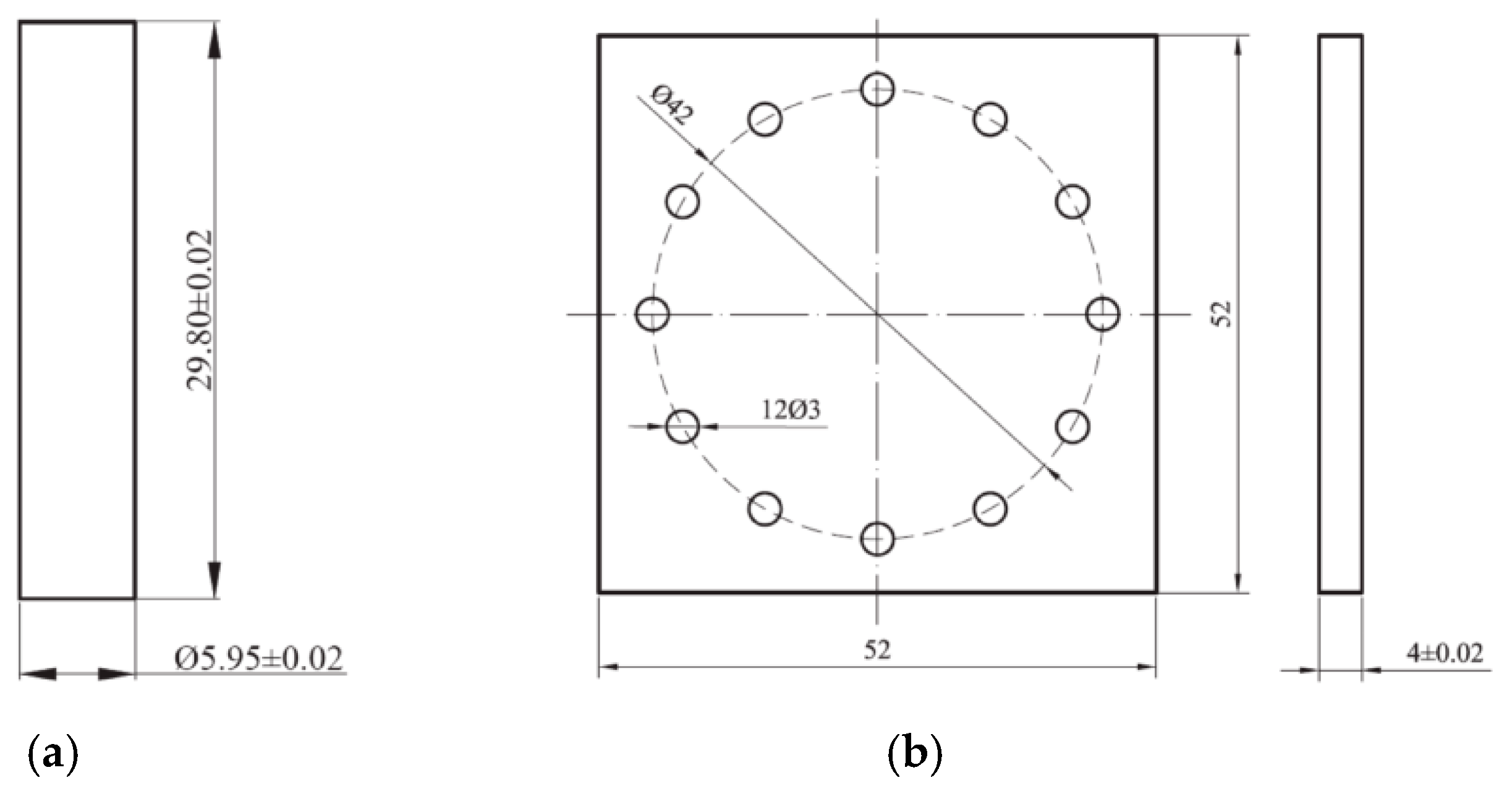
2.2. Targets and Projectiles
2.3. Experimental Results
3. Numerical Model
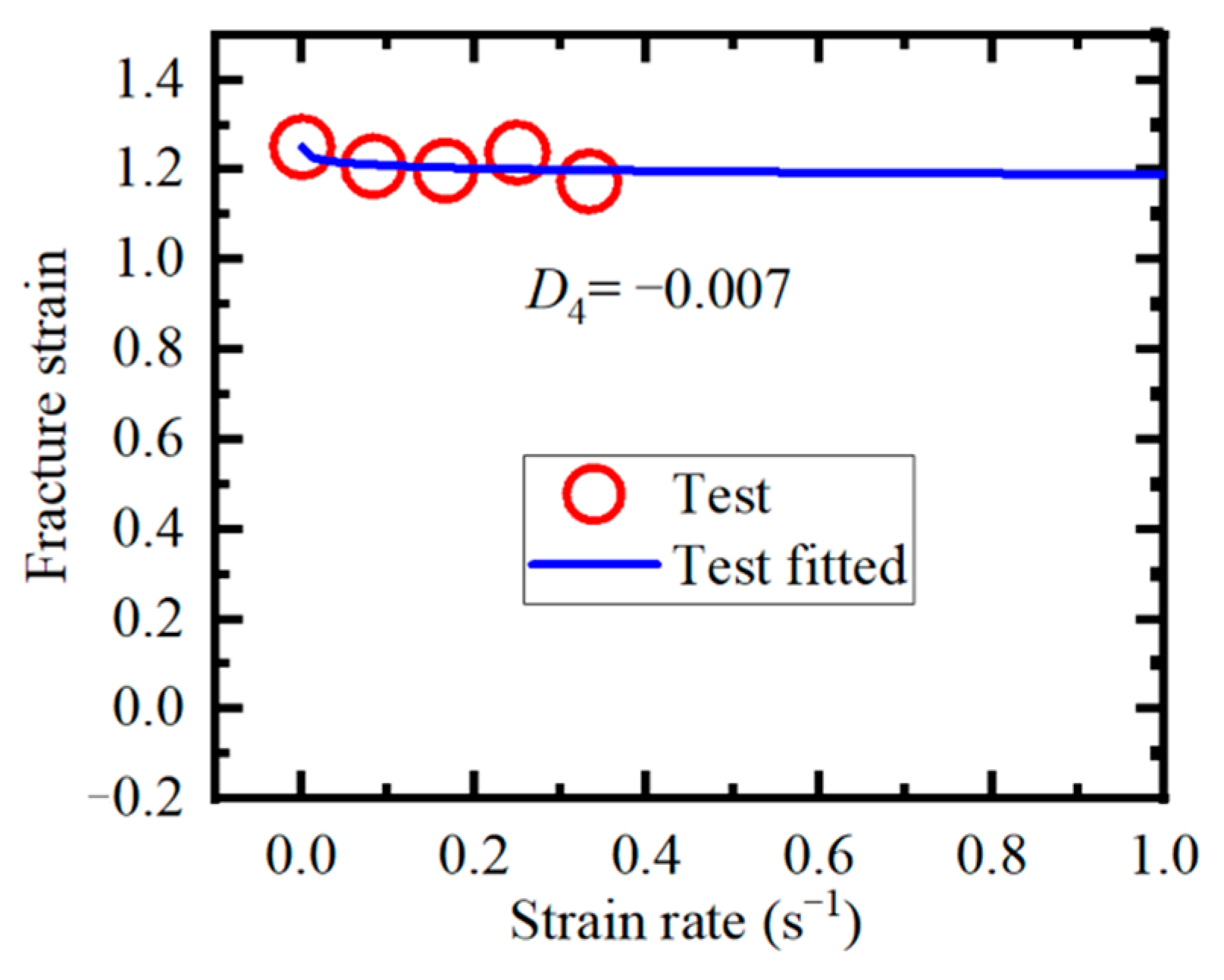
3.1. Material Model
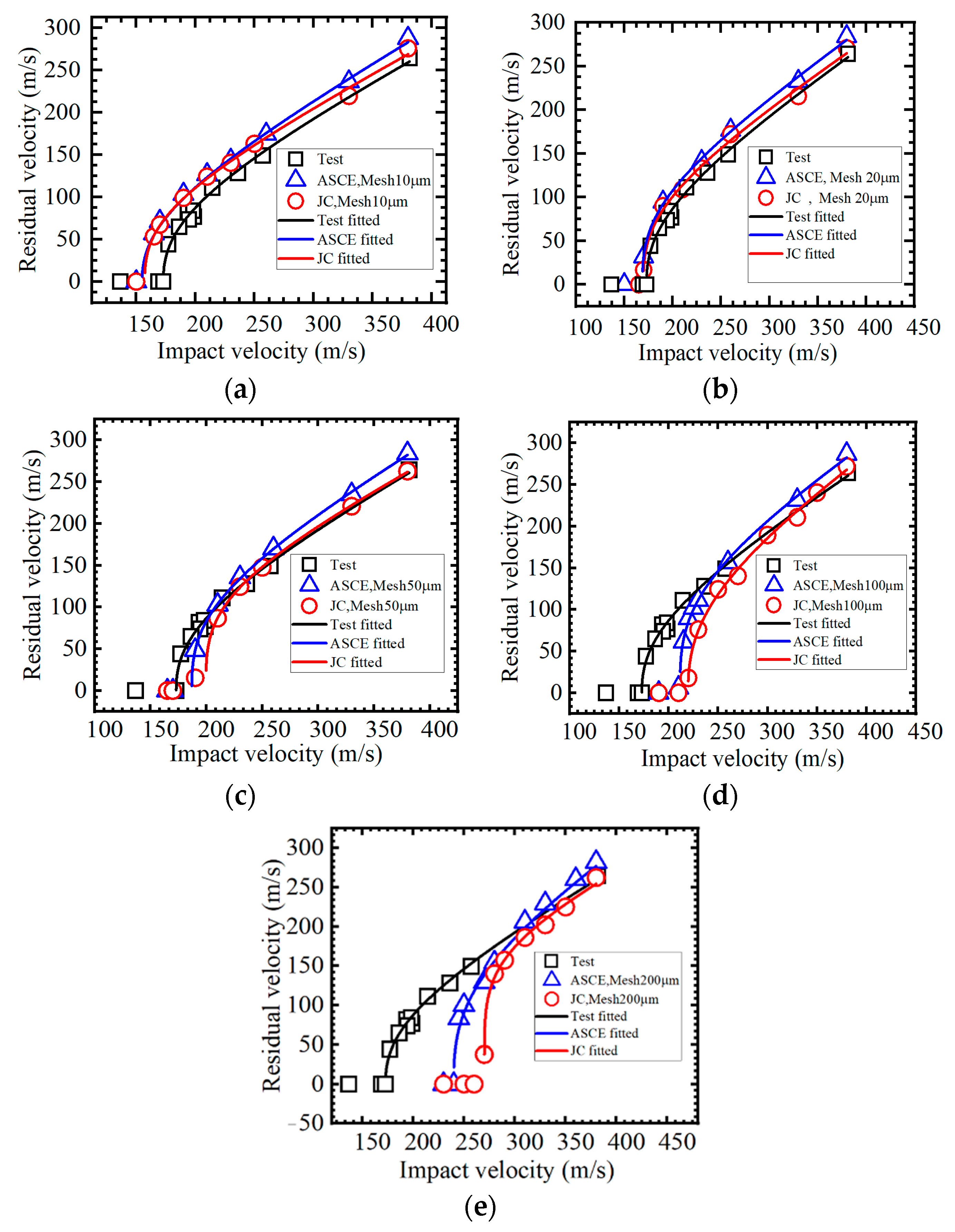
3.2. Numerical Model and Simulation Results
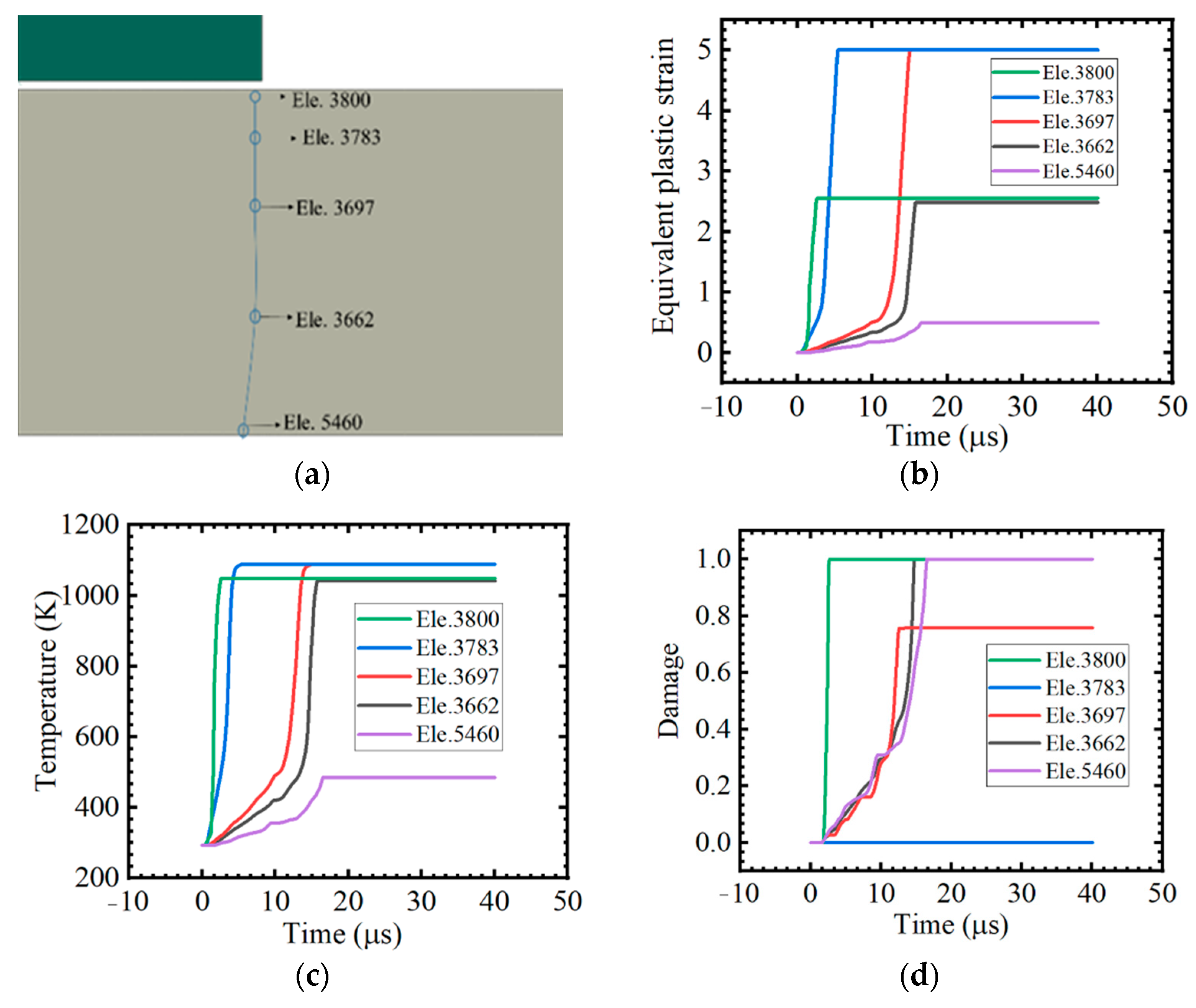
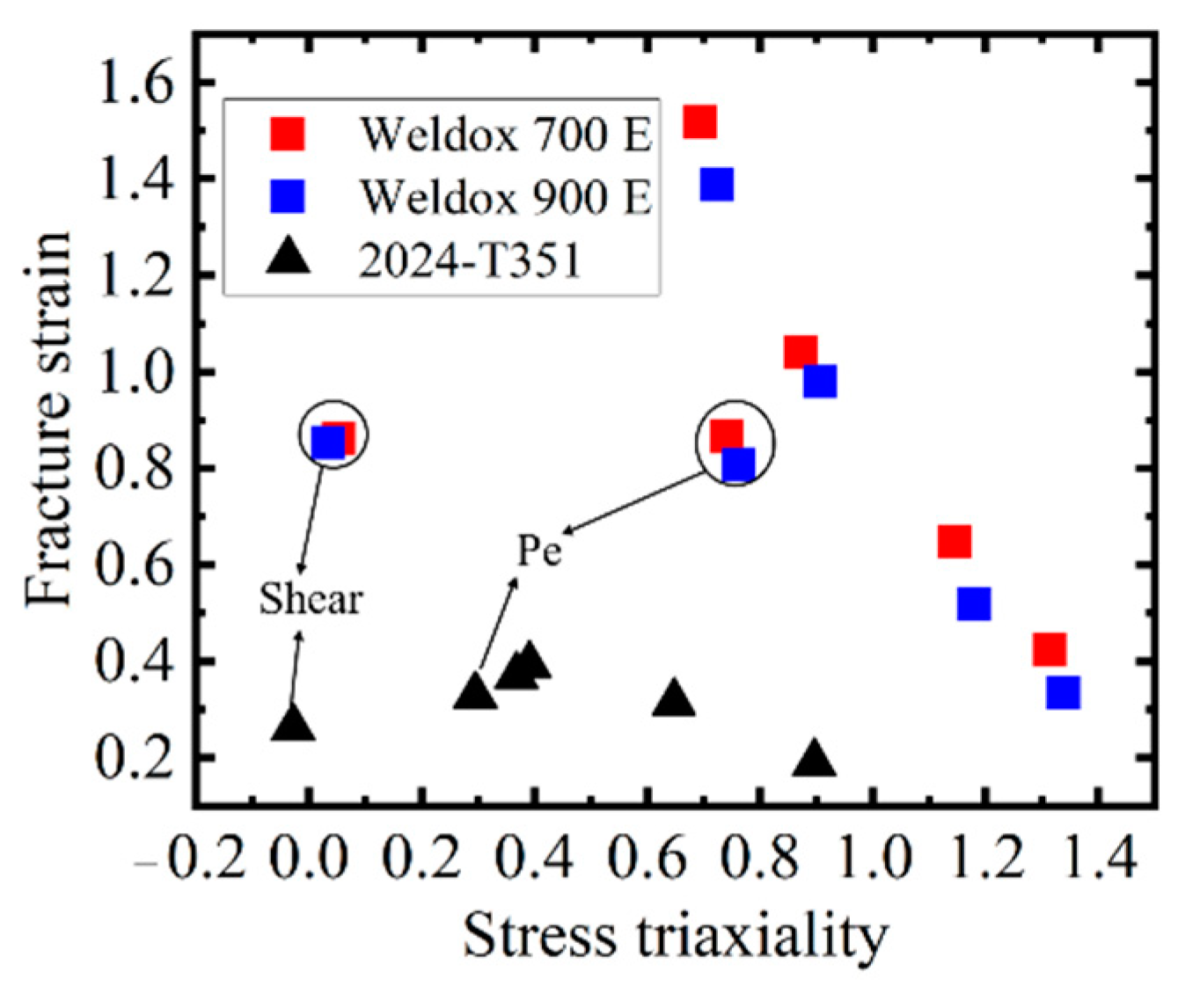
4. Discussion
5. Conclusions
- The fracture locus of Weldox 900 E high-strength steel is obviously related to Lode angle;
- The introduction of Lode parameter into the fracture criterion does not improve the prediction accuracy of the BLV. Additionally, the BLV of the Weldox 900 E high-strength steel has a high mesh size sensitivity;
- For Weldox 900 E high-strength steel, the adiabatic temperature rise effect is very obvious in ballistic tests. This rapid temperature rise will seriously soften the elements on the fracture path, resulting in larger plastic strains, which will cover the influence mechanism of the Lode parameter on fracture strain.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Moćko, W.; Janiszewski, J.; Radziejewska, J.; Grązka, M. Analysis of deformation history and damage initiation for 6082-T6 aluminium alloy loaded at classic and symmetric Taylor impact test conditions. Int. J. Impact Eng. 2015, 75, 203–213. [Google Scholar] [CrossRef]
- Dey, S.; Børvik, T.; Hopperstad, O.; Leinum, J.; Langseth, M. The effect of target strength on the perforation of steel plates using three different projectile nose shapes. Int. J. Impact Eng. 2004, 30, 1005–1038. [Google Scholar] [CrossRef]
- Balos, S.; Howard, D.; Brezulianu, A.; Zlatanović, D.L. Perforated Plate for Ballistic Protection—A Review. Metals 2021, 11, 526. [Google Scholar] [CrossRef]
- Zochowski, P.; Bajkowski, M.; Grygoruk, R.; Magier, M.; Burian, W.; Pyka, D.; Bocian, M.; Jamroziak, K. Ballistic Impact Resistance of Bulletproof Vest Inserts Containing Printed Titanium Structures. Metals 2021, 11, 225. [Google Scholar] [CrossRef]
- Børvik, T.; Langseth, M.; Hopperstad, O.; Malo, K.A. Ballistic penetration of steel plates. Int. J. Impact Eng. 1999, 22, 855–886. [Google Scholar] [CrossRef]
- Dey, S.; Børvik, T.; Hopperstad, O.; Langseth, M. On the influence of fracture criterion in projectile impact of steel plates. Comput. Mater. Sci. 2006, 38, 176–191. [Google Scholar] [CrossRef]
- Zhang, W.; Xiao, X.K.; Guo, Z.T.; Mu, Z.C.; Wei, G. Investigation on the Ballistic Resistance of Double-Layered A3 Steel Targets Against Blunt Projectile Impact. Chin. J. High Press. Phys. 2012, 26, 163–170. (In Chinese) [Google Scholar] [CrossRef]
- Rodríguez-Millán, M.; Díaz-Álvarez, A.; Bernier, R.; Miguélez, M.H.; Loya, J.A.; Millán, R. Loya Experimental and Numerical Analysis of Conical Projectile Impact on Inconel 718 Plates. Metals 2019, 9, 638. [Google Scholar] [CrossRef] [Green Version]
- Sima, Y.Z.; Xiao, X.K.; Wang, Y.P.; Zhang, W. Tests and numerical simulation for anti- penetrating behavior of a high strength 7A04- T6 aluminium alloy plate against a blunt projectile’s impact. J. Vib. Shock. 2017, 36, 1–8. (In Chinese) [Google Scholar] [CrossRef]
- Xiao, X.; Zhang, W.; Wei, G.; Mu, Z.; Guo, Z. Experimental and numerical investigation on the deformation and failure behavior in the Taylor test. Mater. Des. 2011, 32, 2663–2674. [Google Scholar] [CrossRef]
- Xiao, X.; Pan, H.; Bai, Y.; Lou, Y.; Chen, L. Application of Lode dependent fracture criterion in predicting fracture of 6061-T6511H aluminium alloy Taylor rods. J. Vib. Shock. 2018, 37, 142–149. (In Chinese) [Google Scholar] [CrossRef]
- Zhang, W.; Xiao, X.K.; Wei, G. Constitutive relation and fracture model of 7A04 aluminum alloy. Explos. Shock. Waves 2011, 31, 81–87. (In Chinese) [Google Scholar]
- Zhang, W.; Xiao, X.-K.; Wei, G.; Guo, Z. Evaluation of five fracture models in Taylor impact fracture. Am. Phys. Soc. 2012, 1426, 1125–1128. [Google Scholar] [CrossRef] [Green Version]
- Xiao, X.K.; Mu, Z.C.; Pan, H.; Lou, Y.S. Effect of the Lode parameter in predicting shear cracking of 2024-T351 aluminum alloy Taylor rods. Int. J. Impact Eng. 2018, 120, 185–201. [Google Scholar] [CrossRef]
- Jia, Y.; Bai, Y. Ductile fracture prediction for metal sheets using all-strain-based anisotropic eMMC model. Int. J. Mech. Sci. 2016, 115–116, 516–531. [Google Scholar] [CrossRef]
- Pan, H.; Liu, J.; Choi, Y.; Xu, C.; Bai, Y.; Atkins, T. Zones of material separation in simulations of cutting. Int. J. Mech. Sci. 2016, 115–116, 262–279. [Google Scholar] [CrossRef]
- Shabahang, S.; Tao, G.; Kaufman, J.J.; Qiao, Y.; Wei, L.; Bouchenot, T.; Gordon, A.P.; Fink, Y.; Bai, Y.; Hoy, R.S.; et al. Controlled fragmentation of multimaterial fibers and films via polymer cold-drawing. Nature 2016, 534, 529–533. [Google Scholar] [CrossRef]
- Teng, X.; Wierzbicki, T.; Hiermaier, S.; Rohr, I. Numerical prediction of fracture in the Taylor test. Int. J. Solids Struct. 2005, 42, 2929–2948. [Google Scholar] [CrossRef]
- Teng, X.; Wierzbicki, T. Evaluation of six fracture models in high velocity perforation. Eng. Fract. Mech. 2006, 73, 1653–1678. [Google Scholar] [CrossRef]
- Xiao, X.; Pan, H.; Bai, Y.; Lou, Y.; Chen, L. Application of the modified Mohr–Coulomb fracture criterion in predicting the ballistic resistance of 2024-T351 aluminum alloy plates impacted by blunt projectiles. Int. J. Impact Eng. 2018, 123, 26–37. [Google Scholar] [CrossRef]
- Gilioli, A.; Manes, A.; Giglio, M.; Wierzbicki, T. Predicting ballistic impact failure of aluminium 6061-T6 with the rate-independent Bao–Wierzbicki fracture model. Int. J. Impact Eng. 2015, 76, 207–220. [Google Scholar] [CrossRef]
- Wierzbicki, T.; Bao, Y.; Lee, Y.-W.; Bai, Y. Calibration and evaluation of seven fracture models. Int. J. Mech. Sci. 2005, 47, 719–743. [Google Scholar] [CrossRef]
- Xue, L. Damage accumulation and fracture initiation in uncracked ductile solids subject to triaxial loading. Int. J. Solids Struct. 2007, 44, 5163–5181. [Google Scholar] [CrossRef] [Green Version]
- Bai, Y.; Wierzbicki, T. A new model of metal plasticity and fracture with pressure and Lode dependence. Int. J. Plast. 2008, 24, 1071–1096. [Google Scholar] [CrossRef]
- Johnson, G.R.; Chocron, S.; Anderson, C.E., Jr.; Beissel, S.R.; Holmquist, T.J. Effect of the third invariant on strength and failure for 10 metals. In Proceedings of the 27th International Symposiumon Ballistics, Freiburg, Germany, 22–26 April 2013; pp. 1–12. [Google Scholar]
- Yoon, J.W.; Lou, Y.; Yoon, J.; Glazoff, M.V. Asymmetric yield function based on the stress invariants for pressure sensitive metals. Int. J. Plast. 2014, 56, 184–202. [Google Scholar] [CrossRef]
- Li, Y.; Du, Z.; Wu, W.; Zhu, H.; Zhang, L.; Zhang, W. Dynamic failure criterion of ship-build metal based on stress trixiality and Lode parameter. J. Harbin Inst. Technol. 2017, 49, 153–157. (In Chinese) [Google Scholar] [CrossRef]
- Deng, Y.; Zhang, Y.; Xiao, X.; Hu, A.; Wu, H.; Xiong, J. Experimental and numerical study on the ballistic impact behavior of 6061-T651 aluminum alloy thick plates against blunt-nosed projectiles. Int. J. Impact Eng. 2020, 144, 103659. [Google Scholar] [CrossRef]
- Xiao, X.; Chen, L.; Du, T. Tests and numerical simulation for shear plugging of 7075-T651 aluminum alloy targets. J. Vib. Shock. 2019, 38, 51–58. [Google Scholar] [CrossRef]
- Xiao, X.; Wang, Y.; Vershinin, V.V.; Chen, L.; Lou, Y. Effect of Lode angle in predicting the ballistic resistance of Weldox 700 E steel plates struck by blunt projectiles. Int. J. Impact Eng. 2019, 128, 46–71. [Google Scholar] [CrossRef]
- Recht, R.F.; Ipson, T.W. Ballistic Perforation Dynamics. J. Appl. Mech. 1963, 30, 384–390. [Google Scholar] [CrossRef]
- Johnson, G.R.; Cook, W.H. A constitutive model and data for metals subjected to large strains, high strain rates and high temperatures. In Proceedings of the seventh inter-national symposium on ballistics, The Hague, The Netherlands, 19–21 April 1983; pp. 541–547. [Google Scholar]
- Knysh, P.; Korkolis, Y.P. Determination of the fraction of plastic work converted into heat in metals. Mech. Mater. 2015, 86, 71–80. [Google Scholar] [CrossRef]
- Wen, H.; Mahmoud, H. New model for ductile fracture of metal alloys. I: Monotonic loading. J. Eng. Mech. 2016, 142, 04015088. [Google Scholar] [CrossRef]
- Jiao, B. Research and Application of Weldox 900 E Steel Constitutive Model and Fracture Criterion. Master’s Thesis, Zhengzhou University, Zhengzhou, Henan, 1 May 2019. (In Chinese). [Google Scholar]
- Lindholm, U.S.; Johnson, G.R. Strain rate effects in metals at large shear strains. In Proceedings of the 29th Sagamore Army Materials Conference entitled Material Behaviour Under High Stress and Ultrahigh Loading Rates, New York, NY, USA, 19–23 July 1982. [Google Scholar]


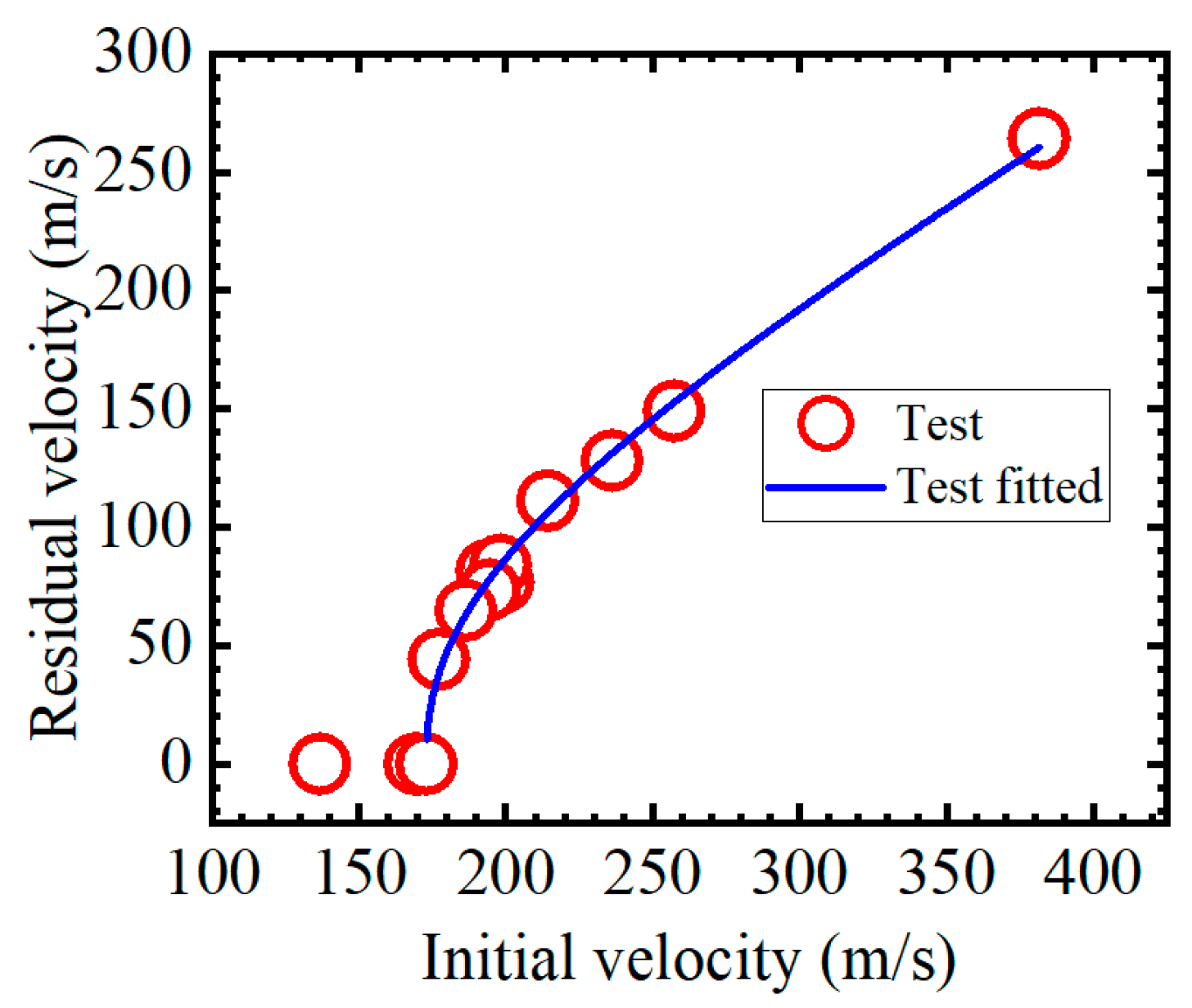
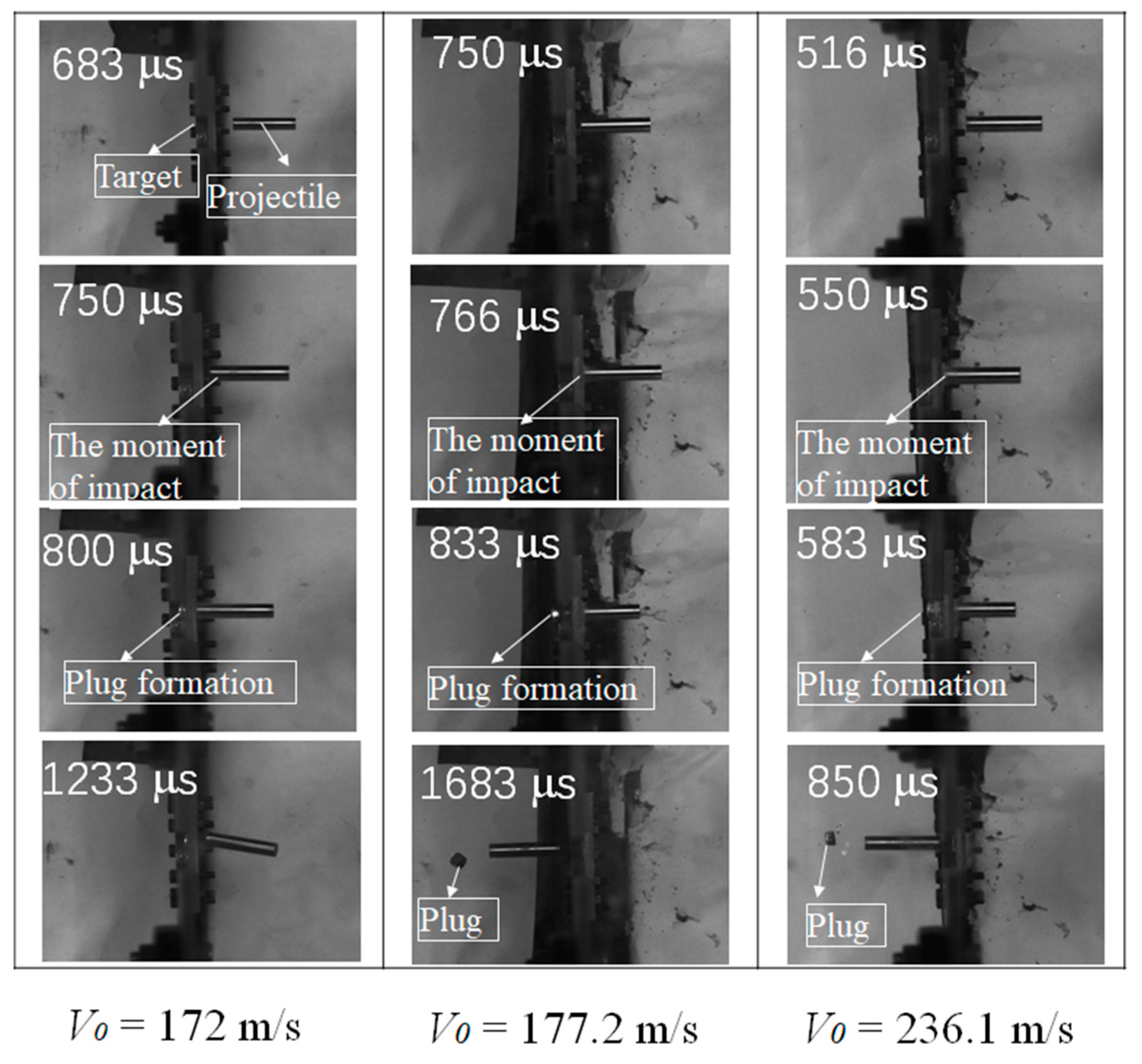
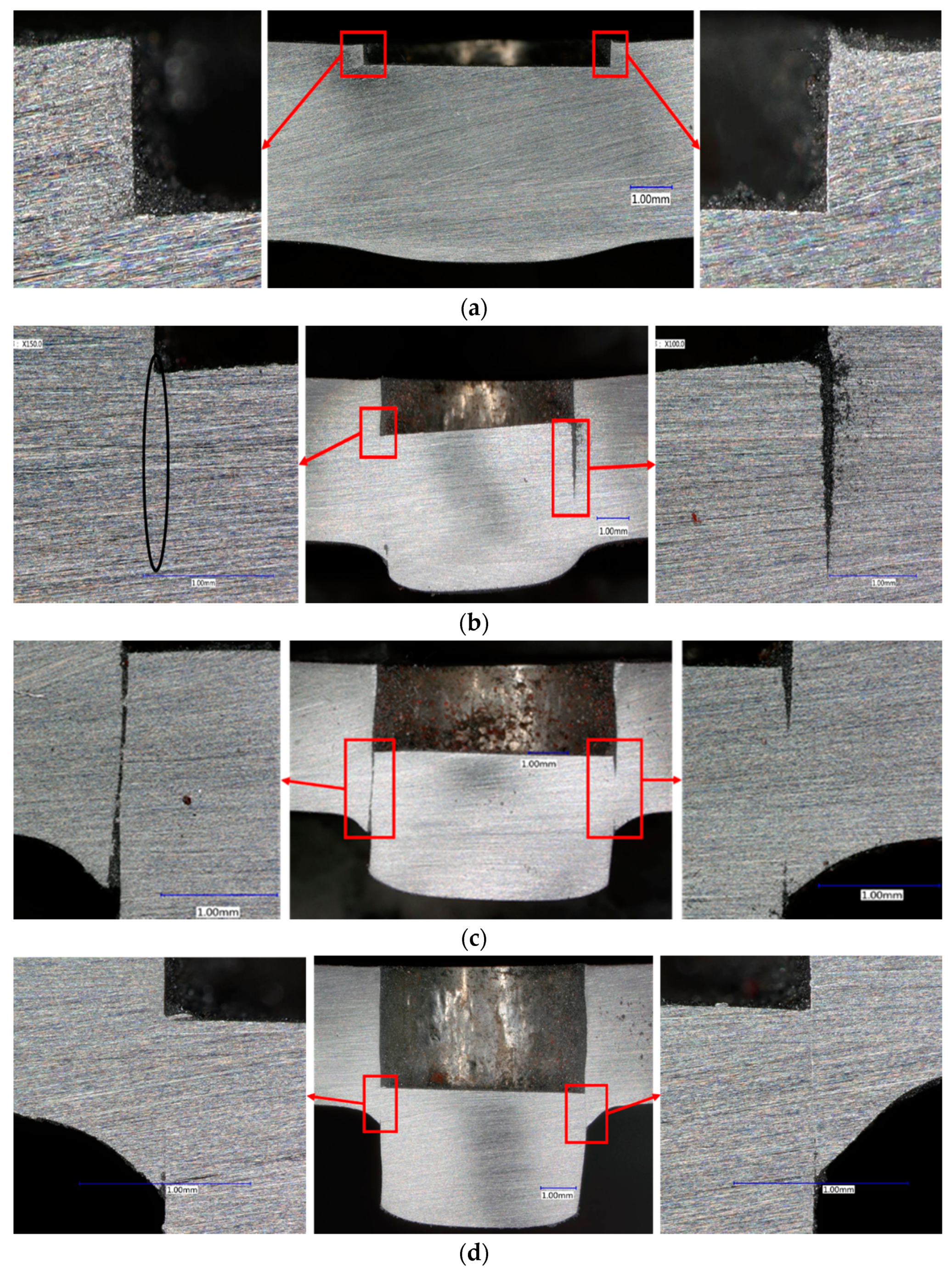






| No. | D (mm) | L (mm) | m (g) | V0 (m/s) | Vr (m/s) | Status |
|---|---|---|---|---|---|---|
| 1 | 5.94 | 29.82 | 6.39 | 257.23 | 149.13 | P |
| 2 | 5.94 | 29.8 | 6.40 | 236.11 | 128.18 | P |
| 3 | 5.94 | 29.8 | 6.39 | 214.20 | 111.24 | P |
| 4 | 5.93 | 29.81 | 6.39 | 198.99 | 76.63 | P |
| 5 | 5.96 | 29.82 | 6.39 | 193.52 | 81.78 | P |
| 6 | 5.94 | 29.82 | 6.39 | 198.13 | 84.07 | P |
| 7 | 5.93 | 29.81 | 6.39 | 194.46 | 73.64 | P |
| 8 | 5.94 | 29.83 | 6.39 | 186.32 | 64.84 | P |
| 9 | 5.95 | 29.78 | 6.39 | 177.15 | 44.15 | P |
| 10 | 5.94 | 29.8 | 6.39 | 381.42 | 264.23 | P |
| 11 | 5.94 | 29.77 | 6.39 | 136.63 | 0 | B |
| 12 | 5.95 | 29.79 | 6.39 | 170.31 | 0 | B |
| 13 | 5.95 | 29.78 | 6.39 | 168.92 | 0 | B |
| 14 | 5.95 | 29.8 | 6.39 | 172.93 | 0 | I |
| 15 | 5.93 | 29.79 | 6.39 | 257.23 | 149.13 | P |
| Modulus of Elasticity | Poisson’s Ratio | Density | Yield Strength | Tangent Modulus |
|---|---|---|---|---|
| E(GPa) | v | ρ(kg/m3) | Y(MPa) | Et(MPa) |
| 204 | 0.33 | 7750 | 1900 | 15,000 |
| Parameter Name | Notation | Numerical Value |
|---|---|---|
| Elastic Modulus | E(GPa) | 206 |
| Poisson’s ratio | μ | 0.3 |
| Density | (kg/m3) | 7800 |
| Yield Strength | A (MPa) | 947.48 |
| Strain hardening constant | B (MPa) | 396.5 |
| n | 0.383 | |
| Strain rate constant | C | 0.023 |
| Reference strain rate | (s−1) | 4.2*10−4 |
| Thermal softening constant | p | 5.931 |
| m | 2.768 | |
| Room temperature | T0 (K) | 293 |
| Melting temperature | Tm (K) | 1800 |
| Specific heat | Cp (J/kg K) | 460 |
| JC | D1 | −0.458 |
| D2 | 5.037 | |
| D3 | −1.385 | |
| D4 | −0.007 | |
| D5 | 7.57 | |
| D6 | 2.738 | |
| ASCE | C1 | 1.061 |
| C2 | −1.302 | |
| C3 | −7.531 |
| Results | Mesh Size | Fracture criterion | a | p | Vbl (m/s) | Vbl |
|---|---|---|---|---|---|---|
| Test result | 0.73 | 2.57 | 172.93 | |||
| FE result | 10 μm | JC | 0.73 | 2.65 | 157.26 | 9.21% |
| ASCE | 0.78 | 0.32 | 155 | 9.29% | ||
| 20 μm | JC | 0.73 | 2.75 | 169.74 | 1.84% | |
| ASCE | 0.76 | 2.76 | 168.71 | 2.44% | ||
| 50 μm | JC | 0.71 | 3.37 | 199 | 15.07% | |
| ASCE | 0.86 | 2.48 | 187 | 8.15% | ||
| 100 μm | JC | 0.79 | 2.49 | 219.65 | 26.6% | |
| ASCE | 0.79 | 3.06 | 211.46 | 22.28% | ||
| 200 μm | JC | 0.69 | 4.89 | 269.97 | 56.11% | |
| ASCE | 0.82 | 2.71 | 240 | 38.78% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shi, Y.; Hu, A.; Du, T.; Xiao, X.; Jia, B. Experimental and Numerical Study on the Protective Behavior of Weldox 900 E Steel Plates Impacted by Blunt-Nosed Projectiles. Metals 2022, 12, 141. https://doi.org/10.3390/met12010141
Shi Y, Hu A, Du T, Xiao X, Jia B. Experimental and Numerical Study on the Protective Behavior of Weldox 900 E Steel Plates Impacted by Blunt-Nosed Projectiles. Metals. 2022; 12(1):141. https://doi.org/10.3390/met12010141
Chicago/Turabian StyleShi, Yahui, Ang Hu, Taisheng Du, Xinke Xiao, and Bin Jia. 2022. "Experimental and Numerical Study on the Protective Behavior of Weldox 900 E Steel Plates Impacted by Blunt-Nosed Projectiles" Metals 12, no. 1: 141. https://doi.org/10.3390/met12010141
APA StyleShi, Y., Hu, A., Du, T., Xiao, X., & Jia, B. (2022). Experimental and Numerical Study on the Protective Behavior of Weldox 900 E Steel Plates Impacted by Blunt-Nosed Projectiles. Metals, 12(1), 141. https://doi.org/10.3390/met12010141






