Simulation of the Influence of the as-Deposited Wall Thickness on Arc Shape and Stability during Wire Arc Additive Manufacturing
Abstract
:1. Introduction
2. Mathematical Models and Methods
2.1. Assumptions
- (1)
- The arc plasma is considered to be continuous, laminar Newton flow, and the arc plasma is in a steady state and satisfies the local thermal equilibrium (LTE) state [12].
- (2)
- Due to the constant change in the shape of the wire tip in the actual WAAM process, it is not easy to describe it by a unified body. To simplify the numerical model, the wire tip is simplified as a plane [13].
- (3)
- Since the arc is not sensitive to the electrode surface temperature, the surface of the single-pass multi-layer wall is set to 2000 K, and the wire tip is set to 3000 K [28].
- (4)
- Since the arc velocity is much greater than the metal flow velocity, the influence of the droplet transfer on the arc is ignored [28], and only the arc simulation is studied, so the steady-state calculation method is adopted.
- (5)
- To simplify the mathematical model, it is assumed that the upper surface of the single-pass multi-layer wall is a circular arc surface. The corner angle between the circular arc surface and the sidewall is fixed at 30° in this study.
2.2. Governing Equations
2.3. The Experimental System and Boundary Conditions

| No. | Welding Speed (mm/s) | Shielding Gas Flow Rate (L/min) | Designed δ (mm) | Measured δ (mm) |
|---|---|---|---|---|
| 1 | 24 | 20 | 1 | 1.4 |
| 2 | 22 | 20 | 2 | 1.9 |
| 3 | 18 | 20 | 3 | 3.1 |
| 4 | 10 | 20 | 4 | 4.2 |
| 5 | 8 | 20 | 5 | 5.1 |
| 6 | 4.5 | 20 | 6 | 6.2 |
| 7 | 3.5 | 20 | 8 | 8.1 |
| 8 | 2.8 | 20 | 10 | 10.2 |
| 9 | 2.5 | 20 | 12 | 12.2 |
| 10 | 2 | 20 | 14 | 14.3 |
| 11 | 4 | 20 | +∞ (a single bead depositing) | +∞ (a single bead depositing) |
| Boundaries | (m/s) | T (K) | (V) | (Wb/m) |
|---|---|---|---|---|
| wire-bottom | — | 3000 | ||
| wire-wall | — | coupled | coupled | |
| gas-in | 300 | |||
| gas-out | — | 300 | 0 | |
| workpiece | — |
3. Results and Discussion
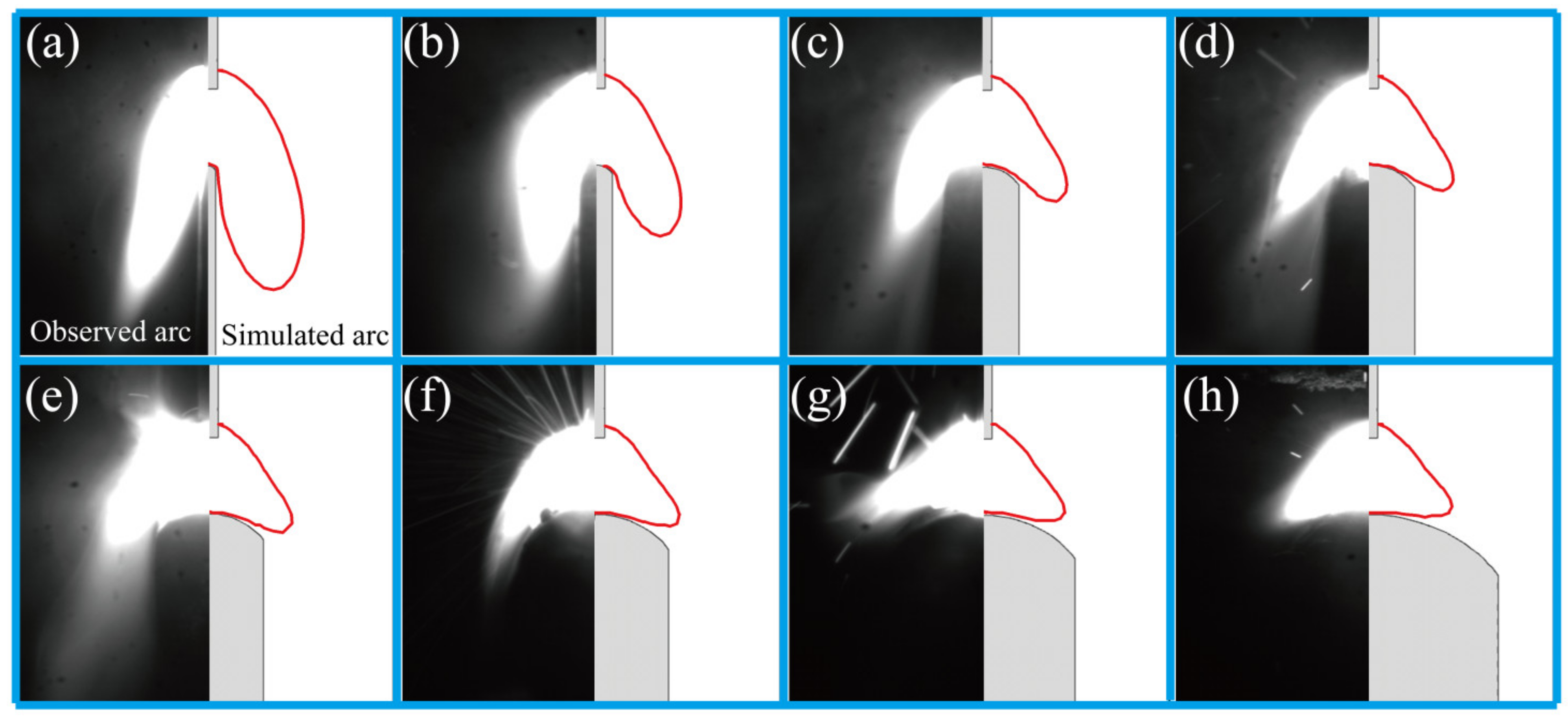
3.1. The Arc Shape and Temperature Fields
3.2. Velocity Fields
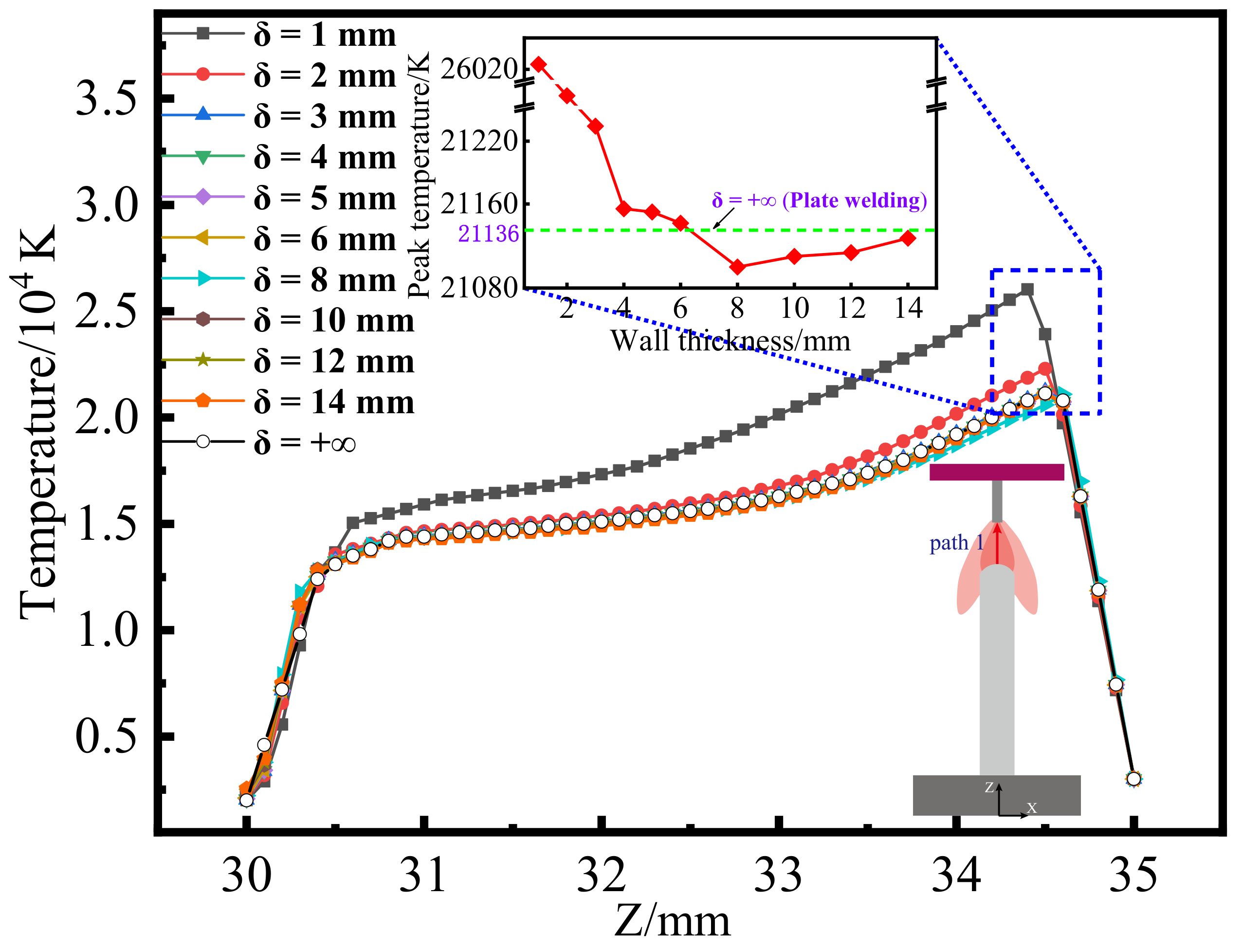
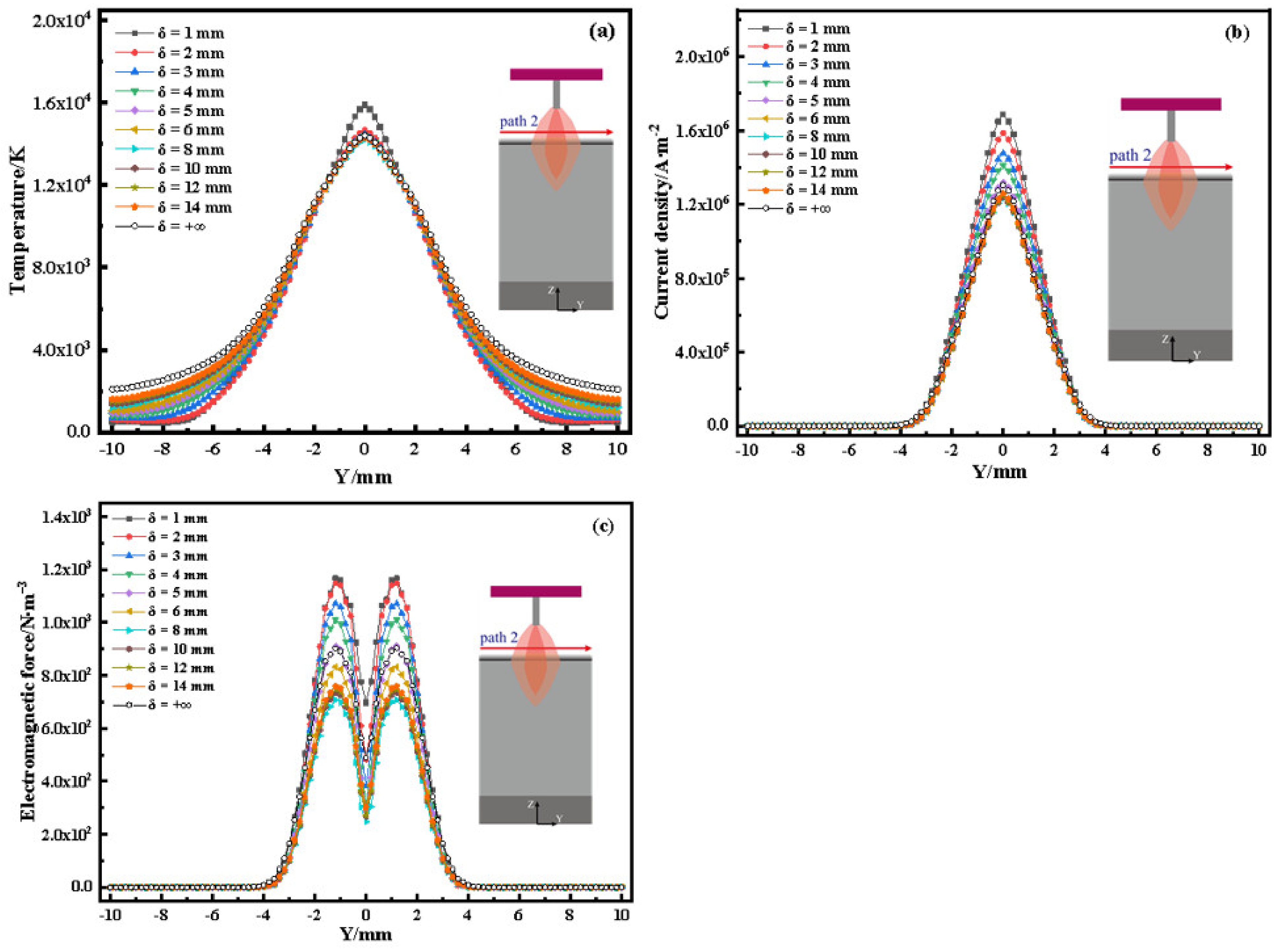
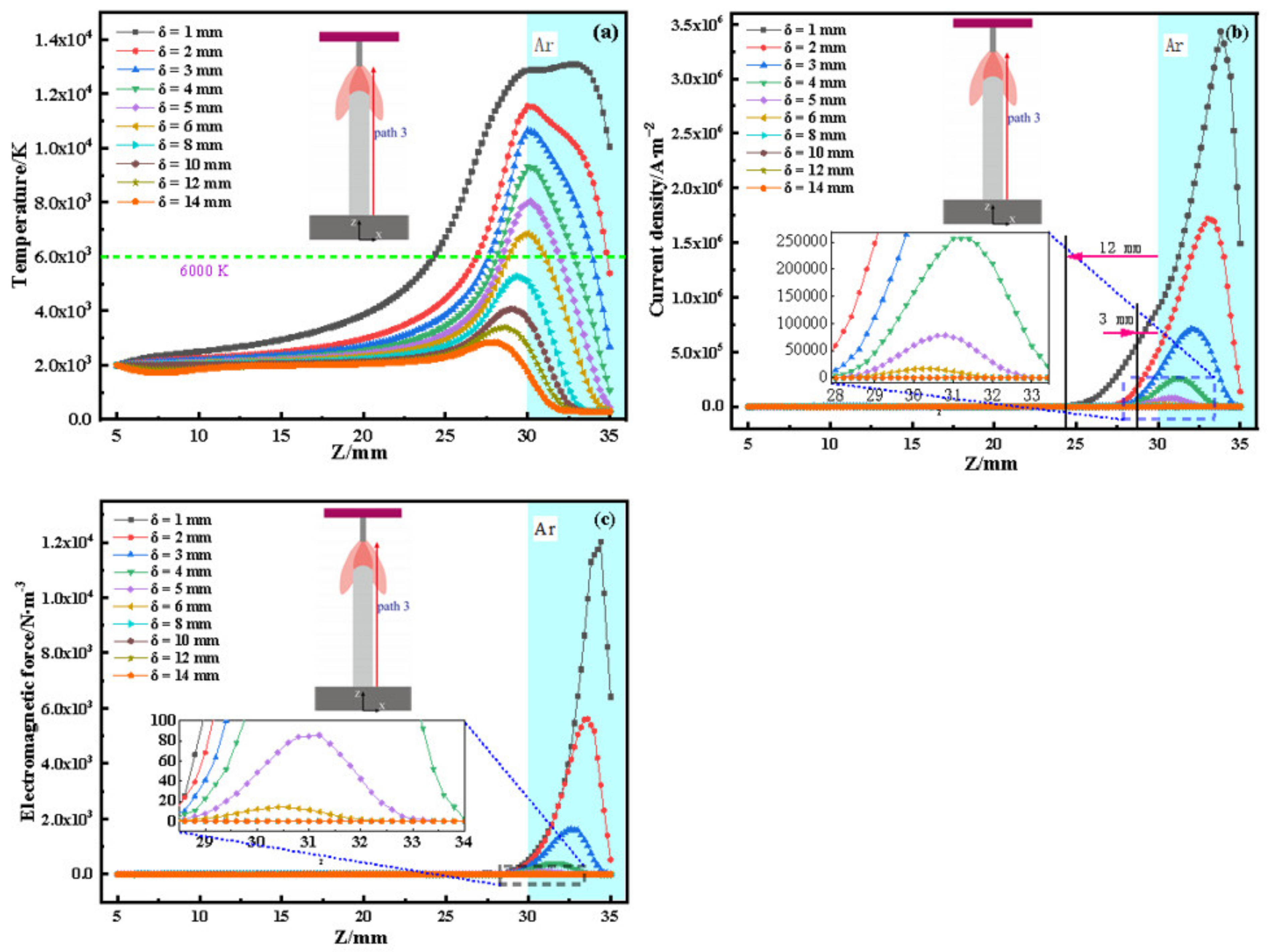
3.3. The Arc Parameters Distribution along the Characteristic Path
4. Conclusions
- (1)
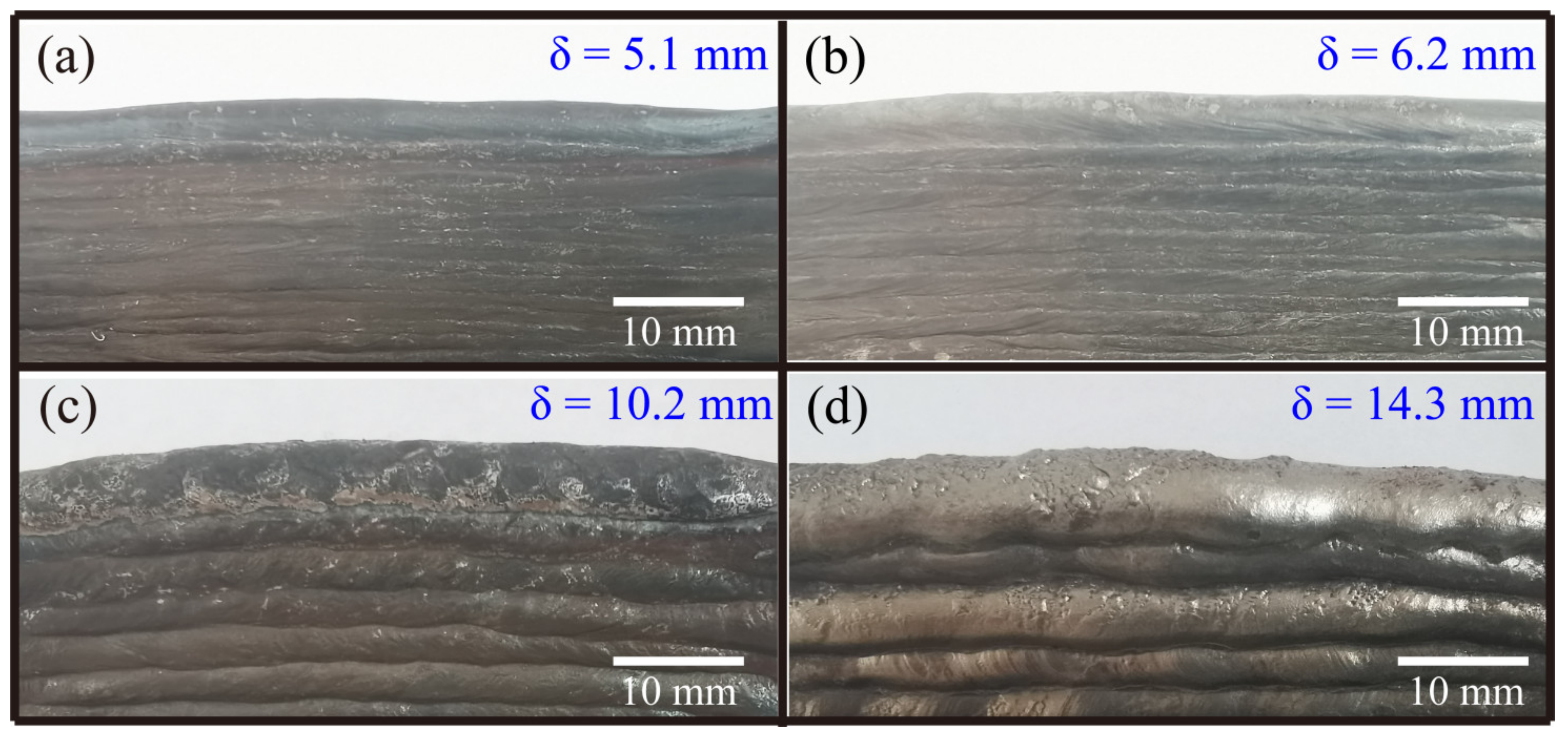
- The length of the arc along the sidewall decreases with the increase in the wall thickness, and the arc shape tends to be bell-shaped. The wall thickness has little influence on the temperature, velocity, current density, and electromagnetic force distribution along the welding direction, which is similar to that of the plate welding. The coverage of shielding gas on the sidewall decreases with the increase in wall thickness. When δ is slightly less than Φ, the forming quality of the deposited wall is the best.
- (2)
- When δ < Φ, the peak temperature of the arc centre, the peak current density, and peak and valley electromagnetic force along the welding direction decrease with the increase in the wall thickness, and the increase in wall thickness will lead to the reduction in arc contraction.
- (3)
- When δ > Φ, the peak temperature of the arc centre, the peak current density, and peak and valley electromagnetic force along the welding direction increase slowly with the increase in wall thickness, and similar to the case of plate welding, the small cathode area on the top surface of the wall will lead to a weak trend of the arc contraction.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Williams, S.W.; Martina, F.; Addison, A.C.; Ding, J.; Colegrove, P. Wire + Arc Additive Manufacturing. Mater. Sci. Technol. 2016, 32, 641–647. [Google Scholar]
- Cunningham, C.; Flynn, J.; Shokrani, A.; Dhokia, V.; Newman, S. Invited review article: Strategies and processes for high quality wire arc additive manufacturing. Addit. Manuf. 2018, 22, 672–686. [Google Scholar] [CrossRef]
- Ortega, A.G.; Galvan, L.C.; Deschaux-Beaume, F.; Mezrag, B.; Rouquette, S. Effect of process parameters on the quality of aluminium alloy Al5Si deposits in wire and arc additive manufacturing using a cold metal transfer process. Sci. Technol. Weld. Join. 2018, 23, 316–332. [Google Scholar] [CrossRef]
- Ikram, A.; Chung, H. Numerical simulation of arc, metal transfer and its impingement on weld pool in variable polarity gas metal arc welding. J. Manuf. Process. 2021, 64, 1529–1543. [Google Scholar]
- Manqing, C.H.E.N.G.; Yanli, A.N.; Huayun, D.U.; Yinghui, W.E.I.; Ding, F.A.N. Effect of current changes on velocity and temperature profiles of GTAW arc. Trans. China Weld. Inst. 2010, 31, 33–37. [Google Scholar]
- Bachmann, B.; Kozakov, R.; Gött, G.; Ekkert, K.; Bachmann, J.-P.; Marques, J.-L.; Schöpp, H.; Uhrlandt, D.; Schein, J. High-speed three-dimensional plasma temperature determination of axially symmetric free-burning arcs. J. Phys. 2013, 46, 125203. [Google Scholar] [CrossRef]
- Hsu, K.C.; Etemadi, K.; Pfender, E. Study of the freeburning highintensity argon arc. J. Appl. Phys. 1983, 54, 1293. [Google Scholar]
- Nahed, C.; Gounand, S.; Medale, M. A numerical study of the effects of cathode geometry on tungsten inert gas type electric arcs. Int. J. Heat Mass Transf. 2022, 182, 121923. [Google Scholar] [CrossRef]
- Hu, J.; Tsai, H.L. Heat and mass transfer in gas metal arc welding. Part I: The arc. Int. J. Heat Mass Transf. 2007, 50, 833–846. [Google Scholar] [CrossRef]
- Hu, J.; Tsai, H.L. Heat and mass transfer in gas metal arc welding. Part II: The metal. Int. J. Heat Mass Transf. 2007, 50, 808–820. [Google Scholar] [CrossRef]
- Cadiou, S.; Courtois, M.; Carin, M.; Berckmans, W.; Le Masson, P. 3D heat transfer, fluid flow and electromagnetic model for cold metal transfer wire arc additive manufacturing (Cmt-Waam). Addit. Manuf. 2020, 36, 101541. [Google Scholar] [CrossRef]
- Zhou, X.; Zhang, H.; Wang, G.; Bai, X. Three-dimensional numerical simulation of arc and metal transport in arc welding based additive manufacturing. Int. J. Heat Mass Transf. 2016, 103, 521–537. [Google Scholar] [CrossRef]
- Zhou, X.; Tian, Q.; Du, Y.; Zhang, Y.; Bai, X.; Zhang, Y.; Zhang, H.; Zhang, C.; Yuan, Y. Investigation of the effect of torch tilt and external magnetic field on arc during overlapping deposition of wire arc additive manufacturing. Rapid Prototyp. J. 2020, 27, 1355–2046. [Google Scholar] [CrossRef]
- Wang, X.; Luo, Y.; Chi, L.; Fan, D. Numerical investigation of transport phenomena of arc plasma in argon-oxygen gas mixture. Int. J. Heat Mass Transf. 2020, 154, 119708. [Google Scholar] [CrossRef]
- Wang, L.L.; Lu, F.G.; Wang, H.P.; Murphy, A.; Tang, X.H. Effects of shielding gas composition on arc profile and molten pool dynamics in gas metal arc welding of steels. J. Phys. D Appl. Phys. 2014, 47, 465202. [Google Scholar] [CrossRef]
- Murphy, A.B.; Tanaka, M.; Yamamoto, K.; Tashiro, S.; Sato, T.; Lowke, J.J. Modelling of thermal plasmas for arc welding: The role of the shielding gas properties and of metal vapour. J. Phys. D Appl. Phys. 2009, 42, 194006. [Google Scholar] [CrossRef]
- Savaş, A.; Ceyhun, V. Finite element analysis of GTAW arc under different shielding gases. Comput. Mater. Sci. 2011, 51, 57–71. [Google Scholar]
- Xiao, L.; Fan, D.; Huang, J.-K. Numerical Study on Arc Plasma Behaviors in GMAW with Applied Axial Magnetic Field. J. Phys. Soc. Jpn. 2019, 88, 074502. [Google Scholar]
- Han, Y.; Chen, J.; Li, L.; Wang, L.; Wu, C. Numerical Investigation of Arc-Pool-Metal Vapor Behavior in GTAW with an External Magnetic Field. Metals 2020, 10, 1199. [Google Scholar]
- Wang, L.; Chen, J.; Wu, C. Numerical investigation on the effect of process parameters on arc and metal transfer in magnetically controlled gas metal arc welding. Vacuum 2020, 177, 109391. [Google Scholar] [CrossRef]
- Wang, L.; Chen, J.; Wu, C.; Luan, S. Numerical analysis of arc and droplet behaviors in gas metal arc welding with external compound magnetic field. J. Mater. Process. Technol. 2020, 282, 116638. [Google Scholar] [CrossRef]
- Wang, X.; Luo, Y.; Wu, G.; Chi, L.; Fan, D. Numerical Simulation of Metal Vapour Behavior in Double Electrodes TIG Welding. Plasma Chem. Plasma Process. 2018, 38, 1095–1114. [Google Scholar] [CrossRef]
- Kanemaru, S.; Sasaki, T.; Sato, T.; Era, T.; Tanaka, M. Study for the mechanism of TIG-MIG hybrid welding process. Weld. World 2015, 59, 261–268. [Google Scholar] [CrossRef]
- Wu, D.; Huang, J.; Kong, L.; Hua, X.; Wang, M. Coupled mechanisms of arc, weld pool and weld microstructures in high speed tandem TIG welding. Int. J. Heat Mass Transf. 2020, 154, 119641. [Google Scholar] [CrossRef]
- Bai, X.; Colegrove, P.; Ding, J.; Zhou, X.; Diao, C.; Bridgeman, P.; Hönnige, J.R.; Zhang, H.; Williams, S. Numerical analysis of heat transfer and fluid flow in multilayer deposition of PAW-based wire and arc additive manufacturing. Int. J. Heat Mass Transf. 2018, 124, 504–516. [Google Scholar] [CrossRef]
- Zhao, W.; Wei, Y.; Long, J.; Chen, J.; Liu, R.; Ou, W. Modeling and simulation of heat transfer, fluid flow and geometry morphology in GMAW-based wire arc additive manufacturing. Weld. World 2021, 20, 1571–1590. [Google Scholar] [CrossRef]
- Choudhury, S.S.; Marya, S.K.; Amirthalingam, M. Improving arc stability during wire arc additive manufacturing of thin-walled titanium components. J. Manuf. Processes 2021, 66, 53–69. [Google Scholar] [CrossRef]
- Zhou, X.; Zhang, H.; Wang, G.; Bai, X. Simulation of the influences of surface top ography of dep osited layer on arc shap e and state in arc based additive forming. Acta Phys. Sin. 2016, 65, 331–342. [Google Scholar]
- Xinxin, W.; Ding, F.; Jiankang, H.; Yong, H. Numerical Simulation of Heat Transfer and Fluid Flow for Arc-weld Pool in TIG Welding. J. Mech. Eng. 2015, 50, 69–78. [Google Scholar]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, C.; Zhang, X.; Peng, Y.; Huang, Y.; Wang, K.; Wang, J.; Zhou, M. Simulation of the Influence of the as-Deposited Wall Thickness on Arc Shape and Stability during Wire Arc Additive Manufacturing. Metals 2022, 12, 1563. https://doi.org/10.3390/met12101563
Zhou C, Zhang X, Peng Y, Huang Y, Wang K, Wang J, Zhou M. Simulation of the Influence of the as-Deposited Wall Thickness on Arc Shape and Stability during Wire Arc Additive Manufacturing. Metals. 2022; 12(10):1563. https://doi.org/10.3390/met12101563
Chicago/Turabian StyleZhou, Chundong, Xiaoyong Zhang, Yong Peng, Yong Huang, Kehong Wang, Jianchun Wang, and Ming Zhou. 2022. "Simulation of the Influence of the as-Deposited Wall Thickness on Arc Shape and Stability during Wire Arc Additive Manufacturing" Metals 12, no. 10: 1563. https://doi.org/10.3390/met12101563
APA StyleZhou, C., Zhang, X., Peng, Y., Huang, Y., Wang, K., Wang, J., & Zhou, M. (2022). Simulation of the Influence of the as-Deposited Wall Thickness on Arc Shape and Stability during Wire Arc Additive Manufacturing. Metals, 12(10), 1563. https://doi.org/10.3390/met12101563





