Computational Investigation on Cracking Behaviors of AerMet 100
Abstract
:1. Introduction
2. Methods
2.1. Microstructure of AerMet 100 Steel
2.2. Modeling
2.3. Computational Method of Crack Propagation Angle and SIF
- Method 1: 10 points in front of the crack tip are selected;
- Method 2: the first 2 points are excluded, but the remaining 8 points are selected;
- Method 3: the first 3 points are selected;
- Method 4: the first 2 points are excluded, but the consecutive 3 points are selected.
3. Results
3.1. Propagation Behavior of Typical In-Plane Crack
3.2. Propagation Behavior of Crack with an Offset
3.3. Propagation Behavior of Crack with an Initial Angle
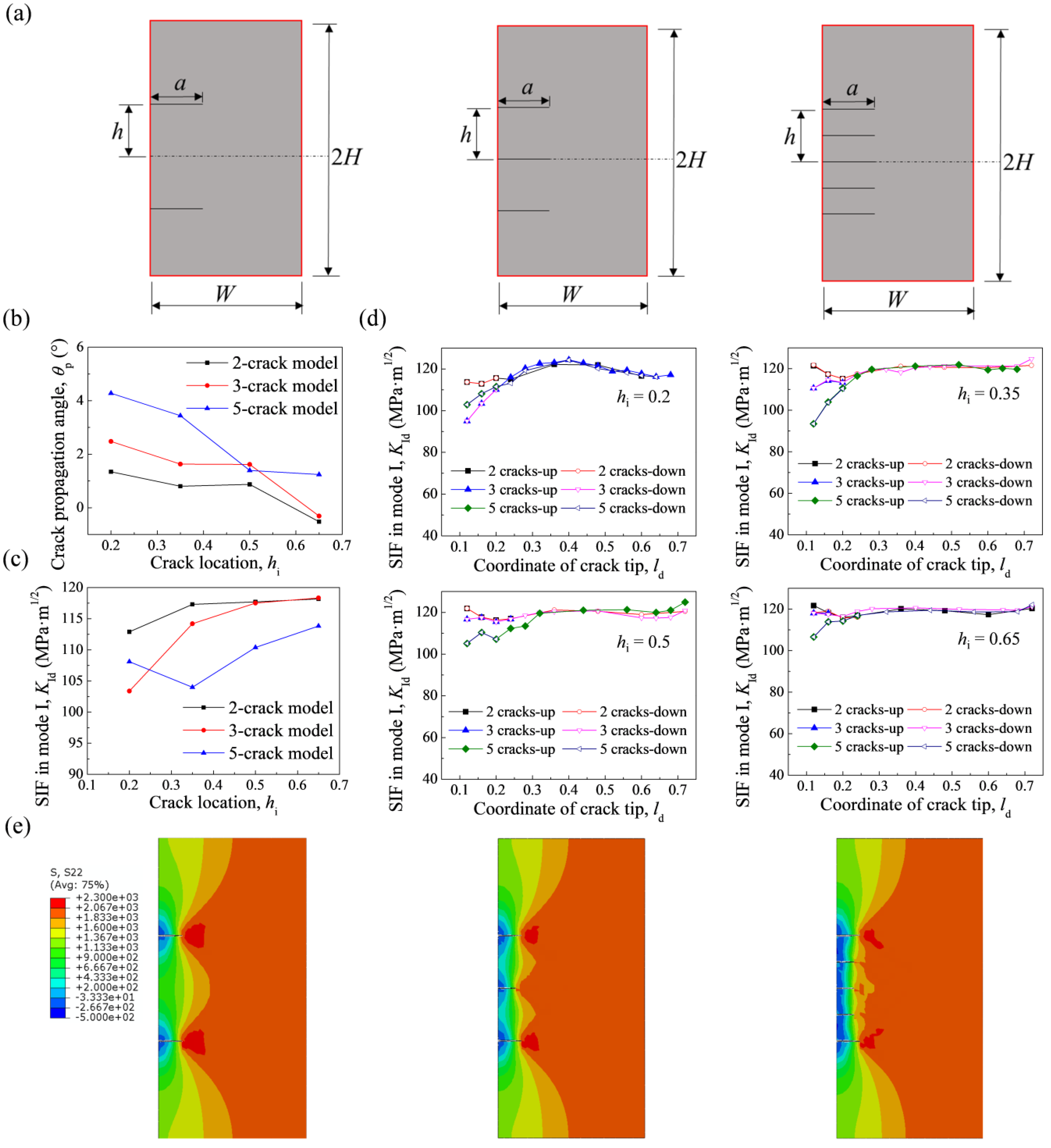
3.4. Propagation Behavior of Multi-Crack
4. Discussion
4.1. Influence of Initial Crack Length
4.2. Influence of Crack Location
4.3. Influence of Initial Crack Angle
4.4. Influence of Crack Number
5. Conclusions
- The crack propagation angle showed a significant increasing trend when the initial crack length and the initial crack location increased, which could be attributed to the asymmetry of the stress contour in front of the crack tip.
- The initial crack length had little influence on KId, while KId exhibited a smaller value as the crack location increased casing the declined of the stress level.
- The inward crack propagation angle slowly increased and finally turned into an outward angle until the initial crack angle reached about 45°, while KId rarely changed.
- More cracks in the model would enhance the deflection in the propagation process and lead to a smaller KId value in the crack tip.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| KIC | Critical stress intensity factor for opening mode |
| GIC | Critical fracture energy for opening mode |
| EA | Energy absorption |
| SIF | Stress intensity factor |
| E | Young’s modulus |
| μ | Poisson ratio |
| σe | Yield strength |
| σu | Ultimate strength |
| ρ | Mass density |
| η | Breaking elongation |
| W | Width of the model |
| H | Half of the model height |
| a | Initial crack length |
| h | Distance between the crack and the middle line of the plate |
| li | Dimensionless initial crack length |
| hi | Dimensionless crack location |
| ld | Dimensionless crack location in X coordinate |
| yd | Crack location in Y coordinate |
| Crack propagation angle | |
| Initial crack angle | |
| KId | Dynamic stress intensity factor for opening mode |
| KIId | Dynamic stress intensity factor for in-plane shearing mode |
| KIIId | Dynamic stress intensity factor for anti-plane shearing mode |
| /S22 | Stress component normal to the crack propagation direction |
| /S12 | Stress component locally perpendicular to the crack edge |
| /S23 | Stress component tangent to the crack edge |
| r | the distance of points to the crack tip |
| dc | Dimensionless distance to the crack tip |
| FQ | The peak reaction force in the three-bending test |
References
- Wang, C.; Gao, Y.; Li, Y.; Han, S.; Liu, S.; Zhang, P. Effects of solid-solution temperature on microstructure and mechanical properties of a novel 2000 MPa grade ultra-high-strength steel. J. Iron Steel Res. Int. 2020, 27, 710–718. [Google Scholar] [CrossRef]
- Gibbons, S.; Abrahams, R.; Vaughan, M.; Barber, R.; Harris, R.; Arroyave, R.; Karaman, I. Microstructural refinement in an ultra-high strength martensitic steel via equal channel angular pressing. Mater. Sci. Eng. A 2018, 725, 57–64. [Google Scholar] [CrossRef]
- Oehlert, A.; Atrens, A. Stress corrosion crack propagation in AerMet 100. J. Mater. Sci. 1998, 33, 775–781. [Google Scholar] [CrossRef]
- Zhong, J.; Yu, M.; Li, S.; Macdonald, D.D.; Xiao, B.; Liu, J. Theoretical and experimental studies of passivity breakdown of Aermet 100 ultra-high stainless steel in chloride ion medium. Mater. Corros. 2019, 70, 2020–2032. [Google Scholar] [CrossRef]
- Shi, X.; Zeng, W.; Zhao, Q.; Peng, W.; Kang, C. Study on the microstructure and mechanical properties of Aermet 100 steel at the tempering temperature around 482 °C. J. Alloys Compd. 2016, 679, 184–190. [Google Scholar] [CrossRef]
- Wei, R.; Benn, R.C.; Cooper, C.V. High Intensity plasma ion nitriding of AerMet 100 martensitic steel. Plasma Process. Polym. 2007, 4, S700–S706. [Google Scholar] [CrossRef]
- Manigandan, K.; Srivatsan, T.S.; Tammana, D.; Poorganji, B.; Vasudevan, V.K. Influence of microstructure on strain-controlled fatigue and fracture behavior of ultra high strength alloy steel AerMet 100. Mater. Sci. Eng. A 2014, 601, 29–39. [Google Scholar] [CrossRef]
- Hu, D.Y.; Meng, K.P.; Jiang, H.L.; Xu, J.; Liu, R.R. Strain rate dependent constitutive behavior investigation of AerMet 100 steel. Mater. Des. 2015, 87, 759–772. [Google Scholar] [CrossRef]
- Lu, T.; Li, Y.; Zhao, H.; Wang, C.; Han, S. Dynamic constitutive behavior investigation of a novel low alloy ultra-high strength steel. Mater. Res. Express 2021, 8, 016508. [Google Scholar] [CrossRef]
- Sun, X.; Yao, P.; Qu, S.; Yu, S.; Zhang, X.; Wang, W.; Huang, C.; Chu, D. Material properties and machining characteristics under high strain rate in ultra-precision and ultra-high-speed machining process: A review. Int. J. Adv. Manuf. Technol. 2022, 120, 7011–7042. [Google Scholar] [CrossRef]
- Hirobe, S.; Imakita, K.; Aizawa, H.; Kato, Y.; Urata, S.; Oguni, K. Simulation of catastrophic failure in a residual stress field. Phys. Rev. Lett. 2021, 127, 064301. [Google Scholar] [CrossRef] [PubMed]
- Mao, L.; Wang, W.; Liu, Z.; Yang, G.; Song, C.; Qu, S. Research on causes of fatigue cracking in the motor hangers of high-speed trains induced by current fluctuation. Eng. Fail. Anal. 2021, 127, 105508. [Google Scholar] [CrossRef]
- Wen, M.; Sun, Y.; Yu, J.; Yang, S.; Hou, X.; Yang, Y.; Sun, X.; Zhou, Y. Amelioration of weld-crack resistance of the M951 superalloy by engineering grain boundaries. J. Mater. Sci. Technol. 2021, 78, 260–267. [Google Scholar] [CrossRef]
- Liu, Y.; Cong, P.; Wu, Y.; Li, J.; Wang, X. Failure analysis and design optimization of shrouded fan blade. Eng. Fail. Anal. 2021, 122, 105208. [Google Scholar] [CrossRef]
- Hu, L.; Wang, Y.; Feng, P.; Wang, H.; Qiang, H. Debonding development in cracked steel plates strengthened by CFRP laminates under fatigue loading: Experimental and boundary element method analysis. Thin-Walled Struct. 2021, 166, 108038. [Google Scholar] [CrossRef]
- Thiyahuddin, M.; Gu, Y.; Gover, R.; Thambiratnam, D. Fluid–structure interaction analysis of full scale vehicle-barrier impact using coupled SPH–FEA. Eng. Anal. Bound. Elem. 2014, 42, 26–36. [Google Scholar] [CrossRef]
- Jena, J.; Singh, S.; Gaur, V.; Singh, I.V.; Natarajan, S. A new framework based on XFEM for cracked semipermeable piezoelectric material. Eng. Fract. Mech. 2021, 253, 107874. [Google Scholar] [CrossRef]
- Xiao, G.; Wen, L.; Tian, R. Arbitrary 3D crack propagation with Improved XFEM: Accurate and efficient crack geometries. Comput. Methods Appl. Mech. Eng. 2021, 377, 113659. [Google Scholar] [CrossRef]
- Muixí, A.; Marco, O.; Rodríguez-Ferran, A.; Fernández-Méndez, S. A combined XFEM phase-field computational model for crack growth without remeshing. Comput. Mech. 2021, 67, 231–249. [Google Scholar] [CrossRef]
- Nikfam, M.; Zeinoddini, M.; Aghebati, F.; Arghaei, A. Experimental and XFEM modelling of high cycle fatigue crack growth in steel welded T-joints. Int. J. Mech. Sci. 2019, 153, 178–193. [Google Scholar] [CrossRef]
- Gee, B.; Parchei-Esfahani, M.; Gracie, R. XFEM simulation of a mixed-mode fracture experiment in PMMA. Eng. Fract. Mech. 2020, 229, 106945. [Google Scholar] [CrossRef]
- Yadav, R.N.; Uniyal, P.; Sihota, P.; Kumar, S.; Dhiman, V.; Goni, V.G.; Sahni, D.; Bhadada, S.K.; Kumar, N. Effect of ageing on microstructure and fracture behavior of cortical bone as determined by experiment and Extended Finite Element Method (XFEM). Med. Eng. Phys. 2021, 93, 100–112. [Google Scholar] [CrossRef] [PubMed]
- Kamaya, M. Estimation of elastic–plastic fracture toughness by numerical simulation based on a stress-based criterion for ductile crack initiation. Int. J. Press. Vessels Pip. 2014, 117, 2–8. [Google Scholar] [CrossRef]
- Safdar, A.; Alizada, A.; Fries, T.-P. Model-independent approaches for the XFEM in fracture mechanics. Int. J. Numer. Methods Eng. 2010, 1, 0–10. [Google Scholar]
- Rezanezhad, M.; Lajevardi, S.A.; Karimpouli, S. Crack growth in porous media using XFEM: Comparison of modeling strategies on the abaqus. J. Anal. Numer. Methods Min. Eng. 2020, 10, 27–40. [Google Scholar]
- Sutula, D.; Kerfriden, P.; Van Dam, T.; Bordas, S.P. Minimum energy multiple crack propagation. Part-II: Discrete solution with XFEM. Eng. Fract. Mech. 2018, 191, 225–256. [Google Scholar] [CrossRef]
- Lal, A.; Mishra, K. Stochastic MMSIF of multiple edge cracks FGMs plates subjected to combined loading using XFEM. Curved Layer Struct. 2020, 7, 35–47. [Google Scholar] [CrossRef]
- ASTM. Standard test method for measurement of fracture toughness. In Annual Book of ASTM Standards; ASTM International (ASTM): Philadelphia, PA, USA, 2006. [Google Scholar]
- Burlayenko, V.N.; Altenbach, H.; Sadowski, T.; Dimitrova, S.D. Computational simulations of thermal shock cracking by the virtual crack closure technique in a functionally graded plate. Comput. Mater. Sci. 2016, 116, 11–21. [Google Scholar] [CrossRef]
- Fernandez Martinez, R.; Lostado Lorza, R.; Santos Delgado, A.A.; Piedra Pullaguari, N.O. Optimizing presetting attributes by softcomputing techniques to improve tapered roller bearings working conditions. Adv. Eng. Softw. 2018, 123, 13–24. [Google Scholar] [CrossRef]
- Iniguez-Macedo, S.; Lostado-Lorza, R.; Escribano-Garcia, R.; Martinez-Calvo, M.A. Finite element model updating combined with multi-response optimization for hyper-elastic materials characterization. Materials 2019, 12, 1019. [Google Scholar] [CrossRef]
- Lostado Lorza, R.; Escribano García, R.; Fernandez Martinez, R.; Martínez Calvo, M. Using genetic algorithms with multi-objective optimization to adjust finite element models of welded joints. Metals 2018, 8, 230. [Google Scholar] [CrossRef]
- Tada, H.; Paris, P.C.; Irwin, G.R. The Analysis of Cracks Handbook; ASME Press: New York, NY, USA, 2000. [Google Scholar]
- Zhu, X.K.; Leis, B.N. Effective methods to determine stress intensity factors for 2D and 3D cracks. In Proceedings of the 2014 10th International Pipeline Conference, Calgary, AB, Canada, 29 September–3 October 2014. [Google Scholar]
- Han, Q.; Wang, Y.; Yin, Y.; Wang, D. Determination of stress intensity factor for mode I fatigue crack based on finite element analysis. Eng. Fract. Mech. 2015, 138, 118–126. [Google Scholar] [CrossRef]

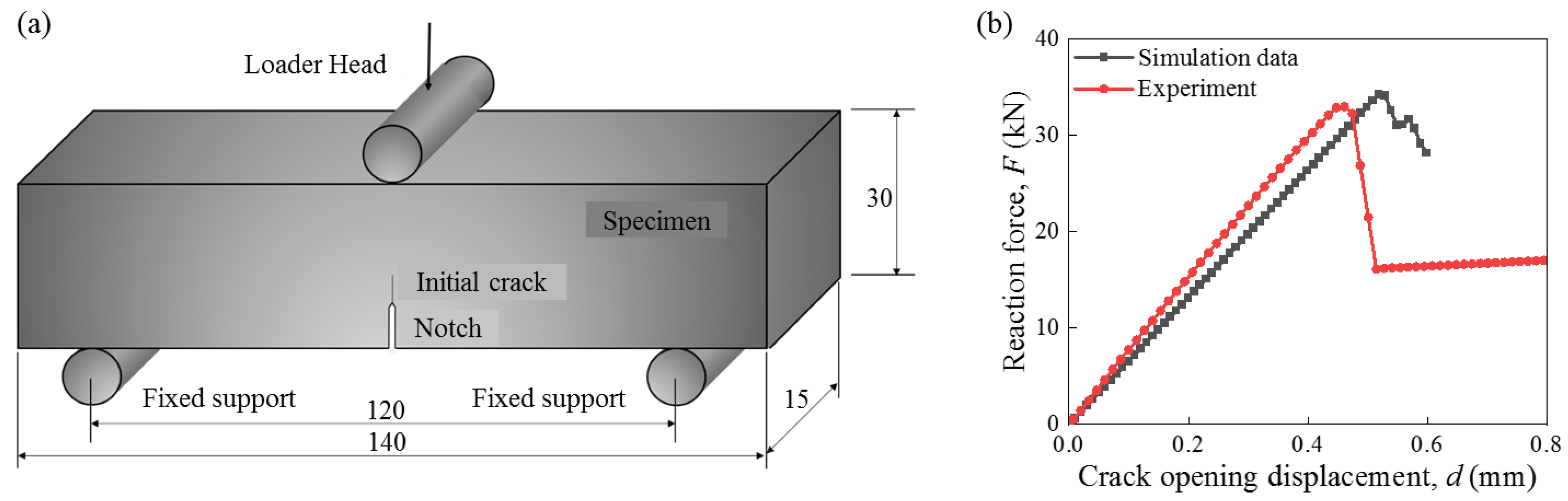

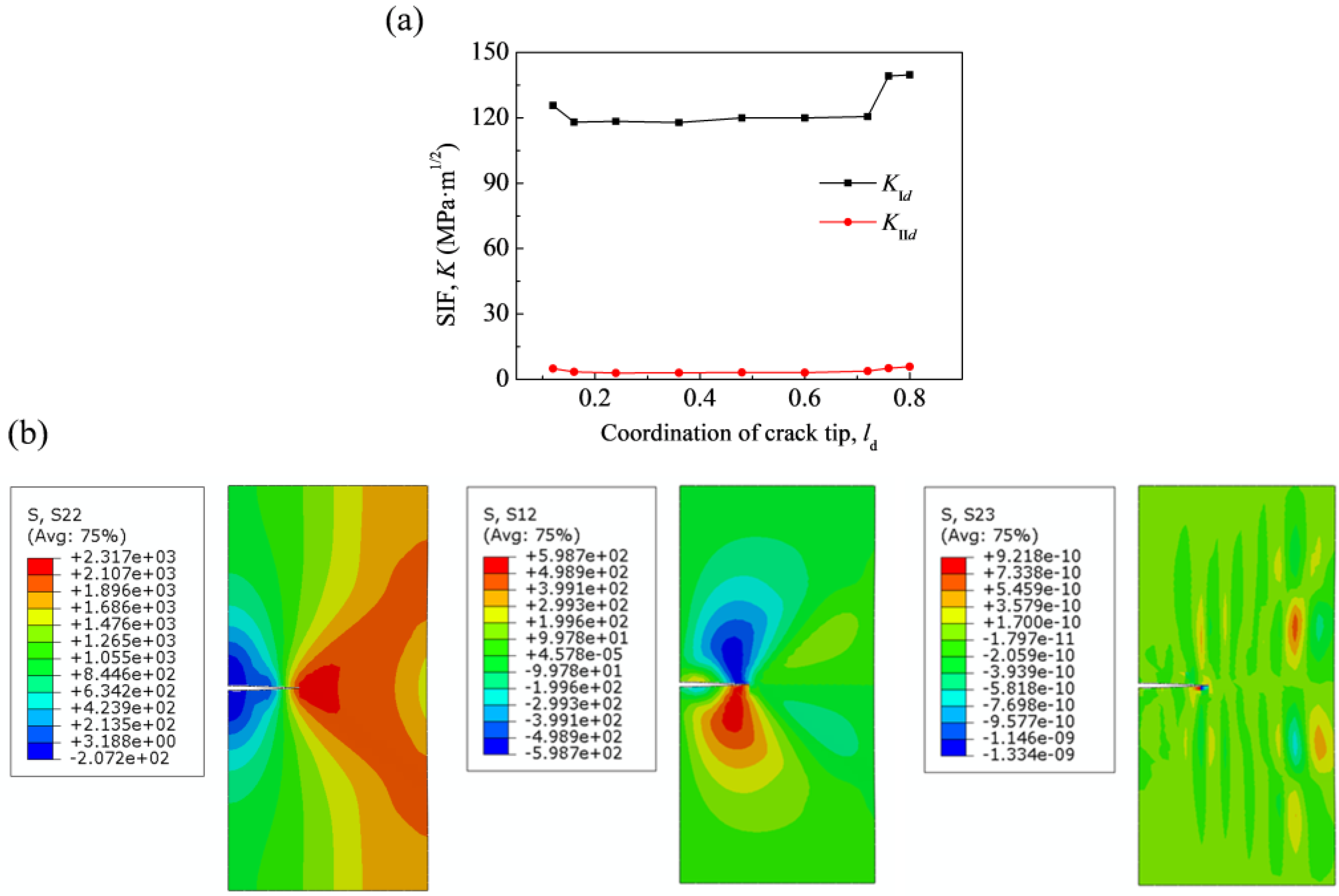
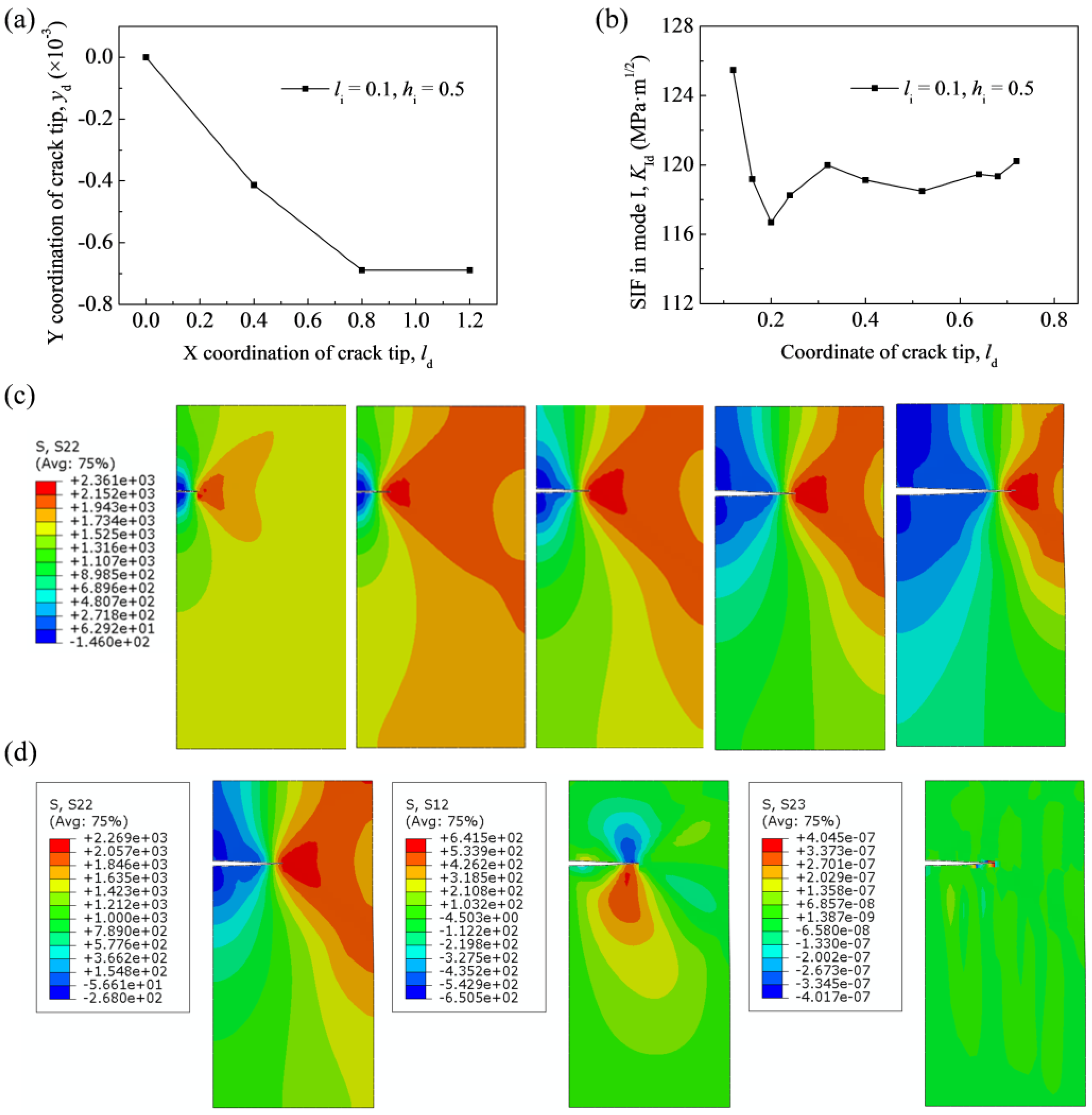




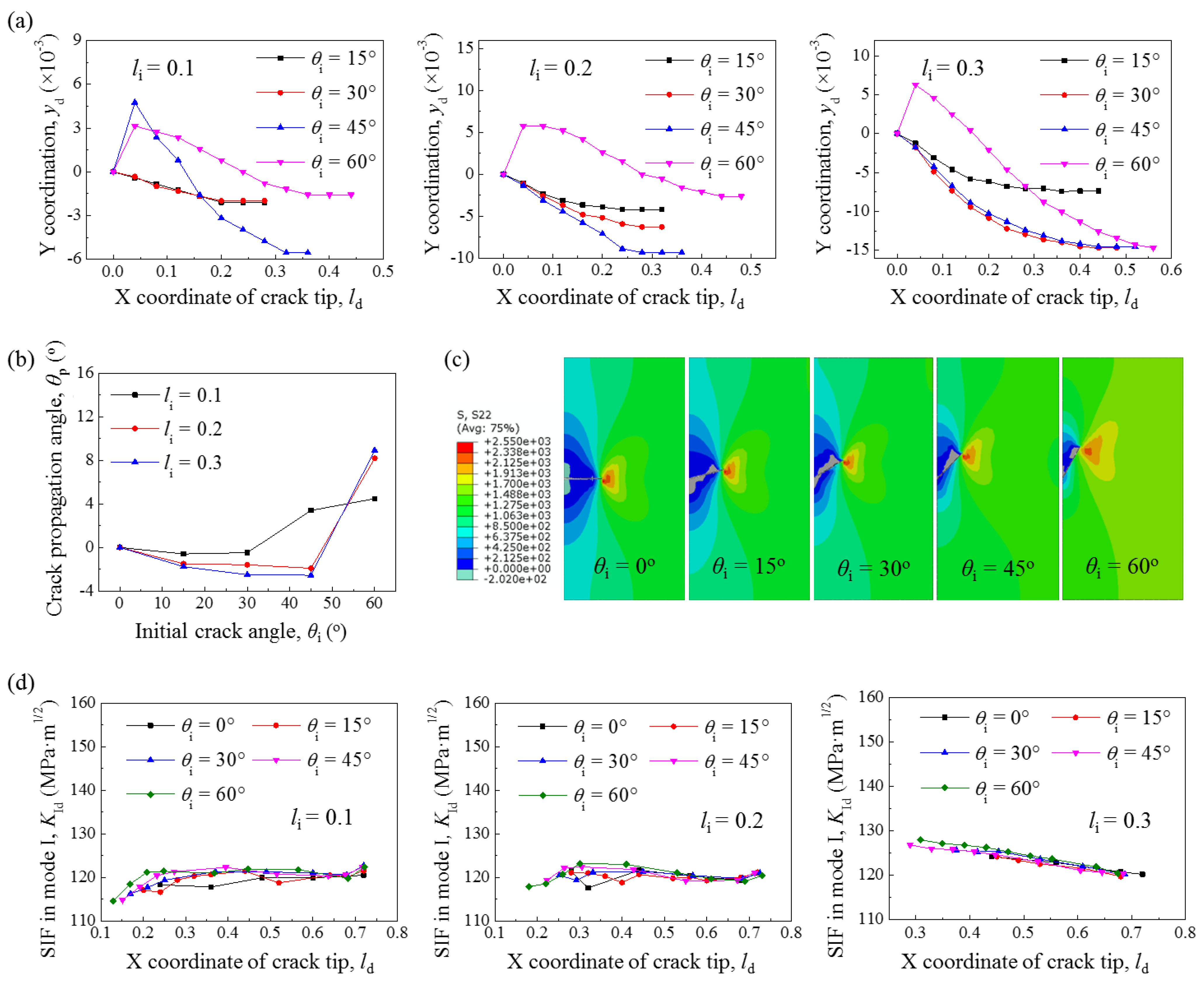
| Material Parameter | Young’s Modulus, E (GPa) | Yield Strength, σe (MPa) | Ultimate Strength, σu (MPa) | Density, ρ (kg/m3) | Poisson’s Ratio, μ | Elongation, η |
|---|---|---|---|---|---|---|
| Value | 187.20 | 1724.51 | 1986.9 | 7.8 × 103 | 0.35 | 0.166 |
| Material Parameters | A | B | n | C | (s−1) |
|---|---|---|---|---|---|
| Value | 1724.51 | 90.92 | 0.002 | 0.018 | 0.001 |
| Method | 1 | 2 | 3 | 4 | Computation Formula |
|---|---|---|---|---|---|
| Result Value (MPa·m1/2) | 114.41 | 141.16 | 72.39 | 121.77 | 123.5 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, H.; Hu, D.; Meng, K. Computational Investigation on Cracking Behaviors of AerMet 100. Metals 2022, 12, 1650. https://doi.org/10.3390/met12101650
Zhang H, Hu D, Meng K. Computational Investigation on Cracking Behaviors of AerMet 100. Metals. 2022; 12(10):1650. https://doi.org/10.3390/met12101650
Chicago/Turabian StyleZhang, Hongbo, Dayong Hu, and Kangpei Meng. 2022. "Computational Investigation on Cracking Behaviors of AerMet 100" Metals 12, no. 10: 1650. https://doi.org/10.3390/met12101650
APA StyleZhang, H., Hu, D., & Meng, K. (2022). Computational Investigation on Cracking Behaviors of AerMet 100. Metals, 12(10), 1650. https://doi.org/10.3390/met12101650







