The Dependence of the Strength of a Carbon Fiber/Aluminum Matrix Composite on the Interface Shear Strength between the Matrix and Fiber
Abstract
:1. Introduction
2. Materials and Methods
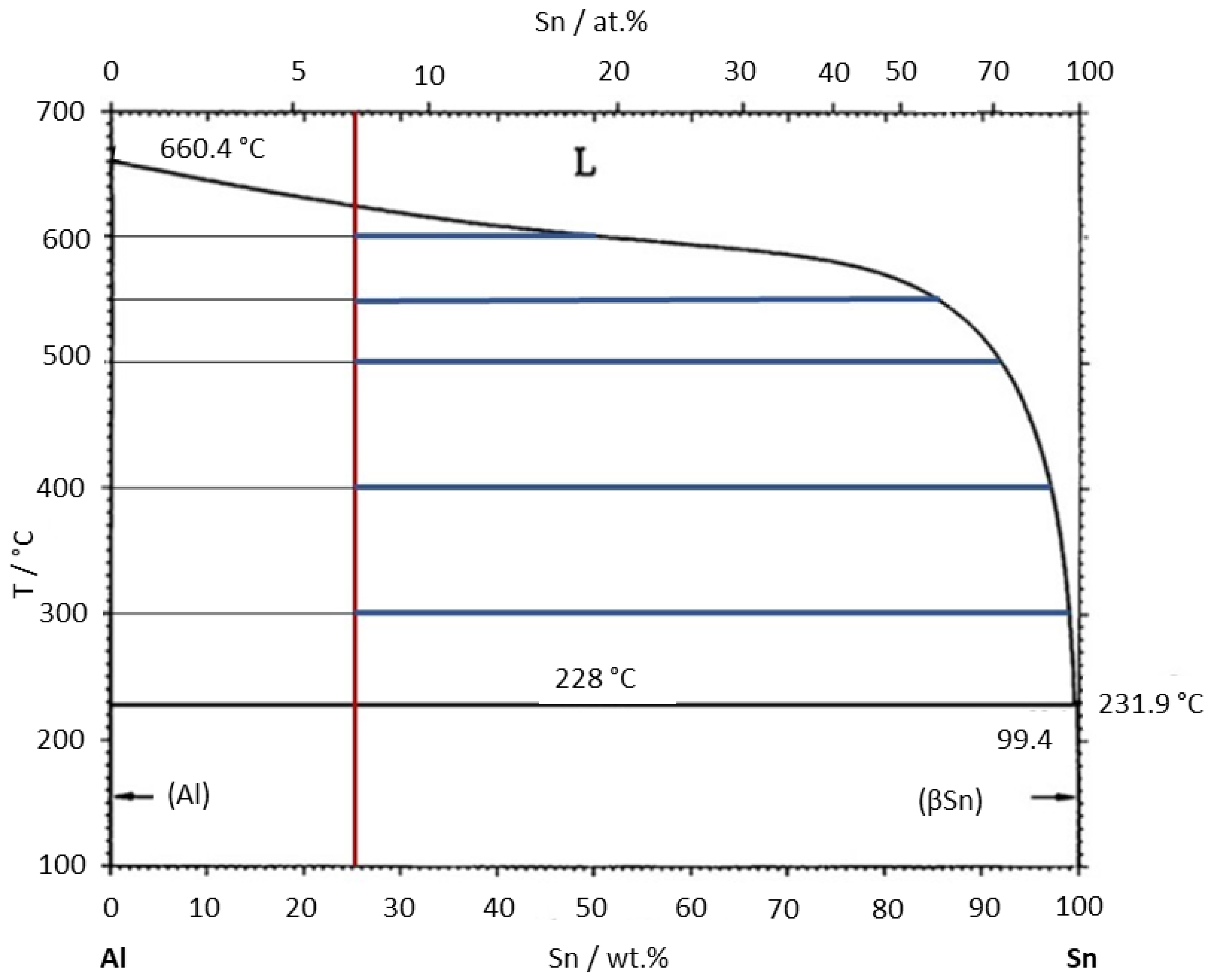
2.1. Production of a Composite Carbon–Aluminum Wire
2.2. Determination of Mechanical Properties
2.3. Scanning Electron Microscopy and Elemental Analysis
2.4. X-ray Diffraction Analysis
2.5. Strength of the Interface between the Matrix and the Fiber
3. Results
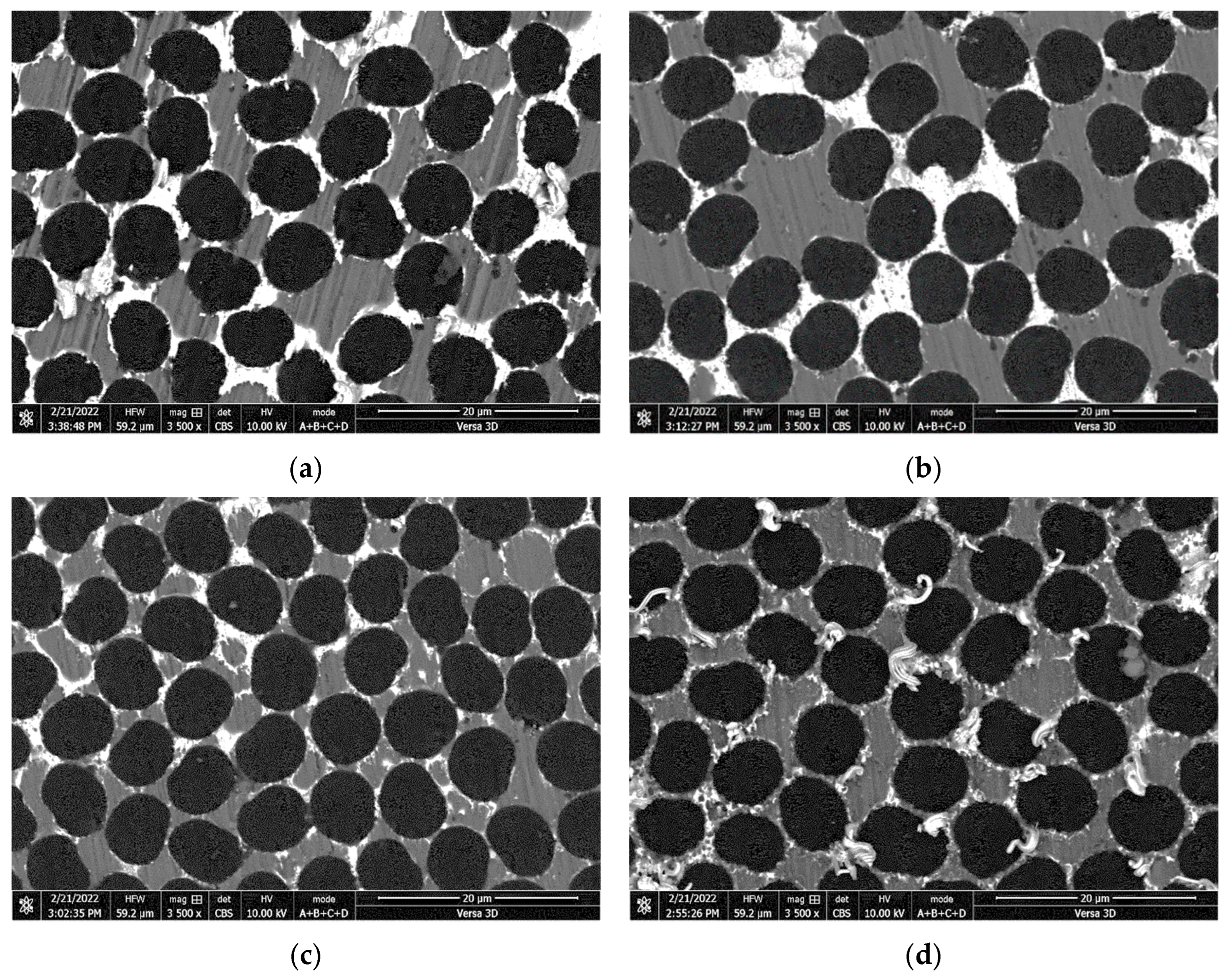
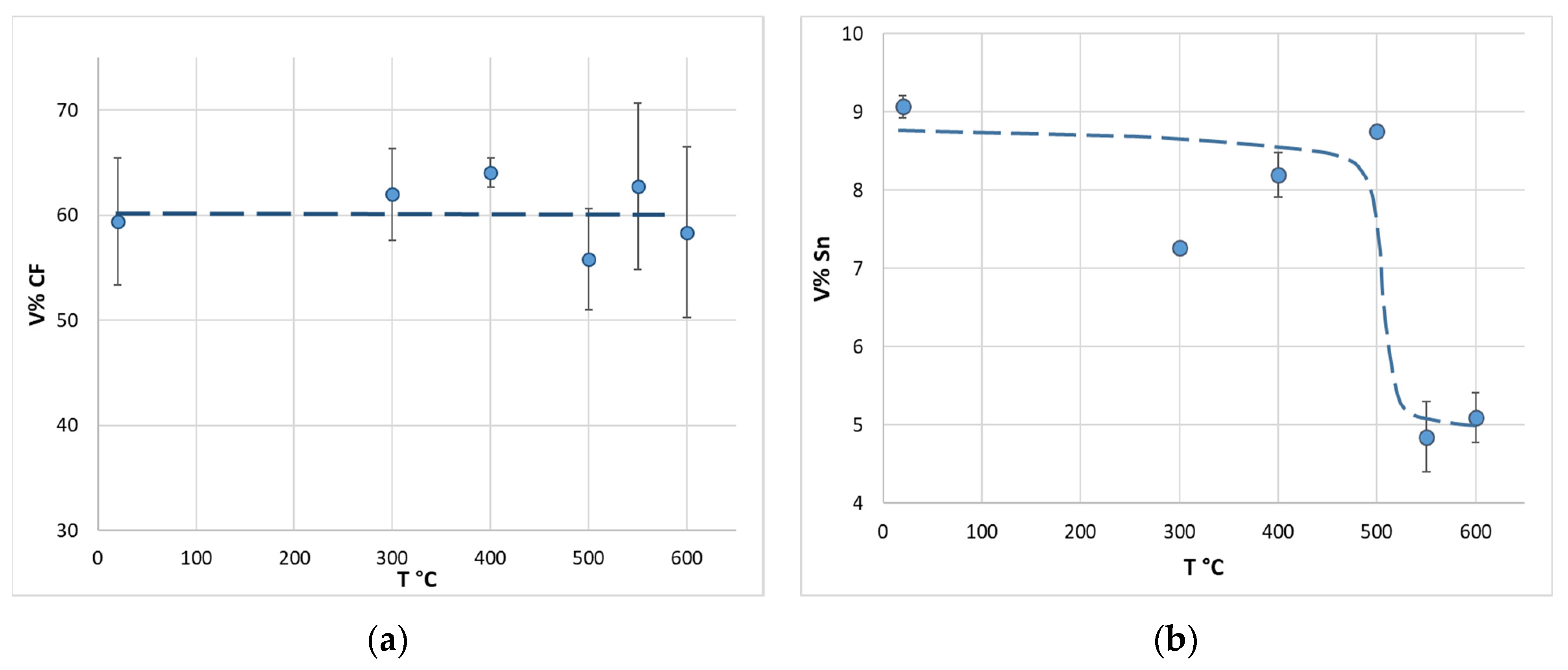
3.1. Microstructure
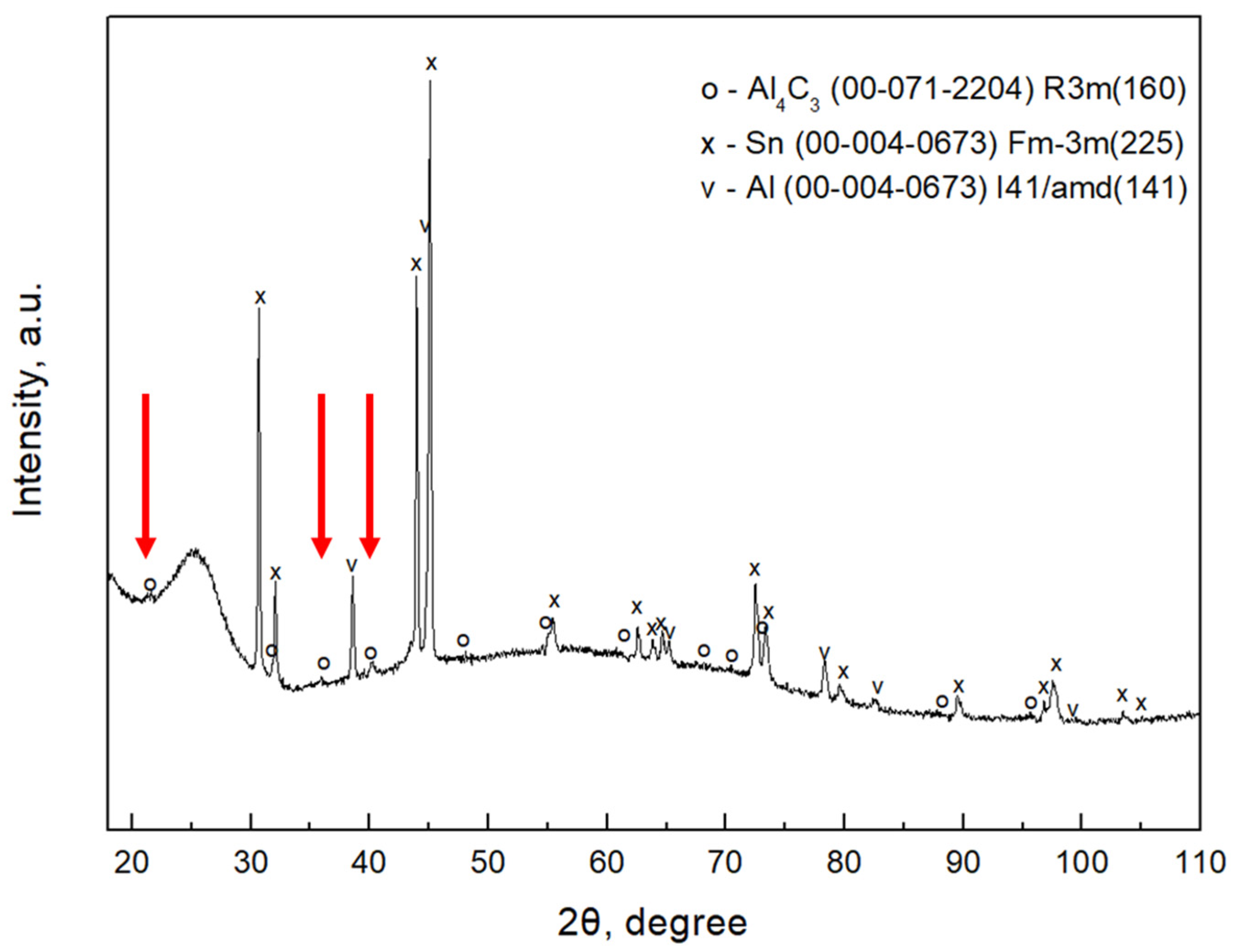
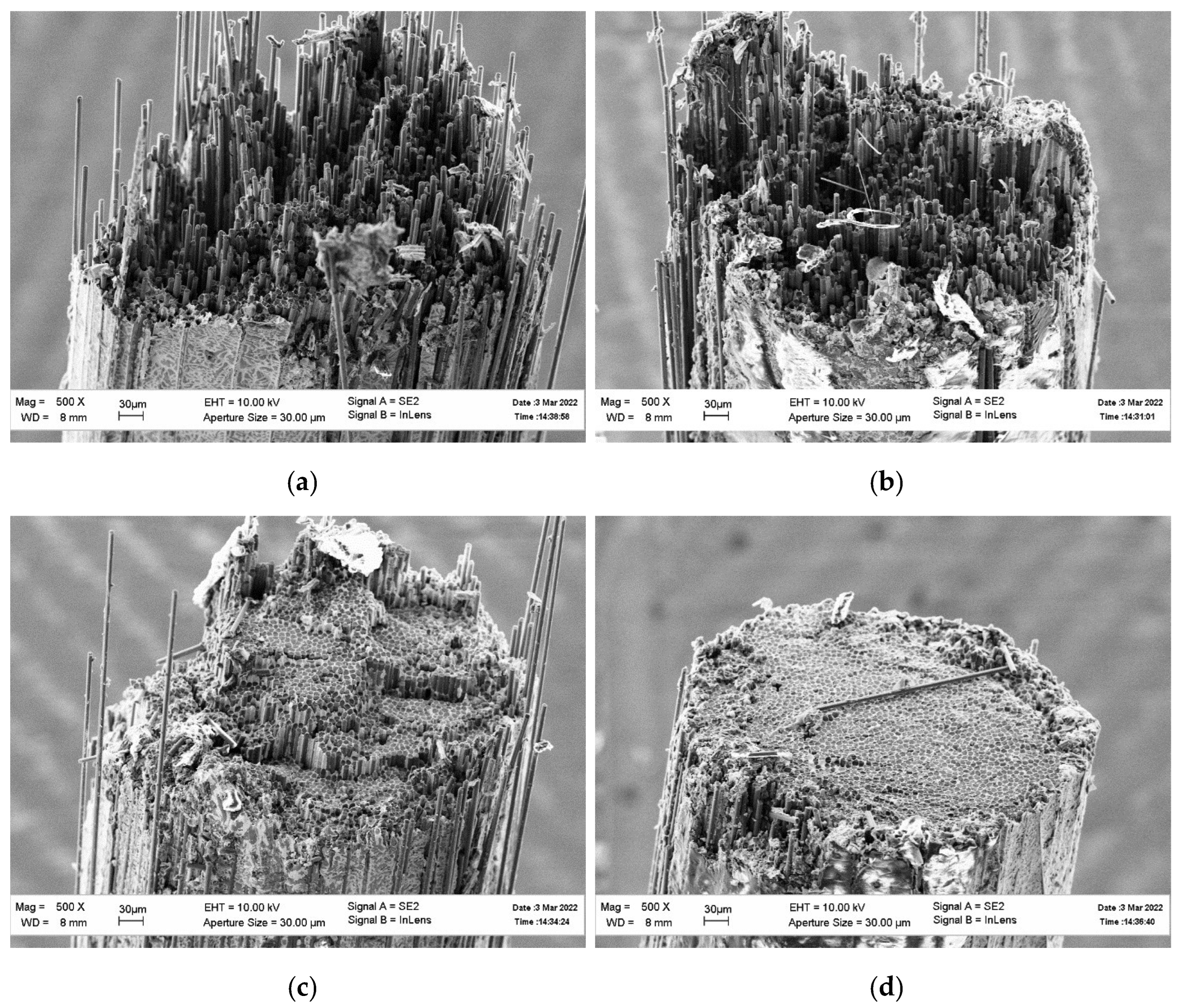
3.2. Fiber Surface
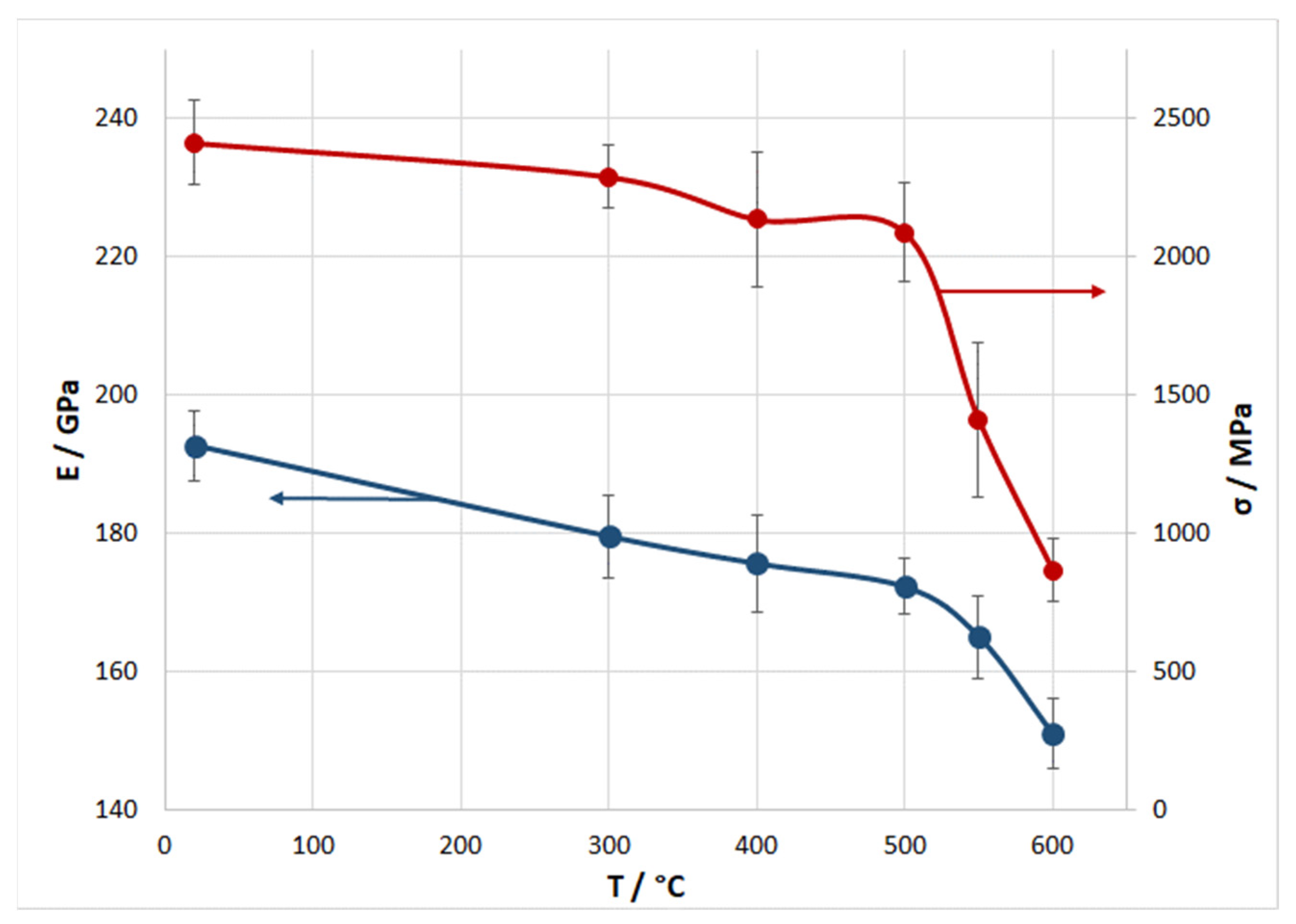
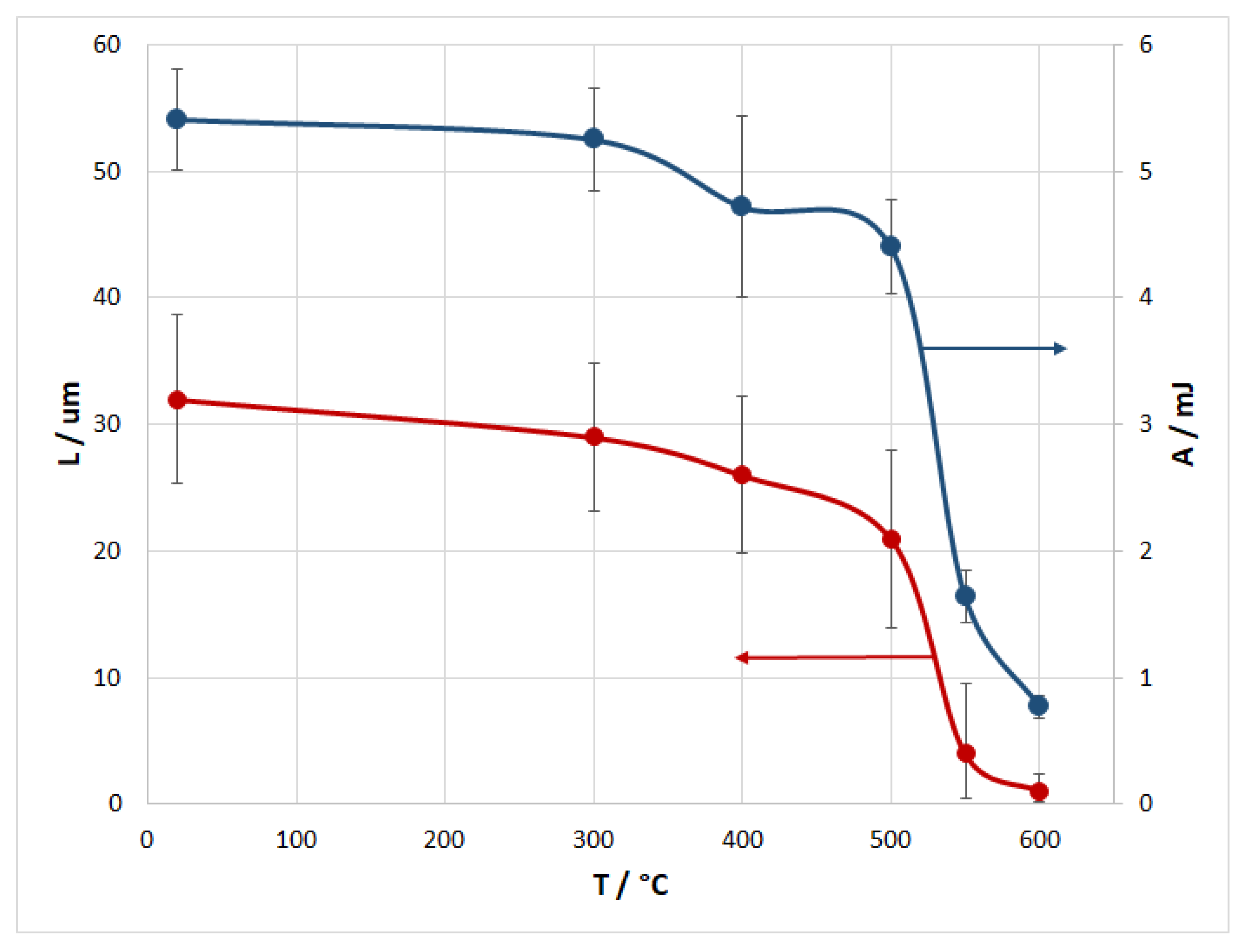
3.3. Strength and the Fracture Energy
4. Discussion
4.1. Microstructure
4.2. Fiber Surface
4.3. Strength and the Fracture Energy
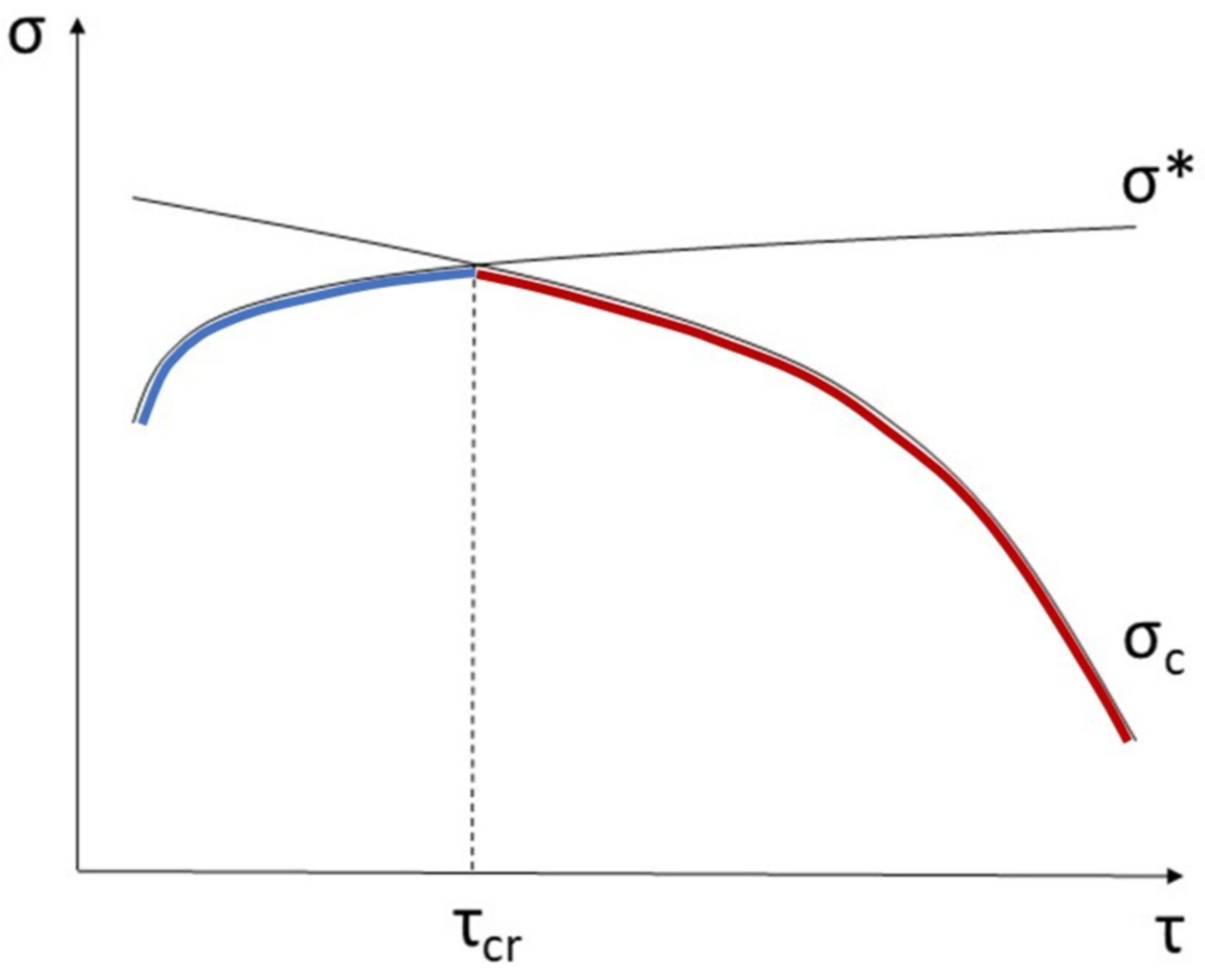
5. Numerical Calculations
5.1. Expression of the σ* Curve
5.2. Expression of the σc Curve
6. Conclusions
- Taking the example of a composite wire with an Al-25% Sn alloy matrix reinforced with carbon fiber, the dependence of the bending strength on the shear strength of the interface between the matrix and the fiber was determined. For this purpose, a series of composite wire specimens were obtained and subjected to heat treatment at temperatures from 300 to 600 °C. An analysis of the microstructure of the composite wire in an initial state and after heat treatment showed that there were almost no changes that could affect the strength of the composite.
- The examination of the surface of the carbon fiber extracted from the composite in an initial state and after heat treatment showed that the fiber in the initial state almost did not differ from the fiber in the delivered state. The fiber from the composite treated at 300 °C had a rougher surface. A further increase in the treatment temperature led to the formation of aluminum carbide crystals on the fiber surface, the size and number of which increased with increasing temperature.
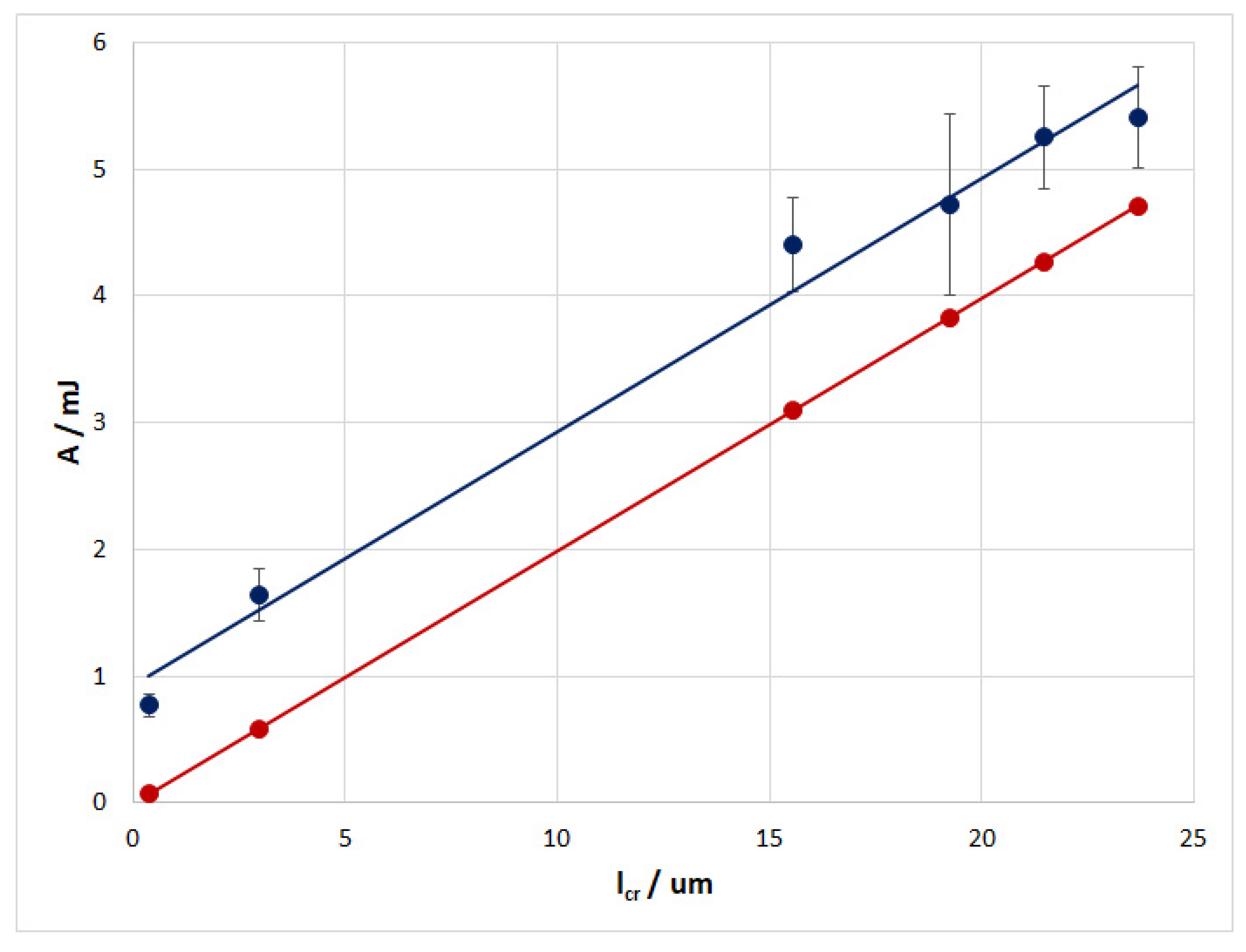
- The study of the fracture surfaces and the comparison with the results of the investigation of the fiber surface showed that the emergence of carbide crystals at the interface led to a decrease in the length of the pulled-out part of the fiber. This explicitly indicated a relation with an increase in the strength of the interface. The evaluation of the work of fracture of the composite with different strengths of the interface between the matrix and the fiber demonstrated that as the strength of the interface increased, the work of fracture decreased, due to the premature fracture of the composite through crack propagation in one plane. In the case of weak interfaces, the trajectory of crack propagation is not in one plane, which leads to an increase in the fracture work.
- The effect of the shear strength of the interface between the matrix and the fiber on the strength, the modulus of elasticity, and the nature of the composite fracture was studied. The highest bending strength of 2450 MPa was observed for composite wire samples with the lowest interface shear strength. With an increase in the shear strength of the interface, a decrease in the composite wire strength below 900 MPa was observed. Along with the strength, as the shear strength of the interface increased, the flexural modulus also decreased, from 190 to 150 GPa.
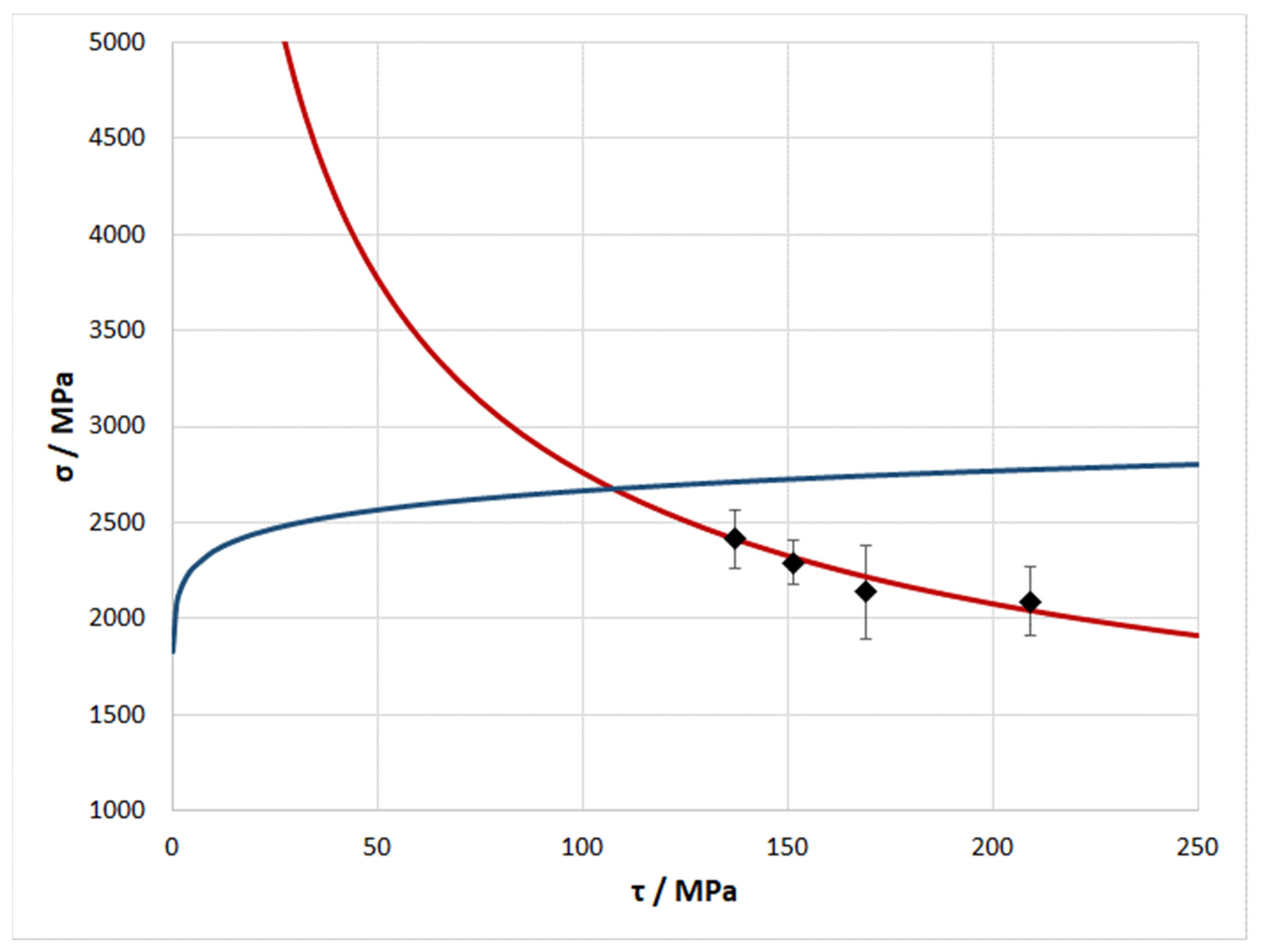
- Based on the experimental data, as well as the refinement of the mixture rule according to the Weibull distribution and the estimation of the critical stress of crack propagation according to the Griffith–Orowan–Irwin concept, a numerical assessment of the dependence of the composite strength on the shear strength of the interface was made. On the basis of this, the critical shear strength was calculated, at which the greatest strength of the composite was achieved, the values being τcr = 107 MPa and σmax = 2675 MPa, respectively. It has been shown that the contribution of the work of overcoming the friction force to the total work of fracture at relatively small values of shear strength can be several times greater than the total contribution of all other types of energy. This indicates that a composite with weak interfaces can remain stable even after the onset of plastic deformation of the matrix and the breakage of some of the fibers in the bulk of the material.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Curtin, W.A. Ultimate strengths of fibre-reinforced ceramics and metals. Composites 1993, 24, 98–102. [Google Scholar] [CrossRef]
- Deve, H.E.; McCullough, C. Continuous-fiber reinforced composites: A new generation. J. Miner. Met. Mater. Soc. 1995, 47, 33–37. [Google Scholar] [CrossRef]
- Evans, A. The mechanical properties of reinforced ceramic, metal and intermetallic matrix composites. Mater. Sci. Eng. A 1991, 143, 63–76. [Google Scholar] [CrossRef]
- Galyshev, S.; Orlov, V.; Atanov, B.; Kolyvanov, E.; Averichev, O.; Akopdzhanyan, T. The Effect of Tin Content on the Strength of a Carbon Fiber/Al-Sn-Matrix Composite Wire. Metals 2021, 11, 2057. [Google Scholar] [CrossRef]
- Vidal-Sétif, M.; Lancin, M.; Marhic, C.; Valle, R.; Raviart, J.-L.; Daux, J.-C.; Rabinovitch, M. On the role of brittle interfacial phases on the mechanical properties of carbon fibre reinforced Al-based matrix composites. Mater. Sci. Eng. A 1999, 272, 321–333. [Google Scholar] [CrossRef]
- Yu, J.K.; Li, H.L.; Shang, B.L. A functionally gradient coating on carbon fibre for C/Al composites. J. Mater. Sci. 1994, 29, 2641–2647. [Google Scholar] [CrossRef]
- Zhu, C.; Su, Y.; Zhang, D.; Ouyang, Q. Effect of Al2O3 coating thickness on microstructural characterization and mechanical properties of continuous carbon fiber reinforced aluminum matrix composites. Mater. Sci. Eng. A 2020, 793, 139839. [Google Scholar] [CrossRef]
- Gomzin, A.I.; Gallyamova, R.F.; Galyshev, S.N.; Paramonov, R.M.; Zaripov, N.G.; Musin, F.F. The effect of titanium dioxide coated carbon fibers on the aluminum alloy matrix composite strength. IOP Conf. Ser. Mater. Sci. Eng. 2019, 537, 022057. [Google Scholar] [CrossRef]
- Galyshev, S.; Postnova, E. Electrochemical Deposition of SiO2-Coatings on a Carbon Fiber. Fibers 2021, 9, 33. [Google Scholar] [CrossRef]
- Wang, X.; Jiang, D.; Wu, G.; Li, B.; Li, P. Effect of Mg content on the mechanical properties and microstructure of Grf/Al composite. Mater. Sci. Eng. A 2008, 497, 31–36. [Google Scholar] [CrossRef]
- Zhang, Y.; Wu, G. Comparative study on the interface and mechanical properties of T700/Al and M40/Al composites. Rare Metals 2010, 29, 102–107. [Google Scholar] [CrossRef]
- Galyshev, S.; Gomzin, A.; Gallyamova, R.; Khodos, I.; Musin, F. On the liquid-phase technology of carbon fiber/aluminum matrix composites. Int. J. Miner. Met. Mater. 2019, 26, 1578–1584. [Google Scholar] [CrossRef]
- Lee, M.; Choi, Y.; Sugio, K.; Matsugi, K.; Sasaki, G. Effect of aluminum carbide on thermal conductivity of the unidirectional CF/Al composites fabricated by low pressure infiltration process. Compos. Sci. Technol. 2014, 97, 1–5. [Google Scholar] [CrossRef]
- Galyshev, S. On the Strength of the CF/Al-Wire Depending on the Fabrication Process Parameters: Melt Temperature, Time, Ultrasonic Power, and Thickness of Carbon Fiber Coating. Metals 2021, 11, 1006. [Google Scholar] [CrossRef]
- Massalski, T.B.; Okamoto, H.; Subramanian, P.; Kacprzak, L.; Scott, W.W. (Eds.) Binary Alloy Phase Diagrams; American Society for Metals: Metals Park, OH, USA, 1986; Volume 1, p. 2. [Google Scholar]
- Oden, L.L.; Gokcen, N.A. Sn-C and Al-Sn-C phase diagrams and thermodynamic properties of C in the alloys: 1550 °C to 2300 °C. Met. Mater. Trans. A 1993, 24, 53–58. [Google Scholar] [CrossRef]
- Mileiko, S.T. Metal and Ceramic Based Composite; Elsevier: Amsterdam, The Netherlands, 1997; p. 690. [Google Scholar]
- Zhong, K.; Zhou, J.; Zhao, C.; Yun, K.; Qi, L. Effect of interfacial transition layer with CNTs on fracture toughness and failure mode of carbon fiber reinforced aluminum matrix composites. Compos. Part A Appl. Sci. Manuf. 2022, 163, 107201. [Google Scholar] [CrossRef]
- Parton, V.Z. Fracture Mechanics: From Theory to Practice; CRC Press: Boca Raton, FL, USA, 1992; p. 208. ISBN 9782881247804. [Google Scholar]
- Watt, W.; Perov, B.V. Strong Fibres Handbook of Composites; North-Holland Publishing Company: Amsterdam, The Netherlands, 1985; Volume 1, p. 752. ISBN 0-444-87505-0. [Google Scholar] [CrossRef]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Galyshev, S.; Atanov, B. The Dependence of the Strength of a Carbon Fiber/Aluminum Matrix Composite on the Interface Shear Strength between the Matrix and Fiber. Metals 2022, 12, 1753. https://doi.org/10.3390/met12101753
Galyshev S, Atanov B. The Dependence of the Strength of a Carbon Fiber/Aluminum Matrix Composite on the Interface Shear Strength between the Matrix and Fiber. Metals. 2022; 12(10):1753. https://doi.org/10.3390/met12101753
Chicago/Turabian StyleGalyshev, Sergei, and Bulat Atanov. 2022. "The Dependence of the Strength of a Carbon Fiber/Aluminum Matrix Composite on the Interface Shear Strength between the Matrix and Fiber" Metals 12, no. 10: 1753. https://doi.org/10.3390/met12101753
APA StyleGalyshev, S., & Atanov, B. (2022). The Dependence of the Strength of a Carbon Fiber/Aluminum Matrix Composite on the Interface Shear Strength between the Matrix and Fiber. Metals, 12(10), 1753. https://doi.org/10.3390/met12101753







