Evaluation of the Degree of Dissociation of A Congruent Compound Fe2Ti across the Bjerrum–Guggenheim Coefficient
Abstract
1. Introduction
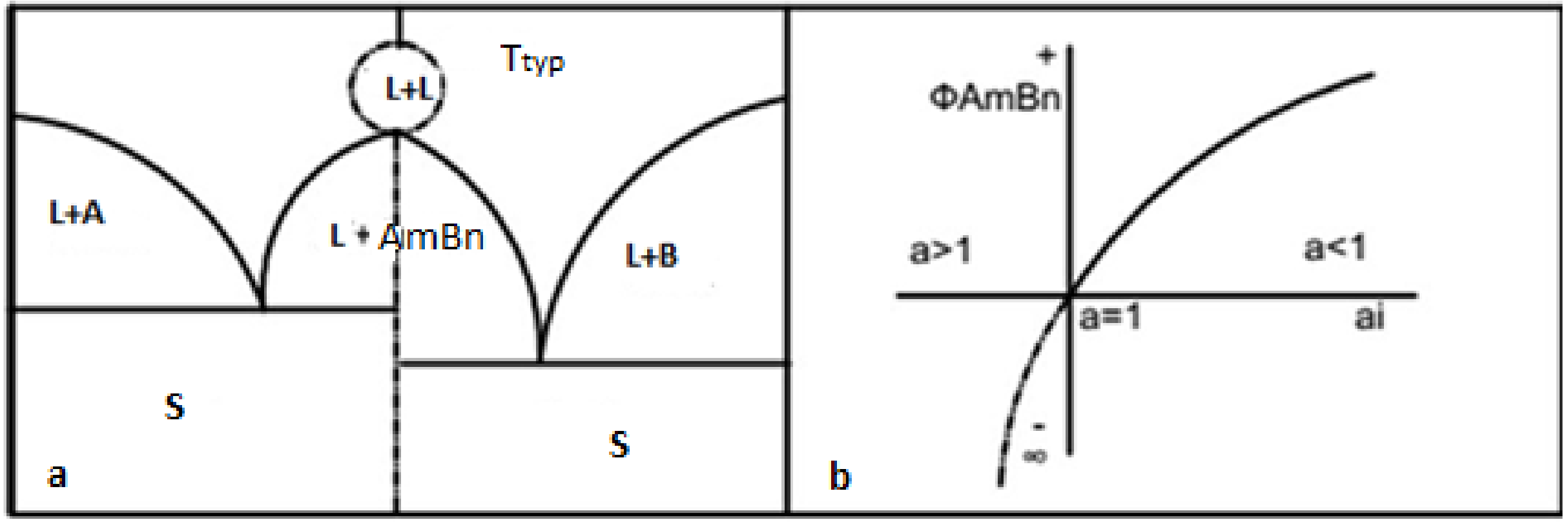
2. The Equilibrium Method to Calculate Dissociation Parameters of the Congruent Compound Based on the Monovariant Equilibrium Line Analysis
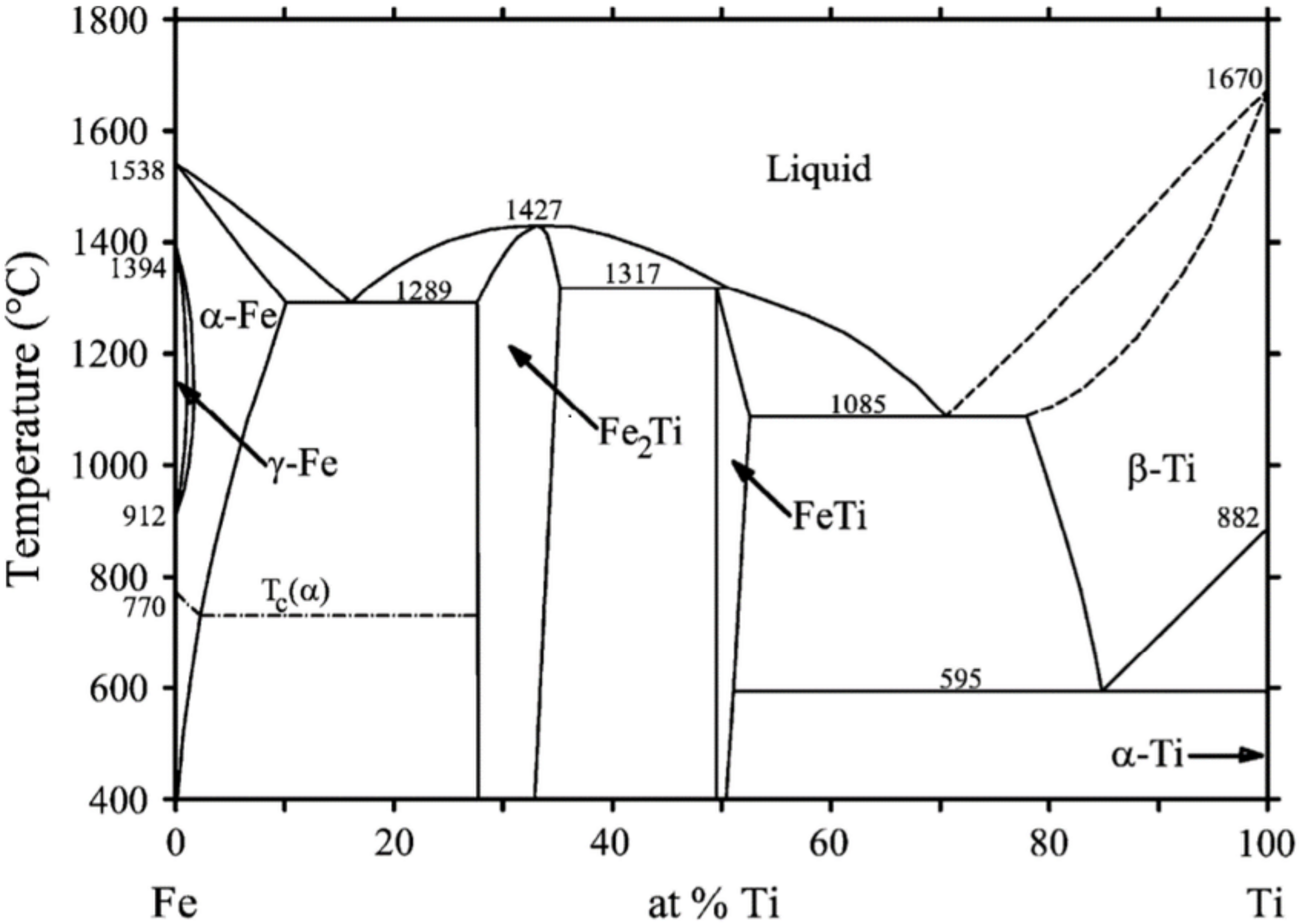
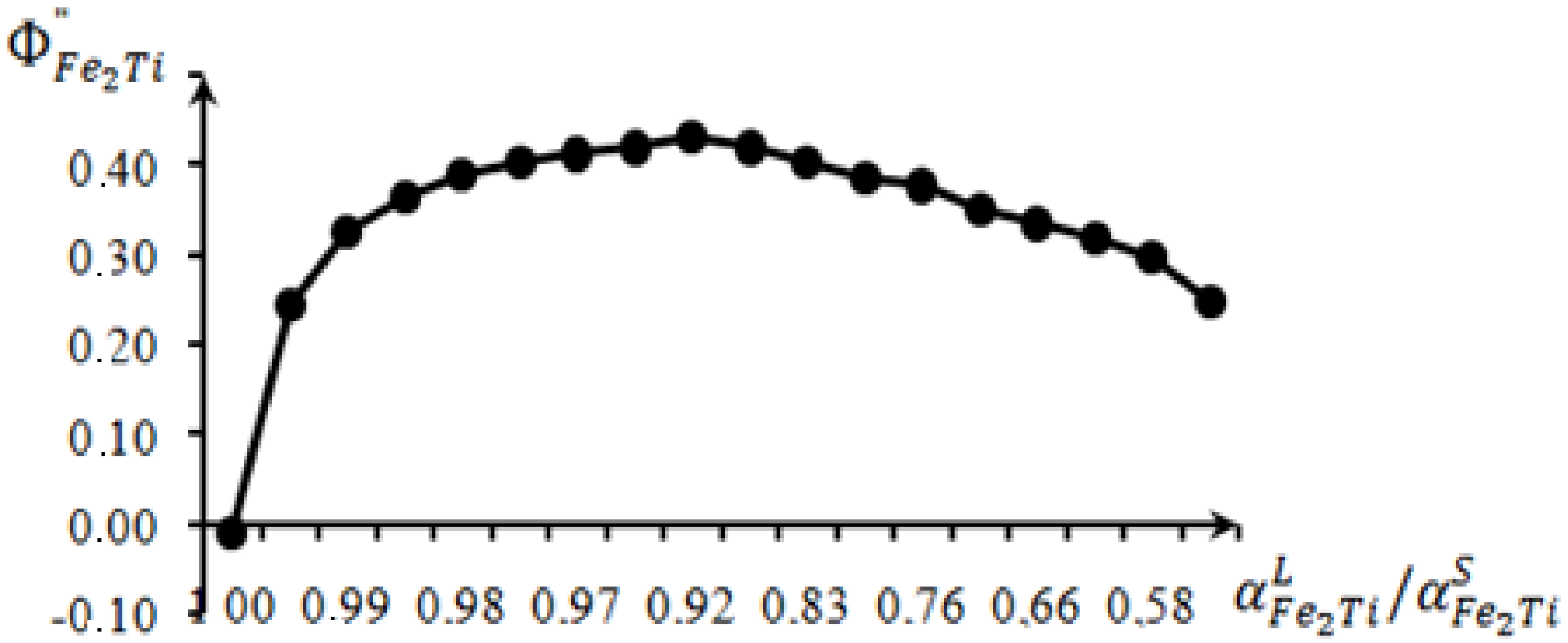
3. A Detailed Study of the Diagrams
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kulikov, I.S. Thermal dissociation of compounds. In Metallurgy; Springer: Cham, Switzerland, 1966; p. 251. (In Russian) [Google Scholar]
- Kulikov, I.S. Thermodynamics of oxygen. In Metallurgy; Springer: Cham, Switzerland, 1986; p. 344. (In Russian) [Google Scholar]
- Kulikov, I.S. Thermodynamicscarbides and Nitrides. In Metallurgy; Springer: Cham, Switzerland, 2012; pp. 198–320. (In Russian) [Google Scholar]
- Patrick Fleming. Pressure Dependence of Kp—Le Châtelier’s Principle, 13 April 2022. Available online: https://chem.libretexts.org/Bookshelves/Physical_and_Theoretical_Chemistry_Textbook_Maps (accessed on 16 October 2022).
- Denisov, E.T.; Sarkisov, O.M.; Likhtenshtein, G.I. Reactions of ions and radical ions. In Chemical Kinetics, Fundamentals and New Developments; Elsevier: Amsterdam, The Netherlands, 2003; pp. 250–304. [Google Scholar]
- Ozerov, R.P.; Vorobyev, A.A. Molecular Physics, Physics for Chemists; Elsevier: Amsterdam, The Netherlands, 2007; pp. 169–250. [Google Scholar]
- Glazov, V.M.; Pavlova, L.M. Chemical thermodynamics and phase equilibria. In Metallurgy; Springer: Cham, Switzerland, 1981; p. 336. [Google Scholar]
- Kurnakov, N.S. Fav. Tr. T.1.–M.; Publishing House of the Academy of Sciences of the USSR: Moscow, Russian, 1961; p. 635. (In Russian) [Google Scholar]
- Glazov, V.M.; Pavlova, L.M. Chemical thermodynamics and phase equilibria. In Metallurgy; Springer: Cham, Switzerland, 1988; p. 555. [Google Scholar]
- Osipov, A.A.; Ivanov, K.D.; Ashadullin, R.S. Equilibrium dissociation model. In Nuclear Reactor Constants; State Scientific Centre of the Russian Federation: Moscow, Russia, 2018. (In Russian) [Google Scholar]
- Baisanov, S.O.; Tolokonnikova, V.V. Development of the Fundamental Foundations of the Theory of the Liquid State for Binary Systems from the Standpoint of Thermodynamics of Heterogeneous Phase Equilibria; Glassir: Karaganda, Kazahstan, 2017; p. 129. (In Russian) [Google Scholar]
- Ponamarev, A.B.; Pikuleva, A.A. Methodology of Scientific Research; Publishing House Perm National Research Polytechnic University: Perm, Russia, 2014; p. 186. (In Russian) [Google Scholar]
- Tolokonnikova, V.; Baisanov, S.; Narikbayeva, G.; Narikbayeva, I. Assessment of dissociation rate of FeCr2O4 using the Bjerrum-Guggenheim coefficient. Metalurgija 2021, 60, 303–305. [Google Scholar]
- Kubashevsky, O. State diagrams of iron-based binary systems. In Metallurgy; Springer: Cham, Switzerland, 1985; p. 184. (In Russian) [Google Scholar]
- Lukas, H.L.; Fries, S.G.; Sundman, B. Computational Thermodynamics, the Calphad Method; Cambridge University Press: Cambridge, UK, 2007; p. 324. [Google Scholar]
- Krestovnikov, A.N.; Vladimirov, L.P.; Gulyanitsky, V.S.; Fischer, A.Y. Handbook on Calculations of Equilibria of Metallurgical Reactions; GNTI Literature on Ferrous and Non-Ferrous Metallurgy: Moscow, Russia, 1968; p. 416. (In Russian) [Google Scholar]
- Ruzinov, L.P.; Gulyanitsky, V.S. Equilibrium transformations of metallurgical reactions. In Metallurgy; Springer: Cham, Switzerland, 1975; p. 417. (In Russian) [Google Scholar]
- Database. Available online: http://www.outokumpu.fi/hsc/ (accessed on 16 October 2022).
- Zakharov, M.A. Calculation of the types of the main state diagrams of binary solutions within the framework of a generalized lattice model. Bull. Novgorod State Univ. 2016, 98, 22–26. (In Russian) [Google Scholar]
- Shakhnazarov, K.Y. Signs of intermediate phases in Al-Si, Fe-C and Al-Cu systems. Bull. MSTU 2016, 14, 71–77. (In Russian) [Google Scholar]
- Voronin, G.F. New possibilities of thermodynamic calculation and construction of diagrams of phase states of heterogeneous systems. J. Phys. Chem. 2003, 77, 1874–1883. (In Russian) [Google Scholar]
- Moshchenskaya, E.Y.; Clepushkin, V.V. Method of liquids curves constructing of double eutectic systems. J. Inorg. Chem. 2015, 60, 78–84. [Google Scholar]
- Akberdin, A.A.; Kim, A.S.; Sultangaziev, R.B. Planning of numerical and physical experiment in simulation of technological processes. Izv. Ferr. Metall. 2018, 9, 737–742. [Google Scholar] [CrossRef]
- Nurgali, N.Z.; Kelamanov, B.S.; Tazhiev, E.B.; Sariev, O.R.; Abdirashit, A.M.; Burumbayev, A.G.; Zayakin, O.V. Modeling of metal systems Fe-Ti, Ti-Si, Ti-Al Chemical and metallurgical sciences. In KazUTZU Khabarshysy; Department of Physics, Mathematics And Digital Technology: Kostanay, Kazahstan, 2021; pp. 115–121. (In Russian) [Google Scholar]
| T, K. | ||||
|---|---|---|---|---|
| 1700 | 0.66667 | 1.00001 | 1.0000 | 0.00000 |
| 1699 | 0.67070 | 0.96456 | 0.9949 | 0.14031 |
| 1698 | 0.66689 | 0.99799 | 0.9899 | 0.23459 |
| 1697 | 0.66691 | 0.99781 | 0.9849 | 0.29215 |
| 1696 | 0.66693 | 0.99763 | 0.9799 | 0.33315 |
| 1695 | 0.66695 | 0.99745 | 0.9749 | 0.35075 |
| 1694 | 0.66697 | 0.99727 | 0.9700 | 0.36756 |
| 1693 | 0.66710 | 0.99611 | 0.9651 | 0.37043 |
| 1683 | 0.67000 | 0.97059 | 0.9168 | 0.35836 |
| 1673 | 0.72500 | 0.61111 | 0.8704 | 0.33753 |
| 1663 | 0.75000 | 0.50000 | 0.8258 | 0.32054 |
| 1653 | 0.77600 | 0.40580 | 0.7830 | 0.30373 |
| 1648 | 0.78600 | 0.37413 | 0.7623 | 0.28073 |
| 1633 | 0.81600 | 0.29114 | 0.7026 | 0.26019 |
| 1623 | 0.84900 | 0.21633 | 0.6649 | 0.24180 |
| 1613 | 0.85900 | 0.19638 | 0.6288 | 0.22312 |
| 1600 | 0.87800 | 0.16138 | 0.5841 | 0.20019 |
| T, K | |||||
|---|---|---|---|---|---|
| 1700 | 0.9997 | 1.0000 | 1.00000 | 0.00000 | −0.00971 |
| 1699 | 0.96731 | 0.9795 | 0.99495 | 0.15233 | 0.24500 |
| 1698 | 0.95273 | 0.9695 | 0.98992 | 0.20924 | 0.32754 |
| 1697 | 0.93786 | 0.9595 | 0.8491 | 0.23704 | 0.36749 |
| 1696 | 0.92304 | 0.9494 | 0.97992 | 0.25334 | 0.39048 |
| 1695 | 0.91004 | 0.9393 | 0.97494 | 0.26918 | 0.40501 |
| 1694 | 0.89665 | 0.9292 | 0.96999 | 0.27929 | 0.41472 |
| 1693 | 0.88274 | 0.9191 | 0.96506 | 0.28516 | 0.42143 |
| 1683 | 0.73946 | 0.8185 | 0.91678 | 0.28787 | 0.43379 |
| 1673 | 0.61807 | 0.7197 | 0.87038 | 0.28853 | 0.42198 |
| 1663 | 0.51198 | 0.6240 | 0.82581 | 0.28588 | 0.40576 |
| 1653 | 0.42081 | 0.5327 | 0.78303 | 0.28257 | 0.38841 |
| 1648 | 0.38028 | 0.4892 | 0.76229 | 0.28073 | 0.37965 |
| 1633 | 0.27809 | 0.3687 | 0.70262 | 0.27577 | 0.35369 |
| 1623 | 0.22464 | 0.2978 | 0.66491 | 0.27330 | 0.33691 |
| T, K. | ||||||
|---|---|---|---|---|---|---|
| 1700 | 0.6667 | 0.6667 | 1.0000 | 1.000 | 1 | 0 |
| 1693 | 0.6350 | 0.6615 | 0.8699 | 0.9771 | 0.9641 | 0.2623 |
| 1683 | 0.6010 | 0.6550 | 0.7531 | 0.9493 | 0.9145 | 0.3151 |
| 1673 | 0.5780 | 0.6510 | 0.6848 | 0.9327 | 0.8670 | 0.3770 |
| 1663 | 0.5590 | 0.6470 | 0.6338 | 0.9164 | 0.8214 | 0.4315 |
| 1653 | 0.5450 | 0.6440 | 0.5989 | 0.9045 | 0.7777 | 0.4905 |
| 1648 | 0.5450 | 0.6440 | 0.5989 | 0.9045 | 0.7565 | 0.5443 |
| 1633 | 0.5230 | 0.6400 | 0.5482 | 0.8889 | 0.6957 | 0.6036 |
| 1623 | 0.5140 | 0.6370 | 0.5288 | 0.8774 | 0.6573 | 0.6585 |
| 1613 | 0.5060 | 0.6340 | 0.5121 | 0.8661 | 0.6206 | 0.7128 |
| 1600 | 0.4930 | 0.6300 | 0.4862 | 0.8514 | 0.5754 | 0.7665 |
| T, K | |||||
|---|---|---|---|---|---|
| 1700 | 1.0000 | 1.0000 | 0.00000 | 0.2735 | 1.0000 |
| 1699 | 0.9706 | 0.99495 | 0.169597 | 0.28495 | 0.9824 |
| 1698 | 0.962 | 0.98992 | 0.261448 | 0.29627 | 0.9664 |
| 1697 | 0.9535 | 0.98491 | 0.319282 | 0.30746 | 0.9517 |
| 1696 | 0.9472 | 0.97992 | 0.37384 | 0.31852 | 0.9383 |
| 1695 | 0.9409 | 0.97494 | 0.416694 | 0.32944 | 0.9259 |
| 1694 | 0.936 | 0.96999 | 0.460327 | 0.34022 | 0.9143 |
| 1693 | 0.9306 | 0.9651 | 0.494599 | 0.35088 | 0.9036 |
| 1683 | 0.8514 | 0.9168 | 0.539914 | 0.44999 | 0.8244 |
| 1673 | 0.7821 | 0.8704 | 0.564705 | 0.53545 | 0.7716 |
| 1663 | 0.7255 | 0.8258 | 0.596385 | 0.60696 | 0.7296 |
| 1653 | 0.6765 | 0.783 | 0.625741 | 0.66422 | 0.692 |
| 1648 | 0.6601 | 0.7623 | 0.653437 | 0.68738 | 0.6738 |
| 1633 | 0.5989 | 0.7026 | 0.688427 | 0.73438 | 0.6184 |
| 1623 | 0.5638 | 0.6649 | 0.712218 | 0.74638 | 0.5788 |
| 1613 | 0.5331 | 0.6288 | 0.737472 | 0.74228 | 0.5352 |
| Steh. Coefficient | Substance | T, K | ΔHo J/mol | ΔSo J/mol deg | A | B | C |
|---|---|---|---|---|---|---|---|
| 2 | Fe | 298.16 | 0.00 | 27.20 | 17.50 | 24.80 | 0.00 |
| 1033 | 5104.00 | 4.90 | 37.70 | 0.00 | 0.00 | ||
| 1185 | 912.10 | 0.80 | 7.70 | 19.50 | 0.00 | ||
| 1667 | 1108.00 | 0.70 | 43.90 | 0.00 | 0.00 | ||
| 1811 | 13,811.00 | 7.60 | 43.50 | 0.00 | 0.00 | ||
| 1 | Ti | 298.16 | −113.000 | 7.325 | 6.226 | 1.444 | −0.617 |
| 1120 | 0.000 | 0.000 | 5.280 | 2.400 | 0.000 | ||
| 1933 | 3.160 | 3.591 | 4.340 | 2.200 | 0.000 | ||
| 1941 | 4.450 | 3.562 | 8.500 | 0.000 | 0.000 | ||
| 1 | Fe2Ti | 298.16 | −87,446.00 | 74.48 | 80.96 | 13.81 | −13.18 |
| 1700 | 125,000.00 | 73.53 | 0.00 | 0.00 | 0.00 |
| Crystallization Region Fe2Ti on the Plot-Fe2Ti-Fe | Crystallization Region Fe2Ti on the Plot Fe2Ti-Ti | ||||
|---|---|---|---|---|---|
| ΔG0(KJ) | Kp | α | ΔG0(KJ) | Kp | α |
| 155,177.421 | 0.0000171 | 0.004129518 | 155,177.421 | 0.0000171 | 0.004129518 |
| 154,937.683 | 0.0000166 | 4.92005 × 10−5 | 154,937.683 | 0.0000166 | 0.000110688 |
| 154,593.986 | 0.0000159 | 1.94735 × 10−5 | 154,593.986 | 0.0000159 | 4.8043 × 10−5 |
| 154,248.894 | 0.0000153 | 1.1572 × 10−5 | 154,248.894 | 0.0000153 | 3.24136 × 10−5 |
| 153,902.441 | 0.0000146 | 7.58367 × 10−6 | 153,902.441 | 0.0000146 | 2.43673 × 10−5 |
| 153,554.661 | 0.0000140 | 5.37735 × 10−6 | 153,554.661 | 0.0000140 | 1.98397 × 10−5 |
| 153,380.285 | 0.0000138 | 5.52517 × 10−6 | 153,380.285 | 0.0000138 | 1.94241 × 10−5 |
| 152,855.263 | 0.0000129 | 2.97747 × 10−6 | 152,855.263 | 0.0000129 | 1.43308 × 10−5 |
| 152,503.717 | 0.0000123 | 2.31103 × 10−6 | 152,503.717 | 0.0000123 | 1.24875 × 10−5 |
| 152,150.985 | 0.0000118 | 1.72814 × 10−6 | 152,150.985 | 0.0000118 | 1.09995 × 10−5 |
| 151,690.724 | 0.0000112 | 0.28527 × 10−6 | 151,690.724 | 0.0000112 | 9.08643 × 10−6 |
| 150,370.842 | 0.0000094 | 1.19363 × 10−6 | - | - | - |
| T, K | |||
|---|---|---|---|
| 1699.6 | 0.99798 | 0.13668 | 0.9853 |
| 1699.7 | 0.99848 | 0.10868 | 0.9861 |
| 1699.8 | 0.99899 | 0.07591 | 0.9868 |
| 1699.9 | 0.99949 | 0.03706 | 0.9864 |
| 1700.0 | 1.00000 | −0.00971 | 1.0000 |
| 1700.1 | 1.00051 | −0.06709 | 0.99249 |
| 1700.2 | 1.00101 | −0.13912 | 0.99275 |
| 1700.3 | 1.00152 | −0.3222 | 0.99349 |
| 1700.4 | 1.00203 | −0.35716 | 0.99435 |
| 1700.5 | 1.00253 | −0.53358 | 0.99527 |
| 1700.6 | 1.00304 | −0.80149 | 0.99622 |
| 1700.7 | 1.00355 | −1.25675 | 0.99719 |
| 1700.8 | 1.00405 | −2.20115 | 0.99816 |
| 1700.9 | 1.00456 | −5.33617 | 0.99915 |
| 1701.0 | 1.00507 | 37.11261 | 1.00000 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tolokonnikova, V.V.; Baisanov, S.; Yerekeyeva, G.S.; Narikbayeva, G.I.; Korsukova, I.Y. Evaluation of the Degree of Dissociation of A Congruent Compound Fe2Ti across the Bjerrum–Guggenheim Coefficient. Metals 2022, 12, 2132. https://doi.org/10.3390/met12122132
Tolokonnikova VV, Baisanov S, Yerekeyeva GS, Narikbayeva GI, Korsukova IY. Evaluation of the Degree of Dissociation of A Congruent Compound Fe2Ti across the Bjerrum–Guggenheim Coefficient. Metals. 2022; 12(12):2132. https://doi.org/10.3390/met12122132
Chicago/Turabian StyleTolokonnikova, Vera Vladimirovna, Sailaubai Baisanov, Gauhar Sarsengaliqyzy Yerekeyeva, Gulnar Itimirovna Narikbayeva, and Irina Yaroslavovna Korsukova. 2022. "Evaluation of the Degree of Dissociation of A Congruent Compound Fe2Ti across the Bjerrum–Guggenheim Coefficient" Metals 12, no. 12: 2132. https://doi.org/10.3390/met12122132
APA StyleTolokonnikova, V. V., Baisanov, S., Yerekeyeva, G. S., Narikbayeva, G. I., & Korsukova, I. Y. (2022). Evaluation of the Degree of Dissociation of A Congruent Compound Fe2Ti across the Bjerrum–Guggenheim Coefficient. Metals, 12(12), 2132. https://doi.org/10.3390/met12122132






