1. Introduction
Prediction of the fatigue life is very important task in the design of the machines [
1] and their attachment [
2]. This paper focuses on fatigue in cold stamped parts [
3,
4] (p. 16). Cold forming has a significant impact on fatigue [
5,
6]. Therefore, fatigue tests were performed and the effects affecting the fatigue life were studied. Methods for the prediction of cold formed parts have also been investigated and designed to easily include the effect of forming in high cycle fatigue.
Cold forming leads to plastic deformations, residual stresses initiation and changes in wall thickness. The spring-back effect [
7,
8] is occurring, when the forming tool is opened. It leads to a change in the shape of the part. All these effects can impacting the fatigue life.
Plastic strain influences the shape of the fatigue curve. This effect is studied in [
9] and the Material Law for Steel Sheets (MLSS) method is formulated. A similar method was published by [
10] called Method of Variable Slopes (MVS). MLSS and MVS adjust the fatigue curve based on the effective plastic strain originating during forming. MLSS and MVS are included in FEMFAT 2022a fatigue software which was used for the final fatigue evaluation. The same software and same methods were used in [
11,
12]. To make them easier to use, MLSS and MVS methods do not distinguish what type of pre-strain condition is present. There are also attempts to consider the type of pre-strain, as in [
13].
Also, residual stress which appear during forming influence the lifetime of such parts. Residual stress relaxes when a part is exposed to a cyclic load. According to [
10], if stress amplitude is higher than yield strength then stress relaxation is nearly complete. Therefore, its impact on fatigue can be weak.
From what has been stated it is obvious that effective plastic strain originating during forming is important for fatigue. It is possible to perform an incremental forming simulation as shown in [
14], but the calculation time can be long. Simulation requires knowledge of the forming process in detail, which is not always available, especially not in reverse engineering. Also, other problems which are discussed later could occur if this approach is used.
If incremental simulation cannot be used for some reason, then a simplified calculation method is a welcome alternative. Facchinetti et al. [
15] proposed a calculation method based on the local curvature of a part. Its purpose is to calculate the plastic strain and residual stress of stamped parts. This approach has some limitations. An example of such a limitation can be the fact that this method takes into account only the bending deformation [
16] and neglects the membrane deformation [
17].
Almost no attention has been paid to inverse stamping [
18,
19,
20,
21] used in conjunction with fatigue life evaluation. But in fact, this method provides more accurate results than those offered by the methods described above. At the same time, inverse stamping maintains simple use and short calculation time. Inverse stamping is more complex, so it provides more realistic results even for geometrically complicated bodies. It needs the final shape of the product and the material properties as inputs, i.e., no models of forming tools are required. This is a great advantage if the details of the forming process are unknown. Also, calculation time compared to incremental forming simulation is significantly shorter [
22]. On the other hand, a longer calculation time than for Facchinetti’s method is expected.
An algorithm of inverse stamping is formulated for shell structures [
23,
24,
25]. If the metal sheet thickness is high compared to the body curvature then it makes more sense to model the calculated body as a volumetric body. Also, if a 3D scan [
26] is used as the input for inverse stamping then it is helpful to use a volumetric model in the calculation. This means that the inverse stamping algorithm needs to be adapted from shell structures to volumetric structures. This adaptation is described below.
This article presents a computational method where the effective plastic deformation occurring during cold forming is determined using adapted inverse stamping. Using the MVS and MLSS methods, the effect of plastic deformation on the fatigue curve is determined at each point of the component. Using the modified fatigue curves, the fatigue life of the entire component is then determined.
4. Discussion
Figure 14,
Figure 15,
Figure 16 and
Figure 17 show the results of the calculations and measurements on a semi-logarithmic graph. The horizontal lines denote the results of the experiments. Each horizontal line includes three markers—the minimum, average and maximum number of cycles which were achieved during the testing of the specimen sets. Markers “x” denote experimental data related to specimens with coating, markers “+” denote experimental data related to specimens without coating.
Especially if loading force amplitude is low, then there is a significant difference in the fatigue results. Voorwald et al. [
37] measured the fatigue of steel samples with various surface layers. The test results from specimens with Zn-Ni coatings were highly dependent on the coating thickness. Specimens with coatings thicker than 15 µm had a shorter lifetime than specimens with thinner coatings or specimens without any coating. Our results are in agreement with this. It can be concluded that the thicker the coating, the greater the decrease in the fatigue life of the samples. From our and Voorwald’s results [
37], it can be seen that at lower load levels, where more cycles are achieved, this difference is much more noticeable. For higher load levels and thus lower number of cycles, the results of fatigue tests on coated and uncoated samples can be considered identical. [
38] describes the ways in which fatigue damage to coated components occurs. The first way of failure is the delamination of the coating and the base material (substrate). The crack formed at the interface between the coating and the substrate gradually spreads until it penetrates the substrate. The second way of failure is the formation of a crack directly in the coating. The crack arises as a result of the lower mechanical resistance of the coating and gradually propagates into the substrate. Our observations show that the second mode of damage dominates the fatigue damage of our samples. This is due to the fact that the mechanical resistance of the coating is lower than the mechanical resistance of the substrate and at the same time the Zn-Ni coating exhibits good adhesion to the substrate, so delamination does not occur. Moreover, coating increases surface roughness, which negatively affects fatigue life too. Coating also increases the surface hardness. A harder surface behaves more brittlely and thus again the fatigue life is reduced.
Results from the calculations are represented by non-horizontal lines. Three calculation methods were compared to find a favourable calculation set-up. The first calculation method (green dashed line) did not consider the effect of plastic strain originating during forming. The second calculation was done using the MLSS method (blue dashed line) and the third one was done using the MVS method (orange dashed line). Markers “x” denote calculations where influence of coating wasn’t considered. Only surface roughness Rz = 8 µm was considered via surface roughness factor according to [
39]. Markers “+” denote calculations where surface roughness Rz = 13 µm was considered via surface roughness factor and where a GSTF of 0.97 was used. The purpose of this is to consider the effect of coating. It can be seen from all the graphs that the inclusion of the effect of effective plastic deformation brings the calculation results closer to the experimental results.
Residual stress arises in the part during forming. The residual stress relaxes if the part is exposed to cyclic loading. Hatscher claims [
10] that residual stress relaxation is almost total if cyclic stress amplitude exceeds yield strength. This condition was fulfilled for all loading levels and for all critical spots in the specimens. Therefore, residual stress was neglected. The same assumption regarding residual stress was made in [
40] where a similar problem was studied.
According to [
10], plastic strain originating during forming should not be higher than the material’s uniform elongation. The same publication states a uniform elongation value of 23.3% for DC04 material. Results of our measurement shown in
Table 2 agree with that. Uniform elongation of the S420MC material is about 13% based on our measurement shown in
Table 2. This condition is not fulfilled for whole bodies, but it is satisfied in spots where rupture was expected. In
Figure 18 and in
Figure 19 these spots are marked ‘spot of rupture’.
Specimens are made from rolled metal sheet. The rolling direction can be seen in
Figure 14 and
Figure 15. From the fatigue point of view and in accordance with [
6], the rolling direction has a low influence and it can be neglected in the fatigue evaluation. From the forming point of view, metal sheet orthotropy has some influence as can be seen in [
41]. Nevertheless, the inverse stamping implementation which was used here only enables the user to consider normal anisotropy.
Figure 14 and
Figure 15 also show the relative direction of the principal plastic strain originating during forming and the principal operational stress direction which is caused by cyclic loading. These directions are perpendicular in the critical spots of specimens A and B. For specimens C and D, these directions are parallel. The MVS and the MLSS adjust the local fatigue S-N curve based on effective plastic strain which is a direction-independent parameter. Massendorf claims in his publication [
6], that the relative direction of principal plastic strain and principal operational stress has a low influence on fatigue-life. At the same time he adds that when the direction of plastic strain and stress is equal, this is more unfavourable from a fatigue point of view. Therefore, his MLSS method is based on this situation in order to obtain more conservative results. Relative direction of principal plastic strain direction and principal operational stress direction was studied in [
5]. Its conclusions are somewhat different and the relative direction between strain and stress is quite significant.
The results based on MLSS for Specimens A and B are somewhat conservative due to the relative direction between principal plastic strain and principal operational stress discussed above. MLSS and MVS ignore relative direction influence and take into account plastic strain intensity only. Our results suggest that relative direction inclusion could lead to accuracy increase. However, including this effect will lead to a more demanding computational procedure. A limitation is also that computational procedures taking this effect into account are not currently included in available software. This is a serious limitation for engineering practice.
All A and B samples broke at the same spot, which is shown in
Figure 20 and in
Figure 21. All calculations predicted breakage at the same spot.
All type C specimens broke in the middle, see
Figure 22. Some type D specimens broken in the middle too—this spot is denoted as a dominant cracking area in
Figure 23. Nevertheless, some type D specimens broke in the minor cracking area or cracks appeared in both locations at once,
Figure 23. Breakage in the minor cracking area occurred only for some specimens without coating and for loading levels D1 and D2. All calculations predicted breakage in the dominant cracking area only. Nevertheless, there is high plastic deformation in the minor cracking area and the theory used is outside the validity range. Samples which broke only in the minor cracking area were removed from the evaluation. Therefore,
Figure 17 includes only two specimens without coating at loading level D1. All experimental results are summarized in
Table A1,
Table A2,
Table A3 and
Table A4.
Experimental data showed some differences between parts with Ni-Zn coating and parts without any coating. The difference is more significant for lower loading force amplitudes. Temperatures reached in electroplating are not high (lower than 90 °C), so an impact on the base material is not expected. Nevertheless, surface roughness and hardness are affected and those effects need to be included in fatigue calculations. A detailed discussion of the effect of the coating was made in
Section 4.
The MLSS provided realistic or conservative results, so it seems suitable for similar applications. A significant safety margin was observed in A type samples. The difference could be a result of some simplifications. The influence of rolling direction or the difference between principal plastic strain direction and the principal operational stress direction seem to be more significant than reported in the literature about MLSS and MVS methods.
Author Contributions
Conceptualization, J.K.; Data curation, J.K.; Formal analysis, J.K.; Investigation, J.K., P.B. and V.L.; Project administration, P.B. and V.L.; Software, J.K.; Supervision, P.B. and V.L.; Validation, J.K.; Visualization, J.K.; Writing—original draft, J.K.; Writing—review & editing, P.B. All authors have read and agreed to the published version of the manuscript.
Funding
This paper was funded by the project “Comprehensive support for designing technical equipment” no. SGS-2022-009.
Data Availability Statement
The data presented in this study are available on request from the corresponding author. The data are not publicly available.
Acknowledgments
This paper is based on the cooperation with MUBEA, spol. s.r.o. using the equipment and material for preparation of all specimens.
Conflicts of Interest
The authors declare no conflict of interest.
References
- He, L.; Wang, Z.; Akebono, H.; Sugeta, A. Machine learning-based predictions of fatigue life and fatigue limit for steels. J. Mater. Sci. Technol. 2021, 90, 9–19. [Google Scholar] [CrossRef]
- Lašová, V.; Bernardin, P.; Švagr, M.; Kubíček, J. Alternative solution of a milling head housing using the Computer Aided Systems. MM Sci. J. 2021, 2021, 4304–4309. [Google Scholar] [CrossRef]
- Lalvani, H.; Mandal, P. Cold forming of Al-5251 and al-6082 tailored welded blanks manufactured by laser and electron beam welding. J. Manuf. Process. 2021, 68, 1615–1636. [Google Scholar] [CrossRef]
- Hu, P.; Ma, N.; Liu, L.-Z.; Zhu, Y.-G. Theories, Methods and Numerical Technology of Sheet Metal Cold and Hot Forming: Analysis, Simulation and Engineering Applications; Springer Science & Business Media: London, UK, 2012; p. 16. [Google Scholar]
- Bimal, D.; Akhilendra, S.; Kanwer, S.A.; Mahadev, S.; Surajit, K.P. Influence of pre-straining path on high cycle fatigue performance of DP 600 steel. Int. J. Fatigue 2019, 126, 369–380. [Google Scholar] [CrossRef]
- Souto, C.D.S.; Gomes, V.M.G.; Figueiredo, M.; Correia, J.A.F.O.; Lesiuk, G.; Fernandes, A.A.; De Jesus, A.M.P. Fatigue behaviour of thin-walled cold roll-formed steel sections. Int. J. Fatigue 2021, 149, 106299. [Google Scholar] [CrossRef]
- Meng, Q.; Zhai, R.; Zhang, Y.; Fu, P.; Zhao, J. Analysis of springback for multiple bending considering nonlinear unloading-reloading behavior, stress inheritance and Bauschinger effect. J. Mater. Process. Technol. 2022, 307, 117657. [Google Scholar] [CrossRef]
- Solfronk, P.; Sobotka, J.; Koreček, D. Effect of the computational model and mesh strategy on the springback prediction of the sandwich material. Machines 2022, 10, 114. [Google Scholar] [CrossRef]
- Masendorf, R. Einfluss der Umformung auf die zyklischen Werkstoffkennwerte von Feinblech; Papierflieger: Clausthal, Germany, 2000; ISBN 3-89720-413-4. [Google Scholar]
- Hatscher, A. Abschätzung der zyklischen Kennwerte von Stählen; Papierflieger: Clausthal, Germany, 2004; ISBN 3-89720-719-2. [Google Scholar]
- Dannbqauer, H.; Gaier, C. Integrating the Results from Process Simulation into Fatigue Life Prediction; Technical Report; SAE Technical Paper: Warrendale, PE, USA, 2007. [Google Scholar]
- Gaier, C.; Kose, K.; Hebisch, H.; Pramhas, G. Coupling Forming Simulation and Fatigue Life Prediction of Vehicle Components; MAGNASTEYR, Engineering Center Steyr: Valentin, Austria, 2004; pp. 65–68. [Google Scholar]
- Le, Q.; Kang, H.; Kridli, G.; Khosrovaneh, A.; Yan, B. Modified strain-life equation to consider the effect of different prestrain paths for dual phase sheet steel. J. Mater. Process. Technol. 2009, 209, 3525–3531. [Google Scholar] [CrossRef]
- Merten, M.; Liebold, K.; Haufe, A. Robustness analysis with LS-OPT® and LS-DYNA® for sheet metal forming simulations. IOP Conf. Ser. Mater. Sci. Eng. 2021, 1157, 012088. [Google Scholar] [CrossRef]
- Facchinetti, M.L.; Weber, B.; Doudard, C.; Calloch, S. Coupling of forming process and fatigue design computations: A local approach. Adv. Eng. Mater. 2009, 11, 736–741. [Google Scholar] [CrossRef]
- To, C.W.S.; Liu, M.L. Hybrid strain based three-node flat triangular shell elements. Finite Elem. Anal. Des. 1994, 17, 169–203. [Google Scholar] [CrossRef]
- Farahani, M.K.; Shirin, M.B.; Assempour, A. Development of an inverse finite element method with an initial guess of linear unfolding. Finite Elem. Anal. Des. 2014, 79, 1–8. [Google Scholar] [CrossRef]
- Yu, M.; Du, R. Sensitivity Analysis of the Sheet Metal Stamping Processes Based on Inverse Finite Element Modeling and Monte Carlo Simulation. AIP Conf. Proc. 2005, 778, 813–818. [Google Scholar] [CrossRef]
- Guo, Y.Q.; Batoz, J.L.; Naceur, H.; Bouabdallah, S.; Mercier, F.; Barlet, O. Recent developments on the analysis and optimum design of sheet metal forming parts using a simplified inverse approach. Comput. Struct. 2000, 78, 133–148. [Google Scholar] [CrossRef]
- Kašpar, J.; Švagr, M.; Bernardin, P.; Lašová, V.; Šedivý, O. Dimension reduction using the inverse stamping method. MM Sci. J. 2021, 4, 4810–4817. [Google Scholar] [CrossRef]
- Lan, J.; Dong, X.; Li, Z. Inverse finite element approach and its application in sheet metal forming. J. Mater. Process. Technol. 2005, 170, 624–631. [Google Scholar] [CrossRef]
- Muhammad, A.A.; Qattawi, A. numerical simulation of sheet metal forming: A review. Int. J. Adv. Manuf. Technol. 2017, 89, 1235–1250. [Google Scholar] [CrossRef]
- Lee, C.H.; Huh, H. Blank Design and Strain Prediction of Automobile Stamping Parts by an Inverse Finite Element Approach. J. Mater. Process. Technol. 1997, 63, 645–650. [Google Scholar] [CrossRef]
- Shamloofard, M.; Assempour, A. Development of an inverse isogeometric methodology and its application in sheet metal forming process. Appl. Math. Model. 2019, 73, 266–284. [Google Scholar] [CrossRef]
- Shamloofard, M.; Assempour, A. Simulation of sheet metal forming processes by presenting a bending-dependent inverse isogeometric methodology. Int. J. Adv. Manuf. Technol. 2021, 112, 1389–1408. [Google Scholar] [CrossRef]
- Tóth, T.; Živčák, J. A Comparison of the Output of 3D Scanners. Procedia Eng. 2014, 69, 393–401. [Google Scholar] [CrossRef]
- Krawczyk, J.; Bembenek, M.; Frocisz, Ł.; Śleboda, T.; Paćko, M. The Effect of Sandblasting on Properties and Structures of the DC03/1.0347, DC04/1.0338, DC05/1.0312, and DD14/1.0389 Steels for Deep Drawing. Materials 2021, 14, 3540. [Google Scholar] [CrossRef]
- Fratesi, R.; Roventi, G. Corrosion resistance of Zn-Ni alloy coatings in industrial production. Surf. Coat. Technol. 1996, 82, 158–164. [Google Scholar] [CrossRef]
- Azizi, R.; Assempour, A. Applications of linear inverse finite element method in prediction of the optimum blank in sheet metal forming. Mater. Des. 2008, 29, 1965–1972. [Google Scholar] [CrossRef]
- Kašpar, J.; Bernardin, P.; Lašová, V. Increasing the robustness of an inverse stamping algorithm. MM Sci. J. 2022, 5684–5688. [Google Scholar] [CrossRef]
- Kašpar, J. Výzkum Simulačních Technik Určených k Navrhování za Studena Lisovaných Komponentů s Ohledem na Jejich Únavovou Životnost. Ph.D. Theses, University of West Bohemia, Pilsen, Czech Republic, 2023. [Google Scholar]
- Růžička, M.; Hanke, M.; Rost, M. Dynamická pevnost a životnost; Ediční středisko ČVUT: Prague, Czech Republic, 1987; pp. 16–17. [Google Scholar]
- Hu, J.; Marciniak, Z.; Duncan, J. Mechanics of Sheet Metal Forming; Elsevier Science: Boston, MA, USA, 2002; p. 9. [Google Scholar]
- Ghennai, W.; Boussaid, O.; Bendjama, H.; Haddag, B.; Nouari, M. Experimental and numerical study of DC04 sheet metal behaviour—Plastic anisotropy identification and application to deep drawing. Int. J. Adv. Manuf. Technol. 2019, 100, 361–371. [Google Scholar] [CrossRef]
- Kašpar, J.; Bernardin, P.; Lašová, V. The Fague of Highly Formed Bodies. MM Sci. J. 2023, 2023, 6616–6623. [Google Scholar] [CrossRef]
- Klemenc, J. Influence of fatigue–life data modelling on the estimated reliability of a structure subjected to a constant-amplitude loading. Reliab. Eng. Syst. Saf. 2015, 142, 238–247. [Google Scholar] [CrossRef]
- Voorwald, H.J.C.; Miguel, I.M.; Peres, M.P.; Cioffi, M.O.H. Effect of Electroplated Zinc-Nickel Alloy Coatings on the Fatigue Strength of AlSl 4340 High-Strength Steel. J. Mater. Eng. Perform. 2005, 14, 249–257. [Google Scholar] [CrossRef]
- Nguyen, V.P.; Dang, T.N.; Le, C.C.; Wang, D.-A. Effect of Coating Thickness on Fatigue Behavior of AISI 1045 Steel with HVOF Thermal Spray and Hard Chrome Electroplating. J. Therm. Spray Technol. 2020, 29, 1968–1981. [Google Scholar] [CrossRef]
- Forschungskuratorium Maschinenbau (FKM). Rechnerischer Festigkeitsnachweis für Maschinenbauteile, 5th extended ed.; VDMA Verlag: Frankfurt am Main, Germany, 2003; p. 5. [Google Scholar]
- Meng, J.; Zhu, P.; Liu, Z.; Ji, Q. Integration of multi-step stamping effects in the bending fatigue analysis of a steel wheel. Fatigue Fract. Eng. Mater. Struct. 2013, 36, 795–808. [Google Scholar] [CrossRef]
- Jakubec, M. Anisotropy Influence on Sheet Metal Part Fatigue Life. Master’s Theses, Czech Technical University in Prague, Faculty of Mechanical Engineering, Prague, Czech Republic, 2022. [Google Scholar]
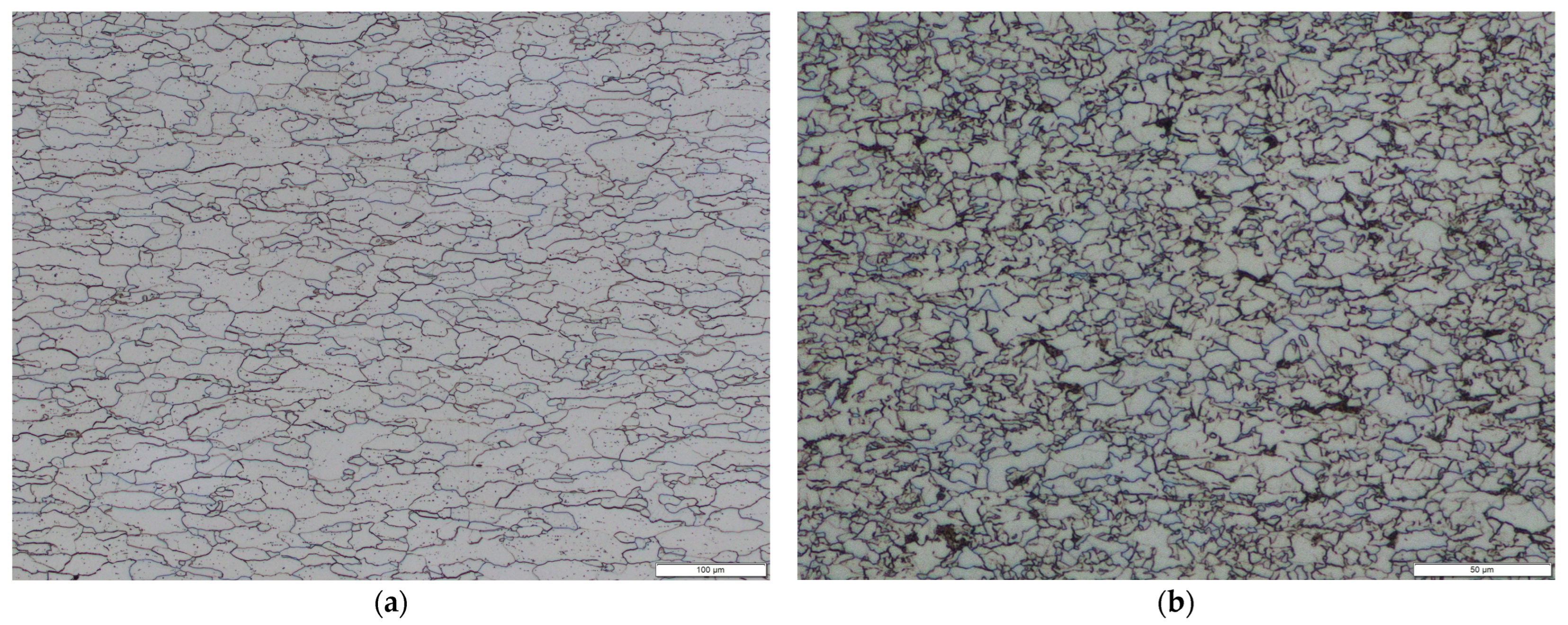
Figure 1.
Microstructure of DC04 (a) and microstructure of S420MC (b).
Figure 2.
Stress-strain curves of DC04 (a) and stress-strain curves of S420MC (b).
Figure 3.
Thickness of coating on DC04 specimen (a) and S420MC specimen (b).
Figure 4.
Cross-section through a volumetric mesh.
Figure 5.
Surface mesh offset. Taken from [
31].
Figure 6.
(a) Cross-section through volumetric mesh (b) Local thickness calculation.
Figure 7.
Protrusion showing non-zero plastic strain (arrows).
Figure 8.
Expected shape and 3D scan.
Figure 9.
Dimensions of specimens A and B.
Figure 10.
Dimensions of specimens C and D.
Figure 11.
Workflow diagram.
Figure 12.
Three point bending test assembly.
Figure 13.
Specimen D in testing machine. Taken from [
31].
Figure 14.
Comparison of experimental and calculated results, specimen A.
Figure 15.
Comparison of experimental and calculated results, specimen B.
Figure 16.
Comparison of experimental and calculated results, specimen C. Taken from [
31].
Figure 17.
Comparison of experimental and calculated results, specimen D. Taken from [
31].
Figure 18.
Rolling direction, principal plastic strain direction and operational stress direction of specimens A and B.
Figure 19.
Rolling direction, principal plastic strain direction and operational stress direction of specimens C and D.
Figure 20.
Fatigue crack of specimen A where coating was applied.
Figure 21.
Fatigue crack of specimen B without coating.
Figure 22.
Fatigue crack of specimen C with coating.
Figure 23.
Fatigue crack of specimen D where coating was not applied.
Table 1.
Chemical composition of used materials.
| Mat. | Chemical Composition [%] |
|---|
| C | Mn | Si | P | S | Al | Nb | Ti | V | Si | Cr | Cu |
|---|
| S420MC | 0.082 | 1.42 | 0.012 | 0.014 | 0.001 | 0.033 | 0.032 | 0.001 | 0.001 | - | - | - |
| DC04 | 0.071 ± 0.026 | 0.24 ± 0.02 | 0.06 | 0.012 ± 0.001 | 0.007 | 0.076 | - | - | - | 0.06 | 0.02 | 0.04 |
Table 2.
Directional dependent material properties.
| Mat. | Direction
[°] | Avg. Rp0.2 [MPa] | Avg. Rm
[MPa] | Avg. Ag
[%] | Avg. r
[-] |
|---|
| DC04 | 0 | 173.0 | 289.1 | 26.1 | 1.699 |
| 45 | 187.6 | 301.0 | 23.2 | 1.196 |
| 90 | 191.6 | 289.6 | 24.2 | 1.794 |
| S420MC | 0 | 464.6 | 539.5 | 13.6 | 0.672 |
| 45 | 469.3 | 528.3 | 13.2 | 1.173 |
| 90 | 490.6 | 554.3 | 12.5 | 0.769 |
Table 3.
Vickers microhardness over depth.
| Spec. Type | Coating | Depth [µm] |
|---|
| 10 | 20 | 30 | 40 | 50 | 60 | 70 | 80 | 90 | 100 |
|---|
| A | No | 128 | 131 | 124 | 130 | 126 | 122 | 130 | 131 | 126 | 124 |
| A | Yes | 508 | 162 | 112 | 134 | 133 | 131 | 88 | 130 | 125 | 132 |
| B | No | 151 | 183 | 180 | 174 | 180 | 191 | 197 | 196 | 182 | 177 |
| B | Yes | 318 | 186 | 189 | 203 | 194 | 193 | 188 | 180 | 177 | 184 |
Table 4.
Material properties used in inverse stamping.
| Mat. | κ [MPa] | n [-] | a [-] |
|---|
| DC04 | 467.1 | 0.1675 | 1.4693 |
| S420MC | 783.4 | 0.1065 | 0.9468 |
Table 5.
Dimensions of specimens A and B.
| Spec. Type | l1 [mm] | l2 [mm] | l3 [mm] | l4 [mm] | l5 [mm] | l6 [mm] | l7 [mm] | r1 [mm] | r2 [mm] |
|---|
| A | 190 | 34 | 6 | 1.14 | 3.92 | 27.02 | 2 | 2.8 | 5.11 |
| B | 380 | 68 | 12 | 2.28 | 7.84 | 54.04 | 4 | 5.6 | 10.22 |
Table 6.
Dimensions of specimens C and D.
| Spec. Type | l8 [mm] | l9 [mm] | l10 [mm] | l11 [mm] | r3 [mm] | r4 [mm] | α [°] |
|---|
| C | 61.5 | 19 | 7.5 | 2 | 1.5 | 15 | 4.5 |
| D | 123 | 38 | 15 | 4 | 3 | 30 | 3.1 |
Table 7.
Loading levels and loading forces.
| Spec. Type | Loading Level | Min. Force [N] | Max. Force [N] | Mean Force [N] | Force Ampl. [N] |
|---|
| A | A1 | −956 | −64 | −510 | 446 |
| A2 | −984 | −66 | −525 | 459 |
| A3 | −1050 | −70 | −560 | 490 |
| B | B1 | −4500 | −300 | −2400 | 2100 |
| B2 | −4781 | −319 | −2550 | 2231 |
| B3 | −5500 | −367 | −2933.5 | 2566.5 |
| C | C1 | −170 | +170 | 0 | 170 |
| C2 | −185 | +185 | 0 | 185 |
| C3 | −200 | +200 | 0 | 200 |
| D | D1 | −1000 | +1000 | 0 | 1000 |
| D2 | −1100 | +1100 | 0 | 1100 |
| D3 | −1320 | +1320 | 0 | 1320 |
| Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).