New Analytical Model for Determining the Roll Pitch Diameter in Three-Roll Continuous Retained Mandrel Rolling
Abstract
:1. Introduction
2. Modelling Work
2.1. Tube Profile and Tube Stress Data Needed for Further Calculations
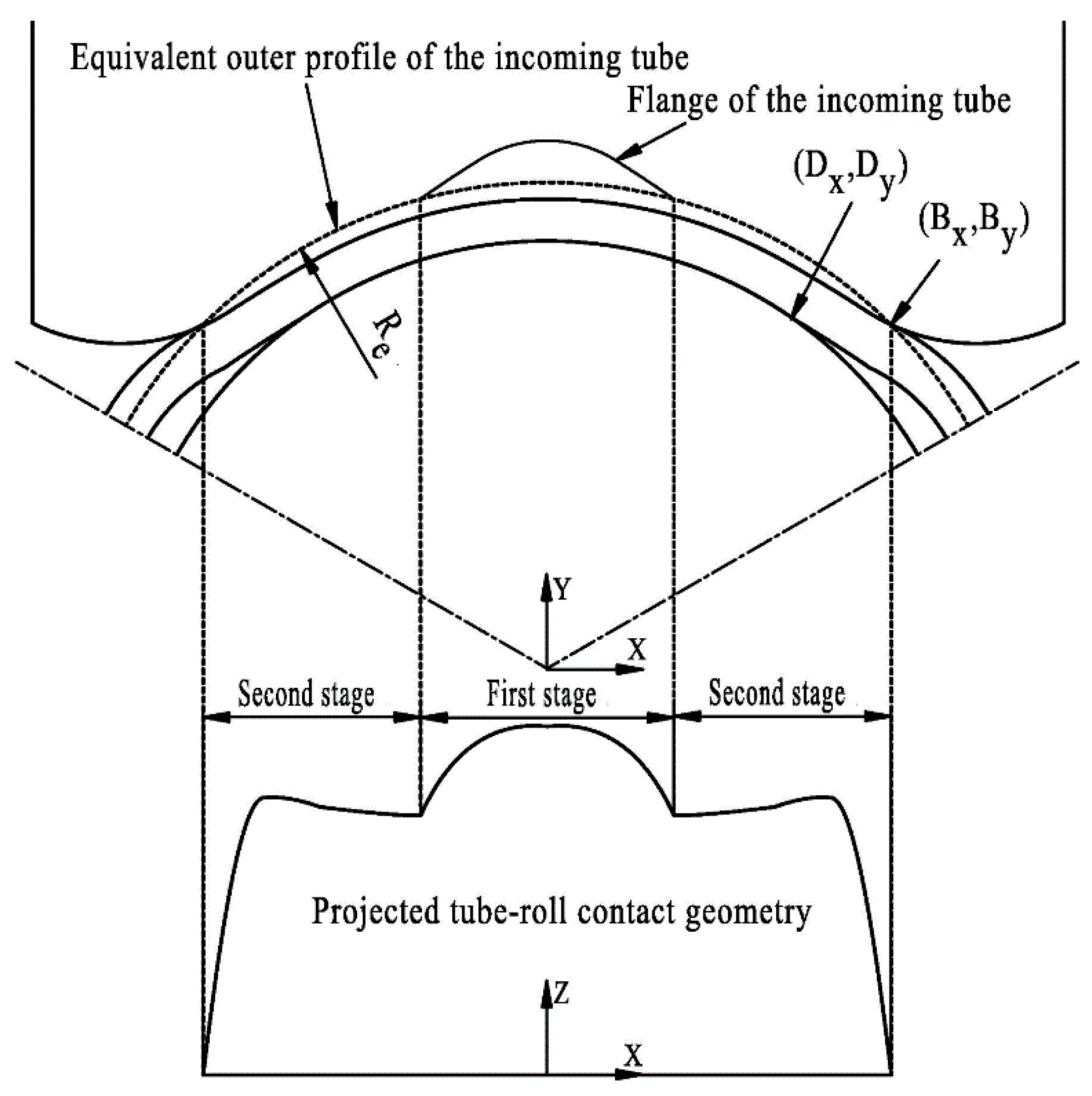
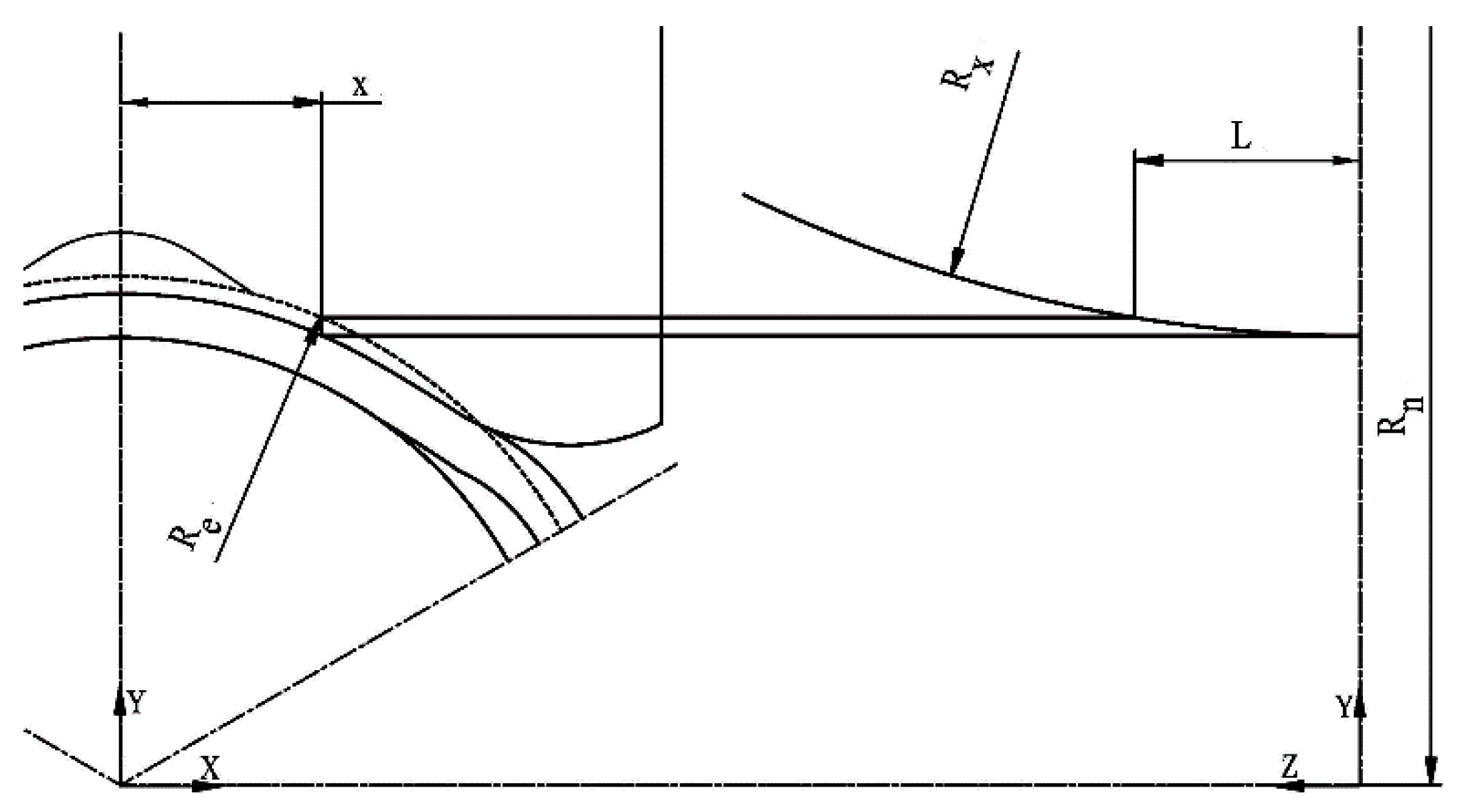
2.2. Modelling for Tube-Roll Contact Geometry
2.3. Equation for Roll Separating Force
2.4. Fundamental Equations for the Roll Pitch Diameter
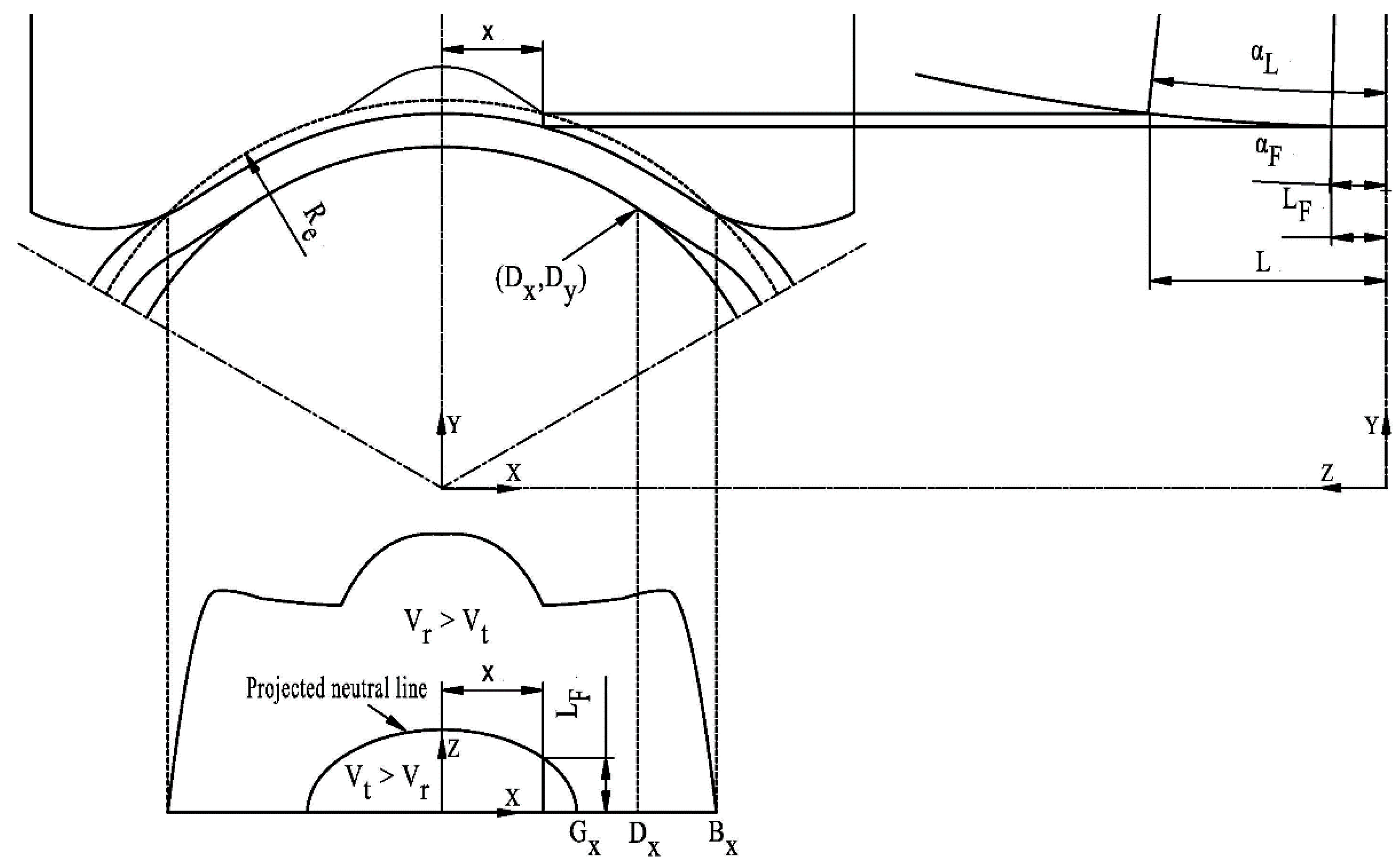
2.4.1. Mechanical Relationship on One Unit Width of the Contact Arc
2.4.2. Determination of the Neutral Angle on A Given Contact Arc
2.4.3. Equations for the Force–Equilibrium in the Rolling Direction
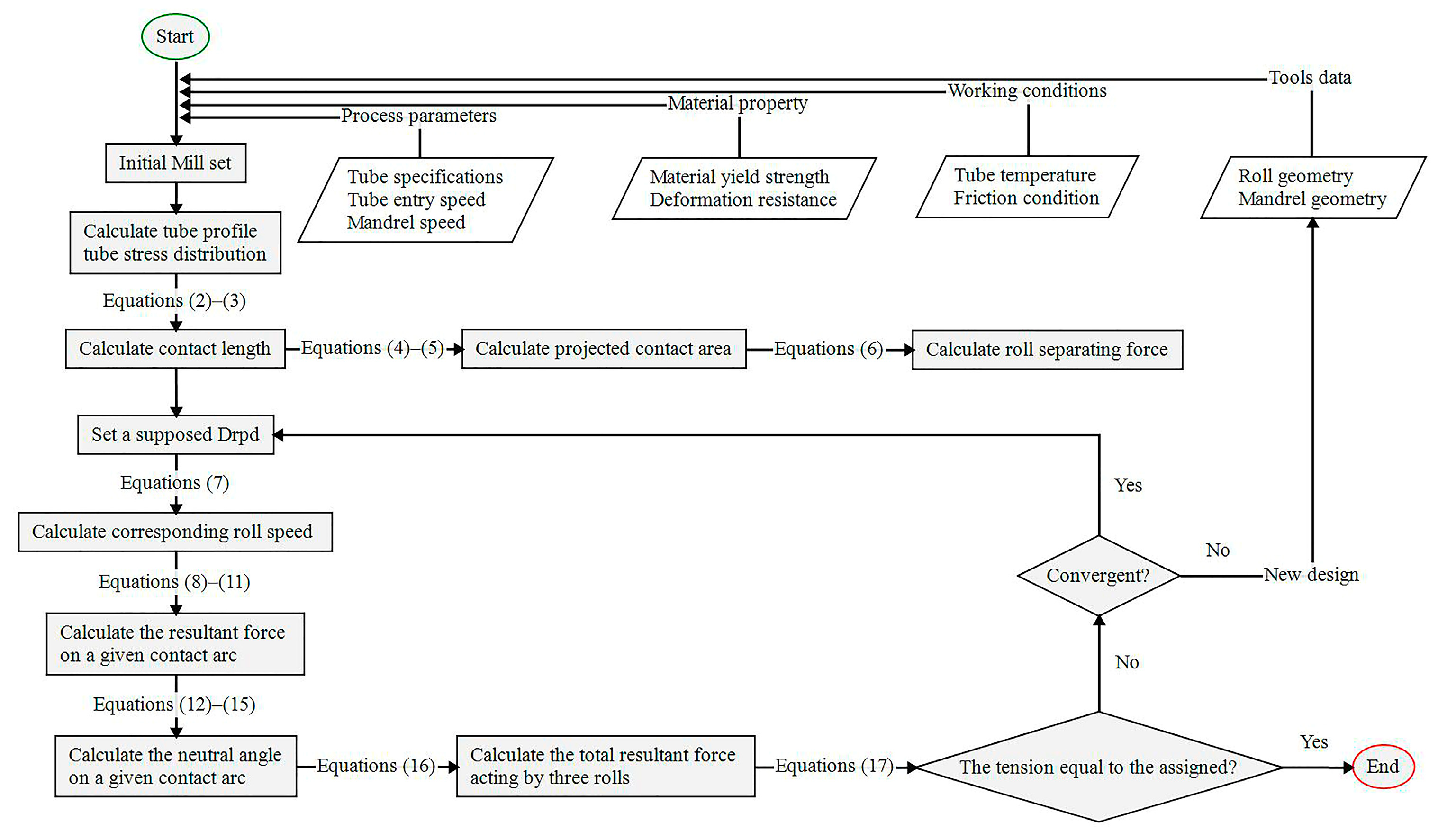
2.5. Implementation of the Model
3. Validation and Discussion
3.1. Validation of the Tube-Roll Contact Geometry Model
3.2. Validation of the Roll Pitch Diameter Model in Field Operation
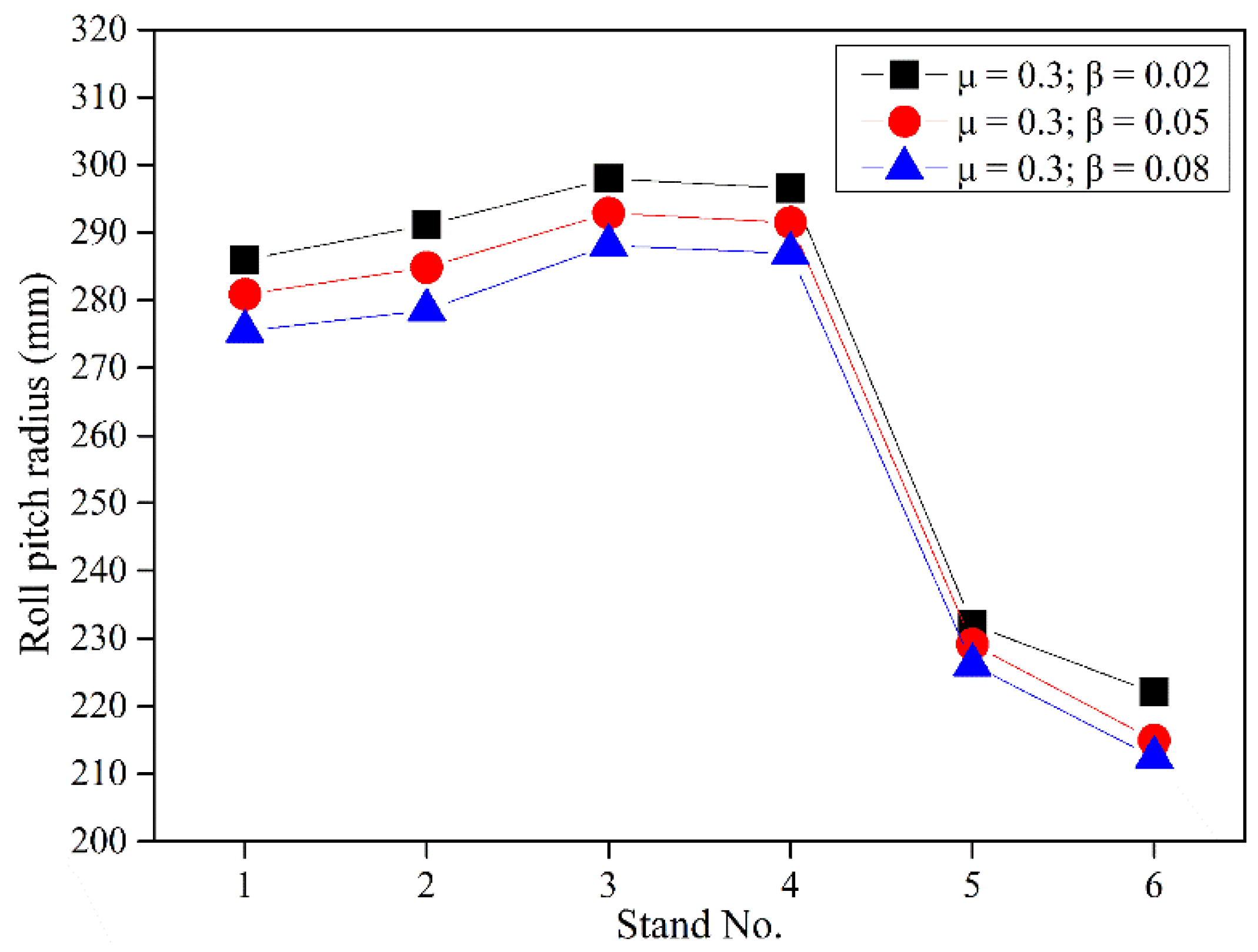
3.3. Influence of Various Friction Coefficients on the Calculated Results of the Roll Pitch Diameter
3.4. Comparison of the Proposed Model and the Conventional Empirical Model
4. Conclusions
- The tube-roll contact geometry in three-roll continuous retained mandrel rolling has been modelled from 3D analytic geometry principles. The model can be used to calculate the contact data necessary for the further analytical calculations of roll pitch diameter under limited conditions. According to the experimental results, the maximum deviation of the calculated total projected contact area is less than 6%;
- The analytical model for determining the roll pitch diameter has been established from force–equilibrium principles. The formulation of the fundamental equations has taken into account the tube-roll contact geometry, roll pressure, inter-stand tensions, mandrel pull forces, and frictional conditions. The roll setting data calculated by using the model have been examined through actual rolling at the plant. With the maximum deviation of the calculated data from the satisfactory data in field operation being less than 3.9%, it can be concluded that the validity of the proposed model has been experimentally verified;
- In applying the proposed model to the actual hot steel tube rolling, the appropriate values of the friction coefficient should be substituted in the calculations. The maximum changing amplitude of the theoretical roll pitch diameter corresponding to the commonly used data range of the friction coefficients can be above 9%;
- Having overcome the shortcomings of the conventional empirical model, the proposed model has the required prediction accuracy and flexibility to be used in flexible tube rolling;
- By building the key algorithms around physical models, this modelling has not only provided a sound theoretical basis for developments in rolling control technology but has also advanced our scientific understanding of the mechanics of continuous retained mandrel rolling process.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Ai | tube cross-sectional area at the i-th stand outlet; |
| projected contact area corresponding to direct deformation zone; | |
| projected contact area corresponding to intermediate deformation zone; | |
| Bx | x-coordinate of the detaching point on the roll; |
| d | actual size of roll pass; |
| Dn | roll nominal diameter; |
| Drpd | roll pitch diameter; |
| Dx | x-coordinate of the detaching point on the mandrel; |
| erpd | pitch coefficient of roll pitch diameter; |
| f | resultant force on a given contact arc in the rolling direction; |
| F | roll separating force; |
| F1 | half of the resultant force acting by the roll in the direct deformation zone where Vt > Vr; |
| F2 | half of the resultant force acting by the roll in the direct deformation zone where Vt < Vr; |
| F3 | half of the resultant force acting by the roll in the intermediate deformation zone where Vt > Vr; |
| Fmpi | mandrel pull force in the roll-bite; |
| Fmpo | mandrel pull force at the stand outlet; |
| Fti | tension force at the stand inlet; |
| Fto | tension force at the stand outlet; |
| Ftotal | total resultant force acting by three rolls in rolling direction; |
| Gx | x-coordinate of the point where Vt = Vr at the outlet plane; |
| i | number of roll pass; |
| L | projected contact length; |
| Pz | roll pressure on the contact arc; |
| Re | equivalent cylinder radius of incoming tube; |
| Rm | mandrel radius; |
| Rn | roll nominal radius; |
| Rr | roll groove arc radius; |
| Rt | tube flange profile radius; |
| Rx | roll radius at the given x-coordinate; |
| Xr | x-coordinate of groove arc center; |
| Yr | y-coordinate of groove arc center; |
| Xt | x-coordinate of tube profile center; |
| Yt | y-coordinate of tube profile center; |
| Vi | outgoing tube speed at the i-th pass; |
| Vr | separating speed of roll peripheral speed; |
| Vt | tube speed in the rolling direction; |
| Vrpm | roll rotational speed. |
| Greek letters | |
| αF | nip angle of the contact arc where the metal forward slip exists; |
| αL | nip angle of the total contact arc; |
| αz | nip angle at a given point on the contact arc; |
| δ | arc length of the tube profile corresponding to one unit width in the x-axis direction; |
| mean outer radial stress in direct deformation zone; | |
| mean outer radial stress in intermediate deformation zone. | |
References
- Lee, Y.; Kim, Y.H. Approximate analysis of roll force in a round-oval-round pass rolling sequence. J. Mater. Process. Technol. 2001, 113, 124–130. [Google Scholar] [CrossRef]
- Vacance, M.; Massoni, E.; Chenot, J.L.; Rovelli; Cumino; Lubrano. Multi stand pipe mill finite element model. J. Mater. Process. Technol. 1990, 24, 421–430. [Google Scholar] [CrossRef]
- Yamada, K.; Ogawa, S.; Hamauzu, S.; Kikuma, T. Three-dimensional analysis of mandrel rolling by rigid-plastic finite element method. Sanjigen Gososei Yugen Yosoho Ni Yoru Mandrel Atsuen No Kaiseki. Shinnettetsu Giho 1991, 342, 9–13. [Google Scholar]
- Cavaliere, M.A.; Goldschmit, M.B.; Dvorkin, E.N. Finite element analysis of steel rolling processes. Comput. Struct. 2001, 79, 2075–2089. [Google Scholar] [CrossRef]
- Sola, G.; Vacance, M.; Massoni, E.; Chenot, J.L. Thermomechanical simulation of seamless tube rolling using a 3D finite element method. J. Mater. Process. Technol. 1994, 45, 187–192. [Google Scholar] [CrossRef]
- Li, S.Z.; Zhang, Z.C.; Bao, H.Y.; Zhou, Z.Y. Influence of roll speed schedule on transverse wall thickness evenness of shell elongated by mandrel mill. Mater. Sci. Forum 2010, 654, 1311–1314. [Google Scholar] [CrossRef]
- Yamamoto, S.; Yamada, K.; Hamauzu, S.; Masuda, I.; Ozak, T. Effect of roll-groove profile on tube deformation in single-stand mandrel rolling. J. Jpn. Soc. Technol. Plast. 1999, 40, 1076–1080. [Google Scholar]
- Li, S.Z.; Jie, X.; Yin, Y.D.; Xue, J.G.; Yuan, F. Mechanism of internal surface crack formation of seamless modified 9Cr-1Mo steel tube rolled by mandrel mill and its application. J. Iron Steel Res. Int. 2007, 14, 273–276. [Google Scholar] [CrossRef]
- Toporov, V.A.; Ibragimov, P.A.; Panasenko, O.A.; Nukhov, D.S.; Khalezov, A.O. Mathematical model for continuous tube rolling and the effect of technological factors on surface defect formation. Russ. Metall. 2020, 2020, 1632–1636. [Google Scholar] [CrossRef]
- Jiang, Y.; Tang, H.; Zhang, X.P. Rotation mechanics and numerical simulation of hot rolling process under asymmetric rolls. Int. J. Mech. Sci. 2019, 151, 785–796. [Google Scholar] [CrossRef]
- Knapinski, M.; Bobarikin, Y.L.; Radkin, Y.I. The rolling tool development in order to improve the geometry of a tube profile and to reduce the wear of mill rolls. New Trends Produc. Eng. 2019, 2, 321–330. [Google Scholar] [CrossRef]
- Okamoto, T.; Hayashi, C. Theory of plasticity on mandrel rolling. In Proceedings of the International Conference on the Science and Technology of Iron and Steel; Iron and Steel Institute of Japan: Tokyo, Japan, 1971; pp. 655–658. [Google Scholar]
- Furugen, M.; Hayashi, C. Application of the theory of plasticity of the cold pilgering of tubes. J. Mater. Process. Technol. 1984, 10, 273–286. [Google Scholar] [CrossRef]
- Wei, Z.H.; Wu, C.J. A new analytical model to predict the profile and stress distribution of tube in three-roll continuous retained mandrel rolling. J. Mater. Process. Technol. 2022, 302, 117491. [Google Scholar] [CrossRef]
- Baines, K. Lead as a model material to simulate mandrel rolling of hot steel tube. J. Mater. Process. Technol. 2001, 118, 422–428. [Google Scholar] [CrossRef]
- Sobkowiak, P. Experimental investigation on the states of strain and stress occurring in the metal during the continuous tube-rolling process. J. Mater. Process. Technol. 1996, 61, 347–353. [Google Scholar] [CrossRef]
- Yan, Z.S. Modern Continuous Hot-rolling Production of Seamless Steel Tubes and Pipes, 1st ed.; Metallurgical Industry Press: Beijing, China, 2009; pp. 117–118. [Google Scholar]
- Lazarus Home Page. Available online: https://www.lazarus-ide.org/index.php (accessed on 23 December 2020).
- Wang, X.M.; Hong, H.P.; Wang, M.; Ma, C.Q.; Cheng, Z.; Liang, P.X. High temperature deformation resistance model and dynamic recrystallization kinetics model of 37Mn5 steel. J. Wuhan Univ. Sci. Technol. 2010, 5, 497–500. [Google Scholar]



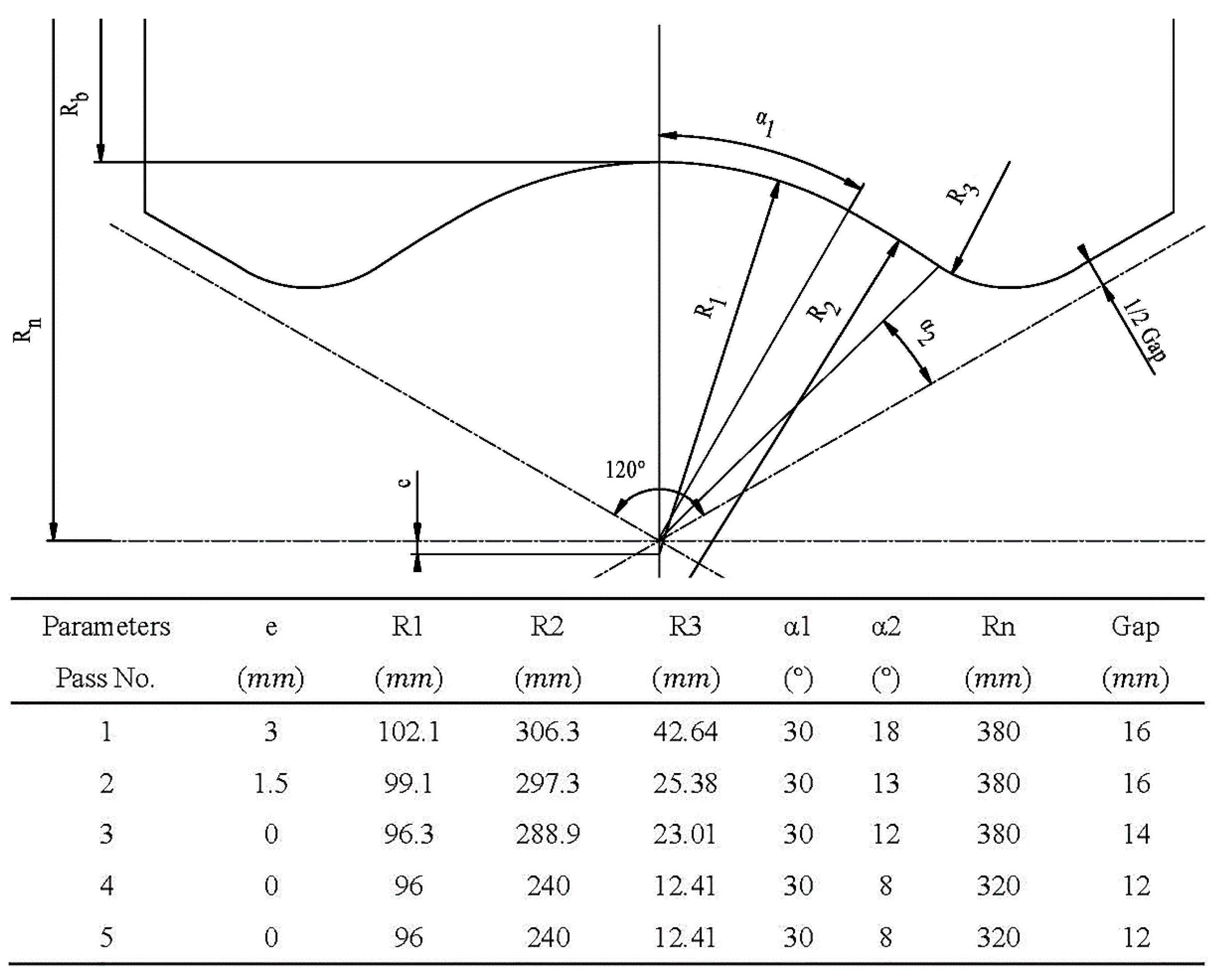





| Pass No. | Pass 1 | Pass 2 | Pass 3 | Pass 4 | Pass 5 |
|---|---|---|---|---|---|
| Predicted (mm2) | 13,478 | 15,145 | 13,028 | 9360 | 6242 |
| Measured (mm2) | 13,537 | 14,956 | 12,917 | 8935 | 5891 |
| Error (%) | −0.44 | 1.26 | 0.86 | 4.75 | 5.95 |
| Tools Data | |||||||
|---|---|---|---|---|---|---|---|
| Parameters | Inlet | Pass 1 | Pass 2 | Pass 3 | Pass 4 | Pass 5 | Pass 6 |
| R1 (mm) | 106.8 | 102.8 | 99.3 | 98.4 | 98 | 98 | |
| R2 (mm) | 320.4 | 308.4 | 297.9 | 270.6 | 245 | 245 | |
| R3 (mm) | 50 | 30 | 27 | 20 | 14 | 14 | |
| e (mm) | 3.3 | 1.65 | 0 | 0 | 0 | 0 | |
| α1 (°) | 60 | 60 | 60 | 60 | 60 | 60 | |
| α2 (°) | 18 | 13 | 12 | 10 | 8 | 8 | |
| Gap (mm) | 16 | 16 | 14 | 14 | 12 | 12 | |
| Rb (mm) | 251.9 | 263.2 | 280 | 274.5 | 211.9 | 202.2 | |
| Size (mm) | 207 | 202.3 | 198.6 | 196.8 | 196 | 196 | |
| Stand spacing (mm) | 870 | 1170 | 870 | 1115 | 760 | ||
| Rm (mm) | 92.45 | 92.45 | 92.45 | 92.45 | 92.45 | 92.45 | |
| Rolling conditions | |||||||
| Inlet | Pass 1 | Pass 2 | Pass 3 | Pass 4 | Pass 5 | Pass 6 | |
| μ | 0.3 | 0.3 | 0.3 | 0.3 | 0.3 | 0.3 | |
| β | 0.05 | 0.05 | 0.05 | 0.05 | 0.05 | 0.05 | |
| TD (°C) | 1103 | 1100.6 | 1099.2 | 1097.3 | 1095.9 | 1094.2 | 1092.9 |
| Fti (kN) | 0 | 0 | 0 | 0 | 0 | 0 | |
| Fto (kN) | 0 | 0 | 0 | 0 | 0 | 0 | |
| Process parameters | |||||||
| Inlet | Pass 1 | Pass 2 | Pass 3 | Pass 4 | Pass 5 | Pass 6 | |
| Gap (mm) | 15.5 | 15.4 | 13.4 | 13.4 | 11.4 | 11.4 | |
| Shell tube O.D. (mm) | 230 | ||||||
| tube W.T. (mm) | 17.5 | 10.7 | 8.3 | 6.4 | 5.5 | 5.1 | 5.1 |
| Tube speed (mm/s) | 1200 | 4500 | |||||
| Mandrel speed (mm/s) | 1040 | 1040 | 1040 | 1040 | 1040 | 1040 | 1040 |
| Calculated data | |||||||
| Inlet | Pass 1 | Pass 2 | Pass 3 | Pass 4 | Pass 5 | Pass 6 | |
| Ai (mm2) | 11,682.8 | 8375.9 | 5945.1 | 4479.8 | 3611.3 | 3211.9 | 3117.5 |
| (MPa) | −187.8 | −197.6 | −188.5 | −203.2 | −184.5 | −106.2 | |
| (MPa) | −3.3 | −2.6 | −2.4 | −2.0 | −1.6 | −0.1 | |
| (mm2) | 11,400 | 10,699 | 9423 | 7056 | 4557 | 3928 | |
| (mm2) | 2309 | 2990 | 2147 | 1676 | 911 | 512 | |
| F (kN) | 2149 | 2123 | 1782 | 1437 | 842 | 417 | |
| Fmpi (kN) | 291.6 | 303.8 | 209.5 | 165.8 | 82.7 | 28.2 | |
| Fmpo (kN) | 148.2 | 172.1 | 99.4 | 120.5 | 82.6 | 79 | |
| Drpd (mm) | 526.6 | 549.1 | 597.8 | 581 | 447.8 | 424.2 | |
| Vrpm (rpm) | 60.69 | 82 | 99.98 | 127.61 | 186.17 | 202.44 | |
| Pass No. | Pass 1 | Pass 2 | Pass 3 | Pass 4 | Pass 5 | Pass 6 |
|---|---|---|---|---|---|---|
| Actual F (kN) | 2002 | 2121 | 1653 | 1601 | 892 | 313 |
| Calculated F (kN) | 2149 | 2123 | 1782 | 1437 | 842 | 417 |
| Error (%) | 7.33 | 0.07 | 7.8 | −10.2 | −6.3 | 33.3 |
| Actual Vrpm (rpm) | 60.67 | 82.53 | 102.26 | 127.96 | 186.47 | 206.48 |
| Calculated Vrpm (rpm) | 60.69 | 82 | 99.98 | 127.61 | 186.17 | 202.44 |
| Error (%) | 0.03 | −0.64 | −2.23 | −0.27 | −0.16 | −1.95 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wei, Z.; Wu, C. New Analytical Model for Determining the Roll Pitch Diameter in Three-Roll Continuous Retained Mandrel Rolling. Metals 2023, 13, 304. https://doi.org/10.3390/met13020304
Wei Z, Wu C. New Analytical Model for Determining the Roll Pitch Diameter in Three-Roll Continuous Retained Mandrel Rolling. Metals. 2023; 13(2):304. https://doi.org/10.3390/met13020304
Chicago/Turabian StyleWei, Zhaohui, and Chunjing Wu. 2023. "New Analytical Model for Determining the Roll Pitch Diameter in Three-Roll Continuous Retained Mandrel Rolling" Metals 13, no. 2: 304. https://doi.org/10.3390/met13020304
APA StyleWei, Z., & Wu, C. (2023). New Analytical Model for Determining the Roll Pitch Diameter in Three-Roll Continuous Retained Mandrel Rolling. Metals, 13(2), 304. https://doi.org/10.3390/met13020304







