Prediction of Fatigue Crack Initiation under Variable Amplitude Loading: Literature Review
Abstract
1. Introduction
2. Methods
3. Overview of Crack Initiation Approach
- First, estimates are made of the stresses and strains at the critical site.
- Second, local stresses and strains are used to compute damage, which is summed up algebraically over time until a critical damage total (failure threshold) is met.
- Third, predicted life is the time in history when the failure criteria were met.
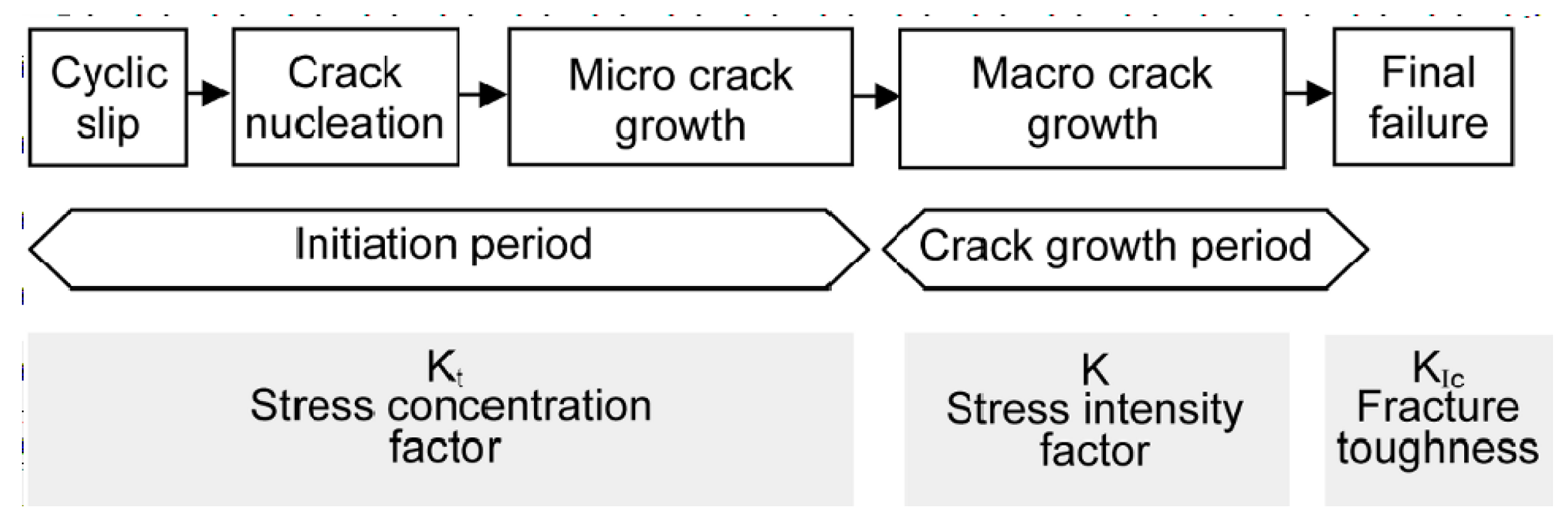
4. Physics of Crack Initiation
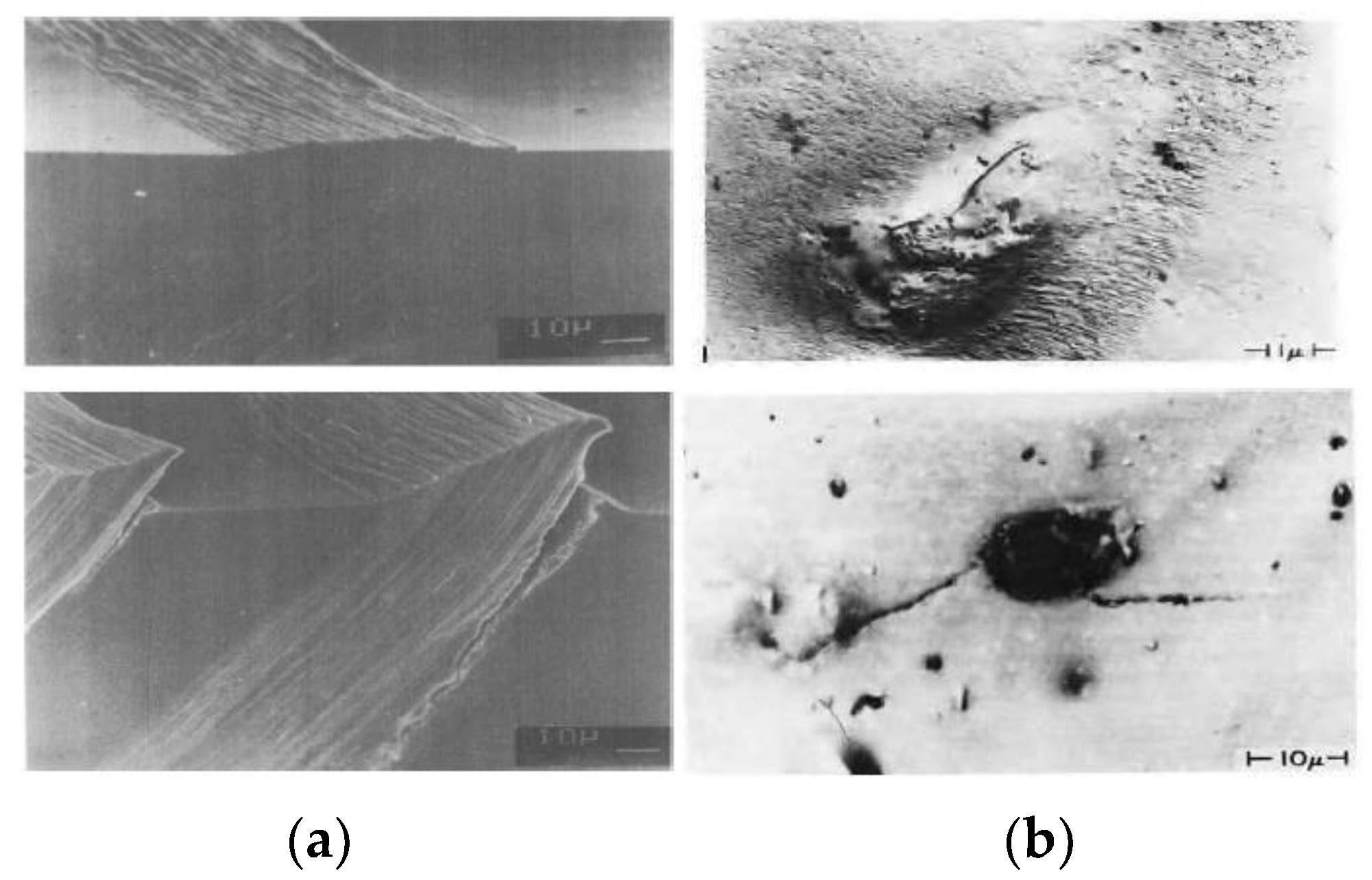
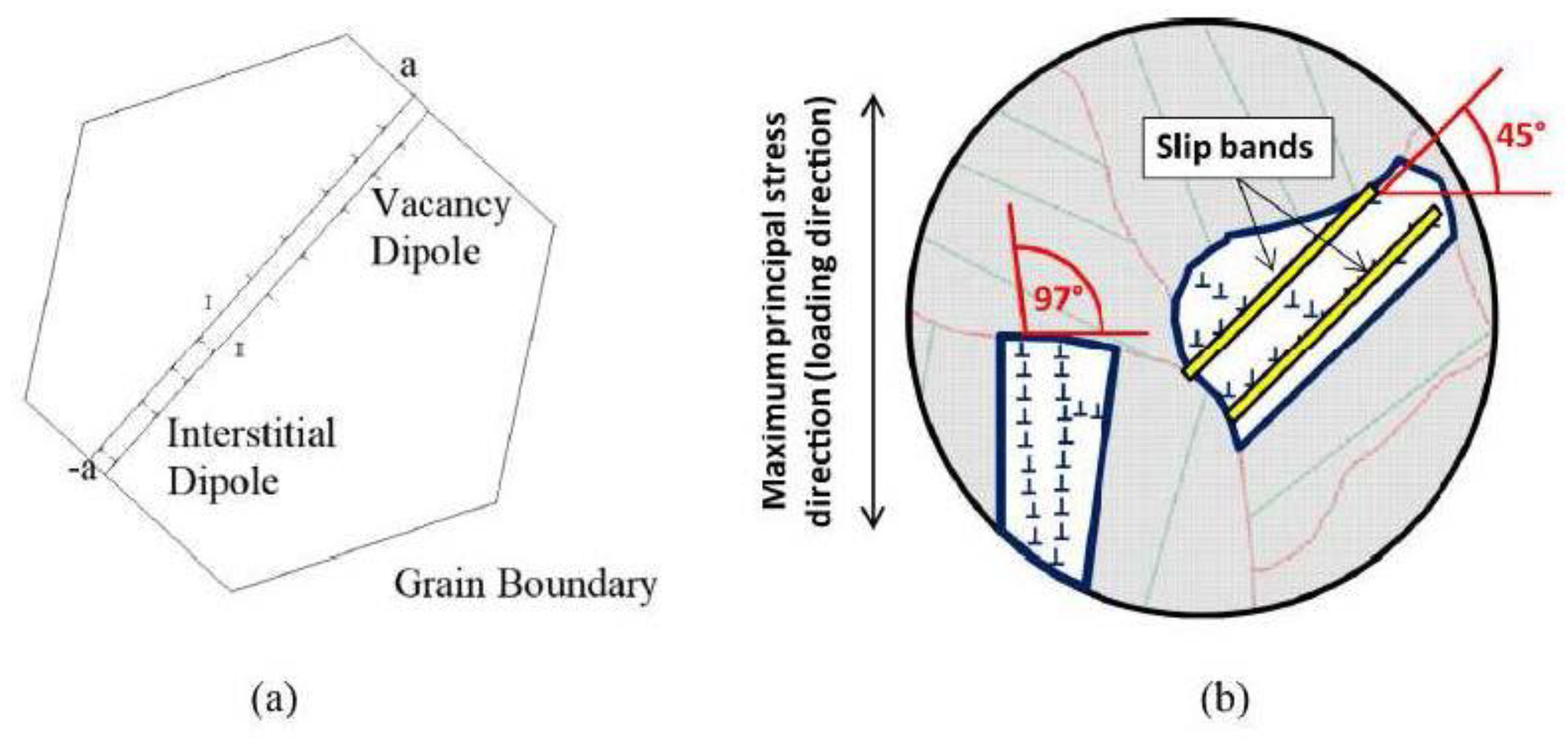
Microstructural Mechanisms of Fatigue Crack Initiation
5. Prediction of Fatigue Crack Initiation
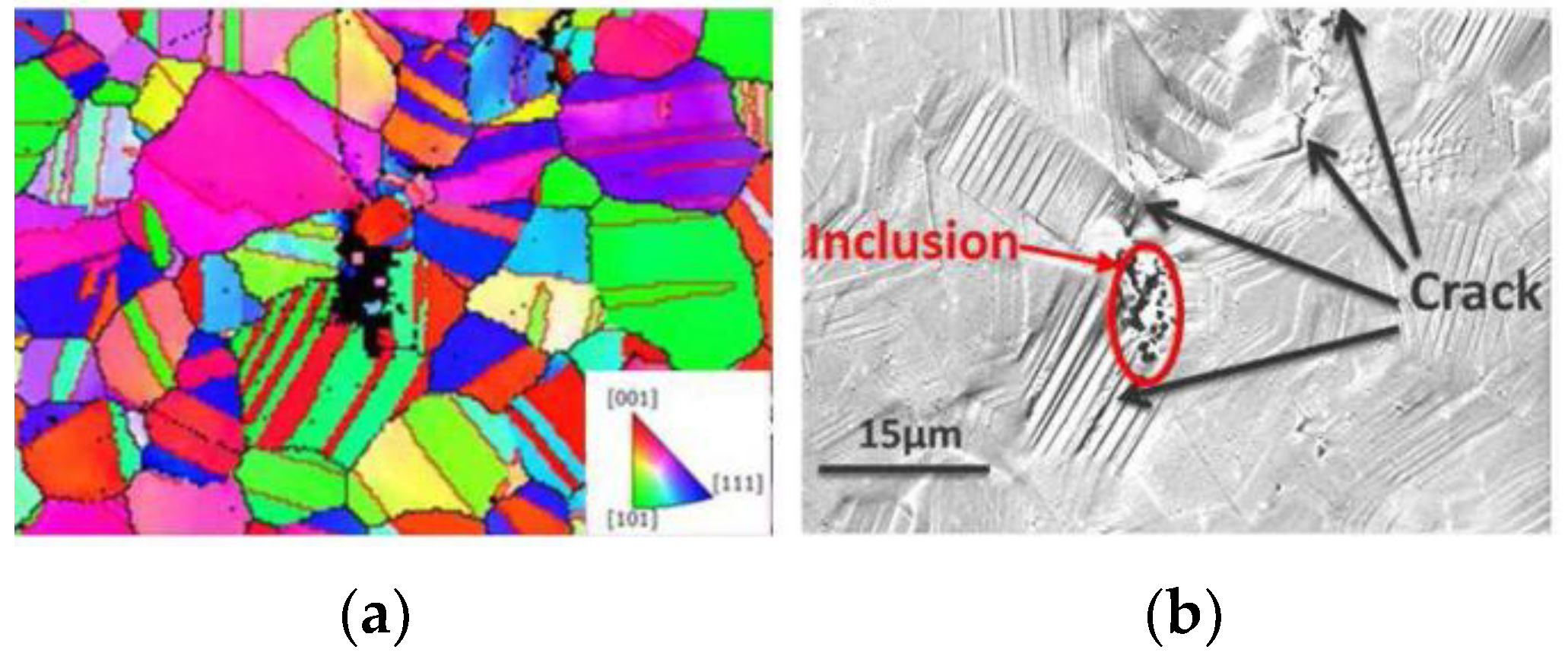
5.1. Modified Tanaka Mura Model
- (i)
- cracks start from inclusions (type A),
- (ii)
- inclusions crack when slip bands hit them (type B), and
- (iii)
- a slip band crack starts from an inclusion that hasn’t cracked (type C).
5.2. Acoustic Second Harmonic Generation
5.3. Probability of Crack Initiation on Defects
6. Methods of Life Prediction Crack Initiation under Variable Amplitude Loading
- Stress-based approach (SN curve approach)
- B.
- Strain based approach for variable amplitude loading
- C.
- Continuum damage mechanics approaches
- Defect-tolerant techniques and
- Total-life approaches.
- It is difficult to establish material parameters and crack growth data for mechanically small cracks through tests.
- The results are sensitive to the initial crack and defect sizes chosen; and.
- It has been difficult to establish approaches that are applicable for engineering calculations.
7. Experimental Methods for Prediction Fatigue Crack Initiation
8. Effects of Temperature on Crack Initiation
9. Conclusions and Outlook
- The fatigue crack initiation areas and external factors, particularly those related to structure geometry, manufacturing processes, and interactions between components when they form a whole mechanical system
- At room temperature, the fatigue cracks formed from the micropores within the fine-grain clusters because the micropores acted as a pre-crack, shortening the crack initiation process.
- Different models for predicting fatigue crack initiation under that include microstructure scale parameters that can be used to predict how fatigue cracks will start under variable amplitude loading have been reviewed. Each model’s scope of applicability varies from case to case and depends on the individual application and the reliability aspects that need to be taken into account.
- A modified version of the energy-based Tanaka-Mura equation for cyclically softening metal was developed and successfully used to predict the influence of strain-range on high temperature low cycle fatigue crack start in cyclically softening steel at various temperatures.
- Material and structure fatigue under variable amplitude Multiaxial loading is still a complicated domain with many factors interacting (loading, mechanical behaviour of the material, geometry of the structure, surface roughness, residual stresses, temperature, environment, and so on).
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Cui, W. A state-of-the-art review on fatigue life prediction methods for metal structures. J. Mar. Sci. Technol. 2002, 7, 43–56. [Google Scholar] [CrossRef]
- Pande, C.S. Fundamentals of Fatigue Crack Initiation and Propagation: Some Thoughts; Srivatsan, T.S., Imam, M.A., Srinivasan, R., Eds.; Springer International Publishing: Cham, Switzerland, 2016; pp. 3–15. [Google Scholar] [CrossRef]
- Bathias, C.; Pineau, A. Fatigue of Materials and Structures: Application to Design and Damage; John Wiley & Sons: Hoboken, NJ, USA, 2013. [Google Scholar]
- Zhu, M.; Liu, X.; Lai, J.; Luo, J. Fatigue life of a pressure vessel based on residual strength and crack size. Trans. Can. Soc. Mech. Eng. 2022, 46, 391–399. [Google Scholar] [CrossRef]
- Benedetti, M.; du Plessis, A.; Ritchie, R.; Dallago, M.; Razavi, S.; Berto, F. Architected cellular materials: A review on their mechanical properties towards fatigue-tolerant design and fabrication. Mater. Sci. Eng. R Rep. 2021, 144, 100606. [Google Scholar] [CrossRef]
- Schijve, J. Fatigue of structures and materials in the 20th century and the state of the art. Int. J. Fatigue 2003, 25, 679–702. [Google Scholar] [CrossRef]
- Zhang, Q.; Zhu, Y.; Gao, X.; Wu, Y.; Hutchinson, C. Training high-strength aluminum alloys to withstand fatigue. Nat. Commun. 2020, 11, 5198. [Google Scholar] [CrossRef]
- Krupp, U. Fatigue Crack Propagation in Metals and Alloys: Microstructural Aspects and Modelling Concepts; John Wiley & Sons: Hoboken, NJ, USA, 2007. [Google Scholar]
- Lin, P.-H.; Tobushi, H.; Hashimoto, T.; Shimeno, Y.; Takata, K. Fatigue Properties of TiNi Shape Memory Alloy. J. Soc. Mater. Sci. Jpn. 2001, 50, 103–110. [Google Scholar] [CrossRef]
- Susmel, L.; Tovo, R. Estimating fatigue damage under variable amplitude multiaxial fatigue loading. Fatigue Fract. Eng. Mater. Struct. 2011, 34, 1053–1077. [Google Scholar] [CrossRef]
- Cui, W.; Wang, F.; Huang, X. A unified fatigue life prediction method for marine structures. Mar. Struct. 2011, 24, 153–181. [Google Scholar] [CrossRef]
- Suresh, S. Fatigue of Materials; Cambridge university press: Cambridge, UK, 1998. [Google Scholar]
- Lassen, T.; Recho, N. Proposal for a more accurate physically based S–N curve for welded steel joints. Int. J. Fatigue 2009, 31, 70–78. [Google Scholar] [CrossRef]
- Deepthi, T.V.; Reddy, C.S.; Satyadevi, A. Recent trends in elastic-plastic analysis using elastic solutions. Mater. Today Proc. 2015, 2, 2188–2197. [Google Scholar] [CrossRef]
- Yao, J.; Kozin, F.; Wen, Y.K.; Yang, J.N.; Schueller, G.; Ditlevsen, O. Stochastic fatigue, fracture and damage analysis. Struct. Saf. 1986, 3, 231–267. [Google Scholar] [CrossRef]
- Fatemi, A.; Yang, L. Cumulative fatigue damage and life prediction theories: A survey of the state of the art for homogeneous materials. Int. J. Fatigue 1998, 20, 9–34. [Google Scholar] [CrossRef]
- Wood, W. Recent Observations on Fatigue Failure in Metals; ASTM International: West Conshohocken, PA, USA, 1959. [Google Scholar]
- Demir, O. Prediction of crack initiation angle in brittle structures containing inclined cracks. Mech. Solids 2021, 56, 1066–1075. [Google Scholar] [CrossRef]
- Morrow, J. Fatigue design handbook. Adv. Eng. 1968, 4, 21–29. [Google Scholar]
- Smith, C. Small specimen data for predicting life of full-scale structures. In Symposium on Fatigue Tests of Aircraft Structures: Low-Cycle, Full-Scale, and Helicopters; ASTM International: West Conshohocken, PA, USA, 1963. [Google Scholar]
- Morrow, J.; Martin, J.; Dowling, N.E. Local Stress-Strain Approach to Cumulative Fatigue Damage Analysis. Department of Theoretical and Applied Mechanics, College of Engineering, University of Illinois in Urbana: Urbana, IL, USA, 1974. [Google Scholar]
- Crews, J.; Hardrath, H. A study of cyclic plastic stresses at a notch root. Exp. Mech. 1966, 6, 313–320. [Google Scholar] [CrossRef]
- Sangid, M.D. The physics of fatigue crack initiation. Int. J. Fatigue 2013, 57, 58–72. [Google Scholar] [CrossRef]
- Srivatsan, S.; Sudarshan, T. Mechanisms of fatigue crack initiation in metals: Role of aqueous environments. J. Mater. Sci. 1988, 23, 1521–1533. [Google Scholar] [CrossRef]
- Sinclair, G.; Dolan, T.J. Influence of Grain Size on Work Hardening and Fatigue Characteristics of Alpha Brass. ASM Trans. Q 1952, 44, 929–948. [Google Scholar]
- Thompson, A.W. The comparison of yield and fatigue strength dependence on grain size. Scr. Metall. 1971, 5, 859–863. [Google Scholar] [CrossRef]
- Wood, W. Formation of fatigue cracks. Philos. Mag. 1958, 3, 692–699. [Google Scholar] [CrossRef]
- Cottrell, A.H.; Hull, D. Extrusion and intrusion by cyclic slip in copper. Proc. R. Soc. Lond. Ser. A Math. Phys. Sci. 1957, 242, 211–213. [Google Scholar]
- Broom, T.; Summerton, J. The fatigue of zinc single crystals. Philos. Mag. 1963, 8, 1847–1862. [Google Scholar] [CrossRef]
- Basinski, Z.; Basinski, S. Fundamental aspects of low amplitude cyclic deformation in face-centred cubic crystals. Prog. Mater. Sci. 1992, 36, 89–148. [Google Scholar] [CrossRef]
- Seeger, A.; Diehl, J.; Mader, S.; Rebstock, H. Work-hardening and work-softening of face-centred cubic metal crystals. Philos. Mag. 1957, 2, 323–350. [Google Scholar] [CrossRef]
- Friedel, J.; Feltham, P. A discussion on work-hardening and fatigue in metals. Proc. R. Soc. Lond. Ser. A Math. Phys. Sci. 1957, 242, 145. [Google Scholar]
- Basinski, Z.; Korbel, A.; Basinski, S. The temperature dependence of the saturation stress and dislocation substructure in fatigued copper single crystals. Acta Metall. 1980, 28, 191–207. [Google Scholar] [CrossRef]
- Christ, H.J.; Mughrabi, H. Cyclic stress-strain response and microstructure under variable amplitude loading. Fatigue Fract. Eng. Mater. Struct. 1996, 19, 335–348. [Google Scholar] [CrossRef]
- Lerch, B.A.; Jayaraman, N.; Antolovich, S.D. A study of fatigue damage mechanisms in Waspaloy from 25 to 800 C. Mater. Sci. Eng. 1984, 66, 151–166. [Google Scholar] [CrossRef]
- Healy, J.; Grabowski, L.; Beevers, C. Short-fatigue-crack growth in a nickel-base superalloy at room and elevated temperature. Int. J. Fatigue 1991, 13, 133–138. [Google Scholar] [CrossRef]
- Yates, J.; Zhang, W.; Miller, K. The initiation and propagation behaviour of short fatigue cracks in Waspaloy subjected to bending. Fatigue Fract. Eng. Mater. Struct. 1993, 16, 351–362. [Google Scholar] [CrossRef]
- Smith, R.; Liu, Y.; Grabowski, L. Short fatigue crack growth behaviour in Waspaloy at room and elevated temperatures. Fatigue Fract. Eng. Mater. Struct. 1996, 19, 1505–1514. [Google Scholar] [CrossRef]
- Toh, S.F.; Rainforth, W. Fatigue of a nickel base superalloy with bimodal grain size. Mater. Sci. Technol. 1996, 12, 1007–1014. [Google Scholar] [CrossRef]
- Pang, H.; Reed, P. Fatigue crack initiation and short crack growth in nickel-base turbine disc alloysthe effects of microstructure and operating parameters. Int. J. Fatigue 2003, 25, 1089–1099. [Google Scholar] [CrossRef]
- Barsom, J.; McNicol, R. Effect of Stress Concentration on Fatigue-Crack Initiation in HY-130 Steel; ASTM International: West Conshohocken, PA, USA, 1974. [Google Scholar]
- Nesterenko, B.; Nesterenko, G.I.; Basov, V.N. Fracture behaviour of skin materials of civil airplane structures. In ICAF 2009, Bridging the Gap between Theory and Operational Practice; Springer: Berlin/Heidelberg, Germany, 2009; pp. 661–683. [Google Scholar]
- Kim, W.H.; Laird, C. Crack nucleation and stage I propagation in high strain fatigue II. Mechanism. Acta Metall. 1978, 26, 789–799. [Google Scholar] [CrossRef]
- Figueroa, J.; Laird, C. Crack initiation mechanisms in copper polycrystals cycled under constant strain amplitudes and in step tests. Mater. Sci. Eng. 1983, 60, 45–58. [Google Scholar] [CrossRef]
- Liang, F.L.; Laird, C. Control of intergranular fatigue cracking by slip homogeneity in copper I: Effect of grain size. Mater. Sci. Eng. A 1989, 117, 95–102. [Google Scholar] [CrossRef]
- Tanaka, K.; Mura, T. A dislocation model for fatigue crack initiation. J. Appl. Mech. Mar. 1981, 48, 97–103. [Google Scholar] [CrossRef]
- Mughrabi, H. A model of high-cycle fatigue-crack initiation at grain boundaries by persistent slip bands. In Defects, Fracture and Fatigue; Springer: Berlin/Heidelberg, Germany, 1983; pp. 139–146. [Google Scholar]
- Mughrabi, H.; Wang, R.; Differt, K.; Essmann, U. Fatigue crack initiation by cyclic slip irreversibilities in high-cycle fatigue. In Fatigue Mechanisms: Advances in Quantitative Measurement of Physical Damage: A Conference; ASTM International: West Conshohocken, PA, USA, 1983; Volume 811, p. 1. [Google Scholar]
- Christ, H.J. On the orientation of cyclic-slip-induced intergranular fatigue cracks in face-centered cubic metals. Mater. Sci. Eng. A 1989, 117, L25–L29. [Google Scholar] [CrossRef]
- Liu, W.; Bayerlein, M.; Mughrabi, H.; Day, A.; Quested, P. Crystallographic features of intergranular crack initiation in fatigued copper polycrystals. Acta Metall. Mater. 1992, 40, 1763–1771. [Google Scholar] [CrossRef]
- Burmeister, H.J.; Richter, R. Investigations on the origin of grain boundary cracks in fatigued fcc metals. Acta Mater. 1997, 45, 709–714. [Google Scholar] [CrossRef]
- Zhang, Z.; Wang, Z. Dependence of intergranular fatigue cracking on the interactions of persistent slip bands with grain boundaries. Acta Mater. 2003, 51, 347–364. [Google Scholar] [CrossRef]
- Mazánová, V.; Heczko, M.; Polák, J. On the mechanism of fatigue crack initiation in high-angle grain boundaries. Int. J. Fatigue 2022, 158, 106721. [Google Scholar] [CrossRef]
- Li, W.B.; Umezawa, O. A review of subsurface crack initiation models in high-cycle fatigue for titanium alloys. Key Eng. Mater. 2017, 741, 76–81. [Google Scholar] [CrossRef]
- Santecchia, E.; Hamouda, A.; Musharavati, F.; Zalnezhad, E.; Cabibbo, M.; El Mehtedi, M.; Spigarelli, S. A review on fatigue life prediction methods for metals. Adv. Mater. Sci. Eng. 2016, 2016, 9573524. [Google Scholar] [CrossRef]
- Zhao, Z.; Zhang, F.; Dong, C.; Yang, X.; Chen, B. Initiation and early-stage growth of internal fatigue cracking under very-high-cycle fatigue regime at high temperature. Metall. Mater. Trans. A 2020, 51, 1575–1592. [Google Scholar] [CrossRef]
- Grosskreutz, J. The mechanisms of metal fatigue (I). Phys. Status Solidi B 1971, 47, 11–31. [Google Scholar] [CrossRef]
- Laird, C. Mechanisms of fatigue crack nucleation. Corros Fatigue NACE-2 1972, 88–117. [Google Scholar]
- Zhao, Z.; Liang, Z.; Li, Q.; Zhang, F.; Chen, B. Crack initiation and propagation behavior under high-temperature very-high cycle fatigue: Directionally solidified columnar-grained vs. single-crystal superalloys. Mater. Sci. Eng. A 2022, 836, 142711. [Google Scholar] [CrossRef]
- Zhou, J.; Barrett, R.A.; Leen, S.B. A physically-based method for predicting high temperature fatigue crack initiation in P91 welded steel. Int. J. Fatigue 2021, 153, 106480. [Google Scholar] [CrossRef]
- Osterstock, S.; Robertson, C.F.; Sauzay, M.; Degallaix, S.; Aubin, V. Prediction of the scatter of crack initiation under high cycle fatigue. In Key engineering materials; Trans Tech Publications Ltd.: Zurich, Switzerland, 2007; Volume 345, pp. 363–366. [Google Scholar]
- Lee, H.W.; Basaran, C. Predicting high cycle fatigue life with unified mechanics theory. Mech. Mater. 2022, 164, 104116. [Google Scholar] [CrossRef]
- Jiang, J.; Dunne, F.P.; Britton, T.B. Toward predictive understanding of fatigue crack nucleation in Ni-based superalloys. Jom 2017, 69, 863–871. [Google Scholar] [CrossRef] [PubMed]
- Mughrabi, H. Microstructural mechanisms of cyclic deformation, fatigue crack initiation and early crack growth. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2015, 373, 20140132. [Google Scholar] [CrossRef] [PubMed]
- Giroux, P.F. Experimental Study and Simulation of Cyclic Softening of Tempered Martensite Ferritic Steels. PhD Thesis, École Nationale Supéri des Mines de Paris, Paris, France, 2011. [Google Scholar]
- Mura, T. A theory of fatigue crack initiation. Mater. Sci. Eng. A 1994, 176, 61–70. [Google Scholar] [CrossRef]
- Sauzay, M.; Blondel, P. Can polycrystalline microstructure account for the scatter in the number of cycles to initiation of a high-cycle fatigue crack? Eng. Mech. 2004, 11, 377–381. [Google Scholar]
- Chan, K.S. A microstructure-based fatigue-crack-initiation model. Metall. Mater. Trans. A 2003, 34, 43–58. [Google Scholar] [CrossRef]
- Wu, X. On Tanaka-Mura’s fatigue crack nucleation model and validation. Fatigue Fract. Eng. Mater. Struct. 2018, 41, 894–8993. [Google Scholar] [CrossRef]
- Tanaka, K.; Mura, T. A theory of fatigue crack initiation at inclusions. Metall. Trans. A 1982, 13, 117–123. [Google Scholar] [CrossRef]
- Venkataraman, G.; Chung, Y.W.; Nakasone, Y.; Mura, T. Free energy formulation of fatigue crack initiation along persistent slip bands: Calculation of S N curves and crack depths. Acta Metall. Mater. 1990, 38, 31–40. [Google Scholar] [CrossRef]
- Venkataraman, G.; Chung, Y.W.; Mura, T. Application of minimum energy formalism in a multiple slip band model for fatigue II. Crack nucleation and derivation of a generalised Coffin-Manson law. Acta Metall. Mater. 1991, 39, 2631–2638. [Google Scholar] [CrossRef]
- Harvey, S.; Marsh, P.; Gerberich, W. Atomic force microscopy and modeling of fatigue crack initiation in metals. Acta Metall. Mater. 1994, 42, 3493–3502. [Google Scholar] [CrossRef]
- Marchand, N.; Baïlon, J.P.; Dickson, J. Near-threshold fatigue crack growth in copper and alpha-brass: Grain-size and environmental effects. Metall. Trans. A 1988, 19, 2575–2587. [Google Scholar] [CrossRef]
- Ogi, H.; Hirao, M.; Aoki, S. Noncontact monitoring of surface-wave nonlinearity for predicting the remaining life of fatigued steels. J. Appl. Phys. 2001, 90, 438–442. [Google Scholar] [CrossRef]
- Morris, W.; Buck, O.; Inman, R. Acoustic harmonic generation due to fatigue damage in high-strength aluminum. J. Appl. Phys. 1979, 50, 6737–6741. [Google Scholar] [CrossRef]
- Kulkarni, S.S.; Sun, L.; Moran, B.; Krishnaswamy, S.; Achenbach, J. A probabilistic method to predict fatigue crack initiation. Int. J. Fract. 2006, 137, 9–17. [Google Scholar] [CrossRef]
- Melander, A.; Larsson, M. The effect of stress amplitude on the cause of fatigue crack initiation in a spring steel. Int. J. Fatigue 1993, 15, 119–131. [Google Scholar] [CrossRef]
- De Bussac, A.; Lautridou, J. A probabilistic model for prediction of LCF surface crack initiation in PM alloys. Fatigue Fract. Eng. Mater. Struct. 1993, 16, 861–874. [Google Scholar] [CrossRef]
- De Bussac, A. Prediction of the competition between surface and internal fatigue crack initiation in PM alloys. Fatigue Fract. Eng. Mater. Struct. 1994, 17, 1319–1325. [Google Scholar] [CrossRef]
- Dunne, F. Fatigue crack nucleation: Mechanistic modelling across the length scales. Curr. Opin. Solid State Mater. Sci. 2014, 18, 170–179. [Google Scholar] [CrossRef]
- Makkonen, M. Statistical size effect in the fatigue limit of steel. Int. J. Fatigue 2001, 23, 395–402. [Google Scholar] [CrossRef]
- Makkonen, M. Notch size effects in the fatigue limit of steel. Int. J. Fatigue 2003, 25, 17–26. [Google Scholar] [CrossRef]
- Kelestemur, M.H.; Chaki, T. The effect of overload on the fatigue crack growth behaviour of 304 stainless steel in hydrogen. Fatigue Fract. Eng. Mater. Struct. 2001, 24, 15–22. [Google Scholar] [CrossRef]
- Wei, L.; De los Rios, E.; James, M. Experimental study and modelling of short fatigue crack growth in aluminium alloy Al7010-T7451 under random loading. Int. J. Fatigue 2002, 24, 963–975. [Google Scholar] [CrossRef]
- Moreno, B.; Zapatero, J.; Dominguez, J. An experimental analysis of fatigue crack growth under random loading. Int. J. Fatigue 2003, 25, 597–608. [Google Scholar] [CrossRef]
- Ahmadi, A.; Zenner, H. Lifetime simulation under multiaxial random loading with regard to the microcrack growth. Int. J. Fatigue 2006, 28, 954–962. [Google Scholar] [CrossRef]
- Kim, S.T.; Tadjiev, D.; Yang, H.T. Fatigue life prediction under random loading conditions in 7475-T7351 aluminum alloy using the RMS model. Int. J. Damage Mech. 2006, 15, 89–102. [Google Scholar] [CrossRef]
- Yu, S.; Xie, X.; Zhang, J.; Zhao, Z. Ductile fracture modeling of initiation and propagation in sheet-metal blanking processes. J. Mater. Process. Technol. 2007, 187, 169–172. [Google Scholar] [CrossRef]
- Paris, P.; Erdogan, F. A critical analysis of crack propagation laws. J. Basic Eng. Dec. 1963, 85, 528–534. [Google Scholar] [CrossRef]
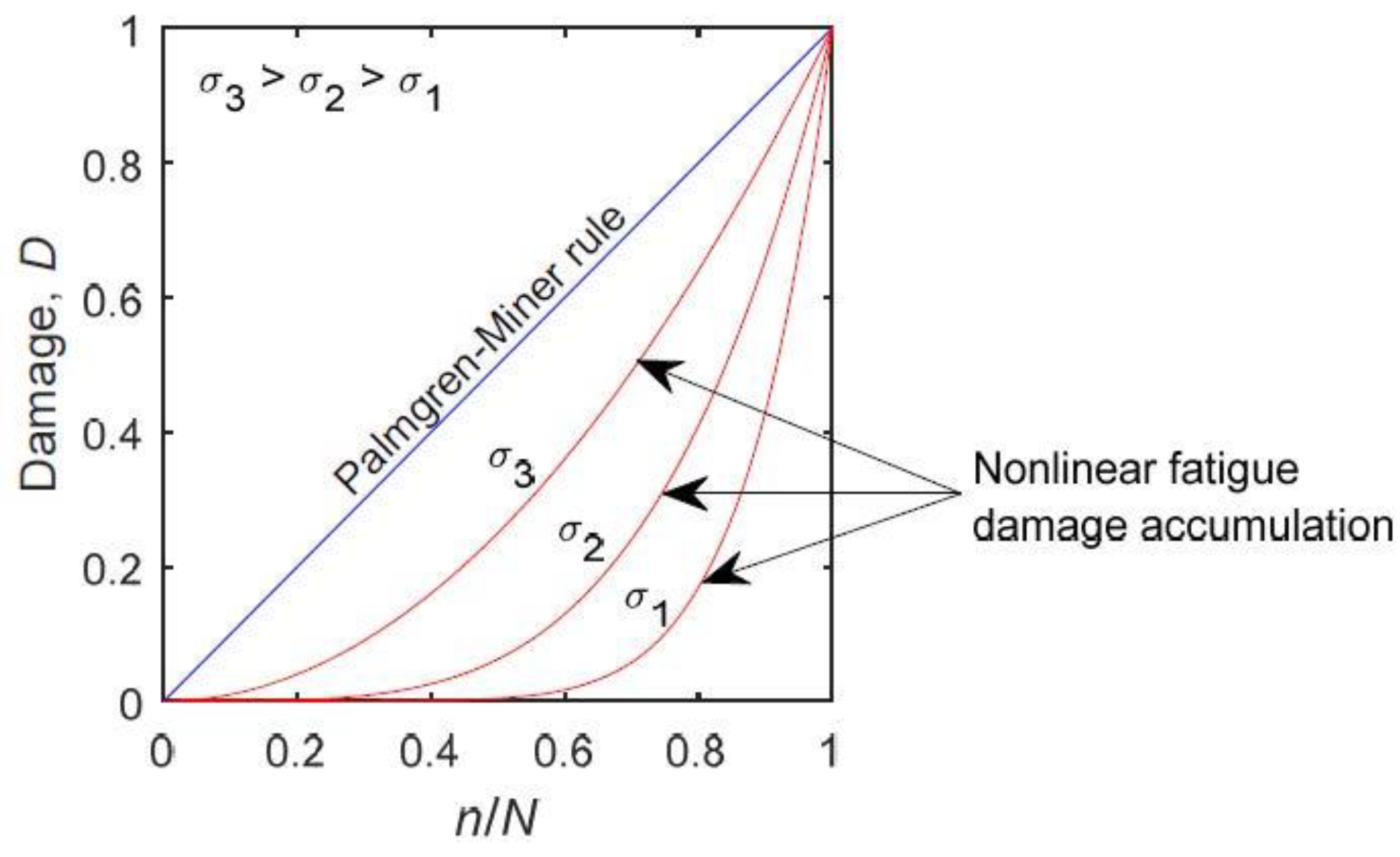
- Rege, K.; Pavlou, D.G. A one-parameter nonlinear fatigue damage accumulation model. Int. J. Fatigue 2017, 98, 234–246. [Google Scholar] [CrossRef]
- Liu, Y.; Mahadevan, S. Stochastic fatigue damage modeling under variable amplitude loading. Int. J. Fatigue 2007, 29, 1149–1161. [Google Scholar] [CrossRef]
- Marco, S.; Starkey, W. A concept of fatigue damage. Trans. Am. Soc. Mech. Eng. 1954, 76, 627–632. [Google Scholar] [CrossRef]
- Miner, M.A. Cumulative damage in fatigue. J. Appl. Mech. Sep. 1945, 12, A159–A164. [Google Scholar] [CrossRef]
- Yang, L.; Fatemi, A. Cumulative fatigue damage mechanisms and quantifying parameters: A literature review. J. Test. Eval. 1998, 26, 89–100. [Google Scholar]
- Manson, S.; Freche, J.C.; Ensign, C.R. Application of a Double Linear Damage Rule to Cumulative Fatigue; National Aeronautics and Space Administration: Washington, DC, USA, 1976; Volume 3839. [Google Scholar]
- Manson, S. Interfaces between fatigue, creep, and fracture. Int. J. Fract. Mech. 1966, 2, 327. [Google Scholar] [CrossRef]
- Basquin, O. The exponential law of endurance tests. Proc. Am. Soc. Test Mater. 1990, 10, 625–630. [Google Scholar]
- Berge, S. Proceedings of the 13th International Ship and Offshore Structures Congress; Pergamon Press: Oxford, UK, 1997; Volume 1. [Google Scholar]
- Wu, Y.S.; Zhou, G.J.; Cui, W.C. Practical Design of Ships and Other Floating Structures: Eighth International Symposium-PRADS 2001 (2 Volume set); Elsevier: Amsterdam, The Netherlands, 2001; Volume 1. [Google Scholar]
- Manson, S.S. Fatigue behaviour in strain cycling in the low-and intermediate-cycle range. In Proceedings of the 10th Sagamore Army Materials Research Conference, Dagamore, NY, USA, 13–16 August 1963. [Google Scholar]
- Coffin, L.F.; Tavernelli, J. The cyclic straining and fatigue of metals. Trans Met. Ing. Soc. AIME 1959, 215, 794–806. [Google Scholar]
- Kachanov, L. Introduction to Continuum Damage Mechanics; Springer Science & Business Media: Berlin/Heidelberg, Germany, 1986; Volume 10. [Google Scholar]
- Chaboche, J.; Lesne, P. A non-linear continuous fatigue damage model. Fatigue Fract. Eng. Mater. Struct. 1988, 11, 1–17. [Google Scholar] [CrossRef]
- Bannantine, J.; Comer, J.; Handrock, J. Fundamentals of Metal Fatigue Analysis. In Research Supported by the University of Illinois; Prentice Hall: Englewood Cliffs, NJ, USA, 1990; p. 286. [Google Scholar]
- Fang, Z.; Wang, L.; Wang, Z.; He, Y. A Comparison of Two Methods Modeling High-Temperature Fatigue Crack Initiation in Ferrite–Pearlite Steel. Crystals 2022, 12, 718. [Google Scholar] [CrossRef]
- Cheong, K.S.; Smillie, M.J.; Knowles, D.M. Predicting fatigue crack initiation through image-based micromechanical modeling. Acta Mater. 2007, 55, 1757–1768. [Google Scholar] [CrossRef]
- McDowell, D.; Dunne, F. Microstructure-sensitive computational modeling of fatigue crack formation. Int. J. Fatigue 2010, 32, 1521–1542. [Google Scholar] [CrossRef]
- D’Angela, D.; Ercolino, M.; Bellini, C.; Di Cocco, V.; Iacoviello, F. Failure criteria for real-time assessment of ductile cast irons subjected to various loading conditions. Smart Mater. Struct. 2020, 30, 017001. [Google Scholar] [CrossRef]
- He, C.; Kitamura, K.; Yang, K.; Liu, Y.J.; Wang, Q.Y.; Chen, Q. Very high cycle fatigue crack initiation mechanism in nugget zone of AA 7075 friction stir welded joint. Adv. Mater. Sci. Eng. 2017, 2017, 7189369. [Google Scholar] [CrossRef]
- Grabulov, A.; Petrov, R.; Zandbergen, H. EBSD investigation of the crack initiation and TEM/FIB analyses of the microstructural changes around the cracks formed under Rolling Contact Fatigue (RCF). Int. J. Fatigue 2010, 32, 576–583. [Google Scholar] [CrossRef]
- Liu, J.; Wei, Y.; Yan, C.; Lang, S. Method for predicting crack initiation life of notched specimen based on damage mechanics. J. Shanghai Jiaotong Univ. Sci. 2018, 23, 286–290. [Google Scholar] [CrossRef]
- Zhu, Z.; Li, G.; Dai, G.; Zhao, J.; Xu, L.; Zhang, Q. Mechanisms and new parameter attribute reduction of high-speed railway wheel rim steel subjected to low temperature fatigue. Mater. Sci. Eng. A 2016, 673, 476–491. [Google Scholar] [CrossRef]
- Zhang, D.; Li, Z.; Wu, H.; Huang, F. Experimental study on fatigue behavior of Q420 high-strength steel at low temperatures. J. Constr. Steel Res. 2018, 145, 116–127. [Google Scholar] [CrossRef]
- Liao, X.; Wang, Y.; Qian, X.; Shi, Y. Fatigue crack propagation for Q345qD bridge steel and its butt welds at low temperatures. Fatigue Fract. Eng. Mater. Struct. 2018, 41, 675–687. [Google Scholar] [CrossRef]
- Walters, C.L.; Alvaro, A.; Maljaars, J. The effect of low temperatures on the fatigue crack growth of S460 structural steel. Int. J. Fatigue 2016, 82, 110–1186. [Google Scholar] [CrossRef]
- Moody, N.; Gerberich, W. Fatigue crack propagation in iron and two iron binary alloys at low temperatures. Mater. Sci. Eng. 1979, 41, 271–280. [Google Scholar] [CrossRef]
- Rice, J.R. Some remarks on elastic crack-tip stress fields. Int. J. Solids Struct. 1972, 8, 751–758. [Google Scholar] [CrossRef]
- Choe, H.; Chen, D.; Schneibel, J.; Ritchie, R. Ambient to high temperature fracture toughness and fatigue-crack propagation behavior in a Mo–12Si–8.5 B (at.%) intermetallic. Intermetallics 2001, 9, 319–329. [Google Scholar] [CrossRef]
- Thurston, K.V.; Gludovatz, B.; Hohenwarter, A.; Laplanche, G.; George, E.P.; Ritchie, R.O. Effect of temperature on the fatigue-crack growth behavior of the high-entropy alloy CrMnFeCoNi. Intermetallics 2017, 88, 65–72. [Google Scholar] [CrossRef]
- Kim, Y.W.; Oh, D.J.; Lee, J.M.; Noh, B.J.; Sung, H.J.; Ando, R.; Matsumoto, T.; Kim, M.H. An experimental study for fatigue performance of 7% nickel steels for type b liquefied natural gas carriers. J. Offshore Mech. Arct. Eng. 2016, 138, 031401. [Google Scholar] [CrossRef]
- Hu, G.; Cai, X.; Rong, Y. Fundamentals of Materials Science; Shanghai Jiao Tong University Press: Shanghai, China, 2010. [Google Scholar]
- Kolbe, M. The high temperature decrease of the critical resolved shear stress in nickel-base superalloys. Mater. Sci. Eng. A 2001, 319, 383–387. [Google Scholar] [CrossRef]
- Vennemann, A.; Langmaack, E.; Nembach, E. On the temperature dependence of the critical resolved shear stress of the g -strengthened superalloy NIMONIC PE16. Scr. Mater. 2002, 46, 723–728. [Google Scholar] [CrossRef]
- Li, X.; Zhang, R.; Wang, X.; Liu, Y.; Wang, C.; Zhang, H.; Li, L.; He, C.; Wang, Q. Effect of high temperature on crack initiation of super austenitic stainless steel 654SMO in very high cycle fatigue. Mater. Des. 2020, 193, 108750. [Google Scholar] [CrossRef]
- Xu, S.; Wu, X.; Han, E.; Ke, W.; Katada, Y. Crack initiation mechanisms for low cycle fatigue of type 316Ti stainless steel in high temperature water. Mater. Sci. Eng. 2008, 490, 16–25. [Google Scholar] [CrossRef]
- Pan, S.; Chen, R. Fatigue crack and evolution prediction of compacted graphite iron under thermal loading with variable amplitude. Eng. Fail. Anal. 2019, 102, 284–292. [Google Scholar] [CrossRef]
- Gu, T.; Stopka, K.S.; Xu, C.; McDowell, D.L. Prediction of maximum fatigue indicator parameters for duplex Ti–6Al–4V using extreme value theory. Acta Mater. 2020, 188, 504–516. [Google Scholar] [CrossRef]
- Dunne, F.; Wilkinson, A.; Allen, R. Experimental and computational studies of low cycle fatigue crack nucleation in a polycrystal. Int. J. Plast. 2007, 23, 273–295. [Google Scholar] [CrossRef]
- Hallberg, H.; Ås, S.K.; Skallerud, B. Crystal plasticity modeling of microstructure influence on fatigue crack initiation in extruded Al6082-T6 with surface irregularities. Int. J. Fatigue 2018, 111, 16–32. [Google Scholar] [CrossRef]
- Taylor, G.I.; Elam, C. The plastic extension and fracture of aluminium crystals. Proc. R. Soc. Lond. Ser. 1925, 108, 28–51. [Google Scholar]
- Taylor, G.I. The mechanism of plastic deformation of crystals. Part I. Theoretical. Proc. R. Soc. Lond. Ser. A 1934, 145, 362–387. [Google Scholar]
- Taylor, G.I.; Elam, C.F. Bakerian lecture: The distortion of an aluminium crystal during a tensile test. Proc. R. Soc. Lond. Ser. A 1923, 102, 643–667. [Google Scholar]
- Hill, R. Generalized constitutive relations for incremental deformation of metal crystals by multislip. J. Mech. Phys. Solids 1966, 14, 95–102. [Google Scholar] [CrossRef]
- Hill, R.; Rice, J. Constitutive analysis of elastic-plastic crystals at arbitrary strain. J. Mech. Phys. Solids 1972, 20, 401–413. [Google Scholar] [CrossRef]
- Huang, Y. A User-Material Subroutine Incroporating Single Crystal Plasticity in the ABAQUS Finite Element Program; Harvard University: Cambridge, UK, 1991. [Google Scholar]
- Li, H.; Kang, G.; Yu, C. Modeling uniaxial ratchetting of magnesium alloys by a new crystal plasticity considering dislocation slipping, twinning and detwinning mechanisms. Int. J. Mech. Sci. 2020, 179, 105660. [Google Scholar] [CrossRef]
- Li, Z.; Wang, Q.; Luo, A.A.; Fu, P.; Peng, L.; Wang, Y.; Wu, G. High cycle fatigue of cast Mg-3Nd-0.2 Zn magnesium alloys. Metall. Mater. Trans. A 2013, 44, 5202–52151. [Google Scholar] [CrossRef]
- Bergara, A.; Dorado, J.I.; Martin-Meizoso, A.; Martinez-Esnaola, J.M. Fatigue crack propagation in complex stress fields: Experiments and numerical simulations using the Extended Finite Element Method (XFEM). Int. J. Fatigue 2017, 103, 112–121. [Google Scholar] [CrossRef]



| Analysis Type | Advantages | Limitations |
|---|---|---|
| Stress based life analysis |
|
|
| Strain based analysis [19,105] |
|
|
| Continuum damage mechanics approaches [65,93] |
|
|
| Methodology | Findings | Refrences |
|---|---|---|
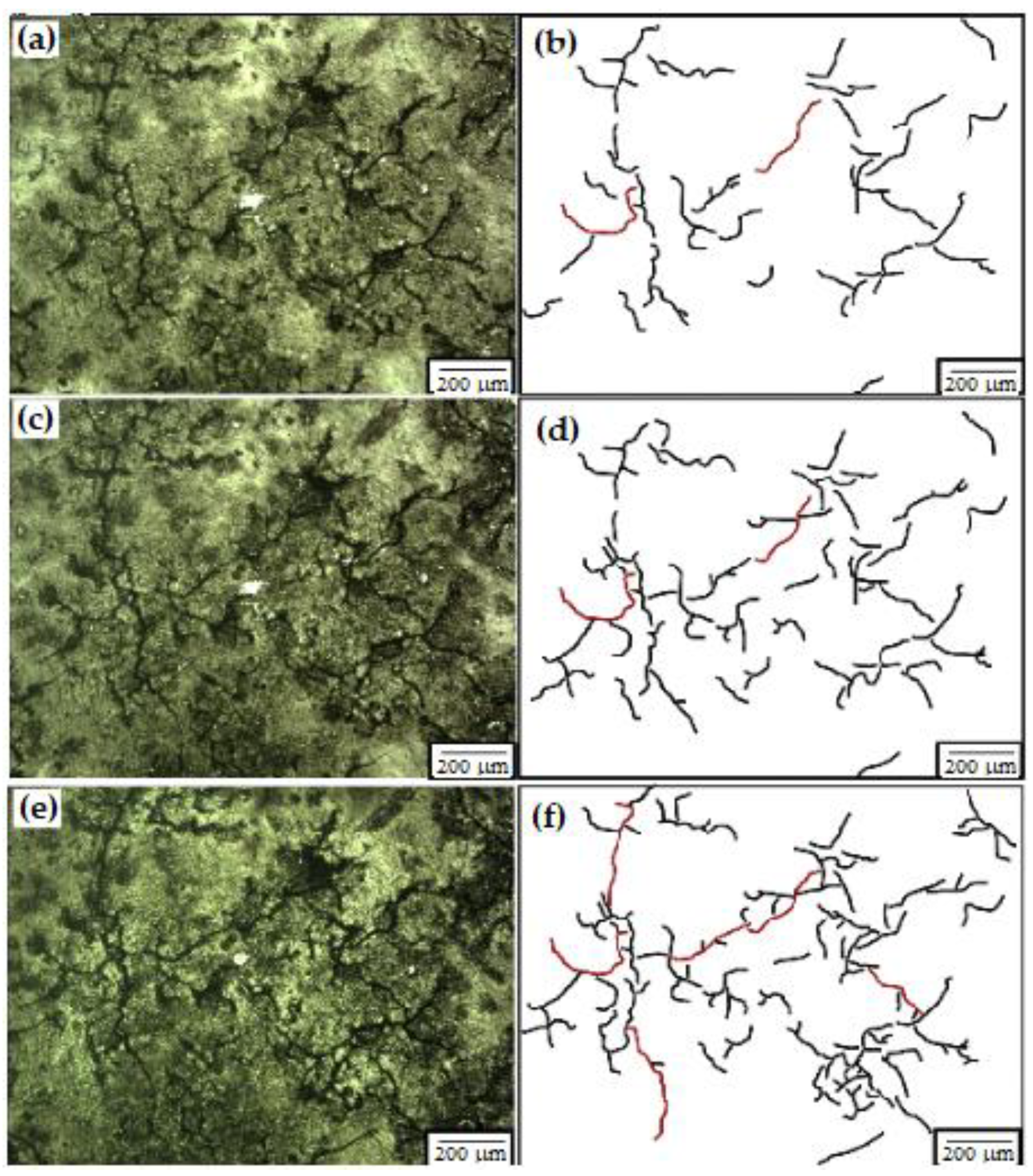
| Image-based micro-mechanical modeling | Fatigue cracks are more likely to start in grains that are poorly aligned with the loading direction. | [107] |
| Ultrasonic fatigue testing system (20 kHz) | The fatigue cracks started at the base of the friction stir weld, where the welding flaws were. | [110] |
| Transmission Electron Microscopy (TEM), Electron Backscattered Diffraction (EBSD), and Focused Ion Beam are examples of state-of-the-artelectron imaging techniques (FIB). | EBSD measurements showed a high level of local grain misorientation at the interface of Al2O3 and steel matrix. This suggests that microcracks could start there. | [111] |
| Measurement of elastic distortion using the high-angular resolution electron backscatter diffraction method (HR-EBSD) | After 20 cycles, the inclusion developed a tiny crack that propagated deeper into the matrix | [63] |
| The fracture initiation life of notched specimens under tension-compression loading is estimated using a model based on damage mechanics theory | Based on the damage theory and the closed-form solution, the damage evolution equation accounts for not only the loading circumstances and material quality, but also the geometric parameters of the notch specimen | [112] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kedir, Y.A.; Lemu, H.G. Prediction of Fatigue Crack Initiation under Variable Amplitude Loading: Literature Review. Metals 2023, 13, 487. https://doi.org/10.3390/met13030487
Kedir YA, Lemu HG. Prediction of Fatigue Crack Initiation under Variable Amplitude Loading: Literature Review. Metals. 2023; 13(3):487. https://doi.org/10.3390/met13030487
Chicago/Turabian StyleKedir, Yahiya Ahmed, and Hirpa G. Lemu. 2023. "Prediction of Fatigue Crack Initiation under Variable Amplitude Loading: Literature Review" Metals 13, no. 3: 487. https://doi.org/10.3390/met13030487
APA StyleKedir, Y. A., & Lemu, H. G. (2023). Prediction of Fatigue Crack Initiation under Variable Amplitude Loading: Literature Review. Metals, 13(3), 487. https://doi.org/10.3390/met13030487






