Terahertz Metamaterial Absorber Based on Ni–Mn–Sn Ferromagnetic Shape Memory Alloy Films
Abstract
:1. Introduction
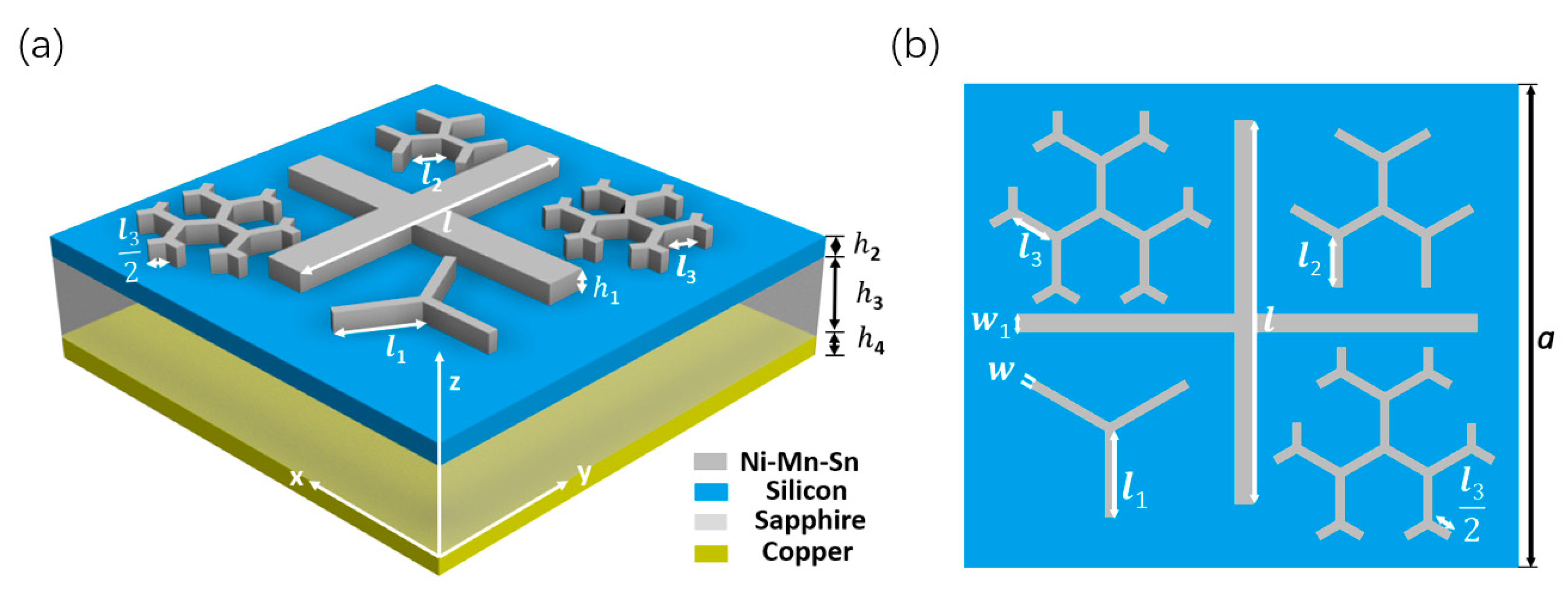
2. Structural Design and Method Simulation
3. Results and Discussion
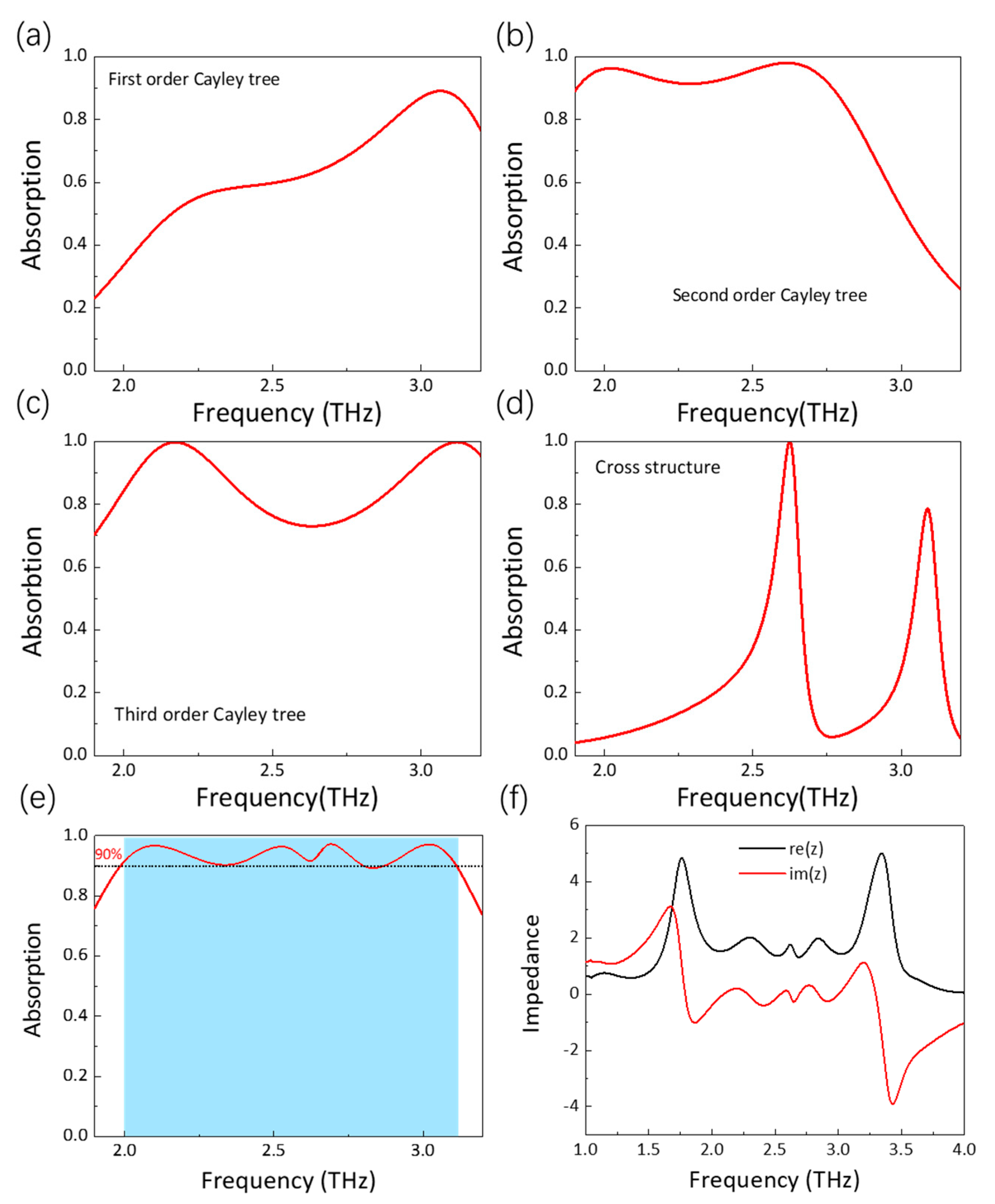
3.1. Broadband THz Absorber
3.2. Switchability between Broadband and Narrowband Absorption under the Magnetic Field
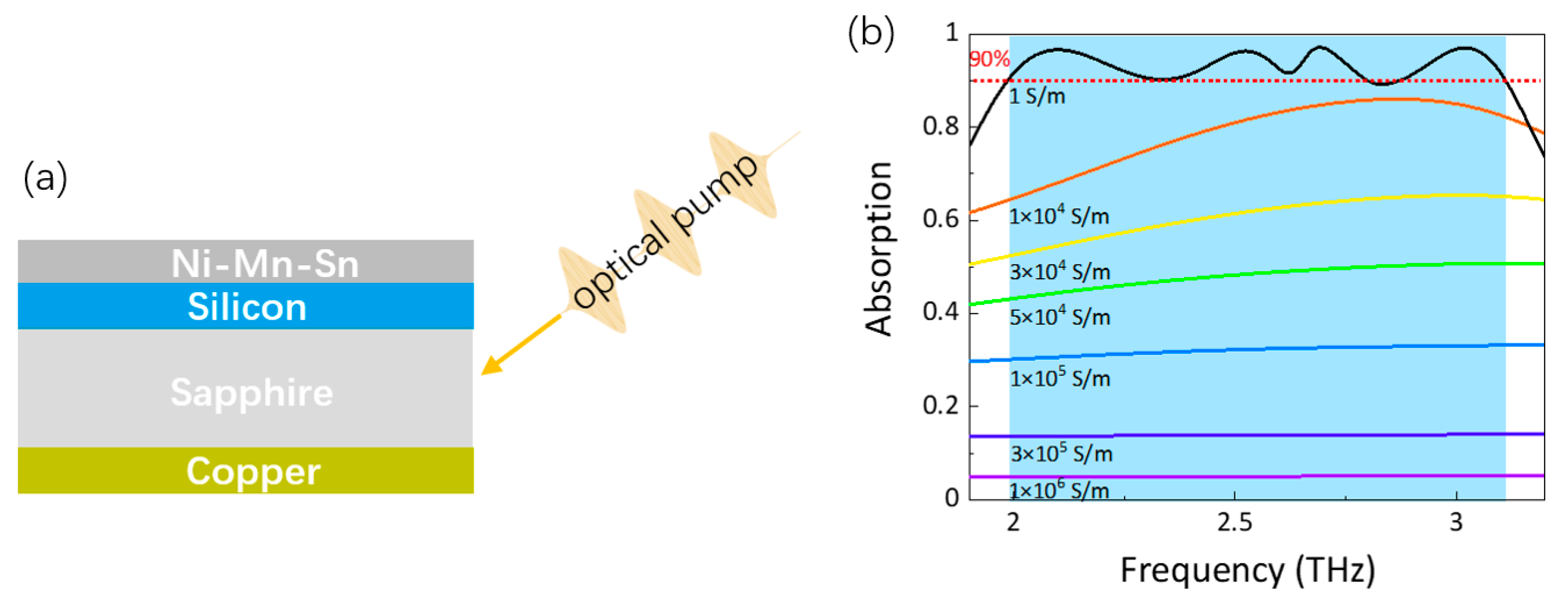
3.3. Multifunction under the Optical Pump
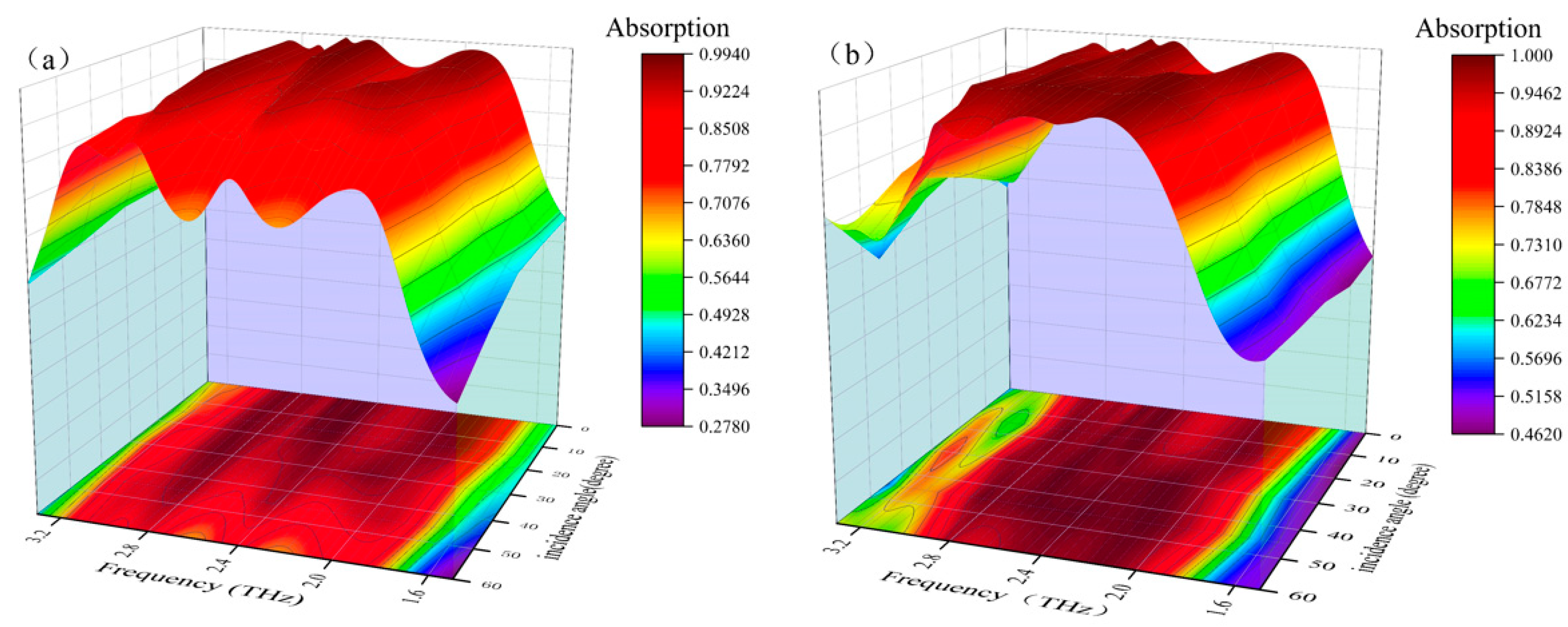
3.4. Incident Angle Sensitivity Characteristics
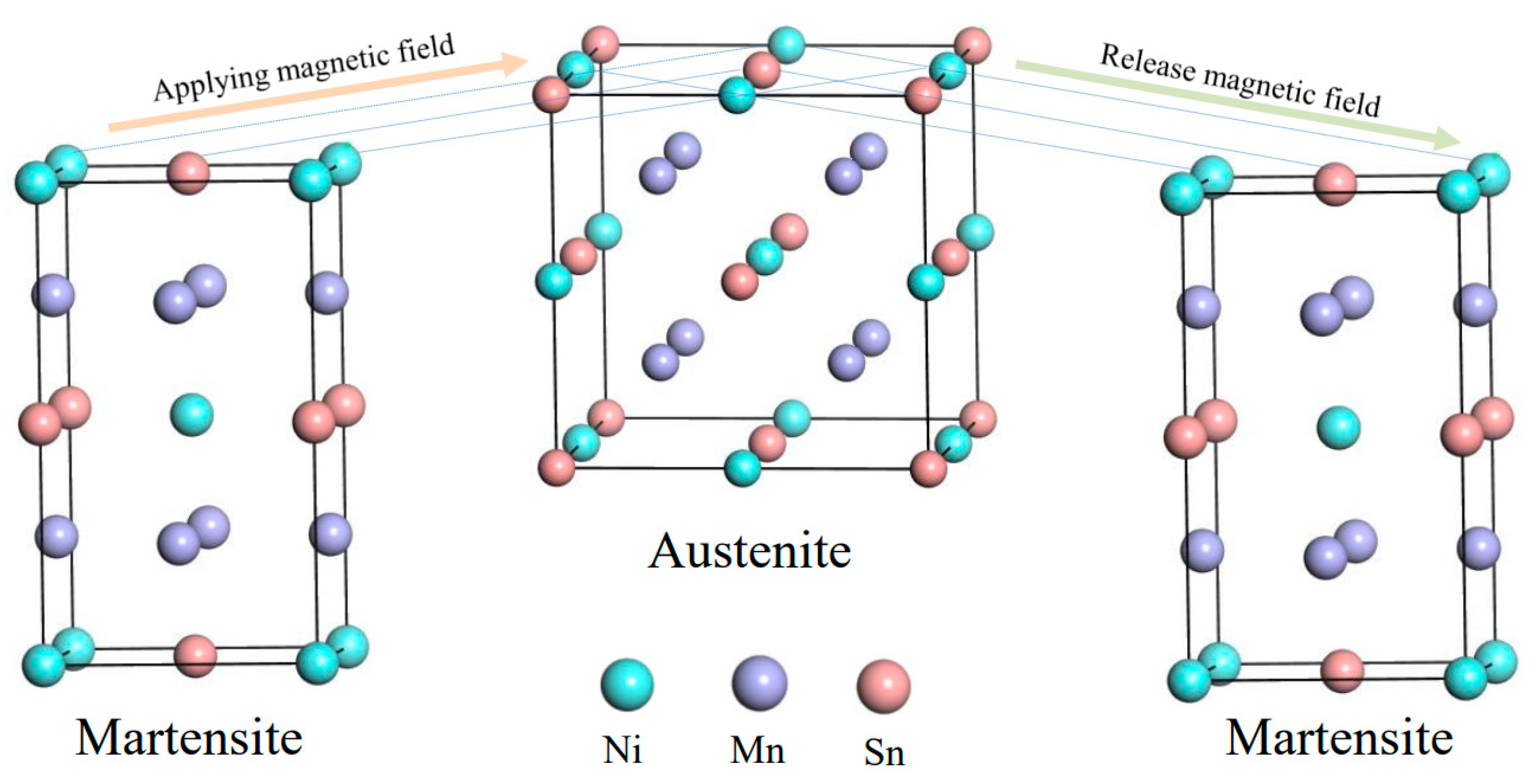
3.5. Mechanism of Wide/Narrow Band Absorption Conversion under Magnetic Field
3.6. The Feasibility of Experimental Demonstration
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Lu, M.; Shen, J.; Li, N.; Zhang, Y.; Zhang, C.; Liang, L.; Xu, X. Detection and identification of illicit drugs using terahertz imaging. J. Appl. Phys. 2006, 100, 103104. [Google Scholar] [CrossRef]
- Davies, A.G.; Burnett, A.D.; Fan, W.; Linfield, E.H.; Cunningham, J.E. Terahertz spectroscopy of explosives and drugs. Mater. Today 2008, 11, 18–26. [Google Scholar] [CrossRef]
- Beard, M.C.; Turner, G.M.; Schmuttenmaer, C.A. Terahertz spectroscopy. J. Phys. Chem. B 2002, 106, 7146–7159. [Google Scholar] [CrossRef]
- Taylor, Z.D.; Singh, R.S.; Bennett, D.B.; Tewari, P.; Kealey, C.P.; Bajwa, N.; Culjat, M.; Stojadinovic, A.; Lee, H.; Hubschman, J.-P.; et al. THz medical imaging: In vivo hydration sensing. IEEE Trans. Terahertz Sci. Technol. 2011, 1, 201–219. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Federici, J.; Moeller, L. Review of terahertz and subterahertz wireless communications. J. Appl. Phys. 2010, 107, 6–323. [Google Scholar] [CrossRef] [Green Version]
- Chen, H.T.; Padilla, W.J.; Zide, J.M.O.; Gossard, A.C.; Taylor, A.J.; Averitt, R.D. Active terahertz metamaterial devices. Nature 2006, 444, 597–600. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ju, L.; Geng, B.; Horng, J.; Girit, C.; Martin, M.; Hao, Z.; Bechtel, H.A.; Liang, X.; Zettl, A.; Shen, Y.R.; et al. Graphene plasmonics for tunable terahertz metamaterials. Nat. Nanotechnol. 2011, 6, 630–634. [Google Scholar] [CrossRef]
- Linden, S.; Enkrich, C.; Wegener, M.; Zhou, J.; Koschny, T.; Soukoulis, C.M. Magnetic response of metamaterials at 100 terahertz. Science 2004, 306, 1351–1353. [Google Scholar] [CrossRef] [Green Version]
- Bai, J.; Pang, Z.; Shen, P.; Chen, T.; Shen, W.; Wang, S.; Chang, S. A terahertz photo-thermoelectric detector based on metamaterial absorber. Opt. Commun. 2021, 497, 127184. [Google Scholar] [CrossRef]
- Carranza, I.E.; Grant, J.P.; Gough, J.; Cumming, D. Terahertz metamaterial absorbers implemented in CMOS technology for imaging applications: Scaling to large format focal plane arrays. IEEE J. Sel. Top. Quantum Electron. 2016, 23, 1–8. [Google Scholar] [CrossRef] [Green Version]
- Che, Z.; Zhang, G.; Ren, P.; Yue, J.; Li, Z.; Lun, Y.; Suo, J.; Zhu, J.; Zhang, Q.; Feng, Y. Narrow bandpass filter based on vanadium dioxide can be used for terahertz stealth. J. Opt. 2022, 51, 336–342. [Google Scholar] [CrossRef]
- Landy, N.I.; Sajuyigbe, S.; Mock, J.J.; Smith, D.R.; Padilla, W.J. Perfect metamaterial absorber. Phys. Rev. Lett. 2008, 100, 207402. [Google Scholar] [CrossRef]
- Shen, X.; Yang, Y.; Zang, Y.; Gu, J.; Han, J.; Zhang, W.; Jun Cui, T. Triple-band terahertz metamaterial absorber: Design, experiment, and physical interpretation. Appl. Phys. Lett. 2012, 101, 154102. [Google Scholar] [CrossRef]
- Zhang, Y.; Feng, Y.; Zhu, B.; Zhao, J.; Jiang, T. Graphene based tunable metamaterial absorber and polarization modulation in terahertz frequency. Opt. Express 2014, 22, 22743–22752. [Google Scholar] [CrossRef] [PubMed]
- Huang, L.; Chowdhury, D.R.; Ramani, S.; Reiten, M.T.; Luo, S.N.; Taylor, A.J.; Chen, H.T. Experimental demonstration of terahertz metamaterial absorbers with a broad and flat high absorption band. Opt. Express 2012, 37, 154–156. [Google Scholar] [CrossRef] [Green Version]
- Agarwal, S.; Srivastava, G.; Prajapati, Y.K. Dual band Vis-IR absorber using bismuth based helical metamaterial surface. Opt. Quantum Electron. 2022, 54, 772. [Google Scholar] [CrossRef]
- Islam, M.S.; Sultana, J.; Biabanifard, M.; Vafapour, Z.; Nine, M.J.; Dinovitser, A.; Cordeiro, C.M.B.; Ng, B.W.H.; Abbott, D. Tunable localized surface plasmon graphene metasurface for multiband superabsorption and terahertz sensing. Carbon 2020, 158, 559–567. [Google Scholar] [CrossRef]
- Du, C.; Zhou, D.; Guo, H.H.; Pang, Y.Q.; Shi, H.Y.; Liu, W.F.; Su, J.Z.; Singh, C.; Trukhanov, S.; Trukhanov, A.; et al. An ultra-broadband terahertz metamaterial coherent absorber using multilayer electric ring resonator structures based on anti-reflection coating. Nanoscale 2020, 12, 9769–9775. [Google Scholar] [CrossRef]
- Liu, Y.; Gu, S.; Luo, C.; Zhao, X. Ultra-thin broadband metamaterial absorber. Appl. Phys. A 2012, 108, 19–24. [Google Scholar] [CrossRef]
- Agarwal, S.; Prajapati, Y.K. Design of broadband absorber using 2-D materials for thermo-photovoltaic cell application. Opt. Commun. 2018, 413, 39–43. [Google Scholar] [CrossRef]
- Gu, S.; Barrett, J.P.; Hand, T.H.; Popa, B.I.; Cummer, S.A. A broadband low-reflection metamaterial absorber. J. Appl. Phys. 2010, 108, 064913. [Google Scholar] [CrossRef] [Green Version]
- Hedayati, M.K.; Javaherirahim, M.; Mozooni, B.; Abdelaziz, R.; Tavassolizadeh, A.; Chakravadhanula, V.S.K.; Zaporojtchenko, V.; Strunkus, T.; Faupel, F.; Elbahri, M. Design of a perfect black absorber at visible frequencies using plasmonic metamaterials. Adv. Mater. 2011, 23, 5410–5414. [Google Scholar] [CrossRef] [Green Version]
- Yu, P.; Besteiro, L.V.; Huang, Y.; Wu, J.; Fu, L.; Tan, H.H.; Jagadish, C.; Wiederrecht, G.P.; Govorov, A.O.; Wang, Z. Broadband metamaterial absorbers. Adv. Opt. Mater. 2019, 7, 1800995. [Google Scholar] [CrossRef] [Green Version]
- Xie, T.; Chen, D.; Yang, H.; Xu, Y.; Zhang, Z.; Yang, J. Tunable broadband terahertz waveband absorbers based on fractal technology of graphene metamaterial. Nanomaterials 2021, 11, 269. [Google Scholar] [CrossRef]
- Naveed, M.A.; Bilal, R.M.H.; Baqir, M.A.; Bashir, M.M.; Ali, M.M.; Rahim, A.A. Ultrawideband fractal metamaterial absorber made of nickel operating in the UV to IR spectrum. Opt. Express 2021, 29, 42911–42923. [Google Scholar] [CrossRef]
- Bilal, R.M.H.; Naveed, M.A.; Baqir, M.A.; Ali, M.M.; Rahim, A.A. Design of a wideband terahertz metamaterial absorber based on Pythagorean-tree fractal geometry. Opt. Mater. Express 2020, 10, 3007–3020. [Google Scholar] [CrossRef]
- Gottheim, S.; Zhang, H.; Govorov, A.O.; Halas, N.J. Fractal nanoparticle plasmonics: The Cayley tree. ACS Nano 2015, 9, 3284–3292. [Google Scholar] [CrossRef]
- Zubair, A.; Zubair, M.; Danner, A.; Mehmood, M.Q. Engineering multimodal spectrum of Cayley tree fractal meta-resonator supercells for ultrabroadband terahertz light absorption. Nanophotonics 2020, 9, 633–644. [Google Scholar] [CrossRef] [Green Version]
- Agarwal, S.; Prajapati, Y.K. Analysis of metamaterial-based absorber for thermo-photovoltaic cell applications. IET Optoelectron. 2017, 11, 208–212. [Google Scholar] [CrossRef]
- Huang, J.; Li, J.; Yang, Y.; Li, J.; Li, J.; Zhang, Y.; Yao, J. Broadband terahertz absorber with a flexible, reconfigurable performance based on hybrid-patterned vanadium dioxide metasurfaces. Opt. Express 2020, 28, 17832–17840. [Google Scholar] [CrossRef]
- Dao, R.N.; Kong, X.R.; Zhang, H.F.; Su, X.R. A tunable broadband terahertz metamaterial absorber based on the vanadium dioxide. Optik 2019, 180, 619–625. [Google Scholar] [CrossRef]
- Zhou, R.; Jiang, T.; Peng, Z.; Li, Z.; Zhang, M.; Wang, S.; Li, L.; Liang, H.; Ruan, S.; Su, H. Tunable broadband terahertz absorber based on graphene metamaterials and VO2. Opt. Mater. 2021, 114, 110915. [Google Scholar] [CrossRef]
- Agarwal, S.; Prajapati, Y.K. Broadband and polarization-insensitive helix metamaterial absorber using graphene for terahertz region. Appl. Phys. A 2016, 122, 561. [Google Scholar] [CrossRef]
- Badri, S.H.; Gilarlue, M.M.; SaeidNahaei, S.; Kim, J.S. Narrowband-to-broadband switchable and polarization-insensitive terahertz metasurface absorber enabled by phase-change material. J. Opt. 2022, 24, 025101. [Google Scholar] [CrossRef]
- Badri, S.H.; Soofi, H.; SaeidNahaei, S. Thermally reconfigurable extraordinary terahertz transmission using vanadium dioxide. JOSA B 2022, 39, 1614–1621. [Google Scholar] [CrossRef]
- Zhong, Y.; Huang, Y.; Zhong, S.; Lin, T.; Luo, M.; Shen, Y.; Ding, J. Tunable terahertz broadband absorber based on MoS2 ring-cross array structure. Opt. Mater. 2021, 114, 110996. [Google Scholar] [CrossRef]
- Liu, L.; Liu, W.; Song, Z. Ultra-broadband terahertz absorber based on a multilayer graphene metamaterial. J. Appl. Phys. 2020, 128, 093104. [Google Scholar] [CrossRef]
- Wu, T.; Shao, Y.; Ma, S.; Wang, G.; Gao, Y. Broadband terahertz absorber with tunable frequency and bandwidth by using Dirac semimetal and strontium titanate. Opt. Express 2021, 29, 7713–7723. [Google Scholar] [CrossRef] [PubMed]
- Liu, Y.; Huang, R.; Ouyang, Z. Numerical Investigation of Graphene and STO Based Tunable Terahertz Absorber with Switchable Bifunctionality of Broadband and Narrowband Absorption. Nanomaterials 2021, 11, 2044. [Google Scholar] [CrossRef]
- Song, Z.; Chen, A.; Zhang, J. Terahertz switching between broadband absorption and narrowband absorption. Opt. Express 2020, 28, 2037–2044. [Google Scholar] [CrossRef] [PubMed]
- Liu, W.; Song, Z. Terahertz absorption modulator with largely tunable bandwidth and intensity. Carbon 2021, 174, 617–624. [Google Scholar] [CrossRef]
- Otsuka, K.; Ren, X. Physical metallurgy of Ti–Ni-based shape memory alloys. Prog. Mater. Sci. 2005, 50, 511–678. [Google Scholar] [CrossRef]
- Cao, J.; Zhou, J.; Li, M.; Chen, J.; Zhang, Y.; Liu, X. Insightful understanding of three-phase interface behaviors in 1T-2H MoS2/CFP electrode for hydrogen evolution improvement. Chin. Chem. Lett. 2022, 33, 3745–3751. [Google Scholar] [CrossRef]
- Tan, C.; Liu, J.; Tian, X.; Zhu, J.; Zhang, K. Multifunctional and dynamically tunable terahertz metamaterials based on TiNi shape memory alloy films with a simple design. Results Phys. 2021, 24, 104165. [Google Scholar] [CrossRef]
- Zhang, K.; Ma, T.; Liu, J.; Tian, X.; Zhu, J.; Tan, C. Dynamically tunable and polarization-insensitive dual-band terahertz metamaterial absorber based on TiNi shape memory alloy films. Results Phys. 2021, 23, 104001. [Google Scholar] [CrossRef]
- Kainuma, R.; Imano, Y.; Ito, W.; Sutou, Y.; Morito, H.; Okamoto, S.; Kitakami, O.; Oikawa, K.; Fujita, A.; Kanomata, T.; et al. Magnetic-field-induced shape recovery by reverse phase transformation. Nature 2006, 439, 957–960. [Google Scholar] [CrossRef] [PubMed]
- Thomas, M.; Heczko, O.; Buschbeck, J.; Lai, Y.W.; McCord, J.; Kaufmann, S.; Schultz, L.; Fähler, S. Stray-Field-Induced Actuation of Free-Standing Magnetic Shape-Memory Films. Adv. Mater. 2009, 21, 3708–3711. [Google Scholar] [CrossRef]
- Zhang, K.; Tan, C.; Zhao, W.; Guo, E.; Tian, X. Computation-Guided Design of Ni–Mn–Sn Ferromagnetic Shape Memory Alloy with Giant Magnetocaloric Effect and Excellent Mechanical Properties and High Working Temperature via Multielement Doping. ACS Appl. Mater. Interfaces 2019, 11, 34827–34840. [Google Scholar] [CrossRef]
- Wang, X.; Liu, X.; Zhang, K.; Liu, R.; Zhu, J.; Tian, X.; Tan, C. Magnetic-induced dual-function tunable THz polarization conversion metamaterial based on Ni-Mn-Sn shape memory alloy films. Results Opt. 2022, 9, 100274. [Google Scholar] [CrossRef]
- Zheng, C.; Li, J.; Wang, S.; Li, J.; Li, M.; Zhao, H.; Hao, X.; Zang, H.; Zhang, Y.; Yao, J. Optically tunable all-silicon chiral metasurface in terahertz band. Appl. Phys. Lett. 2021, 118, 051101. [Google Scholar] [CrossRef]
- Shabanpour, J. Programmable anisotropic digital metasurface for independent manipulation of dual-polarized THz waves based on a voltage-controlled phase transition of VO2 microwires. J. Mater. Chem. C 2020, 8, 7189–7199. [Google Scholar] [CrossRef] [Green Version]
- Pan, W.; Yan, Y.; Ma, Y.; Shen, D. A terahertz metamaterial based on electromagnetically induced transparency effect and its sensing performance. Opt. Commun. 2019, 431, 115–119. [Google Scholar] [CrossRef]
- Bisht, S.; Saini, S.; Prakash, V.; Nautiyal, B. Study the various feeding techniques of microstrip antenna using design and simulation using CST microwave studio. Int. J. Emerg. Technol. Adv. Eng. 2014, 4, 318–324. [Google Scholar]
- Liu, A.Q.; Zhu, W.M.; Tsai, D.P.; Zheludev, N.I. Micromachined tunable metamaterials: A review. J. Opt. 2021, 14, 114009. [Google Scholar] [CrossRef] [Green Version]
- Kim, H.; Melinger, J.S.; Khachatrian, A.; Charipar, N.A.; Auyeung, R.C.Y.; Piqué, A. Fabrication of terahertz metamaterials by laser printing. Opt. Lett. 2010, 35, 4039–4041. [Google Scholar] [CrossRef]
- Walther, M.; Ortner, A.; Meier, H.; Löffelmann, U.; Smith, P.J.; Korvink, J.G. Terahertz metamaterials fabricated by inkjet printing. Appl. Phys. Lett. 2009, 95, 251107. [Google Scholar] [CrossRef]
- Smith, D.R.; Vier, D.C.; Koschny, T.; Soukoulis, C.M. Electromagnetic parameter retrieval from inhomogeneous metamaterials. Phys. Rev. E 2005, 71, 036617. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Smith, D.R.; Schultz, S.; Markoš, P.; Soukoulis, C.M. Determination of effective permittivity and permeability of metamaterials from reflection and transmission coefficients. Phys. Rev. B 2002, 65, 195104. [Google Scholar] [CrossRef] [Green Version]
- Auge, A.; Teichert, N.; Meinert, M.; Reiss, G.; Hütten, A.; Yüzüak, E.; Dincer, I.; Elerman, Y.; Ennen, I.; Schattschneider, P. Thickness dependence of the martensitic transformation, magnetism, and magnetoresistance in epitaxial Ni-Mn-Sn ultrathin films. Phys. Rev. B 2012, 85, 214118. [Google Scholar] [CrossRef]
- Krenke, T.; Acet, M.; Wassermann, E.F.; Moya, X.; Mañosa, L.; Planes, A. Martensitic transitions and the nature of ferromagnetism in the austenitic and martensitic states of Ni-Mn-Sn alloys. Phys. Rev. B 2005, 72, 014412. [Google Scholar] [CrossRef] [Green Version]
- Rao, Y.; Pan, L.; Ouyang, C.; Xu, Q.; Liu, L.; Li, Y.; Zhang, W. Asymmetric transmission of linearly polarized waves based on Mie resonance in all-dielectric terahertz metamaterials. Opt. Express 2020, 28, 29855–29864. [Google Scholar] [CrossRef]
- Chen, H.T. Interference theory of metamaterial perfect absorbers. Opt. Express 2012, 20, 7165–7172. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gu, J.; Singh, R.; Liu, X.; Zhang, X.; Ma, Y.; Zhang, S.; Zhang, W. Active control of electromagnetically induced transparency analogue in terahertz metamaterials. Nat. Commun. 2012, 3, 1151. [Google Scholar] [CrossRef] [Green Version]
- Li, J.S.; Li, X.J. Switchable tri-function terahertz metasurface based on polarization vanadium dioxide and photosensitive silicon. Opt. Express 2022, 30, 12823. [Google Scholar] [CrossRef] [PubMed]
- Bing, P.; Guo, X.; Wang, H.; Li, Z.; Yao, J. Characteristic analysis of a photoexcited tunable metamaterial absorber for terahertz waves. J. Opt. 2019, 48, 179–183. [Google Scholar] [CrossRef]
- Pitchappa, P.; Kumar, A.; Liang, H.; Prakash, S.; Wang, N.; Bettiol, A.A.; Venkatesan, T.; Lee, C.; Singh, R. Frequency-Agile Temporal Terahertz Metamaterials. Adv. Opt. Mater. 2020, 8, 2000101. [Google Scholar] [CrossRef]
- Ou, J.Y.; Plum, E.; Zhang, J.; Zheludev, N.I. An electromechanically reconfigurable plasmonic metamaterial operating in the near-infrared. Nat. Nanotechnol. 2013, 8, 252–255. [Google Scholar] [CrossRef]
- Butera, F. Shape memory actuators for automotive applications. Adv. Mater. Process. 2008, 166, 37. [Google Scholar]
- Jani, J.M.; Leary, M.; Subic, A.; Gibson, M.A. A review of shape memory alloy research, applications and opportunities. Mater. Des. 2014, 56, 1078–1113. [Google Scholar] [CrossRef]
- Strelec, J.K.; Lagoudas, D.C.; Khan, M.A.; Yen, J. Design and implementation of a shape memory alloy actuated reconfigurable airfoil. J. Intell. Mater. Syst. Struct. 2003, 14, 257–273. [Google Scholar] [CrossRef]
- Karami, M.; Chen, X. Nanomechanics of shape memory alloys. Mater. Today Adv. 2021, 10, 100141. [Google Scholar] [CrossRef]
- Gupta, B.; Pandey, S.; Nahata, A.; Zhang, T.; Nahata, A. Bistable physical geometries for terahertz plasmonic structures using shape memory alloys. Adv. Opt. Mater. 2017, 5, 1601008. [Google Scholar] [CrossRef]
- Zhou, H.; Zhang, T.; Guruswamy, S.; Nahata, A. An electrically tunable terahertz plasmonic device based on shape memory alloys and liquid metals. Adv. Opt. Mater. 2018, 6, 1700684. [Google Scholar] [CrossRef] [Green Version]
- Valente, J.; Ou, J.Y.; Plum, E.; Youngs, I.J.; Zheludev, N.I. Reconfiguring photonic metamaterials with currents and magnetic fields. Appl. Phys. Lett. 2015, 106, 111905. [Google Scholar] [CrossRef] [Green Version]


| References | Function | Working Bandwidth | Absorptance | Tunable Range | Material | Regulating Method |
|---|---|---|---|---|---|---|
| [30] | Switching | 1.85–4.3 THz | >90% | 4–100% | Vo2 | Temperature |
| [31] | Switching | 4.29–5.52 THz | >90% | 4–100% | Vo2 | Electric field |
| [32] | Switching | 1–2.03 THz | >90% | 0–99% | Graphene, Vo2 | Temperature, Electric field |
| [34] | Wide-narrow | 3.57–8.45 THz | >50% | — | Vo2 | Temperature |
| [36] | Switching | 1.2–2.67 THz | >90% | 0–95% | MoS2 | Temperature |
| [16] | Wide-band | 500–3000 nm | >90% | — | — | — |
| [20] | Dual-band | 200–1000 nm | >90% | — | Au/Cr/3 layers of grapheme/TMDs pair | — |
| This Work | Wide-narrow/Switching | 1.950–3.08 THz | >90% | 5–95% | Ni–Mn–Sn | Magnetic Fields |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, R.; Wang, X.; Zhu, J.; Tian, X.; Zhao, W.; Tan, C.; Zhang, K. Terahertz Metamaterial Absorber Based on Ni–Mn–Sn Ferromagnetic Shape Memory Alloy Films. Metals 2023, 13, 1162. https://doi.org/10.3390/met13071162
Liu R, Wang X, Zhu J, Tian X, Zhao W, Tan C, Zhang K. Terahertz Metamaterial Absorber Based on Ni–Mn–Sn Ferromagnetic Shape Memory Alloy Films. Metals. 2023; 13(7):1162. https://doi.org/10.3390/met13071162
Chicago/Turabian StyleLiu, Rui, Xiaochuan Wang, Jiachen Zhu, Xiaohua Tian, Wenbin Zhao, Changlong Tan, and Kun Zhang. 2023. "Terahertz Metamaterial Absorber Based on Ni–Mn–Sn Ferromagnetic Shape Memory Alloy Films" Metals 13, no. 7: 1162. https://doi.org/10.3390/met13071162
APA StyleLiu, R., Wang, X., Zhu, J., Tian, X., Zhao, W., Tan, C., & Zhang, K. (2023). Terahertz Metamaterial Absorber Based on Ni–Mn–Sn Ferromagnetic Shape Memory Alloy Films. Metals, 13(7), 1162. https://doi.org/10.3390/met13071162









