Large-Scale Atomistic Simulation of Sintering Process and Mechanical Properties of Al Matrix Composite with Different Reinforcements
Abstract
:1. Introduction
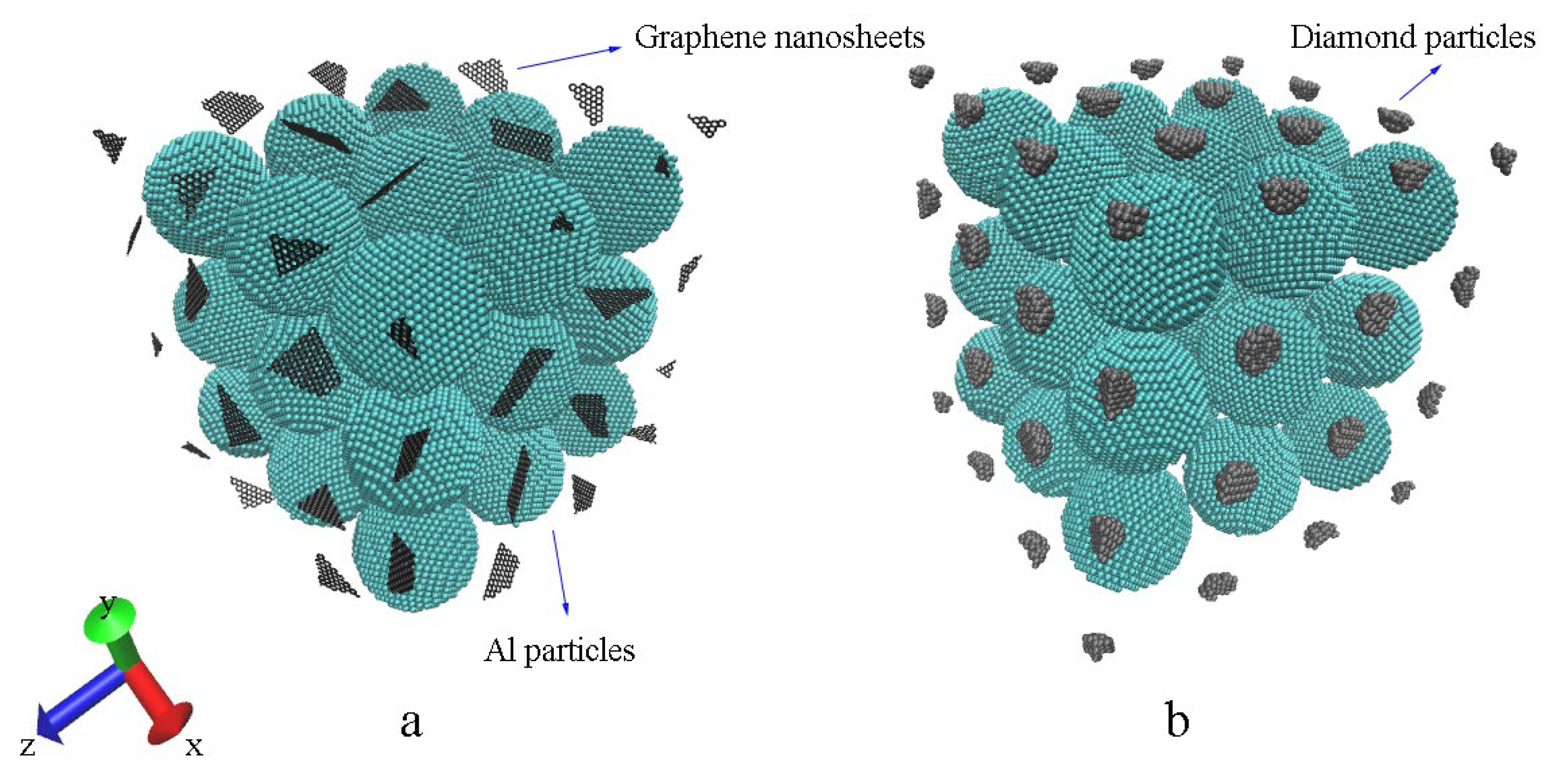
2. Model Methods
3. Results and Discussion
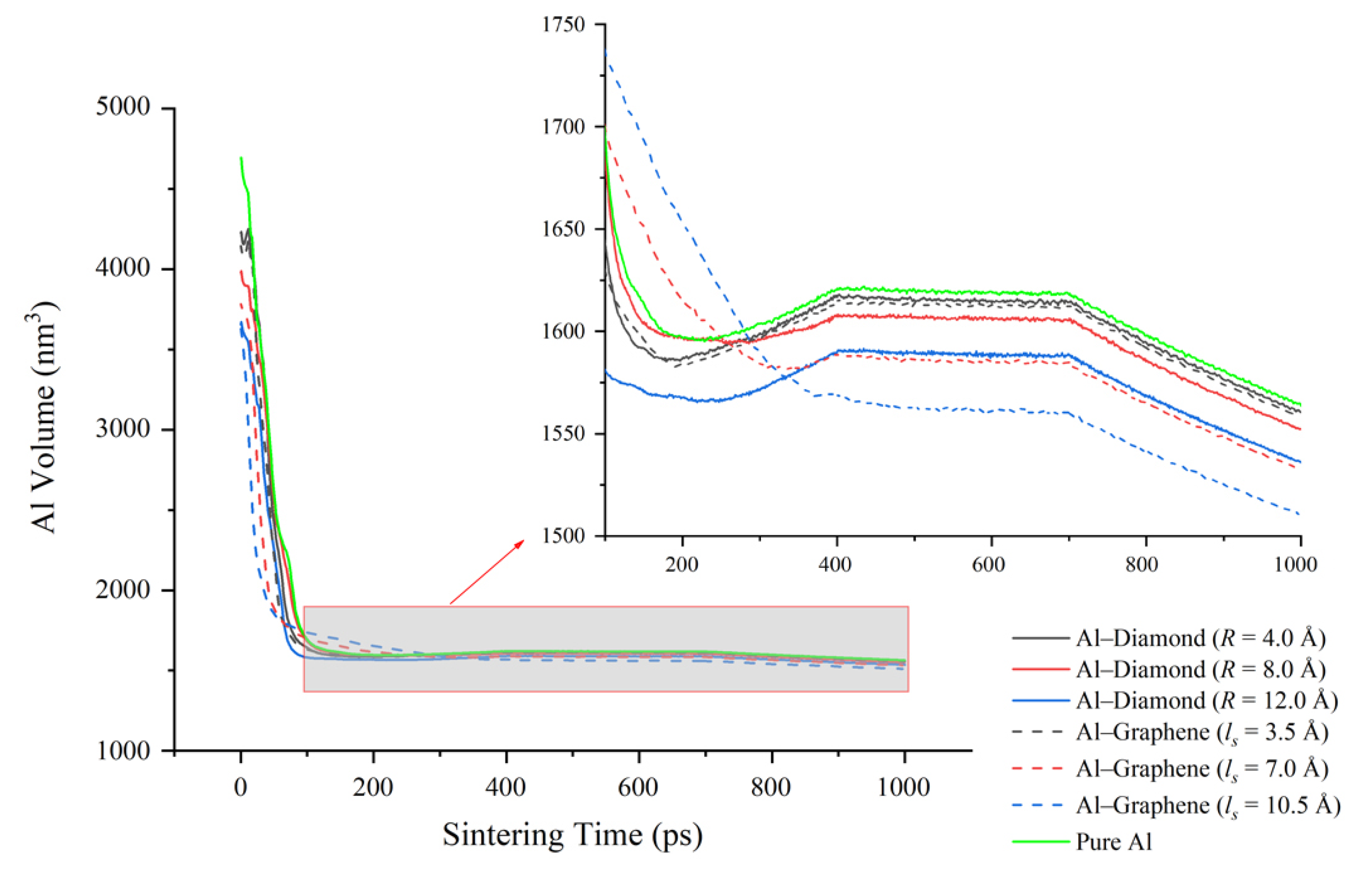
3.1. The Sintering Process
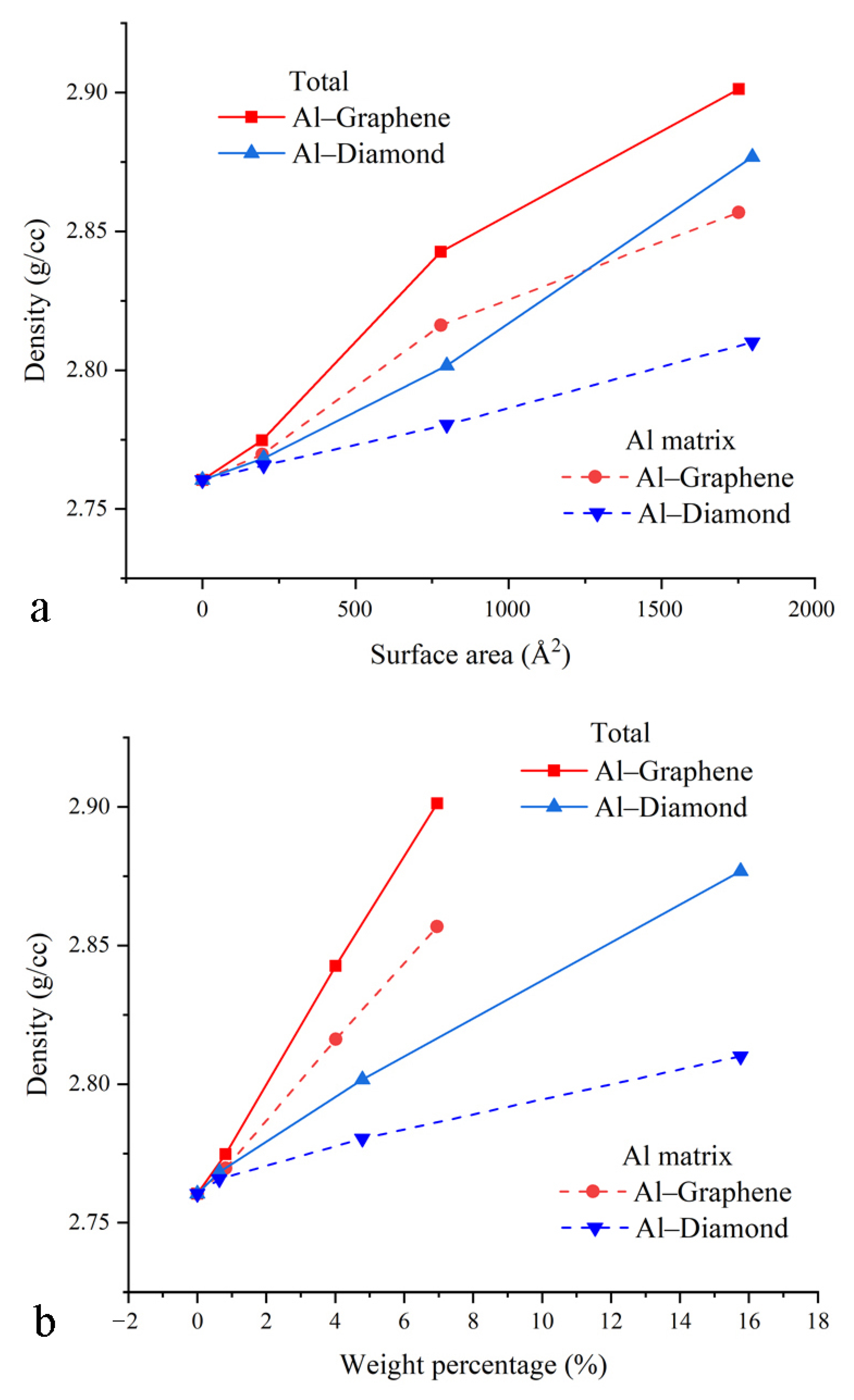
3.2. The Sintered Structure
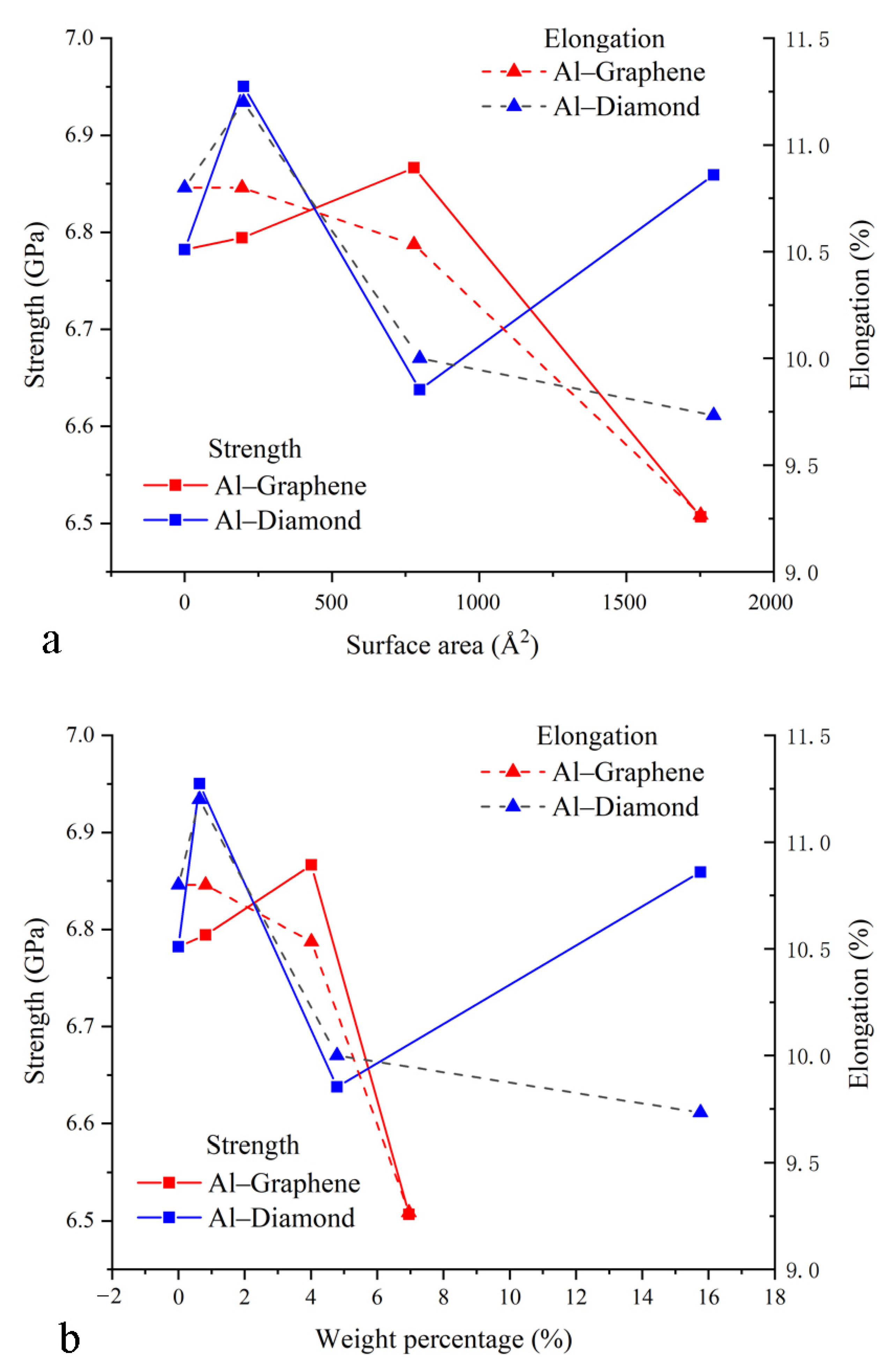
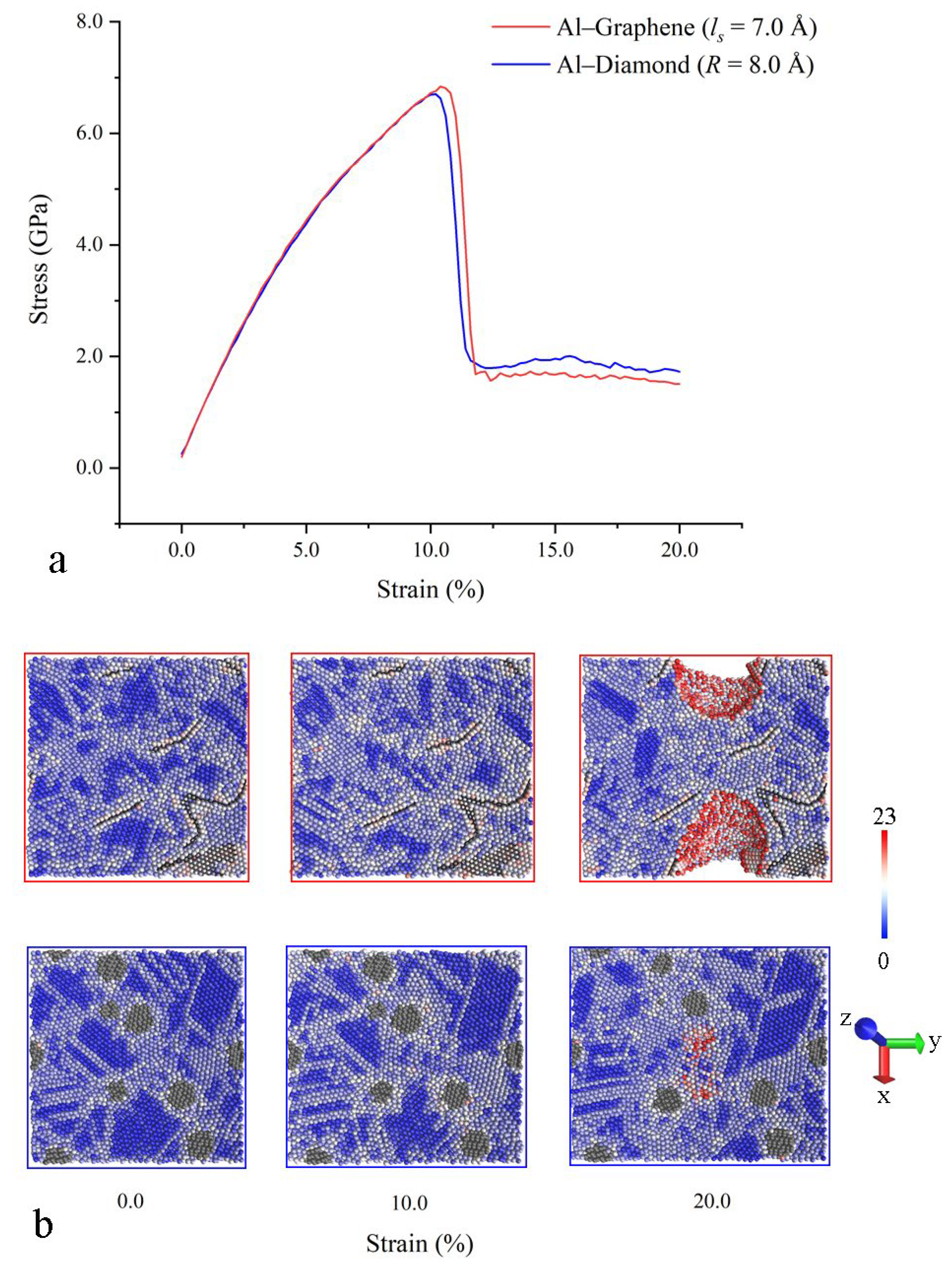
3.3. The Tensile Process
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Tiwari, S.K.; Sahoo, S.; Wang, N.N.; Huczko, A. Graphene research and their outputs: Status and prospect. J. Sci. Adv. Mater. Devices 2020, 5, 10–29. [Google Scholar] [CrossRef]
- Kumar, S.N.; Keshavamurthy, R.; Haseebuddin, M.R.; Koppad, P.G. Mechanical Properties of Aluminium-Graphene Composite Synthesized by Powder Metallurgy and Hot Extrusion. Trans. Indian Inst. Met. 2017, 70, 605–613. [Google Scholar] [CrossRef]
- Kim, Y.; Lee, J.; Yeom, M.S.; Shin, J.W.; Kim, H.; Cui, Y.; Kysar, J.W.; Hone, J.; Jung, Y.; Jeon, S.; et al. Strengthening effect of single-atomic-layer graphene in metal-graphene nanolayered composites. Nat. Commun. 2013, 4, 2114. [Google Scholar] [CrossRef] [PubMed]
- Zhao, S.; Zhang, Y.; Yang, J.; Kitipornchai, S. Folded graphene reinforced nanocomposites with superior strength and toughness: A molecular dynamics study. J. Mater. Sci. Technol. 2022, 120, 196–204. [Google Scholar] [CrossRef]
- Liu, J.; Zhang, Y.; Zhang, H.; Yang, J. Mechanical properties of graphene-reinforced aluminium composite with modified substrate surface: A molecular dynamics study. Nanotechnology 2021, 32, 085712. [Google Scholar] [CrossRef]
- Zhu, J.; Liu, X.; Wang, Z.; Yang, Q. Wrinkles-assisted nanocrystalline formation and mechanical properties of wrinkled graphene/aluminum matrix composites. Model. Simul. Mater. Sci. Eng. 2021, 29, 055017. [Google Scholar] [CrossRef]
- Srivastava, A.K.; Pathak, V.K.; Singh, R.; Dikshit, M.K. Stress-strain behaviour of graphene reinforced aluminum nanocomposite under compressive loading using molecular dynamics. Mater. Today Proc. 2021, 44, 4521–4525. [Google Scholar] [CrossRef]
- Elkady, O.A.; Yehia, H.M.; Ibrahim, A.A.; Elhabak, A.M.; Elsayed, E.M.; Mahdy, A.A. Direct Observation of Induced Graphene and SiC Strengthening in Al–Ni Alloy via the Hot Pressing Technique. Crystals 2021, 11, 1142. [Google Scholar] [CrossRef]
- Lou, S.; Ran, L.; Liu, Y.; Chen, P.; Su, C.; Wang, Q. Effect of Hot Extrusion Ratio on the Mechanical Properties and Microstructure of a 0.5 wt.% Graphene Nanoplatelet-Reinforced Aluminum Matrix Composite. J. Mater. Eng. Perform. 2022, 31, 6533–6544. [Google Scholar] [CrossRef]
- Zeng, M.; Chen, H.; Tao, X.; Ouyang, Y. Mechanical Property and Corrosion Behavior of Powder-Metallurgy-Processed 3D Graphene-Networks-Reinforced Al Matrix Composites. Crystals 2023, 13, 485. [Google Scholar] [CrossRef]
- Zhou, S.; Zhang, W.; Liu, M.; Ren, W.; Yang, Y.; Zhou, Q.; Ma, S.; Shi, L. Microstructure evolution and tensile properties tailoring of graphene nanoplatelets/nickel composites fabricated by two-step 3D vibration milling. J. Alloys Compd. 2022, 918, 165676. [Google Scholar] [CrossRef]
- Yang, W.; Zhao, Q.; Xin, L.; Qiao, J.; Zou, J.; Shao, P.; Yu, Z.; Zhang, Q.; Wu, G. Microstructure and mechanical properties of graphene nanoplates reinforced pure Al matrix composites prepared by pressure infiltration method. J. Alloys Compd. 2018, 732, 748–758. [Google Scholar] [CrossRef]
- Wang, J.; Guo, L.-N.; Lin, W.-M.; Chen, J.; Liu, C.-L.; Zhang, S.; Zhen, T.-T. Effect of the graphene content on the microstructures and properties of graphene/aluminum composites. New Carbon. Mater. 2019, 34, 275–285. [Google Scholar] [CrossRef]
- Wang, Z.-G.; Li, C.-P.; Wang, H.-Y.; Zhu, X.; Wu, M.; Jiang, Q.-C. Effect of nano-SiC content on mechanical properties of SiC/2014Al composites fabricated by powder metallurgy combined with hot extrusion. Powder Metall. 2016, 59, 236–241. [Google Scholar] [CrossRef]
- Kumar, G.B.V.; Panigrahy, P.P.; Nithika, S.; Pramod, R.; Rao, C.S.P. Assessment of mechanical and tribological characteristics of Silicon Nitride reinforced aluminum metal matrix composites. Compos. Part B Eng. 2019, 175, 107138. [Google Scholar] [CrossRef]
- Sharma, P.; Sharma, S.; Khanduja, D. Production and some properties of Si3N4 reinforced aluminium alloy composites. J. Asian Ceram. Soc. 2015, 3, 352–359. [Google Scholar] [CrossRef]
- Zhu, Y.; Li, N.; Li, W.; Niu, L.; Li, Z. Atomistic Study on the Sintering Process and the Strengthening Mechanism of Al-Graphene System. Materials 2022, 15, 2644. [Google Scholar] [CrossRef]
- Sun, H.; Li, N.; Zhu, Y.; Liu, K. A Model for Direct Effect of Graphene on Mechanical Property of Al Matrix Composite. Metals 2023, 13, 1351. [Google Scholar] [CrossRef]
- Dandekar, C.R.; Shin, Y.C. Molecular dynamics based cohesive zone law for describing Al–SiC interface mechanics. Compos. Part A 2011, 42, 355–363. [Google Scholar] [CrossRef]
- Han, R.Q.; Song, H.Y.; An, M.R. Atomic simulation of interaction mechanism between dislocation and graphene in graphene/aluminum composites. Comput. Mater. Sci. 2021, 197, 110604. [Google Scholar] [CrossRef]
- Porwal, H.; Saggar, R.; Tatarko, P.; Grasso, S.; Saunders, T.; Dlouhý, I.; Reece, M.J. Effect of lateral size of graphene nano-sheets on the mechanical properties and machinability of alumina nano-composites. Ceram. Int. 2016, 42, 7533–7542. [Google Scholar] [CrossRef]
- Zhao, M.; Xiong, D.-B.; Tan, Z.; Fan, G.; Guo, Q.; Guo, C.; Li, Z.; Zhang, D. Lateral size effect of graphene on mechanical properties of aluminum matrix nanolaminated composites. Scr. Mater. 2017, 139, 44–48. [Google Scholar] [CrossRef]
- Conrad, H.; Narayan, J. On the grain size softening in nanocrystalline materials. Scr. Mater. 2000, 42, 1025–1030. [Google Scholar] [CrossRef]
- Krajewski, P.E.; Allison, J.E.; Jones, J.W. The influence of matrix microstructure and particle reinforcement on the creep behavior of 2219 aluminum. Metall. Mater. Trans. A 1993, 24, 2731–2741. [Google Scholar] [CrossRef]


| Graphene | Diamond | ||||
|---|---|---|---|---|---|
| Side Length (ls) (Å) | Surface Area (Å2) | Weight Content (%) | Radius (R) (Å) | Surface Area (Å2) | Weight Content (%) |
| 3.5 | 194.6 | 0.6 | 4.0 | 199.6 | 0.8 |
| 7.0 | 778.5 | 4.8 | 8.0 | 798.2 | 4.0 |
| 10.5 | 1751.7 | 15.8 | 12.0 | 1796.0 | 7.0 |
| Sizes of Reinforcements (Å) | Tensile Strength (GPa) | |||
|---|---|---|---|---|
| Along X Axis | Along Y Axis | Along Z Axis | on Average | |
| \ | 6.80 | 6.83 | 6.71 | 6.78 |
| ls = 3.5 | 6.78 | 7.02 | 7.05 | 6.95 |
| ls = 7.0 | 6.53 | 6.70 | 6.69 | 6.64 |
| ls = 10.5 | 6.97 | 6.73 | 6.86 | 6.86 |
| R = 4.0 | 6.79 | 6.88 | 6.72 | 6.79 |
| R = 8.0 | 6.83 | 6.84 | 6.92 | 6.87 |
| R = 12.0 | 6.62 | 6.26 | 6.63 | 6.51 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, Y.; Sui, C.; Li, N.; Sun, L.; Li, S. Large-Scale Atomistic Simulation of Sintering Process and Mechanical Properties of Al Matrix Composite with Different Reinforcements. Metals 2024, 14, 1312. https://doi.org/10.3390/met14111312
Zhu Y, Sui C, Li N, Sun L, Li S. Large-Scale Atomistic Simulation of Sintering Process and Mechanical Properties of Al Matrix Composite with Different Reinforcements. Metals. 2024; 14(11):1312. https://doi.org/10.3390/met14111312
Chicago/Turabian StyleZhu, Yongchao, Can Sui, Na Li, Lijuan Sun, and Songtao Li. 2024. "Large-Scale Atomistic Simulation of Sintering Process and Mechanical Properties of Al Matrix Composite with Different Reinforcements" Metals 14, no. 11: 1312. https://doi.org/10.3390/met14111312
APA StyleZhu, Y., Sui, C., Li, N., Sun, L., & Li, S. (2024). Large-Scale Atomistic Simulation of Sintering Process and Mechanical Properties of Al Matrix Composite with Different Reinforcements. Metals, 14(11), 1312. https://doi.org/10.3390/met14111312






