The Effect of Microstructure on the Very High Cycle Fatigue Behavior of Ti-6Al-4V Alloy
Abstract
1. Introduction
2. Experimental Details
2.1. Material
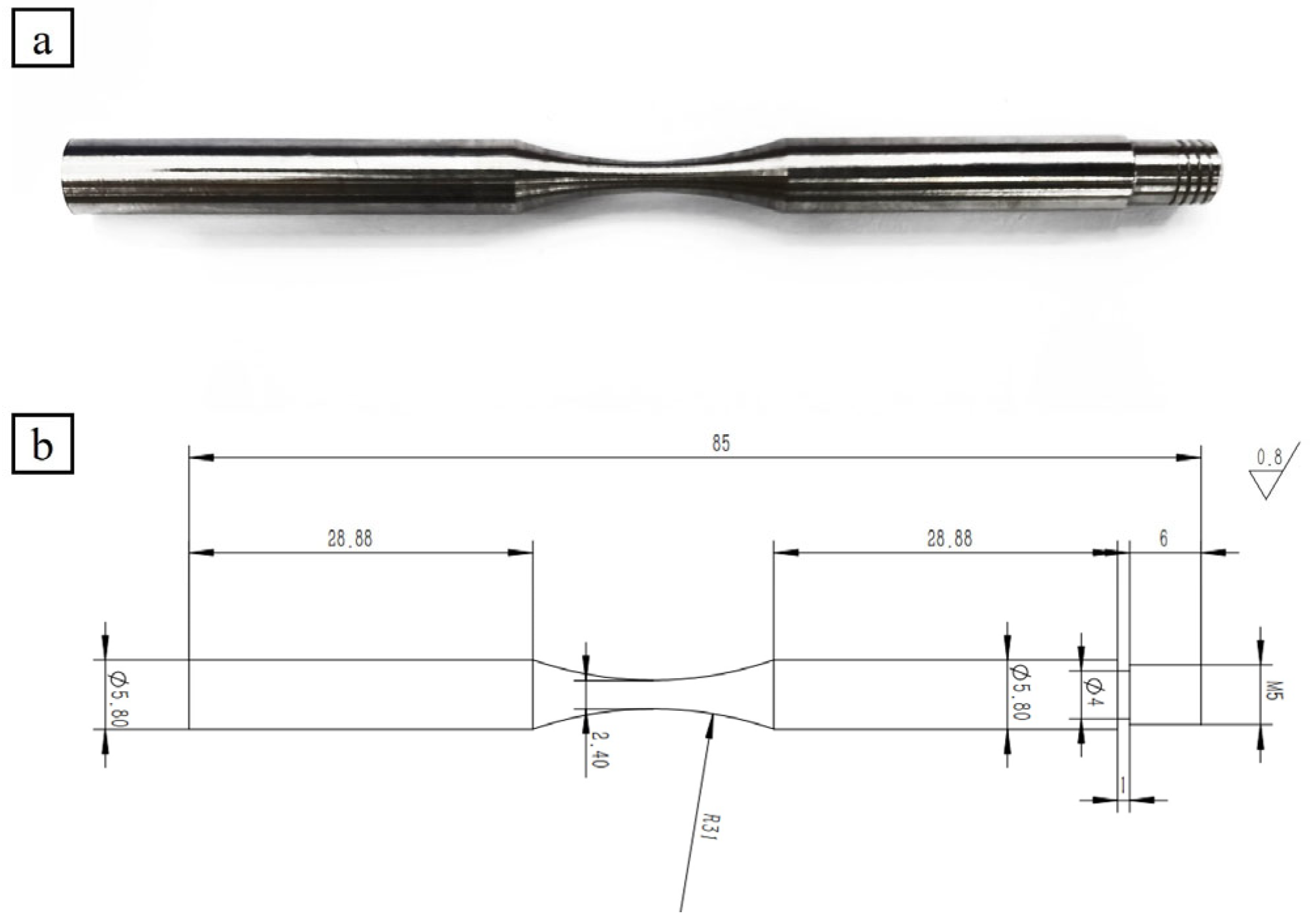
2.2. Fatigue Testing Procedure
2.3. Characterization Methods
3. Results
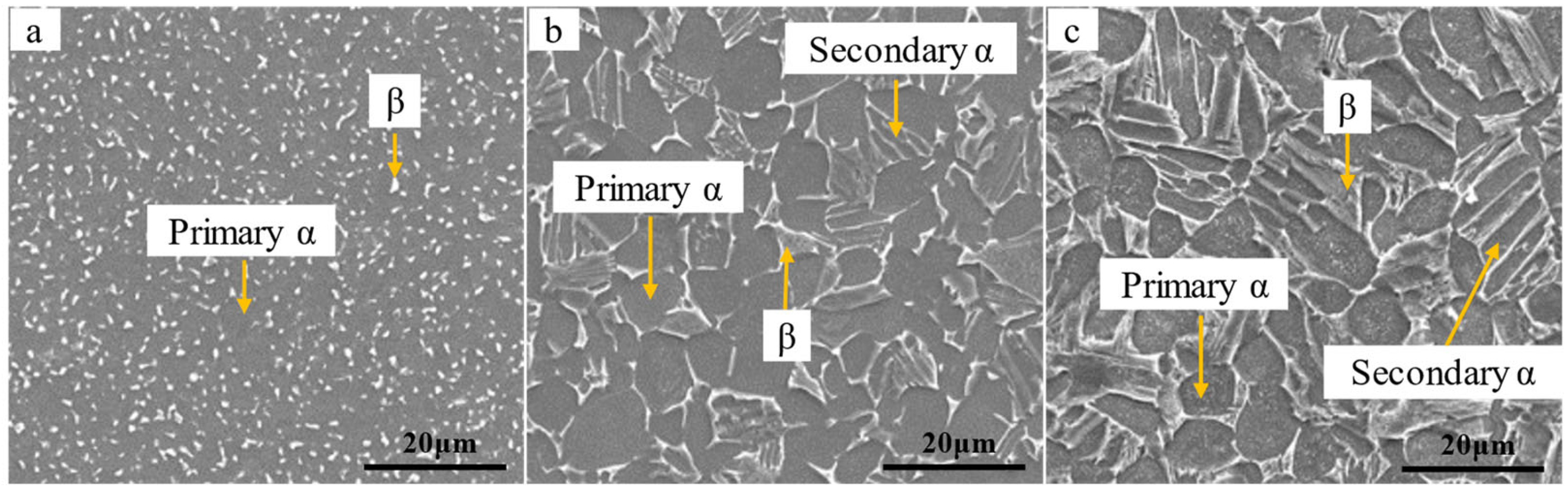
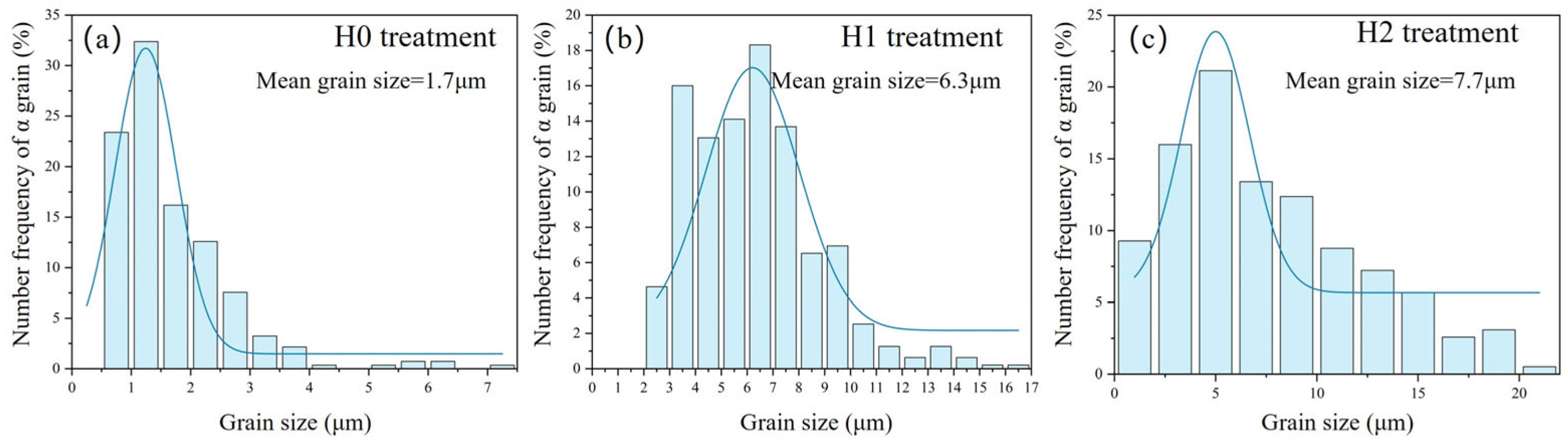
3.1. Microstructure Transformation
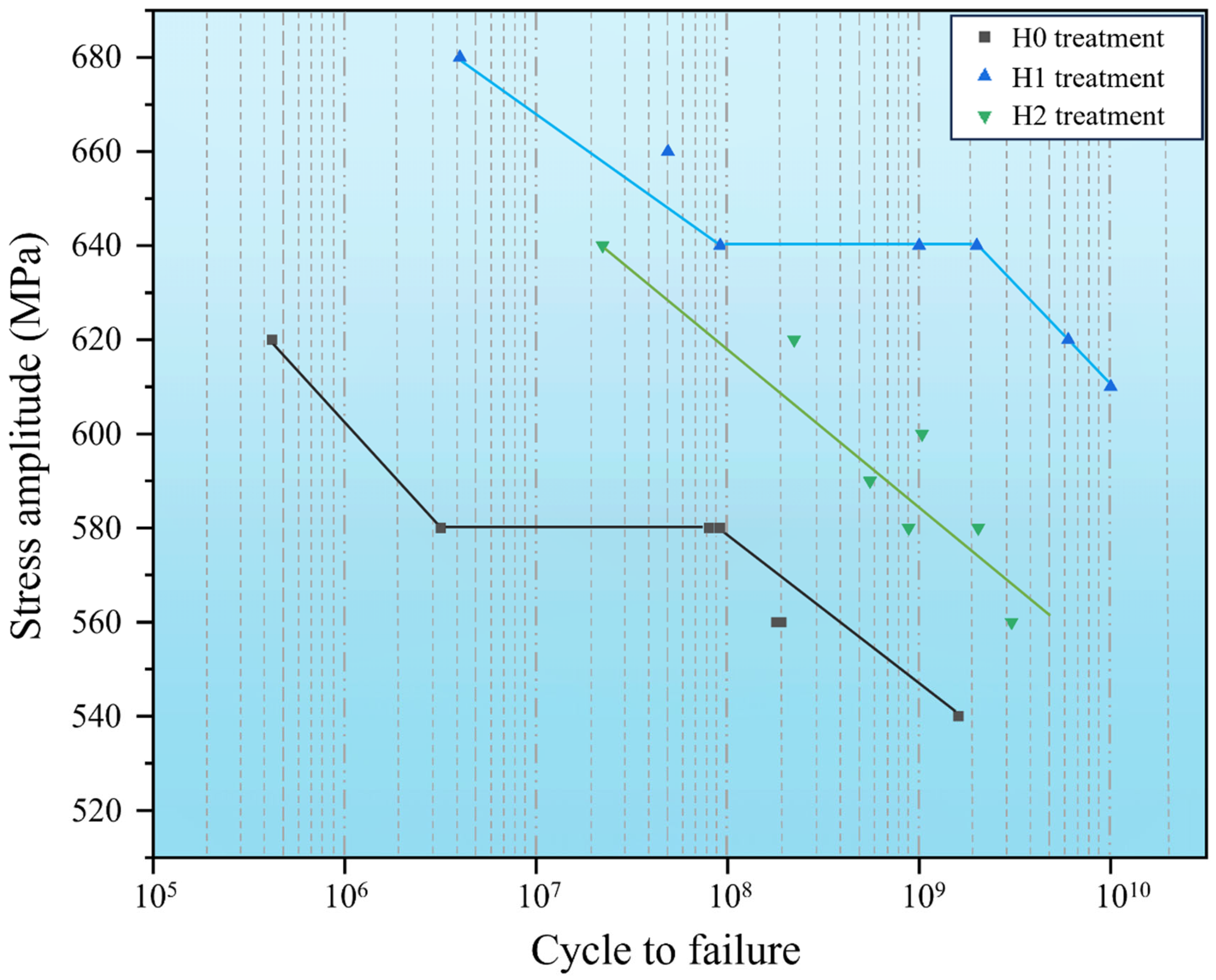
3.2. Fatigue Test Results
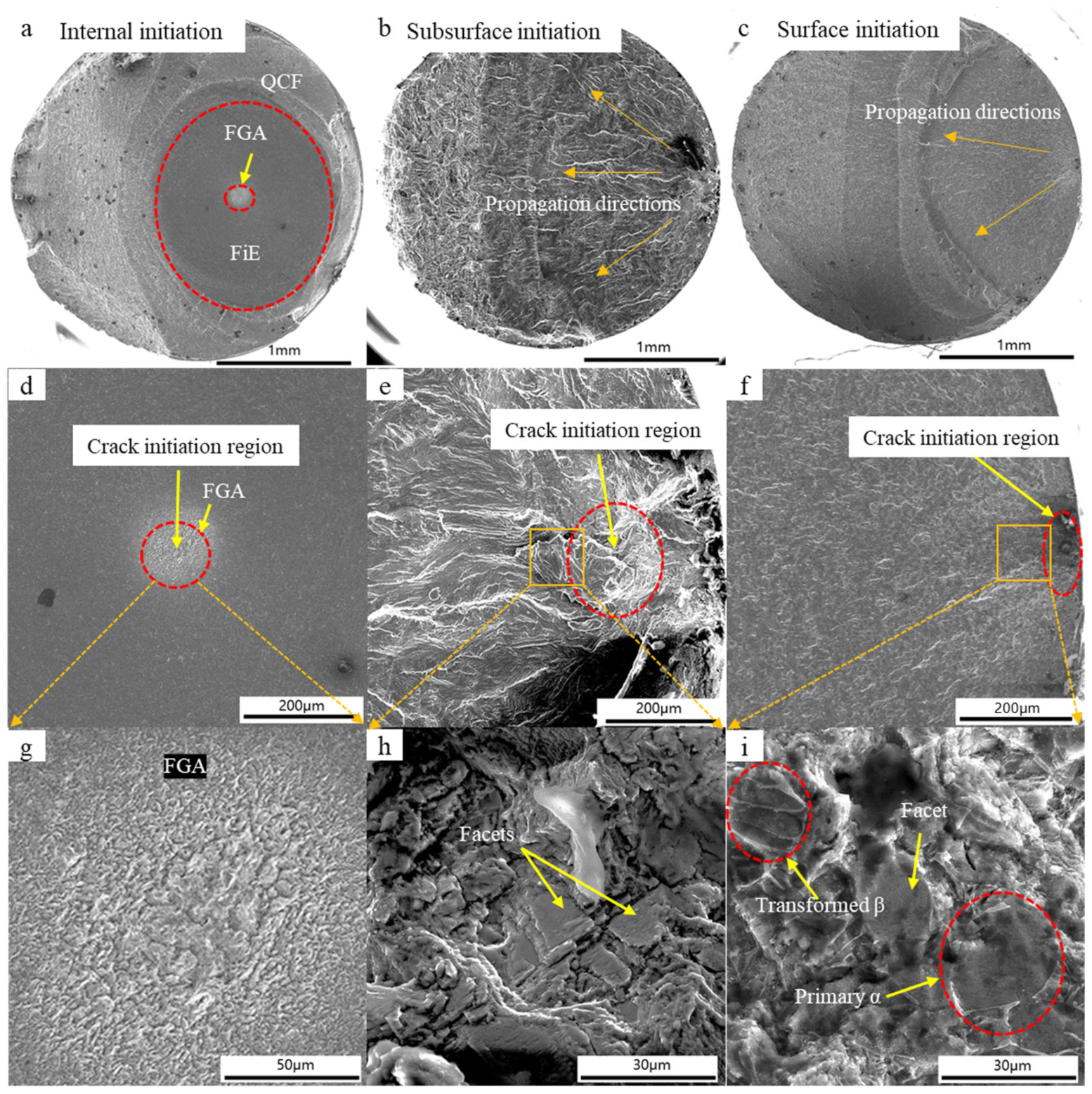
3.3. Fracture Morphology
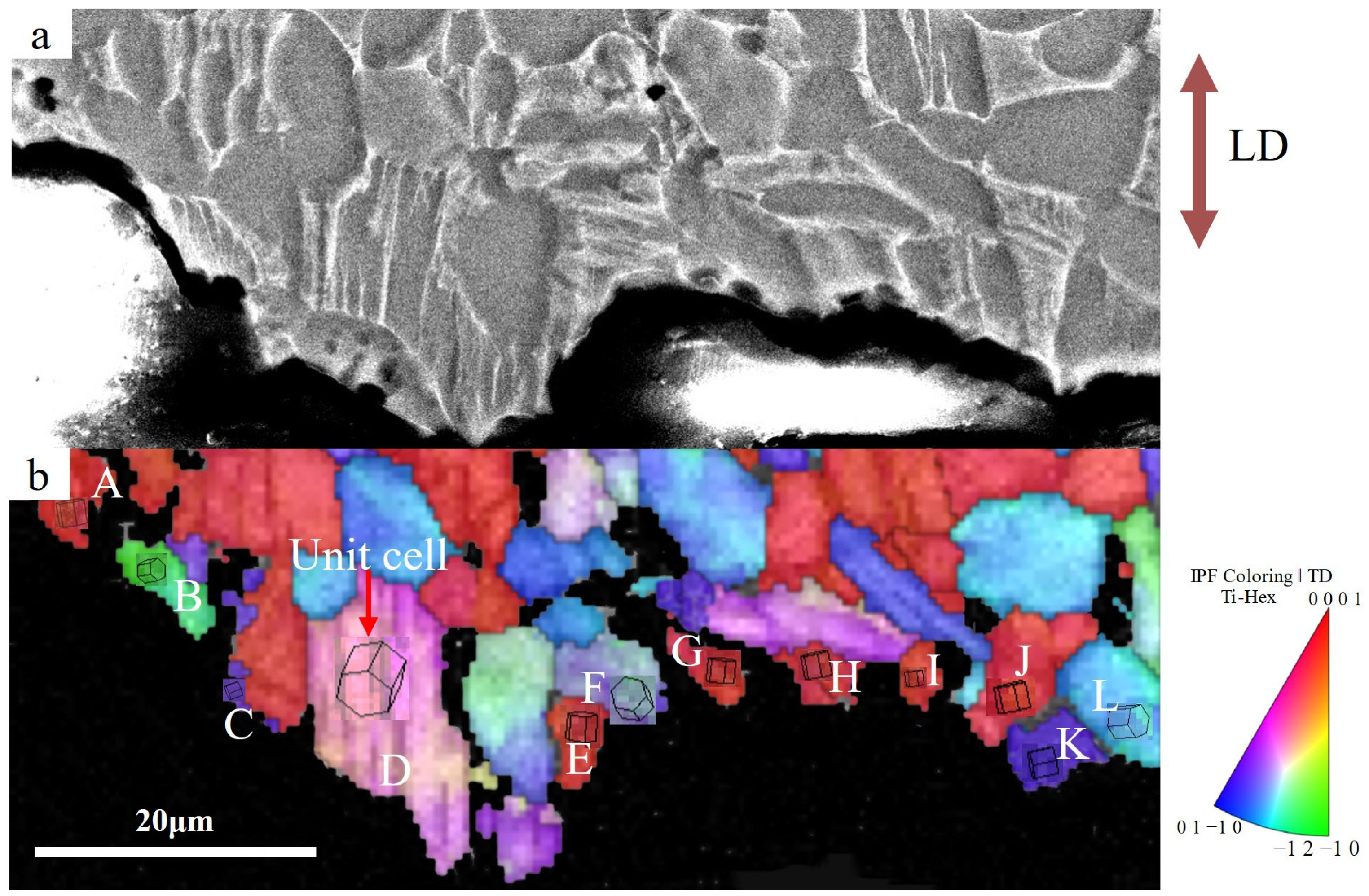
3.4. Microtexture Evolution
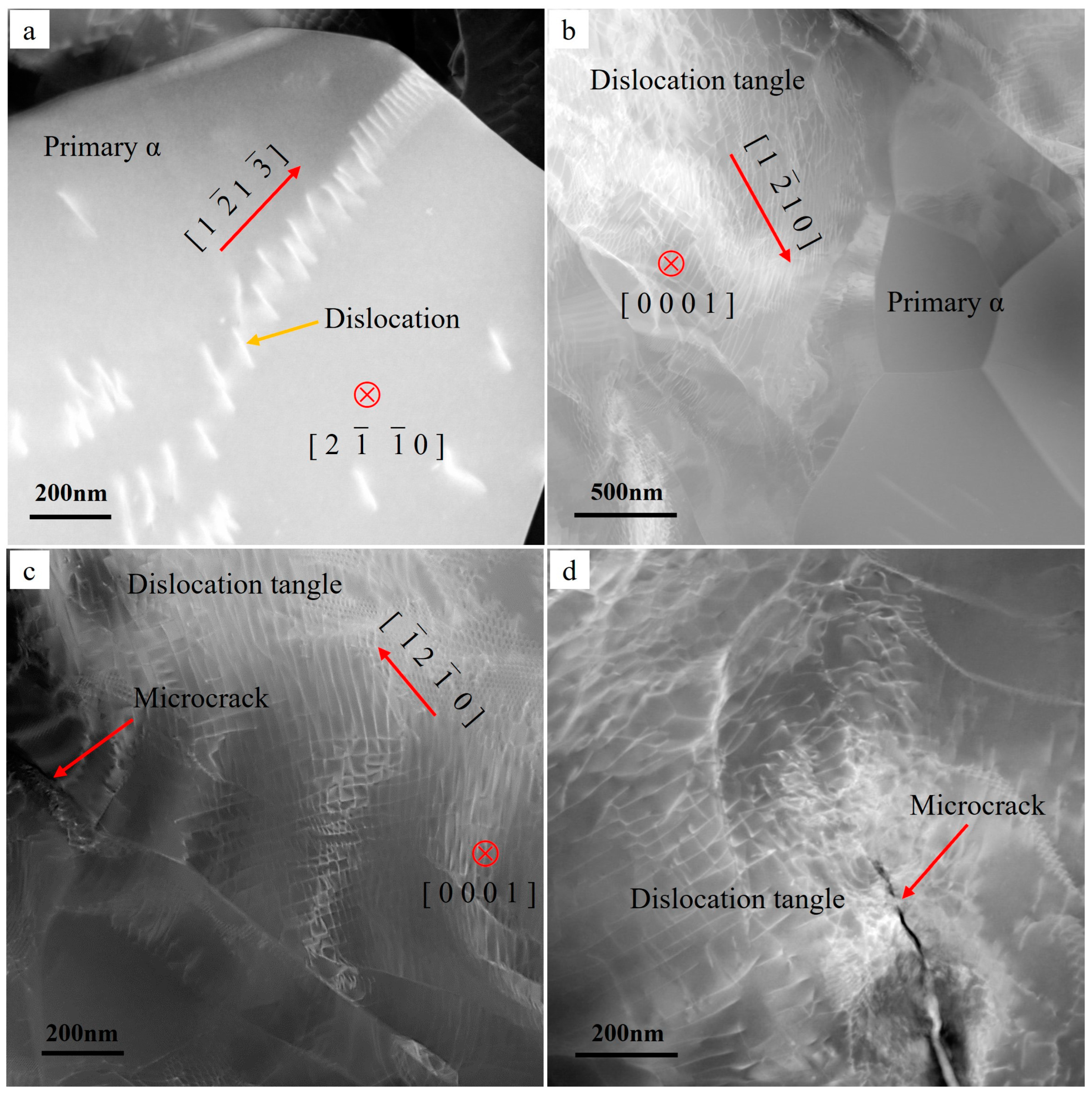
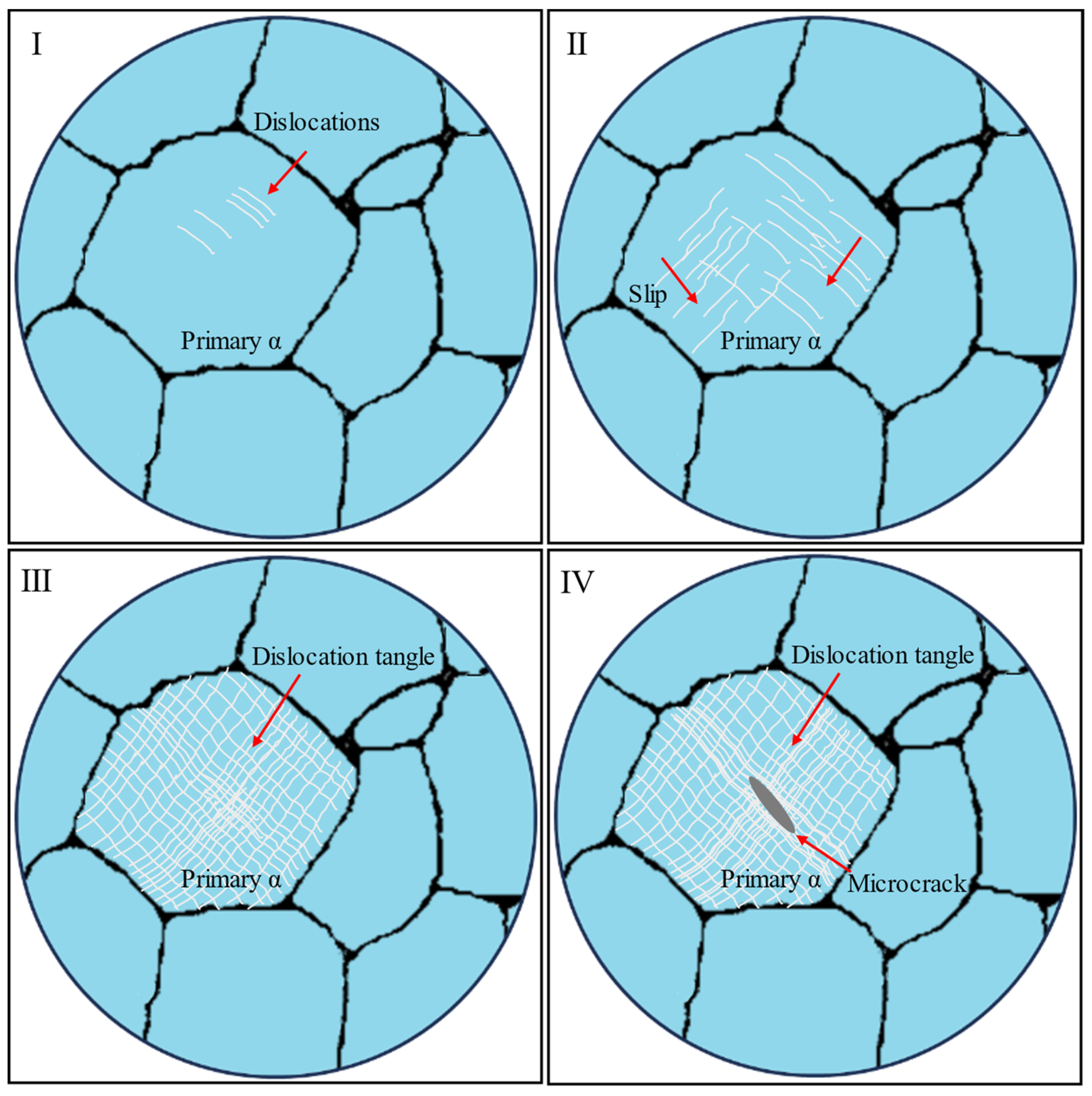
3.5. Fracture and Microcracks
4. Discussion
4.1. Microstructure Changes after Heat Treatment
4.2. Factors Affecting the Fatigue Performance of VHCF
4.2.1. Sphericity and Grain Size Uniformity
4.2.2. Impact of Microstructure and Microtexture on VHCF Performance
5. Conclusions
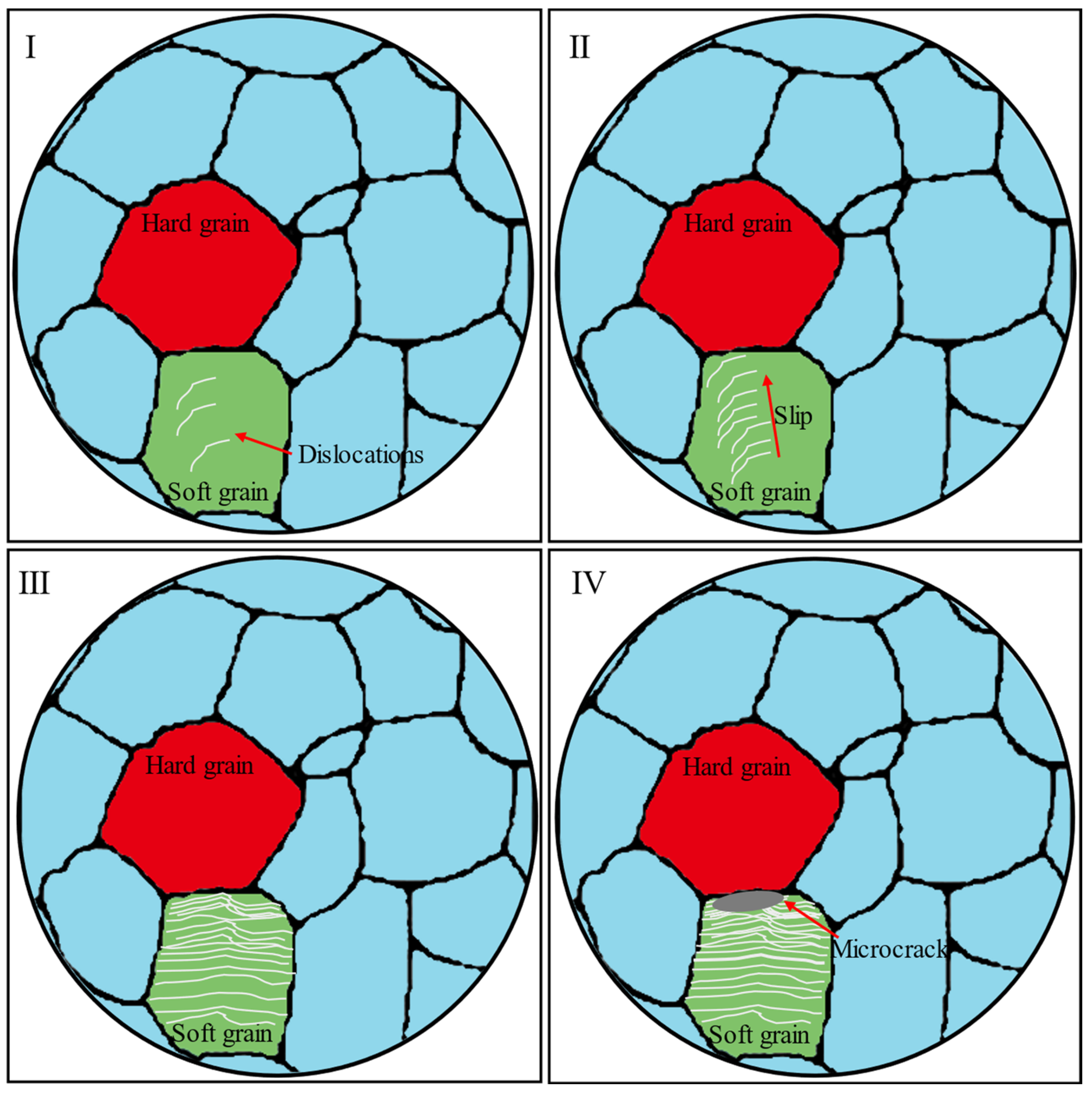
- The crack initiation modes in equiaxed and bimodal microstructures of Ti-6Al-4V are different, and are related to the distinct microtextures of the two. In the equiaxed microstructure, slip and multiplication of dislocations within large grains are more prone to occur, leading to the initiation of microcracks under stress loading. In the bimodal microstructure, grains oriented {0 0 0 1}||RD form rogue grain combinations with grains oriented {1 0 −1 0}||RD, causing the accumulation of dislocations and the formation of microcracks on the soft grain side.
- The VHCF performance of the bimodal microstructure is superior to that of the equiaxed microstructure. Bimodal microstructure samples obtained through heat treatments at 970 °C × 60 min, FAC + 730 °C × 75 min, AC exhibit an 18% improvement in fatigue strength at 109 cycles compared to the original equiaxed microstructure samples.
- According to the test results, in the bimodal microstructure, an increase in grain size and the presence of rogue grain combinations lead to a deterioration in VHCF performance. Therefore, obtaining a bimodal microstructure while simultaneously controlling grain size and microtexture is a viable approach to enhance VHCF performance.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Demulsant, X.; Mendez, J. Influence of Environment on Low Cycle Fatigue Damage in Ti6Al4V and Ti 6246 Titanium Alloys. Mater. Sci. Eng. A 1996, 219, 202–211. [Google Scholar] [CrossRef]
- Chang, L.; Lv, C.; Kitamura, T.; Zhang, W.; Zhou, C.-Y. Slip Dominated Planar Anisotropy of Low Cycle Fatigue Behavior of Commercially Pure Titanium. Mater. Sci. Eng. A 2022, 854, 143807. [Google Scholar] [CrossRef]
- Sinha, S.; Gurao, N.P. The Role of Crystallographic Texture on Load Reversal and Low Cycle Fatigue Performance of Commercially Pure Titanium. Mater. Sci. Eng. A 2017, 691, 100–109. [Google Scholar] [CrossRef]
- Li, G.; Ke, L.; Ren, X.; Sun, C. High Cycle and Very High Cycle Fatigue of TC17 Titanium Alloy: Stress Ratio Effect and Fatigue Strength Modeling. Int. J. Fatigue 2023, 166, 107299. [Google Scholar] [CrossRef]
- Wu, H.; Sun, C.; Xu, W.; Chen, X.; Wu, X. A Novel Evaluation Method for High Cycle and Very High Cycle Fatigue Strength. Eng. Fract. Mech. 2023, 290, 109482. [Google Scholar] [CrossRef]
- Kazymyrovych, V. Very High Cycle Fatigue of Engineering Materials: A Literature Review; Karlstad University: Karlstad, Sweden, 2009. [Google Scholar]
- Hong, Y.; Lei, Z.; Sun, C.; Zhao, A. Propensities of Crack Interior Initiation and Early Growth for Very-High-Cycle Fatigue of High Strength Steels. Int. J. Fatigue 2014, 58, 144–151. [Google Scholar] [CrossRef]
- Sharma, A.; Oh, M.C.; Ahn, B. Recent Advances in Very High Cycle Fatigue Behavior of Metals and Alloys—A Review. Metals 2020, 10, 1200. [Google Scholar] [CrossRef]
- Cui, W.; Chen, X.; Cheng, L.; Ding, J.; Wang, C.; Wang, B. Fatigue Property and Failure Mechanism of TC4 Titanium Alloy in the HCF and VHCF Region Considering Different Forging Processes. Mater. Res. Express 2021, 8, 046524. [Google Scholar] [CrossRef]
- Meier, M.L.; Lesuer, D.R.; Mukherjee, A.K. α Grain Size and β Volume Fraction Aspects of the Superplasticity of Ti-6Al-4V. Mater. Sci. Eng. A 1991, 136, 71–78. [Google Scholar] [CrossRef]
- Sajadifar, S.V.; Wegener, T.; Yapici, G.G.; Niendorf, T. Effect of Grain Size on the Very High Cycle Fatigue Behavior and Notch Sensitivity of Titanium. Theor. Appl. Fract. Mech. 2019, 104, 102362. [Google Scholar] [CrossRef]
- Everaerts, J.; Verlinden, B.; Wevers, M. The Influence of the Alpha Grain Size on Internal Fatigue Crack Initiation in Drawn Ti-6Al-4V Wires. Procedia Struct. Integr. 2016, 2, 1055–1062. [Google Scholar] [CrossRef]
- Ueki, S.; Mine, Y.; Chiu, Y.-L.; Bowen, P.; Takashima, K. Effects of Crystallographic Orientation and Lamellar Configuration on Fatigue Crack Propagation in Single-Colony Structures of Ti-6Al-4V Alloy: Alternating Shear Crack Growth vs. Damage Accumulation Crack Propagation. Mater. Sci. Eng. A 2024, 890, 145885. [Google Scholar] [CrossRef]
- Xu, S.; Zhang, H.; Xiao, N.; Qiu, R.; Cui, Z.; Fu, M. Mechanisms of Macrozone Elimination and Grain Refinement of near α Ti Alloy via the Spheroidization of the Widmannstätten Structure. Acta Mater. 2023, 260, 119339. [Google Scholar] [CrossRef]
- Lavogiez, C.; Hémery, S.; Villechaise, P. On the Mechanism of Fatigue and Dwell-Fatigue Crack Initiation in Ti-6Al-4V. Scr. Mater. 2020, 183, 117–121. [Google Scholar] [CrossRef]
- Bantounas, I.; Dye, D.; Lindley, T.C. The Role of Microtexture on the Faceted Fracture Morphology in Ti-6Al-4V Subjected to High-Cycle Fatigue. Acta Mater. 2010, 58, 3908–3918. [Google Scholar] [CrossRef]
- Bantounas, I.; Dye, D.; Lindley, T.C. The Effect of Grain Orientation on Fracture Morphology during High-Cycle Fatigue of Ti–6Al–4V. Acta Mater. 2009, 57, 3584–3595. [Google Scholar] [CrossRef]
- Bridier, F.; Villechaise, P.; Mendez, J. Slip and Fatigue Crack Formation Processes in an α/β Titanium Alloy in Relation to Crystallographic Texture on Different Scales. Acta Mater. 2008, 56, 3951–3962. [Google Scholar] [CrossRef]
- Briffod, F.; Bleuset, A.; Shiraiwa, T.; Enoki, M. Effect of Crystallographic Orientation and Geometrical Compatibility on Fatigue Crack Initiation and Propagation in Rolled Ti-6Al-4V Alloy. Acta Mater. 2019, 177, 56–67. [Google Scholar] [CrossRef]
- Pan, X.; Su, H.; Sun, C.; Hong, Y. The Behavior of Crack Initiation and Early Growth in High-Cycle and Very-High-Cycle Fatigue Regimes for a Titanium Alloy. Int. J. Fatigue 2018, 115, 67–78. [Google Scholar] [CrossRef]
- Morrissey, R.J.; Nicholas, T. Fatigue Strength of Ti-6Al-4V at Very Long Lives. Int. J. Fatigue 2005, 27, 1608–1612. [Google Scholar] [CrossRef]
- Heinz, S.; Eifler, D. Crack Initiation Mechanisms of Ti6Al4V in the Very High Cycle Fatigue Regime. Int. J. Fatigue 2016, 93, 301–308. [Google Scholar] [CrossRef]
- Gao, T.; Xue, H.; Sun, Z.; Retraint, D. Investigation of Crack Initiation Mechanism of a Precipitation Hardened TC11 Titanium Alloy under Very High Cycle Fatigue Loading. Mater. Sci. Eng. A 2020, 776, 138989. [Google Scholar] [CrossRef]
- Smaga, M.; Boemke, A.; Eifler, D.; Beck, T. Very High Cycle Fatigue Behavior of Austenitic Stainless Steels with Different Surface Morphologies. Metals 2022, 12, 1877. [Google Scholar] [CrossRef]
- Dunne, F.P.E.; Rugg, D.; Walker, A. Lengthscale-Dependent, Elastically Anisotropic, Physically-Based Hcp Crystal Plasticity: Application to Cold-Dwell Fatigue in Ti Alloys. Int. J. Plast. 2007, 23, 1061–1083. [Google Scholar] [CrossRef]
- Lavogiez, C.; Hémery, S.; Villechaise, P. Analysis of Deformation Mechanisms Operating under Fatigue and Dwell-Fatigue Loadings in an α/β Titanium Alloy. Int. J. Fatigue 2020, 131, 105341. [Google Scholar] [CrossRef]
- Zhao, Z.B.; Zhang, B.H.; Sun, H.; Wang, Q.J.; Liu, J.R.; Yang, R. Influence of Globularization Process on Local Texture Evolution of a Near-α Titanium Alloy with a Transformed Microstructure. Metall. Mater. Trans. A 2023, 54, 2849–2857. [Google Scholar] [CrossRef]
- Zhao, Z.B.; Zhang, B.H.; Wang, Q.J.; Sun, H.; Yang, J.X.; Liu, Y.J.; Liu, J.R.; Yang, R. Microtexture Evolution of Titanium Alloy During Hot Deformation: For Better Understanding the Role of Primary α Grains. Metall. Mater. Trans. A 2023, 54, 2890–2902. [Google Scholar] [CrossRef]
- Li, C.; Li, W.; Cai, L.; Zhang, Y.; Sun, R.; Li, X.; Imran Lashari, M.; Hamid, U.; Ding, X.; Wang, P. Microstructure Based Cracking Behavior and Life Assessment of Titanium Alloy under Very-High-Cycle Fatigue with Elevated Temperatures. Int. J. Fatigue 2022, 161, 106914. [Google Scholar] [CrossRef]
- Venkatramani, G.; Ghosh, S.; Mills, M. A Size-Dependent Crystal Plasticity Finite-Element Model for Creep and Load Shedding in Polycrystalline Titanium Alloys. Acta Mater. 2007, 55, 3971–3986. [Google Scholar] [CrossRef]
- Whittaker, M.T.; Evans, W.J.; Lancaster, R.; Harrison, W.; Webster, P.S. The Effect of Microstructure and Texture on Mechanical Properties of Ti6-4. Int. J. Fatigue 2009, 31, 2022–2030. [Google Scholar] [CrossRef]
- Echlin, M.P.; Stinville, J.C.; Miller, V.M.; Lenthe, W.C.; Pollock, T.M. Incipient Slip and Long Range Plastic Strain Localization in Microtextured Ti-6Al-4V Titanium. Acta Mater. 2016, 114, 164–175. [Google Scholar] [CrossRef]
- Gao, T.; Xue, H.; Sun, Z. Effect of Transformed β Phase on Fish-Eye Ductile Crack Initiation of a Ti-6Al-4V Alloy in Very High Cycle Fatigue Regime. Mater. Lett. 2021, 287, 129283. [Google Scholar] [CrossRef]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yuan, M.; Zhao, X.; Yue, Q.; Gu, Y.; Zhang, Z. The Effect of Microstructure on the Very High Cycle Fatigue Behavior of Ti-6Al-4V Alloy. Metals 2024, 14, 254. https://doi.org/10.3390/met14030254
Yuan M, Zhao X, Yue Q, Gu Y, Zhang Z. The Effect of Microstructure on the Very High Cycle Fatigue Behavior of Ti-6Al-4V Alloy. Metals. 2024; 14(3):254. https://doi.org/10.3390/met14030254
Chicago/Turabian StyleYuan, Mingyang, Xinbao Zhao, Quanzhao Yue, Yuefeng Gu, and Ze Zhang. 2024. "The Effect of Microstructure on the Very High Cycle Fatigue Behavior of Ti-6Al-4V Alloy" Metals 14, no. 3: 254. https://doi.org/10.3390/met14030254
APA StyleYuan, M., Zhao, X., Yue, Q., Gu, Y., & Zhang, Z. (2024). The Effect of Microstructure on the Very High Cycle Fatigue Behavior of Ti-6Al-4V Alloy. Metals, 14(3), 254. https://doi.org/10.3390/met14030254





