Fatigue Strength Assessment of Welded Mild Steel Joints Containing Bulk Imperfections
Abstract
:1. Introduction
- Manufacturing of different mild steel butt joints exhibiting gas pores as representative imperfections with different sizes, shapes, and locations.
- Characterization of local properties, fatigue testing, and fracture surface analysis to investigate the relationship between material condition, defect size, and strength.
- Application of different fatigue models to evaluate the practicability of these concepts in order to assess the fatigue strength of imperfected mild steel weld joints.
2. Materials and Methods
2.1. Materials and Test Series
2.2. Fatigue Assessment Methods
3. Results
3.1. Evaluation of Local Properties and Fatigue Behaviour
3.1.1. Hardness and Residual Stress Condition
3.1.2. Fatigue Test Results
3.1.3. Fracture Surface Analysis
3.2. Fatigue Strength Assessment by Analytical Models
3.3. Fatigue Strength Assessment by Analytical/Numerical Approach
4. Discussion
5. Conclusions
- Internal imperfections, such as gas pores, significantly affect the fatigue strength of welded mild steel joints and should be considered within a fatigue assessment.
- The √area approach by Murakami [33] is well applicable to assess the mean strength of both micro and macroscopic gas pores depending on the applied slope value m. Within this study, it is presented that the recommended slope of 1/6 with m = 3 fits well to the defects with √area < 1000 µm, and m = 4 is well applicable for √area > 1000 µm.
- This work highlights that to properly assess the fatigue strength of welded mild steel joints containing internal imperfections, it is essential to incorporate fatigue-relevant local properties, such as the hardness and effective residual stress state.
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Hou, C.-Y. Fatigue analysis of welded joints with the aid of real three-dimensional weld toe geometry. Int. J. Fatigue 2007, 29, 772–785. [Google Scholar] [CrossRef]
- Fricke, W. IIW guideline for the assessment of weld root fatigue. Weld. World 2013, 57, 753–791. [Google Scholar] [CrossRef]
- Sonsino, C.M. Effect of residual stresses on the fatigue behaviour of welded joints depending on loading conditions and weld geometry. Int. J. Fatigue 2009, 31, 88–101. [Google Scholar] [CrossRef]
- James, M.N.; Webster, P.J.; Hughes, D.J.; Chen, Z.; Ratel, N.; Ting, S.-P.; Bruni, G.; Steuwer, A. Correlating weld process conditions, residual strain and stress, microstructure and mechanical properties for high strength steel—The role of neutron diffraction strain scanning. Mater. Sci. Eng. A 2006, 427, 16–26. [Google Scholar] [CrossRef]
- James, M.N.; Ting, S.-P.; Bosi, M.; Lombard, H.; Hattingh, D.G. Residual strain and hardness as predictors of the fatigue ranking of steel welds. Int. J. Fatigue 2009, 31, 1366–1377. [Google Scholar] [CrossRef]
- Hobbacher, A. Recommendations for Fatigue Design of Welded Joints and Components; IIW Collection; Springer: Cham, Switzerland, 2016. [Google Scholar]
- Radaj, D.; Sonsino, C.M.; Fricke, W. Recent developments in local concepts of fatigue assessment of welded joints. Int. J. Fatigue 2009, 31, 2–11. [Google Scholar] [CrossRef]
- Stoschka, M.; Leitner, M.; Posch, G.; Eichlseder, W. Effect of high-strength filler metals on the fatigue behavior of butt joints. Weld. World 2013, 57, 85–96. [Google Scholar] [CrossRef]
- Stoschka, M.; Leitner, M.; Fössl, T.; Leitner, H.; Eichlseder, W. Application of fatigue approaches on fillet welds of high strength steel. Mater. Sci. Eng. Technol. 2010, 41, 961–971. [Google Scholar] [CrossRef]
- Barsoum, Z.; Jonsson, B. Influence of weld quality on the fatigue strength in seam welds. Eng. Fail. Anal. 2011, 18, 971–979. [Google Scholar] [CrossRef]
- Lillemäe, I.; Remes, H.; Liinalampi, S.; Itävuo, A. Influence of weld quality on the fatigue strength of thin normal and high strength steel butt joints. Weld. World 2016, 60, 731–740. [Google Scholar] [CrossRef]
- Ottersböck, M.; Leitner, M.; Stoschka, M.; Maurer, W. Effect of weld defects on the fatigue strength of ultra high-strength steels. Procedia Eng. 2016, 160, 214–222. [Google Scholar] [CrossRef]
- Kirkhope, K.J.; Bell, R.; Caron, L.; Basu, R.I.; Ma, K.-T. Weld detail fatigue life improvement techniques. Part 1: Review. Mar. Struct. 1999, 12, 447–474. [Google Scholar] [CrossRef]
- Leitner, M.; Stoschka, M.; Eichlseder, W. Fatigue enhancement of thin-walled, high-strength steel joints by high-frequency mechanical impact treatment. Weld. World 2014, 58, 29–39. [Google Scholar] [CrossRef]
- Leitner, M.; Stoschka, M.; Schanner, R.; Eichlseder, W. Influence of High Frequency Peening on Fatigue of High-Strength Steels. FME Trans. 2012, 40, 99–104. [Google Scholar]
- Leitner, M.; Barsoum, Z.; Schäfers, F. Crack propagation analysis and rehabilitation by HFMI of pre-fatigued welded structures. Weld. World 2016, 60, 581–592. [Google Scholar] [CrossRef]
- Leitner, M.; Mössler, W.; Putz, A.; Stoschka, M. Effect of post-weld heat treatment on the fatigue strength of HFMI-treated mild steel joints. Weld. World 2015, 59, 861–873. [Google Scholar] [CrossRef]
- Jonsson, B.; Samuelsson, J.; Marquis, G.B. Development of Weld Quality Criteria Based on Fatigue Performance. Weld. World 2011, 55, 79–88. [Google Scholar] [CrossRef]
- Jonsson, B.; Dobmann, G.; Hobbacher, A.; Kassner, M.; Marquis, G.B. IIW Guidelines on Weld Quality in Relationship to Fatigue Strength; IIW Collection; Springer: Cham, Switzerland, 2016. [Google Scholar]
- Zhu, M.-L.; Xuan, F.-Z.; Du, Y.-N.; Tu, S.-T. Very high cycle fatigue behavior of a low strength welded joint at moderate temperature. Int. J. Fatigue 2012, 40, 74–83. [Google Scholar] [CrossRef]
- Yeom, H.; Choi, B.; Seol, T.; Lee, M.; Jeon, Y. Very High Cycle Fatigue of Butt-Welded High-Strength Steel Plate. Metals 2017, 7, 103. [Google Scholar] [CrossRef]
- Chan, K.S. Roles of microstructure in fatigue crack initiation. Int. J. Fatigue 2010, 32, 1428–1447. [Google Scholar] [CrossRef]
- Sangid, M.D. The physics of fatigue crack initiation. Int. J. Fatigue 2013, 57, 58–72. [Google Scholar] [CrossRef]
- Bayraktar, E.; Garcias, I.M.; Bathias, C. Failure mechanisms of automotive metallic alloys in very high cycle fatigue range. Int. J. Fatigue 2006, 28, 1590–1602. [Google Scholar] [CrossRef]
- Shen, F.; Zhao, B.; Li, L.; Chua, C.K.; Zhou, K. Fatigue damage evolution and lifetime prediction of welded joints with the consideration of residual stresses and porosity. Int. J. Fatigue 2017, 103, 272–279. [Google Scholar] [CrossRef]
- Gou, G.; Zhang, M.; Chen, H.; Chen, J.; Li, P.; Yang, Y.P. Effect of humidity on porosity, microstructure, and fatigue strength of A7N01S-T5 aluminum alloy welded joints in high-speed trains. Mater. Des. 2015, 85, 309–317. [Google Scholar] [CrossRef]
- Tsay, L.W.; Shan, Y.-P.; Chao, Y.-H.; Shu, W.Y. The influence of porosity on the fatigue crack growth behavior of Ti–6Al–4V laser welds. J. Mater. Sci. 2006, 41, 7498–7505. [Google Scholar] [CrossRef]
- Leitner, M.; Murakami, Y.; Remes, H.; Stoschka, M. Damage Tolerant Fatigue Design of Seam Imperfections in Mild Steel Butt Joints by Analytical and Numerical Approaches; International Institute of Welding (IIW): Yutz, France, XIII-WG4-158-17; 2017. [Google Scholar]
- Javaheri, E.; Hemmesi, K.; Tempel, P.; Farajian, M. Fatigue Assessment of the Welded Joints Containing Process Relevant Imperfections; International Institute of Welding (IIW): Yutz, France, XIII-2477-13; 2013. [Google Scholar]
- Farajian, M.; Fenzl, R.; Beckmann, C.; Tempel, P.; Kranz, B.; Wagner, S.; Siegele, D. Quantifizierung des Einflusses der Nahtqualität auf die Ermüdungsfestigkeit von Schweißverbindungen; Report number IGF-17.559B/DVS-Nr. 09.055; Fraunhofer-Institut für Werkstoffmechanik IWM: Halle, Germany, 2016. (In German) [Google Scholar]
- McEvily, A.J.; Endo, M.; Murakami, Y. On the √area relationship and the short crack fatigue threshold. Fatigue Fract. Eng. Struct. 2003, 26, 269–278. [Google Scholar] [CrossRef]
- Murakami, Y.; Kodama, S.; Konuma, S. Quantitative Evaluation of Effects of Nonmetallic Inclusions on Fatigue Strength of High Strength Steel. Trans. Jpn. Soc. Mech. Eng. Eng. A 1988, 54, 688–696. [Google Scholar] [CrossRef]
- Murakami, Y. Metal Fatigue: Effects of Small Defects and Non-Metallic Inclusions; Elsevier: New York, NY, USA, 2002. [Google Scholar]
- Baumgartner, J.; Bruder, T. Influence of weld geometry and residual stresses on the fatigue strength of longitudinal stiffeners. Weld. World 2013, 57, 841–855. [Google Scholar] [CrossRef]
- Kihl, D.P.; Sarkani, S. Mean stress effects in fatigue of welded steel joints. Prob. Eng. Mech. 1999, 14, 97–104. [Google Scholar] [CrossRef]
- Leitner, M. Influence of effective stress ratio on the fatigue strength of welded and HFMI-treated high-strength steel joints. Int. J. Fatigue 2017, 102, 158–170. [Google Scholar] [CrossRef]
- Beretta, S.; Blarasin, A.; Endo, M.; Giunti, T.; Murakami, Y. Defect tolerant design of automotive components. Int. J. Fatigue 1997, 19, 319–333. [Google Scholar] [CrossRef]
- Costa, N.; Machado, N.; Silva, F.S. A new method for prediction of nodular cast iron fatigue limit. Int. J. Fatigue 2010, 32, 988–995. [Google Scholar] [CrossRef]
- De Kazinczy, F. Effect of small defects on the fatigue properties of medium strength cast steel. J. Iron Steel Inst. 1970, 851–855. [Google Scholar]
- Radaj, D. Geometriekorrektur zur Spannungsintensität an elliptischen Rissen. Schw. Schneid. 1977, 29, 398–402. (In German) [Google Scholar]
- Casagrande, A.; Cammarota, G.P.; Micele, L. Relationship between fatigue limit and Vickers hardness in steels. Mater. Sci. Eng. A 2011, 528, 3468–3473. [Google Scholar] [CrossRef]
- Pavlina, E.J.; van Tyne, C.J. Correlation of Yield Strength and Tensile Strength with Hardness for Steels. J. Mater. Eng. Perform. 2008, 17, 888–893. [Google Scholar] [CrossRef]
- Mitchell, M.R. Review of the Mechanical Properties of Cast Steels with Emphasis on Fatigue Behavior and the Influence of Microdiscontinuities. J. Eng. Mater. Technol. 1977, 329–343. [Google Scholar] [CrossRef]
- Davis, T.; Healy, D.; Bubeck, A.; Walker, R. Stress concentrations around voids in three dimensions: The roots of failure. J. Struct. Geol. 2017, 102, 193–207. [Google Scholar] [CrossRef]
- Ostsemin, A.A.; Utkin, P.B. Stress-Concentration Coefficients of Internal Welding Defects. Rus. Eng. Res. 2008, 28, 1165–1168. [Google Scholar] [CrossRef]
- Rossini, N.S.; Dassisti, M.; Benyounis, K.Y.; Olabi, A.G. Methods of measuring residual stresses in components. Mater. Des. 2012, 35, 572–588. [Google Scholar] [CrossRef] [Green Version]
- Leitner, M.; Simunek, D.; Shah, F.S.; Stoschka, M. Numerical fatigue assessment of welded and HFMI-treated joints by notch stress/strain and fracture mechanical approaches. Adv. Eng. Softw. 2016, accepted-in press. [Google Scholar] [CrossRef]
- Alipooramirabad, H.; Paradowska, A.; Ghomashchi, R.; Kotousova, A.; Reid, M. Quantification of residual stresses in multi-pass welds using neutron diffraction. J. Mater. Process. Technol. 2015, 226, 40–49. [Google Scholar] [CrossRef]
- Ottersböck, M.; Stoschka, M.; Thaler, M. Study of kinematic strain hardening law in transient welding simulation. In Mathematical Modelling of Weld Phenomena; Montanuniversität Leoben: Leoben, Austria, 2014; Volume 10, pp. 255–266. [Google Scholar]
- ASTM International. Standard practice for statistical analysis of linear or linearized stress-life (S-N) and strain-life (e-N) fatigue data; ASTM E739-91(1998); ASTM International: West Conshohocken, PA, USA, 1998. [Google Scholar]
- McKelvey, S.A.; Lee, Y.-L.; Barkey, M.E. Stress-Based Uniaxial Fatigue Analysis Using Methods Described in FKM-Guideline. J. Fail. Anal. Prev. 2012, 12, 445–484. [Google Scholar] [CrossRef]
- Leitner, M.; Pauer, P.; Kainzinger, P.; Eichlseder, W. Numerical effects on notch fatigue strength assessment of non-welded and welded components. Comp. Struct. 2017, 191, 51–61. [Google Scholar] [CrossRef]
- Ottersböck, M.; Leitner, M.; Stoschka, M.; Maurer, W. Analysis of fatigue notch effect due to axial misalignment for ultra high-strength steel butt joints; International Institute of Welding (IIW): Yutz, France, XIII-2690-17; 2017. [Google Scholar]
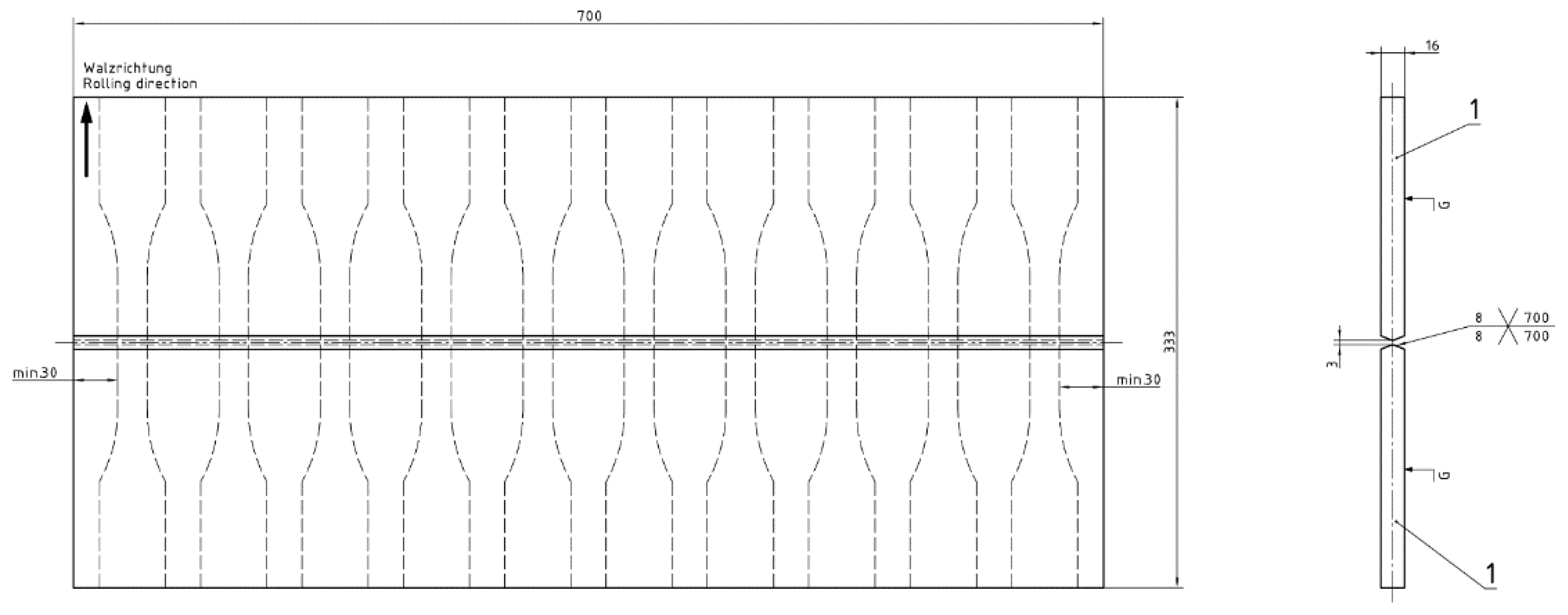

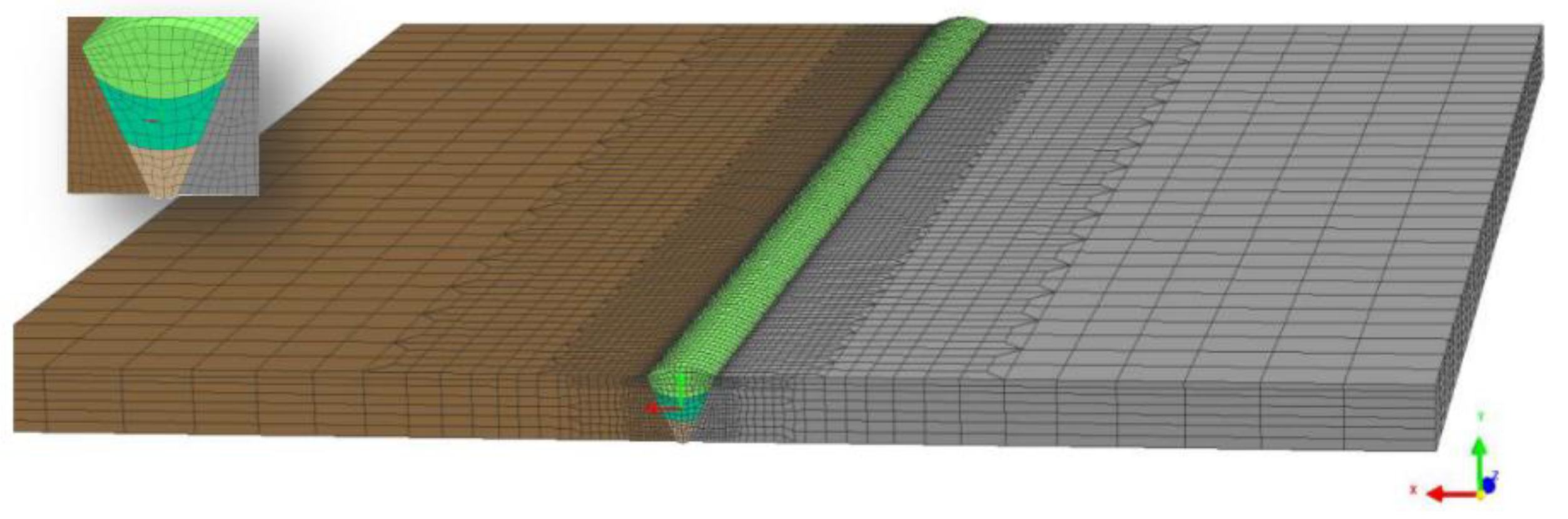



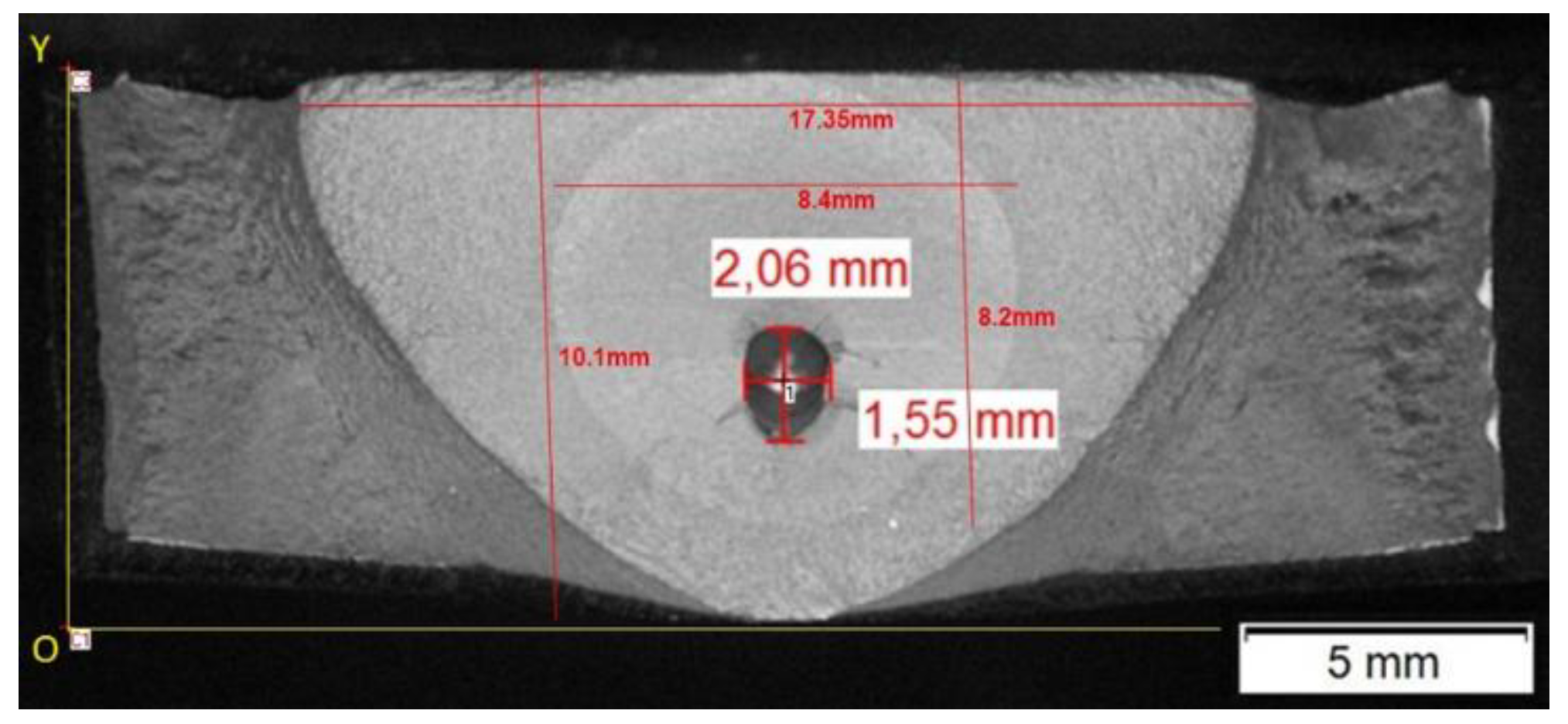
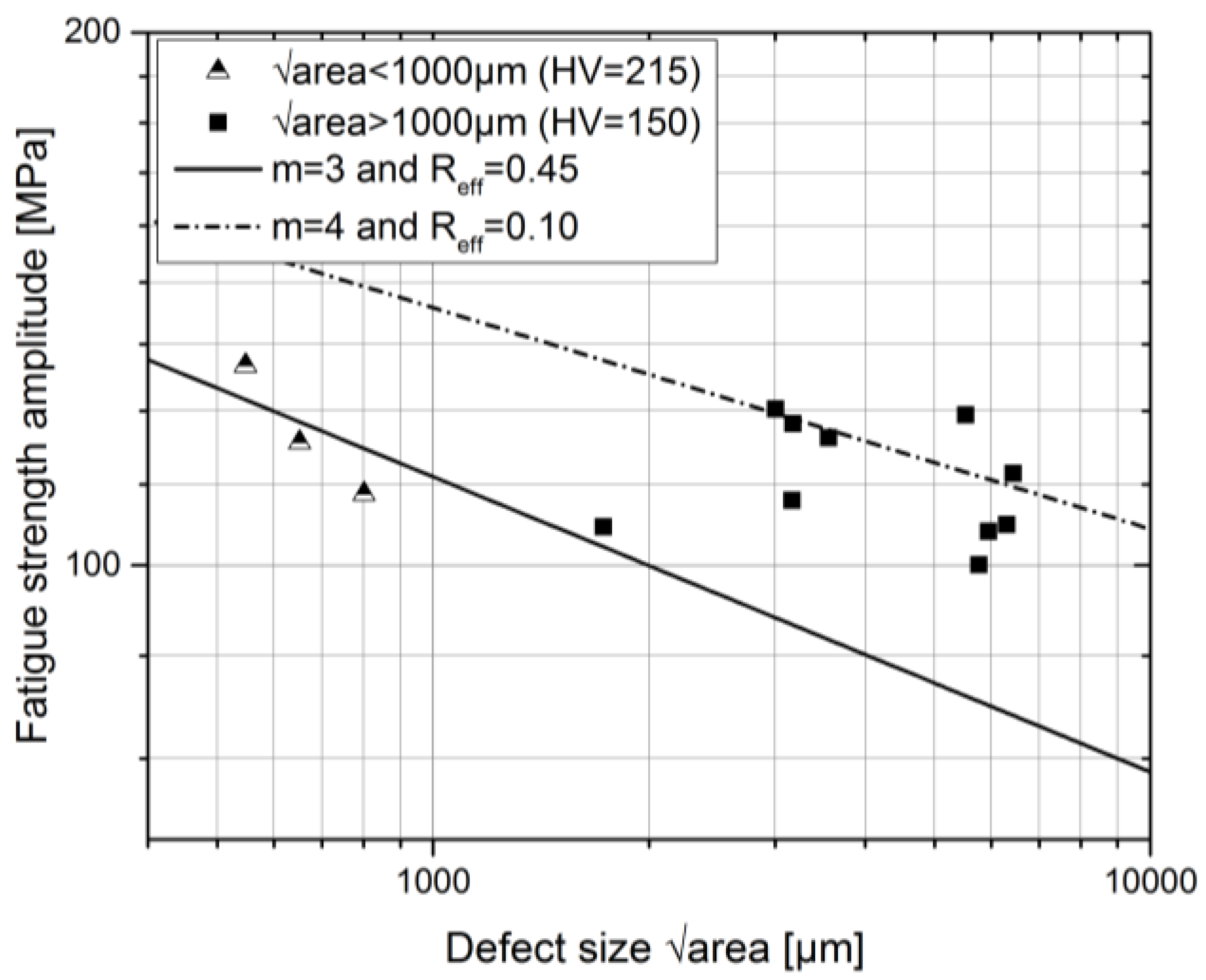




| Denotation | Weld type | Weld-Passes | Area (w by t) | Reference |
|---|---|---|---|---|
| √area < 1000 µm | Butt joint | Two, double-sided | 20 by 16 mm | [28] |
| √area > 1000 µm | Butt joint | Three, single-sided | 30 by 10 mm | [29] |
| Material | C | Mn | Si | P | S | Cr | Ni | Fe | Reference |
|---|---|---|---|---|---|---|---|---|---|
| S355NL | 0.09 | 1.29 | 0.02 | 0.010 | 0.013 | 0.039 | 0.036 | balance | [30] |
| Denotation | Hardness Value (HV5) | Axial Residual Stress (MPa) |
|---|---|---|
| √area < 1000 µm | 215 | approximately 335 |
| √area > 1000 µm | 150 | around zero |
| Length of Gas Pore (mm) | Width of Gas Pore (mm) | Calculated √area (µm) | Vertical Distance from Top Surface (mm) |
|---|---|---|---|
| 0.87 | 0.44 | 548 | 3.5 |
| 0.76 | 0.71 | 651 | 5.1 |
| 1.08 | 0.76 | 801 | 4.2 |
| Length of Gas Pore (mm) | Width of Gas Pore (mm) | Calculated √area (µm) | Vertical Distance from Top Surface (mm) |
|---|---|---|---|
| 2.06 | 1.55 | 3167 | 5.4 |
| 1.95 | 1.95 | 1728 | 3.9 |
| 2.05 | 1.40 | 3003 | 7.3 |
| 3.14 | 3.10 | 5530 | 6.3 |
| 2.12 | 1.90 | 3557 | 6.8 |
| 4.20 | 3.14 | 6437 | 6.3 |
| 3.58 | 2.96 | 5770 | 3.8 |
| 3.72 | 3.03 | 5951 | 4.0 |
| 1.79 | 1.79 | 3173 | 5.9 |
| 3.69 | 3.43 | 6306 | 5.8 |
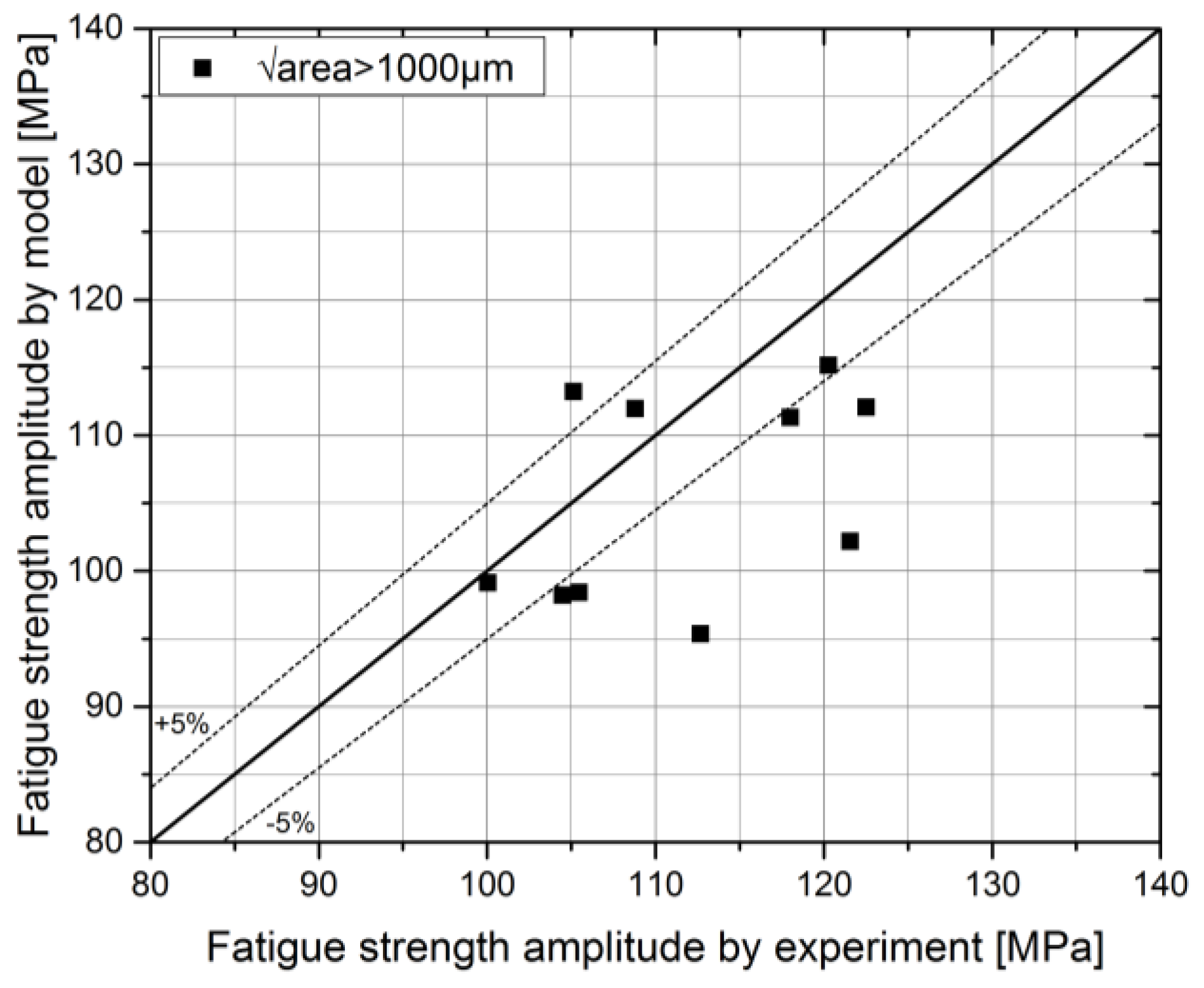
| Denotation | Murakami [33] | De Kazinczy [39] |
|---|---|---|
| √area < 1000 µm | +1% | - |
| √area > 1000 µm | +5% | −5% |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Leitner, M.; Murakami, Y.; Farajian, M.; Remes, H.; Stoschka, M. Fatigue Strength Assessment of Welded Mild Steel Joints Containing Bulk Imperfections. Metals 2018, 8, 306. https://doi.org/10.3390/met8050306
Leitner M, Murakami Y, Farajian M, Remes H, Stoschka M. Fatigue Strength Assessment of Welded Mild Steel Joints Containing Bulk Imperfections. Metals. 2018; 8(5):306. https://doi.org/10.3390/met8050306
Chicago/Turabian StyleLeitner, Martin, Yukitaka Murakami, Majid Farajian, Heikki Remes, and Michael Stoschka. 2018. "Fatigue Strength Assessment of Welded Mild Steel Joints Containing Bulk Imperfections" Metals 8, no. 5: 306. https://doi.org/10.3390/met8050306
APA StyleLeitner, M., Murakami, Y., Farajian, M., Remes, H., & Stoschka, M. (2018). Fatigue Strength Assessment of Welded Mild Steel Joints Containing Bulk Imperfections. Metals, 8(5), 306. https://doi.org/10.3390/met8050306







