Removal Mechanism of Microscale Non-Metallic Inclusions in a Tundish with Multi-Hole-Double-Baffles
Abstract
1. Introduction
2. Structure of MHDB
3. Mathematical Model
3.1. Model Assumptions
- The flow in the tundish is turbulent flow, and the molten steel is an incompressible viscous fluid;
- The inclusions do not influence the flow field, but the flow field does influence the movement of the inclusions;
- The inclusions are spherical;
- The molten steel surface is stable, and the slag layer is not considered;
- The movement of the inclusions is simulated using a stochastic motion model.
3.2. Governing Equations and Boundary Conditions
- The model inlet of tundish was set as the inlet with the velocity derived from the casting speed. Similarly, the inlet of MHDB was set as the velocity inlet, and this velocity is determined by measuring the average velocity of each guide-hole in the simulation of the tundish. The inclusions were injected into the inlet at the same velocity, and the diameter distribution of inclusions is set to “uniform”, which means that the number of inclusion particles for each diameter is the same. Moreover, the inlet velocity of the unused holes is set to zero;
- The liquid surface was set to be a free surface with zero normal velocity and gradient, and the inclusions were trapped at the liquid surface;
- The wall of the tundish was a modeled as a no-slip boundary, and using the Users Defined Function (UDF) in ANSYS Fluent code to define the wall boundary condition, the inclusions could be removed directly from the computing domain when inclusion particles collided with the wall and the speed was less than 0.01 m/s, otherwise it can be reflected from the wall;
- The model outlet was set as the pressure outlet, and for the inclusions, the model outlet was the escape outlet.
4. Results and Discussion
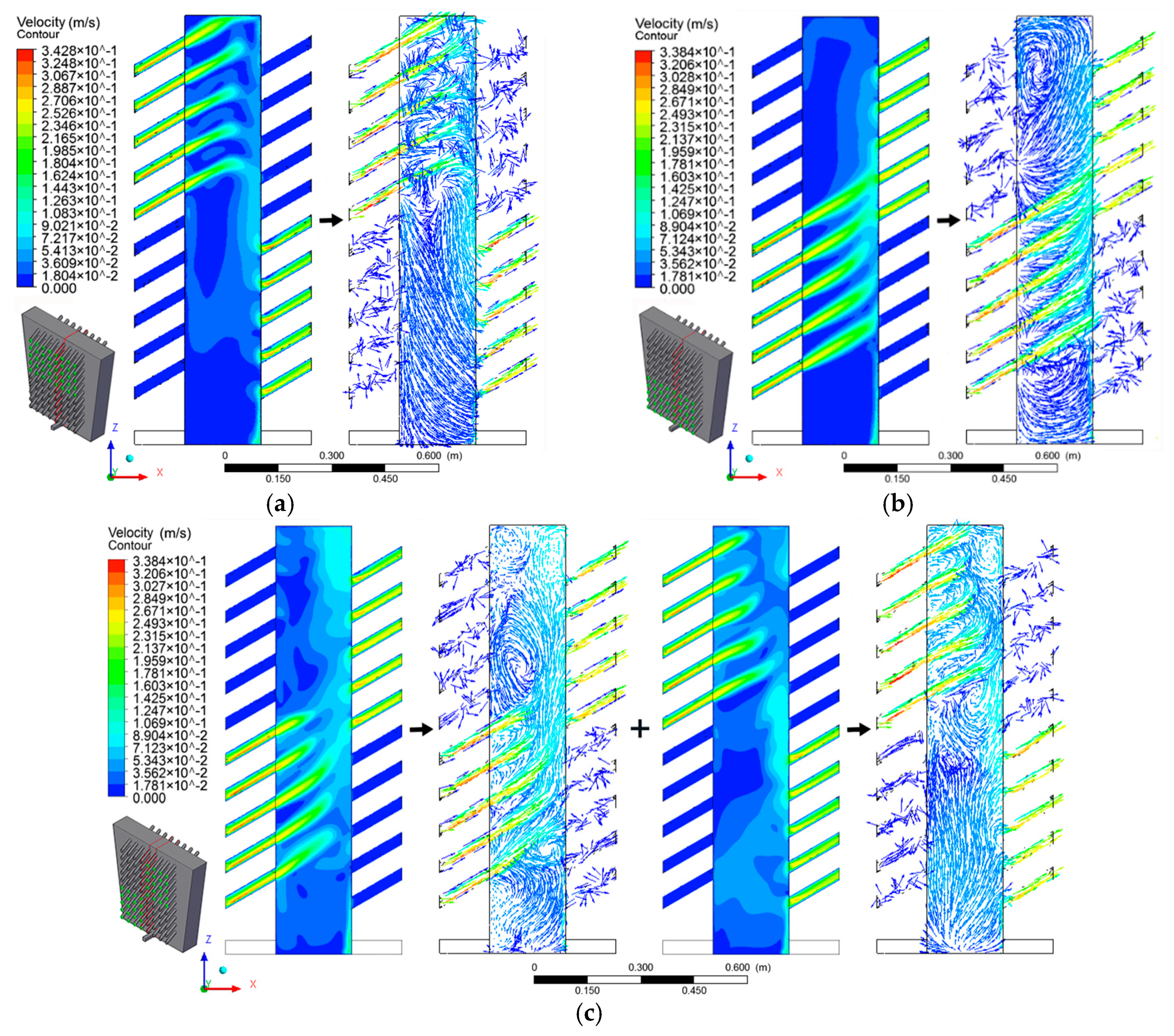
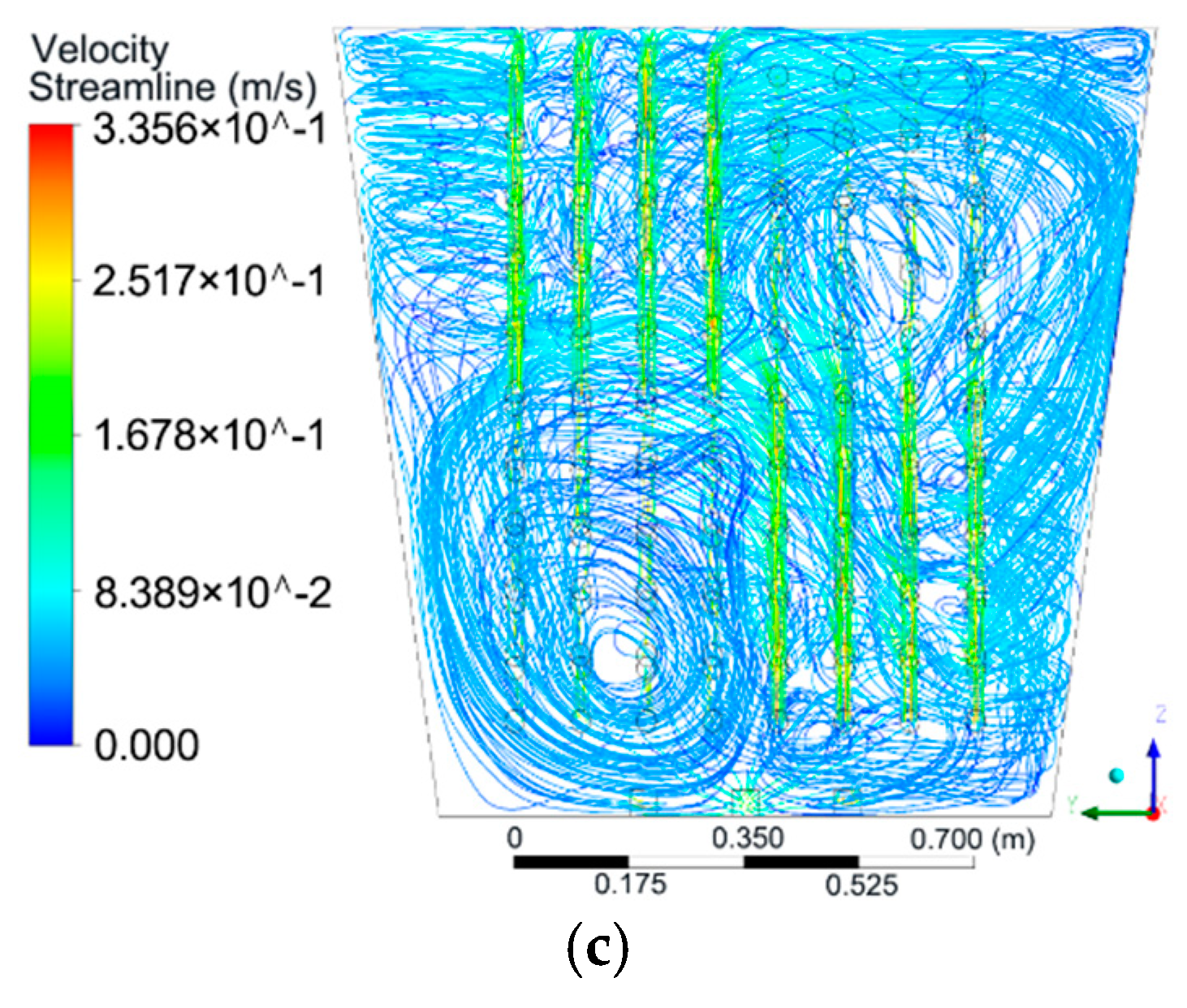
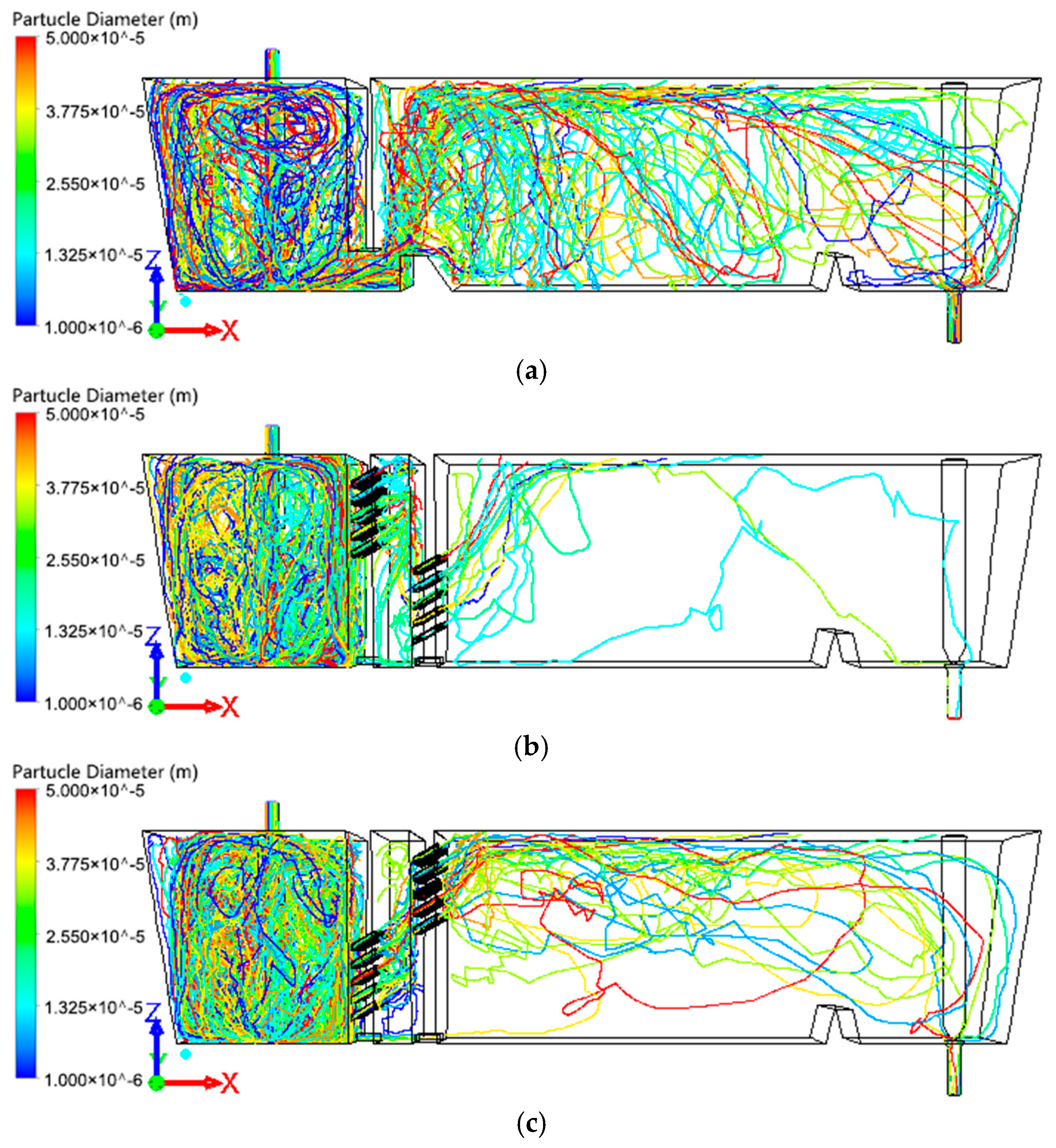
4.1. Flow Field
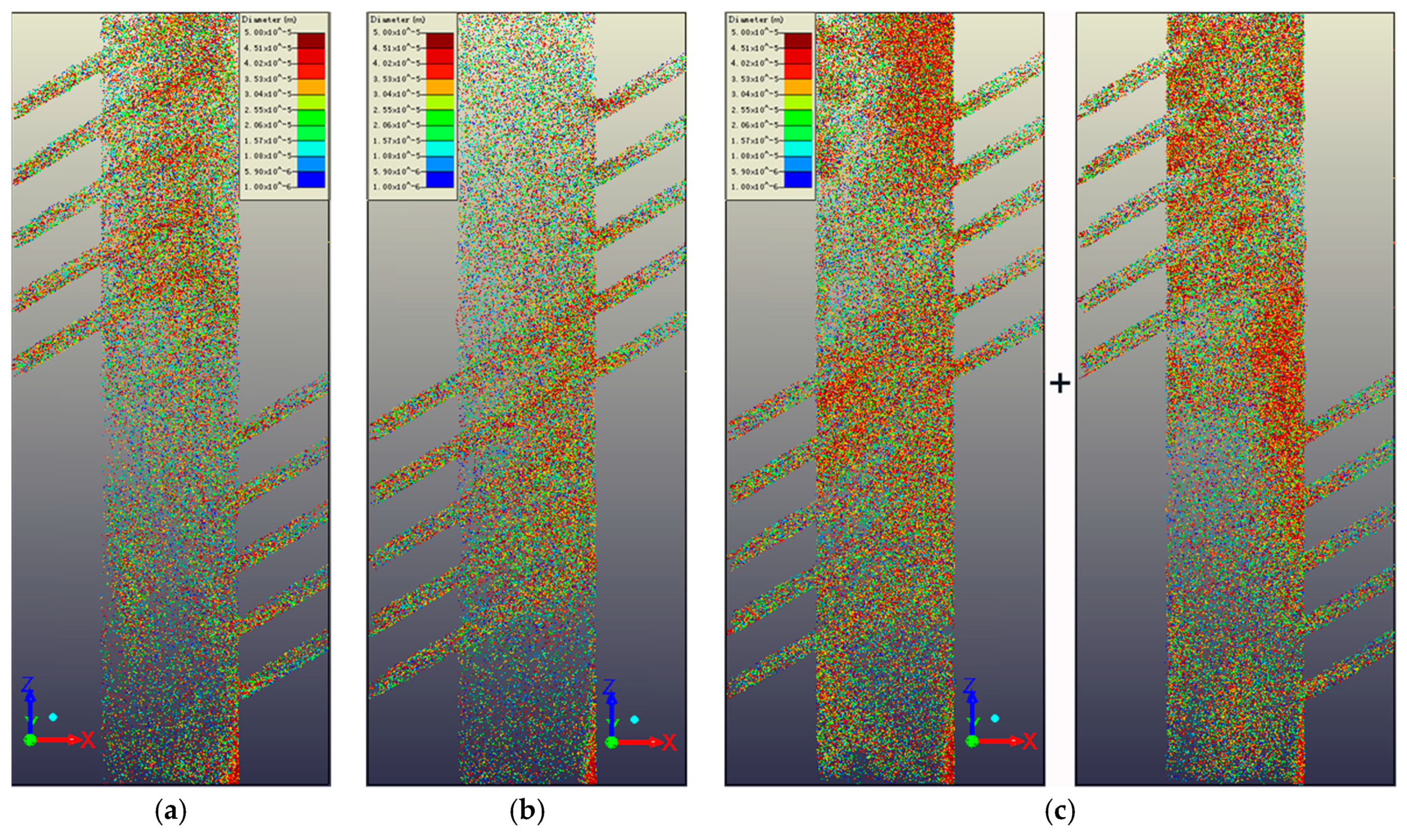
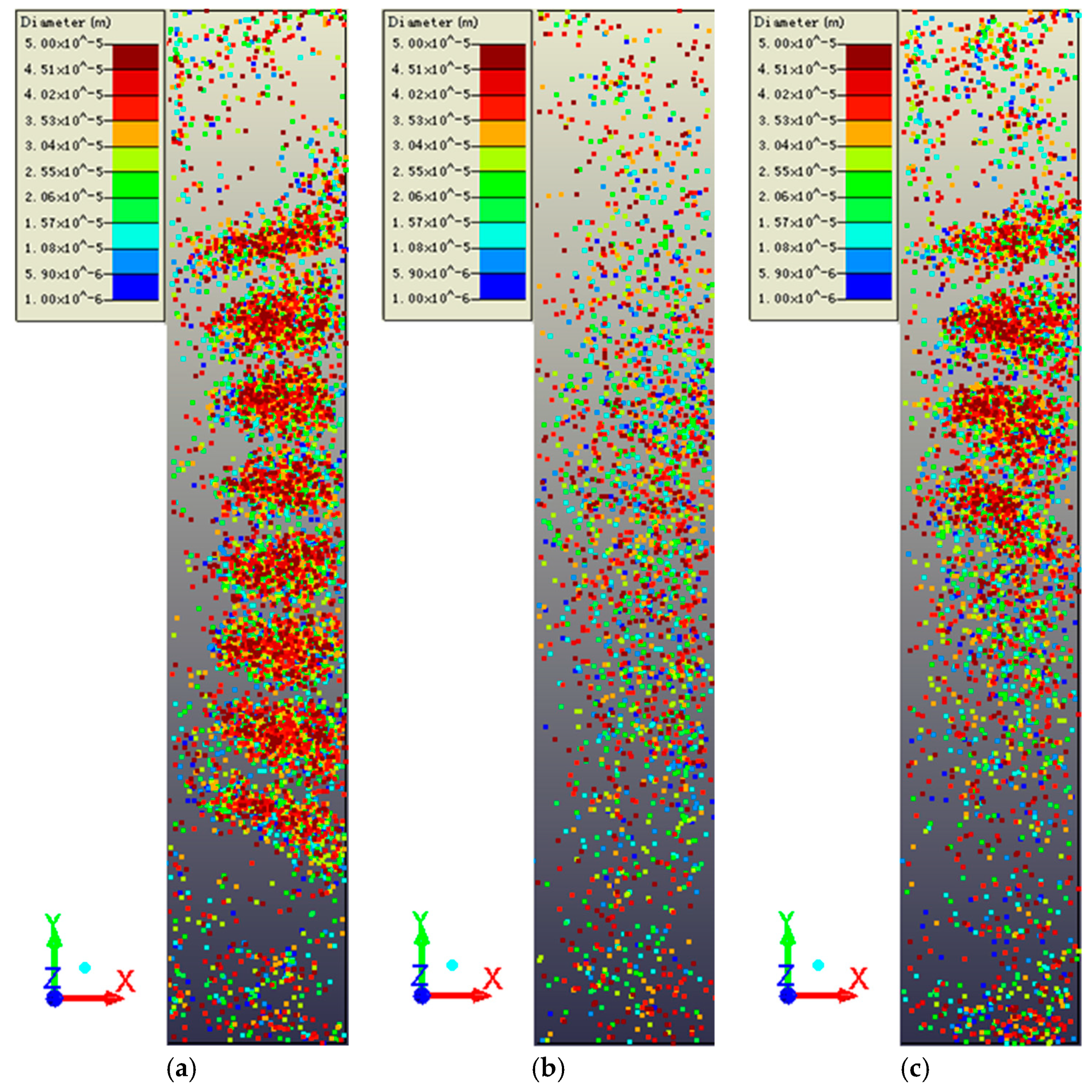
4.2. Distribution of Inclusions
4.2.1. Trajectories of the Inclusions
4.2.2. Residence Time of the Inclusions
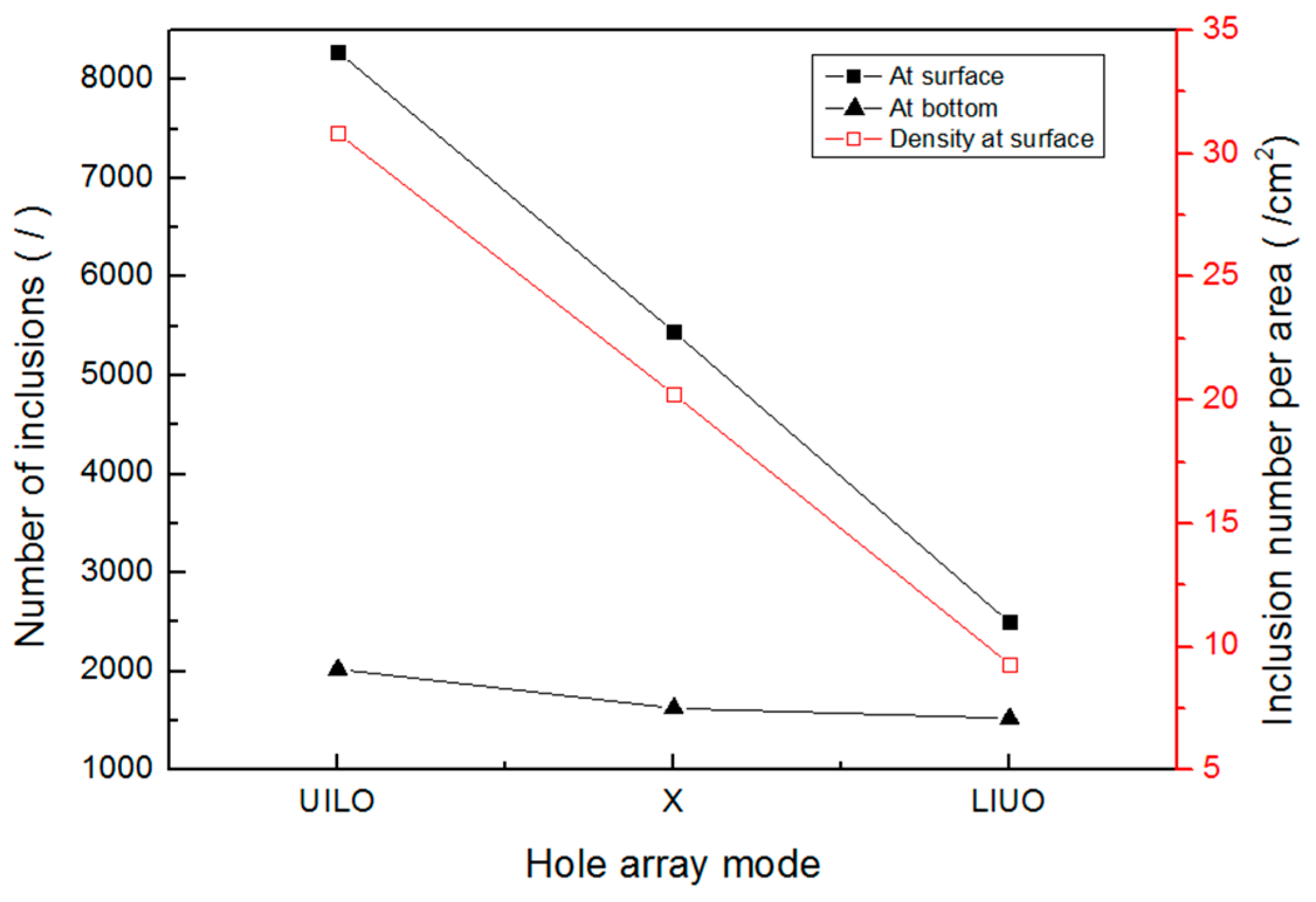
4.2.3. Distribution of the Inclusions
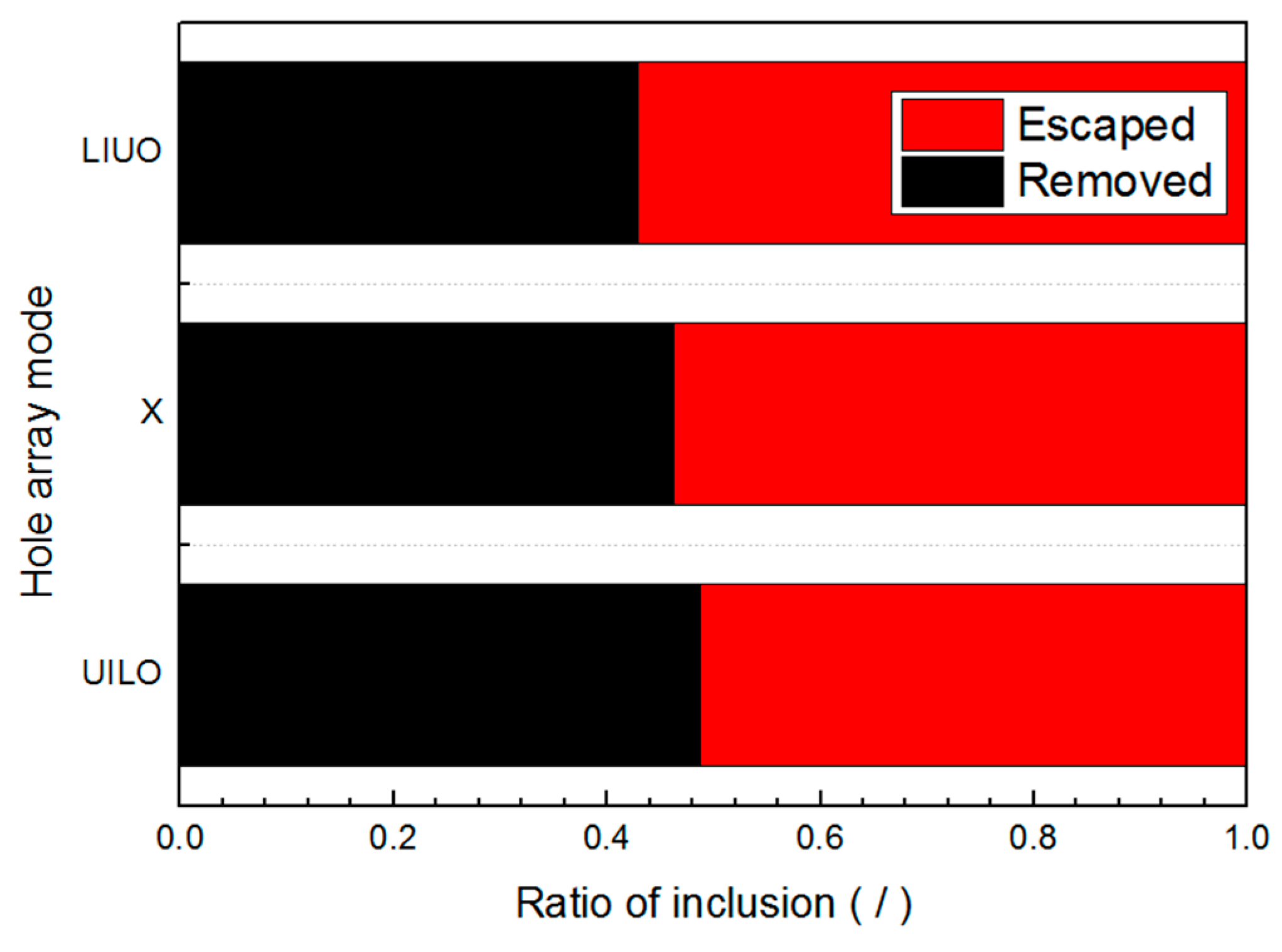
4.3. Inclusion Removing Efficiency
4.4. Comparison with Physical Simulation
5. Conclusions
- The MHDB was a high efficient flow control device in the tundish, and the change of hole array modes of the MHDB could directly affect the trajectories of the micro-inclusions so that the removal effect of inclusions in the MHDB or tundish could be improved.
- Many vortices and cross-flows were formed in the MHDB in the UILO or X modes, increasing the residence time of micro-inclusions. The residence time in the MHDB in the UILO mode was 15 times longer than the theoretical value.
- The tundish with the MHDB device could not only effectively limit the range of pouring area and stabilize the flow field of the tundish, but also form stable upward streams and surface flows in the internal area of MHDB and the downstream area of the tundish, thus promoting the removal of inclusions.
- It was confirmed that the micro-inclusion removal by MHDB in the UILO or X mode was high. In the future, the size and material of the MHDB in the UILO or X modes should be improved in order to trap more inclusions at the molten steel surface and in the secondary baffle.
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Pang, X.F.; Jiang, Y.C.; Gao, L.; Tang, B.; Li, H.B.; Yu, S.P.; Liu, W. Dynamic scheduling system for steelmaking-refining-continuous casting production. In Proceedings of the 2017 29th Chinese Control and Decision Conference, Chongqing, China, 17 July 2017; pp. 4710–4715. [Google Scholar]
- Sahai, Y. Tundish Technology for Casting Clean Steel: A Review. Metall. Trans. B 2016, 47, 2095–2106. [Google Scholar] [CrossRef]
- Sahai, Y. Advances in Tundish Technology for Quality Improvements of Cast Steel. In Proceedings of the 4th International Conference on Continuous Casting of Steel in Developing Countries, Beijing, China, 4–7 November 2008; pp. 643–652. [Google Scholar]
- Chatterjee, S.; Chattopadhyay, K. Tundish Open Eye Formation in Inert Gas-Shrouded Tundishes: A Macroscopic Model from First Principles. Metall. Trans. B 2016, 47, 3099–3114. [Google Scholar] [CrossRef]
- Chatterjee, S.; Chattopadhyay, K. Physical Modeling of Slag ‘Eye’ in an Inert Gas-Shrouded Tundish Using Dimensional Analysis. Metall. Trans. B 2016, 47, 508–521. [Google Scholar] [CrossRef]
- Siddiqui, M.I.H.; Jha, P.K. Effect of Inflow Rate Variation on Intermixing in a Steelmaking Tundish during Ladle Change-Over. Steel Res. Int. 2016, 87, 733–744. [Google Scholar] [CrossRef]
- Gutiérrez, E.; Garcia-Hernandez, S.; José, D.J.B. Mathematical Modeling of Inclusions Deposition at the Upper Tundish Nozzle and the Submerged Entry Nozzle. Steel Res. Int. 2016, 87, 1406–1416. [Google Scholar] [CrossRef]
- CwudziåSki, A. Physical and mathematical simulation of liquid steel mixing zone in one strand continuous casting tundish. Cast Met. 2016, 30, 50–60. [Google Scholar] [CrossRef]
- Yue, Q.; Zhang, C.B.; Pei, X.H. Magnetohydrodynamic flows and heat transfer in a twin-channel induction heating tundish. Ironmak. Steelmak. 2016, 44, 227–236. [Google Scholar] [CrossRef]
- Cheng, C.; Zhang, F.; Li, Y.; Chen, Y.; Jin, Y. Mathematical Modeling on Deformation Behavior of Solidified Shell in Continuous Slab Casting with Soft Reduction. High Temp. Mater. Process. 2017, 36, 389–398. [Google Scholar] [CrossRef]
- Yang, L.; Li, Y.; Xue, Z.L.; Cheng, C.G. Influence of Ti(C,N) precipitates on austenite growth of micro-alloyed steel during continuous casting. China Foundry 2017, 14, 421–428. [Google Scholar] [CrossRef]
- Jin, Y.; Ye, C.; Luo, X.; Yuan, H.; Cheng, C. The Model Analysis of Inclusion Moving in the Swirl Flow Zone Sourcing from the Inner-Swirl-Type Turbulence Controller in Tundish. High Temp. Mater. Process. 2017, 36, 541–550. [Google Scholar] [CrossRef]
- Pirker, S.; Lechner, M.; Ernst, G. Numerical simulation and experimental validation of slag entrainment during tundish draining. Cast Met. 2013, 22, 244–247. [Google Scholar] [CrossRef]
- Yang, L.; Yan, J.; Hui, Y.; Kang, Y.; Fan, A.; Jun, W.; Huang, H.B. Numerical Calculation of Fluid Flow in a Continuous Casting Tundish. Adv. Mater. Res. 2013, 774–776, 316–320. [Google Scholar] [CrossRef]
- Sowa, L. Numerical Modelling of Fluid Flow and Thermal Phenomena in the Tundish of CSC Machine. Arch. Foundry Eng. 2014, 14, 103–106. [Google Scholar] [CrossRef]
- Jha, P.K.; Rao, P.S.; Anupam, D. Effect of Height and Position of Dams on Inclusion Removal in a Six Strand Tundish. Trans. Iron Steel Inst. Jpn. 2008, 48, 154–160. [Google Scholar] [CrossRef]
- Ding, H.; Jin, Y.; Yu, H.; Yang, K. Mathematical Simulation the Influence of Tundish with a Retaining Wall. Adv. Mater. Res. 2013, 774–776, 375–378. [Google Scholar] [CrossRef]
- He, Z.; Zhou, K.; Liu, S.; Xiong, W.; Li, B.K. Numerical modeling of the fluid flow in continuous casting tundish with different control devices. Abstr. Appl. Anal. 2013, 6, 1–8. [Google Scholar] [CrossRef]
- Tripathi, A.; Kumar, A.; Ajmani, S.K.; Singh, J.B.; Mahashabde, V.V. Numerical Investigation of Fluid Flow and Heat Transfer Phenomenon Inside a Single Strand Tundish of Slab Caster. Steel Res. Int. 2016, 86, 1558–1573. [Google Scholar] [CrossRef]
- Ni, P.; Jonsson, L.T.I.; Ersson, M.; Jönsson, P.G. A New Tundish Design to Produce a Swirling Flow in the SEN During Continuous Casting of Steel. Steel Res. Int. 2016, 87, 1356–1365. [Google Scholar] [CrossRef]
- Li, S.; Zhang, L.; Ren, Y.; Fang, W.; Yang, W.; Shao, S.; Yang, J.; Mao, W. Transient Behavior of Inclusions during Reoxidation of Si-killed Stainless Steels in Continuous Casting Tundish. ISIJ Int. 2016, 56, 584–593. [Google Scholar] [CrossRef]
- Gutiérrez, E.; Garcia-Hernandez, S.; Barreto, J.D.J. Mathematical Analysis of the Dynamic Effects on the Deposition of Alumina Inclusions inside the Upper Tundish Nozzle. ISIJ Int. 2016, 56, 1–10. [Google Scholar] [CrossRef]
- Filippov, G.A.; Tyuftyaev, A.S.; Gadzhiev, M.K.; Yusupov, D.I.; Sargsyan, M.A. Effect of Stabilizing Steel Temperature in a Continuous-Caster Tundish by the Plasma Method on the Uniformity of the Mechanical Properties of Plates After Rolling. Metallurgist 2016, 60, 267–273. [Google Scholar] [CrossRef]
- Isayev, O.; Kislitsa, V.; Zhang, C.; Wu, K.; Hress, A. Metallurgical Effects of a Novel Tundish Refining Process for High-Strength Low-Alloy Hot-Rolled Plate Steels. Metallurgist 2016, 59, 980–986. [Google Scholar] [CrossRef]
- Neves, L.; Tavares, R.P. Analysis of the mathematical model of the gas bubbling curtain injection on the bottom and the walls of a continuous casting tundish. Ironmak. Steelmak. 2016, 44, 1–9. [Google Scholar] [CrossRef]
- Bozheskov, A.N.; Kuklev, A.V.; Lebedev, I.V.; Toptygin, A.M.; Anisimov, K.N. Development of Slag-Forming Mixture for Steel-Pouring Ladle and its Application Technology During Production of Steels with High Aluminum and Sulfur Content. Metallurgist 2016, 59, 1081–1085. [Google Scholar] [CrossRef]
- Garcia-Hernandez, S.; Morales, R.D.; Jose, D.J.B.; Calderon-Ramos, I.; Gutierrez, E. Modeling Study of Slag Emulsification During Ladle Change-Over Using a Dissipative Ladle Shroud. Steel Res. Int. 2016, 87, 1154–1167. [Google Scholar] [CrossRef]
- Zhang, H.; Luo, R.; Fang, Q.; Ni, H.; Song, X. Numerical Simulation of Transient Multiphase Flow in a Five-Strand Bloom Tundish during Ladle Change. Metals 2018, 8, 146. [Google Scholar] [CrossRef]
- Ling, H.; Zhang, L. Numerical simulation of the growth and removal of inclusions in the molten steel of a two-strand tundish. JOM 2013, 65, 1155–1163. [Google Scholar] [CrossRef]
- Takahashi, K.; Ando, M.; Ishii, T. Numerical investigation of unsteady molten steel flow and inclusion behavior in the tundish in the ladle change period. ISIJ Int. 2014, 54, 304–310. [Google Scholar] [CrossRef]
- Hou, Q.; Yue, Q.; Wang, H.; Zou, Z.; Yu, A. Modelling of inclusion motion and flow patterns in swirling flow tundishes with symmetrical and asymmetrical structures. ISIJ Int. 2008, 48, 787–792. [Google Scholar] [CrossRef]
- Liu, Z.Q.; Wang, Q.; Li, B.K.; Jiang, M.F. Large eddy simulation for turbulent flow and inclusion distribution in continuous casting tundish. J. Northeast. Univ. 2012, 33, 669–672. [Google Scholar]
- Chattopadhyay, K.; Isac, M.; Lawrence, G.R.I. Modelling of non-isothermal melt flows in a four strand delta shaped billet caster tundish validated by water model experiments. ISIJ Int. 2012, 52, 2026–2035. [Google Scholar] [CrossRef]
- Chattopadhyay, K.; Isac, M.; Guthrie, R.I.L. Physical and mathematical modelling of inert gas shrouding in a tundish. ISIJ Int. 2011, 51, 573–580. [Google Scholar] [CrossRef]
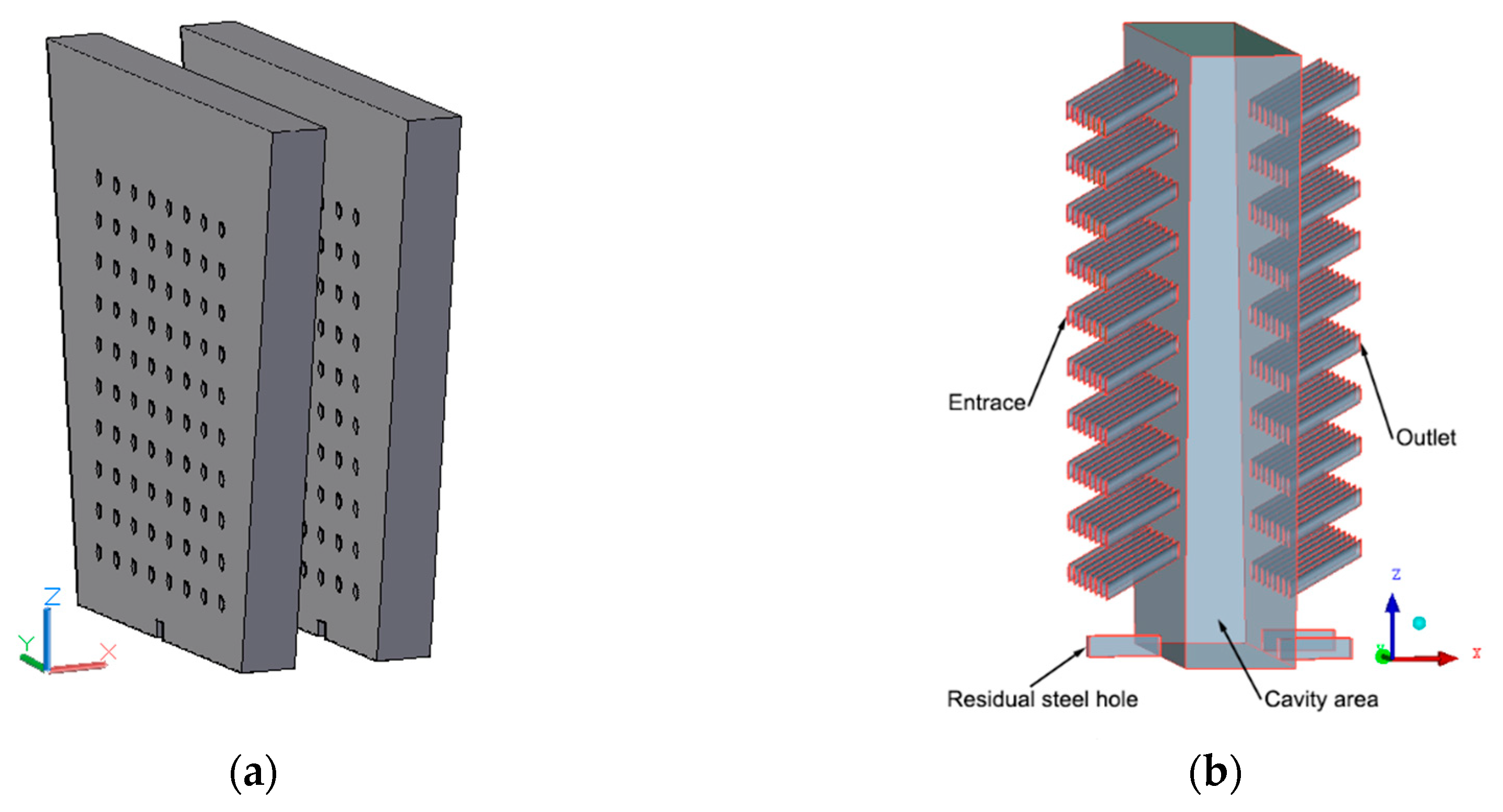
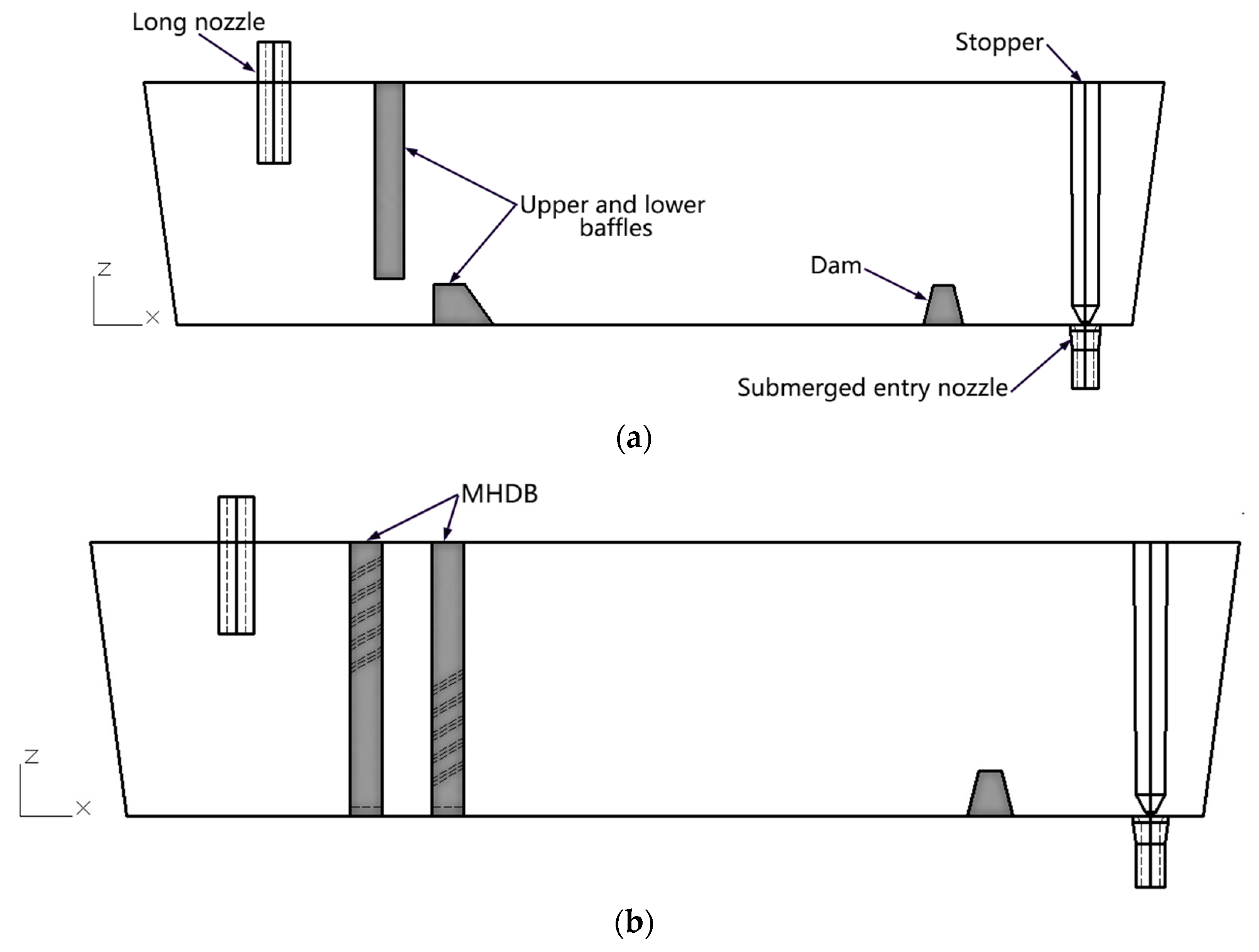



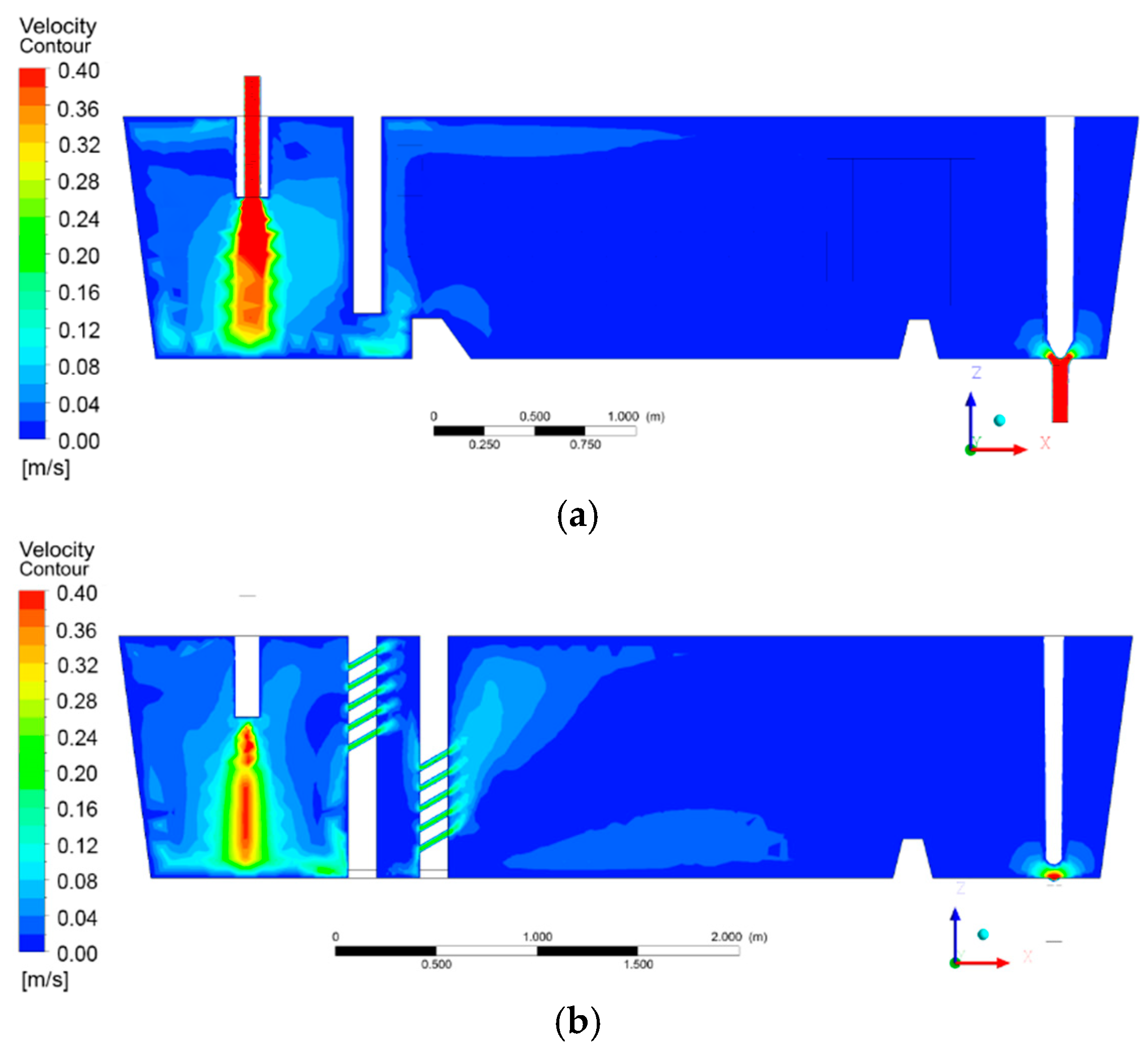
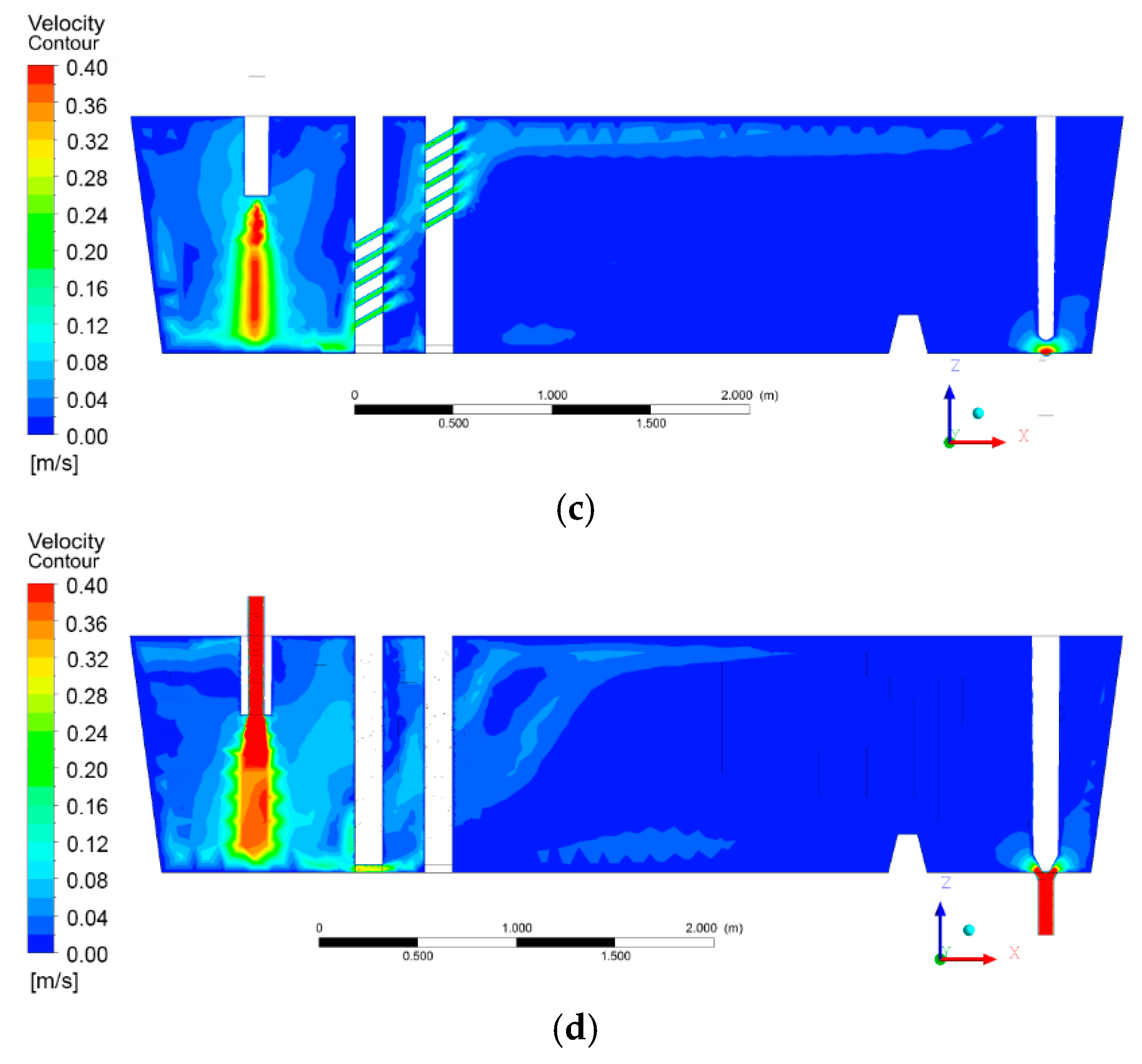







| Item | Parameter | Item | Parameter |
|---|---|---|---|
| Casting speed/m·min−1 | 4 | Working capacity of tundish/t | 60 |
| Diameter of the guide-holes/mm | 30 | Bloom cross section/mm2 | 1250 × 70 |
| Number of the guide-holes | 160 | Height of the upper baffle of UAIB/mm | 1000 |
| Side length of square vertical section of the residual steel hole/mm | 40 | Height of the lower baffle of UAIB/mm | 200 |
| Molten steel temperature/°C | 1600 | Submergence depth of long nozzle/mm | 400 |
| Molten steel density/kg·m−3 | 7000 | Inside and outside diameters of long nozzle/mm | 85/172 |
| Molten steel viscosity/Pa∙s | 0.0065 | Diameters of stopper/mm | 140 |
| Baffle thickness/mm | 140 | Theoretical residence time of tundish/s | 995.29 |
| Horizontal distance between the baffles/mm | 215 | Molten steel depth in the tundish/mm | 1200 |
| Hole Array Mode | Min Residence Time/s | Max Residence Time/s | Mean Residence Time/s |
|---|---|---|---|
| Theoretical value | - | - | 2.53 |
| UILO | 2.95 | 469.3 | 37.96 |
| X | 2.4 | 498.4 | 34.95 |
| LIUO | 2.2 | 473.5 | 25.13 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jin, Y.; Dong, X.; Yang, F.; Cheng, C.; Li, Y.; Wang, W. Removal Mechanism of Microscale Non-Metallic Inclusions in a Tundish with Multi-Hole-Double-Baffles. Metals 2018, 8, 611. https://doi.org/10.3390/met8080611
Jin Y, Dong X, Yang F, Cheng C, Li Y, Wang W. Removal Mechanism of Microscale Non-Metallic Inclusions in a Tundish with Multi-Hole-Double-Baffles. Metals. 2018; 8(8):611. https://doi.org/10.3390/met8080611
Chicago/Turabian StyleJin, Yan, Xiaosen Dong, Fu Yang, Changgui Cheng, Yang Li, and Wei Wang. 2018. "Removal Mechanism of Microscale Non-Metallic Inclusions in a Tundish with Multi-Hole-Double-Baffles" Metals 8, no. 8: 611. https://doi.org/10.3390/met8080611
APA StyleJin, Y., Dong, X., Yang, F., Cheng, C., Li, Y., & Wang, W. (2018). Removal Mechanism of Microscale Non-Metallic Inclusions in a Tundish with Multi-Hole-Double-Baffles. Metals, 8(8), 611. https://doi.org/10.3390/met8080611





